Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting
Abstract
:1. Introduction
2. Characteristics and Optimization Objectives of a Galloping Bluff Body
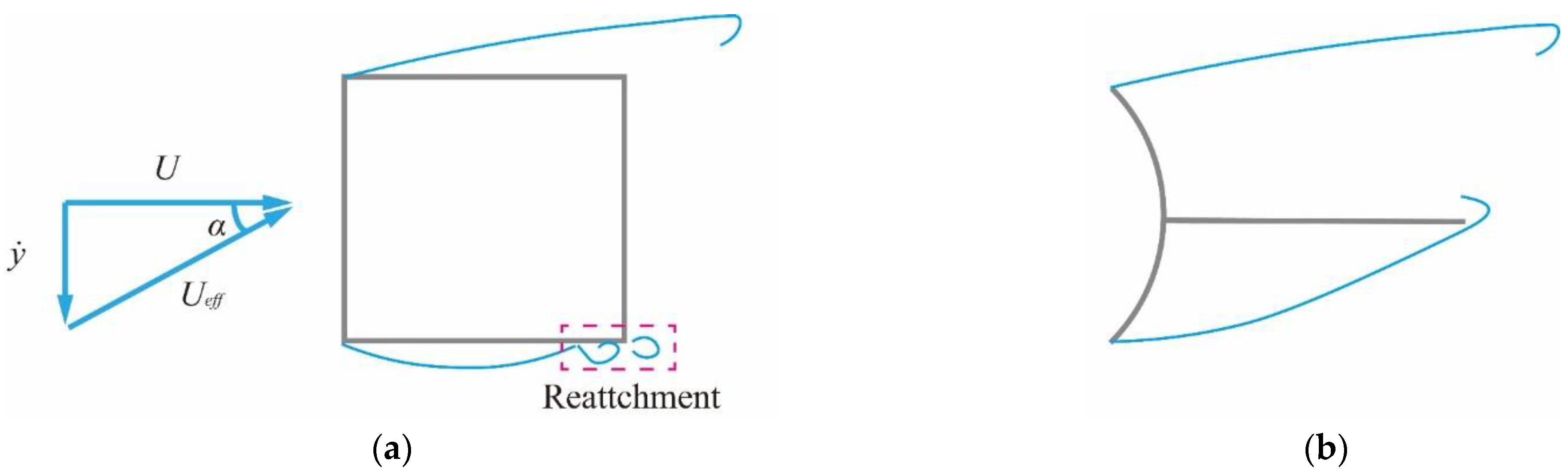
2.1. Characteristics of a Galloping Bluff Body
2.2. Optimization Objectives of a Galloping Bluff Body
3. Surrogate Modeling
3.1. The Kriging Surrogate Modeling Method
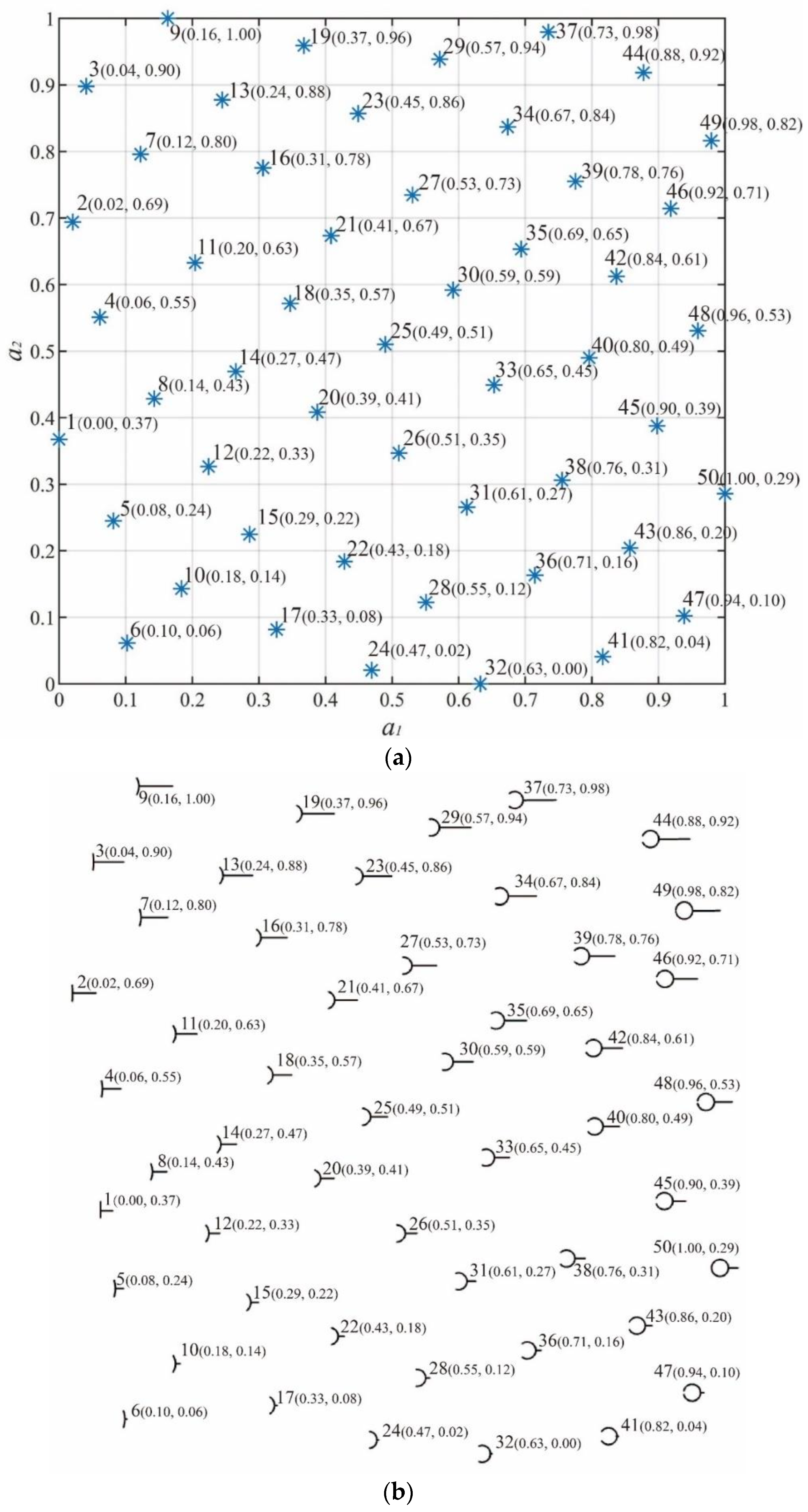
3.2. Design of Experiments
4. Wind Tunnel Test
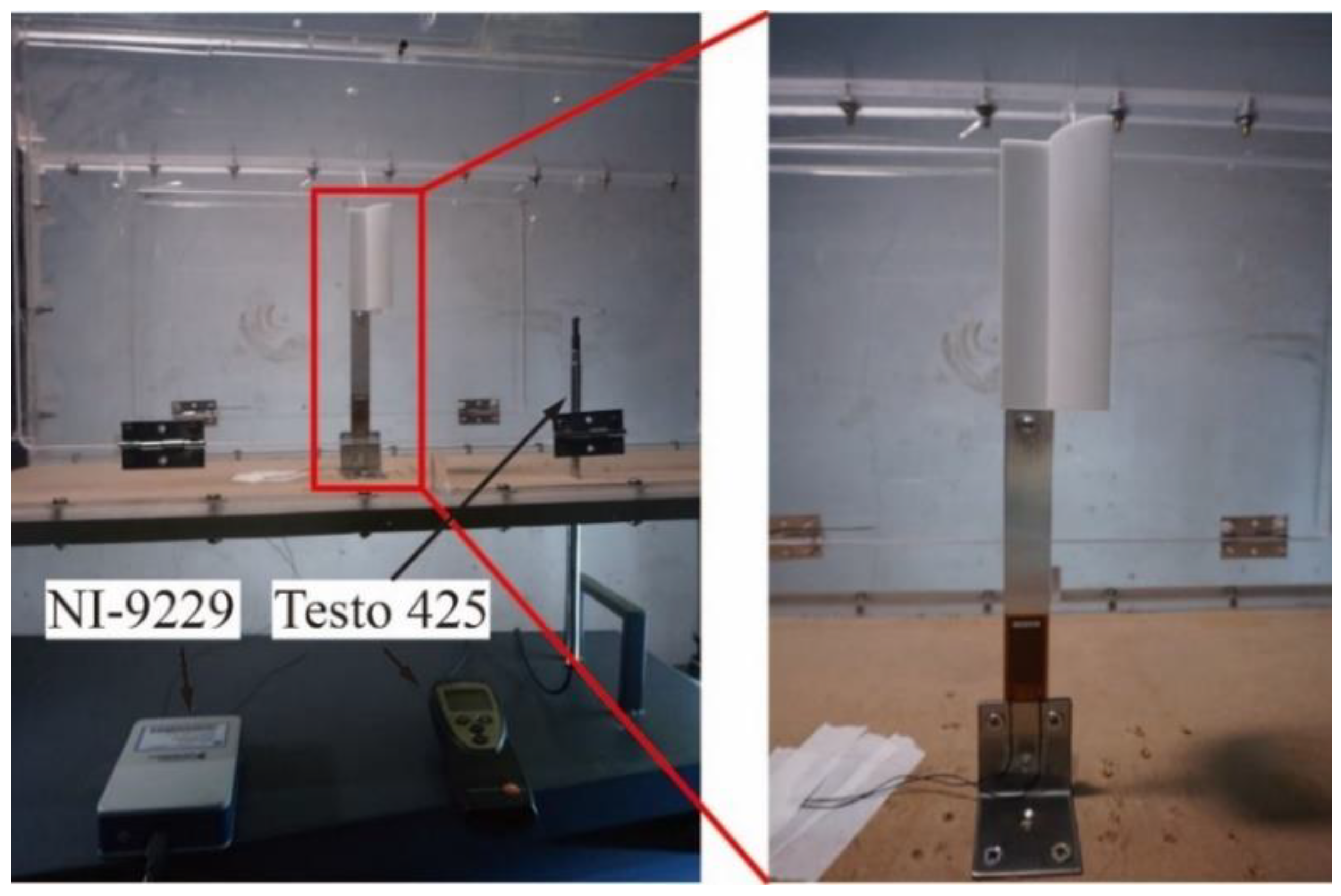
4.1. Piezoelectric Wind Energy Harvesting Tests
4.2. Force Measurement Tests
4.3. Uncertainty Analyses
5. Results and Discussions
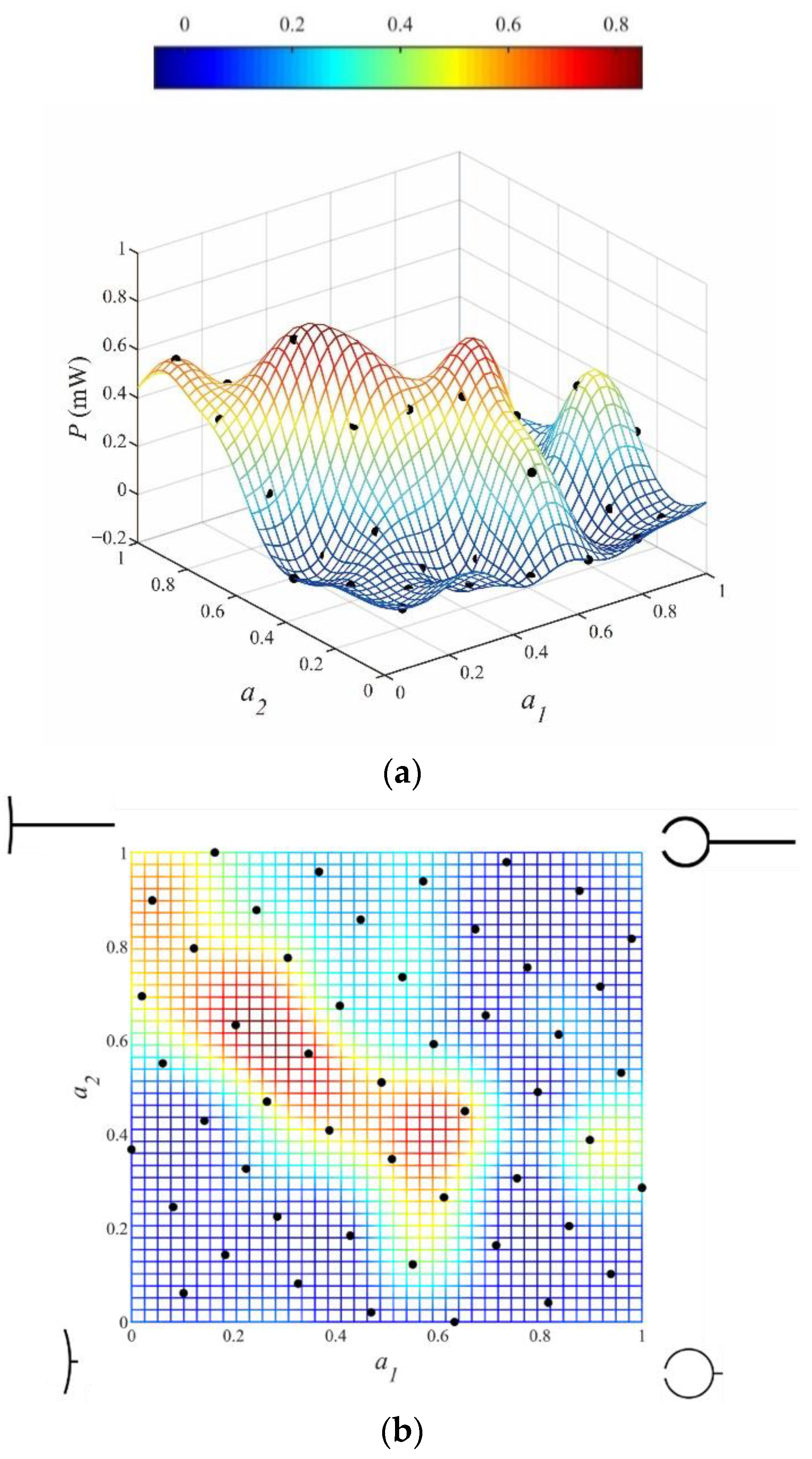
5.1. Surrogate Model
5.2. Output Power
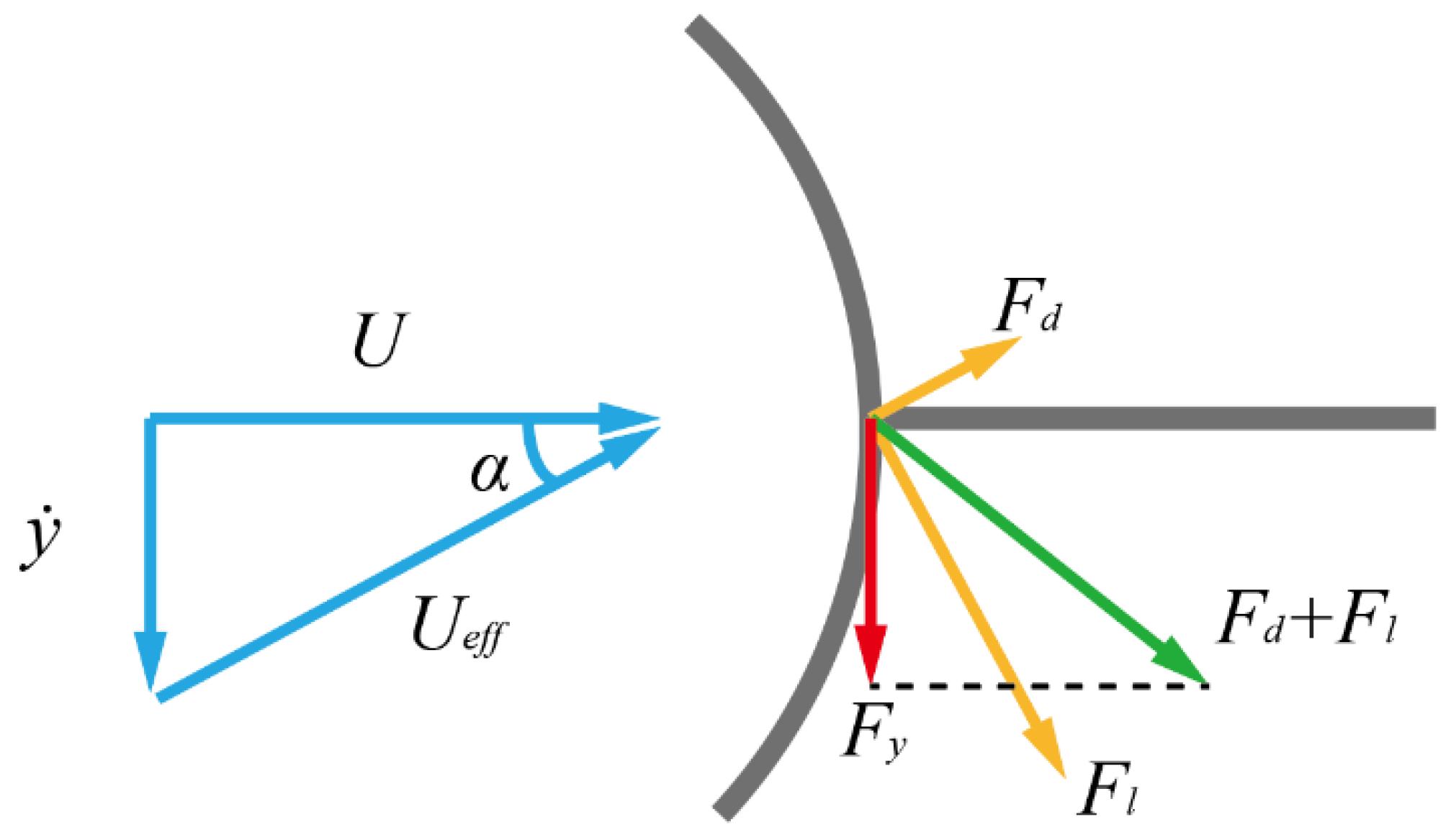
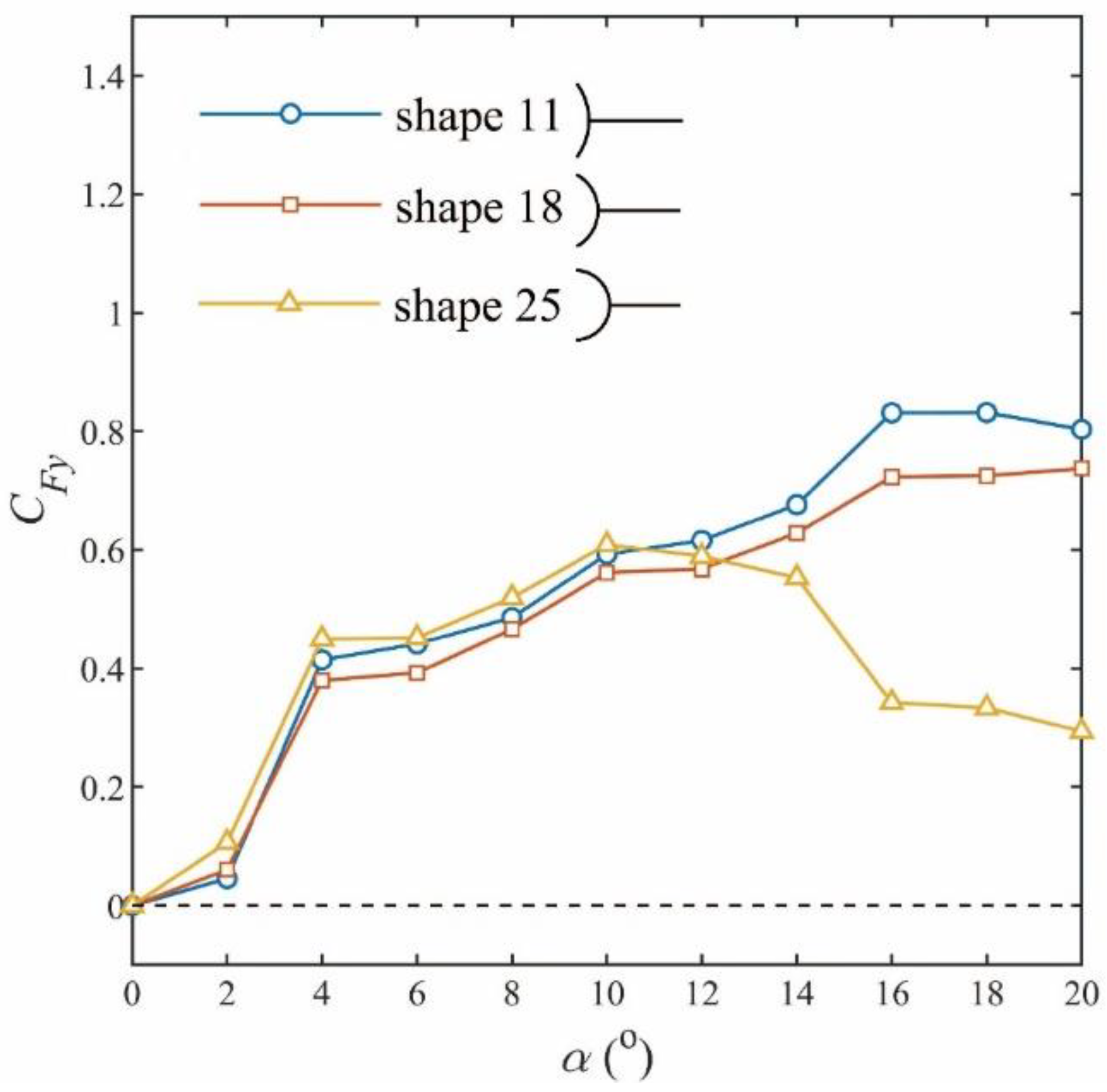
5.3. Force Coefficient
5.4. Comparision and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shaikh, F.K.; Zeadally, S. Energy harvesting in wireless sensor networks: A comprehensive review. Renew. Sustain. Energy Rev. 2016, 55, 1041–1054. [Google Scholar] [CrossRef]
- Alaei, E.; Afrasiab, H.; Dardel, M. Analytical and numerical fluid–structure interaction study of a microscale piezoelectric wind energy harvester. Wind Energy 2020, 23, 1444–1460. [Google Scholar] [CrossRef]
- Larsen, A. A generalized model for assessment of vortex-induced vibrations of flexible structures. J. Wind Eng. Ind. Aerodyn. 1995, 57, 281–294. [Google Scholar] [CrossRef]
- Arunachalam, S.; Lakshmanan, N. Across-wind response of tall circular chimneys to vortex shedding. J. Wind Eng. Ind. Aerodyn. 2015, 145, 187–195. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Hajj, M.R.; Nayfeh, A.H. Phenomena and modeling of piezoelectric energy harvesting from freely oscillating cylinders. Nonlinear Dyn. 2012, 70, 1377–1388. [Google Scholar] [CrossRef]
- Dai, H.; Abdelkefi, A.; Wang, L. Theoretical modeling and nonlinear analysis of piezoelectric energy harvesting from vortex-induced vibrations. J. Intell. Mater. Syst. Struct. 2014, 25, 1861–1874. [Google Scholar] [CrossRef]
- Hu, G.; Tse, K.T.; Kwok, K.C.S. Enhanced performance of wind energy harvester by aerodynamic treatment of a square prism. Appl. Phys. Lett. 2016, 108, 123901. [Google Scholar] [CrossRef]
- Hu, G.; Wang, J.; Su, Z.; Li, G.; Peng, H.; Kwok, K.C.S. Performance evaluation of twin piezoelectric wind energy harvesters under mutual interference. Appl. Phys. Lett. 2019, 115, 073901. [Google Scholar] [CrossRef]
- Javed, U.; Abdelkefi, A. Role of the galloping force and moment of inertia of inclined square cylinders on the performance of hybrid galloping energy harvesters. Appl. Energy 2018, 231, 259–276. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, Y. An impact-based broadband aeroelastic energy harvester for concurrent wind and base vibration energy harvesting. Appl. Energy 2018, 212, 233–243. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Nayfeh, A.H.; Hajj, M.R. Enhancement of power harvesting from piezoaeroelastic systems. Nonlinear Dyn. 2012, 68, 531–541. [Google Scholar] [CrossRef]
- Orrego, S.; Shoele, K.; Ruas, A.; Doran, K.; Caggiano, B.; Mittal, R.; Kang, S.H. Harvesting ambient wind energy with an inverted piezoelectric flag. Appl. Energy 2017, 194, 212–222. [Google Scholar] [CrossRef]
- McCarthy, J.M.; Watkins, S.; Deivasigamani, A.; John, S.J.; Coman, F. An investigation of fluttering piezoelectric energy harvesters in off-axis and turbulent flows. J. Wind Eng. Ind. Aerodyn. 2015, 136, 101–113. [Google Scholar] [CrossRef]
- Doaré, O.; Michelin, S. Piezoelectric coupling in energy-harvesting fluttering flexible plates: Linear stability analysis and conversion efficiency. J. Fluids Struct. 2011, 27, 1357–1375. [Google Scholar] [CrossRef] [Green Version]
- Petrini, F.; Gkoumas, K. Piezoelectric energy harvesting from vortex shedding and galloping induced vibrations inside HVAC ducts. Energy Build. 2018, 158, 371–383. [Google Scholar] [CrossRef]
- Shan, X.; Tian, H.; Cao, H.; Xie, T. Enhancing Performance of a Piezoelectric Energy Harvester System for Concurrent Flutter and Vortex-Induced Vibration. Energies 2020, 13, 3101. [Google Scholar] [CrossRef]
- Yang, K.; Su, K.; Wang, J.; Wang, J.; Yin, K.; Litak, G. Piezoelectric wind energy harvesting subjected to the conjunction of vortex-induced vibration and galloping: Comprehensive parametric study and optimization. Smart Mater. Struct. 2020, 29, 075035. [Google Scholar] [CrossRef]
- Abdelkefi, A. Aeroelastic energy harvesting: A review. Int. J. Eng. Sci. 2016, 100, 112–135. [Google Scholar] [CrossRef]
- Li, D.; Wu, Y.; Da Ronch, A.; Xiang, J. Energy harvesting by means of flow-induced vibrations on aerospace vehicles. Prog. Aeosp. Sci. 2016, 86, 28–62. [Google Scholar] [CrossRef] [Green Version]
- Rostami, A.B.; Armandei, M. Renewable energy harvesting by vortex-induced motions: Review and benchmarking of technologies. Renew. Sustain. Energy Rev. 2017, 70, 193–214. [Google Scholar] [CrossRef]
- Wei, C.; Jing, X. A comprehensive review on vibration energy harvesting: Modelling and realization. Renew. Sustain. Energy Rev. 2017, 74, 1–18. [Google Scholar] [CrossRef]
- Zou, H.; Zhao, L.; Gao, Q.; Zuo, L.; Liu, F.; Tan, T.; Wei, K.; Zhang, W. Mechanical modulations for enhancing energy harvesting: Principles, methods and applications. Appl. Energy 2019, 255, 113871. [Google Scholar] [CrossRef]
- Watson, S.; Moro, A.; Reis, V.; Baniotopoulos, C.; Barth, S.; Bartoli, G.; Bauer, F.; Boelman, E.; Bosse, D.; Cherubini, A.; et al. Future emerging technologies in the wind power sector: A European perspective. Renew. Sustain. Energy Rev. 2019, 113, 109270. [Google Scholar] [CrossRef]
- Wang, J.; Geng, L.; Ding, L.; Zhu, H.; Yurchenko, D. The state-of-the-art review on energy harvesting from flow-induced vibrations. Appl. Energy 2020, 267, 114902. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Hajj, M.R.; Nayfeh, A.H. Power harvesting from transverse galloping of square cylinder. Nonlinear Dyn. 2012, 70, 1355–1363. [Google Scholar] [CrossRef]
- Zhao, L.; Tang, L.; Yang, Y. Comparison of modeling methods and parametric study for a piezoelectric wind energy harvester. Smart Mater. Struct. 2013, 22, 125003. [Google Scholar] [CrossRef]
- Tan, T.; Yan, Z.; Lei, H. Optimization and performance comparison for galloping-based piezoelectric energy harvesters with alternating-current and direct-current interface circuits. Smart Mater. Struct. 2017, 26, 075007. [Google Scholar] [CrossRef]
- Bibo, A.; Daqaq, M.F. An analytical framework for the design and comparative analysis of galloping energy harvesters under quasi-steady aerodynamics. Smart Mater. Struct. 2015, 24, 094006. [Google Scholar] [CrossRef]
- Bibo, A.; Daqaq, M.F. On the optimal performance and universal design curves of galloping energy harvesters. Appl. Phys. Lett. 2014, 104, 023901. [Google Scholar] [CrossRef]
- Yang, Y.; Zhao, L.; Tang, L. Comparative study of tip cross-sections for efficient galloping energy harvesting. Appl. Phys. Lett. 2013, 102, 064105. [Google Scholar] [CrossRef]
- Kluger, J.M.; Moon, F.C.; Rand, R.H. Shape optimization of a blunt body Vibro-wind galloping oscillator. J. Fluids Struct. 2013, 40, 185–200. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Yan, Z.; Hajj, M.R. Performance analysis of galloping-based piezoaeroelastic energy harvesters with different cross-section geometries. J. Intell. Mater. Syst. Struct. 2014, 25, 246–256. [Google Scholar] [CrossRef]
- Hu, G.; Tse, K.T.; Kwok, K.C.S.; Song, J.; Lyu, Y. Aerodynamic modification to a circular cylinder to enhance the piezoelectric wind energy harvesting. Appl. Phys. Lett. 2016, 109, 193902. [Google Scholar] [CrossRef]
- Hu, G.; Liu, F.; Li, L.; Li, C.; Xiao, Y.; Kwok, K.C.S. Wind energy harvesting performance of tandem circular cylinders with triangular protrusions. J. Fluids Struct. 2019, 91, 102780. [Google Scholar] [CrossRef]
- Song, J.; Hu, G.; Tse, K.T.; Li, S.W.; Kwok, K.C.S. Performance of a circular cylinder piezoelectric wind energy harvester fitted with a splitter plate. Appl. Phys. Lett. 2017, 111, 223903. [Google Scholar] [CrossRef]
- Alhadidi, A.H.; Alhussein, H.; Daqaq, M.F. Improving the sensitivity of galloping energy harvesters to flow fluctuations. Appl. Phys. Lett. 2020, 116, 263902. [Google Scholar] [CrossRef]
- Bot, P. Force Variations Related to Flow Pattern Changes Around a High-Camber Thin Wing. AIAA J. 2019, 58, 1906–1912. [Google Scholar] [CrossRef]
- Bot, P.; Rabaud, M.; Thomas, G.; Lombardi, A.; Lebret, C. Sharp Transition in the Lift Force of a Fluid Flowing Past Nonsymmetrical Obstacles: Evidence for a Lift Crisis in the Drag Crisis Regime. Phys. Rev. Lett. 2016, 117, 234501. [Google Scholar] [CrossRef] [Green Version]
- Souppez, J.-B.R.G.; Bot, P.; Viola, I.M. Turbulent flow around circular arcs. Phys. Fluids 2022, 34, 015121. [Google Scholar] [CrossRef]
- Harvey, S.T.; Khovanov, I.A.; Denissenko, P. A galloping energy harvester with flow attachment. Appl. Phys. Lett. 2019, 114, 104103. [Google Scholar] [CrossRef] [Green Version]
- Liu, F.-R.; Zou, H.-X.; Zhang, W.-M.; Peng, Z.-K.; Meng, G. Y-type three-blade bluff body for wind energy harvesting. Appl. Phys. Lett. 2018, 112, 233903. [Google Scholar] [CrossRef]
- Liu, F.-R.; Zhang, W.-M.; Peng, Z.-K.; Meng, G. Fork-shaped bluff body for enhancing the performance of galloping-based wind energy harvester. Energy 2019, 183, 92–105. [Google Scholar] [CrossRef]
- Hu, G.; Tse, K.T.; Wei, M.; Naseer, R.; Abdelkefi, A.; Kwok, K.C.S. Experimental investigation on the efficiency of circular cylinder-based wind energy harvester with different rod-shaped attachments. Appl. Energy 2018, 226, 682–689. [Google Scholar] [CrossRef]
- Wang, J.; Zhou, S.; Zhang, Z.; Yurchenko, D. High-performance piezoelectric wind energy harvester with Y-shaped attachments. Energy Convers. Manag. 2019, 181, 645–652. [Google Scholar] [CrossRef]
- Ding, L.; Zhang, L.; Bernitsas, M.M.; Chang, C.-C. Numerical simulation and experimental validation for energy harvesting of single-cylinder VIVACE converter with passive turbulence control. Renew. Energy 2016, 85, 1246–1259. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Z.; Qin, W.; Zhu, P.; Shang, S. Scavenging wind energy by a Y-shaped bi-stable energy harvester with curved wings. Energy 2018, 153, 400–412. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Han, Z.-H.; Shi, L.; Song, W.-P. Multi-round Surrogate-based Optimization for Benchmark Aerodynamic Design Problems. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; AIAA SciTech Forum. American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2016. [Google Scholar]
- Zhang, X.; Xie, F.; Ji, T.; Zhu, Z.; Zheng, Y. Multi-fidelity deep neural network surrogate model for aerodynamic shape optimization. Comput. Methods Appl. Mech. Eng. 2021, 373, 113485. [Google Scholar] [CrossRef]
- Bernardini, E.; Spence, S.M.J.; Wei, D.; Kareem, A. Aerodynamic shape optimization of civil structures: A CFD-enabled Kriging-based approach. J. Wind Eng. Ind. Aerodyn. 2015, 144, 154–164. [Google Scholar] [CrossRef] [Green Version]
- Ding, F.; Kareem, A. A multi-fidelity shape optimization via surrogate modeling for civil structures. J. Wind Eng. Ind. Aerodyn. 2018, 178, 49–56. [Google Scholar] [CrossRef]
- Kuhn, A.M.; Fennel, K. Evaluating ecosystem model complexity for the northwest North Atlantic through surrogate-based optimization. Ocean Model. 2019, 142, 101437. [Google Scholar] [CrossRef]
- Liu, X.; Zhao, W.; Wan, D. Linear reduced order method for design-space dimensionality reduction and flow-field learning in hull form optimization. Ocean Eng. 2021, 237, 109680. [Google Scholar] [CrossRef]
- Wang, G.G.; Shan, S. Review of Metamodeling Techniques in Support of Engineering Design Optimization. J. Mech. Des. 2006, 129, 370–380. [Google Scholar] [CrossRef]
- Forrester, A.I.J.; Keane, A.J. Recent advances in surrogate-based optimization. Prog. Aeosp. Sci. 2009, 45, 50–79. [Google Scholar] [CrossRef]
- Westermann, P.; Evins, R. Surrogate modelling for sustainable building design—A review. Energy Build. 2019, 198, 170–186. [Google Scholar] [CrossRef]
- Queipo, N.V.; Haftka, R.T.; Shyy, W.; Goel, T.; Vaidyanathan, R.; Tucker, P.K. Surrogate-based analysis and optimization. Prog. Aeosp. Sci. 2005, 41, 1–28. [Google Scholar] [CrossRef] [Green Version]
- Zhao, L.; Yang, Y. Enhanced aeroelastic energy harvesting with a beam stiffener. Smart Mater. Struct. 2015, 24, 032001. [Google Scholar] [CrossRef]
- Coleman, H.W.; Steele, W.G. Experimentation, Validation, and Uncertainty Analysis for Engineers, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Parkinson, G.V.; Brooks, N. On the aeroelastic instability of bluff cylinders. J. Appl. Mech. 1961, 28, 252–258. [Google Scholar] [CrossRef]
- Parkinson, G.V.; Smith, J.D. A square prism as an aeroelastic non-linear oscillator. Q. J. Mech. Appl. Math. 1964, 17, 225–239. [Google Scholar] [CrossRef]
- Tan, T.; Yan, Z.; Hajj, M. Electromechanical decoupled model for cantilever-beam piezoelectric energy harvesters. Appl. Phys. Lett. 2016, 109, 101908. [Google Scholar] [CrossRef]
- Den Hartog, J.P. Mechanical Vibrations; McGraw-Hill Book Company: New York, NY, USA, 1956. [Google Scholar]
- Païdoussis, M.P.; Price, S.J.; de Langre, E. Fluid-Structure Interactions: Cross-Flow-Induced Instabilities; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Bernitsas, M.M.; Raghavan, K.; Ben-Simon, Y.; Garcia, E.M.H. VIVACE (Vortex Induced Vibration Aquatic Clean Energy): A New Concept in Generation of Clean and Renewable Energy from Fluid Flow. J. Offshore Mech. Arct. Eng. 2008, 130, 041101. [Google Scholar] [CrossRef]
- Li, X.; Teng, L.; Tang, H.; Chen, J.; Wang, H.; Liu, Y.; Fu, M.; Liang, J. ViPSN: A Vibration-Powered IoT Platform. IEEE Internet Things J. 2020, 8, 1728–1739. [Google Scholar] [CrossRef]
- Zhao, L.; Tang, L.; Yang, Y. Synchronized charge extraction in galloping piezoelectric energy harvesting. J. Intell. Mater. Syst. Struct. 2016, 27, 453–468. [Google Scholar] [CrossRef]
- Li, S.; Yuan, J.; Lipson, H. Ambient wind energy harvesting using cross-flow fluttering. J. Appl. Phys. 2011, 109, 026104. [Google Scholar] [CrossRef] [Green Version]
- Zhao, D.; Hu, X.; Tan, T.; Yan, Z.; Zhang, W. Piezoelectric galloping energy harvesting enhanced by topological equivalent aerodynamic design. Energy Convers. Manag. 2020, 222, 113260. [Google Scholar] [CrossRef]
- Zhang, B.; Mao, Z.; Song, B.; Ding, W.; Tian, W. Numerical investigation on effect of damping-ratio and mass-ratio on energy harnessing of a square cylinder in FIM. Energy 2018, 144, 218–231. [Google Scholar] [CrossRef]
- Zhang, B.; Song, B.; Mao, Z.; Tian, W.; Li, B. Numerical investigation on VIV energy harvesting of bluff bodies with different cross sections in tandem arrangement. Energy 2017, 133, 723–736. [Google Scholar] [CrossRef]








| Variable | Resolution | Accuracy |
|---|---|---|
| Velocity | 0.01 m/s | ±(0.03 m/s + 5% of the measured value) |
| Width of bluff body | 0.025 mm | ±2.4% |
| Length of bluff body | 0.025 mm | ±0.4% |
| Force | 0.00625 N | ±1% |
| Wind direction | 0.017° | ±0.5% |
| Resistance | 1000 Ω | ±1% |
| Voltage | 3.6 × 10−6 V | ±0.03% |
| U (m/s) | SCFy (%) |
|---|---|
| 1 | 16.2 |
| 2 | 13.3 |
| 3 | 12.3 |
| 4 | 11.8 |
| 5 | 11.5 |
| 6 | 11.3 |
| 7 | 11.2 |
| Bluff Body | Power (mW) | Wind Velocity (m/s) | Efficiency (%) | ||||
|---|---|---|---|---|---|---|---|
| No. | Author | Shape | Width (cm) | Height (cm) | |||
| 1 | Zhao [66] | Square | 2 | 10 | 1.25 | 5 | 0.77 |
| 2 | Sirohi [67] | D-shape | 3 | 23.5 | 1.14 | 4.7 | 0.24 |
| 3 | Hu [33] | Cylinder with rods | 4.8 | 24 | 0.053 | 5.5 | 0.004 |
| 4 | Hu [7] | Square with fins | 2.4 | 24 | 0.034 | 5 | 0.007 |
| 5 | Song [35] | Cylinder with plate | 4.8 | 24 | 0.014 | 5 | 0.002 |
| 6 | Our work | Square | 5 | 10 | 0.50 | 5 | 0.12 |
| 7 | Our work | Arc-plate | 5 | 10 | 1.01 | 5 | 0.25 |
| 8 | Our work | Square | 5 | 10 | 1.12 | 7 | 0.10 |
| 9 | Our work | Arc-plate | 5 | 10 | 2.31 | 7 | 0.21 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, T.; Hu, G.; Zou, L. Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting. Appl. Sci. 2022, 12, 3965. https://doi.org/10.3390/app12083965
Shi T, Hu G, Zou L. Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting. Applied Sciences. 2022; 12(8):3965. https://doi.org/10.3390/app12083965
Chicago/Turabian StyleShi, Tianyi, Gang Hu, and Lianghao Zou. 2022. "Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting" Applied Sciences 12, no. 8: 3965. https://doi.org/10.3390/app12083965
APA StyleShi, T., Hu, G., & Zou, L. (2022). Aerodynamic Shape Optimization of an Arc-Plate-Shaped Bluff Body via Surrogate Modeling for Wind Energy Harvesting. Applied Sciences, 12(8), 3965. https://doi.org/10.3390/app12083965






