A Polynomial Inversion-Based Fast Time-Delay Estimation Method for Wideband Large-Scale Antenna Systems
Abstract
:1. Introduction
- (1)
- Taking the convex parabolic extremum equation determined by the three sets of cross-correlation values as TED and feeding the result of TED into the polynomial inversion equation, an accurate fractional delay estimate with the desired performance and a lower computational complexity can be obtained.
- (2)
- The CRLB is produced to adapt to more scenarios. The bias, variance, and MSE equations of the new algorithm are also derived. Combined with the CRLB and existing approaches, the simulations show that the proposed time-delay estimator is asymptotically unbiased, with its MSE performing on the CRLB.
- (3)
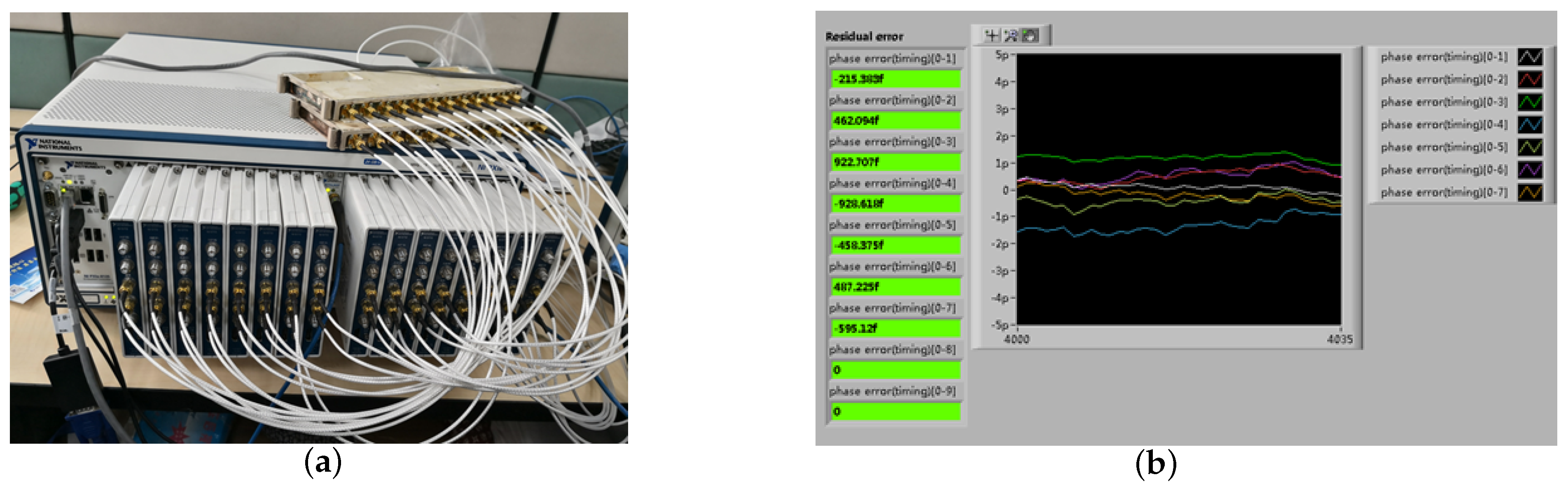
- Applying the algorithm to the RF multichannel broadband acquisition system, the synchronization error between the channels is less than 5 ps.
2. Introduction of Fast Delay Estimation Based on BCC
2.1. Signal Model
2.2. Fast Estimators of Time-Delay
3. Proposed Fast TDE Algorithm
3.1. Principle of Polynomial Inversion-Based Time Delay Estimation
3.2. Summary of the Proposed Algorithm
- (i)
- (ii)
- Conducting correlation calculations using the acquired signals and obtaining , , respectively;
- (iii)
- Substituting the three sets obtained into Equation (8), yielding ;
- (iv)
- Substituting into Equation (13) to yield the fractional time-delay estimate ;
- (v)
- Finally, the time difference is computed as: .
4. Performance Evaluation and Analysis
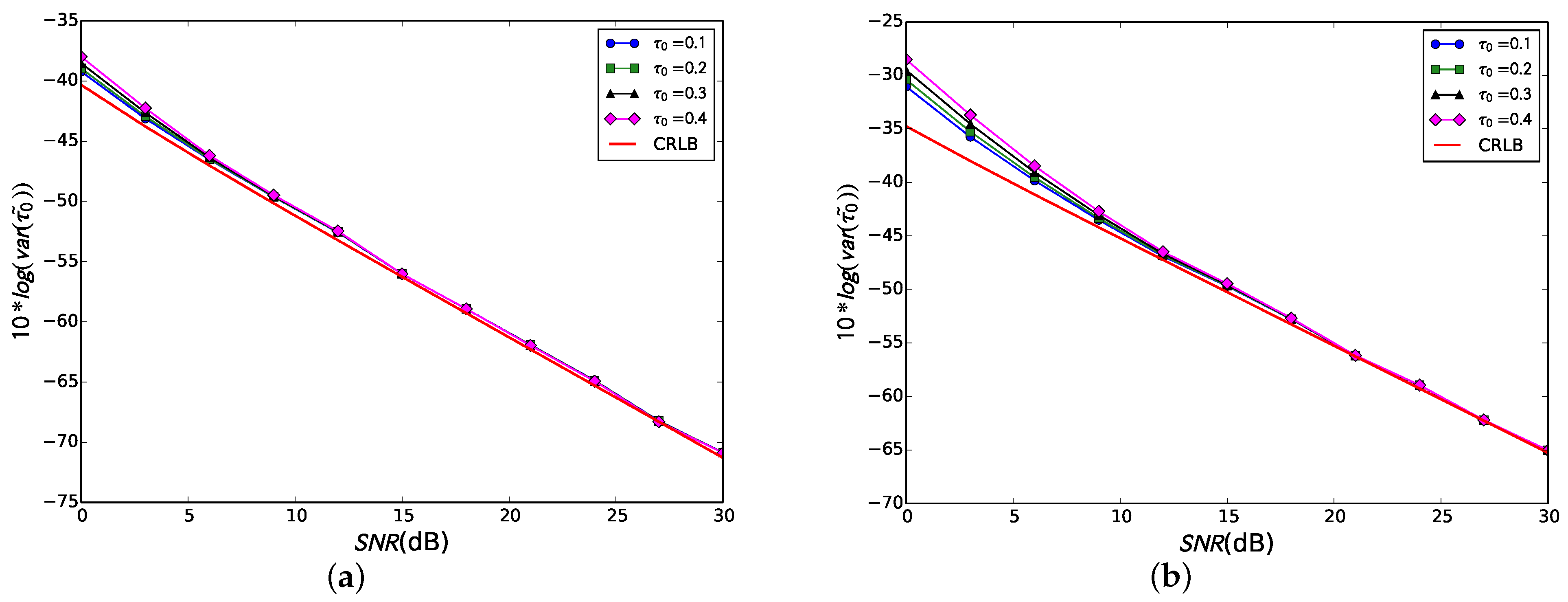
4.1. Modified Performance Limits
4.2. Performance Analysis
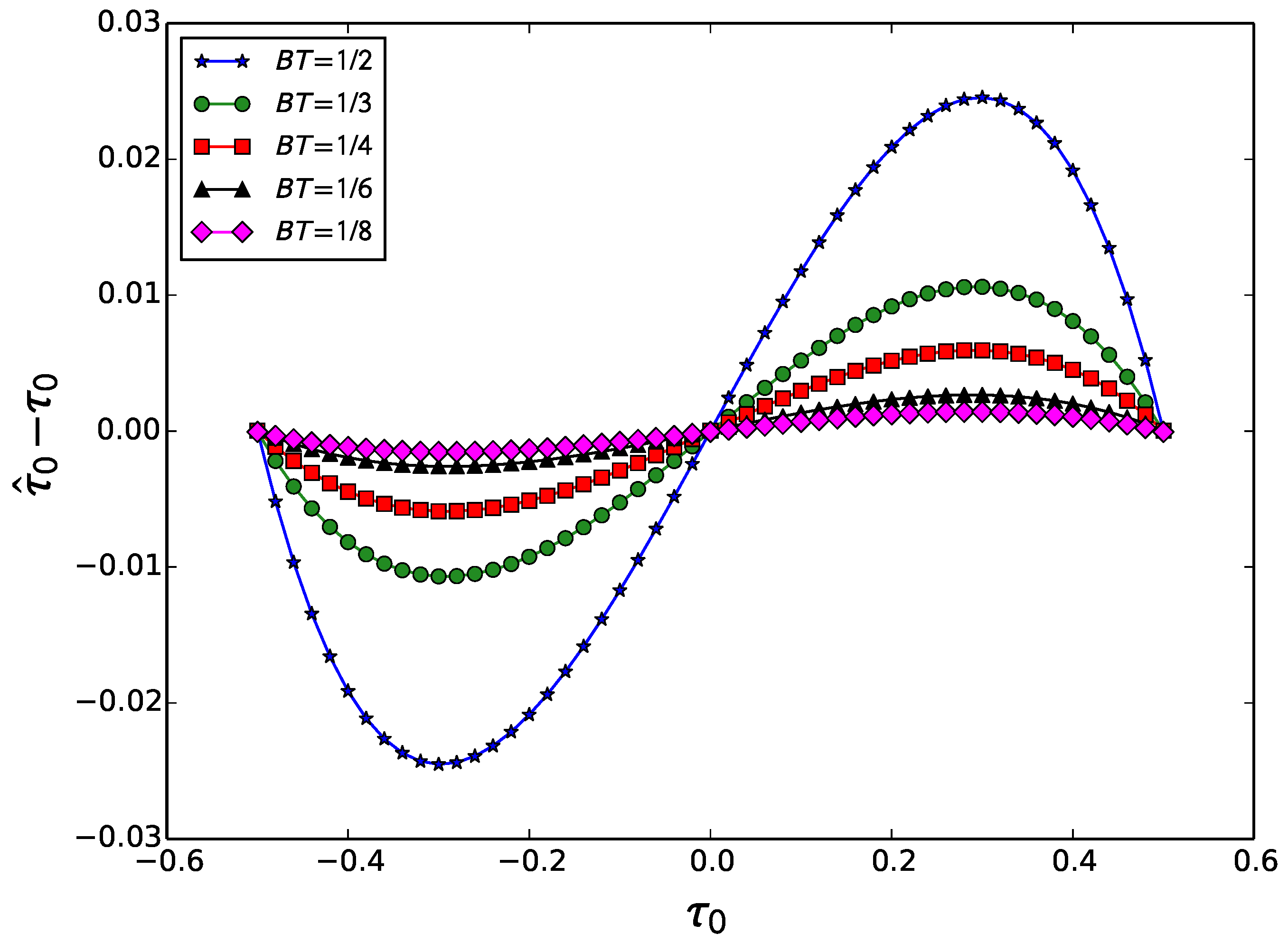
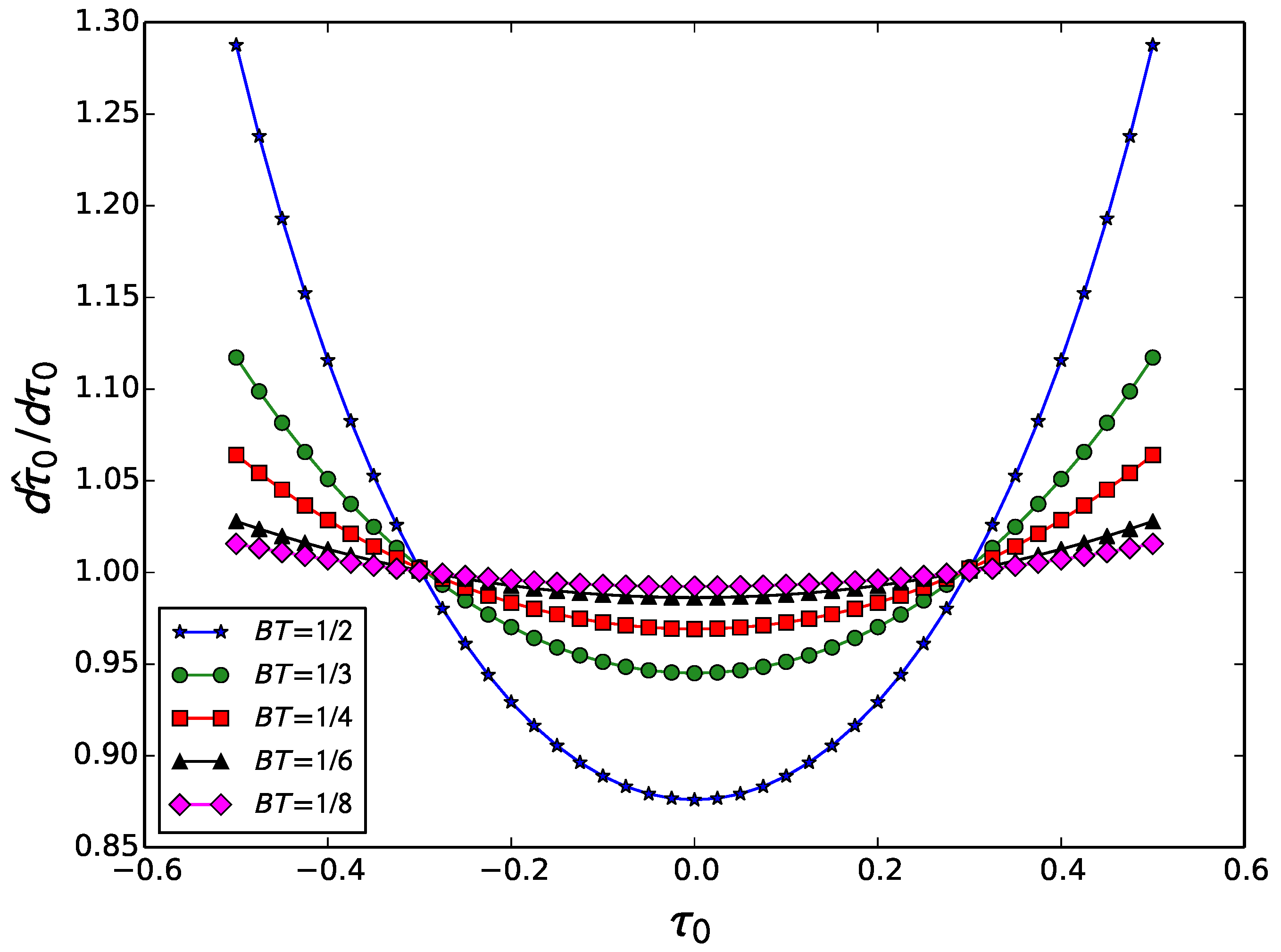
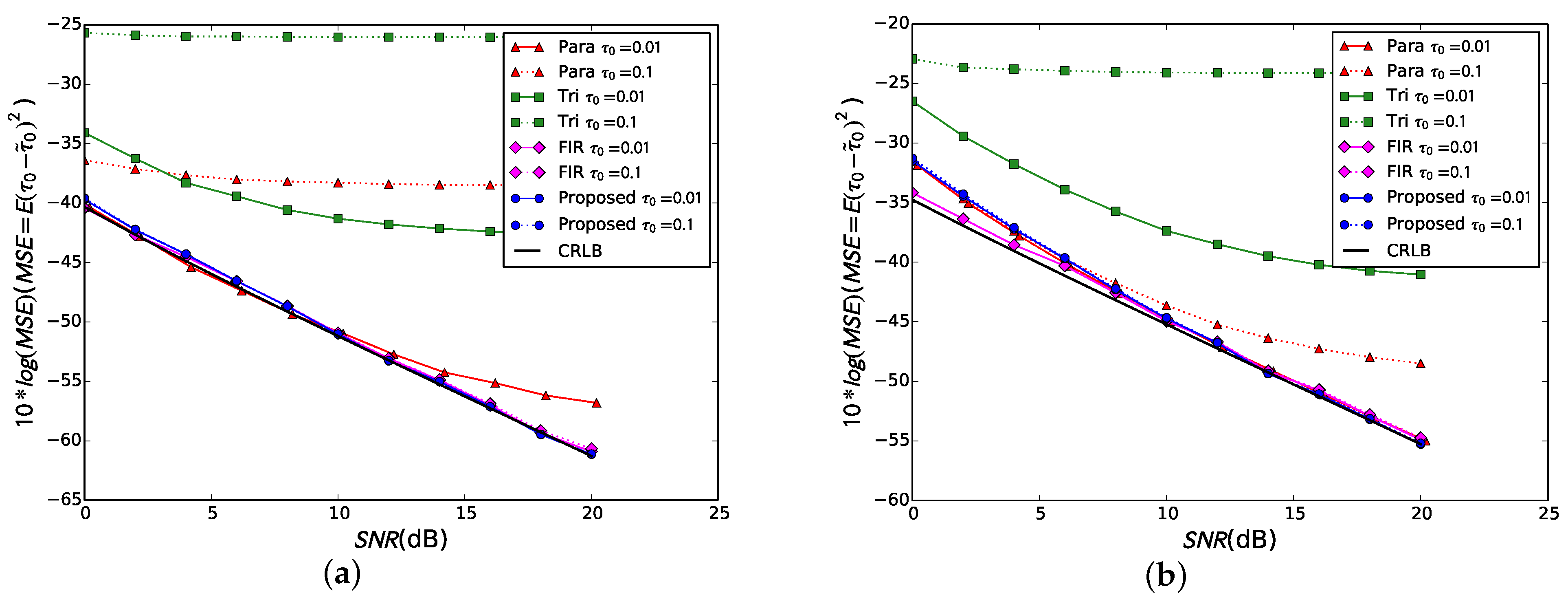
5. Simulations and Discussions
5.1. Simulations
5.2. Evaluations
5.3. Comparison with Existing Approaches
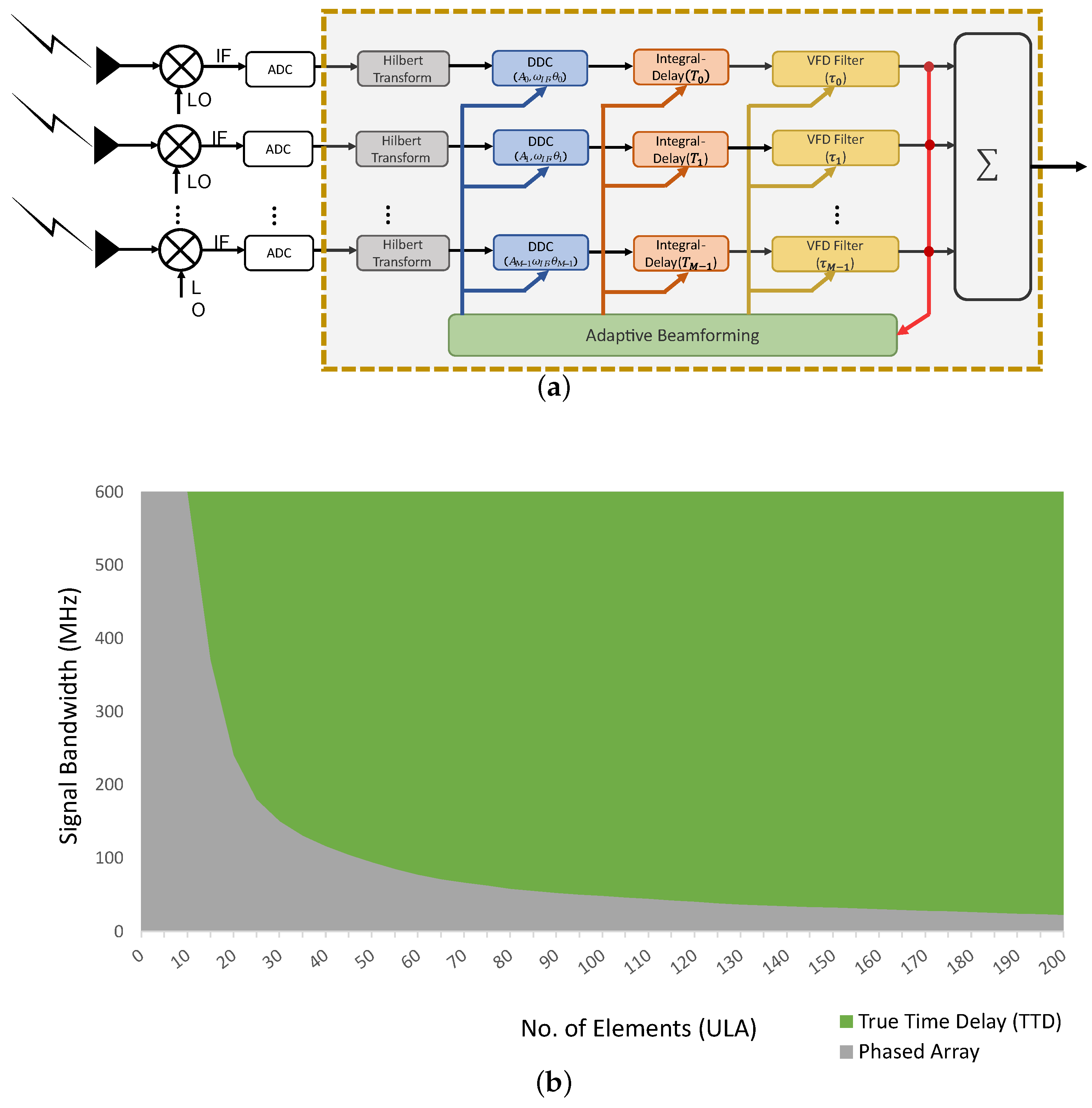
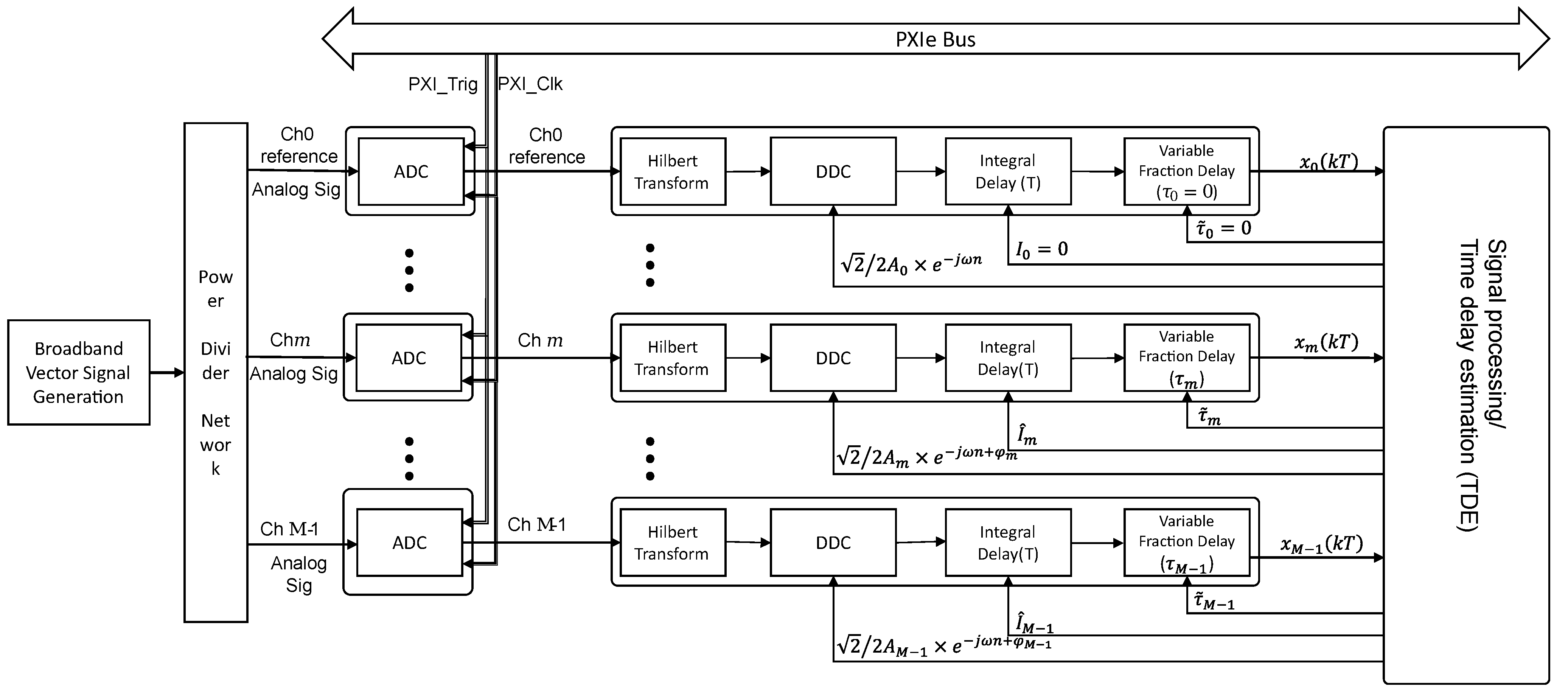
6. TDE-Based Application
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Padois, T.; Doutres, O.; Sgard, F. On the use of modified phase transform weighting functions for acoustic imaging with the generalized cross correlation. J. Acoust. Soc. Am. 2019, 145, 1546–1555. [Google Scholar] [CrossRef] [PubMed]
- Kousiopoulos, G.P.; Papastavrou, G.N.; Kampelopoulos, D.; Karagiorgos, N.; Nikolaidis, S. Comparison of Time Delay Estimation Methods Used for Fast Pipeline Leak Localization in High-Noise Environment. Technologies 2020, 8, 27. [Google Scholar] [CrossRef]
- Fu, L.F.; Xin, G.; Zhang, S.L. Super-Resolution Time-Delays Estimation in HF Channel Sounding Based on Chirp Sequence. Adv. Mater. Res. 2011, 204, 2133–2139. [Google Scholar] [CrossRef]
- Bas, C.U.; Kristem, V.; Wang, R.; Molisch, A.F. Real-Time Ultra-Wideband Channel Sounder Design for 3–18 GHz. IEEE Trans. Commun. 2019, 67, 2995–3008. [Google Scholar] [CrossRef]
- Tian, Y.P.; Chun, S.; Chen, G.; Zong, S.; Huang, Y.; Wang, B. Delay Compensation-Based Time Synchronization Under Random Delays: Algorithm and Experiment. IEEE Trans. Control Syst. Technol. 2021, 29, 80–95. [Google Scholar] [CrossRef]
- Benedetto, F.; Giunta, G. A Fast Time-Delay Estimator of PN Signals. IEEE Trans. Commun. 2011, 59, 2057–2062. [Google Scholar] [CrossRef]
- Barott, W.C.; Milgrome, O.; Wright, M.; MacMahon, D.; Kilsdonk, T.; Backus, P.; Dexter, M. Real-time beamforming using high-speed FPGAs at the Allen Telescope Array: Real-time FPGA beamforming at the ATA. Radio Sci. 2011, 46, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Boljanovic, V.; Yan, H.; Lin, C.C.; Mohapatra, S.; Heo, D.; Gupta, S.; Cabric, D. Fast Beam Training with True-Time-Delay Arrays in Wideband Millimeter-Wave Systems. IEEE Trans. Circuits Syst. I Regul. Pap. 2021, 68, 1727–1739. [Google Scholar] [CrossRef]
- Sarieddeen, H.; Alouini, M.S.; Al-Naffouri, T.Y. An overview of signal processing techniques for terahertz communications. Proc. IEEE 2021, 109, 1628–1665. [Google Scholar] [CrossRef]
- Sikri, D.; Jayasuriya, R.M. Multi-beam phased array with full digital beamforming for SATCOM and 5G. Microw. J. 2019, 62, 64–79. [Google Scholar]
- Giunta, G. Fast estimators of time delay and Doppler stretch based on discrete-time methods. IEEE Trans. Signal Process. 1998, 46, 1785–1797. [Google Scholar] [CrossRef]
- Chao, D.; Zhe, C.; Zhang, Z.; Cheng, Y. Time Delay Estimation Using Cross-power Spectrum Phase. J. Electron. Inf. Technol. 2021, 43, 803–808. [Google Scholar] [CrossRef]
- Dooley, S.; Nandi, A. Adaptive subsample time delay estimation using Lagrange interpolators. IEEE Signal Process. Lett. 1999, 6, 65–67. [Google Scholar] [CrossRef]
- Johansson, H.; Gustafsson, O.; Johansson, K.; Wanhammar, L. Adjustable Fractional-Delay FIR Filters Using the Farrow Structure and Multirate Techniques. In Proceedings of the APCCAS 2006—2006 IEEE Asia Pacific Conference on Circuits and Systems, Singapore, 4–7 December 2006; IEEE: Singapore, 2006; pp. 1055–1058. [Google Scholar] [CrossRef]
- Cao, H.; So, H.C.; Chan, Y.T. Optimum time delay estimation for complex-valued stationary signals. Signal Process. 2017, 131, 434–440. [Google Scholar] [CrossRef]
- Liu, C.; Li, Y.; Fang, L.; Xu, M. New leak-localization approaches for gas pipelines using acoustic waves. Measurement 2019, 134, 54–65. [Google Scholar] [CrossRef]
- Gedalyahu, K.; Eldar, Y.C. Time-Delay Estimation From Low-Rate Samples: A Union of Subspaces Approach. IEEE Trans. Signal Process. 2010, 58, 3017–3031. [Google Scholar] [CrossRef] [Green Version]
- Gu, Y.; Goodman, N.A. Information-theoretic compressive sensing kernel optimization and Bayesian Cramér–Rao bound for time delay estimation. IEEE Trans. Signal Process. 2017, 65, 4525–4537. [Google Scholar] [CrossRef]
- Wen, F.; Liu, W.; Lu, X. An efficient interpolation based two-step time delay estimation method. In Proceedings of the 2015 IEEE International Conference on Digital Signal Processing (DSP), Singapore, 21–24 July 2015; pp. 931–934. [Google Scholar] [CrossRef]
- So, H. On time delay estimation using an FIR filter. Signal Process. 2001, 81, 1777–1782. [Google Scholar] [CrossRef] [Green Version]
- Li, G.; Wu, J.; Tang, T.; Chen, Z.; Chen, J.; Liu, H. Underwater Acoustic Time Delay Estimation Based on Envelope Differences of Correlation Functions. Sensors 2019, 19, 1259. [Google Scholar] [CrossRef] [Green Version]
- Carter, G. Coherence and time delay estimation. Proc. IEEE 1987, 75, 236–255. [Google Scholar] [CrossRef]
- Giunta, G. Fine estimators of two-dimensional parameters and application to spatial shift estimation. IEEE Trans. Signal Process. 1999, 47, 3201–3207. [Google Scholar] [CrossRef]
- Yuan, L.; Liao, J. Parametric Design of a Microwave Radiometer for Land Surface Temperature Retrieval from Moon-Based Earth Observation Platform. Remote Sens. 2020, 12, 4110. [Google Scholar] [CrossRef]
- Liu, X.; Ren, G.; Zhou, X.; Liu, X. Frequency Offset Estimation Algorithm of High-Order M-APSK Modulation Signal Based on DFT. Mob. Netw. Appl. 2022, 1–12. [Google Scholar] [CrossRef]

| Items | Arguments |
|---|---|
| Normalized bandwidth | |
| Delay difference |
| Methods | Para | Tri | FIR () | Proposed () |
|---|---|---|---|---|
| Multiplicative accumulators | 3 | 3 | 41 | 3 |
| Adders | 3 | 2 | 444 | 14 |
| Multipliers | 2 | 2 | 464 | 13 (Horner rule) |
| No. of matrix inversion | 0 | 0 | 1 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Ren, G.; Yin, X.; Zhang, B.; Wang, Y. A Polynomial Inversion-Based Fast Time-Delay Estimation Method for Wideband Large-Scale Antenna Systems. Appl. Sci. 2022, 12, 3378. https://doi.org/10.3390/app12073378
Liu X, Ren G, Yin X, Zhang B, Wang Y. A Polynomial Inversion-Based Fast Time-Delay Estimation Method for Wideband Large-Scale Antenna Systems. Applied Sciences. 2022; 12(7):3378. https://doi.org/10.3390/app12073378
Chicago/Turabian StyleLiu, Xiaowei, Guangliang Ren, Xiaoman Yin, Bo Zhang, and Yu Wang. 2022. "A Polynomial Inversion-Based Fast Time-Delay Estimation Method for Wideband Large-Scale Antenna Systems" Applied Sciences 12, no. 7: 3378. https://doi.org/10.3390/app12073378
APA StyleLiu, X., Ren, G., Yin, X., Zhang, B., & Wang, Y. (2022). A Polynomial Inversion-Based Fast Time-Delay Estimation Method for Wideband Large-Scale Antenna Systems. Applied Sciences, 12(7), 3378. https://doi.org/10.3390/app12073378






