Structural Damage Localization under Unknown Seismic Excitation Based on Mahalanobis Squared Distance of Strain Transmissibility Function
Abstract
:1. Introduction
2. The Strain Transmissibility Function (TF)
2.1. The Strain TF of the Intact Structure under Unknown Ambient Excitation
2.2. The Strain TF of the Damaged Structure under Unknown Seismic Excitation
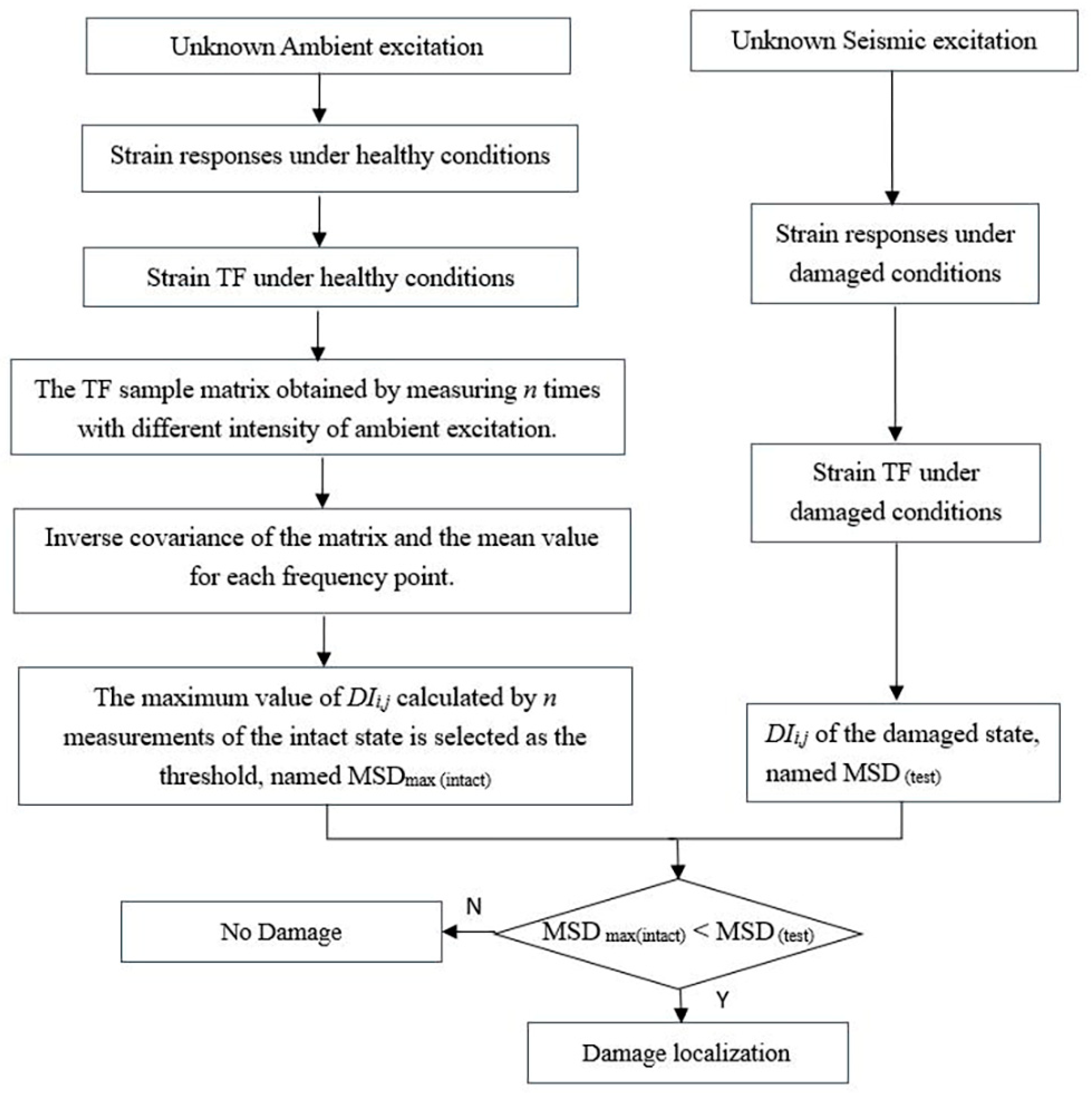
3. The Improved Structural Damage Localization Method
3.1. Mahalanobis Squared Distance (MSD)
3.2. Damage Indicator Based on MSD of Strain TF
4. Numerical Simulation
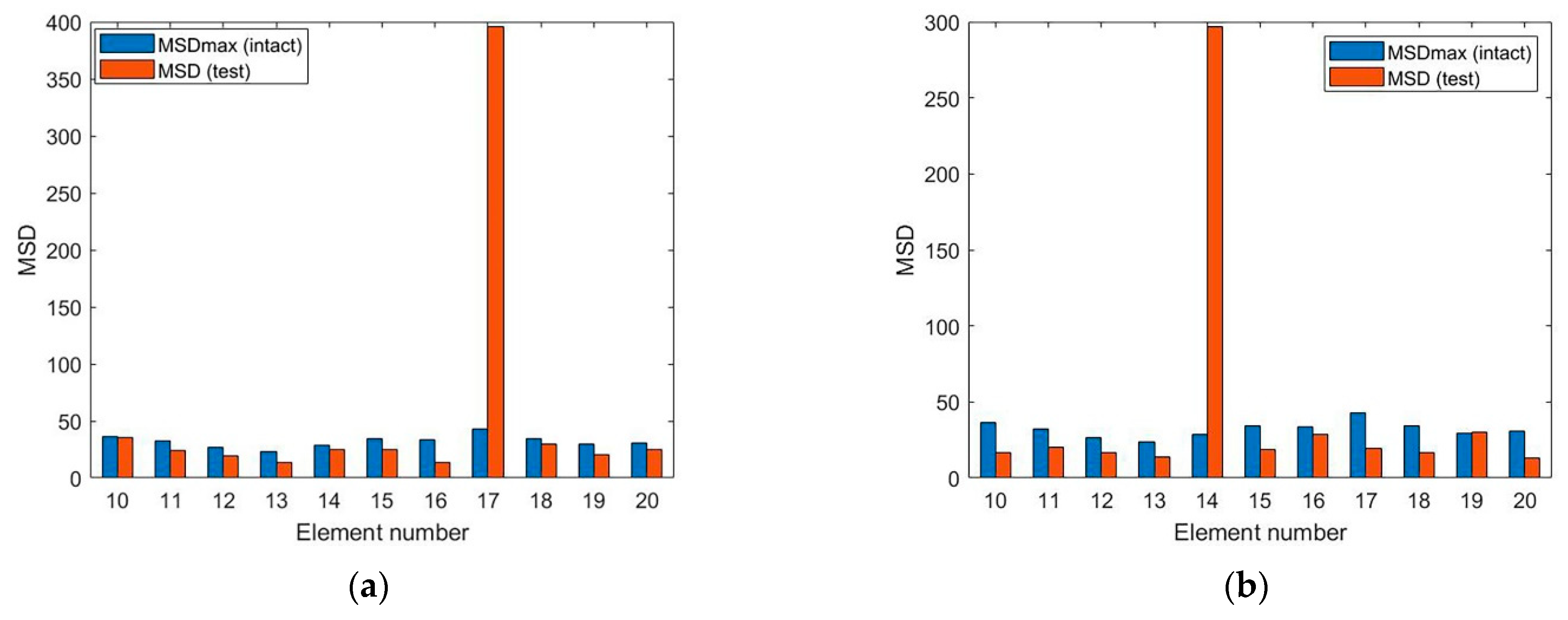
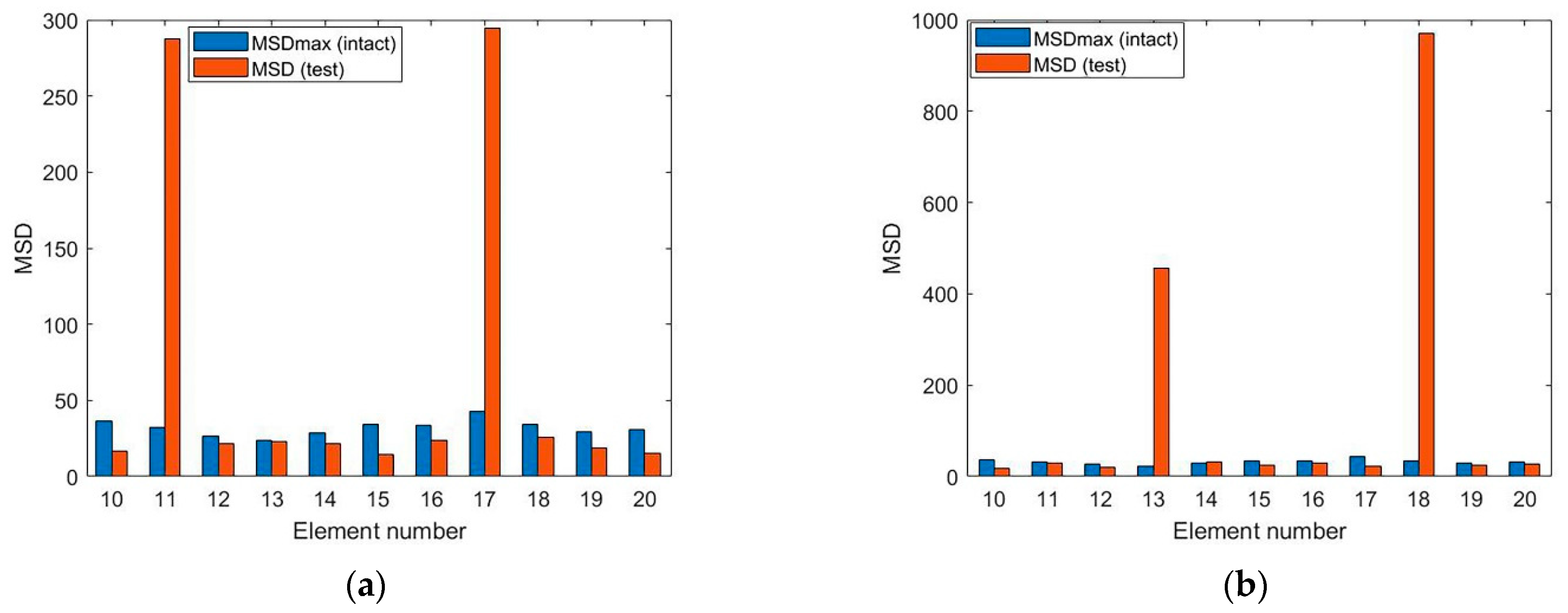
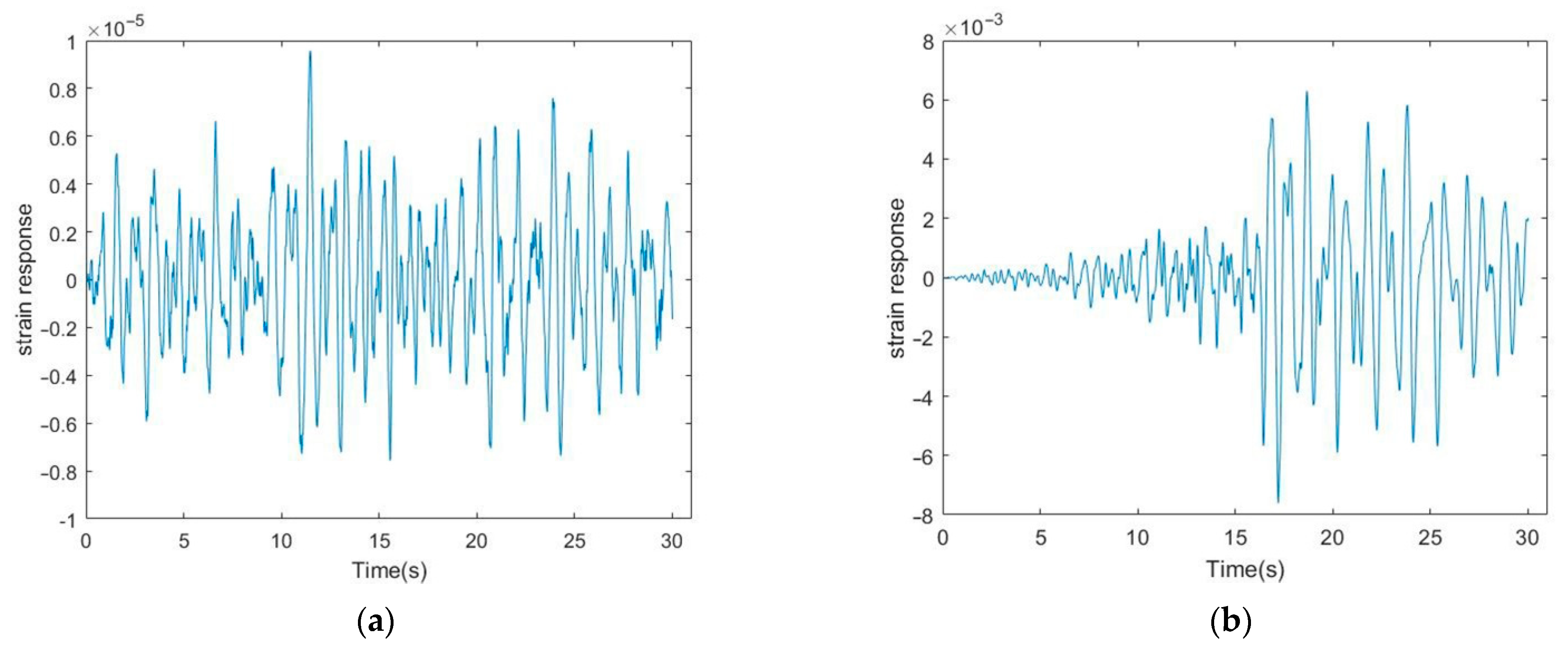
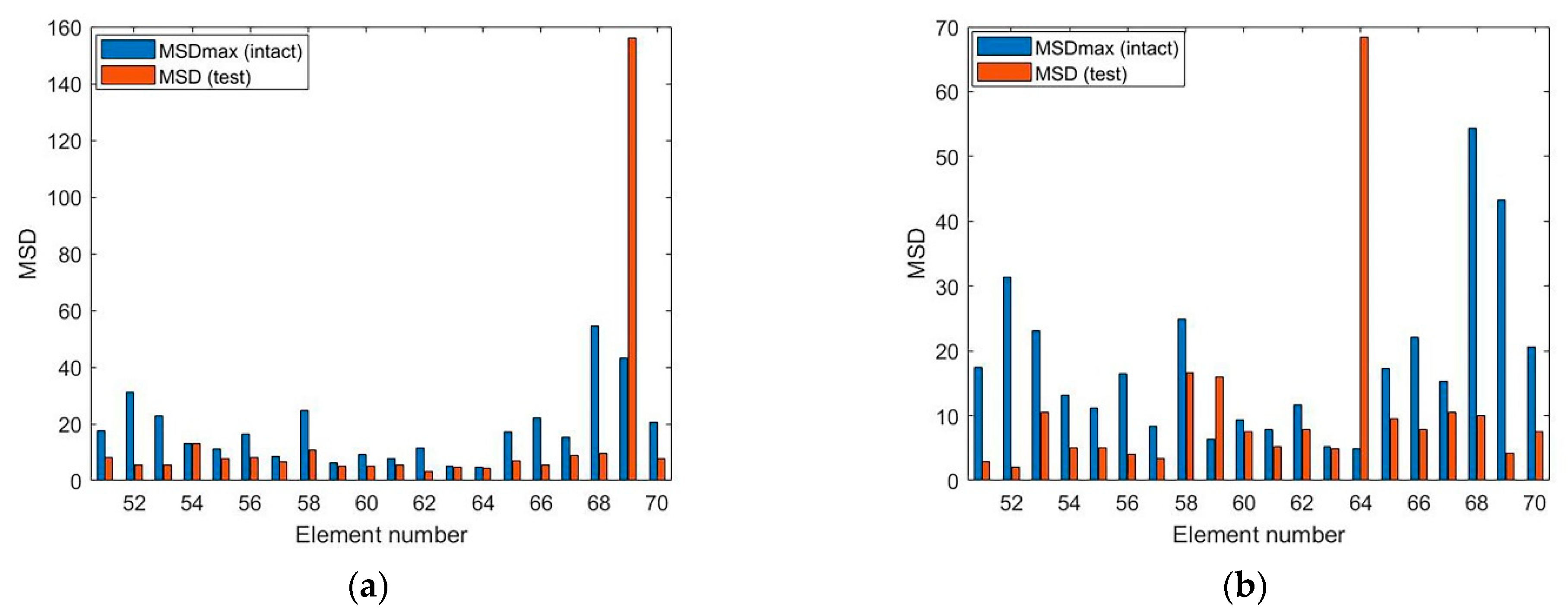
4.1. Damage Localization of a Simply Supported Beam
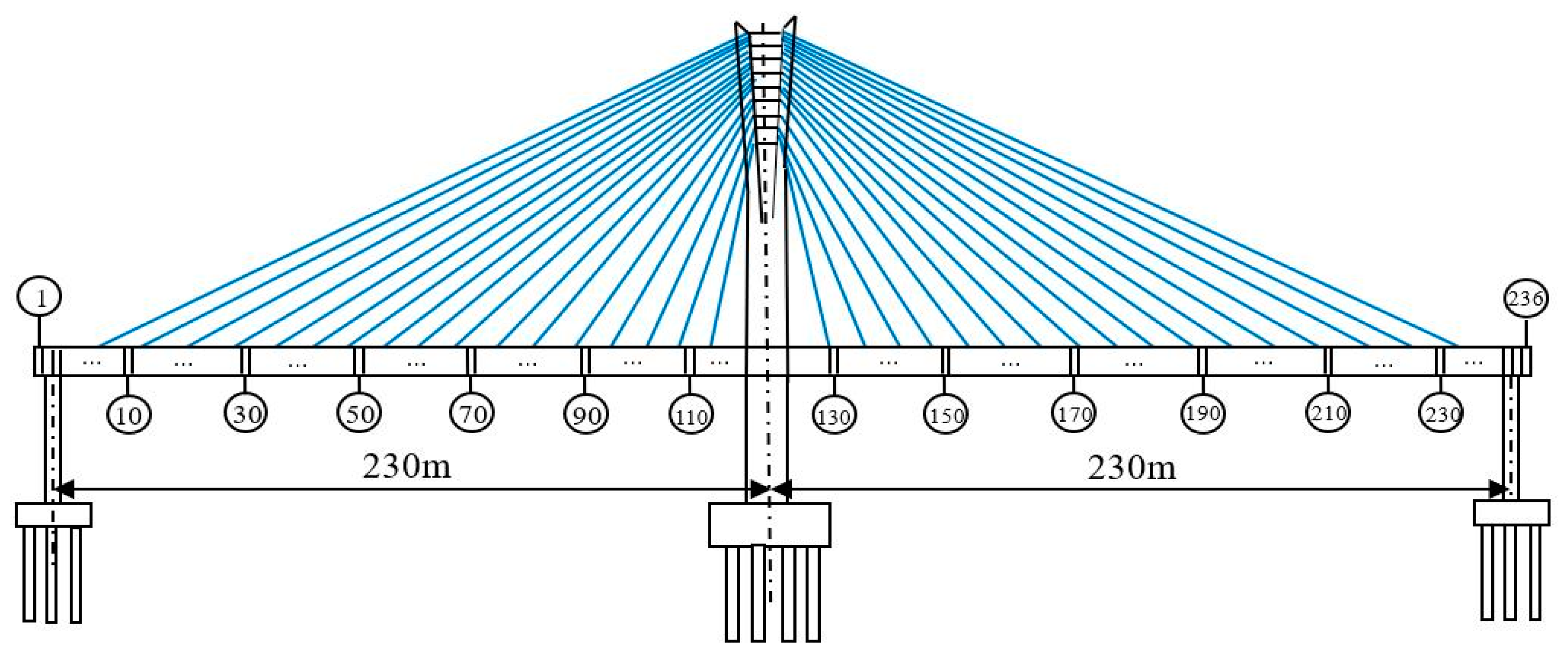
4.2. Damage Localization of a Cable-Stayed Bridge
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rytter, A.; Brincker, R.; Hansen, L.P. Vibration Based Inspection of Civil Engineering Structures. Ph.D. Thesis, Aalborg University, Aalborg, Denmark, 1993. [Google Scholar]
- Fan, W.; Qiao, P.Z. Vibration-based damage identification methods: A review and comparative study. Struct. Health Monit. 2011, 10, 83–111. [Google Scholar] [CrossRef]
- Doebling, S.W.; Farrar, C.R.; Prime, M.B. A summary review of vibration-based damage identification methods. Shock. Vib. Dig. 1998, 30, 91–105. [Google Scholar] [CrossRef] [Green Version]
- Li, J.T.; Zhu, X.Q.; Law, S.S.; Samali, B. A two-step drive-by bridge damage detection using dual Kalman filter. Int. J. Struct. Stab. Dyn. 2020, 20, 2042006. [Google Scholar] [CrossRef]
- Xia, Y.; Lei, X.M.; Wang, P.; Sun, L.M. A data-driven approach for regional bridge condition assessment using inspection reports. Struct. Control Health Monit. 2021, 29, e2915. [Google Scholar] [CrossRef]
- Yan, W.J.; Ren, W.X. An Enhanced Power Spectral Density Transmissibility (EPSDT) approach for operational modal analysis: Theoretical and experimental investigation. Eng. Struct. 2015, 102, 108–119. [Google Scholar] [CrossRef]
- Devriendt, C.; Weijtjens, W.; Sitter, G.D.; Guillaume, P. Combining multiple single-reference transmissibility functions in a unique matrix formulation for operational modal analysis. Mech. Syst. Signal Proc. 2013, 40, 278–287. [Google Scholar] [CrossRef]
- Araújo, I.G.; Sánchez, J.A.G.; Andersen, P. Modal parameter identification based on combining transmissibility functions and blind source separation techniques. Mech. Syst. Signal Proc. 2018, 105, 276–293. [Google Scholar] [CrossRef]
- Yan, W.J.; Zhao, M.Y.; Sun, Q.; Ren, W.X. Transmissibility-based system identification for structural health monitoring: Fundamentals, approaches, and applications. Mech. Syst. Signal Proc. 2019, 117, 453–482. [Google Scholar] [CrossRef]
- Chesne, S.; Deraemaeker, A. Damage localization using transmissibility functions: A critical review. Mech. Syst. Signal Proc. 2013, 38, 569–584. [Google Scholar] [CrossRef] [Green Version]
- Meruane, V. Model updating using antiresonant frequencies identified from transmissibility functions. J. Sound Vibr. 2013, 332, 807–820. [Google Scholar] [CrossRef]
- Yan, W.J.; Katafygiotis, L.S. Application of transmissibility matrix and random matrix to Bayesian system identification with response measurements only. Smart Mater. Struct. 2016, 25, 105017. [Google Scholar] [CrossRef]
- Guasch, O.; García, C.; Jové, J.; Artís, P. Experimental validation of the direct transmissibility approach to classical transfer path analysis on a mechanical setup. Mech. Syst. Signal Proc. 2013, 37, 363–369. [Google Scholar] [CrossRef]
- Seijs, M.V.V.D.; Klerk, D.D.; Rixen, D.J. General framework for transfer path analysis: History, theory and classification of techniques. Mech. Syst. Signal Proc. 2016, 68, 217–244. [Google Scholar] [CrossRef]
- Lage, Y.E.; Maia, N.M.M.; Neves, M.M. Force magnitude reconstruction using the force transmissibility concept. Shock Vib. 2014, 2014, 905912. [Google Scholar] [CrossRef]
- Li, X.W.; Zhao, H.T.; Chen, Z.; Wang, Q.B.; Chen, J.A.; Duan, D.P. Force identification based on a comprehensive approach combining Taylor formula and acceleration transmissibility. Inverse Probl. Sci. Eng. 2017, 26, 1612–1632. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.J.; Waters, T.P.; Lopes, V., Jr. Force and displacement transmissibility of a nonlinear isolator with high-static-low-dynamic stiffness. Int. J. Mech. Sci. 2012, 55, 22–29. [Google Scholar] [CrossRef]
- Maia, N.M.M.; Ribeiro, A.M.R.; Fontul, M.; Montalvão, D.; Sampaio, R.P.C. Using the detection and relative damage quantification indicator (DRQ) with transmissibility. Key Eng. Mater. 2007, 347, 455–460. [Google Scholar] [CrossRef]
- Maia, N.M.M.; Almeida, R.A.B.; Urgueira, A.P.V.; Sampaio, R.P.C. Damage detection and quantification using transmissibility. Mech. Syst. Signal Proc. 2011, 25, 2475–2483. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Figueiredo, E.; Maia, N.; Perera, R. Damage detection and quantification using transmissibility coherence analysis. Shock Vib. 2015, 2015, 290714. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Cao, H.Y.; Liu, Q.M.; Wahab, M.A. Output-based structural damage detection by using correlation analysis together with transmissibility. Materials 2017, 10, 866. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhou, Y.L.; Wahab, M.A. Cosine based and extended transmissibility damage indicators for structural damage detection. Eng. Struct. 2017, 141, 175–183. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Maia, N.M.M.; Sampaio, R.P.C.; Wahab, M.A. Structural damage detection using transmissibility together with hierarchical clustering analysis and similarity measure. Struct. Health Monit. 2017, 16, 711–731. [Google Scholar] [CrossRef]
- Chen, J.B.; Diao, Y.S.; Ren, H. Study on structural damage identification based on the transmissibility function. Struct. Eng. 2014, 30, 32–40. (In Chinese) [Google Scholar]
- Al-Jailawi, S.; Rahmatalla, S. Transmissibility-based damage detection using angular velocity versus acceleration. J. Civ. Struct. Health Monit. 2018, 8, 649–659. [Google Scholar] [CrossRef]
- Cheng, L.L.; Cigada, A. An analytical perspective about structural damage identification based on transmissibility function. Struct. Health Monit. 2020, 19, 142–155. [Google Scholar] [CrossRef]
- Luo, Z.W.; Liu, H.L.; Yu, L. Weighted transmissibility assurance criterion for structural damage detection. J. Aerosp. Eng. 2021, 34, 04021016. [Google Scholar] [CrossRef]
- Luo, Z.W.; Liu, H.L.; Yu, L. A multi-state strategy for structural damage detection using sensitivity of weighted transmissibility function. Int. J. Struct. Stab. Dyn. 2021, 21, 2150144. [Google Scholar] [CrossRef]
- Hou, Z.Q. Structural Damage Identification Based on Cosh Spectral Distance and Transmissibility. Master’s Thesis, Lanzhou University of Technology, Lanzhou, China, 2016. (In Chinese). [Google Scholar]
- Zhou, Y.L.; Figueiredo, E.; Maia, N.; Sampaio, R.; Perera, R. Damage detection in structures using a transmissibility-based Mahalanobis distance. Struct. Control Health Monit. 2015, 22, 1209–1222. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Wahab, M.A. Rapid early damage detection using transmissibility with distance measure analysis under unknown excitation in long-term health monitoring. J. Vibroeng. 2016, 18, 4491–4499. [Google Scholar]
- Chen, J.B. Study on the Structural Damage Identification Based on Acceleration Transmissibility Function. Master’s Thesis, Qingdao Technological University, Qingdao, China, 2013. (In Chinese). [Google Scholar]
- Zhou, Y.L.; Maia, N.M.M.; Wahab, M.A. Damage detection using transmissibility compressed by principal component analysis enhanced with distance measure. J. Vib. Control 2018, 24, 2001–2019. [Google Scholar] [CrossRef]
- Fan, Z.; Feng, X.; Zhou, J. Structural damage detection by using wavelet based transmissibility function. Earthq. Eng. Eng. Dyn. 2014, 34, 28–34. (In Chinese) [Google Scholar]
- Xu, D.F. Structural Damage Identification of Offshore Platform Based on the Vibration Transmissibility Function and Statistical Hypothesis Testing. Master’s Thesis, Qingdao Technological University, Qingdao, China, 2015. (In Chinese). [Google Scholar]
- Chen, C.; Wang, Y.H.; Wang, T.; Yang, X.Y. A Mahalanobis distance cumulant-based structural damage identification method with IMFs and fitting residual of SHM measurements. Math. Probl. Eng. 2020, 2020, 6932463. [Google Scholar] [CrossRef]
- Cheng, L.L.; Busca, G.; Roberto, P.; Vanali, M.; Cigada, A. Damage detection based on strain transmissibility for beam structure by using distributed fiber optics. In Structural Health Monitoring & Damage Detection, Volume 7; Niezrecki, C., Ed.; Springer: Berlin, Germany, 2017; pp. 27–40. [Google Scholar]
- Zhang, X.H.; Zhu, S.; Xu, Y.L.; Homg, X.J. Integrated optimal placement of displacement transducers and strain gauges for better estimation of structural response. Int. J. Struct. Stab. Dyn. 2011, 11, 581–602. [Google Scholar] [CrossRef] [Green Version]
- Shan, D.; Zhou, X.; Yang, J.; Li, Q. Bridge seismic damage identification based on seismic fragility analysis. J. Vib. Shock 2017, 36, 195–201. (In Chinese) [Google Scholar]


| Beam Properties | Value |
|---|---|
| Moment of inertia | 2.326 m4 |
| Young’s modulus | 210 Gpa |
| Unit length mass | 17,555 kg/m |
| Damping ratio | 0.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Zhang, X.; Lei, Y.; Zheng, Z. Structural Damage Localization under Unknown Seismic Excitation Based on Mahalanobis Squared Distance of Strain Transmissibility Function. Appl. Sci. 2022, 12, 3115. https://doi.org/10.3390/app12063115
Liu L, Zhang X, Lei Y, Zheng Z. Structural Damage Localization under Unknown Seismic Excitation Based on Mahalanobis Squared Distance of Strain Transmissibility Function. Applied Sciences. 2022; 12(6):3115. https://doi.org/10.3390/app12063115
Chicago/Turabian StyleLiu, Lijun, Xin Zhang, Ying Lei, and Zhupeng Zheng. 2022. "Structural Damage Localization under Unknown Seismic Excitation Based on Mahalanobis Squared Distance of Strain Transmissibility Function" Applied Sciences 12, no. 6: 3115. https://doi.org/10.3390/app12063115
APA StyleLiu, L., Zhang, X., Lei, Y., & Zheng, Z. (2022). Structural Damage Localization under Unknown Seismic Excitation Based on Mahalanobis Squared Distance of Strain Transmissibility Function. Applied Sciences, 12(6), 3115. https://doi.org/10.3390/app12063115








