Progress in Topological Mechanics
Abstract
:1. Introduction
2. Mechanical Topological Edge Sates
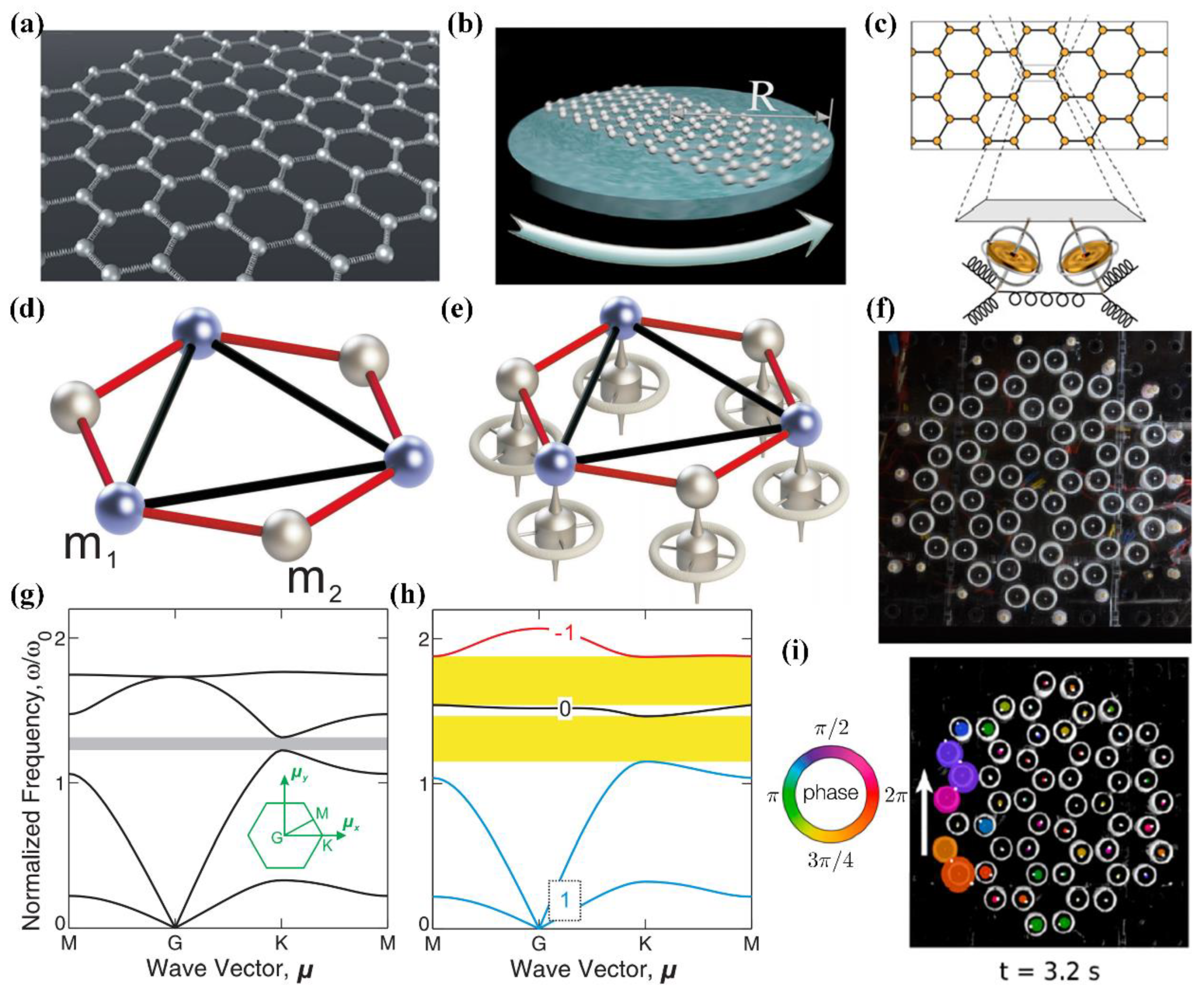
2.1. Analogue Hall Insulators
2.2. Analogue Spin Hall Insulators
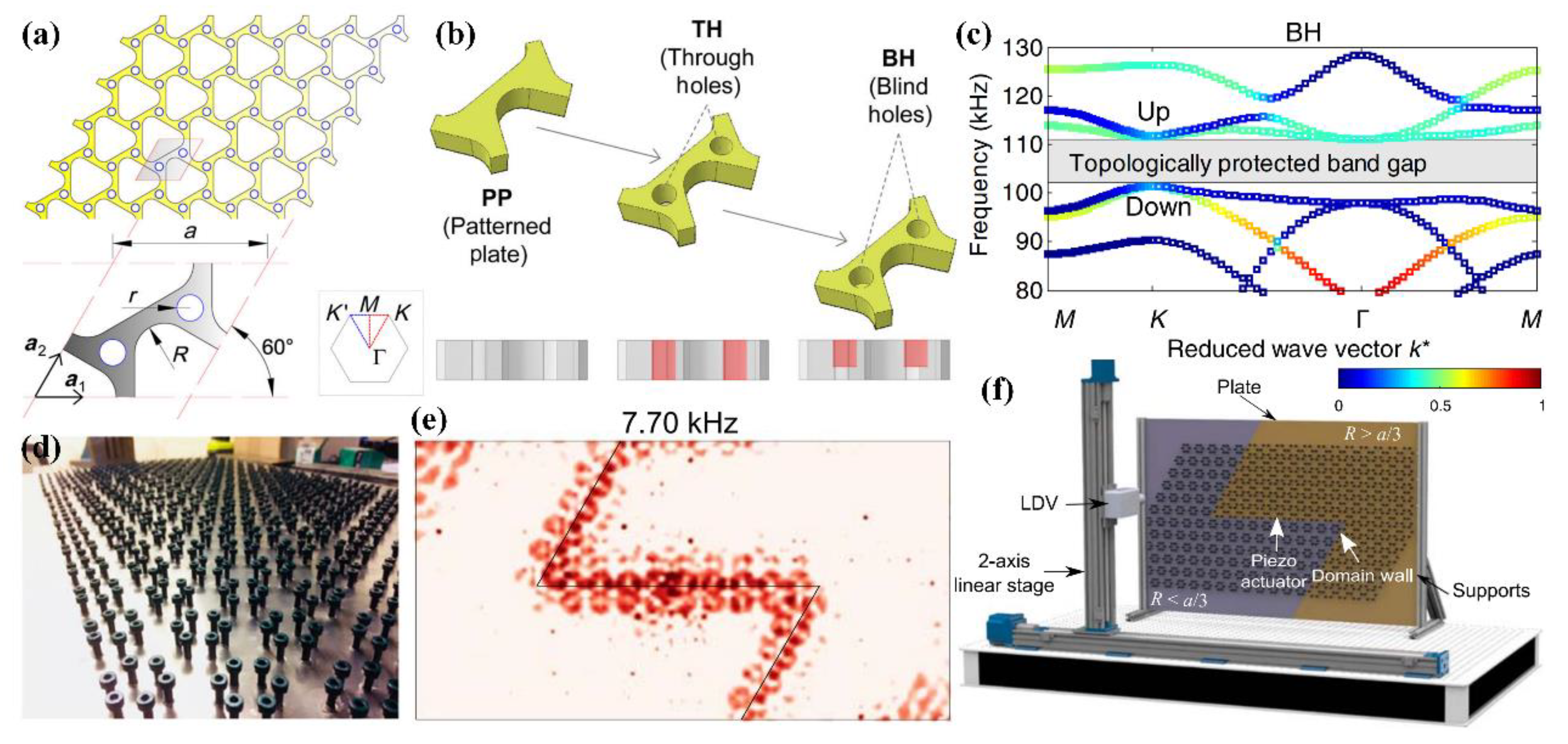
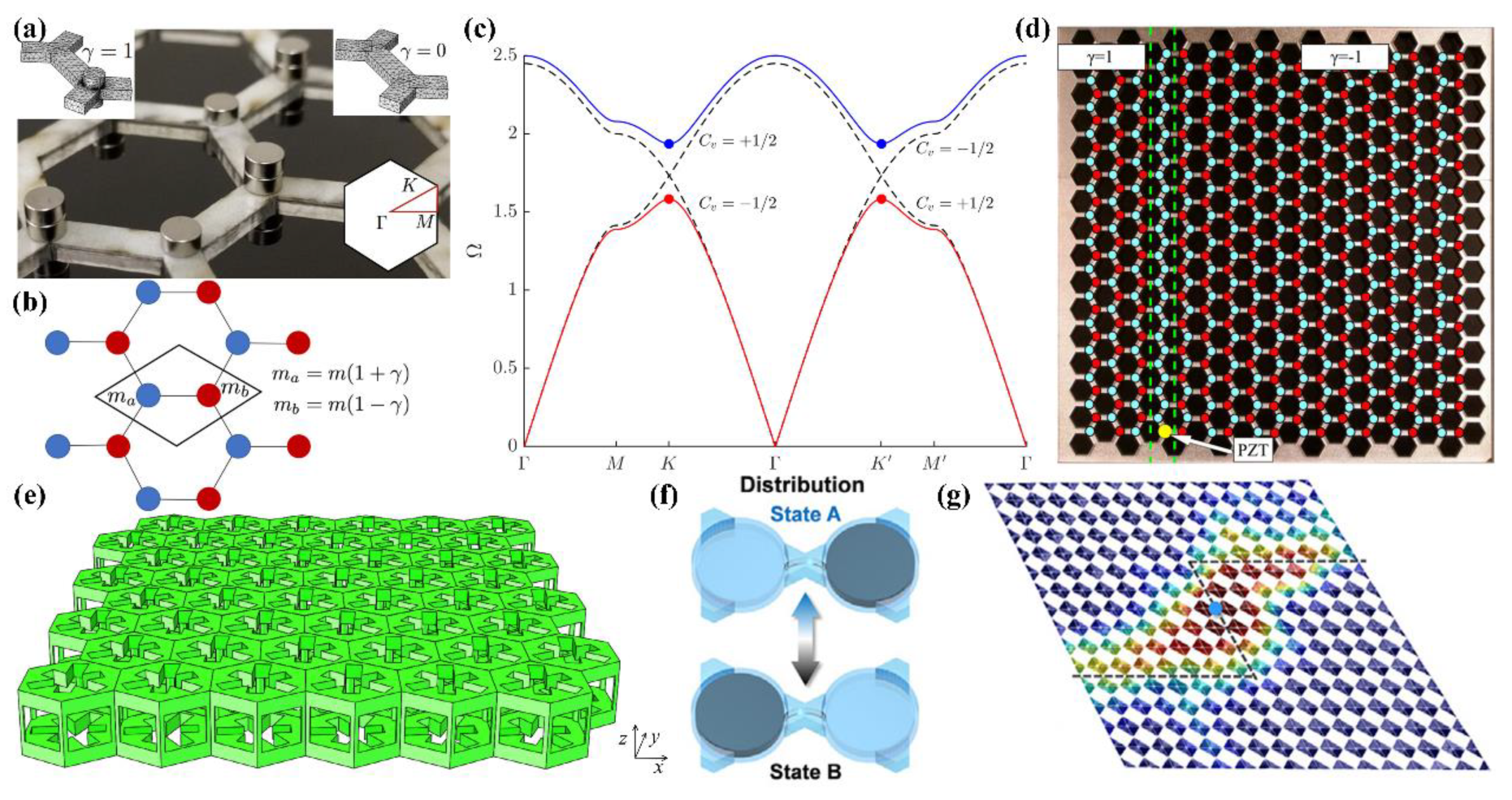
2.3. Analogue Valley Hall Insulators
3. Mechanical Higher-Order Topological Insulators
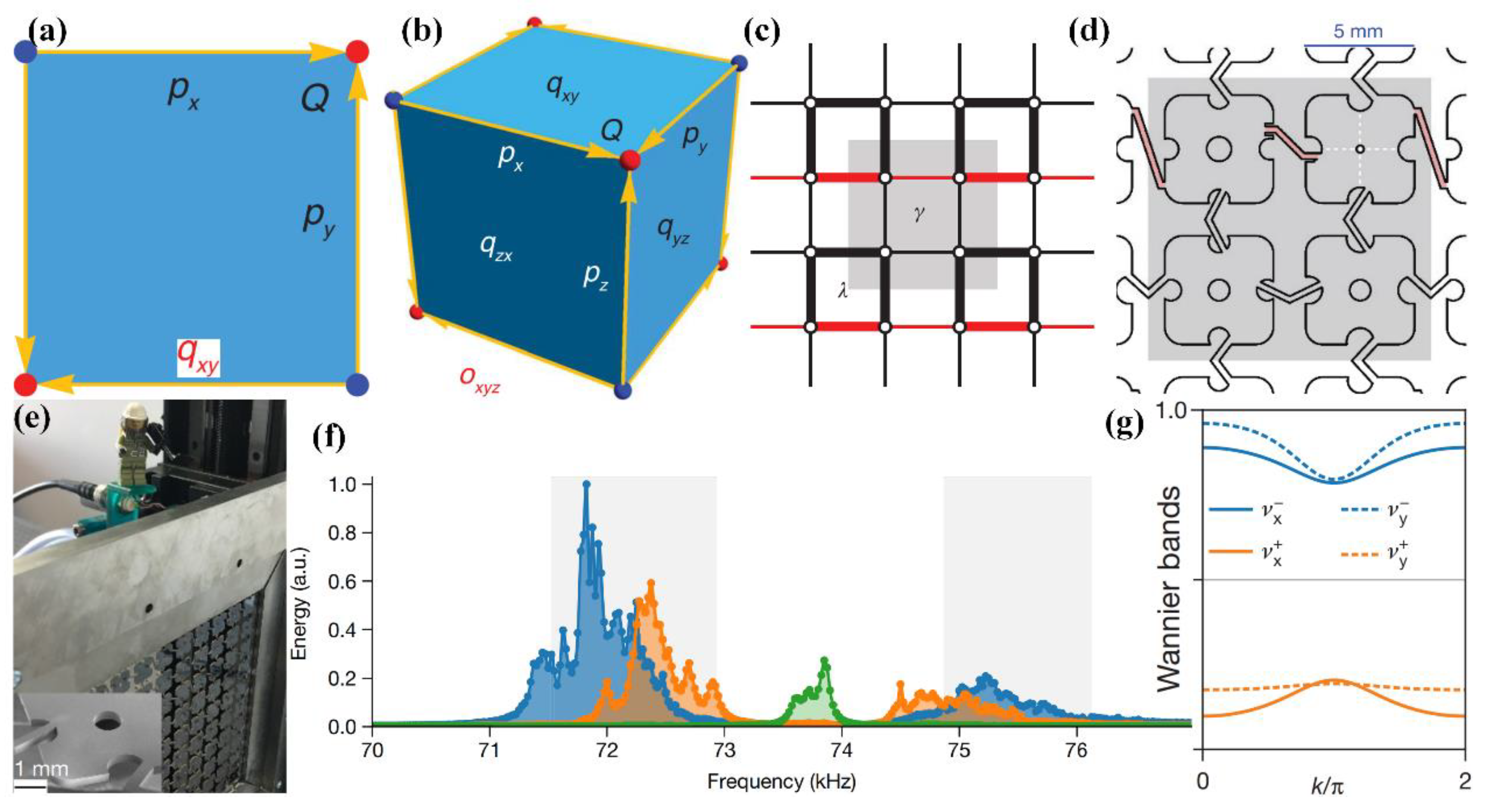
3.1. Quantized Multipole Insulators
3.2. -Symmetric HOTIs
4. Topological Pump
5. Topological Defect States
6. Conclusions and Future Directions
- (1)
- 3D higher-order topological insulators
- (2)
- Higher-order topological pump
- (3)
- Fractional charges
- (4)
- Non-Hermitian topological states
- (5)
- Application implementation
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Klitzing, K.V.; Dorda, G.; Pepper, M. New method for high-accuracy determination of the fine-structure constant based on quantized Hall resistance. Phys. Rev. Lett. 1980, 45, 494. [Google Scholar] [CrossRef] [Green Version]
- Thouless, D.J.; Kohmoto, M.; Nightingale, M.P.; den Nijs, M. Quantized Hall conductance in a two-dimensional periodic potential. Phys. Rev. Lett. 1982, 49, 405. [Google Scholar] [CrossRef] [Green Version]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2010, 82, 3045. [Google Scholar] [CrossRef] [Green Version]
- Kane, C.L.; Mele, E.J. Quantum spin Hall effect in graphene. Phys. Rev. Lett. 2005, 95, 226801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bernevig, B.A.; Zhang, S.-C. Quantum spin Hall effect. Phys. Rev. Lett. 2006, 96, 106802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bernevig, B.A.; Hughes, T.L.; Zhang, S.-C. Quantum spin Hall effect and topological phase transition in HgTe quantum wells. Science 2006, 314, 1757–1761. [Google Scholar] [CrossRef] [Green Version]
- Kane, C.L.; Mele, E.J. Z2 topological order and the quantum spin Hall effect. Phys. Rev. Lett. 2005, 95, 146802. [Google Scholar] [CrossRef] [Green Version]
- Sheng, L.; Sheng, D.; Ting, C.; Haldane, F. Nondissipative spin Hall effect via quantized edge transport. Phys. Rev. Lett. 2005, 95, 136602. [Google Scholar] [CrossRef] [Green Version]
- Rycerz, A.; Tworzydło, J.; Beenakker, C. Valley filter and valley valve in graphene. Nature Phys. 2007, 3, 172–175. [Google Scholar] [CrossRef] [Green Version]
- Xiao, D.; Yao, W.; Niu, Q. Valley-contrasting physics in graphene: Magnetic moment and topological transport. Phys. Rev. Lett. 2007, 99, 236809. [Google Scholar] [CrossRef] [Green Version]
- Benalcazar, W.A.; Bernevig, B.A.; Hughes, T.L. Quantized electric multipole insulators. Science 2017, 357, 61–66. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benalcazar, W.A.; Bernevig, B.A.; Hughes, T.L. Electric multipole moments, topological multipole moment pumping, and chiral hinge states in crystalline insulators. Phys. Rev. B 2017, 96, 245115. [Google Scholar] [CrossRef] [Green Version]
- Schindler, F.; Cook, A.M.; Vergniory, M.G.; Wang, Z.; Parkin, S.S.; Bernevig, B.A.; Neupert, T. Higher-order topological insulators. Sci. Adv. 2018, 4, eaat0346. [Google Scholar] [CrossRef] [Green Version]
- Kim, M.; Jacob, Z.; Rho, J. Recent advances in 2D, 3D and higher-order topological photonics. Light Sci. Appl. 2020, 9, 1–30. [Google Scholar] [CrossRef]
- Xie, B.; Wang, H.-X.; Zhang, X.; Zhan, P.; Jiang, J.-H.; Lu, M.; Chen, Y. Higher-order band topology. Nat. Rev. Phys. 2021, 1–13. [Google Scholar] [CrossRef]
- Kang, B.; Shiozaki, K.; Cho, G.Y. Many-body order parameters for multipoles in solids. Phys. Rev. B 2019, 100, 245134. [Google Scholar] [CrossRef] [Green Version]
- Wheeler, W.A.; Wagner, L.K.; Hughes, T.L. Many-body electric multipole operators in extended systems. Phys. Rev. B 2019, 100, 245135. [Google Scholar] [CrossRef] [Green Version]
- Liu, F.; Wakabayashi, K. Novel topological phase with a zero berry curvature. Phys. Rev. Lett. 2017, 118, 076803. [Google Scholar] [CrossRef] [Green Version]
- Benalcazar, W.A.; Li, T.; Hughes, T.L. Quantization of fractional corner charge in C n-symmetric higher-order topological crystalline insulators. Phys. Rev. B 2019, 99, 245151. [Google Scholar] [CrossRef] [Green Version]
- Thouless, D. Quantization of particle transport. Phys. Rev. B 1983, 27, 6083. [Google Scholar] [CrossRef]
- Lee, C.H.; Wang, Y.; Chen, Y.; Zhang, X. Electromagnetic response of quantum Hall systems in dimensions five and six and beyond. Phys. Rev. B 2018, 98, 094434. [Google Scholar] [CrossRef] [Green Version]
- Petrides, I.; Price, H.M.; Zilberberg, O. Six-dimensional quantum Hall effect and three-dimensional topological pumps. Phys. Rev. B 2018, 98, 125431. [Google Scholar] [CrossRef] [Green Version]
- Benalcazar, W.A.; Noh, J.; Wang, M.; Huang, S.; Chen, K.P.; Rechtsman, M.C. Higher-order topological pumping. arXiv 2020, arXiv:2006.13242. [Google Scholar]
- Lohse, M.; Schweizer, C.; Price, H.M.; Zilberberg, O.; Bloch, I. Exploring 4D quantum Hall physics with a 2D topological charge pump. Nature 2018, 553, 55–58. [Google Scholar] [CrossRef]
- Geier, M.; Fulga, I.C.; Lau, A. Bulk-boundary-defect correspondence at disclinations in rotation-symmetric topological insulators and superconductors. SciPost Phys. 2021, 10, 092. [Google Scholar] [CrossRef]
- Volterra, V. Sur l’équilibre des corps élastiques multiplement connexes. In Annales Scientifiques de l’École Normale Supérieure; Société Mathématique de France: France, 1907; pp. 401–517. [Google Scholar] [CrossRef] [Green Version]
- Kleman, M.; Friedel, J. Disclinations, dislocations, and continuous defects: A reappraisal. Rev. Mod. Phys. 2008, 80, 61. [Google Scholar] [CrossRef] [Green Version]
- Thorngren, R.; Else, D.V. Gauging spatial symmetries and the classification of topological crystalline phases. Phys. Rev. X 2018, 8, 011040. [Google Scholar] [CrossRef] [Green Version]
- Teo, J.C.; Kane, C.L. Topological defects and gapless modes in insulators and superconductors. Phys. Rev. B 2010, 82, 115120. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.; Zhang, Q.; Zhang, Y.-F.; Xia, B.-Z.; Liu, X.-N.; Zhou, X.-M.; Chen, C.-Q.; Hu, G.-K. Research progress of elastic topological materials. Adv. Mech. 2021, 51, 189–256. [Google Scholar]
- Miniaci, M.; Pal, R. Design of topological elastic waveguides. J. Appl. Phys. 2021, 130, 141101. [Google Scholar] [CrossRef]
- Zhang, X.; Xiao, M.; Cheng, Y.; Lu, M.-H.; Christensen, J. Topological sound. Commun. Phys. 2018, 1, 97. [Google Scholar] [CrossRef] [Green Version]
- Haldane, F.; Raghu, S. Possible realization of directional optical waveguides in photonic crystals with broken time-reversal symmetry. Phys. Rev. Lett. 2008, 100, 013904. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Chong, Y.; Joannopoulos, J.D.; Soljačić, M. Observation of unidirectional backscattering-immune topological electromagnetic states. Nature 2009, 461, 772–775. [Google Scholar] [CrossRef] [Green Version]
- Khanikaev, A.B.; Mousavi, S.H.; Tse, W.-K.; Kargarian, M.; MacDonald, A.H.; Shvets, G. Photonic topological insulators. Nat. Mater. 2013, 12, 233–239. [Google Scholar] [CrossRef] [PubMed]
- Ma, T.; Khanikaev, A.B.; Mousavi, S.H.; Shvets, G. Guiding electromagnetic waves around sharp corners: Topologically protected photonic transport in metawaveguides. Phys. Rev. Lett. 2015, 114, 127401. [Google Scholar] [CrossRef] [PubMed]
- He, C.; Sun, X.-C.; Liu, X.-P.; Lu, M.-H.; Chen, Y.; Feng, L.; Chen, Y.-F. Photonic topological insulator with broken time-reversal symmetry. Proc. Natl. Acad. Sci. USA 2016, 113, 4924–4928. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, X. Observing Zitterbewegung for photons near the Dirac point of a two-dimensional photonic crystal. Phys. Rev. Lett. 2008, 100, 113903. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sepkhanov, R.; Nilsson, J.; Beenakker, C. Proposed method for detection of the pseudospin-1/2 Berry phase in a photonic crystal with a Dirac spectrum. Phys. Rev. B 2008, 78, 045122. [Google Scholar] [CrossRef] [Green Version]
- Weick, G.; Woollacott, C.; Barnes, W.L.; Hess, O.; Mariani, E. Dirac-like plasmons in honeycomb lattices of metallic nanoparticles. Phys. Rev. Lett. 2013, 110, 106801. [Google Scholar] [CrossRef]
- Zhang, F. Brought to light. Nat. Phys. 2018, 14, 111–113. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Liu, Z. Extremal transmission and beating effect of acoustic waves in two-dimensional sonic crystals. Phys. Rev. Lett. 2008, 101, 264303. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fleury, R.; Sounas, D.L.; Sieck, C.F.; Haberman, M.R.; Alù, A. Sound isolation and giant linear nonreciprocity in a compact acoustic circulator. Science 2014, 343, 516–519. [Google Scholar] [CrossRef]
- Yang, Z.; Gao, F.; Shi, X.; Lin, X.; Gao, Z.; Chong, Y.; Zhang, B. Topological acoustics. Phys. Rev. Lett. 2015, 114, 114301. [Google Scholar] [CrossRef] [PubMed]
- Khanikaev, A.B.; Fleury, R.; Mousavi, S.H.; Alu, A. Topologically robust sound propagation in an angular-momentum-biased graphene-like resonator lattice. Nat. Commun. 2015, 6, 8260. [Google Scholar] [CrossRef]
- Ni, X.; He, C.; Sun, X.-C.; Liu, X.-p.; Lu, M.-H.; Feng, L.; Chen, Y.-F. Topologically protected one-way edge mode in networks of acoustic resonators with circulating air flow. New J. Phys. 2015, 17, 053016. [Google Scholar] [CrossRef]
- Peng, Y.-G.; Qin, C.-Z.; Zhao, D.-G.; Shen, Y.-X.; Xu, X.-Y.; Bao, M.; Jia, H.; Zhu, X.-F. Experimental demonstration of anomalous Floquet topological insulator for sound. Nat. Commun. 2016, 7, 13368. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, C.; Li, Z.; Ni, X.; Sun, X.-C.; Yu, S.-Y.; Lu, M.-H.; Liu, X.-P.; Chen, Y.-F. Topological phononic states of underwater sound based on coupled ring resonators. Appl. Phys. Lett. 2016, 108, 031904. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Ke, M.; Liu, Z. Valley vortex states in sonic crystals. Phys. Rev. Lett. 2016, 116, 093901. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Qiu, C.; Ye, L.; Fan, X.; Ke, M.; Zhang, F.; Liu, Z. Observation of topological valley transport of sound in sonic crystals. Nat. Phys. 2017, 13, 369–374. [Google Scholar] [CrossRef] [Green Version]
- Xia, B.-Z.; Liu, T.-T.; Huang, G.-L.; Dai, H.-Q.; Jiao, J.-R.; Zang, X.-G.; Yu, D.-J.; Zheng, S.-J.; Liu, J. Topological phononic insulator with robust pseudospin-dependent transport. Phys. Rev. B 2017, 96, 094106. [Google Scholar] [CrossRef] [Green Version]
- Ding, Y.; Peng, Y.; Zhu, Y.; Fan, X.; Yang, J.; Liang, B.; Zhu, X.; Wan, X.; Cheng, J. Experimental demonstration of acoustic Chern insulators. Phys. Rev. Lett. 2019, 122, 014302. [Google Scholar] [CrossRef] [PubMed]
- Zheng, S.; Duan, G.; Xia, B. Underwater acoustic positioning based on valley-chirality locked beam of sonic system. Int. J. Mech. Sci. 2020, 174, 105463. [Google Scholar] [CrossRef]
- Wang, Y.-T.; Luan, P.-G.; Zhang, S. Coriolis force induced topological order for classical mechanical vibrations. New J. Phys. 2015, 17, 073031. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, X.; Hu, G. Topological phase transition in mechanical honeycomb lattice. J. Mech. Phys. Solids 2019, 122, 54–68. [Google Scholar] [CrossRef]
- Wang, P.; Lu, L.; Bertoldi, K. Topological phononic crystals with one-way elastic edge waves. Phys. Rev. Lett. 2015, 115, 104302. [Google Scholar] [CrossRef] [PubMed]
- Nash, L.M.; Kleckner, D.; Read, A.; Vitelli, V.; Turner, A.M.; Irvine, W.T. Topological mechanics of gyroscopic metamaterials. Proc. Natl. Acad. Sci. USA 2015, 112, 14495–14500. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Süsstrunk, R.; Huber, S.D. Observation of phononic helical edge states in a mechanical topological insulator. Science 2015, 349, 47–50. [Google Scholar] [CrossRef] [Green Version]
- Salerno, G.; Berardo, A.; Ozawa, T.; Price, H.M.; Taxis, L.; Pugno, N.M.; Carusotto, I. Spin–orbit coupling in a hexagonal ring of pendula. New J. Phys. 2017, 19, 055001. [Google Scholar] [CrossRef]
- Mousavi, S.H.; Khanikaev, A.B.; Wang, Z. Topologically protected elastic waves in phononic metamaterials. Nat. Commun. 2015, 6, 8682. [Google Scholar] [CrossRef]
- Miniaci, M.; Pal, R.; Morvan, B.; Ruzzene, M. Experimental observation of topologically protected helical edge modes in patterned elastic plates. Phys. Rev. X 2018, 8, 031074. [Google Scholar] [CrossRef] [Green Version]
- Chaunsali, R.; Chen, C.-W.; Yang, J. Subwavelength and directional control of flexural waves in zone-folding induced topological plates. Phys. Rev. B 2018, 97, 054307. [Google Scholar] [CrossRef] [Green Version]
- Chaunsali, R.; Chen, C.-W.; Yang, J. Experimental demonstration of topological waveguiding in elastic plates with local resonators. New J. Phys. 2018, 20, 113036. [Google Scholar] [CrossRef]
- Cha, J.; Kim, K.W.; Daraio, C. Experimental realization of on-chip topological nanoelectromechanical metamaterials. Nature 2018, 564, 229–233. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Nassar, H.; Norris, A.N.; Hu, G.; Huang, G. Elastic quantum spin Hall effect in kagome lattices. Phys. Rev. B 2018, 98, 094302. [Google Scholar] [CrossRef] [Green Version]
- Yu, S.-Y.; He, C.; Wang, Z.; Liu, F.-K.; Sun, X.-C.; Li, Z.; Lu, H.-Z.; Lu, M.-H.; Liu, X.-P.; Chen, Y.-F. Elastic pseudospin transport for integratable topological phononic circuits. Nat. Commun. 2018, 9, 3072. [Google Scholar] [CrossRef]
- Zheng, L.-Y.; Theocharis, G.; Tournat, V.; Gusev, V. Quasitopological rotational waves in mechanical granular graphene. Phys. Rev. B 2018, 97, 060101. [Google Scholar] [CrossRef]
- Li, G.-H.; Ma, T.-X.; Wang, Y.-Z.; Wang, Y.-S. Active control on topological immunity of elastic wave metamaterials. Sci. Rep. 2020, 10, 9376. [Google Scholar] [CrossRef]
- Pal, R.K.; Schaeffer, M.; Ruzzene, M. Helical edge states and topological phase transitions in phononic systems using bi-layered lattices. J. Appl. Phys. 2016, 119, 084305. [Google Scholar] [CrossRef] [Green Version]
- Pal, R.K.; Ruzzene, M. Edge waves in plates with resonators: An elastic analogue of the quantum valley Hall effect. New J. Phys. 2017, 19, 025001. [Google Scholar] [CrossRef]
- Wang, J.; Mei, J. Topological valley-chiral edge states of Lamb waves in elastic thin plates. Appl. Phys. Express 2018, 11, 057302. [Google Scholar] [CrossRef]
- Gao, N.; Qu, S.; Si, L.; Wang, J.; Chen, W. Broadband topological valley transport of elastic wave in reconfigurable phononic crystal plate. Appl. Phys. Lett. 2021, 118, 063502. [Google Scholar] [CrossRef]
- Liu, T.-W.; Semperlotti, F. Tunable acoustic valley–hall edge states in reconfigurable phononic elastic waveguides. Phys. Rev. Appl. 2018, 9, 014001. [Google Scholar] [CrossRef] [Green Version]
- Jiao, J.; Chen, T.; Dai, H.; Yu, D. Observation of topological valley transport of elastic waves in bilayer phononic crystal slabs. Phys. Lett. A 2019, 383, 125988. [Google Scholar] [CrossRef]
- Yao, L.; Zhang, D.; Xu, K.; Dong, L.; Chen, X. Topological phononic crystal plates with locally resonant elastic wave systems. Appl. Acoust. 2021, 177, 107931. [Google Scholar] [CrossRef]
- Vila, J.; Pal, R.K.; Ruzzene, M. Observation of topological valley modes in an elastic hexagonal lattice. Phys. Rev. B 2017, 96, 134307. [Google Scholar] [CrossRef] [Green Version]
- Yan, M.; Lu, J.; Li, F.; Deng, W.; Huang, X.; Ma, J.; Liu, Z. On-chip valley topological materials for elastic wave manipulation. Nat. Mater. 2018, 17, 993–998. [Google Scholar] [CrossRef]
- Zhu, H.; Liu, T.-W.; Semperlotti, F. Design and experimental observation of valley-Hall edge states in diatomic-graphene-like elastic waveguides. Phys. Rev. B 2018, 97, 174301. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Chen, Y.; Zhang, K.; Hu, G. Programmable elastic valley Hall insulator with tunable interface propagation routes. Extrem. Mech. Lett. 2019, 28, 76–80. [Google Scholar] [CrossRef]
- Kariyado, T.; Hatsugai, Y. Manipulation of dirac cones in mechanical graphene. Sci. Rep. 2015, 5, 18107. [Google Scholar] [CrossRef] [Green Version]
- Wu, L.-H.; Hu, X. Scheme for achieving a topological photonic crystal by using dielectric material. Phys. Rev. Lett. 2015, 114, 223901. [Google Scholar] [CrossRef]
- He, C.; Ni, X.; Ge, H.; Sun, X.-C.; Chen, Y.-B.; Lu, M.-H.; Liu, X.-P.; Chen, Y.-F. Acoustic topological insulator and robust one-way sound transport. Nat. Phys. 2016, 12, 1124–1129. [Google Scholar] [CrossRef]
- Lubensky, T.; Kane, C.; Mao, X.; Souslov, A.; Sun, K. Phonons and elasticity in critically coordinated lattices. Rep. Prog. Phys. 2015, 78, 073901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dong, J.-W.; Chen, X.-D.; Zhu, H.; Wang, Y.; Zhang, X. Valley photonic crystals for control of spin and topology. Nat. Mater. 2017, 16, 298–302. [Google Scholar] [CrossRef] [PubMed]
- Gao, F.; Xue, H.; Yang, Z.; Lai, K.; Yu, Y.; Lin, X.; Chong, Y.; Shvets, G.; Zhang, B. Topologically protected refraction of robust kink states in valley photonic crystals. Nat. Phys. 2018, 14, 140–144. [Google Scholar] [CrossRef]
- Huang, H.; Huo, S.; Chen, J. Subwavelength elastic topological negative refraction in ternary locally resonant phononic crystals. Int. J. Mech. Sci. 2021, 198, 106391. [Google Scholar] [CrossRef]
- Zhang, J.; Xia, B.-Z. Topological interface propagation characteristics of valleypolarized three-dimensional elastic phononic crystals induced by lattice defects. Chin. Sci. Bull. 2021, 1–10. [Google Scholar]
- Huo, S.-y.; Chen, J.-j.; Huang, H.-b.; Huang, G.-l. Simultaneous multi-band valley-protected topological edge states of shear vertical wave in two-dimensional phononic crystals with veins. Sci. Rep. 2017, 7, 10335. [Google Scholar] [CrossRef] [Green Version]
- Tong, L.; Fan, H.; Xia, B. Elastic phononic plates with first-order and second-order topological phases. J. Phys. D Appl. Phys. 2020, 53, 115303. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, Y.; Zhang, K.; Hu, G. Dirac degeneracy and elastic topological valley modes induced by local resonant states. Phys. Rev. B 2020, 101, 014101. [Google Scholar] [CrossRef]
- Noh, J.; Benalcazar, W.A.; Huang, S.; Collins, M.J.; Chen, K.P.; Hughes, T.L.; Rechtsman, M.C. Topological protection of photonic mid-gap defect modes. Nat. Photonics 2018, 12, 408–415. [Google Scholar] [CrossRef] [Green Version]
- Mittal, S.; Orre, V.V.; Zhu, G.; Gorlach, M.A.; Poddubny, A.; Hafezi, M. Photonic quadrupole topological phases. Nat. Photonics 2019, 13, 692–696. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.-D.; Deng, W.-M.; Shi, F.-L.; Zhao, F.-L.; Chen, M.; Dong, J.-W. Direct observation of corner states in second-order topological photonic crystal slabs. Phys. Rev. Lett. 2019, 122, 233902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, M.; Rho, J. Topological edge and corner states in a two-dimensional photonic Su-Schrieffer-Heeger lattice. Nanophotonics 2020, 9, 3227–3234. [Google Scholar] [CrossRef]
- Xie, B.; Su, G.; Wang, H.-F.; Liu, F.; Hu, L.; Yu, S.-Y.; Zhan, P.; Lu, M.-H.; Wang, Z.; Chen, Y.-F. Higher-order quantum spin Hall effect in a photonic crystal. Nat. Commun. 2020, 11, 3768. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Lin, Z.K.; Lu, W.; Lai, Y.; Hou, B.; Jiang, J.H. Twisted quadrupole topological photonic crystals. Laser Photonics Rev. 2020, 14, 2000010. [Google Scholar] [CrossRef]
- El Hassan, A.; Kunst, F.K.; Moritz, A.; Andler, G.; Bergholtz, E.J.; Bourennane, M. Corner states of light in photonic waveguides. Nat. Photonics 2019, 13, 697–700. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Xie, B.-Y.; Lu, Y.-H.; Chang, Y.-J.; Wang, H.-F.; Gao, J.; Jiao, Z.-Q.; Feng, Z.; Xu, X.-Y.; Mei, F. Quantum superposition demonstrated higher-order topological bound states in the continuum. Light Sci. Appl. 2021, 10, 173. [Google Scholar] [CrossRef]
- Xue, H.; Yang, Y.; Gao, F.; Chong, Y.; Zhang, B. Acoustic higher-order topological insulator on a kagome lattice. Nat. Mater. 2019, 18, 108–112. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Xie, B.-Y.; Wang, H.-F.; Xu, X.; Tian, Y.; Jiang, J.-H.; Lu, M.-H.; Chen, Y.-F. Dimensional hierarchy of higher-order topology in three-dimensional sonic crystals. Nat. Commun. 2019, 10, 5331. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Wang, H.-X.; Lin, Z.-K.; Tian, Y.; Xie, B.; Lu, M.-H.; Chen, Y.-F.; Jiang, J.-H. Second-order topology and multidimensional topological transitions in sonic crystals. Nat. Phys. 2019, 15, 582–588. [Google Scholar] [CrossRef] [Green Version]
- Xue, H.; Yang, Y.; Liu, G.; Gao, F.; Chong, Y.; Zhang, B. Realization of an acoustic third-order topological insulator. Phys. Rev. Lett. 2019, 122, 244301. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; López, M.R.; Cheng, Y.; Liu, X.; Christensen, J. Non-hermitian sonic second-order topological insulator. Phys. Rev. Lett. 2019, 122, 195501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, X.; Liu, L.; Lu, M.-H.; Chen, Y.-F. Valley-selective topological corner states in sonic crystals. Phys. Rev. Lett. 2021, 126, 156401. [Google Scholar] [CrossRef]
- Serra-Garcia, M.; Peri, V.; Süsstrunk, R.; Bilal, O.R.; Larsen, T.; Villanueva, L.G.; Huber, S.D. Observation of a phononic quadrupole topological insulator. Nature 2018, 555, 342–345. [Google Scholar] [CrossRef] [PubMed]
- Fan, H.; Xia, B.; Tong, L.; Zheng, S.; Yu, D. Elastic higher-order topological insulator with topologically protected corner states. Phys. Rev. Lett. 2019, 122, 204301. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.-W.; Chaunsali, R.; Christensen, J.; Theocharis, G.; Yang, J. Corner states in a second-order mechanical topological insulator. Commun. Mater. 2021, 2, 62. [Google Scholar] [CrossRef]
- Gao, P.; Torrent, D.; Cervera, F.; San-Jose, P.; Sánchez-Dehesa, J.; Christensen, J. Majorana-like zero modes in Kekulé distorted sonic lattices. Phys. Rev. Lett. 2019, 123, 196601. [Google Scholar] [CrossRef] [PubMed]
- Fan, H.; Xia, B.; Zheng, S.; Tong, L. Elastic phononic topological plate with edge and corner sates based on pseudospin-valley-coupling. J. Phys. D Appl. Phys. 2020, 53, 395304. [Google Scholar] [CrossRef]
- Wang, Z.; Wei, Q.; Xu, H.-Y.; Wu, D.-J. A higher-order topological insulator with wide bandgaps in Lamb-wave systems. J. Appl. Phys. 2020, 127, 075105. [Google Scholar] [CrossRef]
- Wu, Y.; Yan, M.; Lin, Z.-K.; Wang, H.-X.; Li, F.; Jiang, J.-H. On-chip higher-order topological micromechanical metamaterials. Sci. Bull. 2021, 66, 1959–1966. [Google Scholar] [CrossRef]
- Wang, Z.; Wei, Q. An elastic higher-order topological insulator based on kagome phononic crystals. J. Appl. Phys. 2021, 129, 035102. [Google Scholar] [CrossRef]
- Imhof, S.; Berger, C.; Bayer, F.; Brehm, J.; Molenkamp, L.W.; Kiessling, T.; Schindler, F.; Lee, C.H.; Greiter, M.; Neupert, T. Topolectrical-circuit realization of topological corner modes. Nat. Phys. 2018, 14, 925–929. [Google Scholar] [CrossRef]
- Wang, Q.; Liu, C.-C.; Lu, Y.-M.; Zhang, F. High-temperature majorana corner states. Phys. Rev. Lett. 2018, 121, 186801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lv, B.; Chen, R.; Li, R.; Guan, C.; Zhou, B.; Dong, G.; Zhao, C.; Li, Y.; Wang, Y.; Tao, H. Realization of quasicrystalline quadrupole topological insulators in electrical circuits. Commun. Phys. 2021, 4, 108. [Google Scholar] [CrossRef]
- Ezawa, M. Higher-order topological insulators and semimetals on the breathing kagome and pyrochlore lattices. Phys. Rev. Lett. 2018, 120, 026801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, Y.; Li, J.; Zhu, J. Topology optimization of quantum spin Hall effect-based second-order phononic topological insulator. Mech. Syst. Signal Process. 2022, 164, 108243. [Google Scholar] [CrossRef]
- Geerligs, L.; Anderegg, V.; Holweg, P.; Mooij, J.; Pothier, H.; Esteve, D.; Urbina, C.; Devoret, M. Frequency-locked turnstile device for single electrons. Phys. Rev. Lett. 1990, 64, 2691. [Google Scholar] [CrossRef] [Green Version]
- Kraus, Y.E.; Lahini, Y.; Ringel, Z.; Verbin, M.; Zilberberg, O. Topological states and adiabatic pumping in quasicrystals. Phys. Rev. Lett. 2012, 109, 106402. [Google Scholar] [CrossRef] [Green Version]
- Verbin, M.; Zilberberg, O.; Lahini, Y.; Kraus, Y.E.; Silberberg, Y. Topological pumping over a photonic Fibonacci quasicrystal. Phys. Rev. B 2015, 91, 064201. [Google Scholar] [CrossRef] [Green Version]
- Kraus, Y.E.; Ringel, Z.; Zilberberg, O. Four-dimensional quantum Hall effect in a two-dimensional quasicrystal. Phys. Rev. Lett. 2013, 111, 226401. [Google Scholar] [CrossRef] [PubMed]
- Kraus, Y.E.; Zilberberg, O. Quasiperiodicity and topology transcend dimensions. Nat. Phys. 2016, 12, 624–626. [Google Scholar] [CrossRef]
- Lohse, M.; Schweizer, C.; Zilberberg, O.; Aidelsburger, M.; Bloch, I. A Thouless quantum pump with ultracold bosonic atoms in an optical superlattice. Nat. Phys. 2016, 12, 350–354. [Google Scholar] [CrossRef] [Green Version]
- Chen, H.; Yao, L.; Nassar, H.; Huang, G. Mechanical quantum hall effect in time-modulated elastic materials. Phys. Rev. Appl. 2019, 11, 044029. [Google Scholar] [CrossRef] [Green Version]
- Nakajima, S.; Tomita, T.; Taie, S.; Ichinose, T.; Ozawa, H.; Wang, L.; Troyer, M.; Takahashi, Y. Topological Thouless pumping of ultracold fermions. Nat. Phys. 2016, 12, 296–300. [Google Scholar] [CrossRef] [Green Version]
- Riva, E.; Casieri, V.; Resta, F.; Braghin, F. Adiabatic pumping via avoided crossings in stiffness-modulated quasiperiodic beams. Phys. Rev. B 2020, 102, 014305. [Google Scholar] [CrossRef]
- Grinberg, I.H.; Lin, M.; Harris, C.; Benalcazar, W.A.; Peterson, C.W.; Hughes, T.L.; Bahl, G. Robust temporal pumping in a magneto-mechanical topological insulator. Nat. Commun. 2020, 11, 974. [Google Scholar] [CrossRef] [Green Version]
- Rosa, M.I.; Pal, R.K.; Arruda, J.R.; Ruzzene, M. Edge states and topological pumping in spatially modulated elastic lattices. Phys. Rev. Lett. 2019, 123, 034301. [Google Scholar] [CrossRef] [Green Version]
- Zilberberg, O.; Huang, S.; Guglielmon, J.; Wang, M.; Chen, K.P.; Kraus, Y.E.; Rechtsman, M.C. Photonic topological boundary pumping as a probe of 4D quantum Hall physics. Nature 2018, 553, 59–62. [Google Scholar] [CrossRef]
- Riva, E.; Rosa, M.I.; Ruzzene, M. Edge states and topological pumping in stiffness-modulated elastic plates. Phys. Rev. B 2020, 101, 094307. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.-G.; Tang, W.; Zhang, R.-Y.; Chen, Z.; Ma, G. Landau-Zener Transition in the Dynamic Transfer of Acoustic Topological States. Phys. Rev. Lett. 2021, 126, 054301. [Google Scholar] [CrossRef]
- Zeng, L.-S.; Shen, Y.-X.; Peng, Y.-G.; Zhao, D.-G.; Zhu, X.-F. Selective Topological Pumping for Robust, Efficient, and Asymmetric Sound Energy Transfer in a Dynamically Coupled Cavity Chain. Phys. Rev. Appl. 2021, 15, 064018. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, H.; Wu, Q.; Huang, Y.; Nguyen, H.; Prodan, E.; Zhou, X.; Huang, G. Physical rendering of synthetic spaces for topological sound transport. arXiv 2020, arXiv:2012.11828. [Google Scholar]
- Lu, L.; Joannopoulos, J.D.; Soljačić, M. Topological photonics. Nat. Photonics 2014, 8, 821–829. [Google Scholar] [CrossRef] [Green Version]
- Xia, Y.; Riva, E.; Rosa, M.I.; Cazzulani, G.; Erturk, A.; Braghin, F.; Ruzzene, M. Experimental observation of temporal pumping in electromechanical waveguides. Phys. Rev. Lett. 2021, 126, 095501. [Google Scholar] [CrossRef] [PubMed]
- Nassar, H.; Chen, H.; Norris, A.; Huang, G. Quantization of band tilting in modulated phononic crystals. Phys. Rev. B 2018, 97, 014305. [Google Scholar] [CrossRef] [Green Version]
- Riva, E.; Castaldini, G.; Braghin, F. Adiabatic edge-to-edge transformations in time-modulated elastic lattices and non-Hermitian shortcuts. arXiv 2021, arXiv:2105.02196. [Google Scholar] [CrossRef]
- Ran, Y.; Zhang, Y.; Vishwanath, A. One-dimensional topologically protected modes in topological insulators with lattice dislocations. Nat. Phys. 2009, 5, 298–303. [Google Scholar] [CrossRef] [Green Version]
- Teo, J.C.; Hughes, T.L. Existence of Majorana-fermion bound states on disclinations and the classification of topological crystalline superconductors in two dimensions. Phys. Rev. Lett. 2013, 111, 047006. [Google Scholar] [CrossRef] [PubMed]
- Tuegel, T.I.; Chua, V.; Hughes, T.L. Embedded topological insulators. Phys. Rev. B 2019, 100, 115126. [Google Scholar] [CrossRef] [Green Version]
- Wang, Q.; Xue, H.; Zhang, B.; Chong, Y. Observation of protected photonic edge states induced by real-space topological lattice defects. Phys. Rev. Lett. 2020, 124, 243602. [Google Scholar] [CrossRef]
- Li, F.-F.; Wang, H.-X.; Xiong, Z.; Lou, Q.; Chen, P.; Wu, R.-X.; Poo, Y.; Jiang, J.-H.; John, S. Topological light-trapping on a dislocation. Nat. Commun. 2018, 9, 2462. [Google Scholar] [CrossRef] [PubMed]
- Grinberg, I.H.; Lin, M.; Benalcazar, W.A.; Hughes, T.L.; Bahl, G. Trapped state at a dislocation in a weak magnetomechanical topological insulator. Phys. Rev. Appl. 2020, 14, 064042. [Google Scholar] [CrossRef]
- Peterson, C.W.; Li, T.; Jiang, W.; Hughes, T.L.; Bahl, G. Trapped fractional charges at bulk defects in topological insulators. Nature 2021, 589, 376–380. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Leung, S.; Li, F.-F.; Lin, Z.-K.; Tao, X.; Poo, Y.; Jiang, J.-H. Bulk–disclination correspondence in topological crystalline insulators. Nature 2021, 589, 381–385. [Google Scholar] [CrossRef]
- Xue, H.; Jia, D.; Ge, Y.; Guan, Y.-J.; Wang, Q.; Yuan, S.-Q.; Sun, H.-X.; Chong, Y.; Zhang, B. Observation of dislocation-induced topological modes in a three-dimensional acoustic topological insulator. arXiv 2021, arXiv:2104.13161. [Google Scholar] [CrossRef] [PubMed]
- Ye, L.; Qiu, C.; Xiao, M.; Li, T.; Du, J.; Ke, M.; Liu, Z. Topological dislocation modes in three-dimensional acoustic topological insulators. arXiv 2021, arXiv:2104.04172. [Google Scholar] [CrossRef]
- Wang, Q.; Ge, Y.; Sun, H.-x.; Xue, H.; Jia, D.; Guan, Y.-j.; Yuan, S.-q.; Zhang, B.; Chong, Y. Vortex states in an acoustic Weyl crystal with a topological lattice defect. Nat. Commun. 2021, 12, 3654. [Google Scholar] [CrossRef]
- Teo, J.C.; Hughes, T.L. Topological defects in symmetry-protected topological phases. Annu. Rev. Condens. Matter Phys. 2017, 8, 211–237. [Google Scholar] [CrossRef]
- Barkeshli, M.; Qi, X.-L. Topological nematic states and non-abelian lattice dislocations. Phys. Rev. X 2012, 2, 031013. [Google Scholar] [CrossRef] [Green Version]
- Juričić, V.; Mesaros, A.; Slager, R.-J.; Zaanen, J. Universal probes of two-dimensional topological insulators: Dislocation and π flux. Phys. Rev. Lett. 2012, 108, 106403. [Google Scholar] [CrossRef] [Green Version]
- Mesaros, A.; Kim, Y.B.; Ran, Y. Changing topology by topological defects in three-dimensional topologically ordered phases. Phys. Rev. B 2013, 88, 035141. [Google Scholar] [CrossRef] [Green Version]
- Benalcazar, W.A.; Teo, J.C.; Hughes, T.L. Classification of two-dimensional topological crystalline superconductors and majorana bound states at disclinations. Phys. Rev. B 2014, 89, 224503. [Google Scholar] [CrossRef] [Green Version]
- Queiroz, R.; Fulga, I.C.; Avraham, N.; Beidenkopf, H.; Cano, J. Partial lattice defects in higher-order topological insulators. Phys. Rev. Lett. 2019, 123, 266802. [Google Scholar] [CrossRef] [Green Version]
- Roy, B.; Juričić, V. Dislocation as a bulk probe of higher-order topological insulators. Phys. Rev. Res. 2021, 3, 033107. [Google Scholar] [CrossRef]
- Yamada, S.S.; Li, T.; Lin, M.; Peterson, C.W.; Hughes, T.L.; Bahl, G. Bound states at partial dislocation defects in multipole higher-order topological insulators. arXiv 2021, arXiv:2105.01050. [Google Scholar]
- Van Miert, G.; Ortix, C. Dislocation charges reveal two-dimensional topological crystalline invariants. Phys. Rev. B 2018, 97, 201111. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; Zhu, P.; Benalcazar, W.A.; Hughes, T.L. Fractional disclination charge in two-dimensional C n-symmetric topological crystalline insulators. Phys. Rev. B 2020, 101, 115115. [Google Scholar] [CrossRef] [Green Version]
- Paulose, J.; Chen, B.G.-g.; Vitelli, V. Topological modes bound to dislocations in mechanical metamaterials. Nat. Phys. 2015, 11, 153–156. [Google Scholar] [CrossRef] [Green Version]
- Xia, B.; Zhang, J.; Tong, L.; Zheng, S.; Man, X. Topologically valley-polarized edge states in elastic phononic plates yielded by lattice defects. Int. J. Solids Struct. 2022, 239–240, 111413. [Google Scholar] [CrossRef]
- Xia, B.; Jiang, Z.; Tong, L.; Zheng, S.; Man, X. Topological bound states in elastic phononic plates induced by disclinations. Acta Mech. Sin. 2021, 1. [Google Scholar]
- Ni, X.; Li, M.; Weiner, M.; Alù, A.; Khanikaev, A.B. Demonstration of a quantized acoustic octupole topological insulator. Nat. Commun. 2020, 11, 2108. [Google Scholar] [CrossRef]
- Weiner, M.; Ni, X.; Li, M.; Alù, A.; Khanikaev, A.B. Demonstration of a third-order hierarchy of topological states in a three-dimensional acoustic metamaterial. Sci. Adv. 2020, 6, eaay4166. [Google Scholar] [CrossRef] [Green Version]
- Xue, H.; Ge, Y.; Sun, H.-X.; Wang, Q.; Jia, D.; Guan, Y.-J.; Yuan, S.-Q.; Chong, Y.; Zhang, B. Observation of an acoustic octupole topological insulator. Nat. Commun. 2020, 11, 2442. [Google Scholar] [CrossRef]
- Zheng, S.; Xia, B.; Man, X.; Tong, L.; Jiao, J.; Duan, G.; Yu, D. Three-dimensional higher-order topological acoustic system with multidimensional topological states. Phys. Rev. B 2020, 102, 104113. [Google Scholar] [CrossRef]
- Bao, J.; Zou, D.; Zhang, W.; He, W.; Sun, H.; Zhang, X. Topoelectrical circuit octupole insulator with topologically protected corner states. Phys. Rev. B 2019, 100, 201406. [Google Scholar] [CrossRef] [Green Version]
- Liu, S.; Ma, S.; Zhang, Q.; Zhang, L.; Yang, C.; You, O.; Gao, W.; Xiang, Y.; Cui, T.J.; Zhang, S. Octupole corner state in a three-dimensional topological circuit. Light Sci. Appl. 2020, 9, 145. [Google Scholar] [CrossRef]
- Peterson, C.W.; Li, T.; Benalcazar, W.A.; Hughes, T.L.; Bahl, G. A fractional corner anomaly reveals higher-order topology. Science 2020, 368, 1114–1118. [Google Scholar] [CrossRef]
- Zhu, X.; Wang, H.; Gupta, S.K.; Zhang, H.; Xie, B.; Lu, M.; Chen, Y. Photonic non-Hermitian skin effect and non-Bloch bulk-boundary correspondence. Phys. Rev. Res. 2020, 2, 013280. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Zhou, Q.; Chen, S. Localization transition, spectrum structure, and winding numbers for one-dimensional non-Hermitian quasicrystals. Phys. Rev. B 2021, 104, 024201. [Google Scholar] [CrossRef]
- Zhou, H.; Peng, C.; Yoon, Y.; Hsu, C.W.; Nelson, K.A.; Fu, L.; Joannopoulos, J.D.; Soljačić, M.; Zhen, B. Observation of bulk Fermi arc and polarization half charge from paired exceptional points. Science 2018, 359, 1009–1012. [Google Scholar] [CrossRef] [Green Version]
- Cerjan, A.; Huang, S.; Wang, M.; Chen, K.P.; Chong, Y.; Rechtsman, M.C. Experimental realization of a Weyl exceptional ring. Nat. Photonics 2019, 13, 623–628. [Google Scholar] [CrossRef] [Green Version]
- Gao, H.; Xue, H.; Gu, Z.; Liu, T.; Zhu, J.; Zhang, B. Non-Hermitian route to higher-order topology in an acoustic crystal. Nat. Commun. 2021, 12, 1888. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Tian, Y.; Jiang, J.-H.; Lu, M.-H.; Chen, Y.-F. Observation of higher-order non-Hermitian skin effect. arXiv 2021, arXiv:2102.09825. [Google Scholar] [CrossRef] [PubMed]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, S.; Duan, G.; Xia, B. Progress in Topological Mechanics. Appl. Sci. 2022, 12, 1987. https://doi.org/10.3390/app12041987
Zheng S, Duan G, Xia B. Progress in Topological Mechanics. Applied Sciences. 2022; 12(4):1987. https://doi.org/10.3390/app12041987
Chicago/Turabian StyleZheng, Shengjie, Guiju Duan, and Baizhan Xia. 2022. "Progress in Topological Mechanics" Applied Sciences 12, no. 4: 1987. https://doi.org/10.3390/app12041987
APA StyleZheng, S., Duan, G., & Xia, B. (2022). Progress in Topological Mechanics. Applied Sciences, 12(4), 1987. https://doi.org/10.3390/app12041987





