Investigation on Spray Morphology, Droplet Dynamics, and Thermal Characteristics of Iso-Pentane Flashing Spray Based on OpenFOAM
Abstract
:1. Introduction
2. Physical Model of Iso-Pentane Flashing Spray
2.1. Gas Phase Equations
2.1.1. The Continuity Equation
2.1.2. The Momentum Equation
2.1.3. The Species Equation
2.1.4. The Energy Equation
2.2. Liquid Phase Equations
2.2.1. Injection Model
2.2.2. Droplet Motion Model
2.2.3. Droplet Breakup Model
2.2.4. Droplet Evaporation/Boiling Model
2.2.5. Droplet Heat Transfer Model
2.2.6. Droplet Dispersion Model
3. Simulation Setup
3.1. Parameter Setup
3.2. Grid Independence Test and Model Validation
4. Results
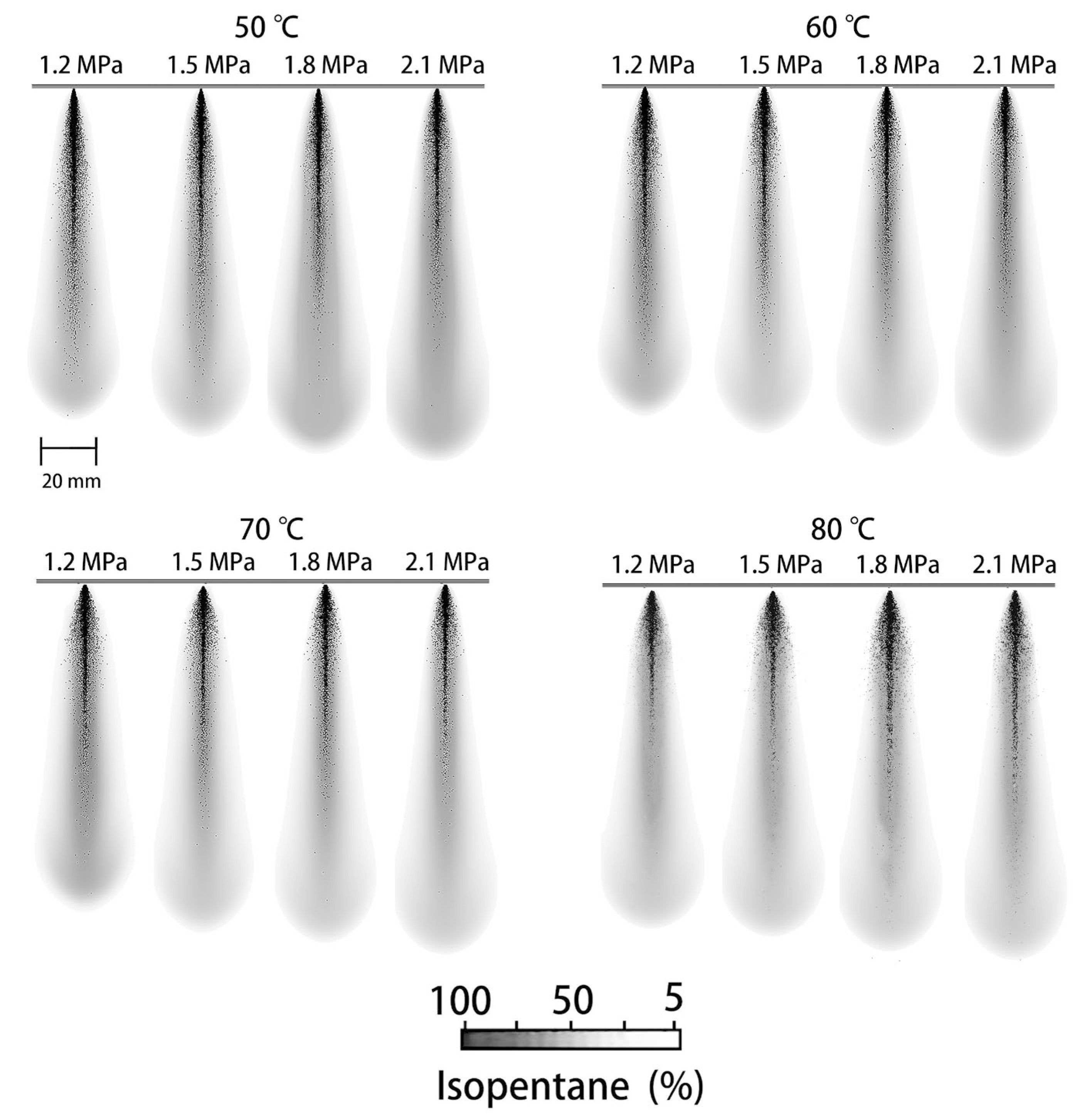
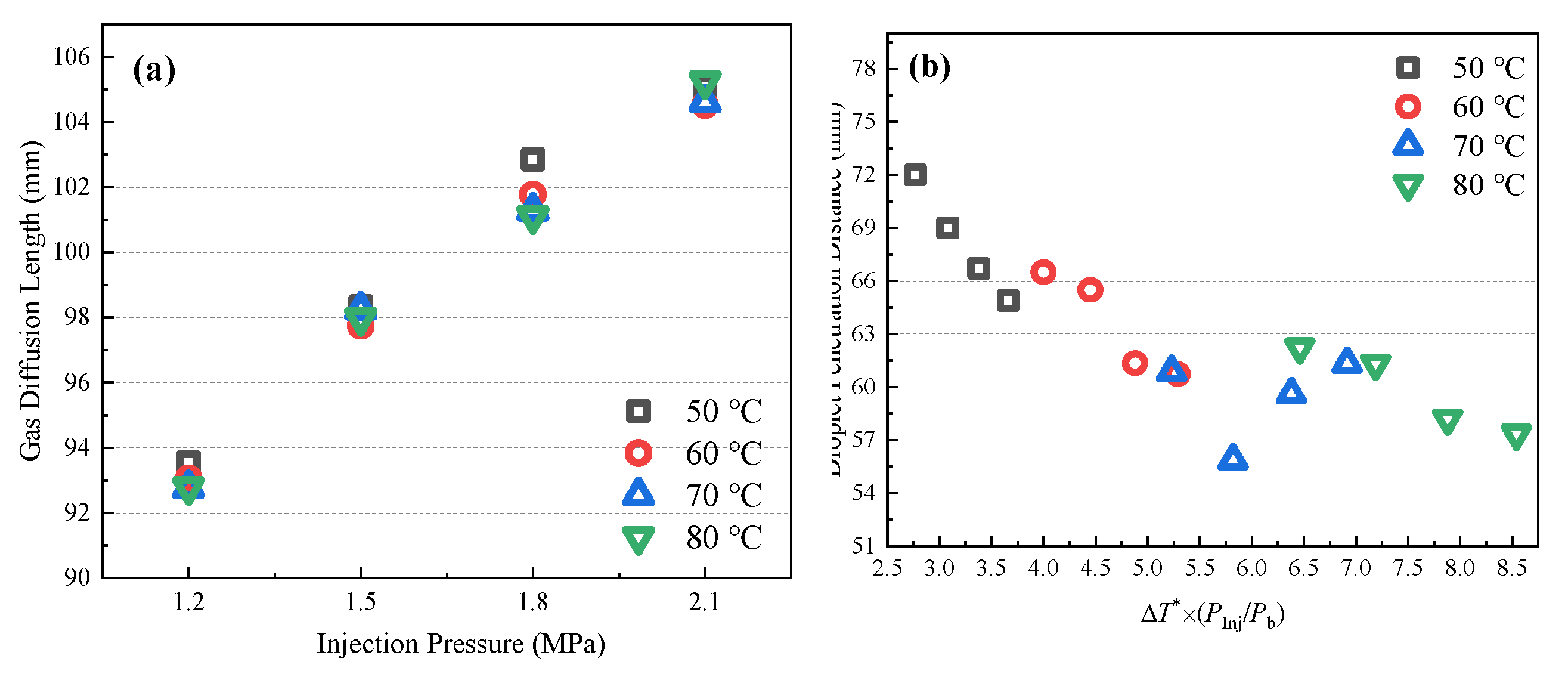
4.1. Spray Morphology
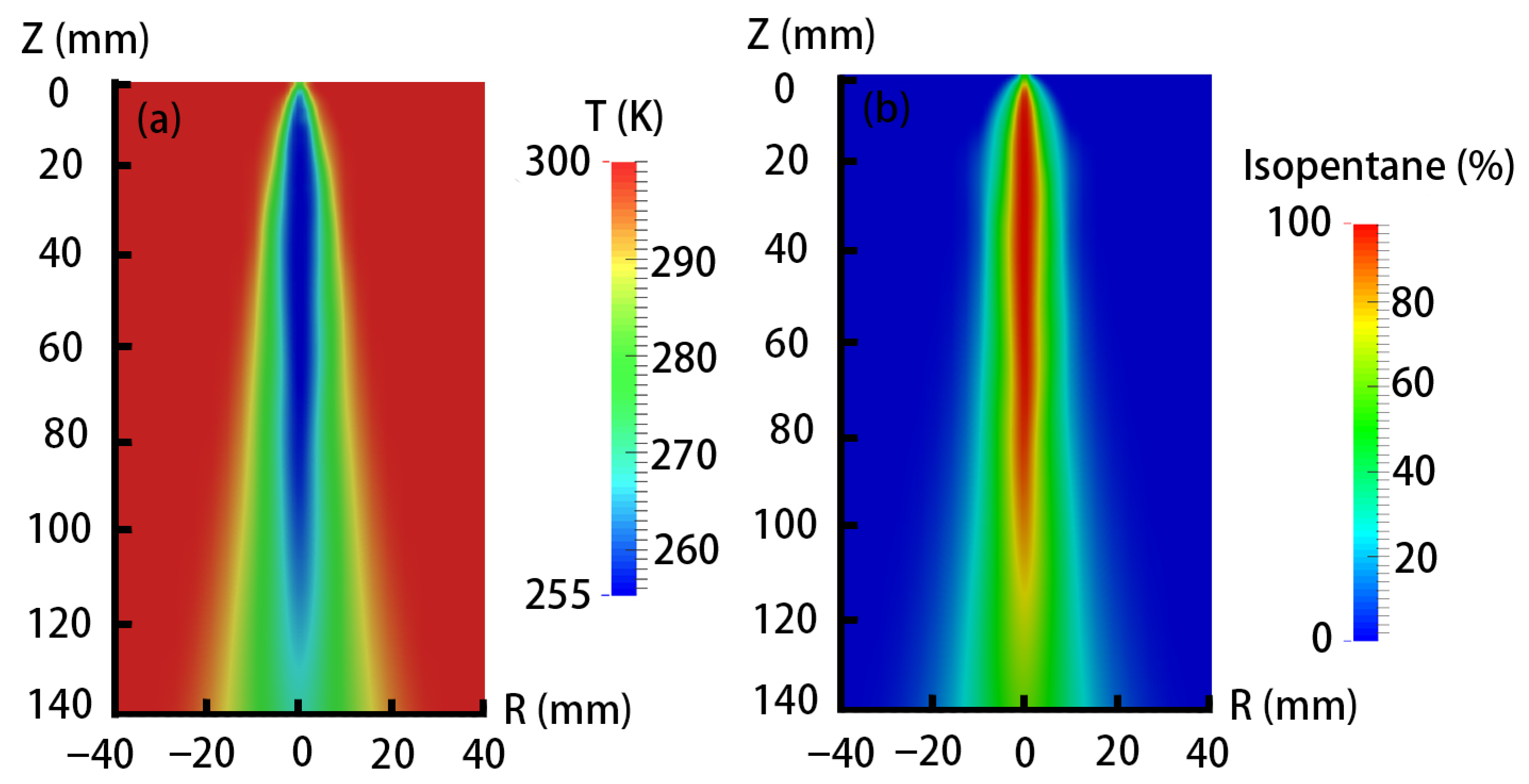
4.2. Spray Temperature and Vapor Concentration
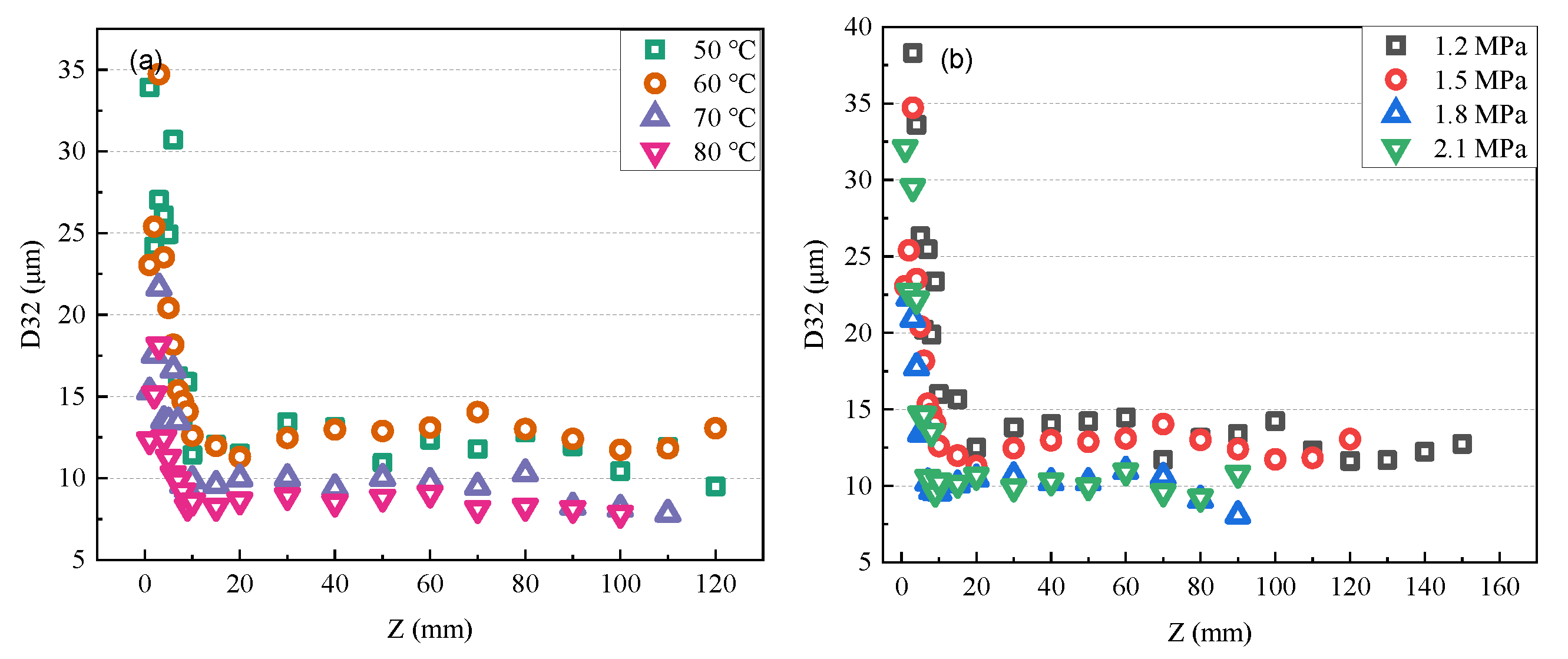
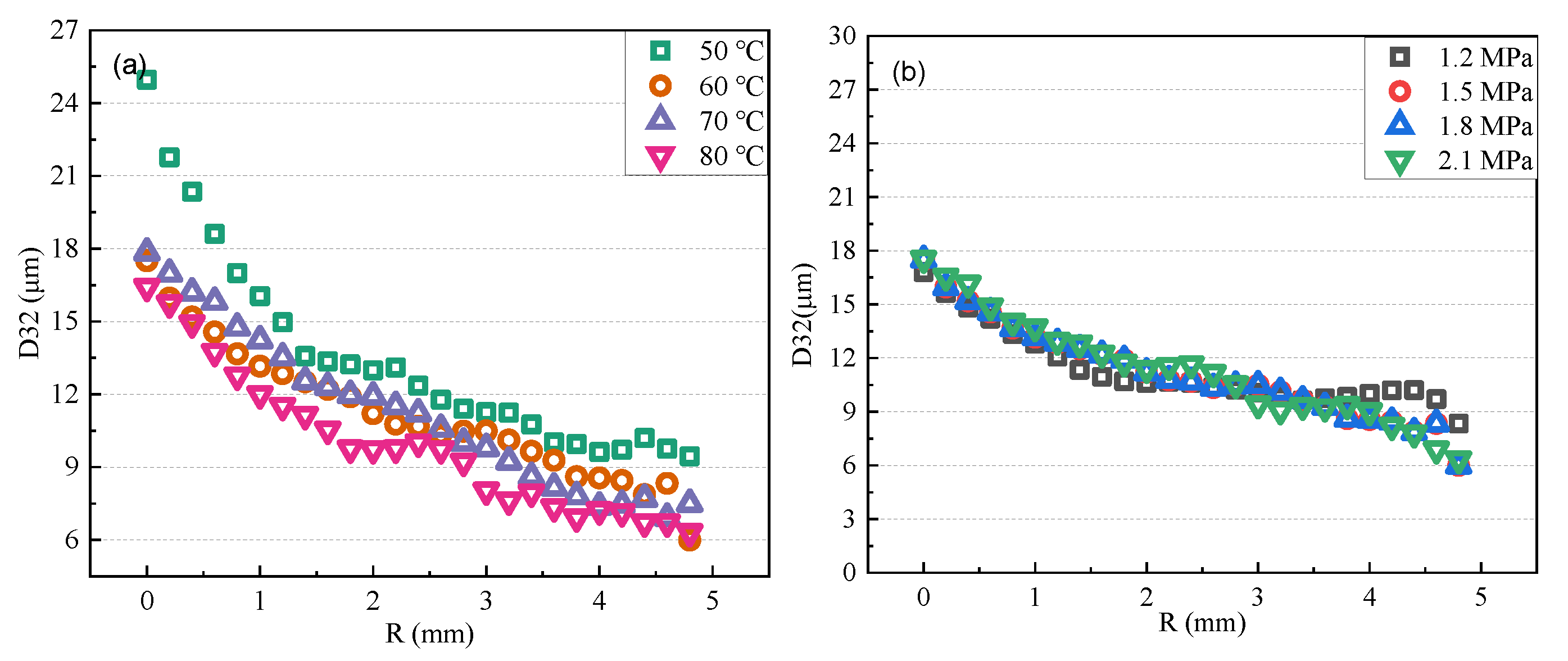
4.3. Spray Droplet Diameter Distribution
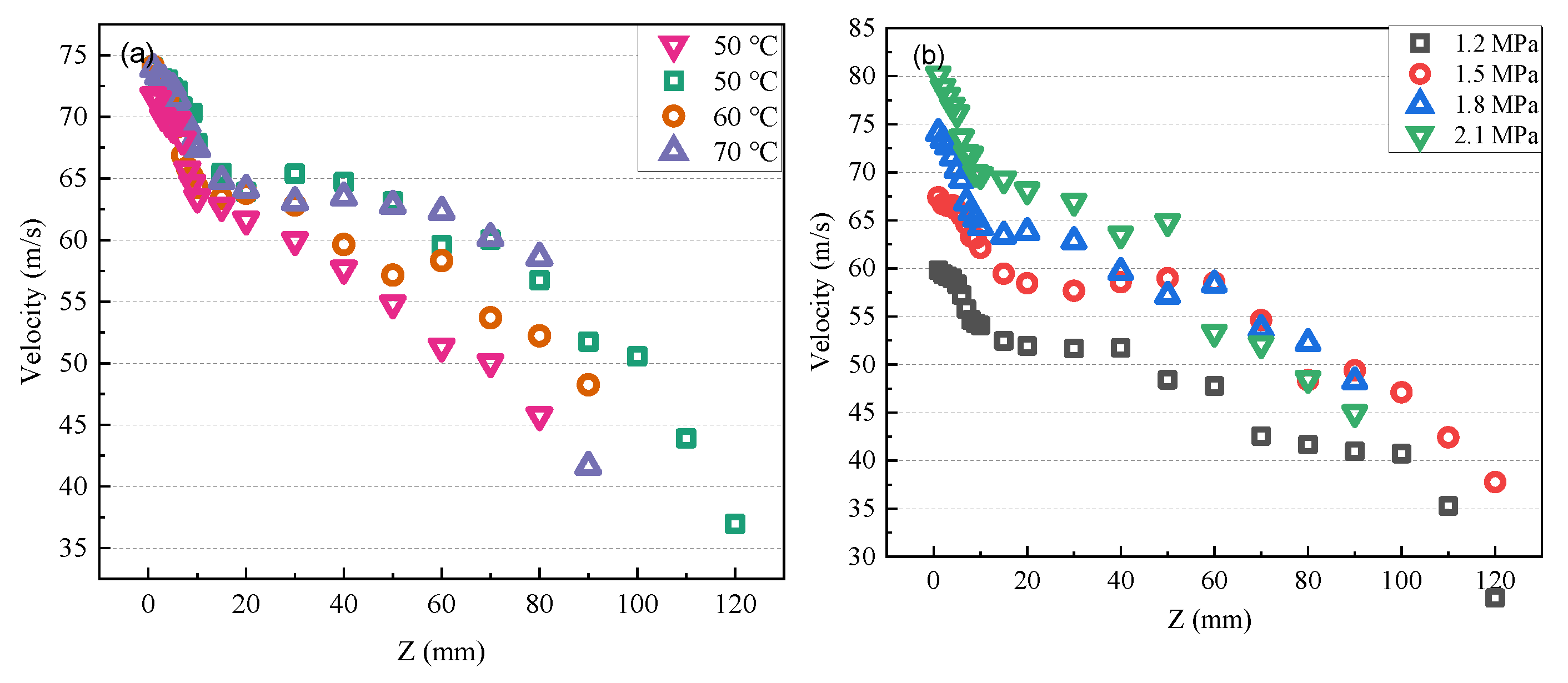
4.4. Spray Droplet Velocity Distribution
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, J.; Cao, B.; Zhang, R.; Li, P. Spray-coated tough thin film composite membrane for pervaporation desalination. Chem. Eng. Res. Des. 2022, 179, 493–501. [Google Scholar] [CrossRef]
- Xu, R.; Wang, G.; Jiang, P. Spray Cooling on Enhanced Surfaces: A Review of the Progress and Mechanisms. J. Electron. Packag. 2021, 144, 010802. [Google Scholar] [CrossRef]
- Zhou, Z.-F.; Chen, B.; Wang, R.; Wang, G.-X. Comparative investigation on the spray characteristics and heat transfer dynamics of pulsed spray cooling with volatile cryogens. Exp. Therm. Fluid Sci. 2017, 82, 189–197. [Google Scholar] [CrossRef]
- Zhou, Z.-f.; Chen, B.; Wang, R.; Bai, F.-l.; Wang, G.-x. Coupling effect of hypobaric pressure and spray distance on heat transfer dynamics of R134a pulsed flashing spray cooling. Exp. Therm. Fluid Sci. 2016, 70, 96–104. [Google Scholar] [CrossRef]
- Zhou, Z.F.; Murad, S.H.M.; Tian, J.M.; Camm, J.; Stone, R. Experimental investigation on heat transfer of n -pentane spray impingement on piston surface. Appl. Therm. Eng. 2018, 138, 197–206. [Google Scholar] [CrossRef]
- Qiu, S.; Xiao, D.; Zhang, X.; Wang, S.; Wang, T.; Li, X.; Xu, M. Experimental investigations of the phase change impacts on flash boiling spray propagations and impingements. Fuel 2022, 312, 122871. [Google Scholar] [CrossRef]
- Tardáguila, R.D.; Herranz, L.E. Attenuation of radioactive releases from NPP under accident conditions: An experimental research on risk-dominant scenarios. Chem. Eng. Res. Des. 2013, 91, 603–613. [Google Scholar] [CrossRef]
- Lee, J.J.; Yun, H.S.; Cho, Y.J.; Park, J.H. Empirical analysis of a steam explosion in a slag yard based on a field investigation and 3D explosion damage simulation. Process Saf. Environ. Prot. 2020, 136, 126–135. [Google Scholar] [CrossRef]
- Wu, F.; Yu, H.; Pan, X.; Zang, X.; Hua, M.; Wang, H.; Jiang, J. Experimental study of methanol atomization and spray explosion characteristic under negative pressure. Process Saf. Environ. Prot. 2022, 161, 162–174. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, H.; Pan, X.; Zhang, L.; Hua, M.; Zhang, C.; Zhou, J.; Yan, C.; Jiang, J. Experimental and numerical simulation research on fire suppression efficiency of dry powder mediums containing molybdenum flame retardant additive. Process Saf. Environ. Prot. 2022, 159, 294–308. [Google Scholar] [CrossRef]
- Hervieu, E.; Veneau, T. Experimental determination of the droplet size and velocity distributions at the exit of the bottom discharge pipe of a liquefied propane storage tank during a sudden blowdown. J. Loss Prev. Process Ind. 1996, 9, 413–425. [Google Scholar] [CrossRef]
- Allen, J.T. Laser-based measurements in two-phase flashing propane jets. Part one: Velocity profiles. J. Loss Prev. Process Ind. 1998, 11, 291–297. [Google Scholar] [CrossRef]
- Allen, J.T. Laser-based measurements in two-phase flashing propane jets. Part two: Droplet size distribution. J. Loss Prev. Process Ind. 1998, 11, 299–306. [Google Scholar] [CrossRef]
- Cleary, V.; Bowen, P.; Witlox, H. Flashing liquid jets and two-phase droplet dispersion: I. Experiments for derivation of droplet atomisation correlations. J. Hazard. Mater. 2007, 142, 786–796. [Google Scholar] [CrossRef] [PubMed]
- Witlox, H.; Harper, M.; Bowen, P.; Cleary, V. Flashing liquid jets and two-phase droplet dispersion: II. Comparison and validation of droplet size and rainout formulations. J. Hazard. Mater. 2007, 142, 797–809. [Google Scholar] [CrossRef] [PubMed]
- Witlox, H.W.M.; Harper, M.; Oke, A.; Bowen, P.J.; Kay, P. Sub-cooled and flashing liquid jets and droplet dispersion I. Overview and model implementation/validation. J. Loss Prev. Process Ind. 2010, 23, 831–842. [Google Scholar] [CrossRef]
- Kay, P.J.; Bowen, P.J.; Witlox, H.W.M. Sub-cooled and flashing liquid jets and droplet dispersion II. Scaled experiments and derivation of droplet size correlations. J. Loss Prev. Process Ind. 2010, 23, 849–856. [Google Scholar] [CrossRef]
- Yildiz, D.; Rambaud, P.; Van Beeck, J.; Buchlin, J. Evolution of the spray characteristics in superheated liquid jet atomization in function of initial flow conditions. In Proceedings of the 10th International Conference on Liquid Atomization and Spray Systems, Kyoto, Japan, 27 August – 1 September 2006. [Google Scholar]
- Yildiz, D.; van Beeck, J.P.A.J.; Riethmuller, M.L. Feasibility Exploration of Laser-based Techniques for Characterization of a Flashing Jet. Part. Part. Syst. Charact. 2004, 21, 390–402. [Google Scholar] [CrossRef]
- Aguilar, G.; Majaron, B.; Verkruysse, W.; Zhou, Y.; Nelson, J.S.; Lavernia, E.J. Theoretical and experimental analysis of droplet diameter, temperature, and evaporation rate evolution in cryogenic sprays. Int. J. Heat Mass Transf. 2001, 44, 3201–3211. [Google Scholar] [CrossRef]
- Vu, H.; García-Valladares, O.; Aguilar, G. Vapor/liquid phase interaction in flare flashing sprays used in dermatologic cooling. Int. J. Heat Mass Transf. 2008, 51, 5721–5731. [Google Scholar] [CrossRef]
- Zhou, Z.-F.; Hu, M.-Y.; Xin, H.; Chen, B.; Wang, G.-X. Experimental and theoretical studies on the droplet temperature behavior of R407C two-phase flashing spray. Int. J. Heat Mass Transf. 2019, 136, 664–673. [Google Scholar] [CrossRef]
- Zhifu, Z.; Weitao, W.; Bin, C.; Guoxiang, W.; Liejin, G. An experimental study on the spray and thermal characteristics of R134a two-phase flashing spray. Int. J. Heat Mass Transf. 2012, 55, 4460–4468. [Google Scholar] [CrossRef]
- Guo, X.; Tan, W.; Liu, L.; Liu, C.; Zhu, G. Experimental study of liquefied gas dynamic leakage behavior from a pressurized vessel. Process Saf. Environ. Prot. 2021, 151, 20–27. [Google Scholar] [CrossRef]
- Hulsbosch-Dam, C.E.C.; Spruijt, M.P.N.; Necci, A.; Cozzani, V. Assessment of particle size distribution in CO2 accidental releases. J. Loss Prev. Process Ind. 2012, 25, 254–262. [Google Scholar] [CrossRef]
- Calay, R.K.; Holdo, A.E. Modelling the dispersion of flashing jets using CFD. J. Hazard. Mater. 2008, 154, 1198–1209. [Google Scholar] [CrossRef]
- Lim, H.; Um, K.; Jung, S. A study on effective mitigation system for accidental toxic gas releases. J. Loss Prev. Process Ind. 2017, 49, 636–644. [Google Scholar] [CrossRef]
- Malekzadeh, S.; Roohi, E. Investigation of Different Droplet Formation Regimes in a T-junction Microchannel Using the VOF Technique in OpenFOAM. Microgravity Sci. Technol. 2015, 27, 231–243. [Google Scholar] [CrossRef]
- Zhou, Z.-F.; Lu, G.-Y.; Chen, B. Numerical study on the spray and thermal characteristics of R404A flashing spray using OpenFOAM. Int. J. Heat Mass Transf. 2018, 117, 1312–1321. [Google Scholar] [CrossRef]
- Liao, Y.; Lucas, D. Computational modelling of flash boiling flows: A literature survey. Int. J. Heat Mass Transf. 2017, 111, 246–265. [Google Scholar] [CrossRef]
- Price, C.; Hamzehloo, A.; Aleiferis, P.; Richardson, D. Numerical modelling of droplet breakup for flash-boiling fuel spray predictions. Int. J. Multiph. Flow 2020, 125, 103183. [Google Scholar] [CrossRef]
- Gerbino, F.; Tretola, G.; Morgan, R.; Atkins, P.; Vogiatzaki, K. Influence of the initial droplet distribution on the prediction of spray dynamics in Eulerian-Lagrangian simulations. Int. J. Multiph. Flow 2021, 141, 103642. [Google Scholar] [CrossRef]
- Liu, K.; Moore, C.; Balachandar, S. A physics-based advanced Euler-Lagrange framework for droplet-droplet interaction in dense spray systems. In Proceedings of the ILASS-Americas 30th Annual Conference on Liquid Atomization and Spray Systems, Tempe, AZ, USA, 12–15 May 2019. [Google Scholar]
- Balachandar, S. A scaling analysis for point–particle approaches to turbulent multiphase flows. Int. J. Multiph. Flow 2009, 35, 801–810. [Google Scholar] [CrossRef]
- Reitz, R.D. Modeling atomization processes in high-pressure vaporizing sprays. At. Spray Technol. 1987, 3, 309–337. [Google Scholar]
- Adachi, M.; McDonell, V.G.; Tanaka, D.; Senda, J.; Fujimoto, H. Characterization of Fuel Vapor Concentration Inside a Flash Boiling Spray; 0148-7191; SAE Technical Paper: Warrendale, PA, USA, 1997; p. 970871. [Google Scholar] [CrossRef]
- Sungkom, R.; Derksen, J.J.; Khinast, J.G. Modeling of aerated stirred tanks with shear-thinning power law liquids. Int. J. Heat Fluid Flow 2012, 36, 153–166. [Google Scholar] [CrossRef]
- Dapelo, D.; Bridgeman, J. Computational fluid dynamics modelling of unconfined gas mixing of wastewater sludge in a full scale anaerobic digester. In Proceedings of the Fifteenth International Conference on Civil, Structural and Environmental Engineering Computing; Civil-Comp Press: Stirlingshire, UK, 2015; 270 p. [Google Scholar] [CrossRef]
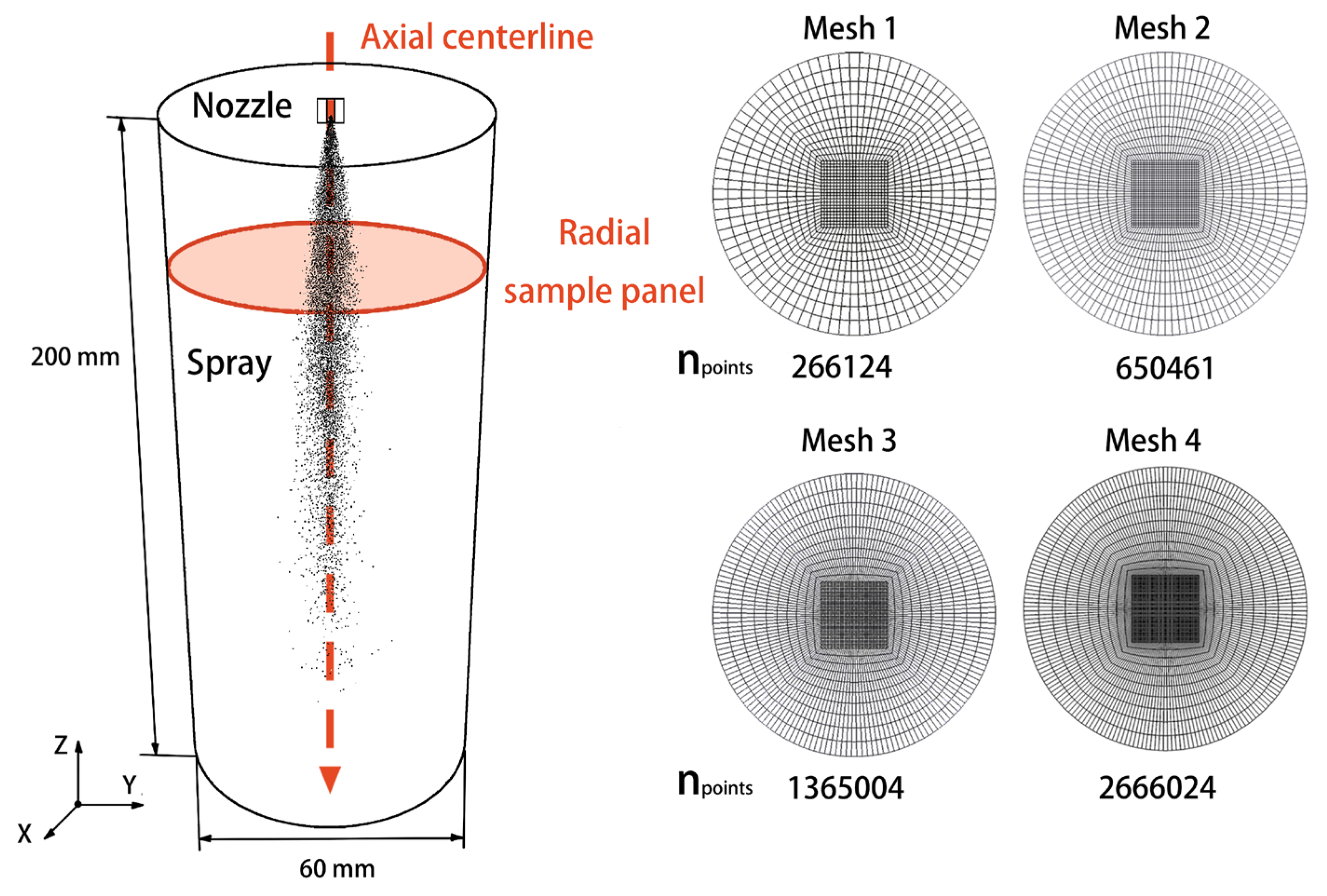
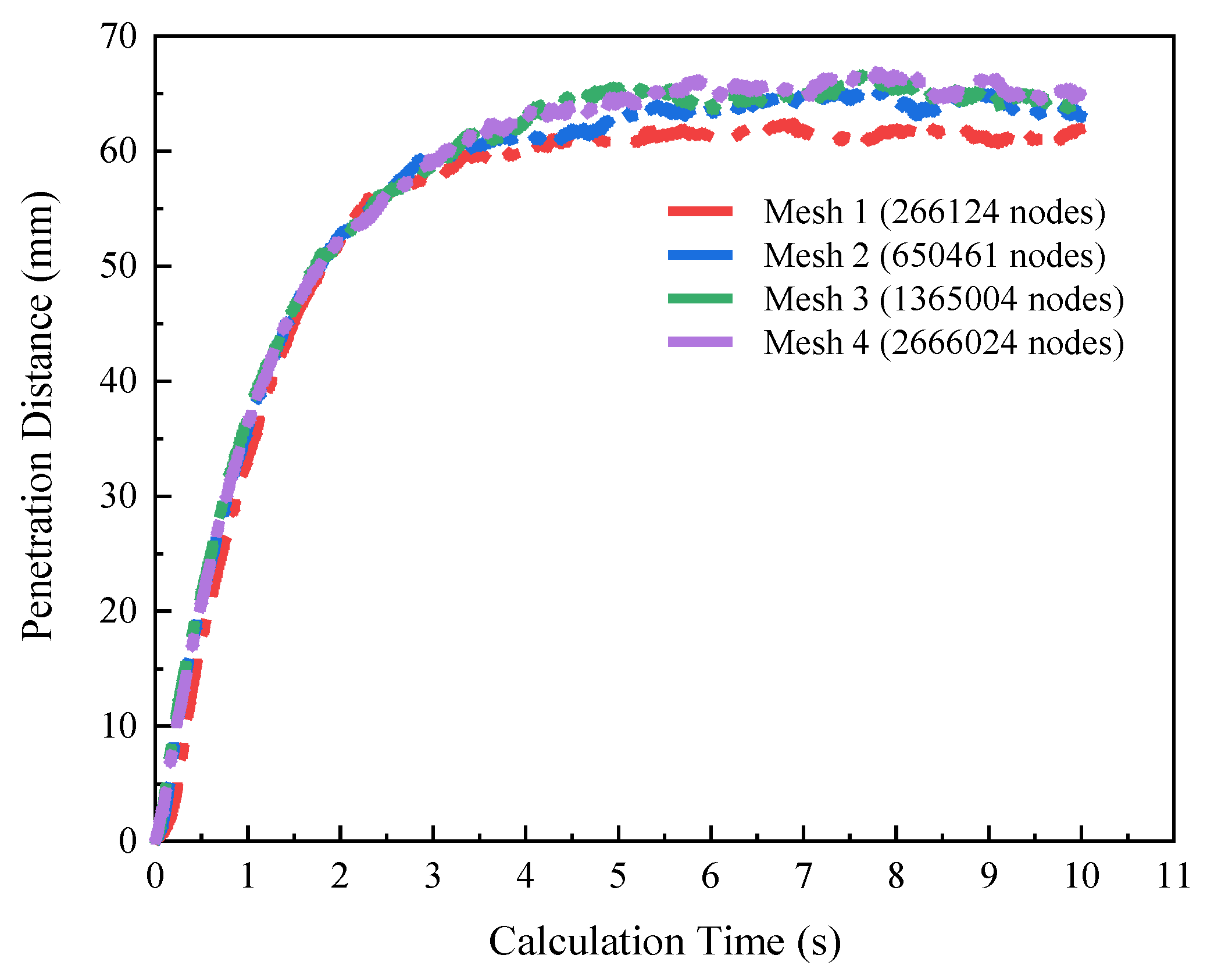
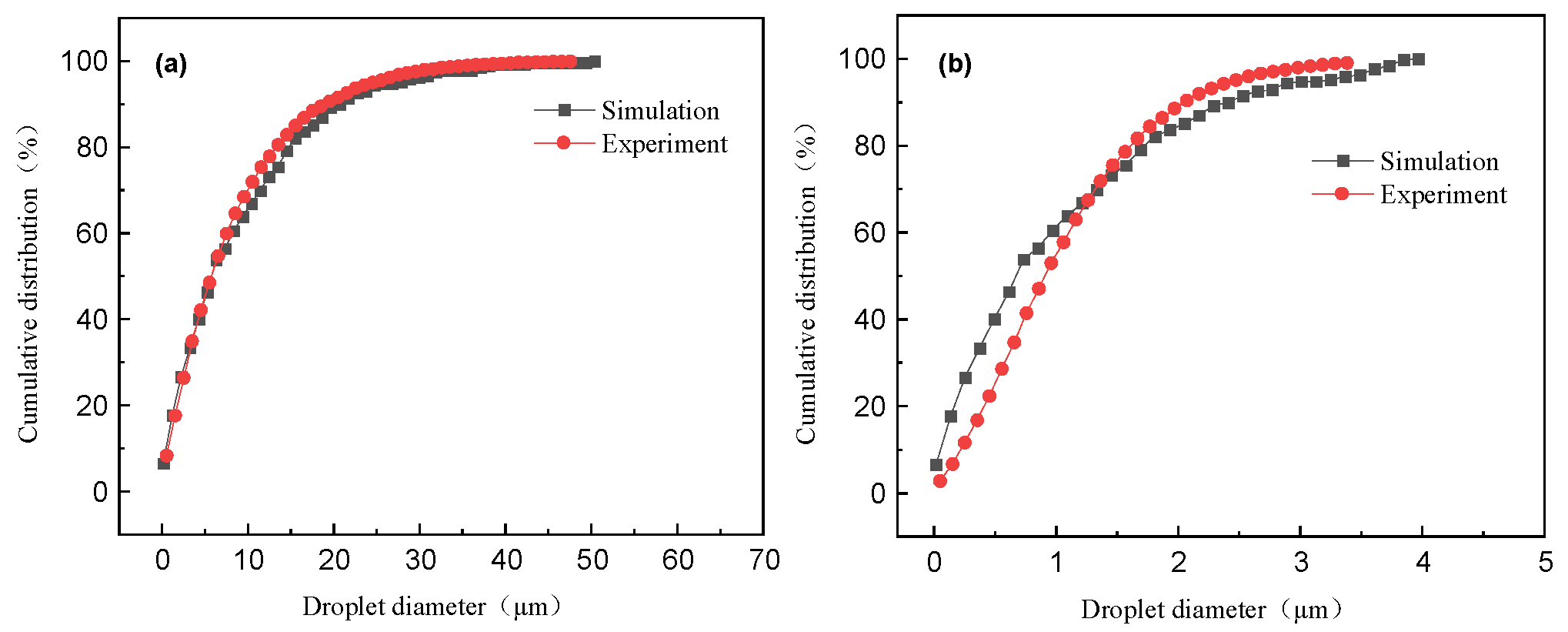
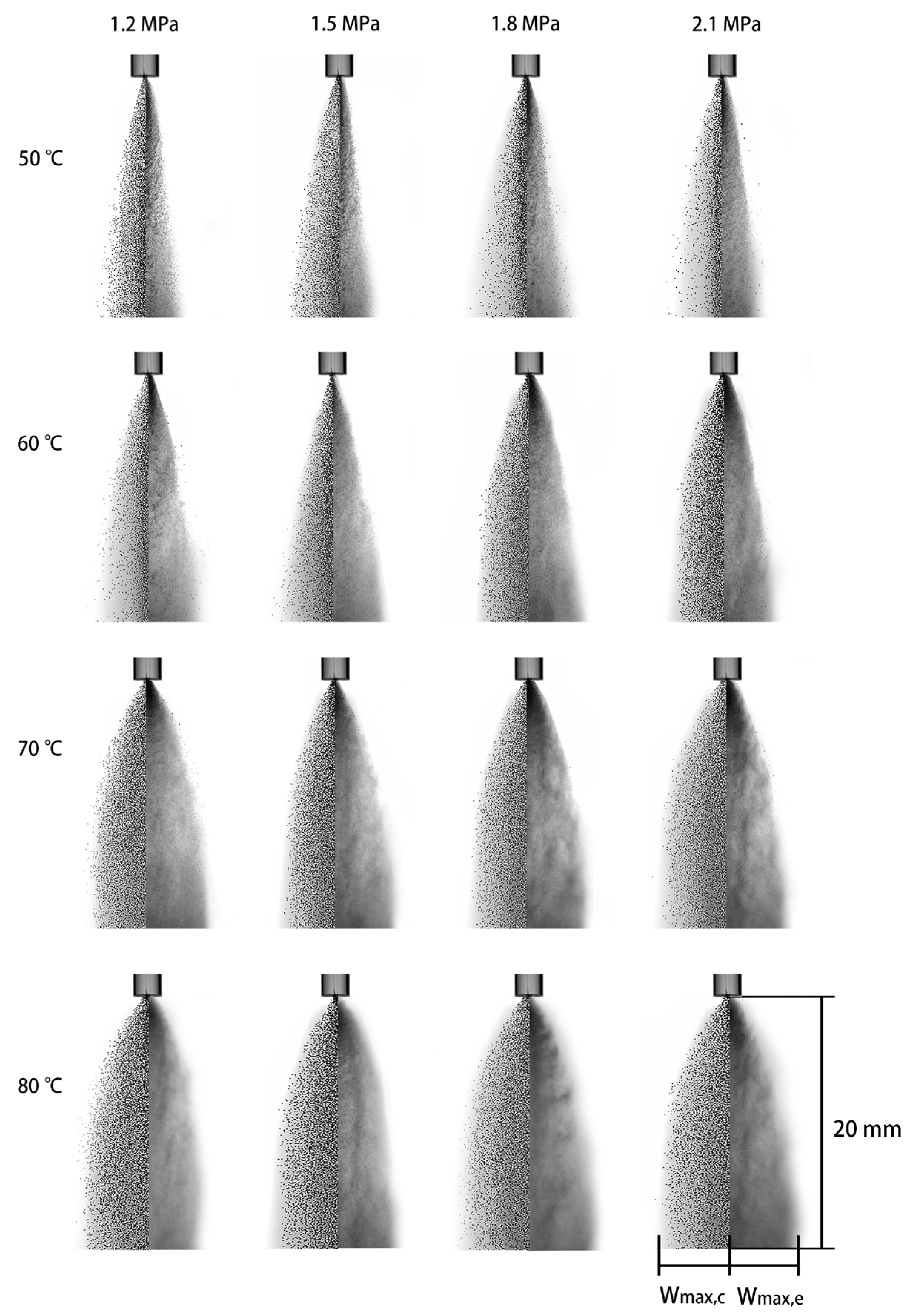

| Parameters | Value |
|---|---|
| Ambient temperature (K) | 300 |
| Ambient pressure (MPa) | 0.1 |
| Iso-pentane initial temperature (°C) | 50, 60, 70, and 80 |
| Iso-pentane inject pressure (MPa) | 1.2, 1.5, 1.8, and 2.1 |
| Diameter of the nozzle (mm) | 0.6 |
| Duration of injection (ms) | 10 |
| Maximum droplet diameter (um) | 60 |
| Minimum droplet diameter (um) | 0.5 |
| Parcels per second | 20,000,000 |
| Inject Pressure (MPa) | Inject Temperature (°C) | Spray Cone Angle (°) | Distribution Parameter | Distribution Parameter |
|---|---|---|---|---|
| 1.2 | 50 | 8.82 | 10.96 | 1.021 |
| 1.5 | 50 | 11.46 | 10.53 | 1.032 |
| 1.8 | 50 | 18.23 | 10.33 | 1.039 |
| 2.1 | 50 | 21.56 | 9.84 | 1.045 |
| 1.2 | 60 | 16.07 | 9.55 | 1.071 |
| 1.5 | 60 | 26.66 | 9.38 | 1.059 |
| 1.8 | 60 | 27.45 | 8.52 | 1.033 |
| 2.1 | 60 | 31.38 | 8.56 | 0.995 |
| 1.2 | 70 | 32.16 | 8.48 | 1.045 |
| 1.5 | 70 | 36.37 | 8.49 | 1.014 |
| 1.8 | 70 | 41.28 | 8.48 | 1.045 |
| 2.1 | 70 | 42.49 | 8.96 | 1.030 |
| 1.2 | 80 | 45.21 | 8.52 | 1.023 |
| 1.5 | 80 | 46.52 | 8.35 | 1.015 |
| 1.8 | 80 | 47.20 | 8.42 | 1.009 |
| 2.1 | 80 | 47.95 | 8.38 | 1.012 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, D.-Q.; Chen, S.-Y.; Xing, H.-J.; Zhou, Z.-F.; Wang, J.-F.; Chen, B. Investigation on Spray Morphology, Droplet Dynamics, and Thermal Characteristics of Iso-Pentane Flashing Spray Based on OpenFOAM. Appl. Sci. 2022, 12, 12899. https://doi.org/10.3390/app122412899
Zhu D-Q, Chen S-Y, Xing H-J, Zhou Z-F, Wang J-F, Chen B. Investigation on Spray Morphology, Droplet Dynamics, and Thermal Characteristics of Iso-Pentane Flashing Spray Based on OpenFOAM. Applied Sciences. 2022; 12(24):12899. https://doi.org/10.3390/app122412899
Chicago/Turabian StyleZhu, Dong-Qing, Shu-Yan Chen, Hong-Jie Xing, Zhi-Fu Zhou, Jia-Feng Wang, and Bin Chen. 2022. "Investigation on Spray Morphology, Droplet Dynamics, and Thermal Characteristics of Iso-Pentane Flashing Spray Based on OpenFOAM" Applied Sciences 12, no. 24: 12899. https://doi.org/10.3390/app122412899
APA StyleZhu, D.-Q., Chen, S.-Y., Xing, H.-J., Zhou, Z.-F., Wang, J.-F., & Chen, B. (2022). Investigation on Spray Morphology, Droplet Dynamics, and Thermal Characteristics of Iso-Pentane Flashing Spray Based on OpenFOAM. Applied Sciences, 12(24), 12899. https://doi.org/10.3390/app122412899






