Removal of Intra-Array Statics in Seismic Arrays Due to Variable Topography and Positioning Errors
Abstract
Featured Application
Abstract
1. Introduction
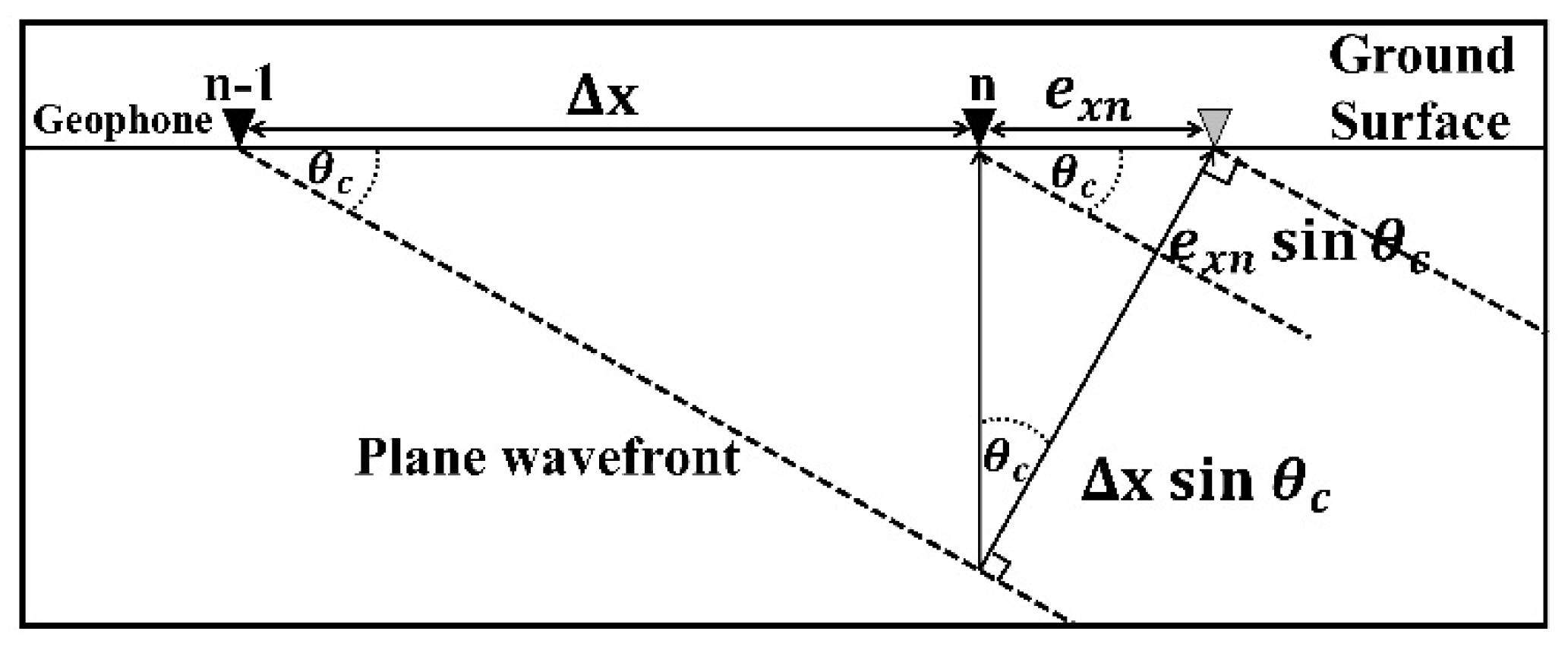
2. Methodology
2.1. Direct Event
2.2. Refraction Event
2.3. Position and Elevation Error
2.3.1. Position Error
2.3.2. Elevation Error
2.4. Traveltimes for Ideal and Error Cases
2.4.1. Perturbed Case
2.4.2. Position’s Error Removal
2.4.3. Elevation Error Removal
2.4.4. Ideal Case
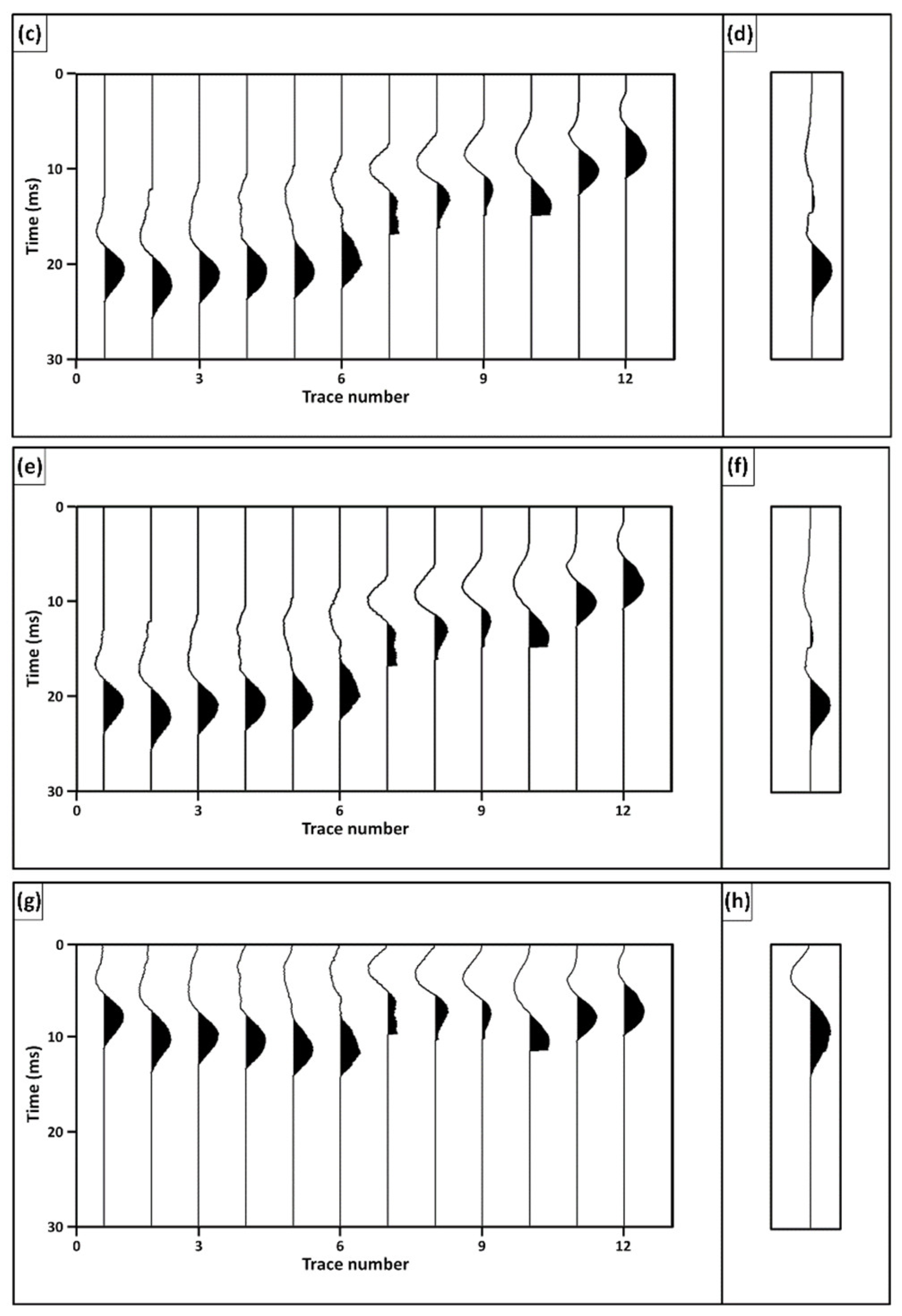
2.5. Wavelet Response and Trace Energy
- Window the first wavelet of the first arrival head-wave event while maintaining the timing information () of the first arrival on each trace.
- Shift each nth windowed trace such that its first sample is at . This produces the ideal trace wavelet response (). Sum across to produce the ideal array response () and calculate its corresponding array trace energy () as follows:where is the number of samples in .
- Shift each by its corresponding to produce the trace wavelet response with position errors only (). Sum across n to produce the array response with position errors only () and calculate its corresponding array trace energy (Ex) as follows:
- 4.
- Starting with again, shift each by its corresponding to produce the trace wavelet response with elevation errors only (). Sum across n to produce the array response with elevation errors only () and calculate its corresponding array trace energy () as follows:
- 5.
- Starting with again, shift each by its corresponding () to produce the perturbed wavelet response of the trace with combined position and elevation errors (). Sum across n to produce the array response with both position and elevation errors () and calculate its corresponding array trace energy () as follows:
3. Results and Discussion
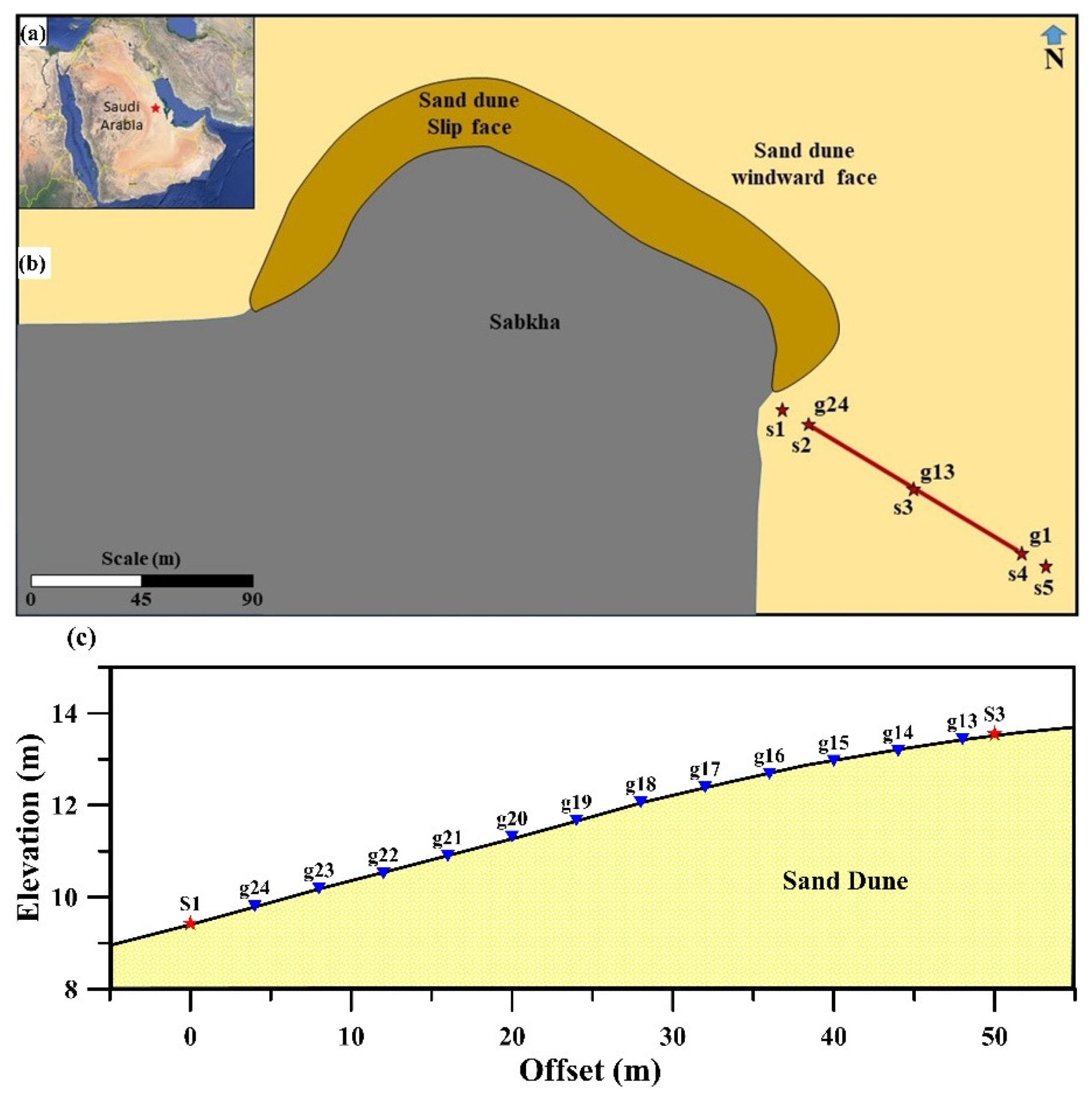
3.1. Geological Setting
3.2. Data Acquisition
3.3. Data Analysis
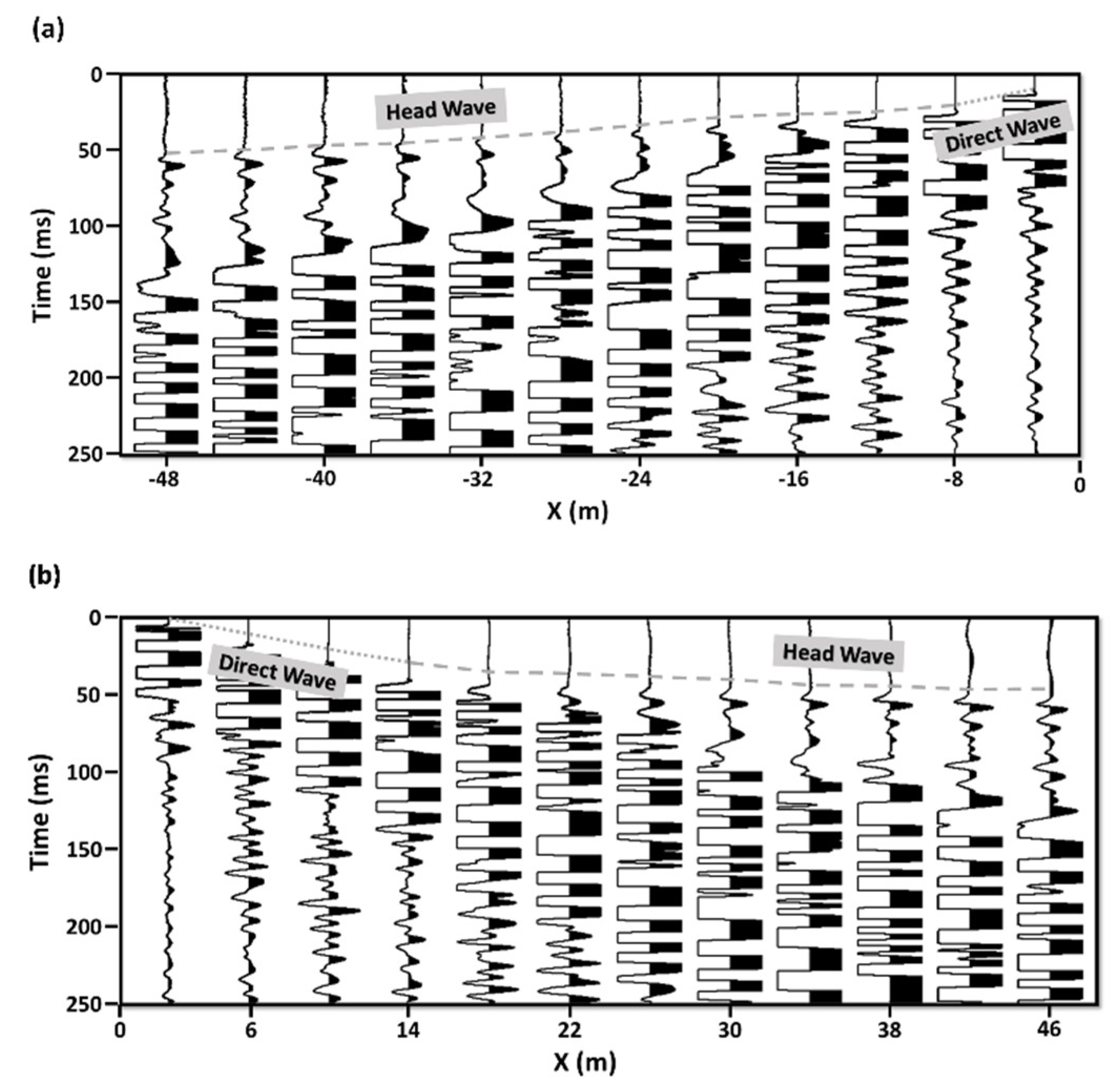
3.3.1. First Arrival Picking
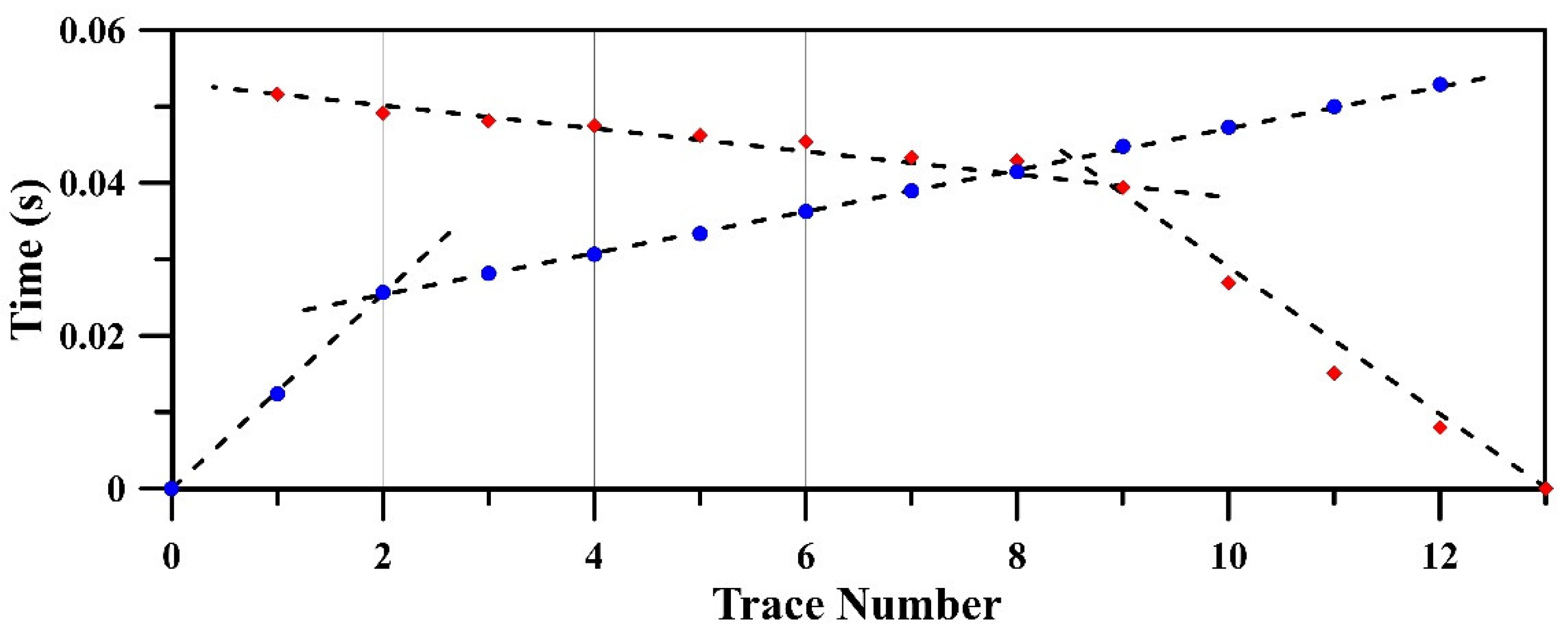
3.3.2. Velocity Calculation
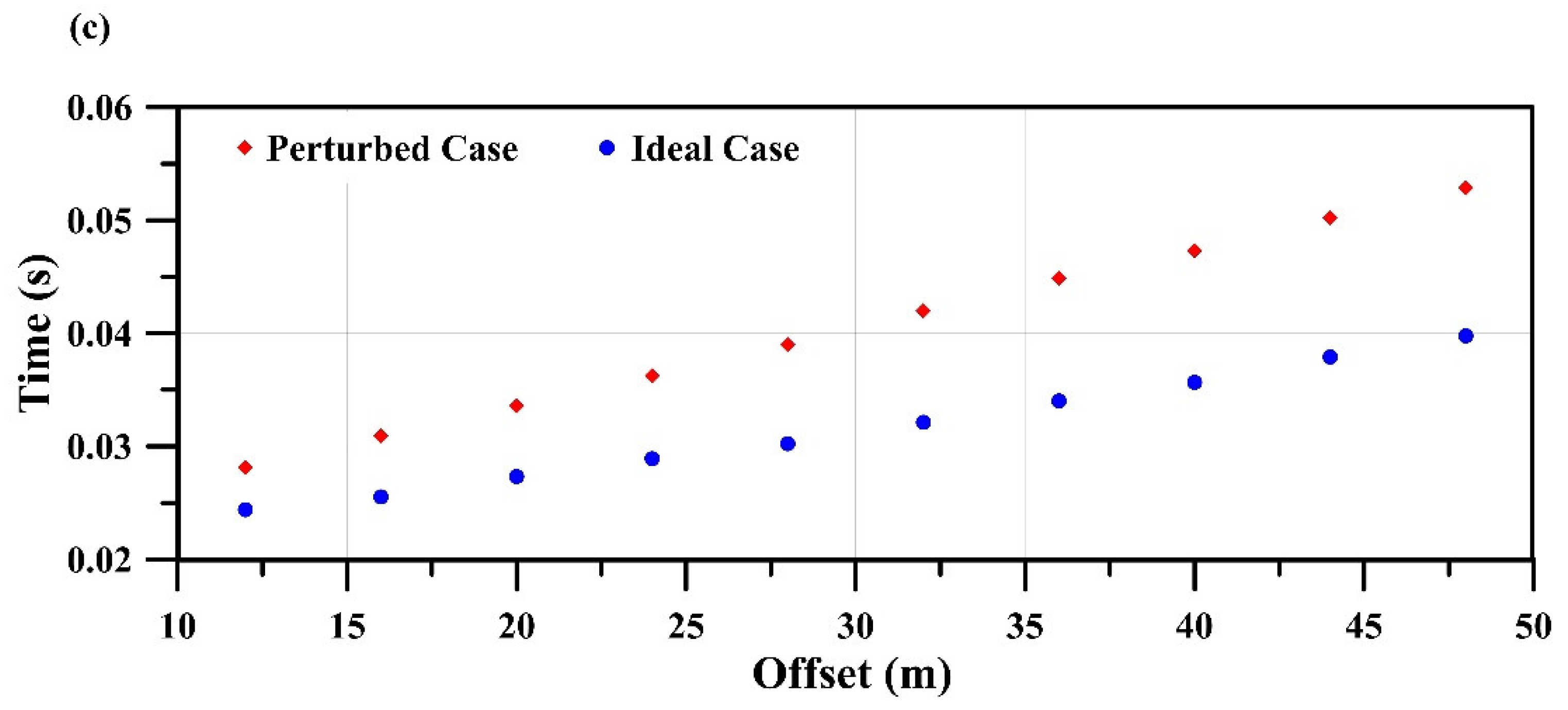
3.3.3. Error Calculation
3.3.4. Responses Calculation
3.3.5. Comparison with Previous Studies
- Generate 12 random position errors from a normal distribution with zero mean and normalized standard deviation of position errors calculated in step 1.
- Generate the array response with position errors only.
- Calculate the corresponding trace energy.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aldridge, D.F. Statistically perturbed geophone array responses. Geophysics 1989, 54, 1306–1318. [Google Scholar] [CrossRef]
- Rost, S.; Thomas, C. Array seismology—Methods and applications. Rev. Geophys. 2002, 40, 1008. [Google Scholar] [CrossRef]
- Douglas, A.; Bowers, D.; Marshall, P.D.; Young, J.B.; Porter, D.; Wallis, N.J. Putting nuclear-test monitoring to the test. Nature 1999, 398, 474–475. [Google Scholar] [CrossRef]
- Douglas, A. Seismometer arrays—Their use in earthquake and test ban seismology. Int. Geophys. Ser. Part A 2002, 81, 357–367. [Google Scholar]
- Kárason, H.; van der Hilst, R.D. Tomographic imaging of the lowermost mantle with differential times of refracted and diffracted core phases (PKP, Pdiff). J. Geophys. Res. 2001, 106, 6569–6587. [Google Scholar] [CrossRef]
- Cao, W.; Hanafy, S.M.; Schuster, G.T.; Zhan, G.; Boonyasiriwat, C. High-resolution and super stacking of time-reversal mirrors in locating seismic sources. Geophys. Prospect. 2012, 60, 1–17. [Google Scholar] [CrossRef]
- Cao, W.; Schuster, G.T.; Zhan, G.; Hanafy, S.M.; Boonyasiriwat, C. Demonstration of super-resolution and super-stacking properties of time reversal mirrors in locating seismic sources. In Proceedings of the 2008 SEG Annual Meeting, Las Vegas, NV, USA, 9–14 November 2008; pp. 3018–3022. [Google Scholar]
- Khalil, M.H.; Hanafy, S.M. Geotechnical parameters from seismic measurements: Two field examples from Egypt and Saudi Arabia. J. Environ. Eng. Geophys. 2016, 21, 13–28. [Google Scholar] [CrossRef][Green Version]
- Lu, K.; Hanafy, S.M.; Stanistreet, I.; Njau, J.; Schick, K.; Toth, N.; Stollhofen, H.; Schuster, G. Seismic imaging of the Olduvai Basin, Tanzania. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2019, 533, 109246. [Google Scholar] [CrossRef]
- Hanafy, S.M.; Soupios, P.; Stampolidis, A.; Koch, C.B.; Al-Ramadan, K.; Al-Shuhail, A.; Solling, T.; Argadestya, I. Comprehensive Geophysical study at Wabar crater, Rub Al-Khali desert, Saudi Arabia. Earth Space Sci. 2021, 8, e2020EA001432. [Google Scholar] [CrossRef]
- Oye, V.; Dando, B.; Wustefeld, A.; Jerkins, A.; Koehler, A. Cost-effective Baseline Studies for Induced Seismicity Monitoring Related to CO2 Storage Site Preparation. In Proceedings of the 15th Greenhouse Gas Control Technologies Conference, Abu Dhabi, United Arab Emirates, 15–18 March 2021. [Google Scholar] [CrossRef]
- Mi, B.; Xia, J.; Tian, G.; Shi, Z.; Xing, H.; Chang, X.; Xi, C.; Liu, Y.; Ning, L.; Dai, T.; et al. Near-surface imaging from traffic-induced surface waves with dense linear arrays: An application in the urban area of Hangzhou, China. Geophysics 2022, 87, 145–158. [Google Scholar] [CrossRef]
- Näsholm, S.P.; Iranpour, K.; Wuestefeld, A.; Dando, B.D.; Baird, A.F.; Oye, V. Array signal processing on distributed acoustic sensing data: Directivity effects in slowness space. J. Geophys. Res. Solid Earth 2022, 127, e2021JB023587. [Google Scholar] [CrossRef]
- Martin, E. A linear algorithm for ambient seismic noise double beamforming without explicit crosscorrelations. Geophysics 2021, 86, F1–F8. [Google Scholar] [CrossRef]
- Yang, J.; Shragge, J. Measuring seasonal velocity variations on an urban DAS array. SEG Technical Program Expanded Abstracts. In Proceedings of the Second International Meeting for Applied Geoscience & Energy, Houston, TX, USA, 28 August–1 September 2022; pp. 626–631. [Google Scholar] [CrossRef]
- Hanafy, S.M. Land-Streamer vs. Conventional Seismic Data for High-Resolution Near-Surface Surveys. Appl. Sci. 2022, 12, 584. [Google Scholar] [CrossRef]
- Ramdani, A.; Khanna, P.; Gairola, G.; Hanafy, S.M.; Vahrenkamp, V. Assessing and Processing 3D Photogrammetry, Sedimentology and Geophysical Data to Build High-fidelity Reservoir Models Based on Carbonate Outcrop Analogs. AAPG Bull. 2022, 106, 1975–2011. [Google Scholar]
- Ramdani, A.; Khanna, P.; De Jong, S.; Gairola, G.S.; Hanafy, S.M.; Vahrenkamp, V. Three-dimensional morphometric analysis and statistical distribution of the Early Kimmeridgian Hanifa Formation stromatoporoid/coral buildups, central Saudi Arabia. Mar. Pet. Geol. 2022, 146, 105934. [Google Scholar] [CrossRef]
- Hoffe, B.H.; Margrave, G.F.; Stewart, R.R.; Foltinek, D.S.; Bland, H.C.; Manning, P.M. Analyzing the effectiveness of receiver arrays for multicomponent seismic exploration. Geophysics 2002, 67, 1853–1868. [Google Scholar] [CrossRef]
- Li, G.; Zheng, H.; Wang, J.; Huang, W. Inversion-based directional deconvolution to remove the effect of a geophone array on seismic signal. J. Appl. Geophys. 2016, 130, 91–100. [Google Scholar] [CrossRef]
- Smith, M.K. Noise analysis and multiple seismometer theory. Geophysics 1956, 21, 337–360. [Google Scholar] [CrossRef]
- Savit, C.H. Transient behavior of patterns. Geophysics 1958, 23, 360–362. [Google Scholar] [CrossRef]
- Newman, P.; Mahoney, J.T. Patterns-with a pinch of salt. Geophys. Prospect. 1973, 21, 197–219. [Google Scholar] [CrossRef]
- Dudgeon, D.E.; Johnson, D.H. Array Signal Processing—Concepts and Techniques; Prentice-Hall Inc.: Hoboken, NJ, USA, 1993; 533p. [Google Scholar]
- Gangi, A.F.; Benson, M.A. Wavelet response of seismic arrays. In SEG Expanded Abstracts; Society of Exploration Geophysicists: Houston, TX, USA, 1989; pp. 663–666. [Google Scholar]
- Al-Shuhail, A.A.; Gangi, A.F. The effect of topography on the wavelet response of the seismic arrays. In SEG Expanded Abstracts; Society of Exploration Geophysicists: Houston, TX, USA, 1994; pp. 895–898. [Google Scholar]
- Al-Shuhail, A.A.; Al-Ghanim, A. Performance of seismic arrays in heterogeneous medium. GeoFrontier 2003, 1, 27–30. [Google Scholar]
- Al-Shuhail, A.A. Seismic array response in the presence of a dipping shallow layer. Signal Image Video Process. 2013, 7, 263–274. [Google Scholar] [CrossRef]
- Akram, J. Seismic Arrays Response in the Presence of Laterally Varying Thickness of the Weathering Layer. Master’s Thesis, King Fahd University of Petroleum and Minerals, Dhahran, Saudi Arabia, 2007. [Google Scholar]
- Akram, J.; Al-Shuhail, A.A. Performance of seismic arrays in the presence of weathering layer variations. Arab. J. Geosci. 2016, 9, 522–529. [Google Scholar] [CrossRef]
- Putra, R.; Al-Shuhail, A.A. Seismic Array Response in the Presence of Intra-Array Variations in Element Weights, Elevations, and Positions. In Proceedings of the 13th Middle East Geosciences Conference and Exhibition, Geo-2018, Manama, Bahrain, 5–8 March 2018. [Google Scholar]
- Sheriff, R.E.; Geldart, L.P. Exploration Seismology, 2nd ed.; Cambridge University Press: Cambridge, UK, 1995; 1582p, ISBN 978-0521468268. [Google Scholar]
- Schiller, J. Schaum’s Outline of Probability and Statistics, 4th ed.; McGraw-Hill Education: New York, NY, USA, 2013; 432p, ISBN 978-0071795579. [Google Scholar]



| Shot Gather No. | Trace No | Offset (m) | First Arrival Time (s) | |
|---|---|---|---|---|
| Shot Gather 1 | Trace 1 | 4 | 0.013 | 311 |
| Trace 2 | 8 | 0.025 | 323 | |
| Shot Gather 2 | Trace 2 | 4 | 0.013 | 299 |
| Trace 3 | 8 | 0.026 | 320 | |
| Shot Gather 3 (left) | Trace 1 * | 2 | 0.004 | 500 |
| Trace 2 | 6 | 0.015 | 349 | |
| Shot Gather 3 (right) | Trace 1 * | 2 | 0.005 | 400 |
| Trace 2 | 6 | 0.016 | 344 | |
| Shot Gather 4 | Trace 2 | 4 | 0.013 | 314 |
| Trace 3 | 8 | 0.027 | 283 | |
| Shot Gather 5 | Trace 1 | 4 | 0.013 | 299 |
| Trace 2 | 8 | 0.027 | 286 | |
| Average velocity V1 (m/s) | 313 | |||
| Trace Number | Shot Gather 1 (Down-dip) | Shot Gather 3 (Up-dip) | ||
|---|---|---|---|---|
| Offset (m) | First Arrival Time (s) | Offset (m) | First Arrival Time (s) | |
| 13 | 48 | 0.053 | 2 | 0.004 |
| 14 | 44 | 0.050 | 6 | 0.015 |
| 15 | 40 | 0.047 | 10 | 0.027 |
| 16 | 36 | 0.045 | 14 | 0.039 |
| 17 | 32 | 0.042 | 18 | 0.043 |
| 18 | 28 | 0.039 | 22 | 0.044 |
| 19 | 24 | 0.036 | 26 | 0.045 |
| 20 | 20 | 0.034 | 30 | 0.046 |
| 21 | 16 | 0.031 | 34 | 0.047 |
| 22 | 12 | 0.028 | 38 | 0.048 |
| 23 | 8 | 0.026 | 42 | 0.049 |
| 24 | 4 | 0.012 | 46 | 0.052 |
| (deg) | 3.2 | |||
| (deg) | 9.2 | |||
| (m/s) | 1455 | 2967 | ||
| (m/s) | 1949 | |||
| Trace | (m) | (m) | Calculated Distance (m) | |
|---|---|---|---|---|
| Reference position | 2,915,129 | 383,141 | ||
| 13 | 2,915,126 | 383,189 | 3.995 | −0.005 |
| 14 | 2,915,126 | 383,185 | 3.945 | −0.055 |
| 15 | 2,915,127 | 383,181 | 4.035 | 0.035 |
| 16 | 2,915,127 | 383,177 | 3.939 | −0.061 |
| 17 | 2,915,127 | 383,173 | 4.025 | 0.025 |
| 18 | 2,915,127 | 383,169 | 3.972 | −0.028 |
| 19 | 2,915,127 | 383,165 | 3.985 | −0.015 |
| 20 | 2,915,127 | 383,161 | 3.938 | −0.062 |
| 21 | 2,915,128 | 383,157 | 4.022 | 0.022 |
| 22 | 2,915,128 | 383,153 | 3.940 | −0.060 |
| 23 | 2,915,128 | 383,149 | 3.986 | −0.014 |
| 24 | 2,915,128 | 383,145 | 4.056 | 0.056 |
| Minimum position error (m) | −0.062 | |||
| Maximum position error (m) | 0.056 | |||
| Mean position error (m) | −0.014 | |||
| Median position error (m) | −0.015 | |||
| Standard deviation of position errors (m) | 0.041 | |||
| Trace | (m) | (m) | Elevation Z (m) | |
|---|---|---|---|---|
| Reference position | 2,915,129 | 383,141 | 9.41 | |
| 13 | 2,915,126 | 383,189 | 13.44 | 4.03 |
| 14 | 2,915,126 | 383,185 | 13.19 | 3.78 |
| 15 | 2,915,127 | 383,181 | 12.97 | 3.56 |
| 16 | 2,915,127 | 383,177 | 12.70 | 3.29 |
| 17 | 2,915,127 | 383,173 | 12.41 | 3.00 |
| 18 | 2,915,127 | 383,169 | 12.08 | 2.67 |
| 19 | 2,915,127 | 383,165 | 11.68 | 2.27 |
| 20 | 2,915,127 | 383,161 | 11.32 | 1.91 |
| 21 | 2,915,128 | 383,157 | 10.90 | 1.50 |
| 22 | 2,915,128 | 383,153 | 10.52 | 1.11 |
| 23 | 2,915,128 | 383,149 | 10.19 | 0.78 |
| 24 | 2,915,128 | 383,145 | 9.81 | 0.40 |
| Minimum elevation error (m) | 0.40 | |||
| Maximum elevation error (m) | 4.03 | |||
| Mean elevation error (m) | 2.36 | |||
| Median elevation error (m) | 2.47 | |||
| Standard deviation of elevation errors (m) | 1.22 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hanafy, S.M.; Al-Mashhor, A.; Al-Shuhail, A.A. Removal of Intra-Array Statics in Seismic Arrays Due to Variable Topography and Positioning Errors. Appl. Sci. 2022, 12, 12810. https://doi.org/10.3390/app122412810
Hanafy SM, Al-Mashhor A, Al-Shuhail AA. Removal of Intra-Array Statics in Seismic Arrays Due to Variable Topography and Positioning Errors. Applied Sciences. 2022; 12(24):12810. https://doi.org/10.3390/app122412810
Chicago/Turabian StyleHanafy, Sherif Mohamed, Abdullah Al-Mashhor, and Abdullatif Abdulrahman Al-Shuhail. 2022. "Removal of Intra-Array Statics in Seismic Arrays Due to Variable Topography and Positioning Errors" Applied Sciences 12, no. 24: 12810. https://doi.org/10.3390/app122412810
APA StyleHanafy, S. M., Al-Mashhor, A., & Al-Shuhail, A. A. (2022). Removal of Intra-Array Statics in Seismic Arrays Due to Variable Topography and Positioning Errors. Applied Sciences, 12(24), 12810. https://doi.org/10.3390/app122412810





