High-Quality Coherent Plane-Wave Compounding Using Enhanced Covariance-Matrix-Based Statistical Beamforming
Abstract
1. Introduction
2. Methods
2.1. Coherent Plane-Wave Compounding (CPWC)
2.2. Delay Multiply and Sum (DMAS)
2.3. Short-Lag Spatial Coherence (SLSC)
2.4. Covariance Matrix-Based Statistical Beamforming (CMSB)
2.5. Proposed Methods
2.5.1. Lag-One Coherence Weighting for CMSB
2.5.2. Adaptive Diagonal Reducing for CMSB
2.5.3. LOC-Weighted CMSB with Adaptive Diagonal Reducing
3. Simulation and Experimental Setups
3.1. Simulated Tissue-Mimicking Phantom Study
3.2. Experimental Phantom and In Vivo Carotid Studies
3.3. Image Quality Metrics
4. Results
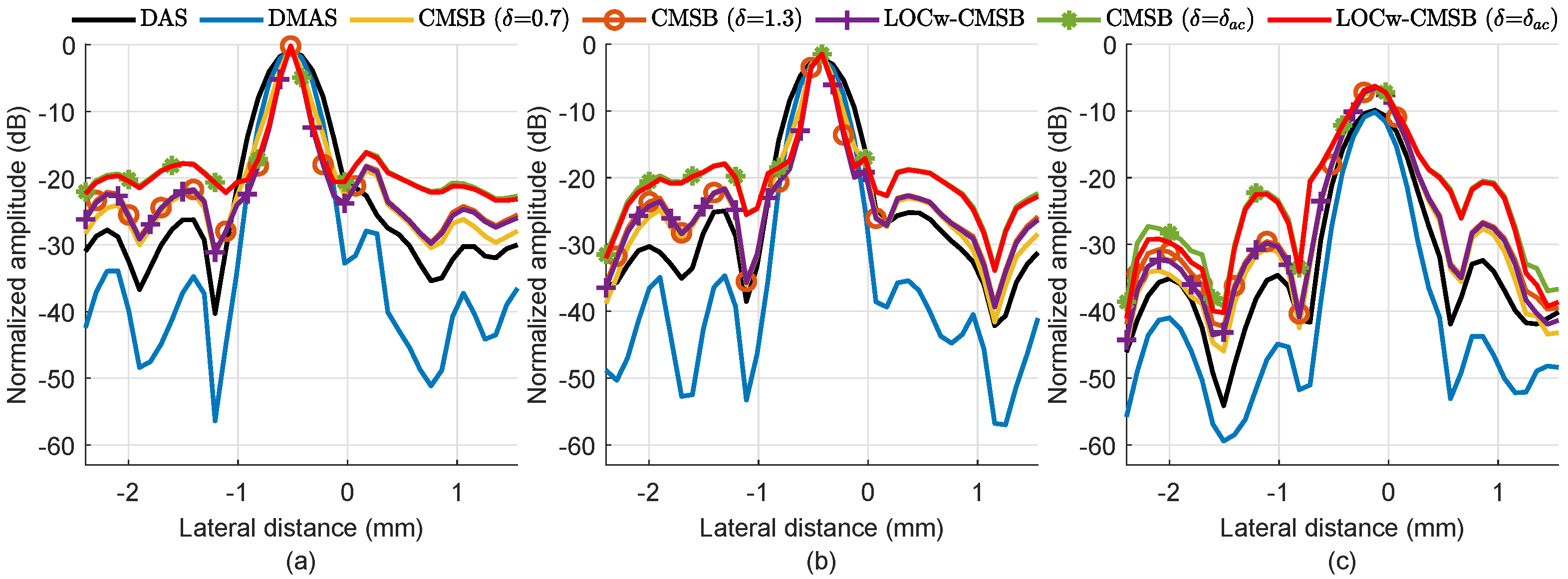
4.1. Simulation
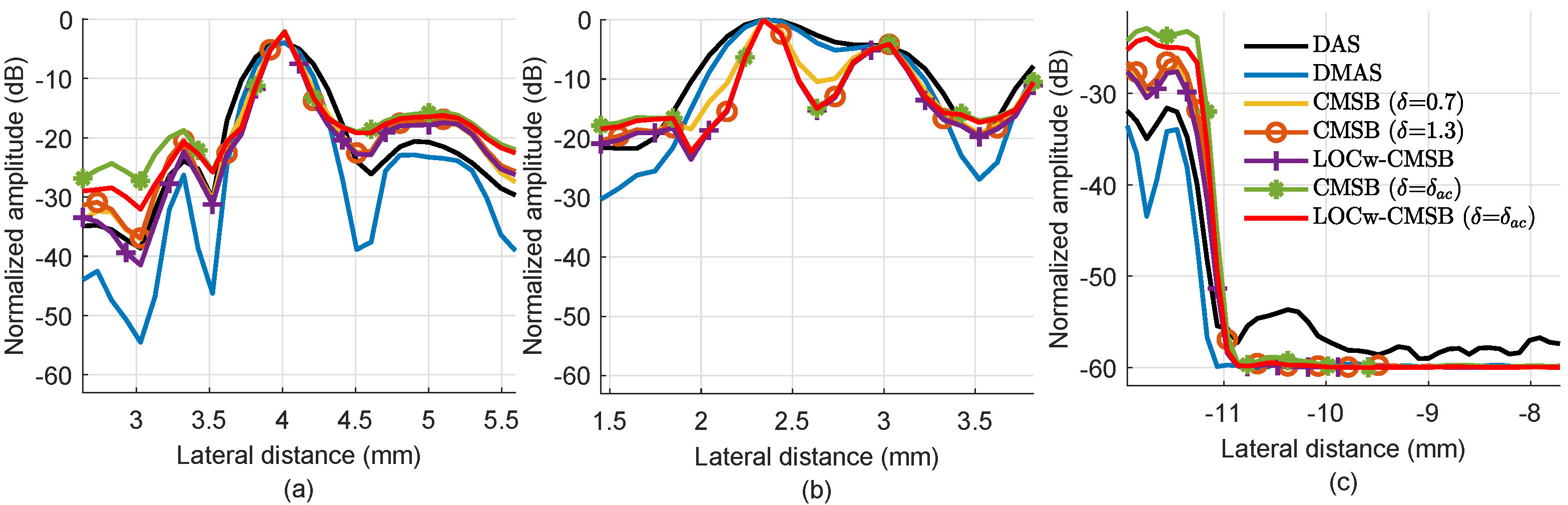
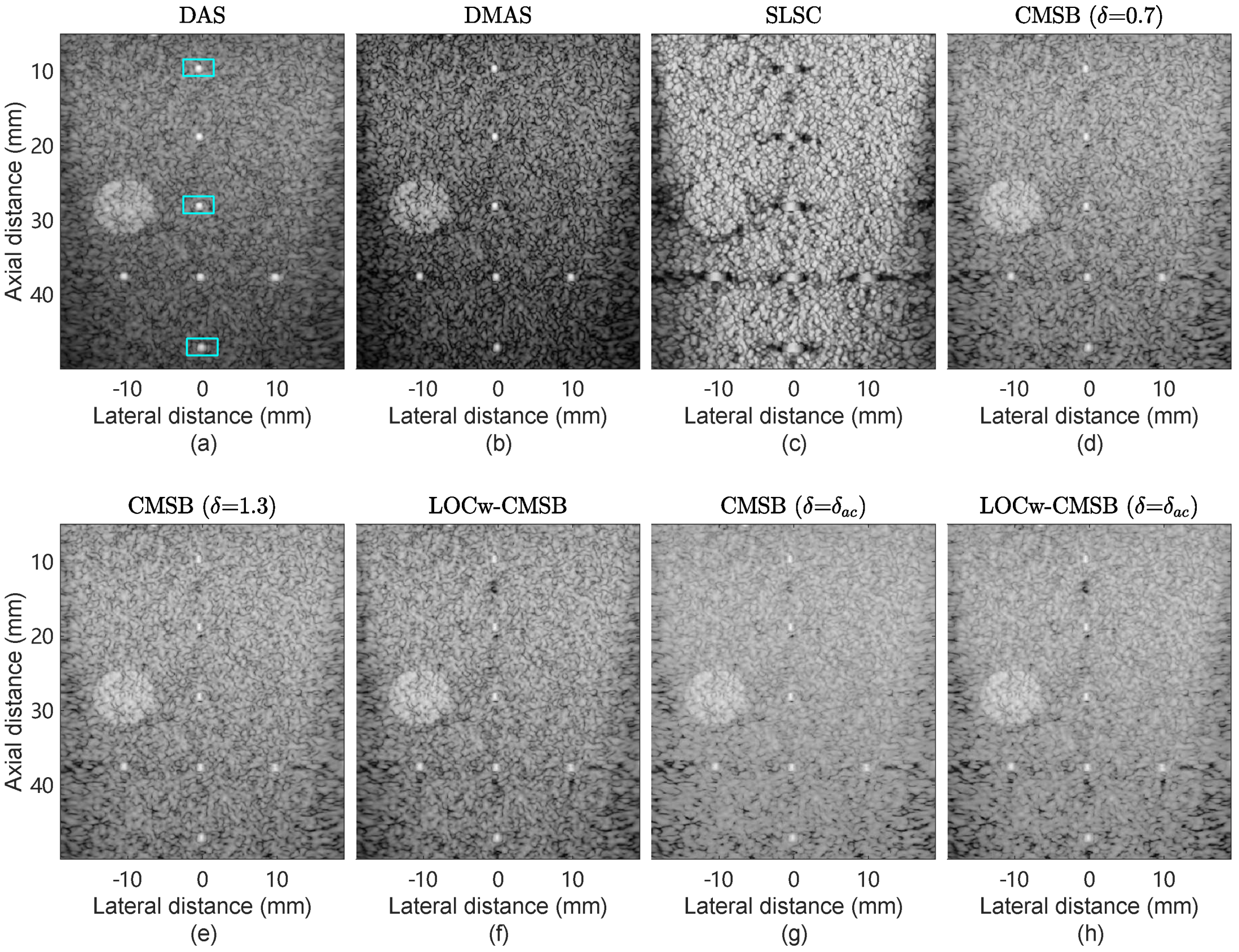
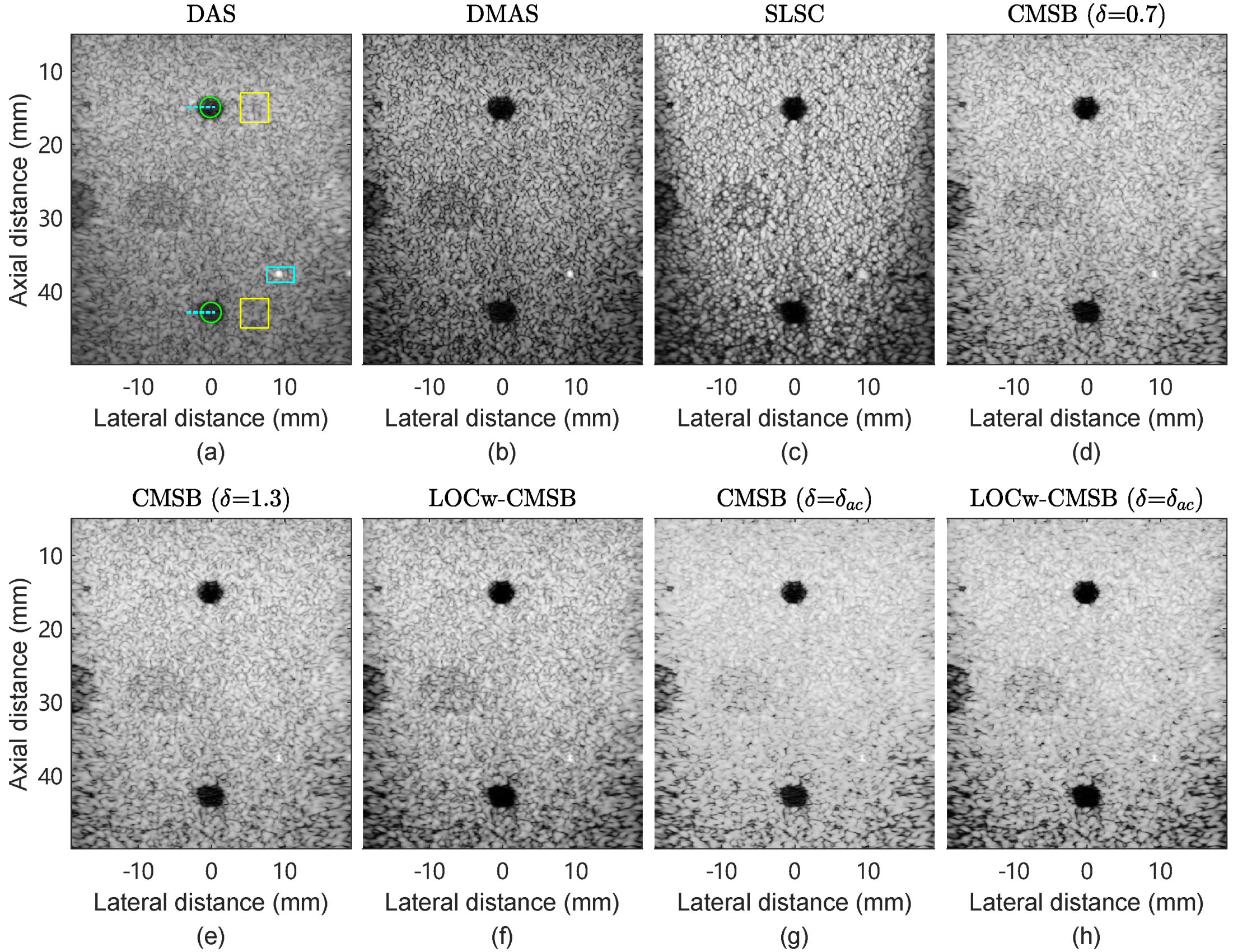
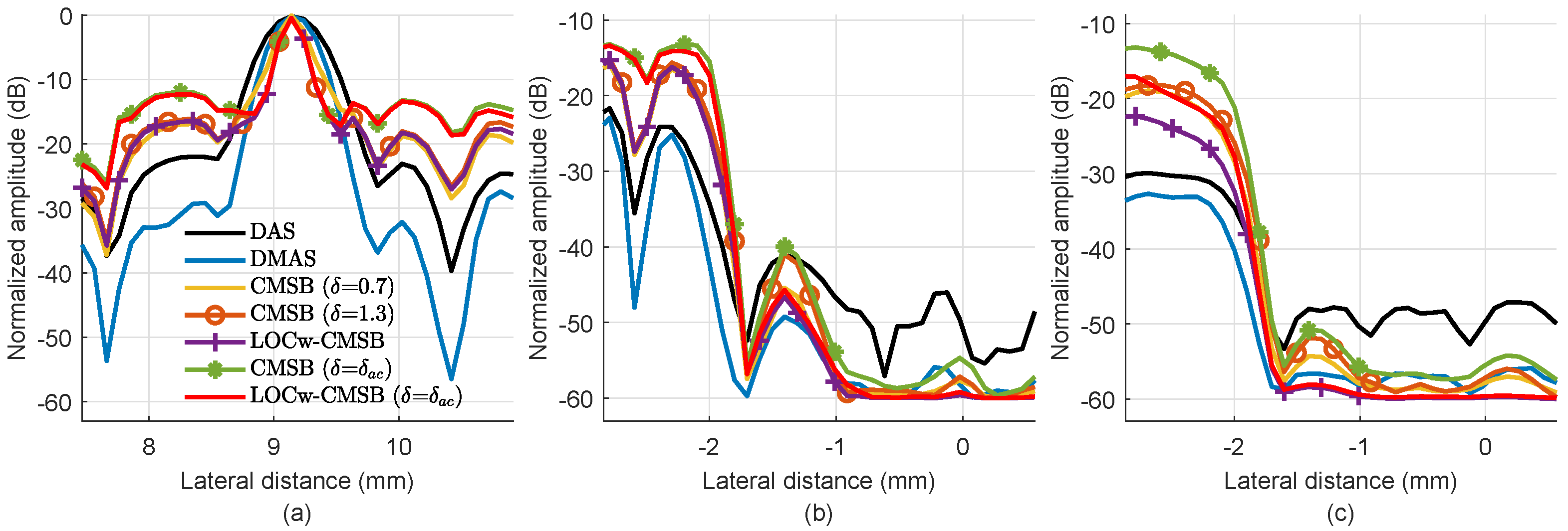
4.2. Experimental Point Phantom
4.3. Experimental Cyst Phantom
4.4. In Vivo Carotid
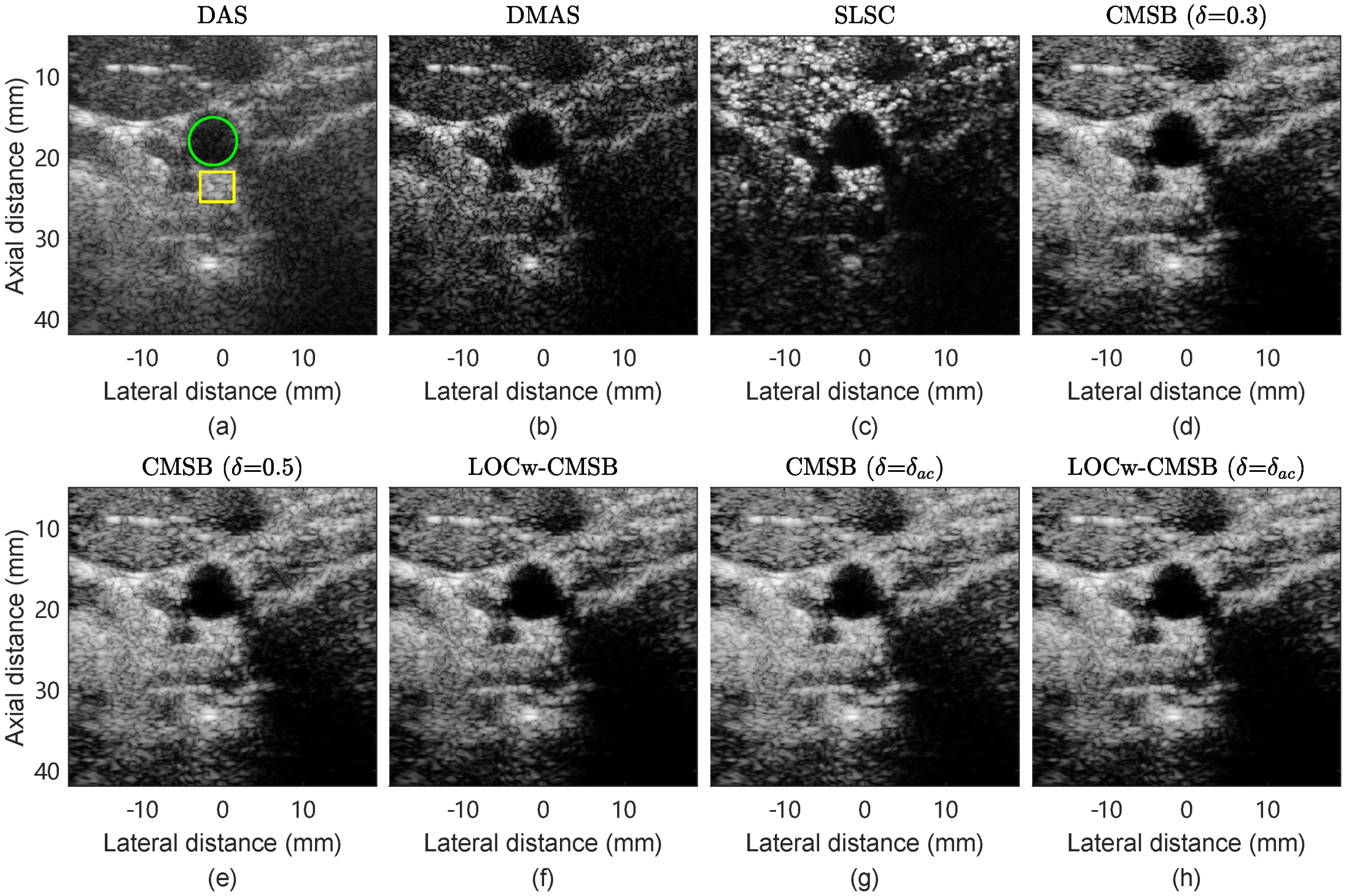
5. Discussion
5.1. Explanation of Results
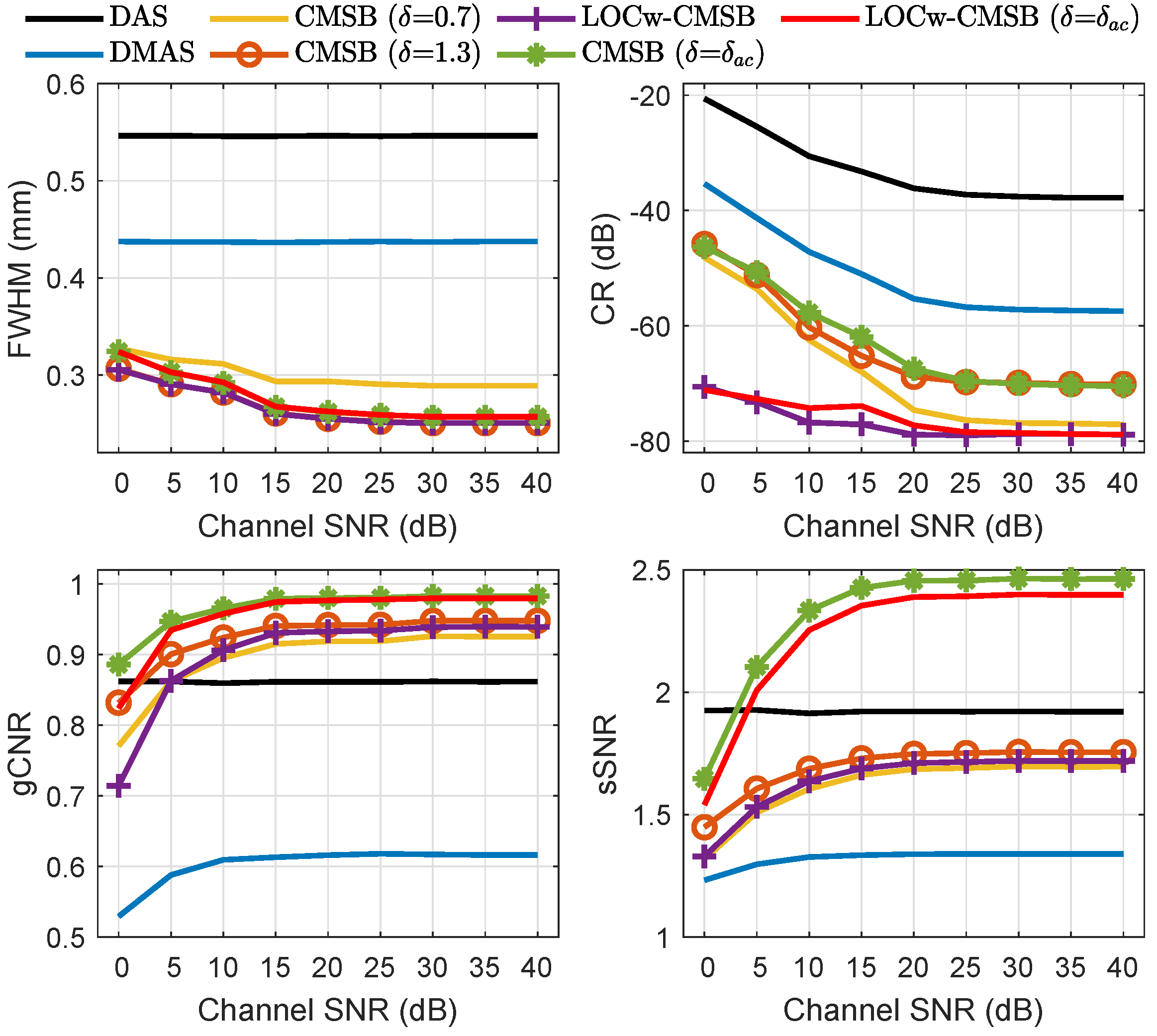
5.2. Robustness to Channel Noise
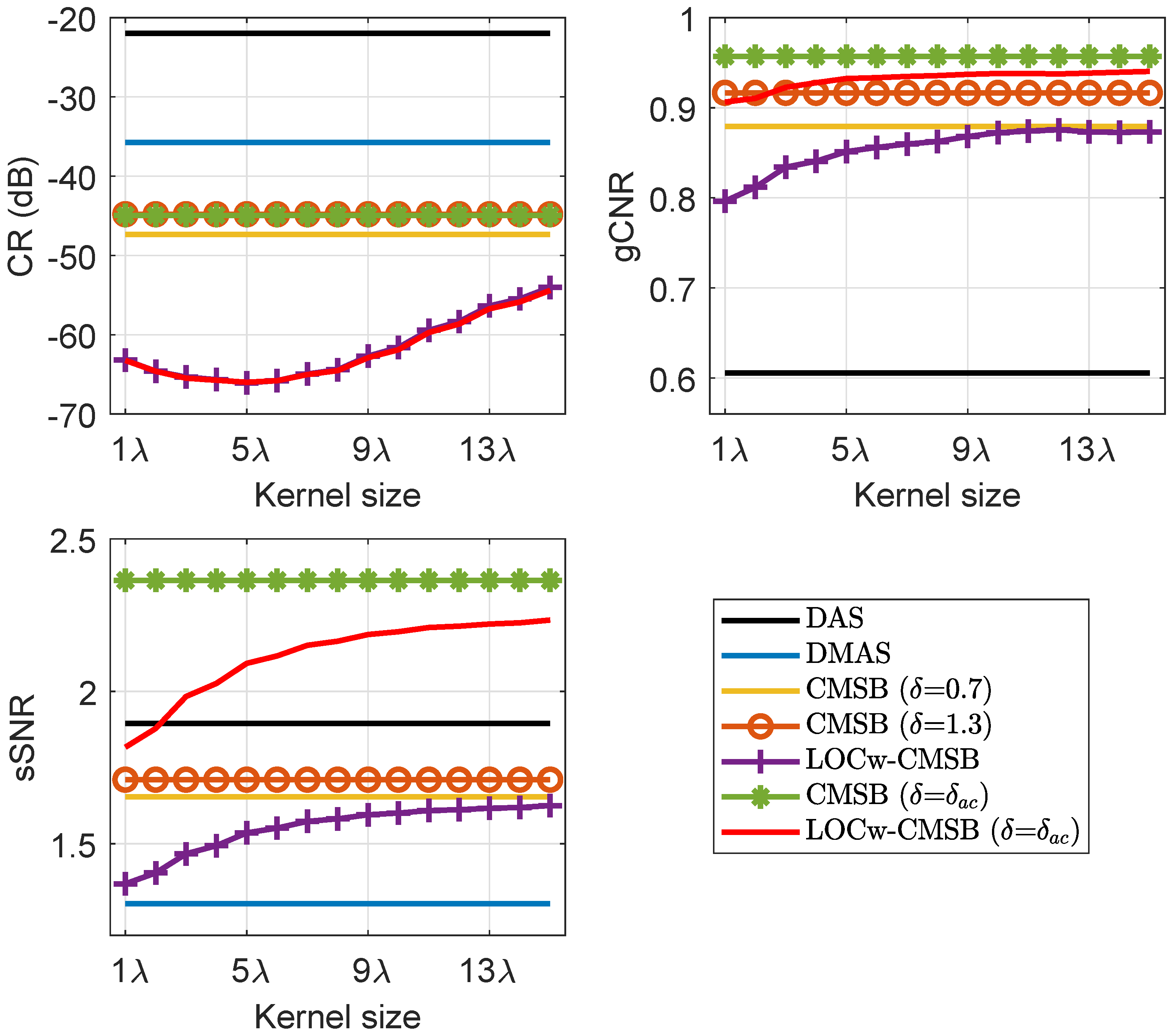
5.3. Impact of the Kernel Size
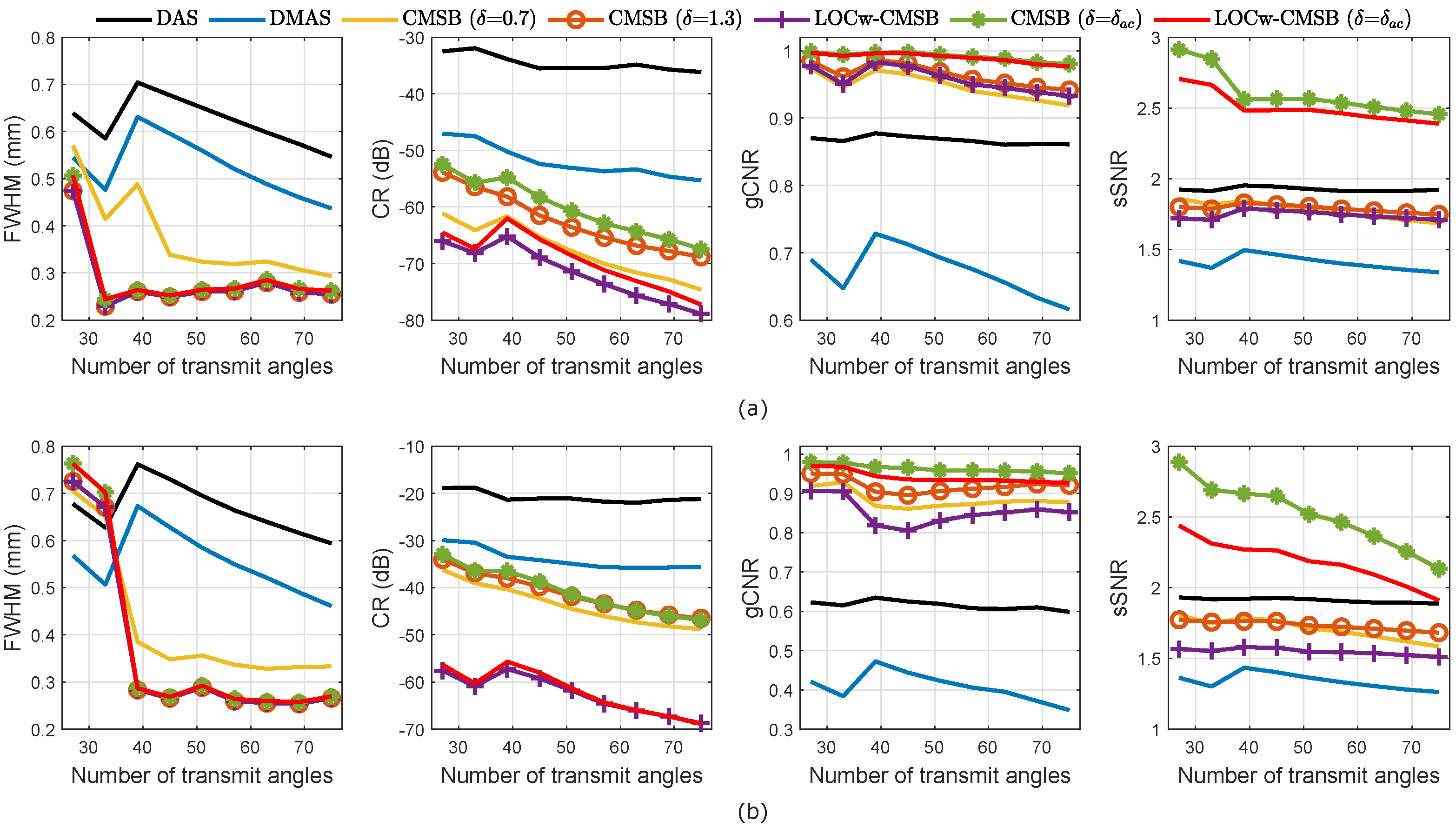
5.4. Influence of the Number of Transmit Angles
5.5. Limitations and Future Works
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| CPWC | Coherent plane-wave compounding |
| CF | Coherence factor |
| DMAS | Delay-multiply-and-sum |
| ASD | Amplitude standard deviation |
| LOC | Lag-one coherence |
References
- Tanter, M.; Fink, M. Ultrafast imaging in biomedical ultrasound. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 102–119. [Google Scholar] [CrossRef] [PubMed]
- Couture, O.; Fink, M.; Tanter, M. Ultrasound contrast plane wave imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 2676–2683. [Google Scholar] [CrossRef] [PubMed]
- Jensen, J.; Stuart, M.B.; Jensen, J.A. Optimized plane wave imaging for fast and high-quality ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2016, 63, 1922–1934. [Google Scholar] [CrossRef] [PubMed]
- Montaldo, G.; Tanter, M.; Bercoff, J.; Benech, N.; Fink, M. Coherent plane-wave compounding for very high frame rate ultrasonography and transient elastography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 489–506. [Google Scholar] [CrossRef] [PubMed]
- Hendriks, G.; Weijers, G.; Chen, C.; Hertel, M.; Lee, C.Y.; Dueppenbecker, P.; Radicke, M.; Milkowski, A.; Hansen, H.; de Korte, C.L. Ultrasound coherent plane-wave compound imaging: Image quality evaluation in phantoms and breast lesions. J. Acoust. Soc. Am. 2021, 150, A88. [Google Scholar] [CrossRef]
- Salles, S.; Liebgott, H.; Basset, O.; Cachard, C.; Vray, D.; Lavarello, R. Experimental evaluation of spectral-based quantitative ultrasound imaging using plane wave compounding. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 1824–1834. [Google Scholar] [CrossRef]
- Porée, J.; Garcia, D.; Chayer, B.; Ohayon, J.; Cloutier, G. Noninvasive Vascular Elastography With Plane Strain Incompressibility Assumption Using Ultrafast Coherent Compound Plane Wave Imaging. IEEE Trans. Med. Imaging 2015, 34, 2618–2631. [Google Scholar] [CrossRef]
- Tang, J.; Zou, B.; Li, C.; Feng, S.; Peng, H. Plane-Wave Image Reconstruction via Generative Adversarial Network and Attention Mechanism. IEEE Trans. Instrum. Meas. 2021, 70, 1–15. [Google Scholar] [CrossRef]
- Bercoff, J.; Tanter, M.; Fink, M. Supersonic shear imaging: A new technique for soft tissue elasticity mapping. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2004, 51, 396–409. [Google Scholar] [CrossRef]
- Demené, C.; Deffieux, T.; Pernot, M.; Osmanski, B.F.; Biran, V.; Gennisson, J.L.; Sieu, L.A.; Bergel, A.; Franqui, S.; Correas, J.M.; et al. Spatiotemporal Clutter Filtering of Ultrafast Ultrasound Data Highly Increases Doppler and fUltrasound Sensitivity. IEEE Trans. Med. Imaging 2015, 34, 2271–2285. [Google Scholar] [CrossRef]
- Errico, C.; Pierre, J.; Pezet, S.; Desailly, Y.; Lenkei, Z.; Couture, O.; Tanter, M. Ultrafast ultrasound localization microscopy for deep super-resolution vascular imaging. Nature 2015, 527, 499–502. [Google Scholar] [CrossRef] [PubMed]
- You, Q.; Dong, Z.; Lowerison, M.R.; Song, P. Pixel-oriented Adaptive Apodization for Planewave Imaging Based on Recovery of the Complete Data Set. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 69, 512–522. [Google Scholar] [CrossRef] [PubMed]
- Seo, C.H.; Yen, J.T. Sidelobe suppression in ultrasound imaging using dual apodization with cross-correlation. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2008, 55, 2198–2210. [Google Scholar] [PubMed]
- Capon, J. High-resolution frequency-wavenumber spectrum analysis. Proc. IEEE 1969, 57, 1408–1418. [Google Scholar] [CrossRef]
- Synnevag, J.F.; Austeng, A.; Holm, S. Adaptive beamforming applied to medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2007, 54, 1606–1613. [Google Scholar] [CrossRef]
- Synnevag, J.F.; Austeng, A.; Holm, S. Benefits of minimum-variance beamforming in medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 1868–1879. [Google Scholar] [CrossRef]
- Asl, B.M.; Mahloojifar, A. Eigenspace-based minimum variance beamforming applied to medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 2381–2390. [Google Scholar] [CrossRef]
- Lediju, M.A.; Trahey, G.E.; Byram, B.C.; Dahl, J.J. Short-lag spatial coherence of backscattered echoes: Imaging characteristics. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 1377–1388. [Google Scholar] [CrossRef]
- Matrone, G.; Savoia, A.S.; Caliano, G.; Magenes, G. The delay multiply and sum beamforming algorithm in ultrasound B-mode medical imaging. IEEE Trans. Med. Imaging 2015, 34, 940–949. [Google Scholar] [CrossRef]
- Cohen, R.; Eldar, Y.C. Sparse Convolutional Beamforming for Ultrasound Imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 2390–2406. [Google Scholar] [CrossRef]
- Lediju Bell, M.A.; Goswami, R.; Kisslo, J.A.; Dahl, J.J.; Trahey, G.E. Short-Lag Spatial Coherence Imaging of Cardiac Ultrasound Data: Initial Clinical Results. Ultrasound Med. Biol. 2013, 39, 1861–1874. [Google Scholar] [CrossRef] [PubMed]
- Wiacek, A.; Rindal, O.M.H.; Falomo, E.; Myers, K.; Fabrega-Foster, K.; Harvey, S.; Lediju Bell, M.A. Robust Short-Lag Spatial Coherence Imaging of Breast Ultrasound Data: Initial Clinical Results. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2019, 66, 527–540. [Google Scholar] [CrossRef] [PubMed]
- Moubark, A.M.; Alomari, Z.; Mohd Zaman, M.H.; Zulkifley, M.A.; Md Ali, S.H.; Nie, L.; Freear, S. Optimizing the lateral beamforming step for filtered-delay multiply and sum beamforming to improve active contour segmentation using ultrafast ultrasound imaging. Biomed. Signal Process. Control 2022, 71, 103267. [Google Scholar] [CrossRef]
- Cohen, R.; Fingerhut, N.; Varray, F.; Liebgott, H.; Eldar, Y.C. Sparse convolutional beamforming for 3-D ultrafast ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 68, 2444–2459. [Google Scholar] [CrossRef]
- Rindal, O.M.H.; Rodriguez-Molares, A.; Austeng, A. The dark region artifact in adaptive ultrasound beamforming. In Proceedings of the 2017 IEEE International Ultrasonics Symposium (IUS), Washington, DC, USA, 6–9 September 2017; pp. 1–4. [Google Scholar] [CrossRef][Green Version]
- Yan, X.; Qi, Y.; Wang, Y.; Wang, Y. Regional-lag Signed Delay Multiply and Sum Beamforming in Ultrafast Ultrasound Imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 69, 580–591. [Google Scholar] [CrossRef]
- Hollman, K.; Rigby, K.; O’donnell, M. Coherence factor of speckle from a multi-row probe. In Proceedings of the 1999 IEEE Ultrasonics Symposium. Proceedings. International Symposium (Cat. No. 99CH37027), IEE, Tahoe, NV, USA, 17–20 October 1999; Volume 2, pp. 1257–1260. [Google Scholar]
- Li, P.C.; Li, M.L. Adaptive imaging using the generalized coherence factor. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 128–141. [Google Scholar]
- Wang, Y.H.; Li, P.C. SNR-dependent coherence-based adaptive imaging for high-frame-rate ultrasonic and photoacoustic imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 1419–1432. [Google Scholar] [CrossRef]
- Xu, M.; Yang, X.; Ding, M.; Yuchi, M. Spatio-temporally smoothed coherence factor for ultrasound imaging [Correspondence]. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 182–190. [Google Scholar] [CrossRef]
- Long, W.; Bottenus, N.; Trahey, G.E. Incoherent Clutter Suppression Using Lag-One Coherence. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2020, 67, 1544–1557. [Google Scholar] [CrossRef]
- Long, W.; Bottenu, N.; Trahey, G.E. Lag-One Coherence as a Metric for Ultrasonic Image Quality. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 1768–1780. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, C.; Peng, H.; Chen, Q. An adaptive beamforming method for ultrasound imaging based on the mean-to-standard-deviation factor. Ultrasonics 2018, 90, 32–41. [Google Scholar] [CrossRef]
- Hasegawa, H.; Nagaoka, R. Converting Coherence to Signal-to-noise Ratio for Enhancement of Adaptive Ultrasound Imaging. Ultrason. Imaging 2020, 42, 27–40. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zheng, C.; Peng, H. Dynamic coherence factor based on the standard deviation for coherent plane-wave compounding. Comput. Biol. Med. 2019, 108, 249–262. [Google Scholar] [CrossRef] [PubMed]
- Bandaru, R.S.; Sornes, A.R.; Hermans, J.; Samset, E.; Dhooge, J. Delay and standard deviation beamforming to enhance specular reflections in ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2016, 63, 2057–2068. [Google Scholar] [CrossRef] [PubMed]
- Paridar, R.; Mozaffarzadeh, M.; Periyasamy, V.; Basij, M.; Mehrmohammadi, M.; Pramanik, M.; Orooji, M. Validation of delay-multiply-and-standard-deviation weighting factor for improved photoacoustic imaging of sentinel lymph node. J. Biophotonics 2019, 12, e201800292. [Google Scholar] [CrossRef] [PubMed]
- Lan, Z.; Zheng, C.; Wang, Y.; Peng, H.; Qiao, H. Adaptive threshold for eigenspace-based minimum variance beamformer for dark region artifacts elimination. IEEE Trans. Instrum. Meas. 2021, 70, 1–16. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, C.; Liu, M.; Peng, H. Covariance Matrix-based Statistical Beamforming for Medical Ultrasound Imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 69, 208–221. [Google Scholar] [CrossRef]
- Paul, S.; Mandal, S.; Singh, M.S. Noise Adaptive Beamforming for Linear Array Photoacoustic Imaging. IEEE Trans. Instrum. Meas. 2021, 70, 1–11. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, C.; Peng, H.; Zhang, C. Coherent plane-wave compounding based on normalized autocorrelation factor. IEEE Access 2018, 6, 36927–36938. [Google Scholar] [CrossRef]
- Long, J.; Long, W.; Bottenus, N.; Pinton, G.F.; Trahey, G.E. Implications of Lag-One Coherence on Real-Time Adaptive Frequency Selection. In Proceedings of the 2018 IEEE International Ultrasonics Symposium (IUS), Kobe, Japan, 22–25 October 2018; pp. 1–9. [Google Scholar] [CrossRef]
- Wiacek, A.; Oluyemi, E.; Myers, K.; Ambinder, E.; Bell, M.A.L. Quantifying the impact of breast density on the lag-one coherence of hypoechoic masses. In Proceedings of the 2021 IEEE International Ultrasonics Symposium (IUS), Xi’an, China, 11–16 September 2021; pp. 1–4. [Google Scholar] [CrossRef]
- Yu, M.; Li, Y.; Ma, T.; Shung, K.K.; Zhou, Q. Intravascular Ultrasound Imaging With Virtual Source Synthetic Aperture Focusing and Coherence Factor Weighting. IEEE Trans. Med. Imaging 2017, 36, 2171–2178. [Google Scholar] [CrossRef]
- Kang, S.; Lee, J.; Chang, J.H. Effectiveness of synthetic aperture focusing and coherence factor weighting for intravascular ultrasound imaging. Ultrasonics 2021, 113, 106364. [Google Scholar] [CrossRef] [PubMed]
- Jensen, J.A.; Svendsen, N.B. Calculation of pressure fields from arbitrarily shaped, apodized, and excited ultrasound transducers. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1992, 39, 262–267. [Google Scholar] [CrossRef] [PubMed]
- Jensen, J.A. FIELD: A Program for Simulating Ultrasound Systems. In Proceedings of the 10th Nordic-Baltic Conference on Biomedical Imaging, Tampere, Finland, 9–13 1996; Volume 34, pp. 351–353. [Google Scholar]
- Liebgott, H.; Rodriguez-Molares, A.; Cervenansky, F.; Jensen, J.; Bernard, O. Plane-Wave Imaging Challenge in Medical Ultrasound. In Proceedings of the 2016 IEEE International Ultrasonics Symposium (IUS), Tours, France, 18–21 September 2016; pp. 1–4. [Google Scholar] [CrossRef]
- Rodriguez-Molares, A.; Rindal, O.M.H.; D’hooge, J.; Måsøy, S.; Austeng, A.; Bell, M.A.L.; Torp, H. The generalized contrast-to-noise ratio: A formal definition for lesion detectability. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2019, 67, 745–759. [Google Scholar] [CrossRef] [PubMed]
- Hoel Rindal, O.M.; Austeng, A.; Rodriguez-Molares, A. Resolution Measured as Separability Compared to Full Width Half Maximum for Adaptive Beamformers. In Proceedings of the 2020 IEEE International Ultrasonics Symposium (IUS), Las Vegas, NV, USA, 7–11 September 2020; pp. 1–4. [Google Scholar] [CrossRef]
- Shen, M.; Zhang, Q.; Li, D.; Yang, J.; Li, B. Adaptive Sparse Representation Beamformer for High-Frame-Rate Ultrasound Imaging Instrument. IEEE Trans. Instrum. Meas. 2012, 61, 1323–1333. [Google Scholar] [CrossRef]
- Song, P.; Urban, M.W.; Manduca, A.; Greenleaf, J.F.; Chen, S. Coded excitation plane wave imaging for shear wave motion detection. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 1356–1372. [Google Scholar] [CrossRef] [PubMed]
- Afrakhteh, S.; Behnam, H. Coherent Plane Wave Compounding Combined With Tensor Completion Applied for Ultrafast Imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 68, 3094–3103. [Google Scholar] [CrossRef]


| Parameters | Value |
|---|---|
| Number of elements | 128 |
| Element pitch | 0.30 mm |
| Element width | 0.27 mm |
| Center frequency | 5.208 MHz |
| Sampling frequency | 20.832 MHz |
| Pulse bandwidth | 67% |
| Excitation | 2.5 cycles |
| Number of transmit angles | 75 |
| Transmit angles | [, ] |
| Angle spacing |
| Methods | FWHM (mm) | CR (dB) | gCNR | sSNR |
|---|---|---|---|---|
| DAS | 0.55 | −36.2 | 0.86 | 1.92 |
| DMAS | 0.44 | −55.3 | 0.62 | 1.34 |
| SLSC | - | −27.1 | 0.98 | 3.35 |
| CMSB ( = 0.7) | 0.29 | −74.6 | 0.92 | 1.69 |
| CMSB ( = 1.3) | 0.26 | −68.8 | 0.94 | 1.75 |
| LOCw-CMSB | 0.26 | −78.9 | 0.93 | 1.71 |
| CMSB ( = ) | 0.26 | −67.4 | 0.98 | 2.46 |
| LOCw-CMSB ( = ) | 0.26 | −77.3 | 0.98 | 2.39 |
| Methods | FWHM (mm) at Depths of | ||
|---|---|---|---|
| 9.6 mm | 28.1 mm | 47 mm | |
| DAS | 0.55 | 0.56 | 0.57 |
| DMAS | 0.44 | 0.44 | 0.46 |
| CMSB ( = 0.7) | 0.29 | 0.32 | 0.51 |
| CMSB ( = 1.3) | 0.23 | 0.26 | 0.49 |
| LOCw-CMSB | 0.23 | 0.26 | 0.49 |
| CMSB ( = ) | 0.23 | 0.27 | 0.56 |
| LOCw-CMSB ( = ) | 0.23 | 0.27 | 0.56 |
| Methods | FWHM (mm) | Cyst at 15 mm Depth | Cyst at 42.9 mm Depth | ||||
|---|---|---|---|---|---|---|---|
| CR (dB) | gCNR | sSNR | CR (dB) | gCNR | sSNR | ||
| DAS | 0.59 | −32.7 | 0.98 | 1.99 | −22.0 | 0.61 | 1.90 |
| DMAS | 0.46 | −50.3 | 0.86 | 1.48 | −35.8 | 0.39 | 1.30 |
| SLSC | - | −29.0 | 1.00 | 4.97 | −32.3 | 1.00 | 2.83 |
| CMSB ( = 0.7) | 0.33 | −62.5 | 1.00 | 2.01 | −47.3 | 0.88 | 1.65 |
| CMSB ( = 1.3) | 0.27 | −59.5 | 1.00 | 2.01 | −44.8 | 0.92 | 1.71 |
| LOCw-CMSB | 0.27 | −74.1 | 1.00 | 1.98 | −66.1 | 0.85 | 1.54 |
| CMSB ( = ) | 0.27 | −56.8 | 1.00 | 2.95 | −44.9 | 0.96 | 2.36 |
| LOCw-CMSB ( = ) | 0.27 | −72.0 | 1.00 | 2.86 | −66.0 | 0.93 | 2.09 |
| Methods | CR (dB) | gCNR | sSNR |
|---|---|---|---|
| DAS | −32.2 | 0.54 | 1.50 |
| DMAS | −48.9 | 0.35 | 1.00 |
| SLSC | −24.8 | 0.91 | 2.06 |
| CMSB ( = 0.3) | −63.5 | 0.79 | 1.43 |
| CMSB ( = 0.5) | −59.8 | 0.83 | 1.50 |
| LOCw-CMSB | −69.2 | 0.81 | 1.46 |
| CMSB ( = ) | −59.7 | 0.89 | 1.76 |
| LOCw-CMSB ( = ) | −68.6 | 0.88 | 1.70 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zheng, C.; Peng, H.; Wang, Y. High-Quality Coherent Plane-Wave Compounding Using Enhanced Covariance-Matrix-Based Statistical Beamforming. Appl. Sci. 2022, 12, 10973. https://doi.org/10.3390/app122110973
Wang Y, Zheng C, Peng H, Wang Y. High-Quality Coherent Plane-Wave Compounding Using Enhanced Covariance-Matrix-Based Statistical Beamforming. Applied Sciences. 2022; 12(21):10973. https://doi.org/10.3390/app122110973
Chicago/Turabian StyleWang, Yuanguo, Chichao Zheng, Hu Peng, and Yadan Wang. 2022. "High-Quality Coherent Plane-Wave Compounding Using Enhanced Covariance-Matrix-Based Statistical Beamforming" Applied Sciences 12, no. 21: 10973. https://doi.org/10.3390/app122110973
APA StyleWang, Y., Zheng, C., Peng, H., & Wang, Y. (2022). High-Quality Coherent Plane-Wave Compounding Using Enhanced Covariance-Matrix-Based Statistical Beamforming. Applied Sciences, 12(21), 10973. https://doi.org/10.3390/app122110973





