Water Retention Curve of Biocemented Sands Using MIP Results
Abstract
Featured Application
Abstract
1. Introduction
2. Model Proposed
3. Materials and Methods
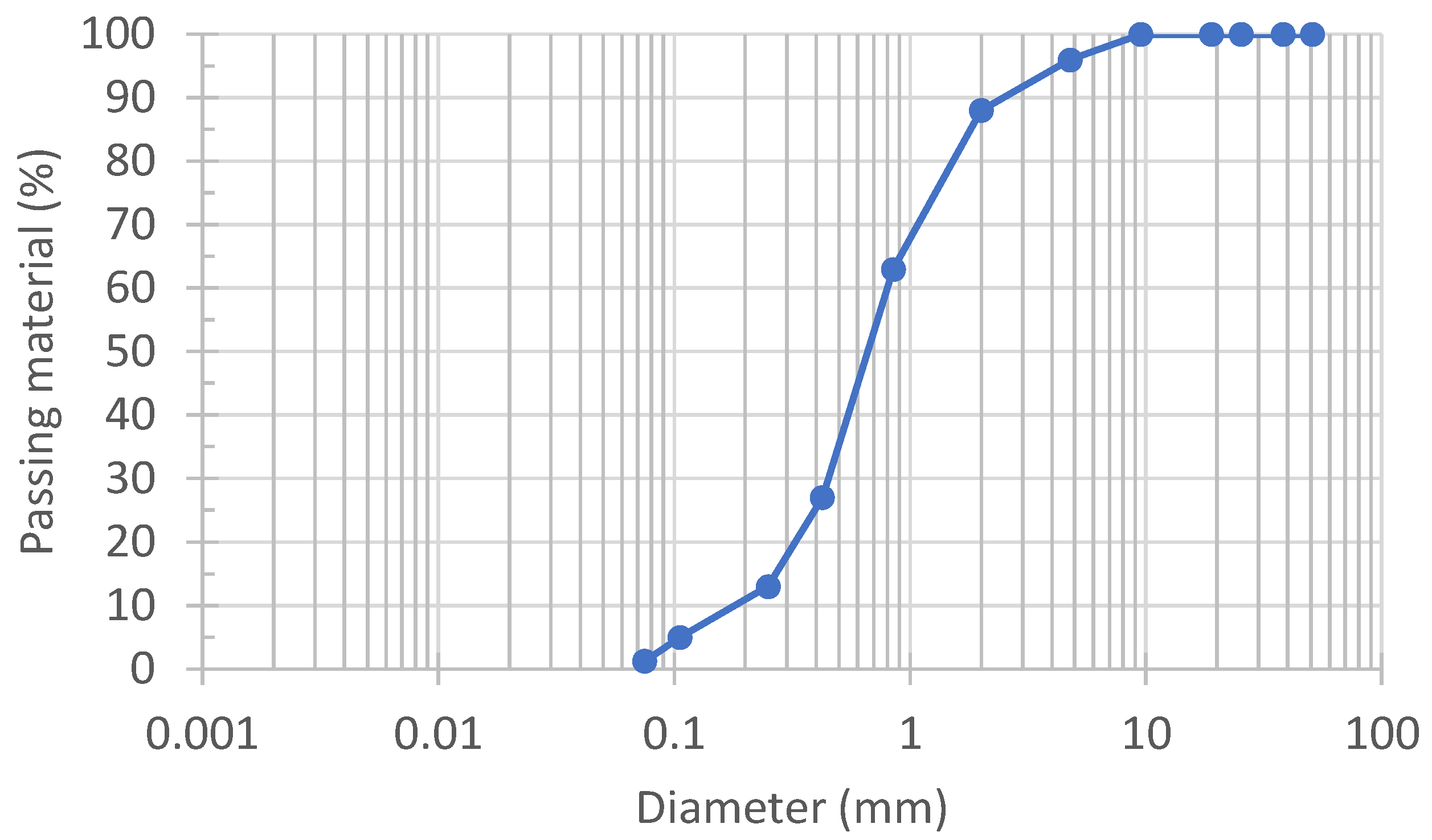
3.1. Soil and Soil Samples
3.2. Bacteria and Feeding Solution
3.3. Biocementation Treatment
3.4. Tests Performed
3.4.1. Oedometer Tests
3.4.2. Saturated Permeability
3.4.3. Tests to Confirm the Presence of Biocement
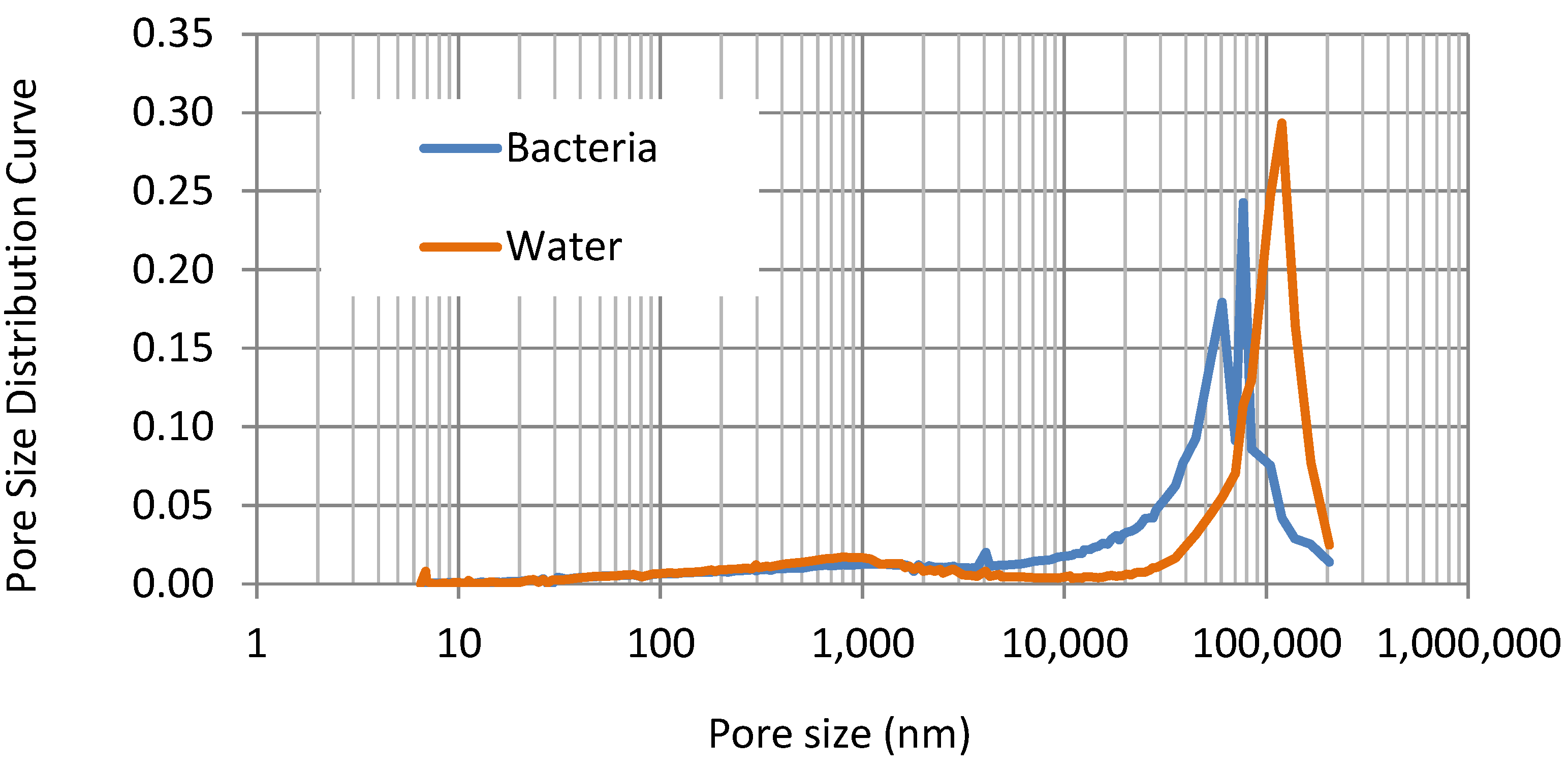
3.4.4. Mercury Intrusion Porosimetry Tests
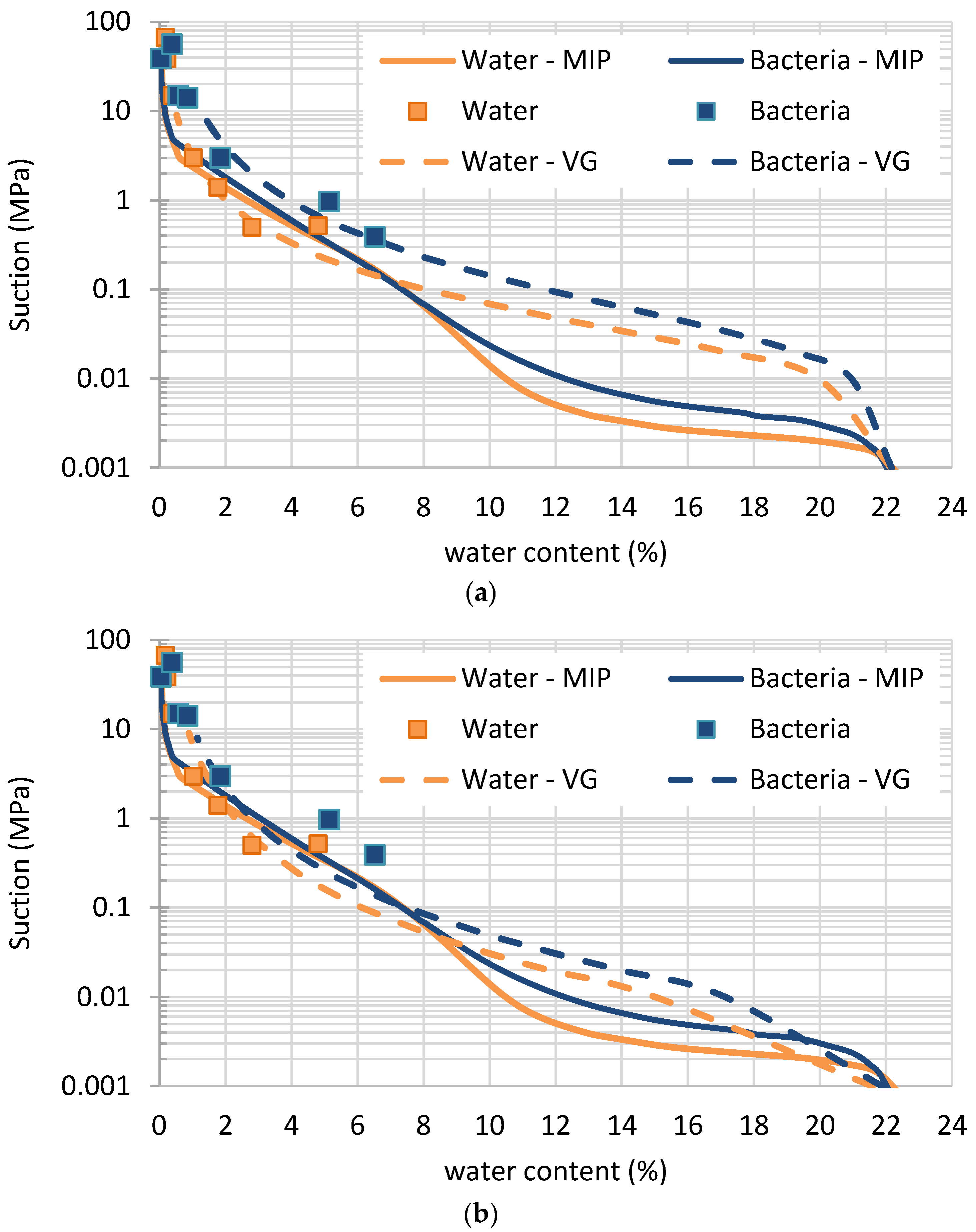
3.4.5. Water Retention Curve
4. Results and Discussion
4.1. Oedometer Tests
4.2. Saturated Permeability
4.3. Presence of Biocement
4.4. Mercury Intrusion Porosimetry Tests and Water Retention Curve
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Inanov, V.; Chu, J.; Stabnikov, V. Chapter 2 basic, construction microbial biotechnology. In Biotechnologies and Biomimetics for Civil Engineering; Pacheco Torgal, F., Labrincha, J.A., Diamanti, M.V., Yu, C.-P., Lee, H.K., Eds.; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Al Qabany, A.; Soga, K.; Santamarina, C. Factors Affecting Efficiency of Microbially Induced Calcite Precipitation. J. Geotech. Geoenviron. Eng. 2012, 138, 992–1001. [Google Scholar] [CrossRef]
- Terzis, D.; Bernier-Latmani, R.; Laloui, L. Fabric characteristics and mechanical response of bio-improved sand to various treatment conditions. Geotech. Lett. 2016, 6, 50–57. [Google Scholar] [CrossRef]
- DeJong, J.T.; Fritzges, M.B.; Nüsslein, K. Microbially Induced Cementation to Control Sand Response to Undrained Shear. J. Geotech. Geoenviron. Eng. 2006, 132, 1381–1392. [Google Scholar] [CrossRef]
- Salifu, E.; MacLachlan, E.; Iyer, K.R.; Knapp, C.W.; Tarantino, A. Application of microbially induced calcite precipitation in erosion mitigation and stabilisation of sandy soil foreshore slopes: A preliminary investigation. Eng. Geol. 2016, 201, 96–105. [Google Scholar] [CrossRef]
- Montoya, B.M.; DeJong, J.; Boulanger, R. Dynamic response of liquefiable sand improved by microbial-induced calcite precipitation. Geotechnique 2013, 63, 302–312. [Google Scholar] [CrossRef]
- Chek, A.; Crowley, R.; Ellis, T.N.; Durnin, M.; Wingender, B. Evaluation of Factors Affecting Erodibility Improvement for MICP-Treated Beach Sand. J. Geotech. Geoenviron. Eng. 2021, 147, 04021001. [Google Scholar] [CrossRef]
- Chu, J.; Ivanov, V.; Stabnikov, V.; Li, B. Microbial method for construction of an aquaculture pond in sand. Géotechnique 2013, 63, 871–875. [Google Scholar] [CrossRef]
- Sisakht, B.N.; Nikooee, E.; Habibagahi, G.; Niazi, A. Stabilisation of collapsible soils: A biological technique. In Proceedings of the XVI ECSMGE Geotechnical Engineering for Infrastructure and Development, Edinburgh, UK, 12–17 September 2015; pp. 2829–2834. [Google Scholar]
- Sun, X.; Miao, L.; Chen, R. The application of bio-cementation for improvement in collapsibility of loess. Int. J. Environ. Sci. Technol. 2020, 18, 2607–2618. [Google Scholar] [CrossRef]
- Phadnis, H.S.; Santamarina, J.C. Bacteria in sediments: Pore size effects. Geotech. Lett. 2011, 1, 91–93. [Google Scholar] [CrossRef]
- Saffari, R.; Nikooee, E.; Habibagahi, G.; van Genuchten, M.T. Effects of Biological Stabilization on the Water Retention Properties of Unsaturated Soils. J. Geotech. Geoenv. Eng. 2019, 145, 04019028. [Google Scholar]
- Saffari, R.; Nikooee, E.; Habibagahi, G. The effect of microbial calcite precipitation on the retention properties of unsaturated fine-grained soils: Discussion of the governing factors. E3S Web Conf. 2020, 195, 05009. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Rahardjo, H. Soil Mechanics for Unsaturated Soils; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Romero, E.; Gens, A.; Lloret, A. Water permeability, water retention and microstructure of unsaturated compacted Boom clay. Eng. Geol. 1999, 54, 117–127. [Google Scholar] [CrossRef]
- Garcia-Bengochea, I.; Altschaeffl, A.G.; Lovell, C.W. Pore Distribution and Permeability of Silty Clays. J. Geotech. Eng. Div. 1979, 105, 839–856. [Google Scholar] [CrossRef]
- van Genuchten, M.T. A Closed-form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Lapierre, C.; Leroueil, S.; Locat, J. Mercury intrusion and permeability of Louisville clay. Can. Geotech. J. 1990, 27, 761–773. [Google Scholar] [CrossRef]
- Romero, E.; Simms, P.H. Microstructure Investigation in Unsaturated Soils: A Review with Special Attention to Contribution of Mercury Intrusion Porosimetry and Environmental Scanning Electron Microscopy. Geotech. Geol. Eng. 2008, 26, 705–727. [Google Scholar] [CrossRef]
- Wang, M.; Pande, G.; Pietruszczak, S.; Zeng, Z. Determination of strain-dependent soil water retention characteristics from gradation curve. J. Rock Mech. Geotech. Eng. 2020, 12, 1356–1360. [Google Scholar] [CrossRef]
- Juang, C.; Holtz, R. Fabric, pore size distribution and permeability for sandy soils. J. Geotech. Eng. 1986, 112, 855–868. [Google Scholar] [CrossRef]
- Delage, P.; Lefebvre, G. Study of the structure of a sensitive Champlain clay and of its evolution during consolidation. Can. Geotech. J. 1984, 21, 21–35. [Google Scholar] [CrossRef]
- Hu, R.; Chen, Y.F.; Liu, H.H.; Zhou, C.B. A water retention curve and unsaturated hydraulic conductivity model for deformable soils: Consideration of the change in pore-size distribution. Géotechnique 2013, 63, 1389–1405. [Google Scholar] [CrossRef]
- Romero, E.; Vecchia, G.D.; Jommi, C. An insight into the water retention properties of compacted clayey soils. Géotechnique 2011, 61, 313–328. [Google Scholar] [CrossRef]
- Romero, E.; Vaunat, J. Retention curves of deformable clays. In Experimental Evidence and Theoretical Approaches in Unsaturated Soils, Proceedings of the Workshop on Unsaturated Soils, Trento, Italy, 10–12 April 2000; Tarantino, A., Mancuso, C., Balkema Rotterdam, A.A., Eds.; Taylor & Francis: New York, NY, USA, 2000; pp. 91–108. [Google Scholar]
- Gallipoli, D.; Gens, A.; Sharma, R.; Vaunat, J. An elasto-plastic model for soil incorporating the effects of suction and degree of saturation on mechanical behavior. Géotechnique 2003, 53, 123–135. [Google Scholar] [CrossRef]
- Maranha, J.R.; Pereira, C.; Cardoso, R. Effective stress in unsaturated soils: Lessons from capillarity in regular sphere arrangements. Geomech. Energy Environ. 2022, 100341. [Google Scholar] [CrossRef]
- Prapaharan, S.; Altschaeffl, A.G.; Dempsey, B. Moisture curve of compacted clay: Mercury intrusion method. J. Geotech. Eng. 1985, 111, 1139–1143. [Google Scholar] [CrossRef]
- Aubertin, M.; Mbonimpa, M.; Bussière, B.; Chapuis, R.P. A model to predict the water retention curve from basic geotechnical properties. Can. Geotech. J. 2003, 40, 1104–1122. [Google Scholar] [CrossRef]
- Zhang, L.M.; Li, X. Microporosity Structure of Coarse Granular Soils. J. Geotech. Geoenviron. Eng. 2010, 136, 1425–1436. [Google Scholar] [CrossRef]
- Wang, J.-P.; Hu, N.; François, B.; Lambert, P. Estimating water retention curves and strength properties of unsaturated sandy soils from basic soil gradation parameters. Water Resour. Res. 2017, 53, 6069–6088. [Google Scholar] [CrossRef]
- Li, P.; Vanapalli, S.; Li, T. Review of collapse triggering mechanism of collapsible soils due to wetting. J. Rock Mech. Geotech. Eng. 2016, 8, 256–274. [Google Scholar] [CrossRef]
- Cheng, Q.; Ng, C.W.W.; Zhou, C.; Tang, C.S. A new water retention model that considers pore non-uniformity and evolution of pore size distribution. Bull. Eng. Geol. Environ. 2019, 78, 5055–5065. [Google Scholar] [CrossRef]
- Sun, W.-J.; Cui, Y.-J. Determining the soil-water retention curve using mercury intrusion porosimetry test in consideration of soil volume change. J. Rock Mech. Geotech. Eng. 2020, 12, 1070–1079. [Google Scholar] [CrossRef]
- Penumadu, D.; Dean, J. Compressibility effect in evaluating the pore-size distribution of kaolin clay using mercury intrusion porosimetry. Can. Geotech. J. 2000, 37, 393–405. [Google Scholar] [CrossRef]
- Simms, P.H.; Yanful, E.K. Predicting soil–water characteristic curves of compacted plastic soils from measured pore-size distributions. Géotechnique 2002, 52, 269–278. [Google Scholar] [CrossRef]
- Vieira, J. Analysis of the Hydromechanical Behavior of a Real Soil Treated by BIO-Cementation. Master’s Thesis, Instituto Superior Técnico, University of Lisbon, Lisboa, Portugal, 2022. (In Portuguese). [Google Scholar]
- ASTM D5333-03; Standard Test Method for Measurement of Collapse Potential of Soils. ASTM International: West Conshohocken, PA, USA, 2003.
- Alonso, E.E.; Gens, A.; Josa, A. A constitutive model for partially saturated soils. Géotechnique 1990, 40, 405–430. [Google Scholar] [CrossRef]
- Leong, E.-C.; Tripathy, S.; Rahardjo, H. Total suction measurement of unsaturated soils with a device using the chilled-mirror dew-point technique. Géotechnique 2003, 53, 173–182. [Google Scholar] [CrossRef]
- Cardoso, R.; Lima, A.; Romero, E.; Ferrari, A. A comparative study of soil suction measurement using two different high-range psychrometers. In Proceedings of the 2nd International Conference, Mechanics of Unsaturated Soils, Weimar, Germany, 7–9 March 2007; Springer: Berlin, Germany, 2007. [Google Scholar]
- Romero, E. Controlled-suction techniques. In Proceedings of the 4th National Brazilian Symposium on Unsaturated Soils, Rio de Janeiro, Brazil, 25–28 July 2011; Gehling, W.Y., Schnaid, F., Eds.; ABMS: Rio de Janeiro, Brazil, 2001; pp. 535–542. [Google Scholar]
- Fredlund, D.; Xing, A. Equations for the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 521–532. [Google Scholar] [CrossRef]
- Mitchell, J.; Soga, K. Fundamentals of Soil Behavior, 3rd ed.; John Wiley and Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Haeri, S.M.; Garakani, A.A.; Khosravi, A.; Meehan, C.L. Assessing the Hydro-Mechanical Behavior of Collapsible Soils Using a Modified Triaxial Test Device. Geotech. Test. J. 2013, 37, 190–204. [Google Scholar] [CrossRef]
- Vandanapu, R.; Omer, J.R.; Attom, M.F. Geotechnical case studies: Emphasis on collapsible soil cases. Proc. Inst. Civ. Eng. Forensic Eng. 2016, 169, 103–110. [Google Scholar] [CrossRef]
- Lee, M.L.; Ng, W.S.; Tanaka, Y. Stress-deformation and compressibility responses of bio-mediated residual soils. Ecol. Eng. 2013, 60, 142–149. [Google Scholar] [CrossRef]
- Cardoso, R.; Borges, I.; Pires, I. Modelling oedometer tests on biocemented soils considering bonds presence. In Congress on Numerical Methods in Engineering; CMN2019: Guimarães, Portugal, 2019. [Google Scholar]
- Harran, R.; Terzis, D.; Laloui, L. Characterizing the Deformation Evolution with Stress and Time of Biocemented Sands. J. Geotech. Geoenviron. Eng. 2022, 148, 04022074. [Google Scholar] [CrossRef]
- Leroueil, S.; Vaughan, P.R. The general and congruent effects of structure in natural soils and weak rocks. Géotechnique 1990, 40, 467–488. [Google Scholar] [CrossRef]
- Cardoso, R.; Pedreira, R.; Duarte, S.O.; Monteiro, G.A. About calcium carbonate precipitation on sand biocementation. Eng. Geol. 2020, 271, 105612. [Google Scholar] [CrossRef]




| Sample | δεvol (%) | Cc | Cs | κ | λ(s) | σ′y (kPa) | |
|---|---|---|---|---|---|---|---|
| Untreated soil—water (initial suction s = 67.5 MPa) | Saturated | -- | 0.137 | 0.014 | 0.034 | 0.316 | 80 |
| Unsaturated | 3.0 | 0.034 | 0.015 | 0.035 | 0.114 | 100 | |
| Biocemented soil—bacteria (initial suction s = 62.7 MPa) | Saturated | -- | 0.162 | 0.013 | 0.031 | 0.372 | 100 |
| Unsaturated | 0.2 | 0.109 | 0.016 | 0.037 | 0.285 | 120 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cardoso, R.; Vieira, J.; Borges, I. Water Retention Curve of Biocemented Sands Using MIP Results. Appl. Sci. 2022, 12, 10447. https://doi.org/10.3390/app122010447
Cardoso R, Vieira J, Borges I. Water Retention Curve of Biocemented Sands Using MIP Results. Applied Sciences. 2022; 12(20):10447. https://doi.org/10.3390/app122010447
Chicago/Turabian StyleCardoso, Rafaela, Joana Vieira, and Inês Borges. 2022. "Water Retention Curve of Biocemented Sands Using MIP Results" Applied Sciences 12, no. 20: 10447. https://doi.org/10.3390/app122010447
APA StyleCardoso, R., Vieira, J., & Borges, I. (2022). Water Retention Curve of Biocemented Sands Using MIP Results. Applied Sciences, 12(20), 10447. https://doi.org/10.3390/app122010447







