Suggestion of Practical Application of Discrete Element Method for Long-Term Wear of Metallic Materials
Abstract
:1. Introduction
2. Numerical Scheme
2.1. Wear Load Calculation Model
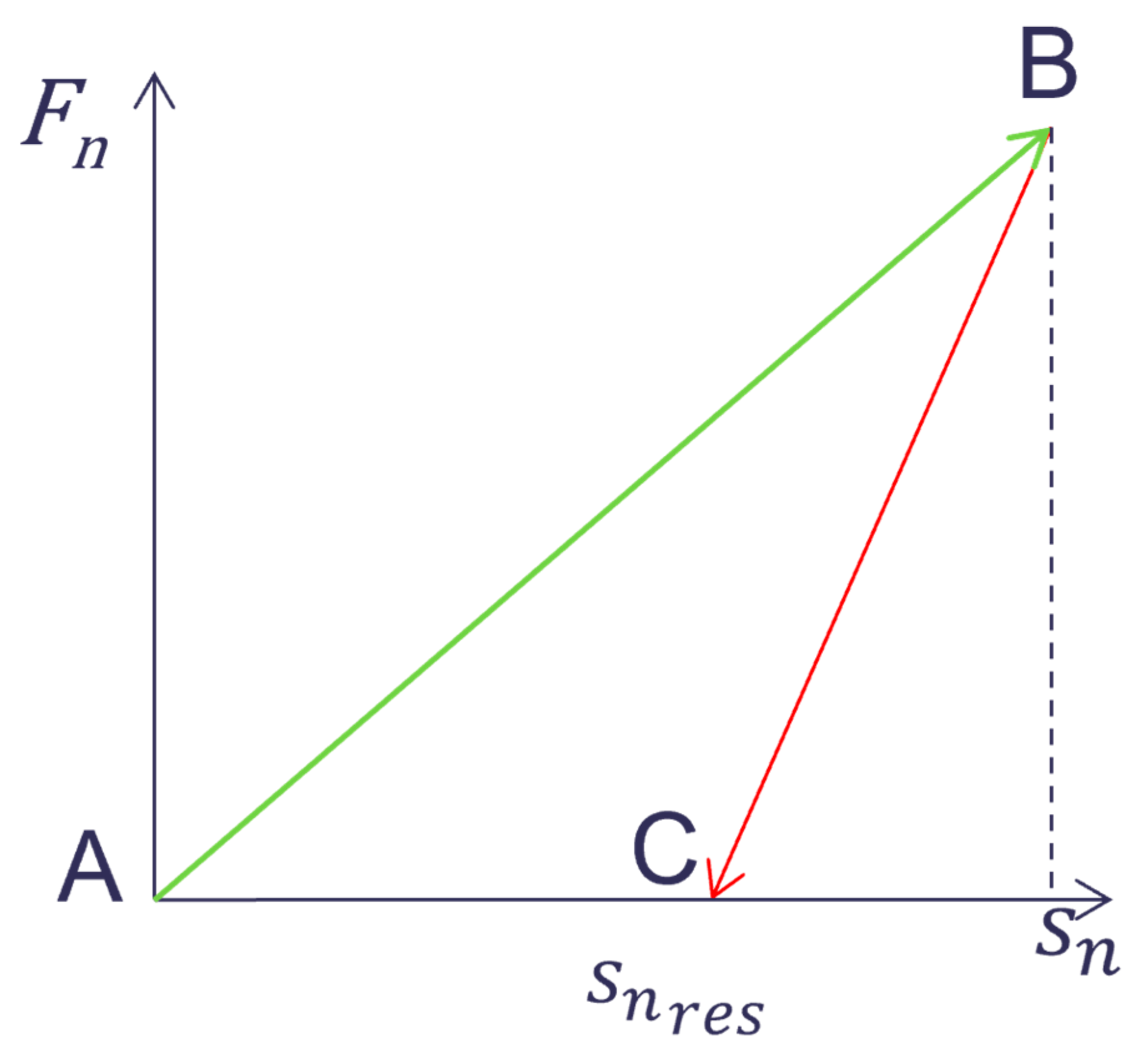
2.2. Archard’s Wear Law
3. Numerical Model for Wear Evaluation
3.1. Numerical Model
3.2. Material Properties
4. Wear Evaluation Results
4.1. Wear Shape Comparison
4.2. Long-Term Wear Rate Prediction Method
4.3. Normal and Tangential Forces
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Holmberg, K.; Kivikytö-Reponen, P.; Valtonen, K.; Erdemir, A. Global energy consumption due to friction and wear in the mining industry. Tribol. Int. 2017, 115, 116–139. [Google Scholar] [CrossRef]
- Kietzig, A.M.; Savvas, G.; Hatzikiriakos, A.; Peter, E. Physics of ice friction. J. Appl. Phys. 2010, 107, 081101. [Google Scholar] [CrossRef]
- Evans, D.C.B.; John, F.N.; Cheeseman, K.J. The kinetic friction of ice. Proc. R. Soc. A Math. Phys. Sci. 1976, 347, 493–512. [Google Scholar]
- Oksanen, P. Coefficient of Friction between Ice and Some Construction Materials, Plastics and Coatings; VTT Technical Research Centre of Finland: Espoo, Finland, 1980. [Google Scholar]
- Kim, J.H.; Kim, Y. Numerical simulation on the ice-induced fatigue damage of ship structural members in broken ice fields. Mar. Struct. 2019, 66, 83–105. [Google Scholar] [CrossRef]
- Johansson, A.; Andersson, C. Out-of-round railway wheels—A study of wheel polygonalization through simulation of three-dimensional wheel–rail interaction and wear. Veh. Syst. Dyn. 2005, 43, 539–559. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Archard, J.F. Wear theory and mechanisms. In Wear Control Handbook; American Society of Mechanical Engineers: New York, NY, USA, 1980. [Google Scholar]
- Põdra, P.; Andersson, S. Simulating sliding wear with finite element method. Tribol. Int. 1999, 32, 71–81. [Google Scholar] [CrossRef]
- Öqvist, M. Numerical Simulations of Wear. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, November 2000. [Google Scholar]
- Tang, Z.; Talke, F.E. Fretting wear simulation of the dimple/gimbal interface. IEEE Trans. Magn. 2019, 55, 18470601. [Google Scholar] [CrossRef]
- Shimizu, K.; Noguchi, T.; Seitoh, H.; Okada, M.; Matsubara, Y. FEM analysis of erosive wear. Wear 2001, 250, 779–784. [Google Scholar] [CrossRef]
- Xie, L.-J.; Schmidt, J.; Schmidt, C.; Biesinger, F. 2D FEM estimate of tool wear in turning operation. Wear 2005, 258, 1479–1490. [Google Scholar] [CrossRef]
- Varga, M.; Goniva, C.; Adam, K.; Badisch, E. Combined experimental and numerical approach for wear prediction in feed pipes. Tribol. Int. 2013, 65, 200–206. [Google Scholar] [CrossRef]
- Zhu, L.; Cheng, X.; Peng, S.S.; Qi, Y.Y.; Zhang, W.F.; Jiang, R.; Yin, C.L. Three-dimensional computational fluid dynamic interaction between soil and plowbreast of horizontally reversal plow. Comput. Electron. Agric. 2016, 123, 1–9. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, S.; Pan, H.; Zheng, Z.; Huang, Y.; Zhu, R. Effect of soil particle size on soil-subsoiler interactions using the discrete element method simulations. Biosyst. Eng. 2019, 182, 138–150. [Google Scholar] [CrossRef]
- Phan, H.T.; Tieu, A.K.; Zhu, H.; Kosasih, B.; Zhu, Q.; Grima, A.; Ta, T.D. A study of abrasive wear on high-speed steel surface in hot rolling by discrete element method. Tribol. Int. 2017, 110, 66–76. [Google Scholar] [CrossRef]
- Rojek, J. Discrete element thermomechanical modelling of rock cutting with valuation of tool wear. Comput. Part. Mech. 2014, 1, 71–84. [Google Scholar] [CrossRef]
- Xie, C.; Zhao, Y. Investigation of the ball wear in a planetary mill by DEM simulation. Powder Technol. 2022, 398, 117057. [Google Scholar] [CrossRef]
- Li, H.; Liu, Y.; Liao, H.; Liang, Z. Accelerated wear test design based on dissipation wear model entropy analysis under mixed lubrication. Lubricants 2022, 10, 71. [Google Scholar] [CrossRef]
- Choudhry, J.; Larsson, R.; Almqvist, A. A stress-state-dependent thermo-mechanical wear model for micro-scale contacts. Lubricants 2022, 10, 223. [Google Scholar] [CrossRef]
- Egidijus, K.; Rostislav, C.; Miloslav, L.; Jankauskas, V. Wear modelling of soil ripper tine in sand and sandy clay by discrete element method. Biosyst. Eng. 2019, 188, 305–319. [Google Scholar]
- ESSS Rocky. Rocky DEM Technical Manual; Release 2022 R1.1; ESSS: Rio de Janeiro, Brazil, 2022. [Google Scholar]
- Finnie, I. Some observations on the erosion of ductile metals. Wear 1972, 19, 81–90. [Google Scholar] [CrossRef]
- Zum Gahr, K.H. Modeling of two-body abrasive wear. Wear 1988, 124, 87–103. [Google Scholar] [CrossRef]
- Rabinowicz, E. Friction and Wear of Materials; Wiley: New York, NY, USA, 1995. [Google Scholar]
- Xiangjun, Q.; Alexander, P.; Ming, S.; Lawrence, N. Prediction of wear of mill lifters using discrete element method. In Proceedings of the SAG Conference, Vancouver, BC, Canada, 30 September 2001. [Google Scholar]





















| Soil Particles | Wear Plate | |
|---|---|---|
| Density [kg/m3] | 1560 | 7850 |
| Bulk Young’s modulus [MPa] (Equation (7)) | 40 | 2 × 105 |
| Poisson’s ratio | 0.3 | 0.3 |
| Shear work ratio [m3/J] (Equation (13)) | -- | 500 |
| Soil Particle–Soil Particle | Soil Particle–Wear Plate | |
|---|---|---|
| Static friction coefficient (Equation (3)) | 0.6 | 0.5 |
| Dynamic friction coefficient (Equation (3)) | 0.3 | 0.5 |
| Tangential stiffness ratio (Equation (8)) | 1 | 1 |
| Restitution coefficient (Equation (6)) | 0.2 | 0.3 |
| Penetration Depth [mm] | Loss Ratio [%] Reference Study [22] | Loss Ratio [%] Present study | |
|---|---|---|---|
| Experiment | Simulation | ||
| 25 | 4.2 | 0.7 | 4.2 |
| 75 | 2.4 | 2.5 | 3.8 |
| 125 | 5.2 | 5.0 | 4.3 |
| 180 | 1.8 | 1.7 | 6.0 |
| 200 | 14.2 | 2.1 | 15.4 |
| 202 | 33.1 | 7.6 | 33.0 |
| 204 | 57.9 | 9.0 | 70.1 |
| Penetration Depth [mm] | Loss Ratio [%] | |||||
|---|---|---|---|---|---|---|
| 0.2 s | 0.4 s | 0.6 s | 0.8 s | 1.0 s | 1.1 s | |
| 25 | 0.7 | 1.4 | 2.0 | 2.9 | 3.5 | 4.2 |
| 75 | 0.6 | 1.2 | 1.9 | 2.5 | 3.1 | 3.8 |
| 125 | 0.5 | 1.2 | 2.0 | 2.7 | 3.3 | 4.3 |
| 180 | 0.9 | 1.8 | 2.8 | 3.8 | 4.8 | 6.1 |
| 200 | 1.6 | 3.8 | 6.4 | 9.4 | 12.4 | 15.4 |
| 202 | 5.6 | 12.4 | 18.1 | 23.5 | 28.4 | 33.0 |
| 204 | 9.0 | 20.4 | 33.3 | 48.0 | 57.8 | 70.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, S.-J.; Lee, J.-H.; Hwang, S.-Y. Suggestion of Practical Application of Discrete Element Method for Long-Term Wear of Metallic Materials. Appl. Sci. 2022, 12, 10423. https://doi.org/10.3390/app122010423
Lee S-J, Lee J-H, Hwang S-Y. Suggestion of Practical Application of Discrete Element Method for Long-Term Wear of Metallic Materials. Applied Sciences. 2022; 12(20):10423. https://doi.org/10.3390/app122010423
Chicago/Turabian StyleLee, Sung-Je, Jang-Hyun Lee, and Se-Yun Hwang. 2022. "Suggestion of Practical Application of Discrete Element Method for Long-Term Wear of Metallic Materials" Applied Sciences 12, no. 20: 10423. https://doi.org/10.3390/app122010423
APA StyleLee, S.-J., Lee, J.-H., & Hwang, S.-Y. (2022). Suggestion of Practical Application of Discrete Element Method for Long-Term Wear of Metallic Materials. Applied Sciences, 12(20), 10423. https://doi.org/10.3390/app122010423







