Design and Simulation of a Solar Tracking System for PV
Abstract
1. Introduction
2. Methodology
2.1. Assumptions
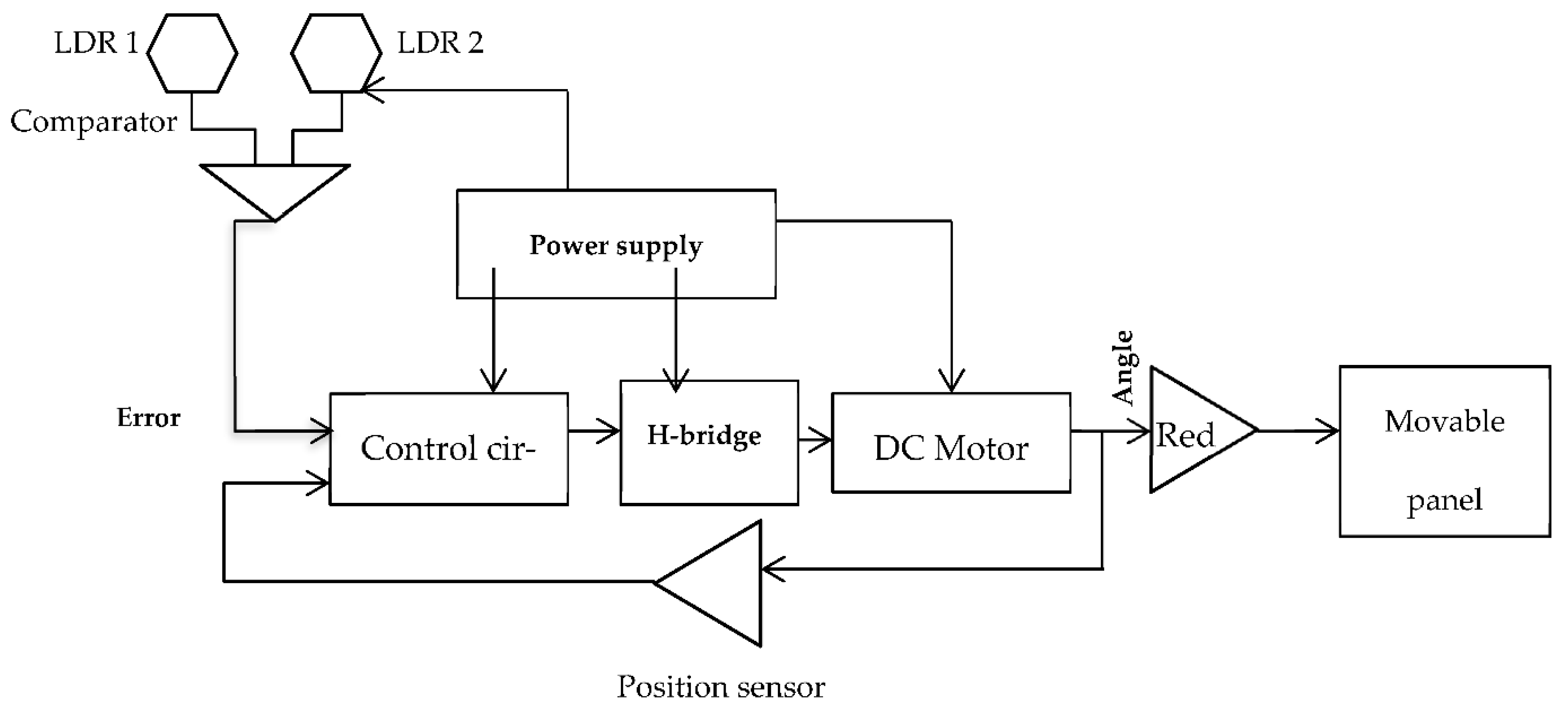
2.2. Block Diagram of a Single-Axis Tracker
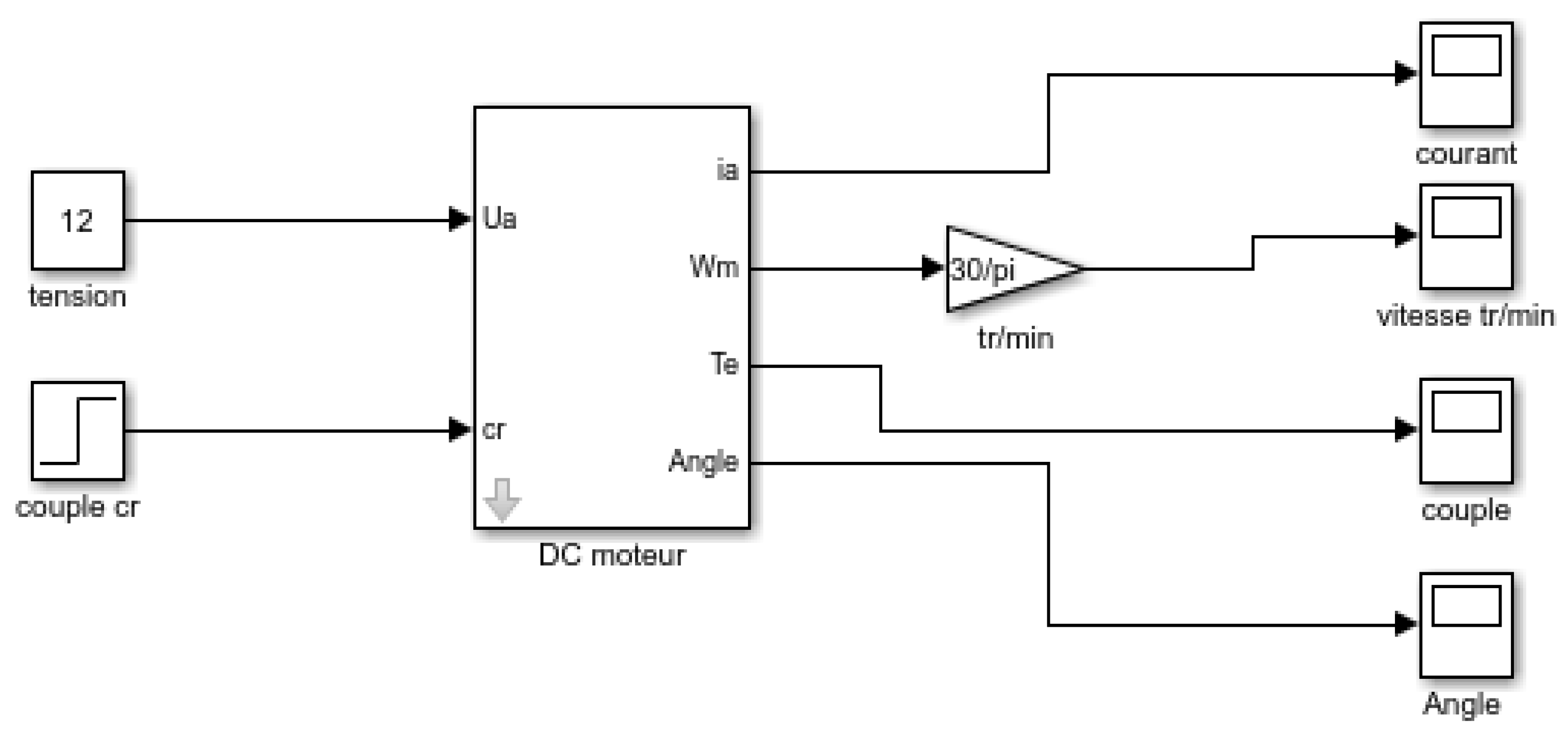
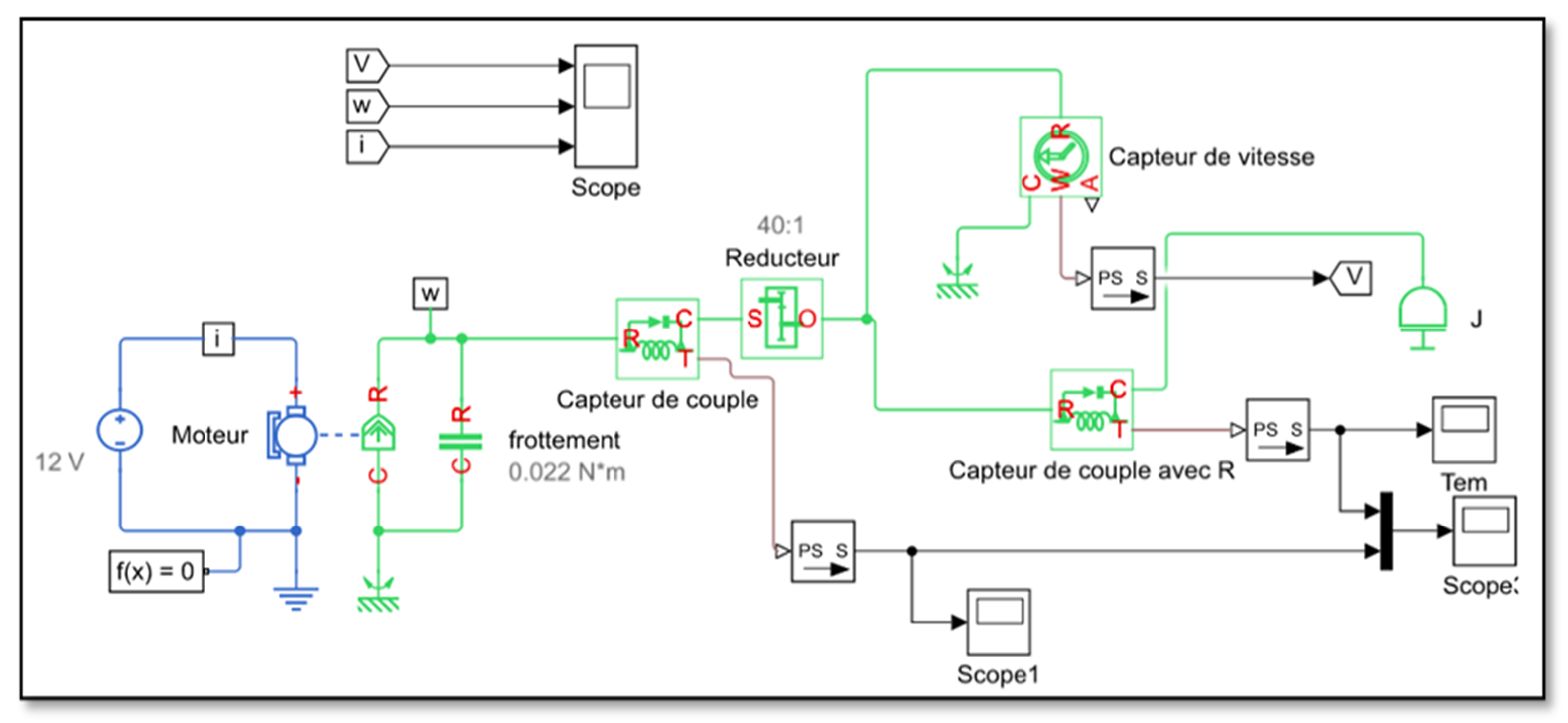
2.3. D.C. Motor Block Controlled by a Chopper
- -
- Variation of the speed for a motor by the P.W.M. (pulse width modulation) command.
- -
- Voltage reversible chopper.
- -
- The average value of the supply voltage.
- -
- Direction inversion control.
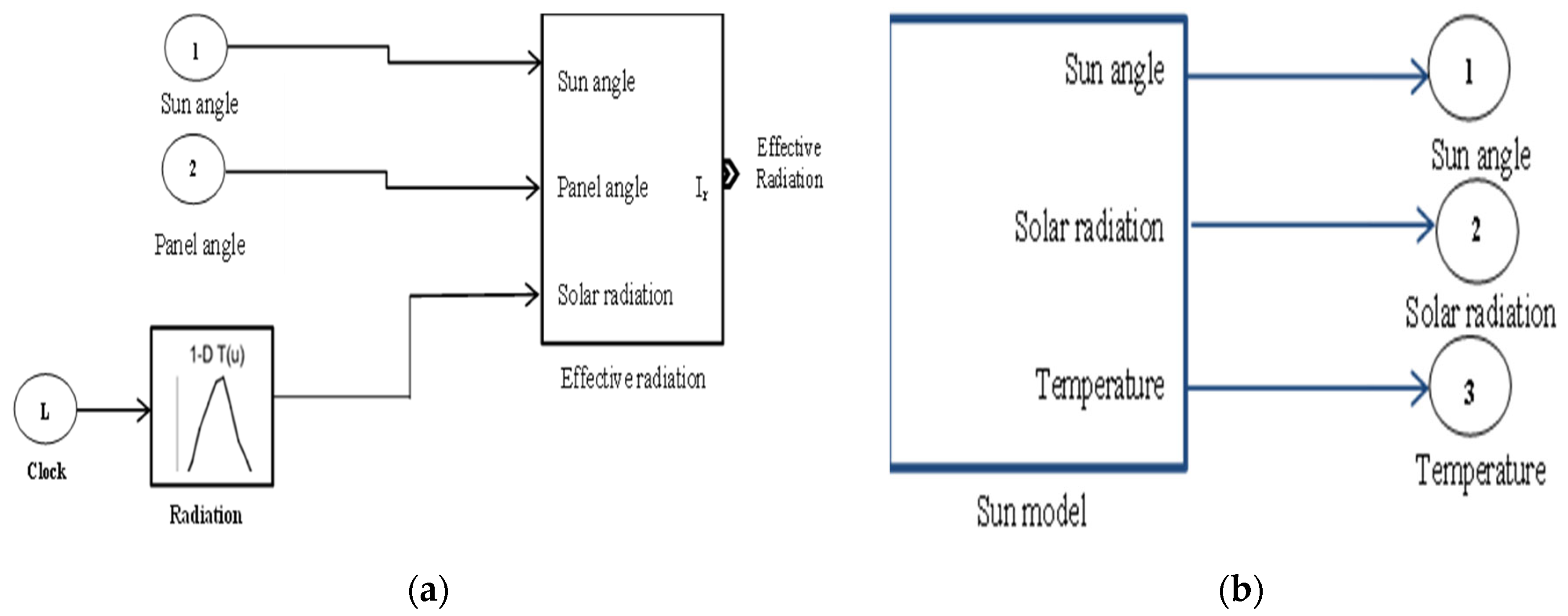
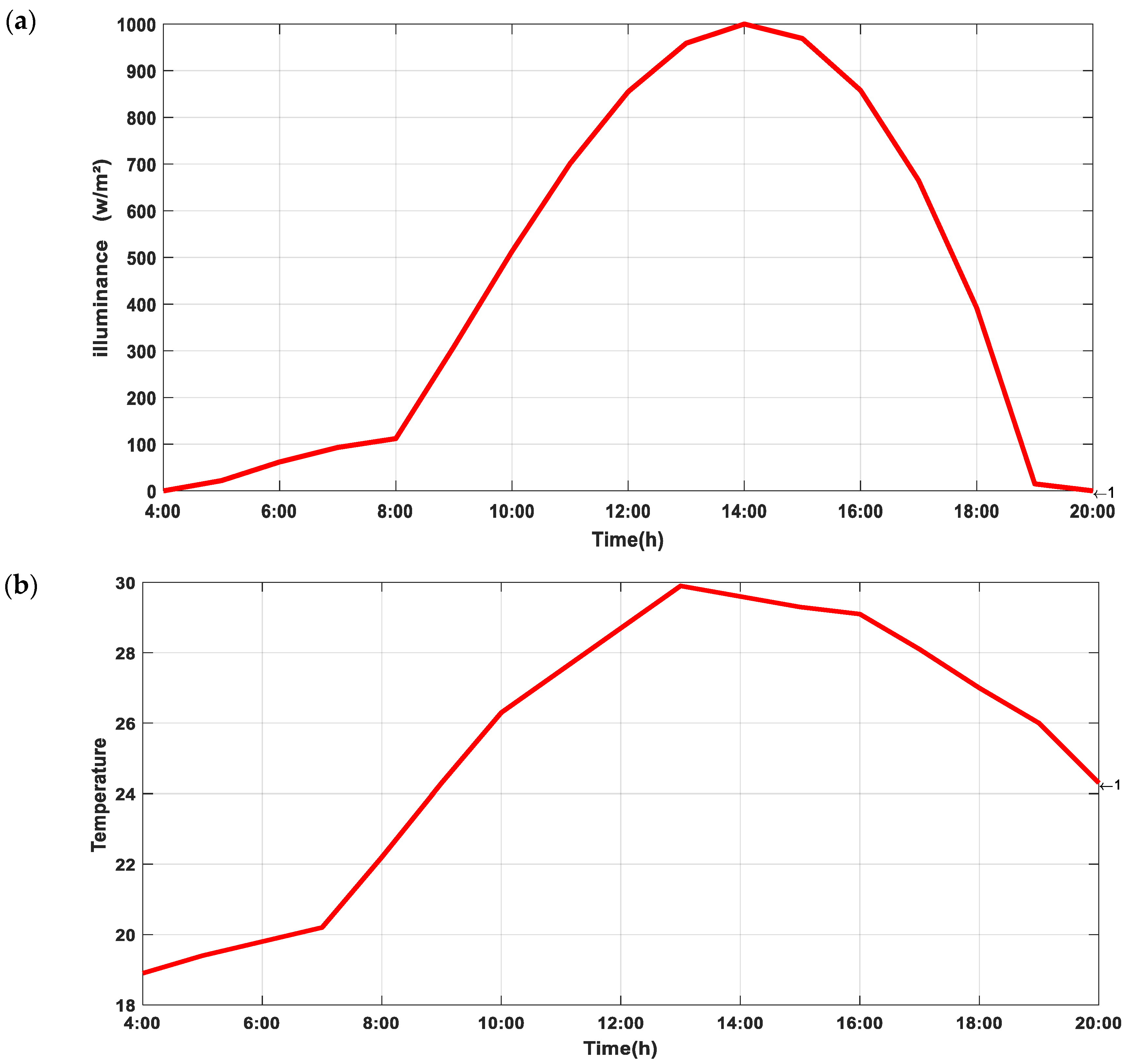
2.4. Effective Radiation Quantity on the Solar Panel and Calculation of Solar Irradiance
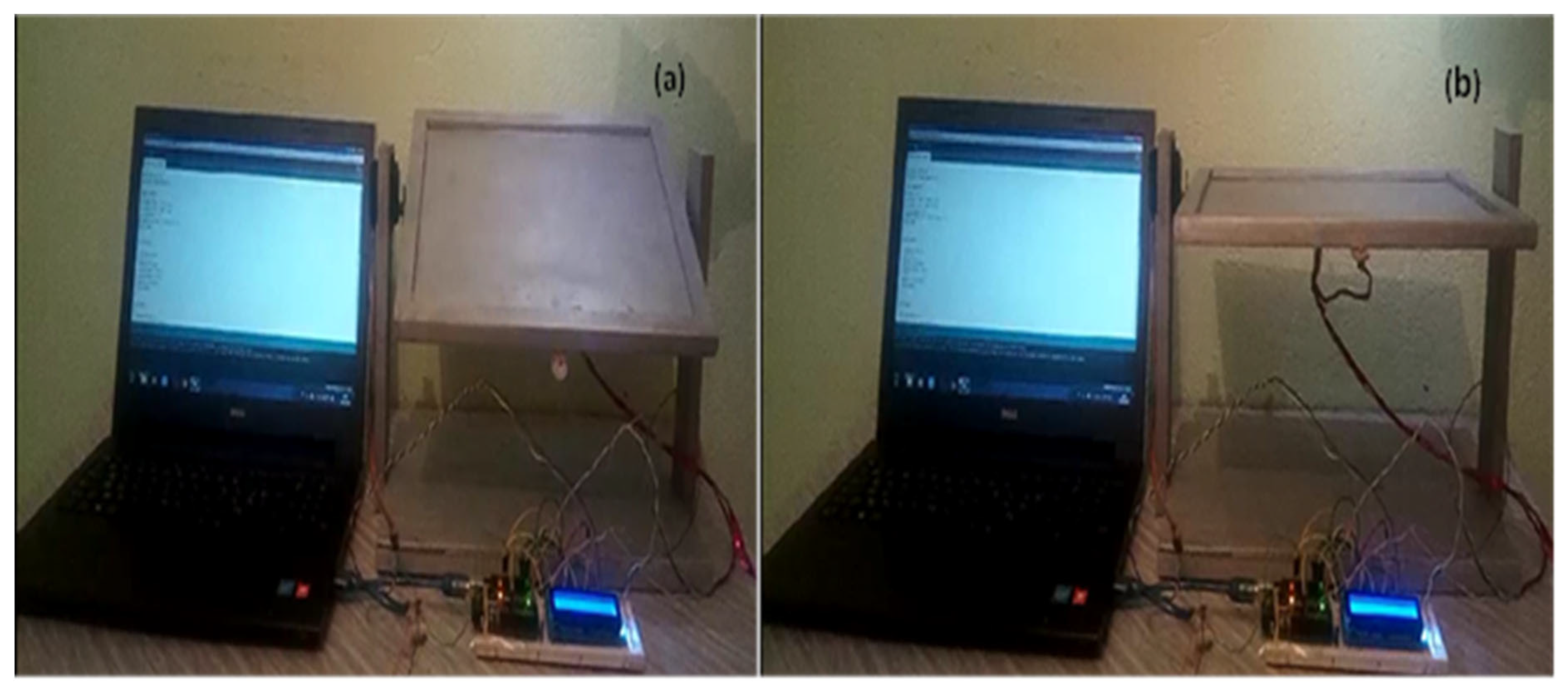
3. Design and Implementation
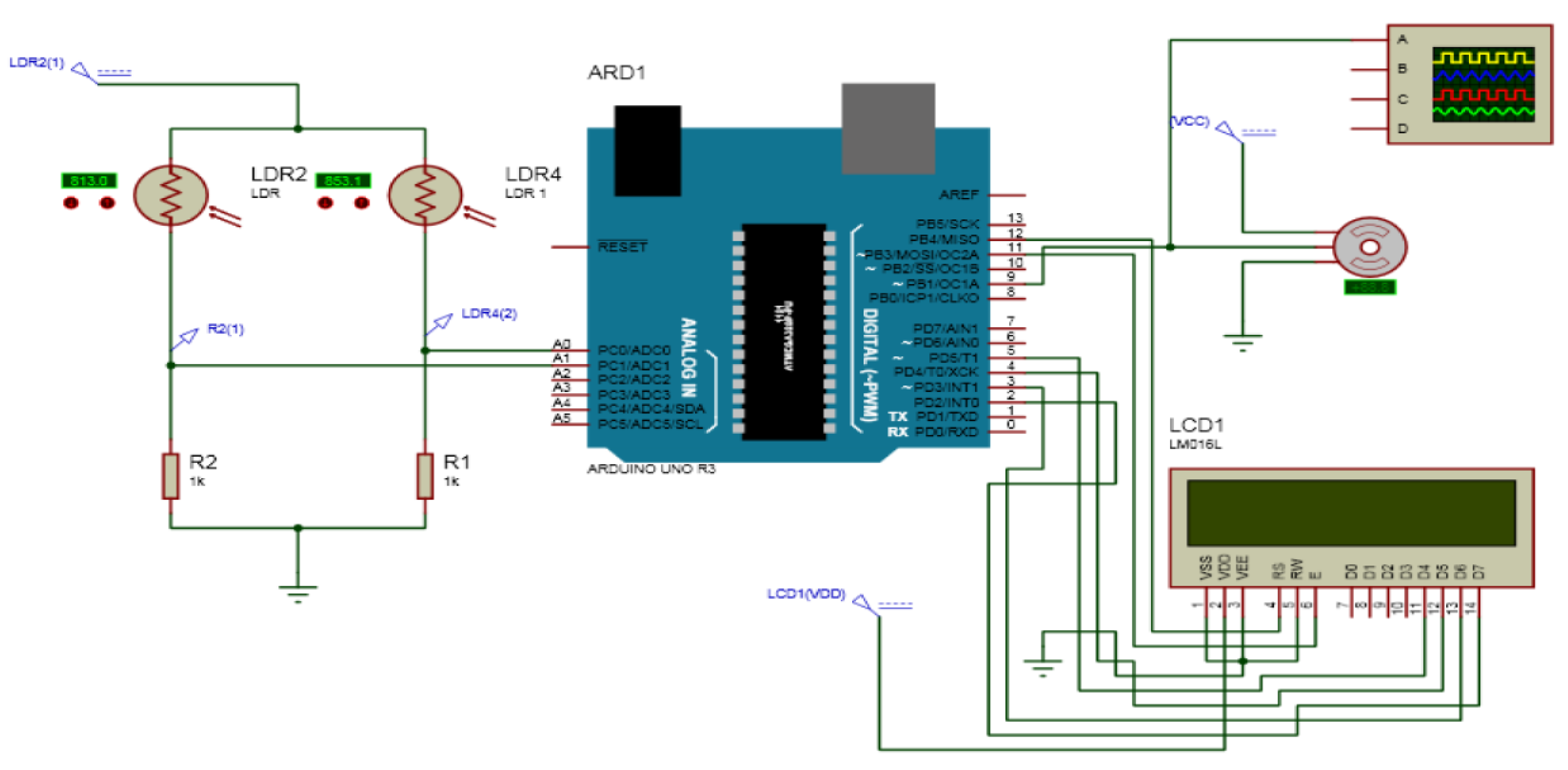
3.1. Electronic Control
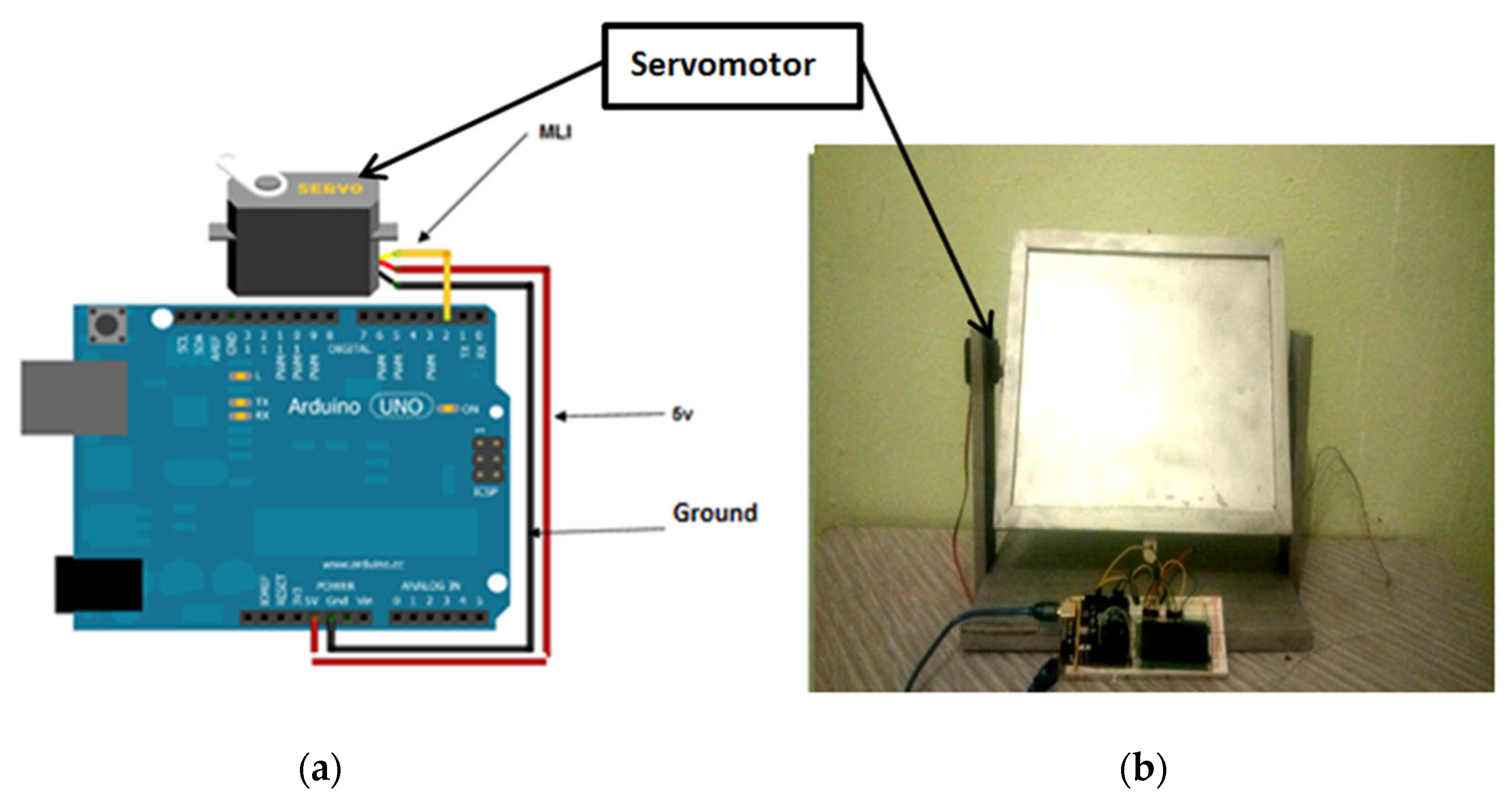
3.2. Servomotor
4. Results and Discussion
4.1. Modeling of a PV Panel
4.2. Command of the Servomotor for Position-Controlled by P.W.M.
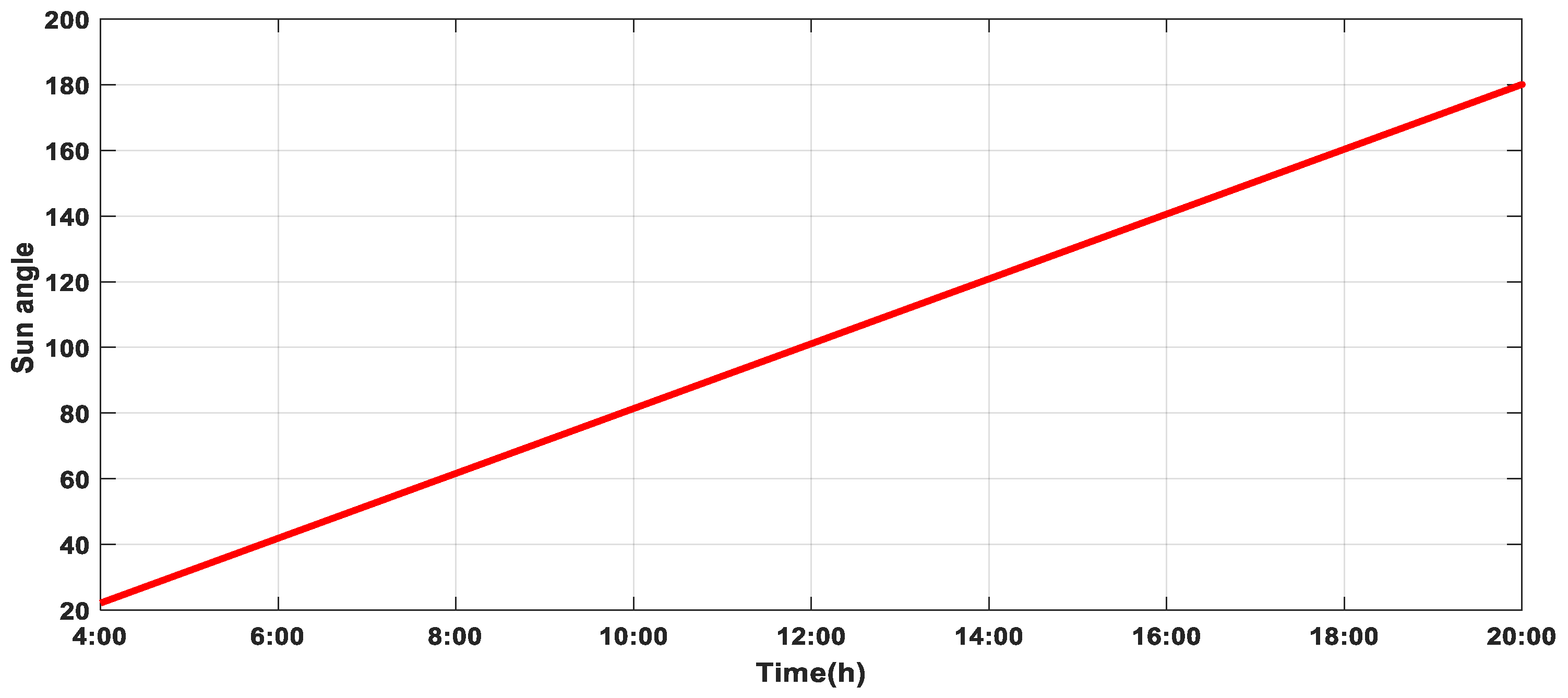
4.3. Simulation of the Sun
- -
- Equal to 0 from 00:45 a.m. to 4:45 a.m. and from 8:45 p.m. to 11:45 p.m.;
- -
- Starts increasing by 11 and 1030 from 5:45 a.m. to 13:45 p.m., then decreases from 1030 to 8 from 2:45 p.m. to 7:45 p.m.
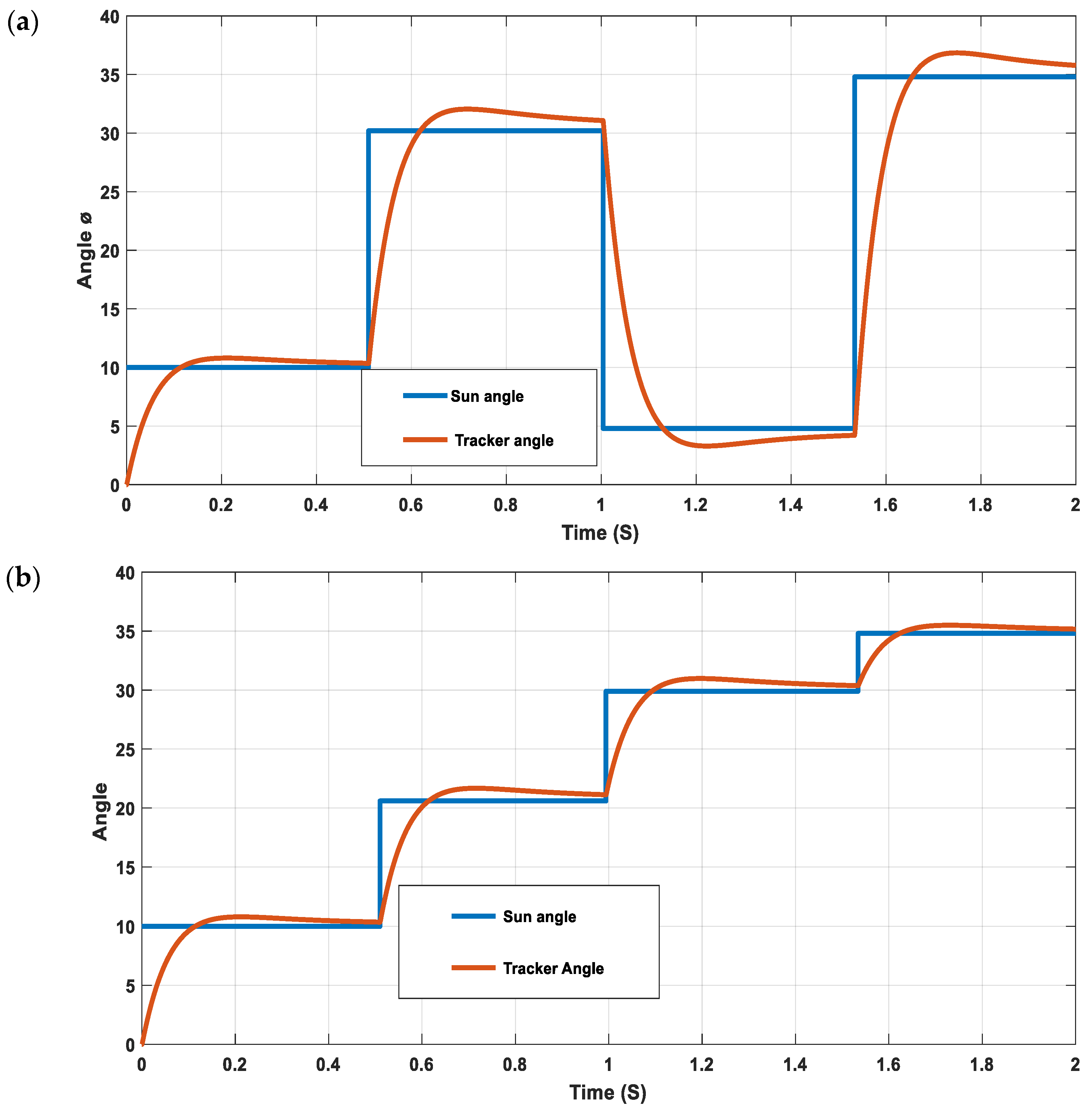
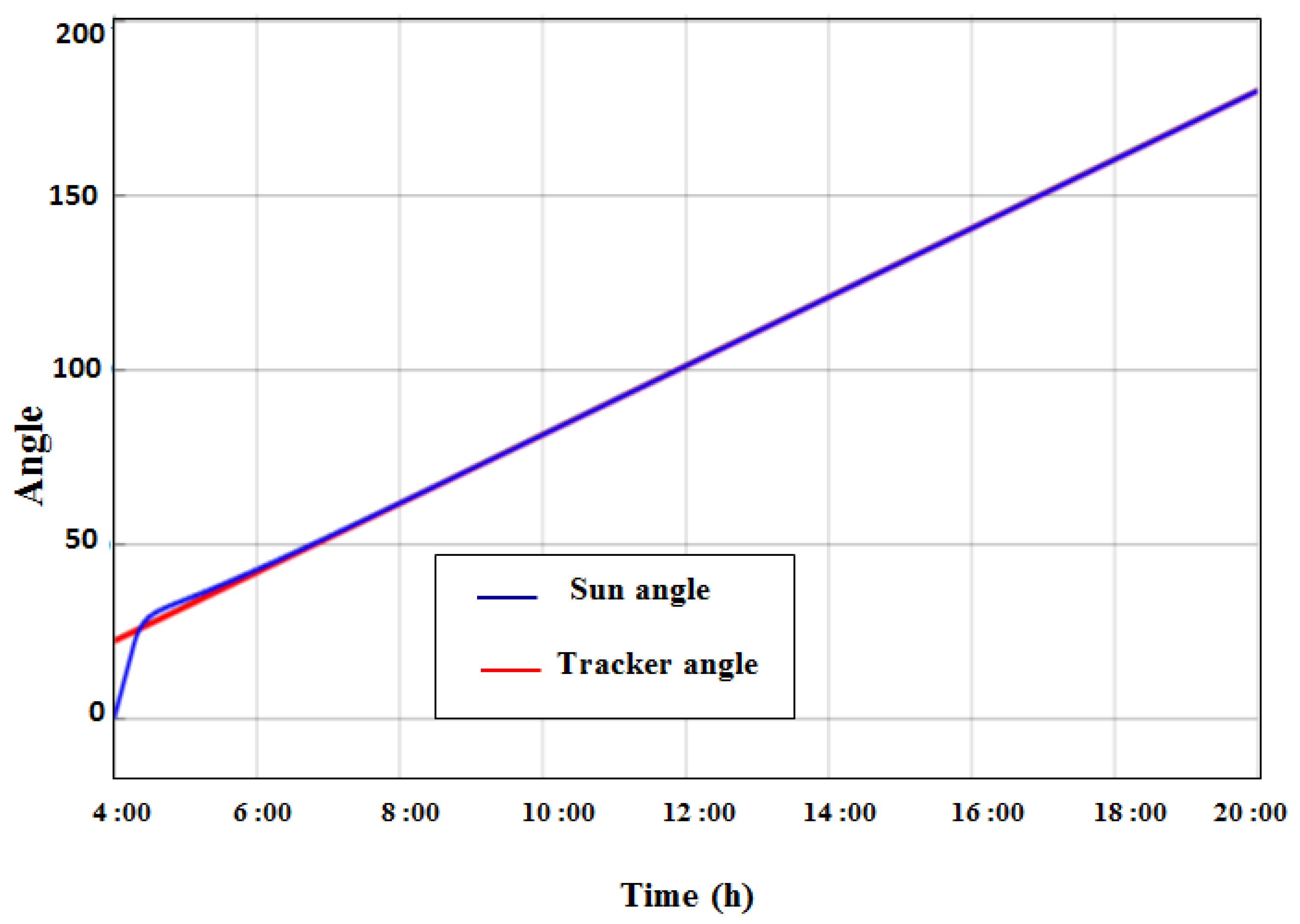
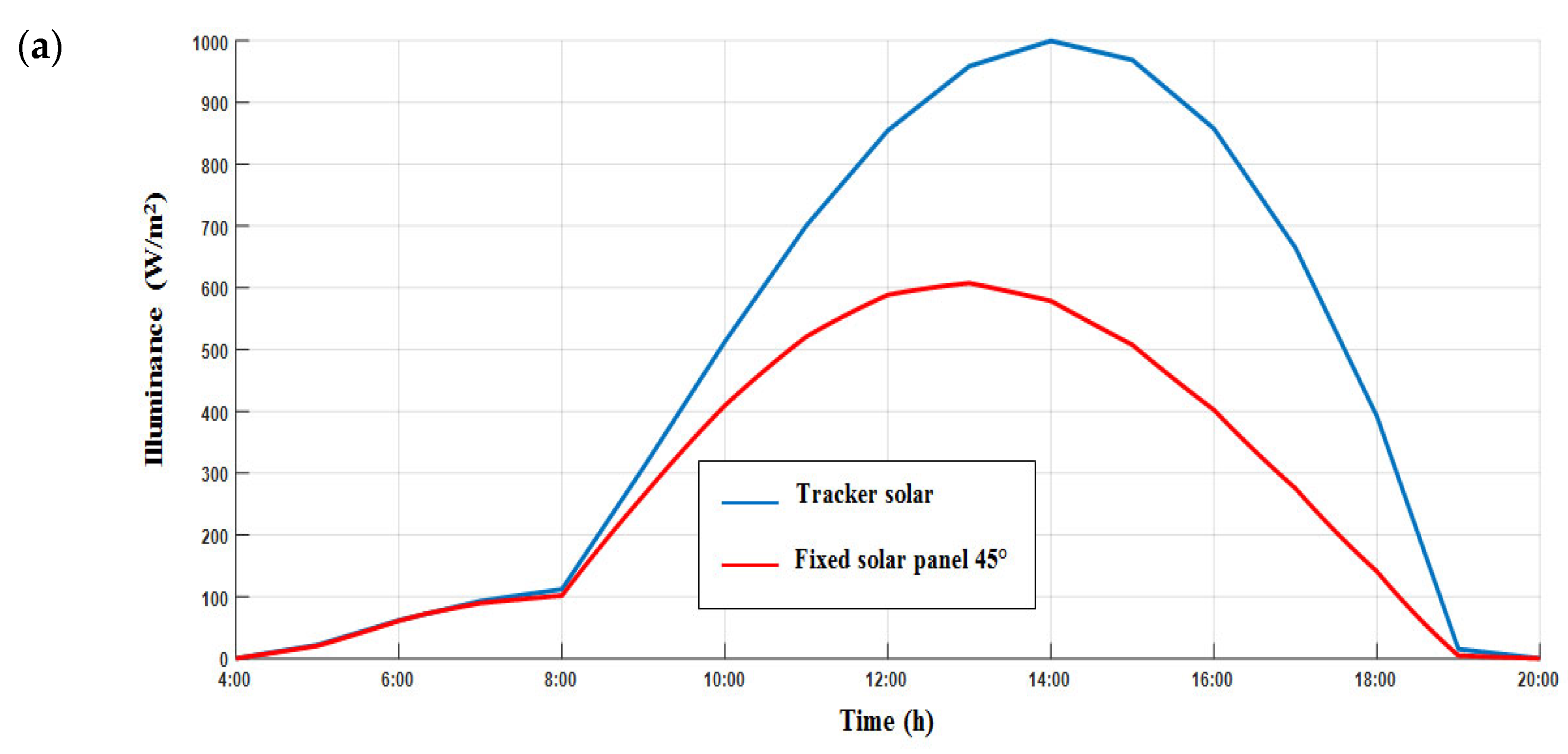
4.4. Tests of the Tracker
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Acknowledgments
Conflicts of Interest
References
- Hua, Z.; Ma, C.; Lian, J.; Pang, X.; Yang, W. Optimal capacity allocation of multiple solar trackers and storage capacity for utility-scale photovoltaic plants considering output characteristics and complementary demand. Appl. Energy 2019, 238, 721–733. [Google Scholar] [CrossRef]
- Gómez-Uceda, F.J.; Moreno-Garcia, I.M.; Jiménez-Martínez, J.M.; López-Luque, R.; Fernández-Ahumada, L.M. Analysis of the Influence of Terrain Orientation on the Design of PV Facilities with Single-Axis Trackers. Appl. Sci. 2020, 10, 8531. [Google Scholar] [CrossRef]
- Chong, K.; Wong, C. General Formula for on-axis sun-tracking system and its application in improving tracking accuracy of solar collector. Sol. Energy 2009, 83, 298–305. [Google Scholar] [CrossRef]
- Ferroudji, F. Conception et Optimisation en Dynamique Forcée D’un Nouveau Prototype de Système de Suiveur Solaire à Deux axes. Ph.D. Thesis, University of Batna, Batna, Algeria, 2015. [Google Scholar]
- Abu-Khadera, M.; Badranb, O.; Abdallah, S. Evaluating multi-axes sun-tracking system at different modes of operation in Jordan. Renew. Sustain. Energy Rev. 2008, 12, 864–873. [Google Scholar] [CrossRef]
- Available online: http://shodh.inflibnet.ac.in/bitstream/123456789/4251/3/03_liteature%20review.pdf (accessed on 22 August 2022).
- Alexandru, C.; Pozna, C. Different tracking strategies for optimizing the energetic efficiency of a photovoltaic system. In Proceedings of the 2008 IEEE International Conference on Automation, Quality and Testing, Robotics, Cluj-Napoca, Romania, 22–25 May 2008; Volume 3, pp. 434–439. [Google Scholar]
- Natarajan, S.K.; Thampi, V.; Shaw, R.; Kumar, V.S.; Nandu, R.S.; Jayan, V.; Rajagopalan, N.; Kandasamy, R.K. Experimental analysis of a two axis tracking system for solar parabolic dish collector. Int. J. Energy Res. 2019, 43, 1012–1018. [Google Scholar] [CrossRef]
- AL-Rousan, N.; Mat Isa, N.A.; Mat Desa, M.K. Correlation analysis and MLP/CMLP for optimum variables to predict orientation and tilt angles in intelligent solar tracking systems. Int. J. Energy Res. 2021, 45, 453–477. [Google Scholar] [CrossRef]
- Farrar-Foley, N.; Rongione, N.A.; Wu, H.; Lavine, A.S.; Hu, Y. Total solar spectrum energy converter with integrated photovoltaics, thermoelectrics, and thermal energy storage: System modeling and design. Int. J. Energy Res. 2021, 46, 5731–5744. [Google Scholar] [CrossRef]
- El Moubarak Bouzid, A.; Mohammed, A. Dimensionnement D’un Système Photovoltaïque Pour L’alimentation D’une Ferme Étude de L’onduleur Triphasé Lié à Cette Application. Engineer Thesis, University of Oran, Es Senia, Algeria, 2008. [Google Scholar]
- Hafez, A.Z.; Yousef, A.M.; Harag, N.M. Solar tracking systems: Technologies and trackers drive types. A review. Renew. Sustain. Energy Rev. 2018, 91, 754–782. [Google Scholar] [CrossRef]
- Merlaud, A.; De Mazière, M.; Hermans, C.; Cornet, A. Equations for Solar Tracking. Sensors 2012, 12, 4074–4090. [Google Scholar] [CrossRef]
- Ruelas, J.; Muñoz, F.; Lucero, B.; Palomares, J. PV Tracking Design Methodology Based on an Orientation Efficiency Chart. Appl. Sci. 2019, 9, 894. [Google Scholar] [CrossRef]
- Visconti, P.; Costantini, P.; Orlando, C.; Lay-Ekuakille, A.; Cavalera, G. Software solution implemented on hardware system to manage and drive multiple bi-axial solar trackers by PC in photovoltaic solar plants. Measurement 2015, 76, 80–92. [Google Scholar] [CrossRef]
- Nsengiyumva, W.; Chen, S.G.; Hu, L.; Chen, X. Recent advancements and challenges in Solar Tracking Systems (S.T.S.): A review. Renew. Sustain. Energy Rev. 2018, 81, 250–279. [Google Scholar] [CrossRef]
- Awasthi, A.; Shukla, A.K.; Manohar S.R., M.; Dondariya, C.; Shukla, K.N.; Porwal, D.; Richhariya, G. Review on sun tracking technology in solar PV system. Energy Rep. 2020, 6, 392–405. [Google Scholar] [CrossRef]
- Ladmi, A.; Arbaoui, K. Modélisation et Optimisation des Performances D’un Suiveur Solaire. Master’s Thesis, Faculty of Sciences and Technology, University of Khemis Miliana, Khemis Miliana, Algeria, 2019. [Google Scholar]
- Hariri, N.G.; AlMutawa, M.A.; Osman, I.S.; AlMadani, I.K.; Almahdi, A.M.; Ali, S. Experimental Investigation of Azimuth- and Sensor-Based Control Strategies for a PV Solar Tracking Application. Appl. Sci. 2022, 12, 4758. [Google Scholar] [CrossRef]
- Alici, H.; Esenboga, B.; Oktem, I.; Demirdelen, T.; Tumay, M. Chapter 7: Designing and performance analysis of solar tracker system: A case st udy of Çukurova region. In Design, Analysis, and Applications of Renewable Energy Systems Advances in Nonlinear Dynamics and Chaos (ANDC); Academic Press: New York, NY, USA, 2021; pp. 165–184. [Google Scholar]
- Balabel, A.; Mahfouz, A.; Salem, F. Design and performance of Solar Tracking Photo-Voltaic system; Research and education. Int. J. Control Autom. Syst. 2013, 1, 49–55. [Google Scholar]
- Etude, K.I. Conception et Réalisation d’un Suiveur de Soleil. Master’s Thesis, University of Abou-Bekr Belkaid Tlemcen-Algeria, Chetouane, Algeria, 2018. [Google Scholar]
- Chin, C.S.; Babu, A.; McBride, W. Design, modeling and testing of a standalone single axis active solar tracker using MATLAB/Simulink. Renew. Energy 2011, 36, 3075–3090. [Google Scholar] [CrossRef]
- Mousazadeh, H.; Keyhani, A.; Javadi, A.; Mobli, H.; Abrinia, K.; Sharifi, A. A review of principle and sun-tracking methods for maximizing solar systems output. Renew. Sustain. Energy Rev. 2009, 13, 1800–1818. [Google Scholar] [CrossRef]
- Afarulrazi, B.A.; Utomo, W.M.; Liew, K.L.; Zarafi, M. Solar tracker robot using microcontroller. In Proceedings of the 2011 International Conference on Business, Engineering and Industrial Applications, Kuala Lumpur, Malaysia, 5–7 June 2011; pp. 47–50. [Google Scholar]
- Nuwayhid, R.Y.; Mrad, F.; Abu-Said, R. The realization of a simple solar tracking concentrator for university research applications. Renew. Energy 2001, 24, 207–222. [Google Scholar] [CrossRef]
- Le Moteur à Courant Continu: Principe, Astuces-Pratiques. 2015. Available online: https://www.astuces-pratiques.fr/electronique/le-moteur-a-courant-continu-principe (accessed on 22 August 2022).
- Eric, G. Le Servomoteur. 2011. Available online: http://ericaeromodelisme974.unblog.fr/2011/06/04/le-servomoteur (accessed on 22 August 2022).
- Rabeh, M.B.; Ayoub, D. Conception et Réalisation d’un Suiveur Solaire Bi-axial à Bas De capteurs de lumière. Master’s Thesis, University of Kasdi Merbah Ouargla, Ouargla, Algeria, 2016. [Google Scholar]
- Electrical Guide 360. D.C. Chopper or DC-to-DC Converter: Working & Its Function; Power Electronics: Valencia, Spain, 2021. [Google Scholar]
- Solar Angles & Tracking Systems. Photovoltaic Efficiency: Lesson 1, Fundamentals Article; Free STEM Curriculum for K-12. Available online: https://www.TeachEngineering.org (accessed on 22 August 2022).
- Telidjane, M. Modélisation des Panneaux Photovoltaïques et Adaptation de la Cyclostationarité pour le Diagnostic. Ph.D. Thesis, University of Lyon, Lyon, France, 13 July 2017. [Google Scholar]
- Marwan; Anshar, M. Controller Design for Solar Tracking System. In Proceedings of the International Conferences on Information System and Technology (CONRIST 2019), Yogyakarta, Indonesia, 5–6 December 2019; pp. 72–77. [Google Scholar] [CrossRef]
- Engr, F. How to Make a Solar Tracker Using Arduino, Arduino Solar Tracker System Its Circuit and Programming; Electronic Clinic: Wan Chai, Hong Kong, 2019. [Google Scholar]
- Aqib, M. Sun Tracking Solar Panel Using Arduino. Microcontroller-Projects. 2017. Available online: https://Circuitdigest.com (accessed on 22 August 2022).
- Necir, A.; Bouchaala, A.D.J. Conception et Réalisation d’un Suiveur du Soleil Commandé par une Carte Arduino. Master’s Thesis, University of El-Oued, El Oued, Algeria, 2018. [Google Scholar]
- Koussa, M.; Cheknane, A.; Hadji, S.; Haddadi, M.; Noureddine, S. Measured and modelled improvement in solar energy yield from flat plate photovoltaic systems utilizing different tracking systems and under a range of environmental conditions. Appl. Energy 2011, 88, 1756–1771. [Google Scholar] [CrossRef]
- Bahrami, A.; Okoye, C.O.; Atikol, U. The effect of latitude on the performance of di erent solar trackers in Europe and Africa. Appl. Energy 2016, 177, 896–906. [Google Scholar] [CrossRef]
- Abdallah, S.; Nijmeh, S. Two axes sun tracking system with PLC control. Energy Convers. Manag. 2004, 45, 1931–1939. [Google Scholar] [CrossRef]
- Antonanzas, J.; Urraca, R.; Martinez-de-Pison, F.J.; Antonanzas, F. Optimal solar tracking strategy to increase irradiance in the plane of array under cloudy conditions: A study across Europe. Sol. Energy 2018, 163, 122–130. [Google Scholar] [CrossRef]
- Batayneh, W.; Bataineh, A.; Soliman, I.; Hafees, S. Investigation of a single-axis discrete solar tracking system for reduced actuations and maximum energy collection. Autom. Constr. 2019, 98, 102–109. [Google Scholar] [CrossRef]
- Manoel Henriques de Sa, C.; Chigueru, T. npTrack: A n-Position Single Axis Solar Tracker Model for Optimized Energy Collection. Energies 2021, 14, 925. [Google Scholar]
- Seme, S.; Stumberger, B.; Hadziselimovic, M.; Sredensek, K. Solar Photovoltaic Tracking Systems for Electricity Generation: A Review. Energies 2020, 13, 4224. [Google Scholar] [CrossRef]
- Kuttybay, N.; Saymbetov, A.; Mekhilef, S.; Nurgaliyev, M.; Tukymbekov, D.; Dosymbetova, G.; Meiirkhanov, A.; Svanbayev, Y. Optimized Single-Axis Schedule Solar Tracker in Different Weather Conditions. Energies 2020, 13, 5226. [Google Scholar] [CrossRef]
- Farajdadian, S.; Hosseini, S.M.H. Conception d’un contrôleur flou optimal pour obtenir une puissance maximale dans un système de production d’énergie solaire. Solar Energy 2019, 182, 161–178. [Google Scholar] [CrossRef]
- Onyeka Okoye, C.; Bahrami, A.; Atikol, U. Evaluating the solar resource potential on different tracking surfaces in Nigeria. Renew. Sustain. Energy Rev. 2018, 81 Pt 1, 1569–1581. [Google Scholar] [CrossRef]


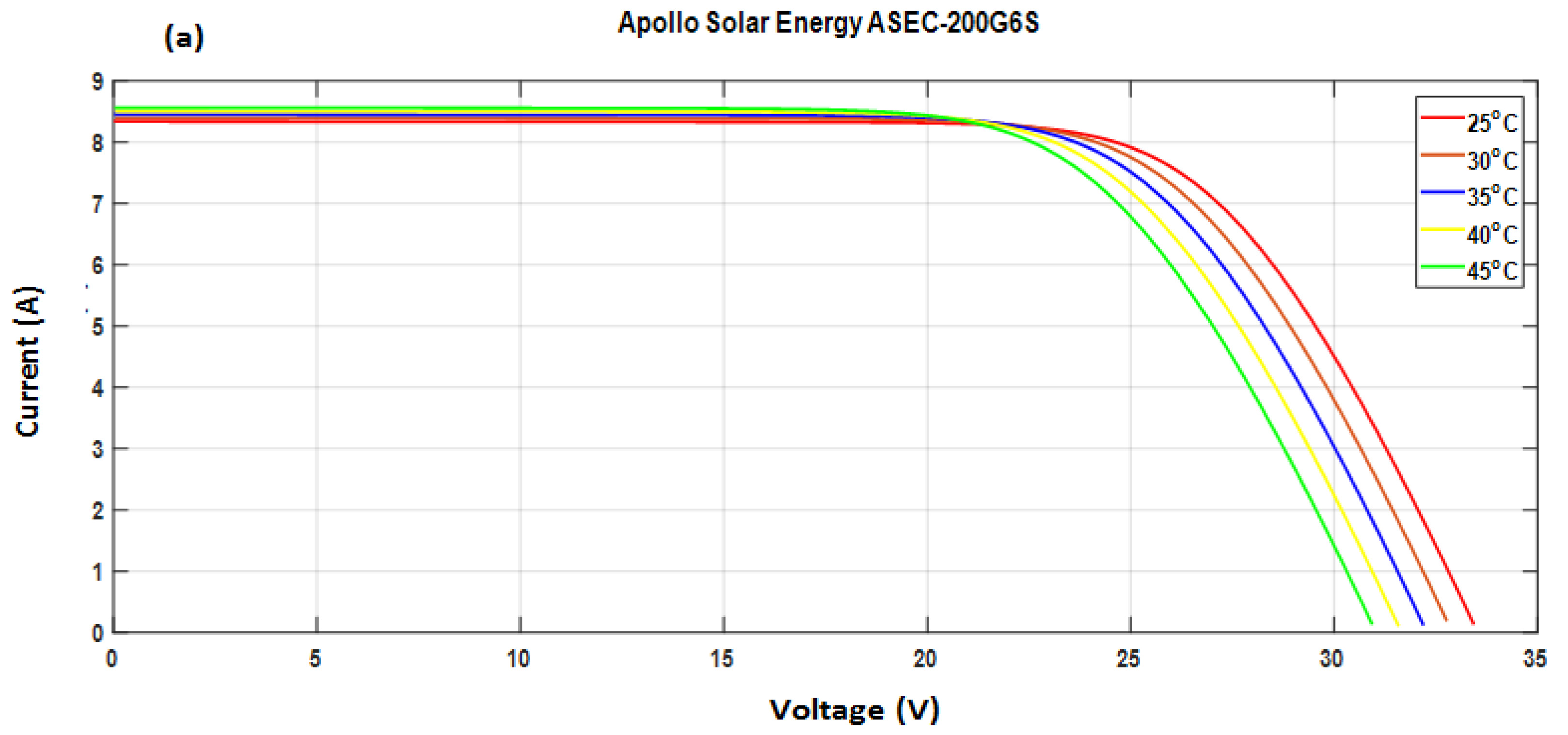
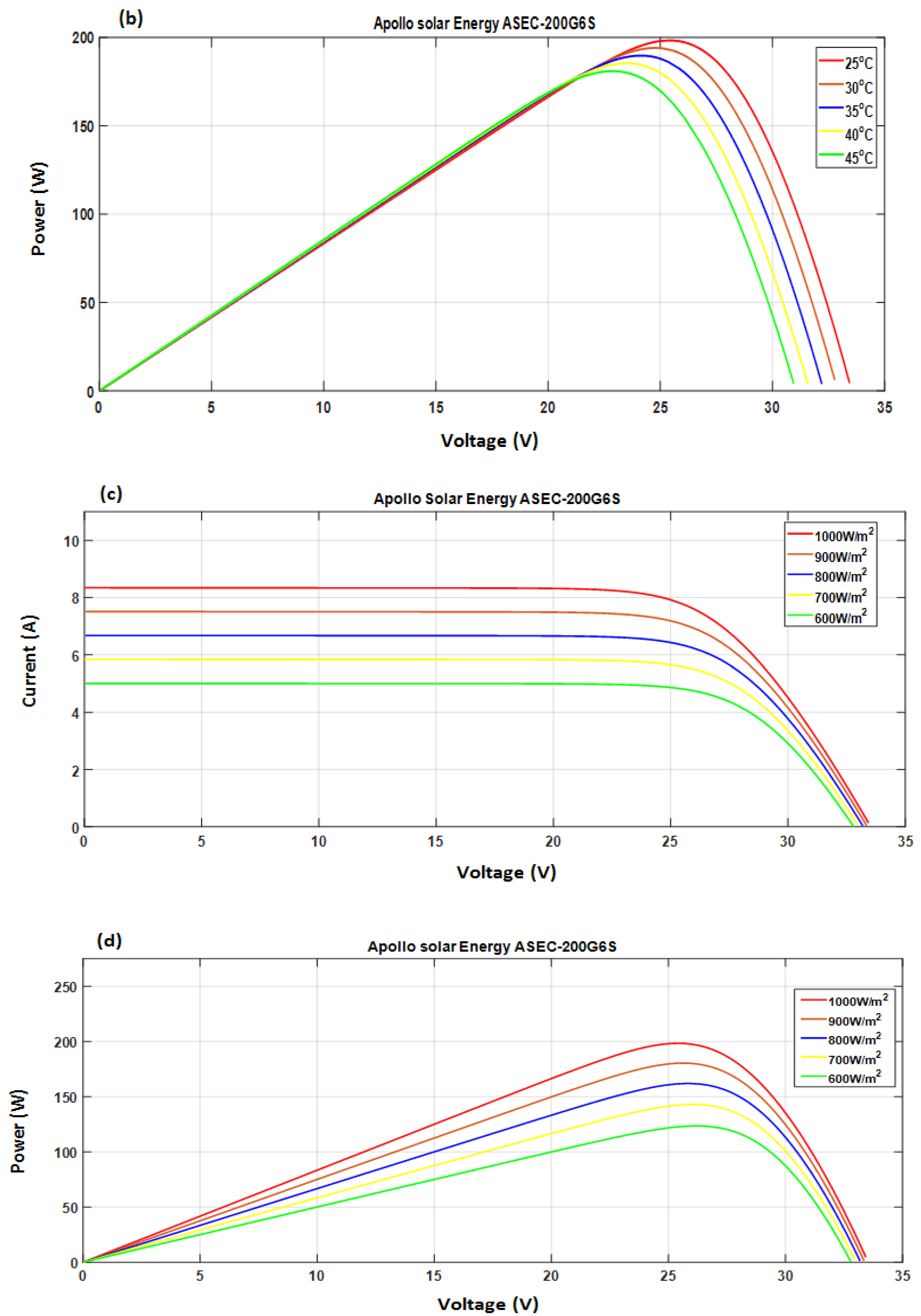


| Parameters/Characteristics | Values |
|---|---|
| Weight | 14.6 g |
| Torque | 2.5 kg.cm (4.8 v) 2.8 kg.cm (6.0 v) |
| Operating speed | Operating: 0.11 s/60 degree (4.8 v) 0.09 s/60 degree (6.0 v) |
| Size | 22.5 × 12.2 × 35 mm |
| Parameters/Characteristics | Values |
|---|---|
| 200 | |
| 25.38 | |
| 7.89 | |
| 33.53 | |
| 8.24 | |
| [%/K] | 0.136 |
| [%/K] | −0.37101 |
| [%/K] | −0.47 |
| Length x width x height [mm] | 1660 × 990 × 50 |
| Number of cells | 54 |
| Cell size [mm] | 156 × 156 |
| Cell material | Monocrystalline Si stalling |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baouche, F.Z.; Abderezzak, B.; Ladmi, A.; Arbaoui, K.; Suciu, G.; Mihaltan, T.C.; Raboaca, M.S.; Hudișteanu, S.V.; Țurcanu, F.E. Design and Simulation of a Solar Tracking System for PV. Appl. Sci. 2022, 12, 9682. https://doi.org/10.3390/app12199682
Baouche FZ, Abderezzak B, Ladmi A, Arbaoui K, Suciu G, Mihaltan TC, Raboaca MS, Hudișteanu SV, Țurcanu FE. Design and Simulation of a Solar Tracking System for PV. Applied Sciences. 2022; 12(19):9682. https://doi.org/10.3390/app12199682
Chicago/Turabian StyleBaouche, Fatima Zohra, Bilal Abderezzak, Abdennour Ladmi, Karim Arbaoui, George Suciu, Traian Candin Mihaltan, Maria Simona Raboaca, Sebastian Valeriu Hudișteanu, and Florin Emilian Țurcanu. 2022. "Design and Simulation of a Solar Tracking System for PV" Applied Sciences 12, no. 19: 9682. https://doi.org/10.3390/app12199682
APA StyleBaouche, F. Z., Abderezzak, B., Ladmi, A., Arbaoui, K., Suciu, G., Mihaltan, T. C., Raboaca, M. S., Hudișteanu, S. V., & Țurcanu, F. E. (2022). Design and Simulation of a Solar Tracking System for PV. Applied Sciences, 12(19), 9682. https://doi.org/10.3390/app12199682









