Fractance with Tunable Fractor’s Order for Microwave Circuit Applications
Abstract
Featured Application
Abstract
1. Introduction
2. State of Art of Fractances’ Implementation
2.1. Equivalent Passive Circuits
2.2. Equivalent Active Circuits
2.3. Single Componet
3. Fractances with Tunable Fractor’s Order
3.1. Equivalent Circuit through Rational Approximation
3.2. Tuning of the Fractor’s Order
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Caputo, M. Linear model of dissipation whose Q is almost frequency independent—II. Geophys. J. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley & Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus: Theory and Application of Differentiation and Integration to Arbitrary Order; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Machado, J.A.T.; Kiryakova, V.; Mainardi, F. Kiryakova and F. Mainardi. Recent History of Fractional Calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef]
- Gutiérrez, R.E.; Rosário, J.M.; Machado, J.A.T. Fractional Order Calculus: Basic Concepts and Engineering Applications. Math. Probl. Eng. 2010, 2010, 375858. [Google Scholar] [CrossRef]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 2003, 3413–3442. [Google Scholar] [CrossRef]
- Dalir, M.; Bashour, M. Applications of Fractional Calculus. Appl. Math. Sci. 2010, 4, 1021–1032. [Google Scholar]
- Dattoli, G.; Ricci, P.; Cesarano, C.; Vazquez, L. Special polynomials and fractional calculus. Math. Comput. Model. 2003, 37, 729–733. [Google Scholar] [CrossRef]
- Assante, D.; Cesarano, C.; Fornaro, C.; Vazquez, L. Higher order and fractional diffusive equations. J. Eng. Sci. Technol. Rev. 2015, 8, 202–204. [Google Scholar] [CrossRef]
- Soltan, A.; Radwan, A.; Soliman, A.M. Fractional order filter with two fractional elements of dependant orders. Microelectron. J. 2012, 43, 818–827. [Google Scholar] [CrossRef]
- Petras, I. Fractional-order nonlinear systems: Modeling, analysis and simulation. Springer Sci. Bus. Media 2011. [Google Scholar]
- Petras, I. Fractional-order memristor-based Chua’s circuit. IEEE Trans. Circuits Syst. II: Express Briefs 2010, 57, 975–979. [Google Scholar] [CrossRef]
- Nakagawa, M.; Sorimachi, K. Basic characteristics of a fractance device. IEICE Trans. Fundam. Electron. Commun. Comput. Sci. 1992, 75, 1814–1819. [Google Scholar]
- Elwakil, A.S. Fractional-order circuits and systems: An emerging interdisciplinary research area. IEEE Circuits Syst. Mag. 2010, 10, 40–50. [Google Scholar] [CrossRef]
- DSierociuk, D.; Podlubny, I.; Petras, I. Experimental evidence of variable-order behavior of ladders and nested ladders. IEEE Trans. Control Syst. Technol. 2013, 21, 459–466. [Google Scholar] [CrossRef]
- Tanwar, R.; Sanjay, K. Analysis & design of fractance based fractional order filter. Int. J. 2013, 1, 113–122. [Google Scholar]
- Upadhyay, D.K.; Mishra, S.K. Realization of fractional order microwave low pass filter. Int. J. Microw. Opt. Technol. 2015, 10, 260–265. [Google Scholar]
- Hamed, E.M.; AbdelAty, A.M.; Said, L.A.; Radwan, A.G. Effect of different approximation techniques on fractional-order KHN filter design. Circuits Syst. Signal Process. 2018, 37, 5222–5252. [Google Scholar] [CrossRef]
- Radwan, A.G.; Elwakil, A.S.; Soliman, A.M. On the generalization of second-order filters to the fractional-order domain. J. Circuits Syst. Comput. 2009, 18, 361–386. [Google Scholar] [CrossRef]
- Abdelouahab, M.S.; Hamri, N. Fractional-order hybrid optical system and its chaos control synchronization. EJTP 2014, 11, 49–62. [Google Scholar]
- Tavazoei, M.S.; Haeri, M. Synchronization of chaotic fractional-order systems via active sliding mode controller. Phys. A Stat. Mech. Its Appl. 2008, 387, 57–70. [Google Scholar] [CrossRef]
- Shahiri, M.; Ghaderi, R.; N., A.R.; Hosseinnia, S.H.; Momani, S. Chaotic fractional-order Coullet system: Synchronization and control approach. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 665–674. [Google Scholar] [CrossRef]
- Adhikary, A.; Khanra, M.; Pal, J.; Biswas, K. Realization of Fractional Order Elements. INAE Lett. 2017, 2, 41–47. [Google Scholar] [CrossRef]
- Clovis, F.; Daou, A.Z.; Moreau, X. Synthesis and implementation of non integer integrators using RLC. Int. J. Electron. 2009, 12, 1207–1223. [Google Scholar]
- Radwan, A.G.; Salama, K.N. Passive and active elements using fractional circuit. IEEE Trans. Circuits Syst. I Regul. Pap. 2011, 58, 2388–2397. [Google Scholar] [CrossRef]
- Krishna, B.T.; Reddy, K. Active and Passive Realization of Fractance Device of Order ½. Act. Passiv. Electron. Compon. 2008, 2008, 369421. [Google Scholar] [CrossRef]
- Sierociuk, D.; Macias, M.; Malesza, W. Analog Modeling of Fractional Switched Order Derivative Using Different Switching Schemes. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 394–403. [Google Scholar] [CrossRef]
- Sugi, M.; Hirano, Y.; Miura, Y.F.; Saito, K. Simulation of fractal immittance by analog circuits: An approach to the optimized circuits. IEICE Trans. Fundam. 1999, 82, 205–209. [Google Scholar]
- Tarunkumar, H.; Ranjan, A.; Kumar, R.; Subrahmanyam, P. Operational Amplifier-Based Fractional Device of Order s±0.5. In Proceeding of International Conference on Intelligent Communication, Control and Devices 2017; Springer: Singapore, 2017; pp. 151–159. [Google Scholar]
- Mondal, D.; Biswas, K. Packaging of single component fractional order element. IEEE Trans. Device Mater. Reliab. 2013, 13, 73–80. [Google Scholar] [CrossRef]
- Elshurafa, A.M.; Almadhoun, M.N.; Salama, K.N.; Alshareef, H.N. Microscale electrostatic fractional capacitors using reduced graphene oxide percolated polymer composites. Appl. Phys. Lett. 2013, 102, 232901. [Google Scholar] [CrossRef]
- Caponetto, R.; Graziani, S.; Pappalardo, F.L.; Sapuppo, F. Experimental characterization of ionic polymer metal composite as a novel fractional order element. Adv. Math. Phys. 2013, 2003, 953695. [Google Scholar] [CrossRef]
- Adhikary, A.; Sen, S.; Biswas, K. Design and hardware realization of a tunable fractional-order series resonator with high quality factor. Circuits Syst. Signal Process. 2017, 36, 3457–3476. [Google Scholar] [CrossRef]
- Wu, M.; Wang, C.; Cai, N.; Meng, W. Fractance circuit design based on a method of constructing the rational approximation function in the form of factorization. In Proceedings of the Control and Decision Conference (IEEE CCDC), Qingdao, China, 23–25 May 2015; pp. 6011–6016. [Google Scholar]
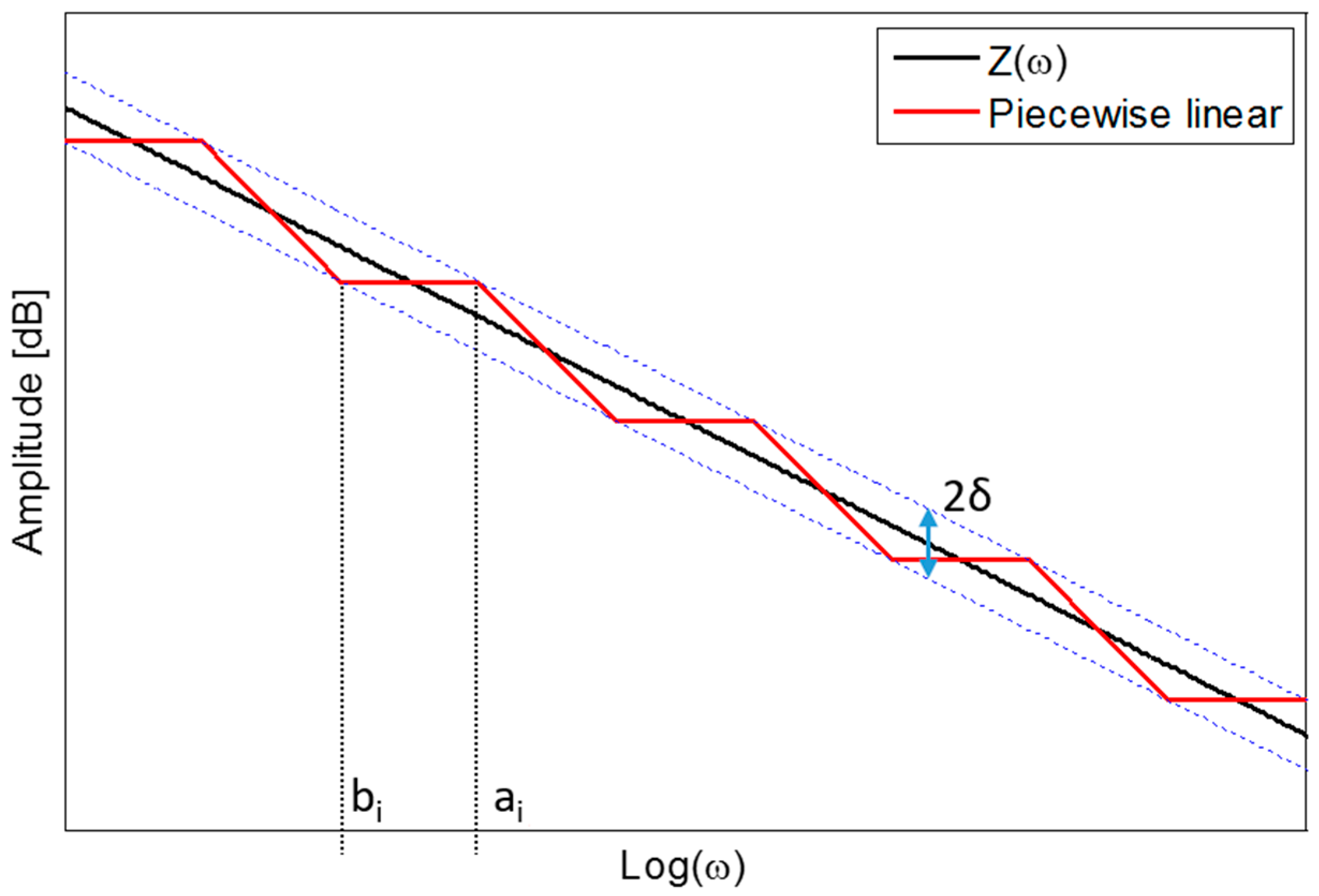
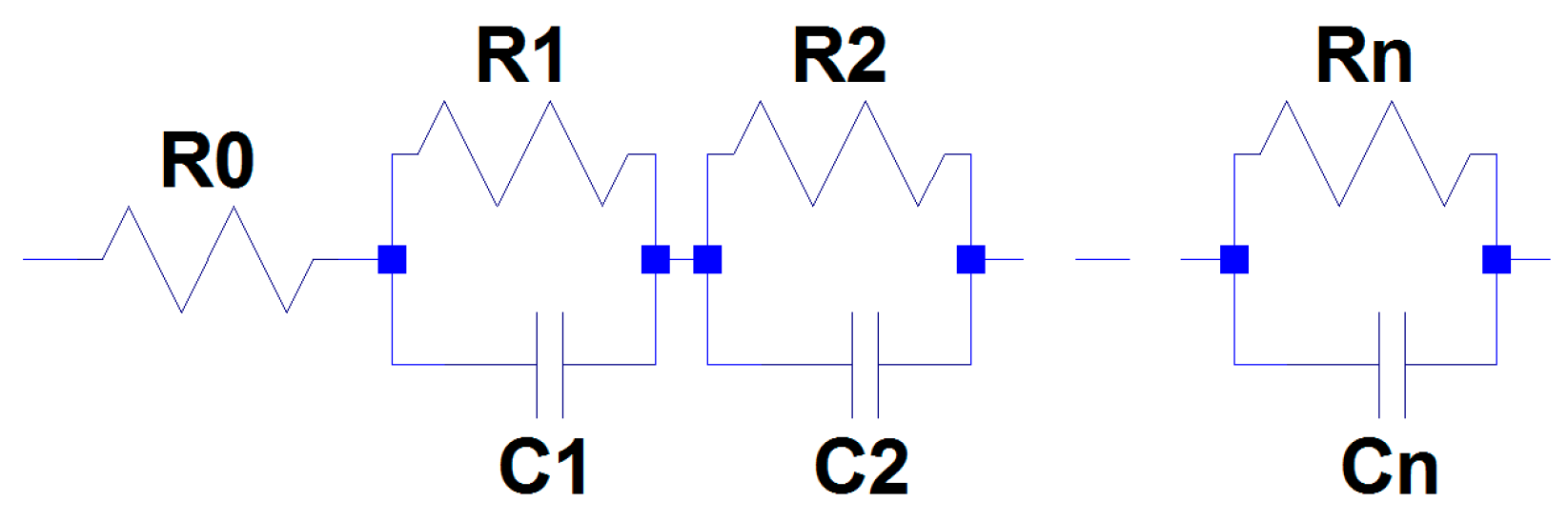
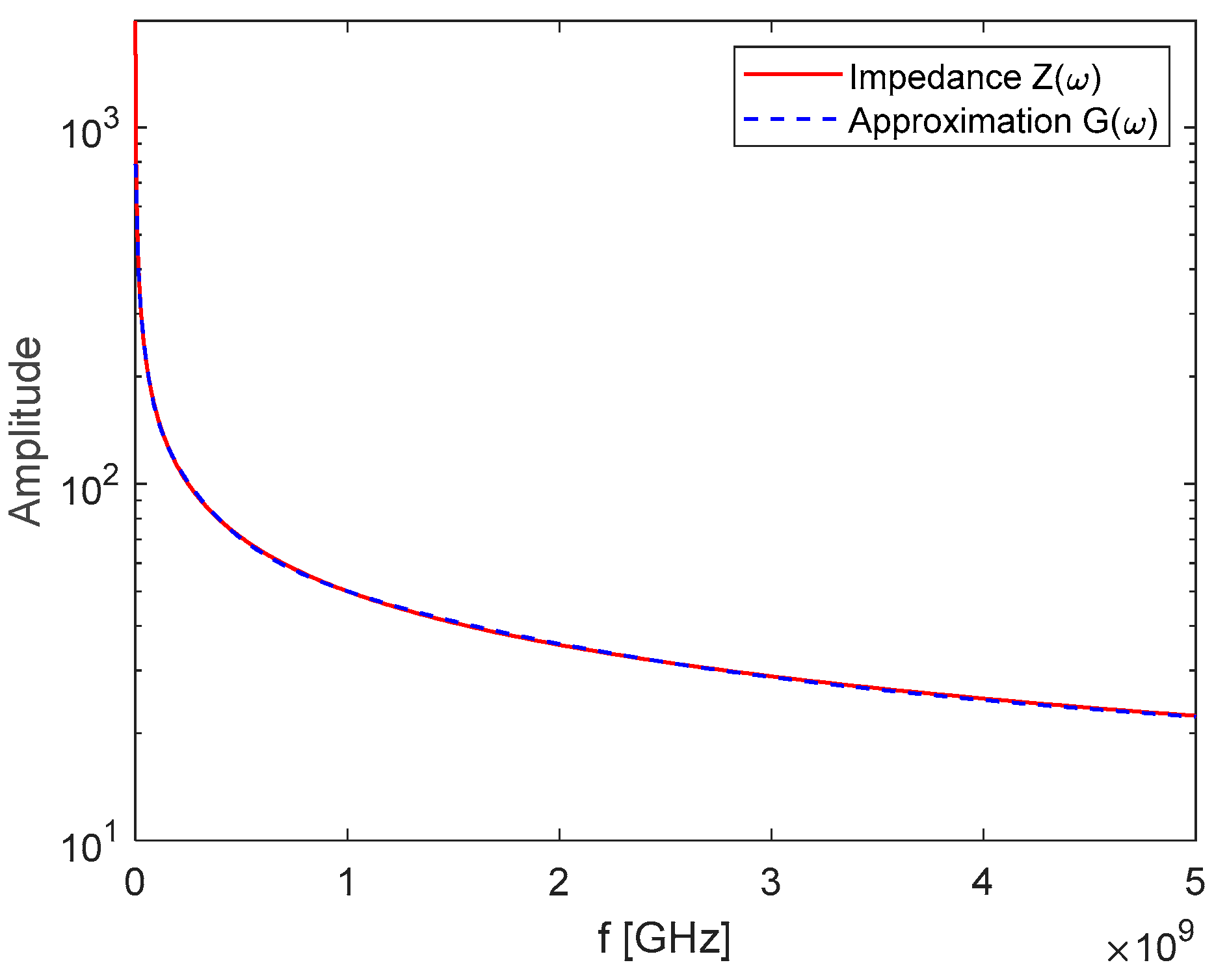

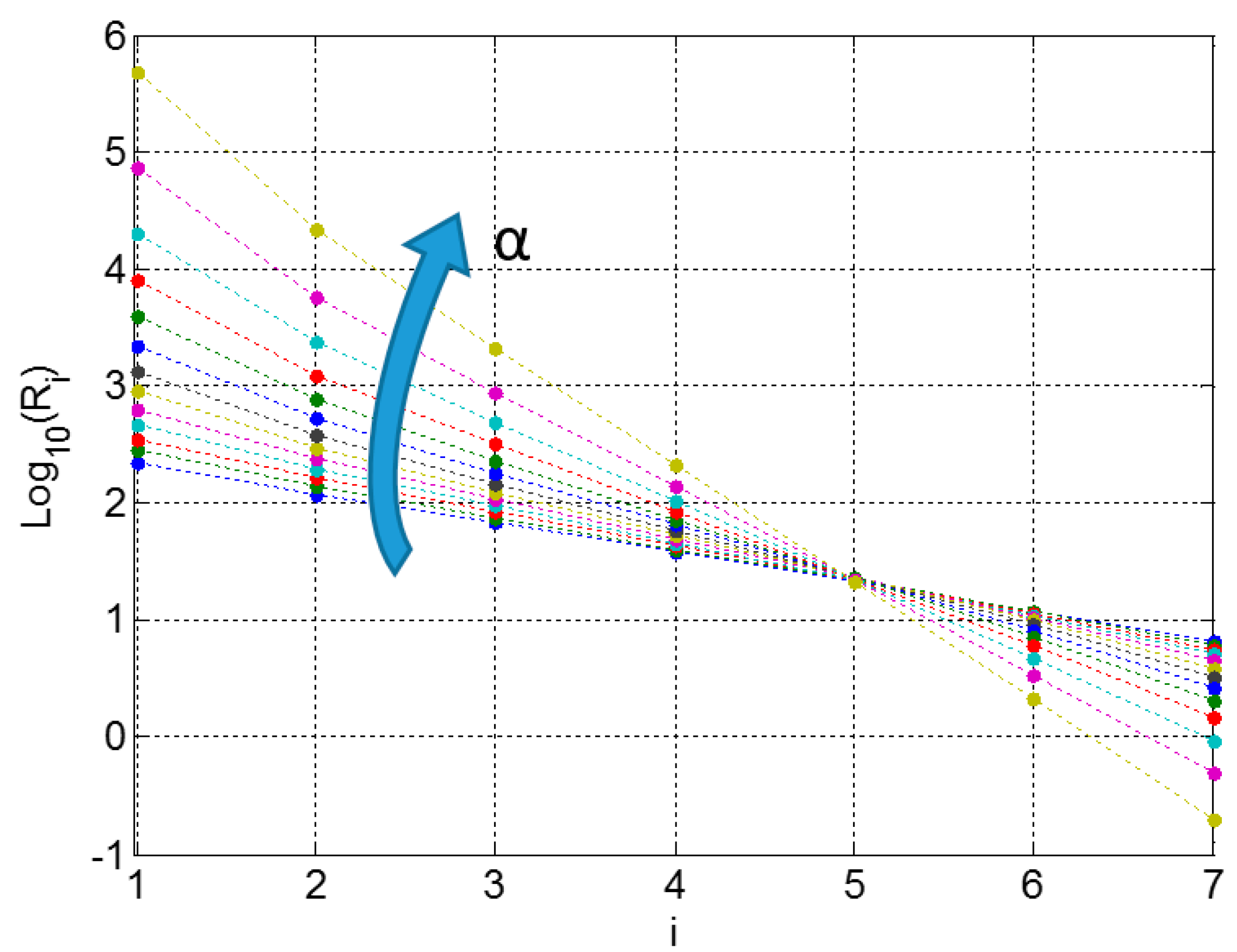


| i | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|---|
| R [Ω] | 3.15 | 541 | 154 | 58.9 | 23.2 | 9.10 | 3.26 |
| C [pF] | - | 0.293 | 0.163 | 0.068 | 0.027 | 0.011 | 0.005 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Assante, D. Fractance with Tunable Fractor’s Order for Microwave Circuit Applications. Appl. Sci. 2022, 12, 10108. https://doi.org/10.3390/app121910108
Assante D. Fractance with Tunable Fractor’s Order for Microwave Circuit Applications. Applied Sciences. 2022; 12(19):10108. https://doi.org/10.3390/app121910108
Chicago/Turabian StyleAssante, Dario. 2022. "Fractance with Tunable Fractor’s Order for Microwave Circuit Applications" Applied Sciences 12, no. 19: 10108. https://doi.org/10.3390/app121910108
APA StyleAssante, D. (2022). Fractance with Tunable Fractor’s Order for Microwave Circuit Applications. Applied Sciences, 12(19), 10108. https://doi.org/10.3390/app121910108






