SimEx: A Tool for the Rapid Evaluation of the Effects of Explosions
Abstract
:1. Introduction
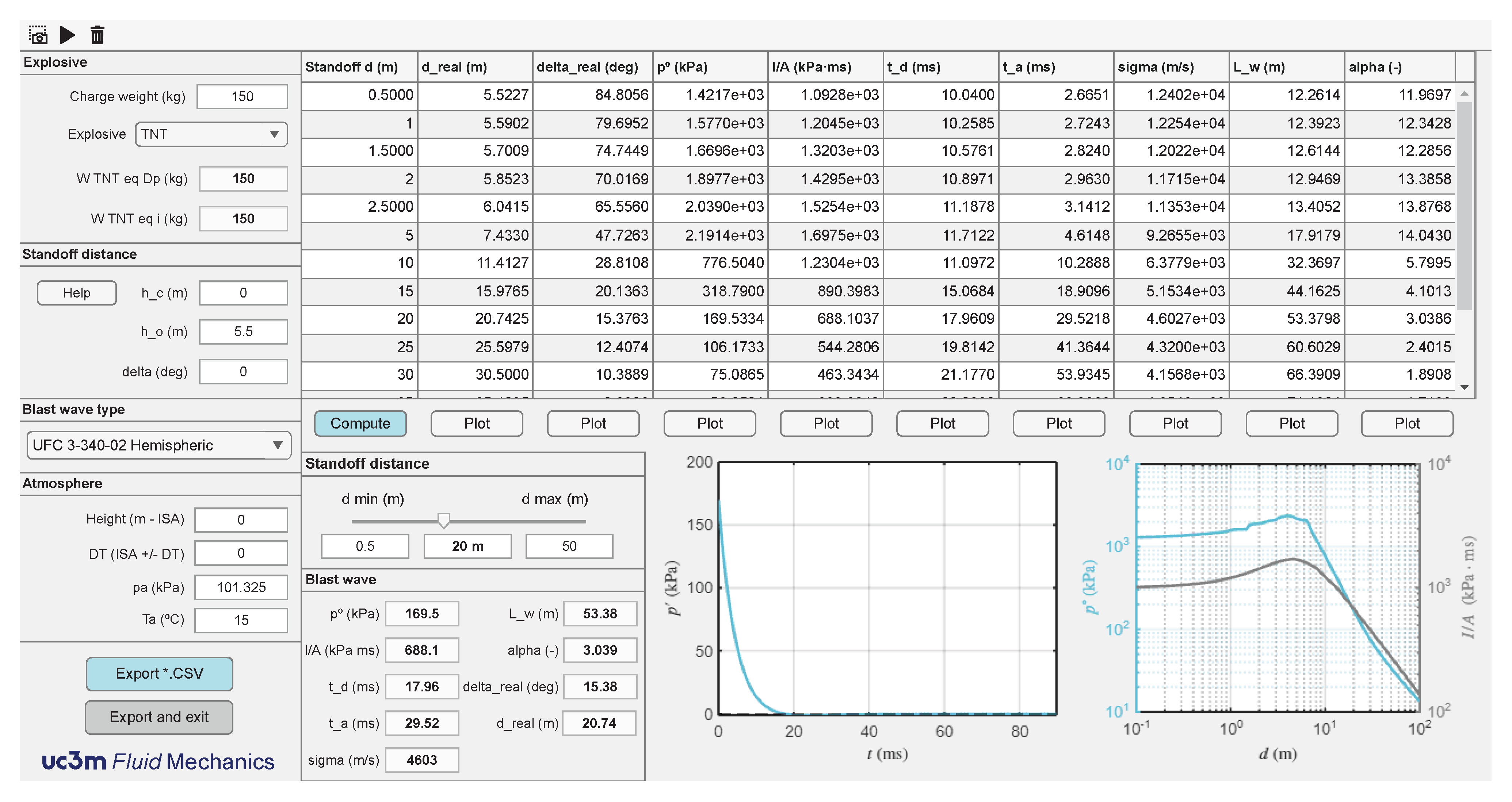
2. SimEx Capabilities
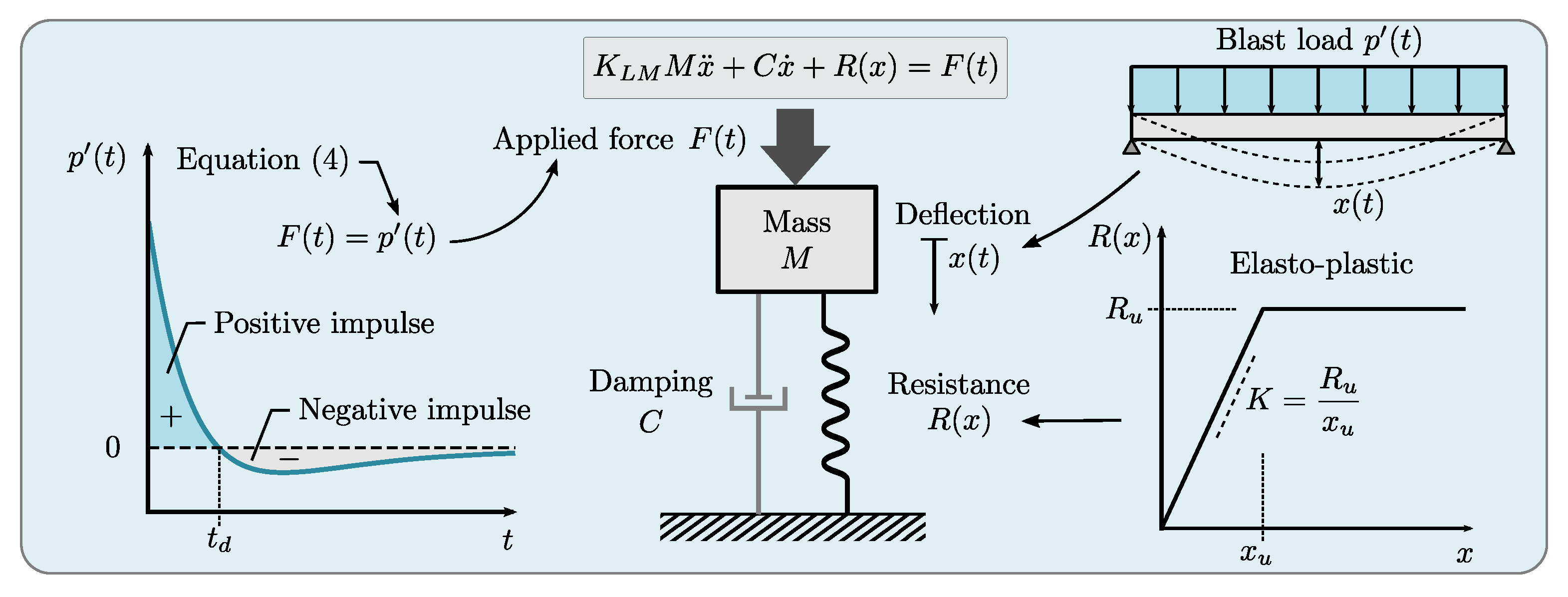
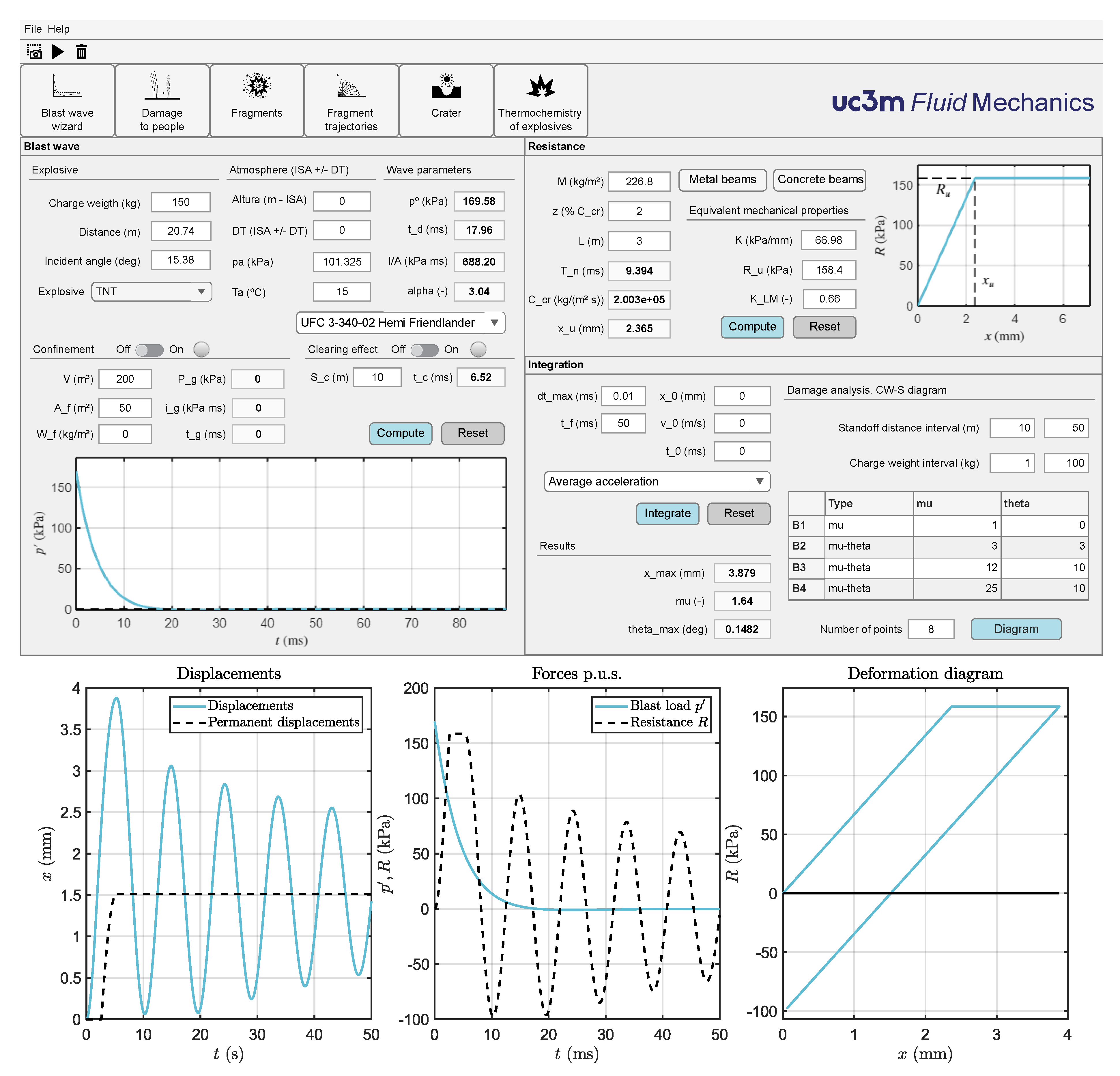
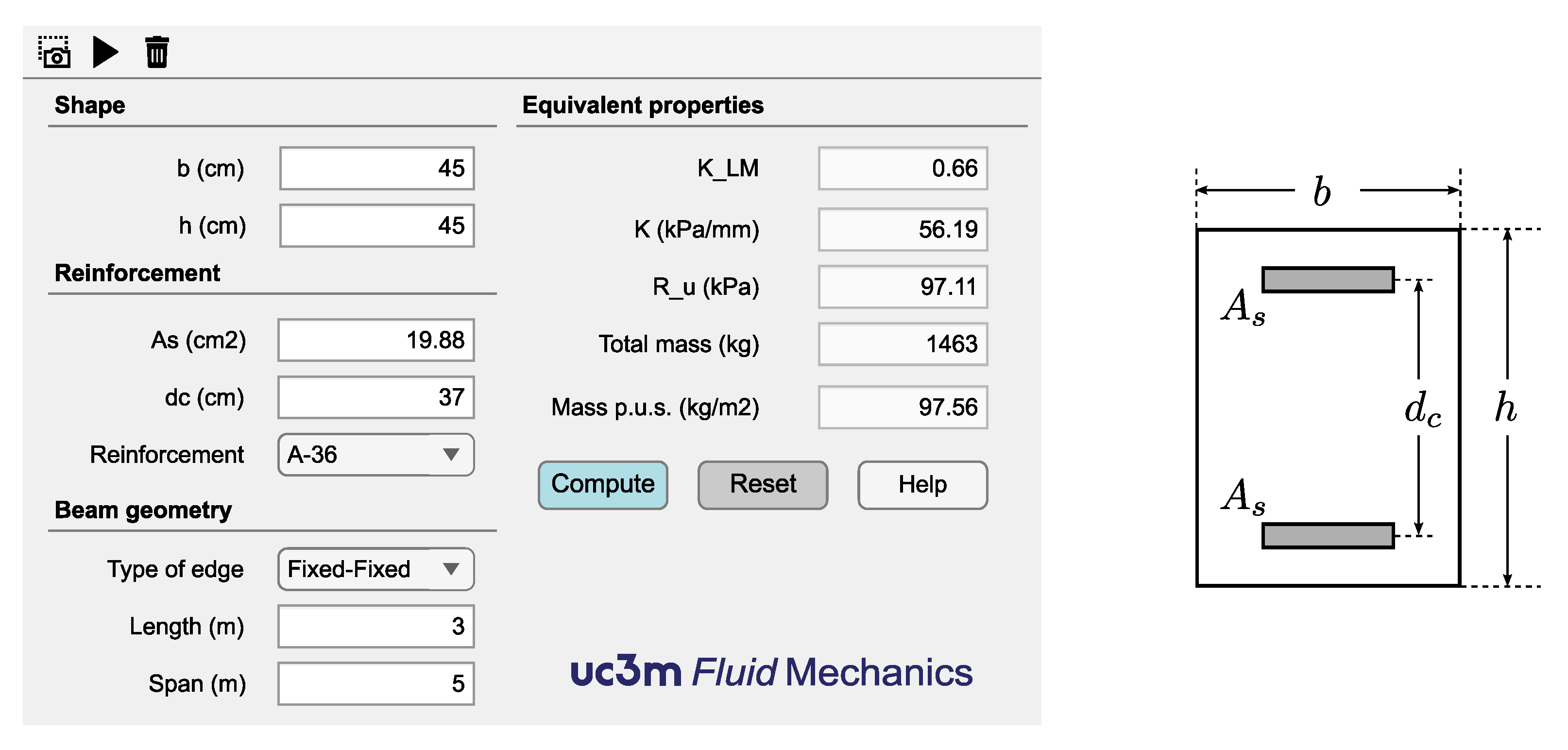
2.1. Single-Degree-of-Freedom System Analysis
2.1.1. Forcing Term
2.1.2. Resistance Term
2.1.3. Numerical Integration
2.1.4. Post-Processing
2.2. Other Calculation Assistants
2.2.1. Assistant for the Calculation of the Thermodynamic Properties of Explosives
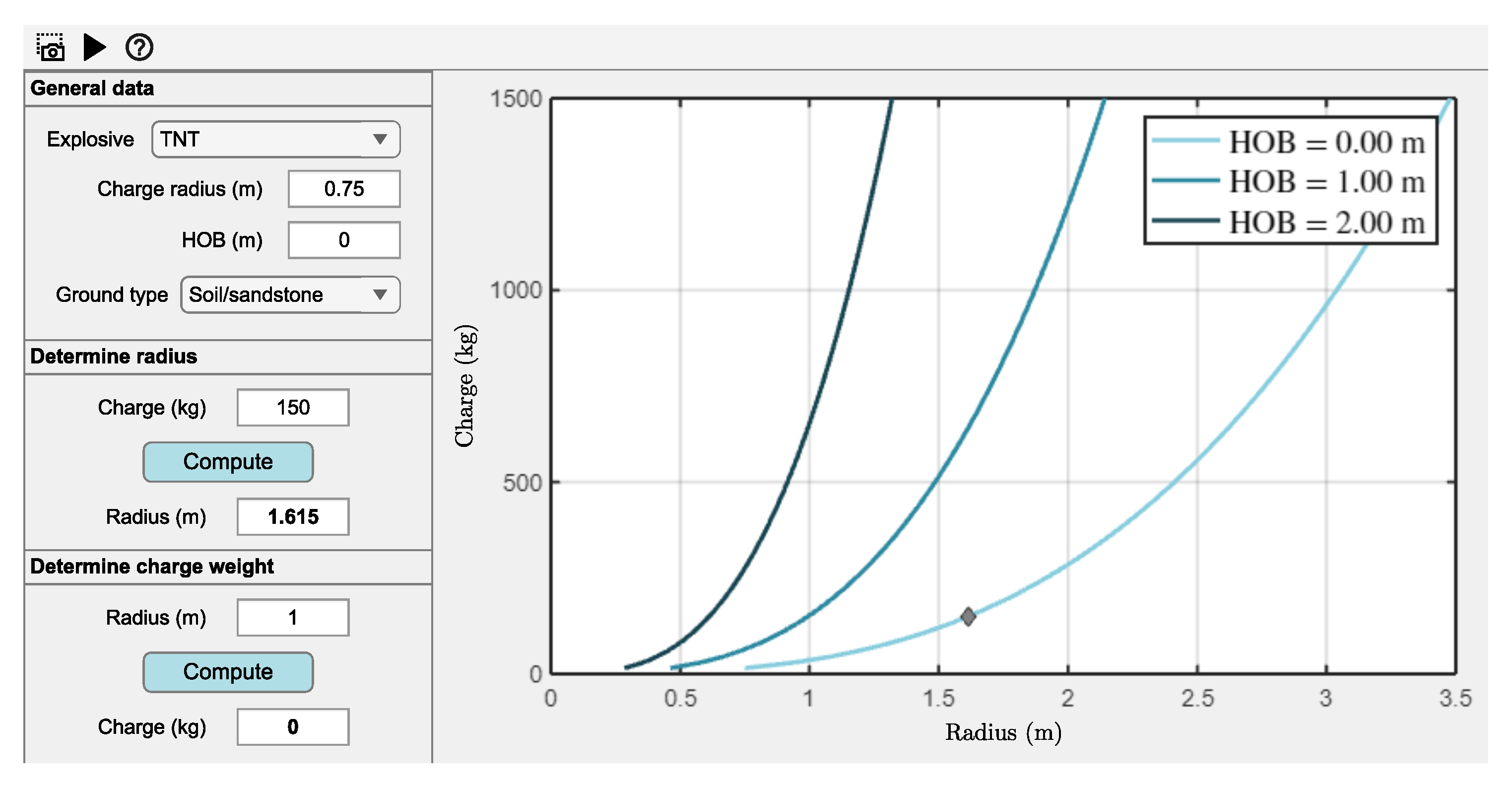
2.2.2. Crater
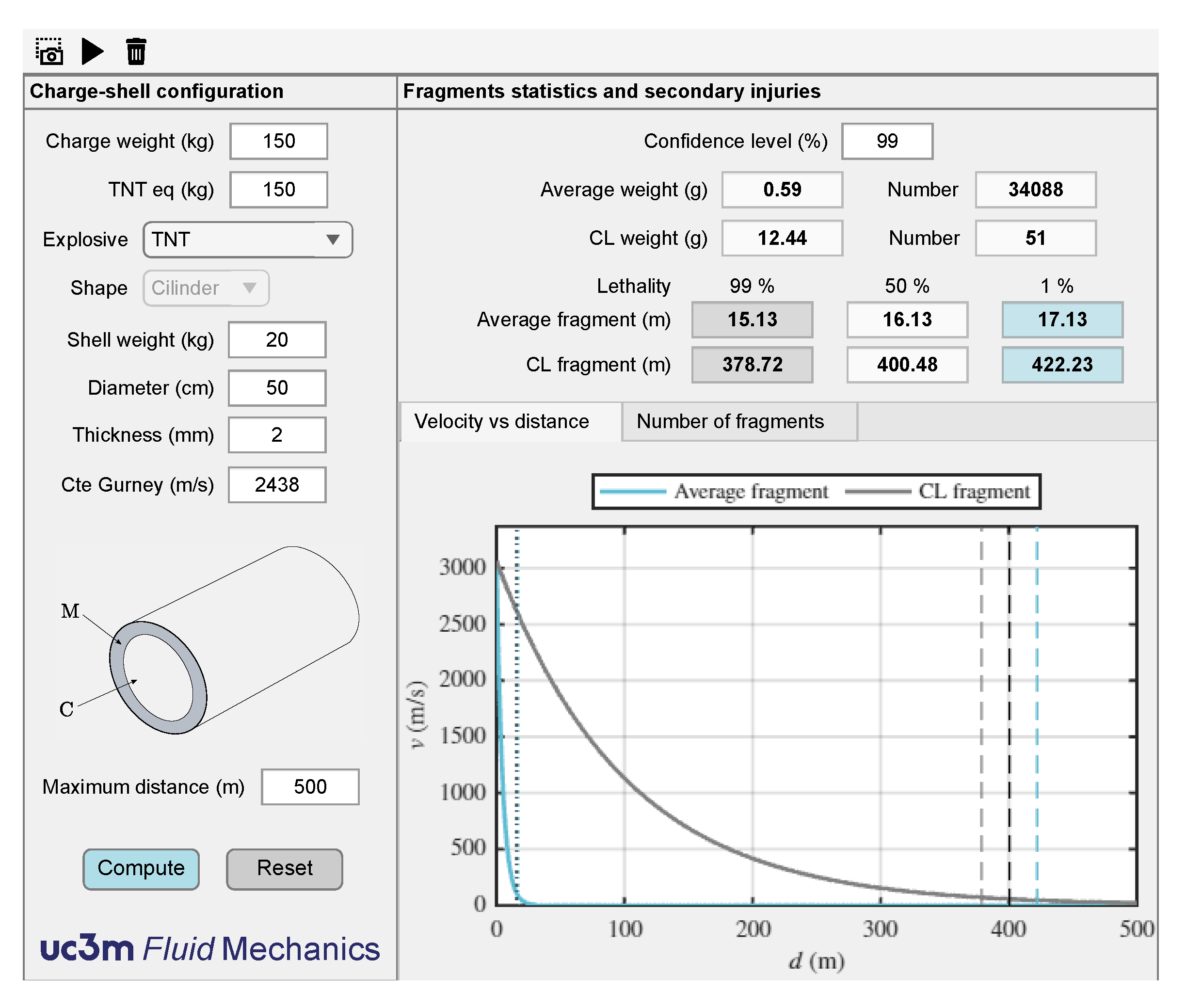
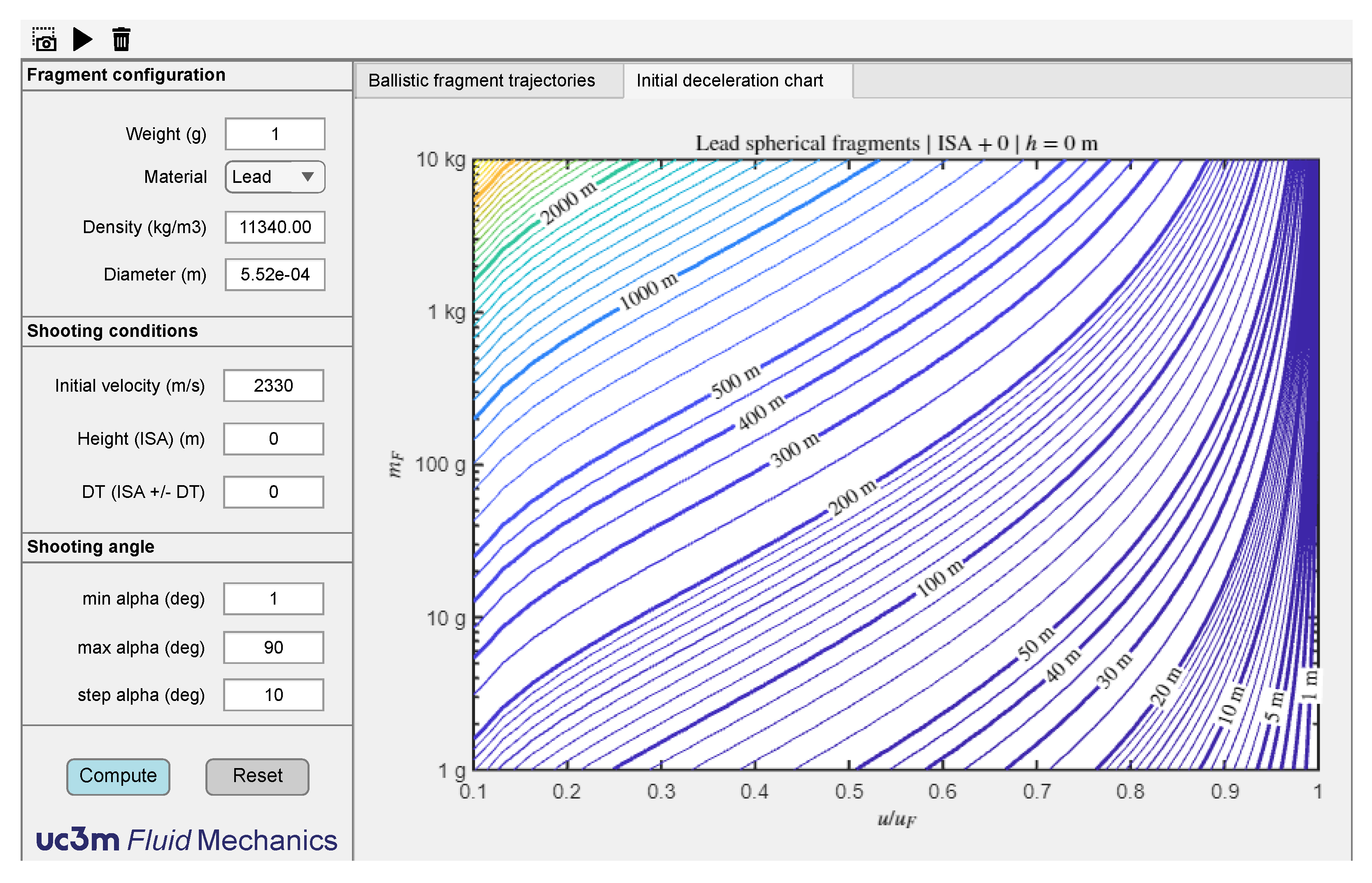
2.2.3. Primary Fragments
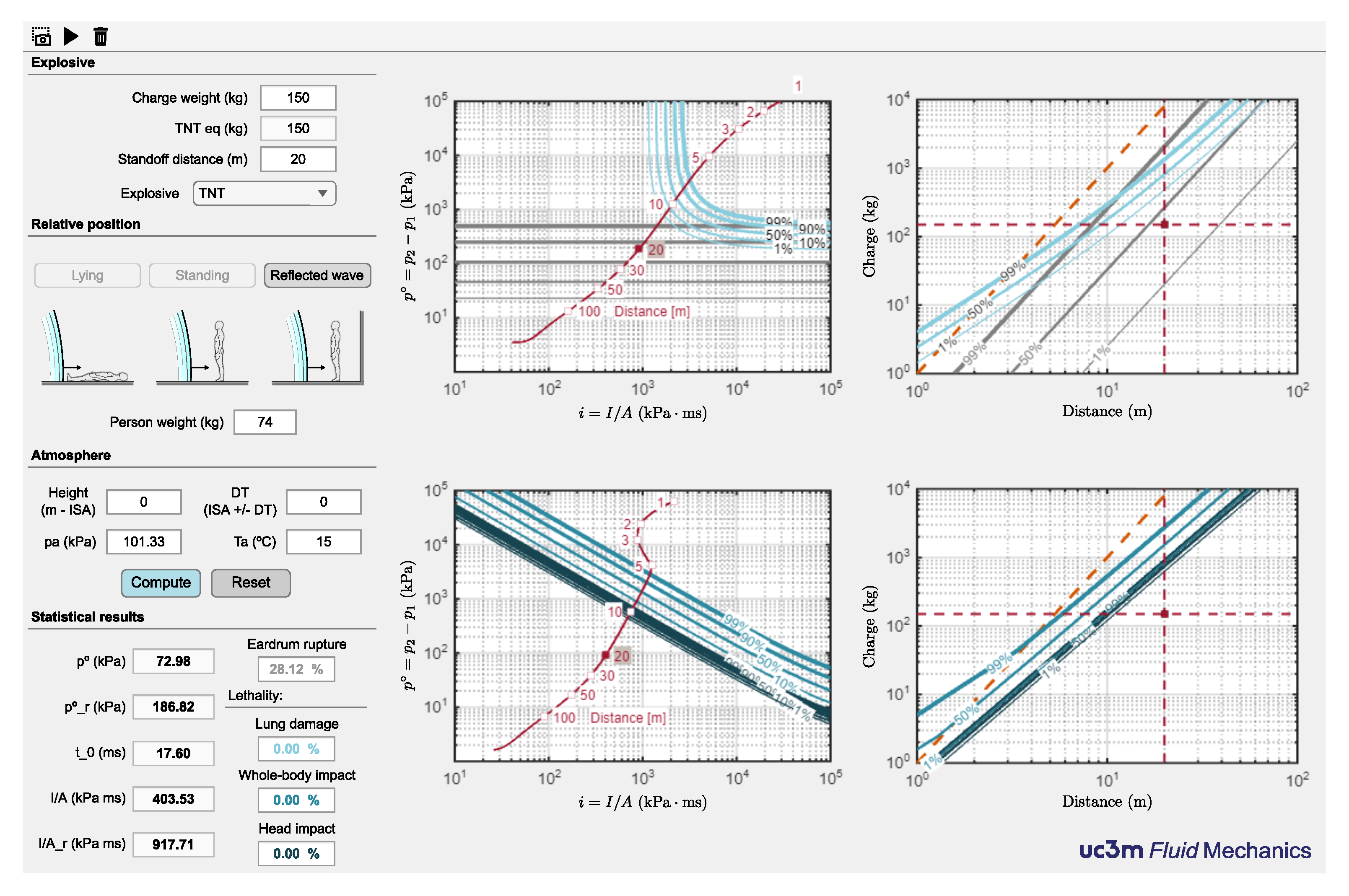
2.2.4. Damage to People
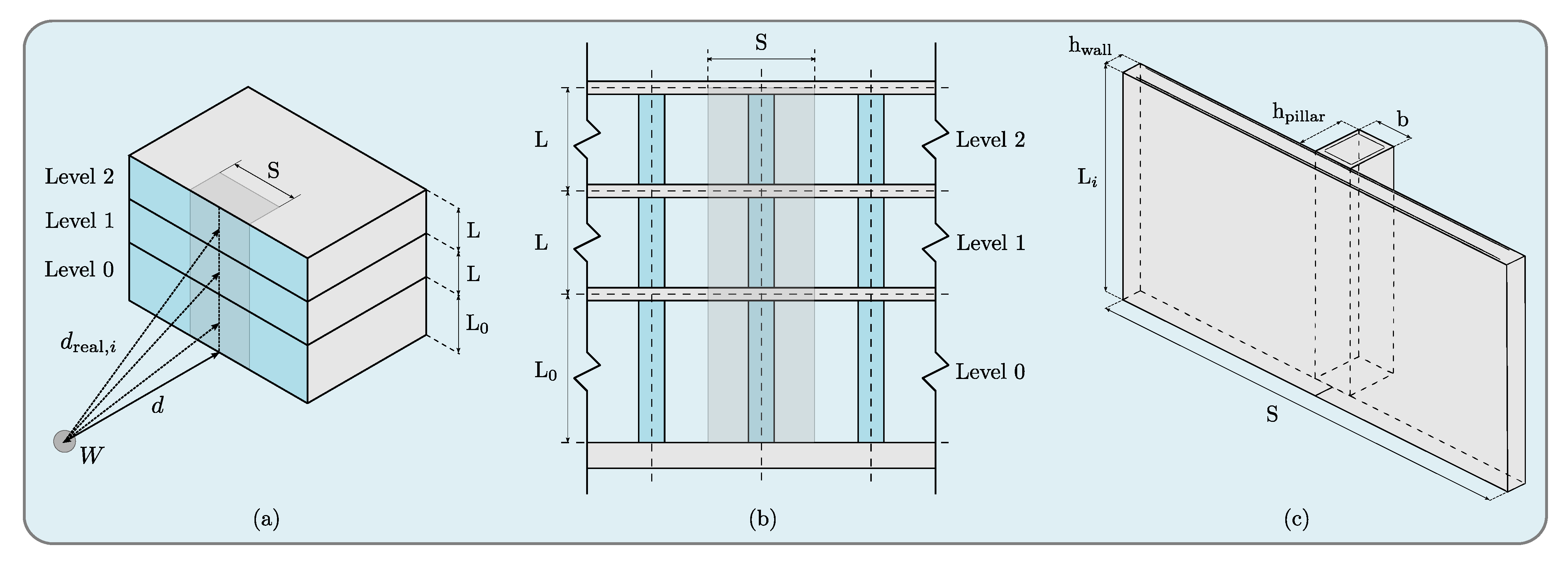
3. Example of Application: Façade of a Building under Blast Loading
3.1. Incident Load
3.2. Estimation of the Equivalent SDOF System Response
3.3. SDOF System Integration and CW–S Damage Diagrams
3.4. Crater, Fragments, and Damage to People
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowldgments
Conflicts of Interest
SimEx License & Distribution
Abbreviations
| BKW | Becker–Kistiakowsky–Wilson EoS |
| CL | Confidence Level |
| CT | Combustion Toolbox |
| CUGC | Centro Universitario de la Guardia Civil |
| CW–S | Charge Weight–Standoff |
| EoS | Equation of State |
| GUI | Graphical User Interface |
| H9 | Heuzé EoS |
| HOB | Height of Burst |
| IED | Improvised Explosive Device |
| ISA | International Standard Atmosphere |
| LOP | Level of Protection |
| PDC | Protective Design Center |
| SDOF | Single Degree of Freedom |
| SEDEX-NRBQ | Explosive Ordnance Disposal (EOD) and CBRN Defense Service |
| UC3M | University Carlos III of Madrid |
| UFC | Unified Facilities Criteria |
| US | United States |
| USACE | United States Army Corps of Engineers |
References
- Kinney, G.F.; Graham, K.J. Explosive Shocks in Air, 2nd ed.; Springer: New York, NY, USA, 1985. [Google Scholar]
- Brode, H.L. Numerical solutions of spherical blast waves. J. Appl. Phys. 1955, 26, 766–775. [Google Scholar] [CrossRef]
- Grady, D. Fragmentation of Ring and Shells, the Legacy of N.F. Mott; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Ambrosini, R.D.; Luccioni, B.M. Craters produced by explosions on the soil surface. J. Appl. Mec. 2006, 73, 890–900. [Google Scholar] [CrossRef]
- Akhavan, J. The Chemistry of Explosives, 3rd ed.; Royal Society of Chemistry: Cambridge, UK, 2011. [Google Scholar]
- Cooper, P.W. Explosives Enginering; Wiley-VCH, Inc.: New York, NY, USA, 1996. [Google Scholar]
- Meyer, R.; Köhler, J.; Homburg, A. Explosives, 6th ed.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2007. [Google Scholar]
- Ngo, T.; Mendis, P.; Gupta, A.; Ramsay, J. Blast loading and blast effects on structures—An overview. Electron. J. Struct. Eng. 2007, 1, 76–91. [Google Scholar] [CrossRef]
- TNO Green Book Methods for the Determination of Possible Damage to People and Objects Resulting from Releases of Hazardous Materials (CPR 16E); CIP-data of the Royal Library: The Hage, The Netherlands, 1992.
- START. National Consortium for the Study of Terrorism and Responses to Terrorism. Global Terror-Ism Database. 2017. Available online: https://www.start.umd.edu/gtd (accessed on 3 September 2022).
- US Department of Homeland Security (DHS). Reference Manual to Mitigate Potential Terrorist At-Tacks Against Buildings, 2nd ed.; Federal Emergency Management Agency: Washington, DC, USA, 2011. [Google Scholar]
- Luccioni, B.; Ambrosini, D.; Danesi, R. Blast load assessment using hydrocodes. Eng. Struct. 2006, 28, 1736–1744. [Google Scholar] [CrossRef]
- Anderson, C.E., Jr. An overview of the theory of hydrocodes. Int. J. Impact Eng. 1987, 5, 33–59. [Google Scholar] [CrossRef]
- Han, Y.; Liu, H. Finite element simulation of medium-range blast loading using LS-DYNA. Shock Vib. 2015, 2015, 631493. [Google Scholar] [CrossRef]
- Protective Design Center Technical Report PDC-TR 06-08; Single Degree of Freedom Structural Response Limits for Antiterrorism Design; US Army Corps of Engineers: Washington, DC, USA, 2008.
- Unified Facilities Criteria (UFC) 3-340-02; Structures to Resist the Effects of Accidental Explosions; US Department of Defense: Washington, DC, USA, 2008.
- Baker, W.E. Explosions in Air; University of Texas Press: Austin, TX, USA, 1973. [Google Scholar]
- Kingery, C.N.; Bulmash, G. Airblast Parameters from TNT Spherical Air Burst and Hemispherical Surface Burst; US Technical Report ARBRL-TR-02555; Aberdeen Proving Ground, Ballistic Research Laboratories (BRL): Aberdeen, MD, USA, 1984. [Google Scholar]
- Needham, C.E. Blast Waves; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Protective Design Center Software, US Army Corps of Engineers. Available online: https://www.nwo.usace.army.mil/About/Centers-of-Expertise/Protective-Design-Center/PDC-Software (accessed on 3 September 2022).
- Sánchez-Monreal, J.; Vera, M. SimEx: SIMulador de EXplosiones mediante sistemas SDOF. In Proceedings of the III Na-tional Congress of R&D in Defense and Security (DESEi+d 2015), Naval Military School of Marín, Pontevedra, Spain, 19–20 November 2015; pp. 993–1000. [Google Scholar]
- Sánchez-Monreal, J.; Vera, M. SIMulador de EXplosiones mediante sistemas SDOF (SimEx): Desarrollo de la aplicación y nuevas funcionalidades. In Proceedings of the IV National Congress of R&D in Defense and Security (DESEi+d 2016), University Defense Center, General Air Academy, San Javier, Spain, 16–18 November 2016; pp. 949–955. Available online: https://www.tecnologiaeinnovacion.defensa.gob.es/.../Actas_DESEi+d2016_1.pdf" (accessed on 3 September 2022).
- Sánchez-Monreal, J.; Vera, M. SimEx: Una herramienta para la evaluación rápida de los efectos de explosiones. Bol. Obs. Tecnol. Def. 2017, 55, 23–27. Available online: https://publicaciones.defensa.gob.es/.../bot_55.pdf (accessed on 3 September 2022).
- Cuadra, A.; Huete, C.; Vera, M. Combustion Toolbox: A MATLAB-GUI Based Open-Source Tool for Solving Gaseous Combustion Problems (v0.9.7). Zenodo: Geneve, Switzerland, 2022.
- Ambrosini, R.D.; Luccioni, B.M.; Danesi, R.F.; Riera, J.D.; Rocha, M.M. Size of craters produced by explo-sive charges on or above the ground surface. Shock Waves 2002, 12, 69–78. [Google Scholar] [CrossRef]
- Mott, N.F. A Theory of Fragmentation of Shells and Bombs; AC4613; Ministry of Supply: Great Britain, UK, 1943. [Google Scholar]
- Mott, N.F. Fragmentation of H.E. Shells: A Theoretical Formula for the Distribution of Weights of Fragments; AC3642; Ministry of Supply: Great Britain, UK, 1943. [Google Scholar]
- Mott, N.F. Fragmentation of shell cases. Proc. R. Soc. 1947, A189, 300–308. [Google Scholar]
- Sánchez-Monreal, J.; Llamazares-Mendo, F.; Vera, M. Estimación de daños de explosiones sobre personas. In Proceedings of the V National Congress of R&D in Defense and Security (DESEi+d 2017), Toledo, Spain, 22–24 November 2017; pp. 1689–1697. Available online: https://publicaciones.defensa.gob.es/.../actas_v_congreso_id_2017.pdf (accessed on 3 September 2022).
- González Duperón, E.; Martínez Gil, M.; Giménez Francés, B.; González Ferradás, E. Analysis of effects of detonation of explosive substances on humans from characteristic curves explosive mass-distance from the origin of the explosion. Process. Saf. Prog. 2016, 35, 233–240. [Google Scholar] [CrossRef]
- Protective Design Center Technical Report PDC TR 06-01; Rev 1, Methodology manual for the Single-Degree-of-Freedom Blast Effects Design Spreadsheets (SBEDS); US Army Corps of Engineers: Washington, DC, USA, 2008.
- Biggs, J.M. Introduction to Structural Dynamics; McGraw-Hill College: New York, NY, USA, 1964. [Google Scholar]
- Brode, H.L. Blast wave from a spherical charge. Phys. Fluids 1959, 2, 217–229. [Google Scholar] [CrossRef]
- Needham, C.E.; Crepeau, J.E. The DNA Nuclear Blast Standard (1 kT); Report No. DNA 5648-T; Systems, Science and Software, Inc.: Alburquerque, Spain, 1981. [Google Scholar]
- Hopkinson, B. British Ordnance Board Minutes 13565; The National Archives: Richmond, UK, 1915. [Google Scholar]
- Sachs, R.G. The Dependence of Blast on Ambient Pressure and Temperature; Technical Report 466; Ballistic Research Laboratories: Aberdeen, MD, USA, 1944. [Google Scholar]
- ISO 2533:1975; Standard Atmosphere. International Organization for Standardization: Geneva, Switzerland, 1975.
- Gallo, E. Quasi static atmospheric model for aircraft trajectory prediction and flight simulation. arXiv 2021, arXiv:2101.10744. [Google Scholar]
- International Ammunition Technical Gudeline (IATG) 01.80 Formulae for Ammunition Management; UNODA Office for Disarmament Affairs: New York, NY, USA, 2013.
- Friedlander, F.G. The diffraction of sound pulses I. Diffraction by a semi-infinite plane. Proc. R. Soc. London Ser. Math. Phys. Sci. 1946, 186, 322–344. [Google Scholar]
- Euronorm 53–62; European Wide Flange Beams. CEN: Brussels, Belgium, 1993.
- Norma UNE 31-002-94; Cálculo de las Principales Características Teóricas de los Explosivos. Asociación Española de Normalización UNE: Biddeford, Maine, 1994.
- European Standard EN 13631-15; Explosives for Civil Use-High Explosives—Part 15: Calculation of the Thermodynamic Properties. UNE: Biddeford, Maine, 2005.
- Arnold, W.; Rottenkolber, E.; Hartmann, T. DRAGON—The German Thermo-Chemical Code Based on the Becker-Kistiakowsky-Wilson Equation of State. Propellants Explos. Pyrotech. 2022, e202100329. [Google Scholar] [CrossRef]
- Hobbs, M.L.; Baer, M.R. Nonideal thermoequilibrium calculations using a large product species data base. Shock Waves 1992, 2, 177–187. [Google Scholar] [CrossRef]
- Mader, C.L. Detonation Properties of Condensed Explosives Computed Using the Becker-Kistiakowsky-Wilson Equation of State; Report LA-2900; Los Alamos Scientific Laboratory: Los Alamos, NM, USA, 1963. [Google Scholar]
- Heuzé, O. Equations of state of detonation products: Influence of the repulsive intermolecular potential. Phys. Rev. A 1986, 34, 428–432. [Google Scholar] [CrossRef] [PubMed]
- López, L.M. Evaluación de la Energía de los Explosivos Mediante Modelos Termodinámicos de Detonación. Ph.D. Thesis, Universidad Politecnica de Madrid, ETSI Minas, Madrid, Spain, 2003. [Google Scholar]
- Sanchidrián, J.A.; López, L.M. Calculation of the energy of explosives with a partial reaction model. Comparison with cylinder test data. Propellants Explos. Pyrotech. 2006, 31, 24–32. [Google Scholar] [CrossRef]
- Cuadra, A.; Huete, C.; Vera, M. Desarrollo de un código termoquímico para la evaluación de las propiedades teóricas de explosivos (CT-EXPLO) y la estimación del rendimiento de motores cohete (CT-ROCKET). In Proceedings of the IX National Congress of R&D in Defense and Se-curity (DESEi+d 2022), Army’s “General Morillo” Base, Pontevedra, Spain, 15–17 November 2022. accepted. [Google Scholar]
- Kamlet, M.J.; Jacobs, S.J. Chemistry of detonations. I. A simple method for calculating detonation proper-ties of CHNO explosives. J. Chem. Phys. 1968, 48, 23–55. [Google Scholar] [CrossRef]
- Politzer, P.; Murray, J.S. Some perspectives on estimating detonation properties of C, H, N, O compounds. Cent. Eur. J. Energetic Mater. 2011, 8, 209–220. [Google Scholar]
- Beveridge, A. Forensic Investigation of Explosions, 2nd ed.; CRC Press: Boca Raton, FL, USA; Tyalor & Francis Group: Boca Raton, FL, USA, 2012. [Google Scholar]
- Westine, P.S. Explosive cratering. J. Terramechanics 1970, 7, 9–19. [Google Scholar] [CrossRef]
- Baker, W.E.; Westine, P.S.; Dodge, F.T. Similarity Methods in Engineering Dynamics: Theory and Practice of Scale Modeling; Fundamental Studies in Engineering No. 12; Elsevier Science Limited: Amsterdam, The Netherlands, 1991. [Google Scholar]
- Gurney, R.W. The Initial Velocities of Fragments from Bombs, Shells and Grenades; Report No 405; Aberdeen Proving Ground, Ballistic Research Laboratories (BRL): Aberdeen, MD, USA, 1943. [Google Scholar]
- Carter, R.T.; Jandir, P.S.; Kress, M.E. Estimating the drag coefficients of meteorites for all Mach number regimes. In Proceedings of the 40th Lunar and Planetary Science Conference, (Lunar and Planetary Science XL), The Wood-Lands, TX, USA, 23–27 March 2009. [Google Scholar]
- Häring, I. Damage Analysis I: Probit Functions and Probability Distributions. In Risk Analysis and Management: Engineering Resilience; Springer: Singapore, 2015; pp. 187–204. [Google Scholar]
- Finney, D.J. Probit Analysis, 3rd ed.; Cambridge University Press: Cambridge, UK, 1971. [Google Scholar]
- Alonso, F.D.; Ferradás, E.G.; Pérez, J.F.S.; Aznar, A.M.; Gimeno, J.R.; Alonso, J.M. Characteristic overpressure–impulse–distance curves for the detonation of explosives, pyrotechnics or unstable substances. J. Loss Prev. Process. Ind. 2006, 19, 724–728. [Google Scholar] [CrossRef]
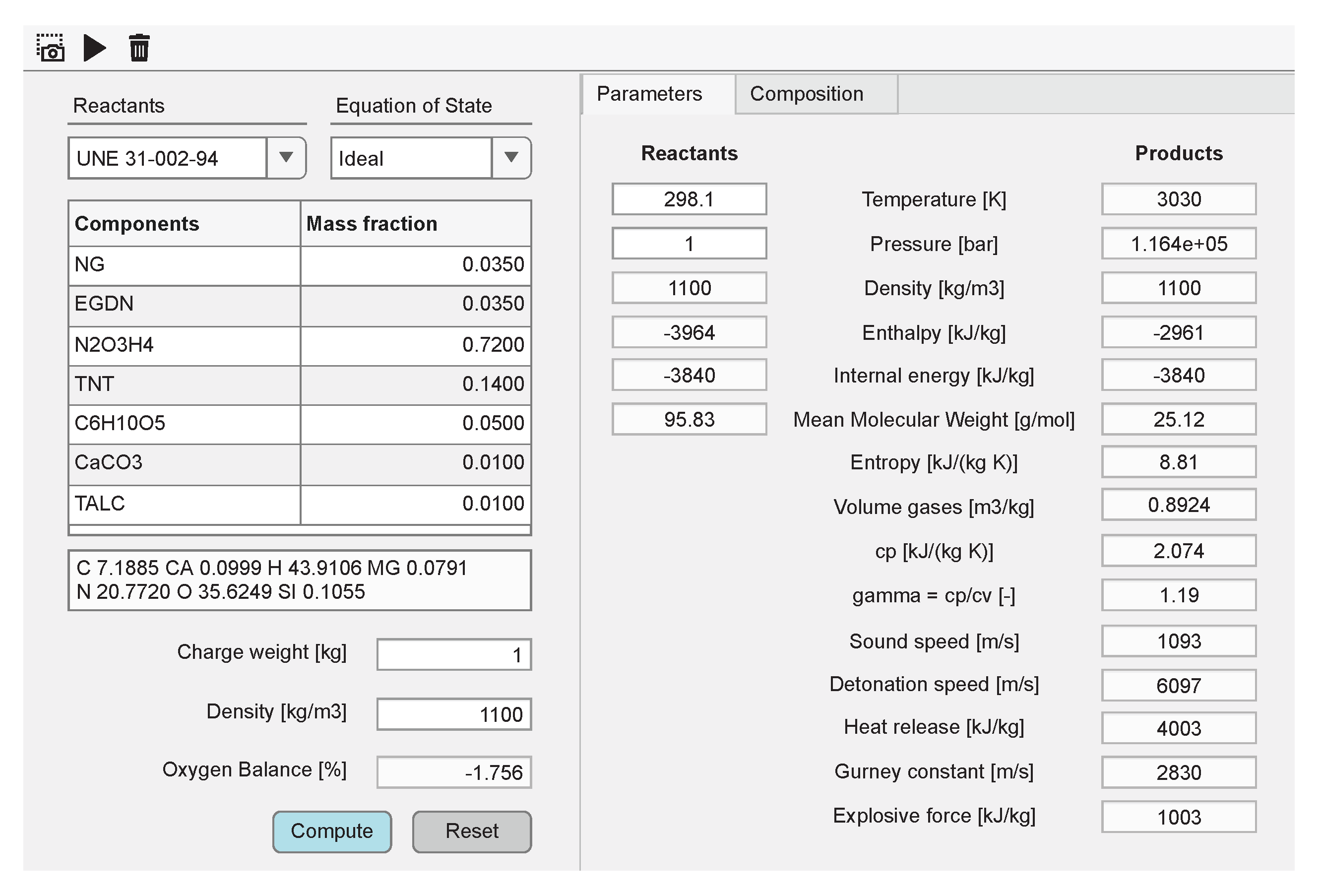



| Explosive | Source | T [K] | [GPa] | [m/s] | [kJ/kg] | [kJ/kg] |
|---|---|---|---|---|---|---|
| ANFO | CT | 2592 | 7.14 | 5353 | 3845 | 943 |
| EN 13631-15 | 2586 | - | - | 3820 | 945 | |
| W-DETCOM | 2919 | 6.62 | 5326 | 3849 | - | |
| ANFO-Al | CT | 3026 | 7.38 | 5442 | 4666 | 1009 |
| EN 13631-15 | 3060 | - | - | 4642 | 1020 | |
| W-DETCOM | 3370 | 6.55 | 5215 | 4655 | - | |
| Emulsion | CT | 2112 | 15.3 | 6549 | 3263 | 766 |
| EN 13631-15 | 2099 | - | - | 3236 | 771 | |
| W-DETCOM | 2438 | 13.9 | 6758 | 3214 | - | |
| Dinamite I | CT | 4173 | 25.03 | 7960 | 6452 | 1147 |
| EN 13631-15 | 4130 | - | - | 6338 | 1138 | |
| Dinamite II | CT | 3165 | 23.58 | 7729 | 5049 | 987 |
| EN 13631-15 | 3151 | - | - | 4989 | 984 |
| Component | ANFO | ANFO-Al | Emulsion | Dinamite I | Dinamite II |
|---|---|---|---|---|---|
| Aluminium | - | 5 | - | - | - |
| Ammonium nitrate | 94 | 91 | 80 | - | 49 |
| Cellulose | - | - | - | - | 3 |
| 2,4-Dinitrotoluene | - | - | - | - | 4 |
| Nitrocellulose 12% | - | - | 10 | - | 4 |
| Nitroglycerin | - | - | - | 45 | 20 |
| Nitroglycol | - | - | - | 45 | 20 |
| Fuel oil | 6 | 4 | 7 | - | - |
| Sodium nitrate | - | - | 5 | - | - |
| Water | - | - | 8 | - | - |
| Density [kg/m] | 850 | 850 | 1300 | 1500 | 1500 |
| Oxygen balance [%] |
| Effect | Probit Function |
|---|---|
| Primary injuries | |
| Eardrum rupture | |
| Death due to lung damage | |
| Tertiary injuries | |
| Death due to displacement and whole-body impact | |
| Death due to displacement and skull impact |
| Variables | Case 1 | Case 2 | Case 3 |
|---|---|---|---|
| d (m) | 12 | 20 | 25 |
| W (kg) | 30 | 150 | 300 |
| Z (m/kg) | 3.86 | 3.76 | 3.73 |
| Level | Type | Variables | Case 1 | Case 2 | Case 3 |
|---|---|---|---|---|---|
| 0 | Incident load parameters | (kPa) | 168.30 | 182.50 | 186.80 |
| () | 406.70 | 724.40 | 922.20 | ||
| (m) | 12.17 | 20.10 | 25.08 | ||
| (deg) | 9.46 | 5.71 | 4.57 | ||
| Damage level indicators | (-) | 1.60 | 3.26 | 4.40 | |
| (deg) | 0.19 | 0.39 | 0.53 | ||
| 1 | Incident load parameters | (kPa) | 139.40 | 169.50 | 178.00 |
| () | 349.90 | 688.10 | 893.00 | ||
| (m) | 13.20 | 20.74 | 25.60 | ||
| (deg) | 24.62 | 15.38 | 12.41 | ||
| Damage level indicators | (-) | 0.90 | 1.64 | 2.10 | |
| (deg) | 0.08 | 0.15 | 0.19 | ||
| 2 | Incident load parameters | (kPa) | 110.20 | 152.00 | 165.40 |
| () | 293.00 | 630.50 | 845.50 | ||
| (m) | 14.71 | 21.73 | 26.41 | ||
| (deg) | 35.31 | 23.03 | 18.78 | ||
| Damage level indicators | (-) | 1.67 | 5.85 | 9.26 | |
| (deg) | 0.87 | 3.05 | 4.83 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez-Monreal, J.; Cuadra, A.; Huete, C.; Vera, M. SimEx: A Tool for the Rapid Evaluation of the Effects of Explosions. Appl. Sci. 2022, 12, 9101. https://doi.org/10.3390/app12189101
Sánchez-Monreal J, Cuadra A, Huete C, Vera M. SimEx: A Tool for the Rapid Evaluation of the Effects of Explosions. Applied Sciences. 2022; 12(18):9101. https://doi.org/10.3390/app12189101
Chicago/Turabian StyleSánchez-Monreal, Juan, Alberto Cuadra, César Huete, and Marcos Vera. 2022. "SimEx: A Tool for the Rapid Evaluation of the Effects of Explosions" Applied Sciences 12, no. 18: 9101. https://doi.org/10.3390/app12189101
APA StyleSánchez-Monreal, J., Cuadra, A., Huete, C., & Vera, M. (2022). SimEx: A Tool for the Rapid Evaluation of the Effects of Explosions. Applied Sciences, 12(18), 9101. https://doi.org/10.3390/app12189101









