Activation Energy and Inclination Magnetic Dipole Influences on Carreau Nanofluid Flowing via Cylindrical Channel with an Infinite Shearing Rate
Abstract
:1. Introduction
2. Novelty of Physical Model
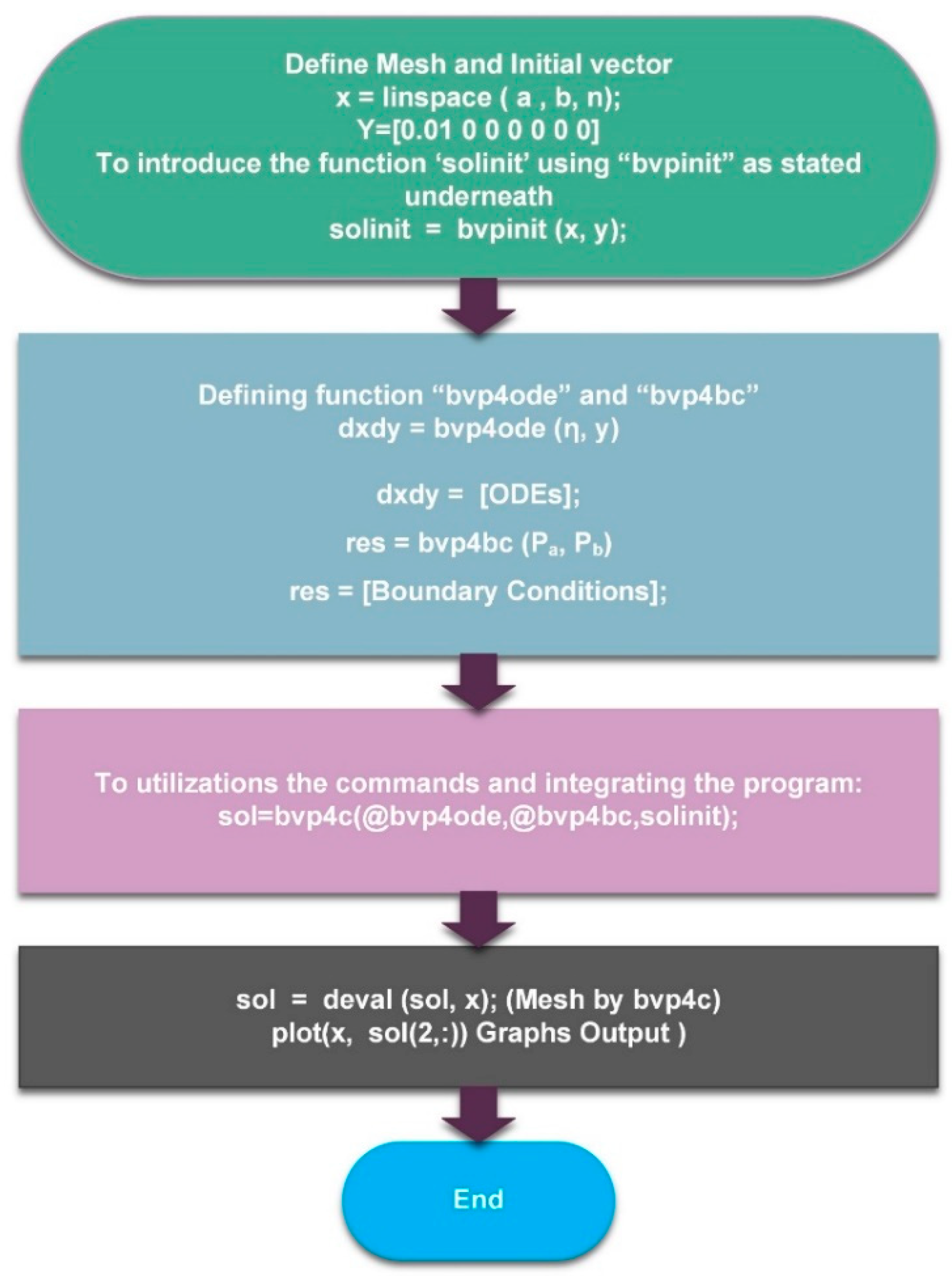
3. Methodology
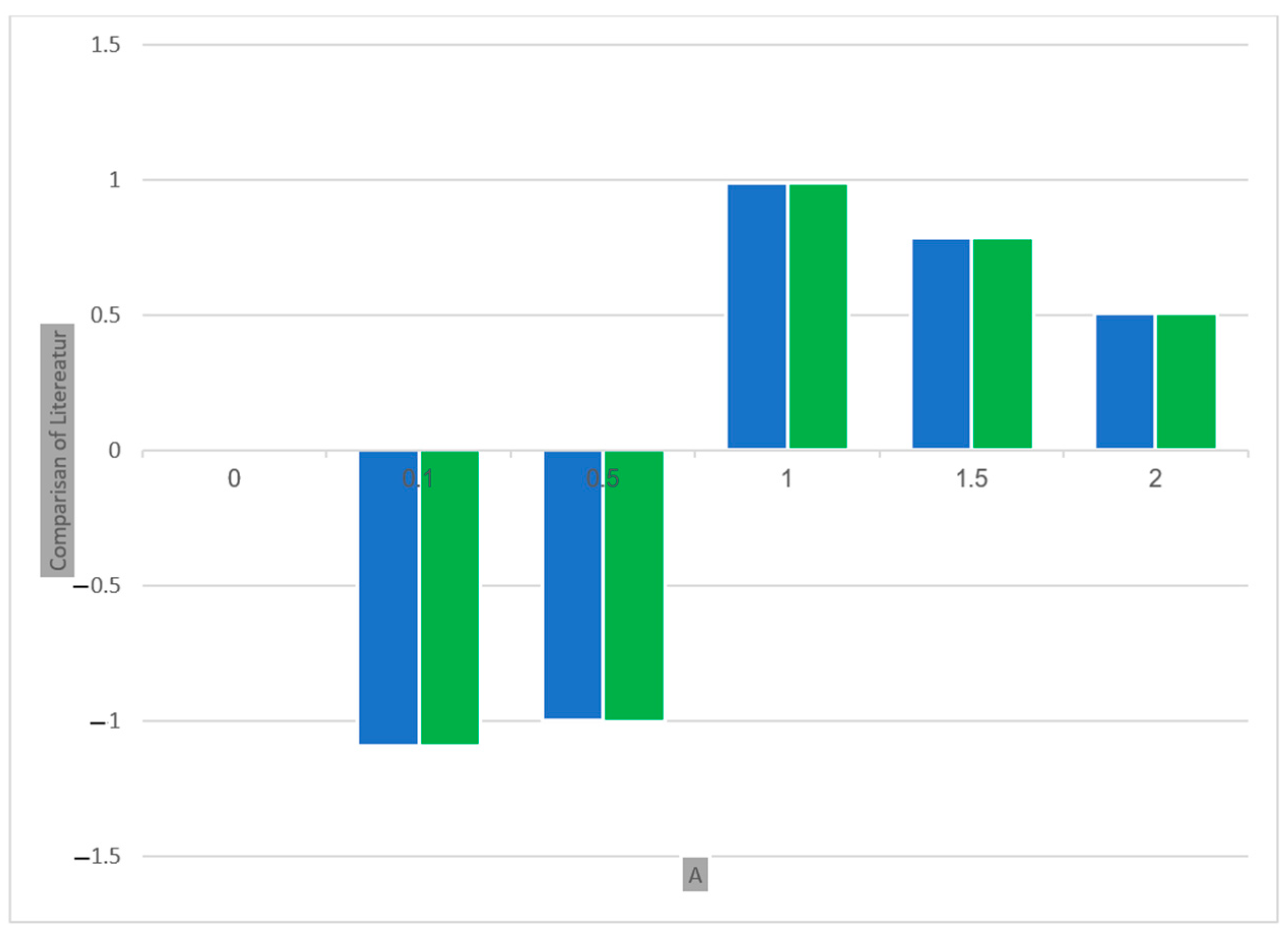
4. Validity of the Study in Pictorial Form
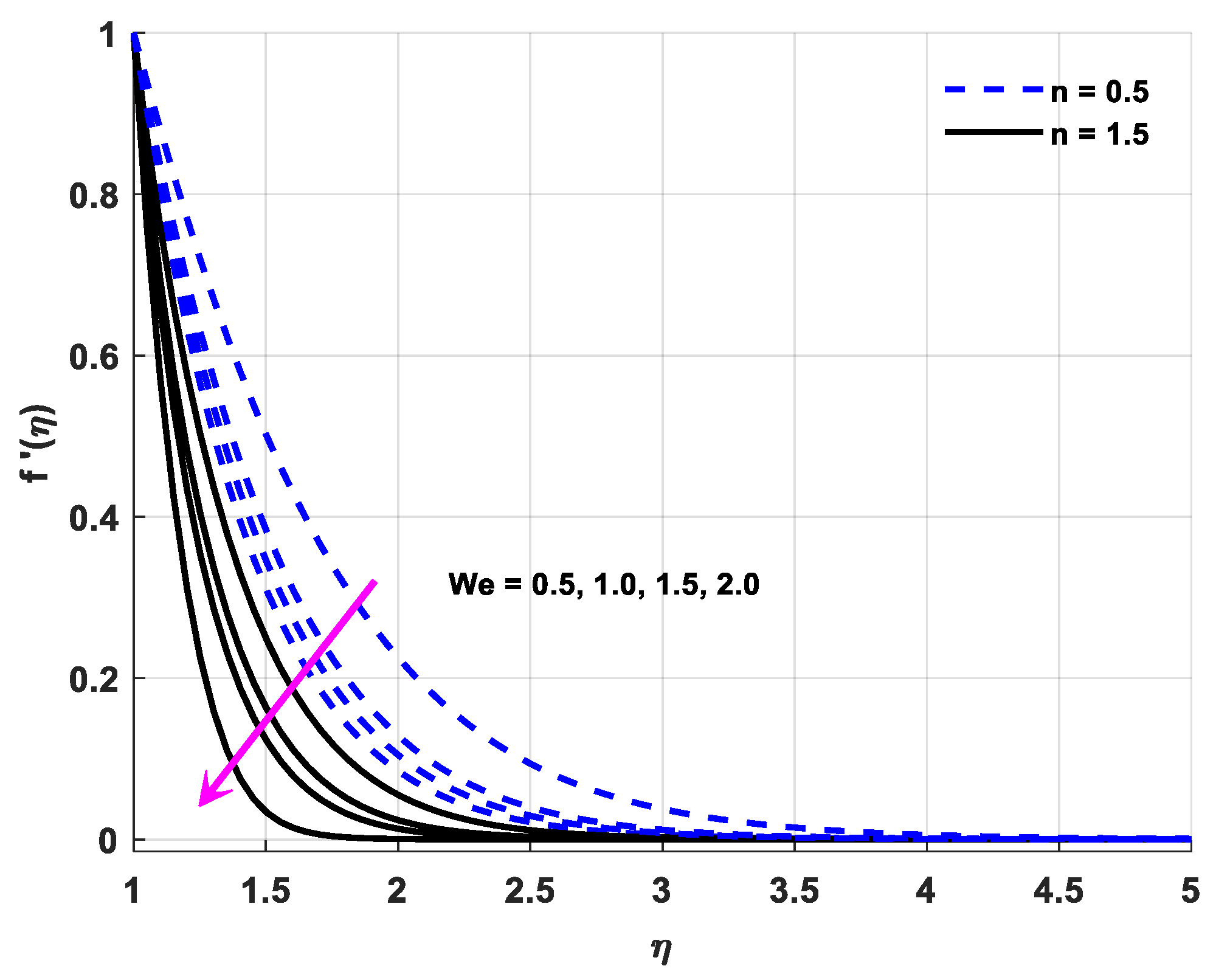
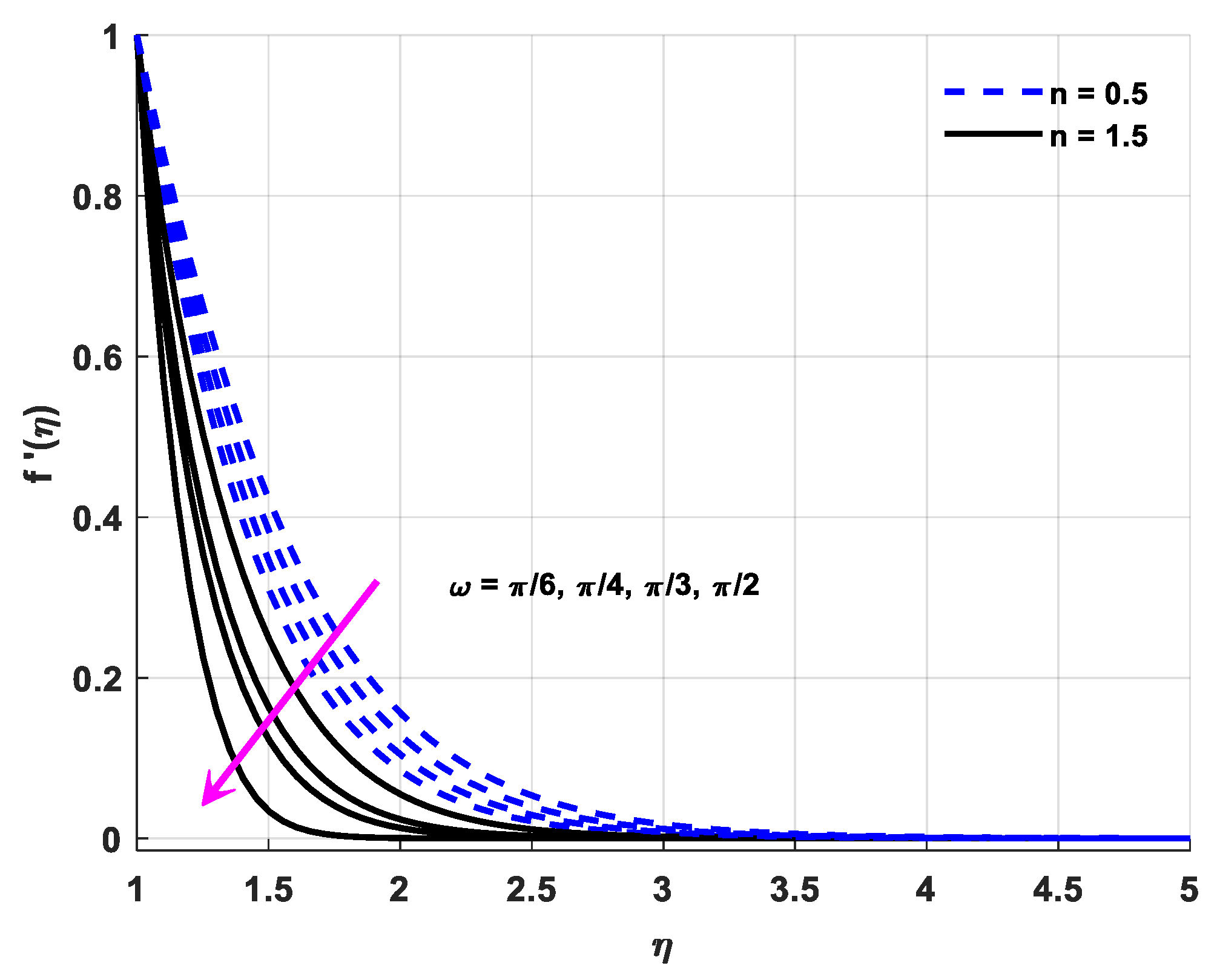
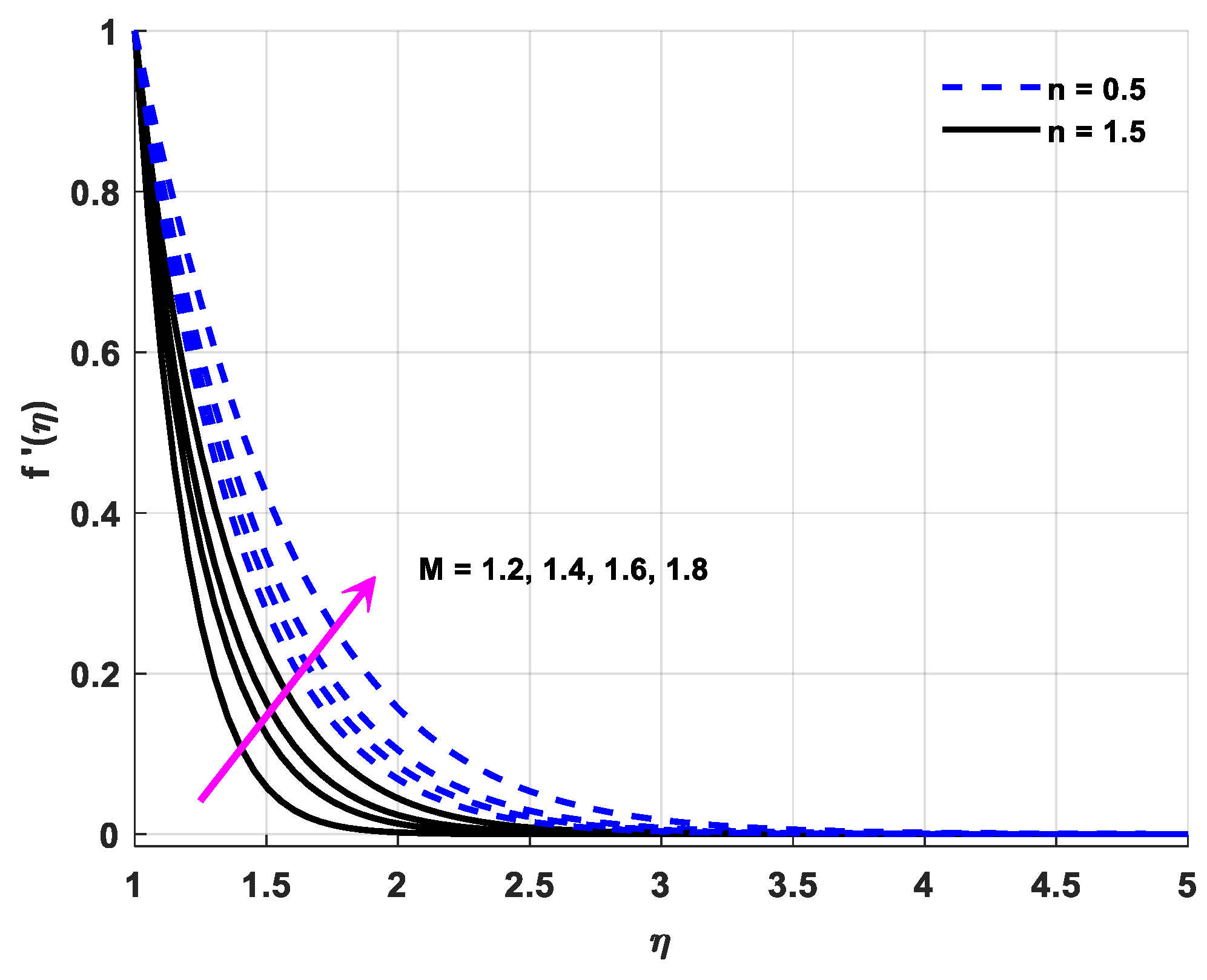
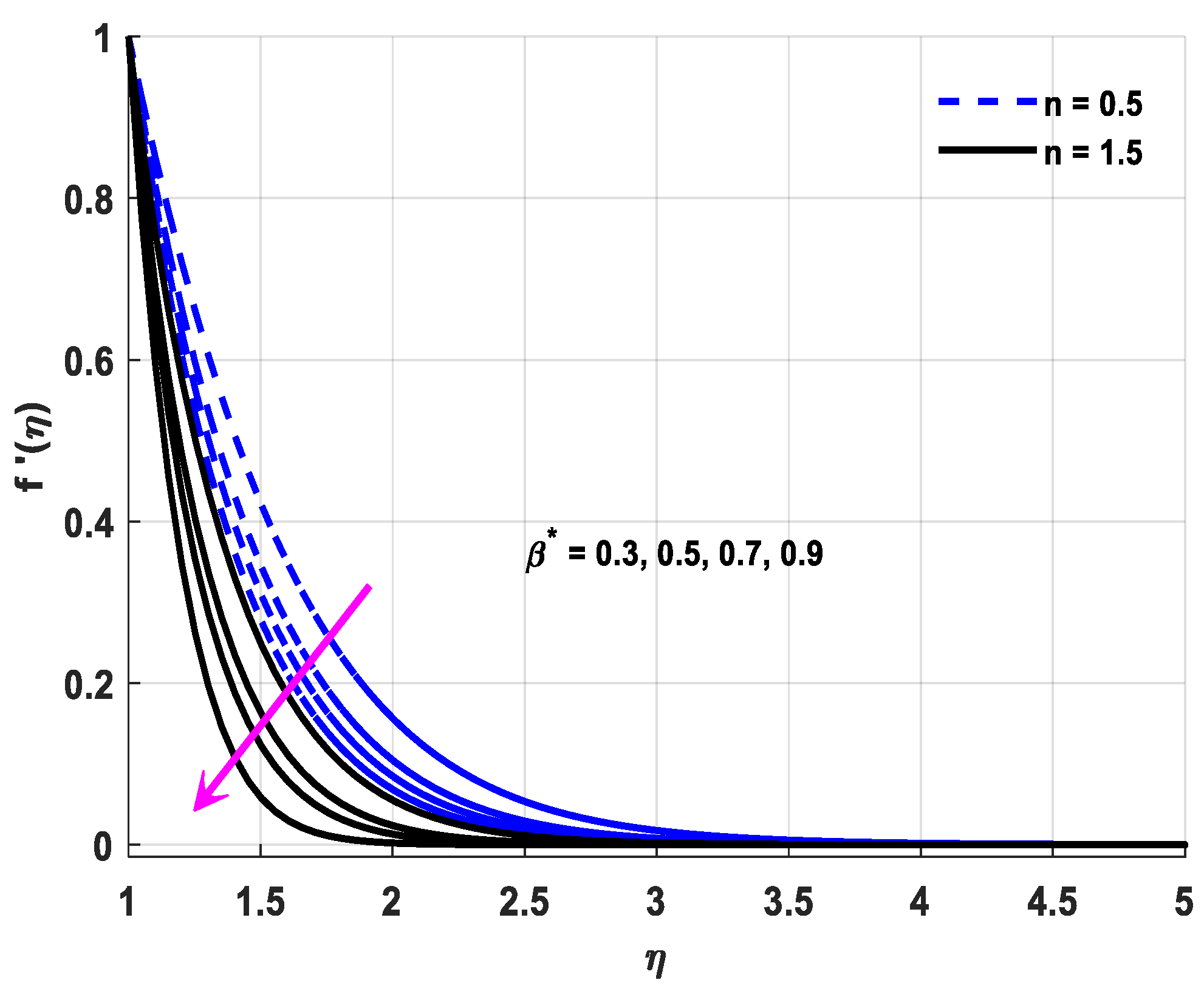
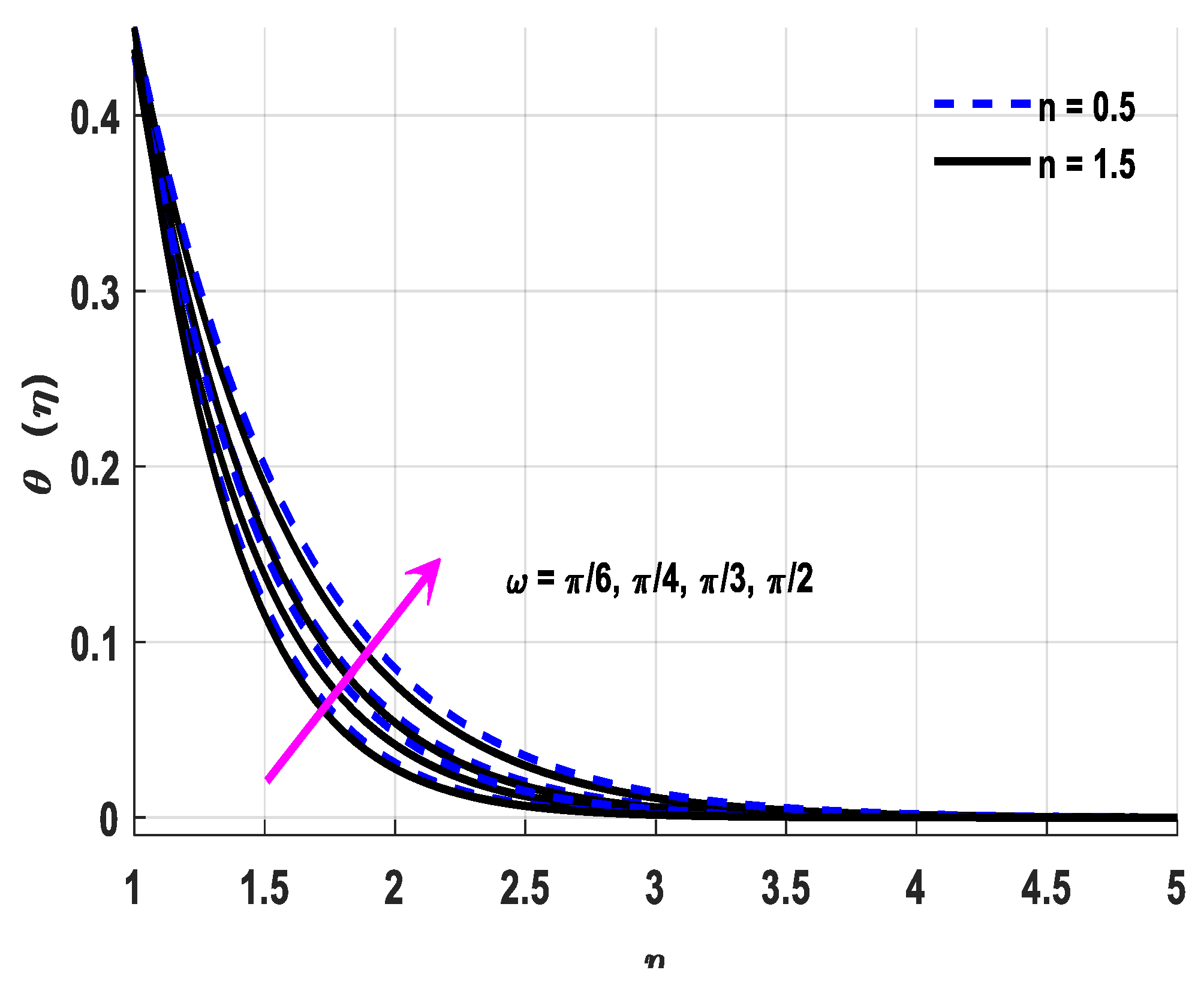
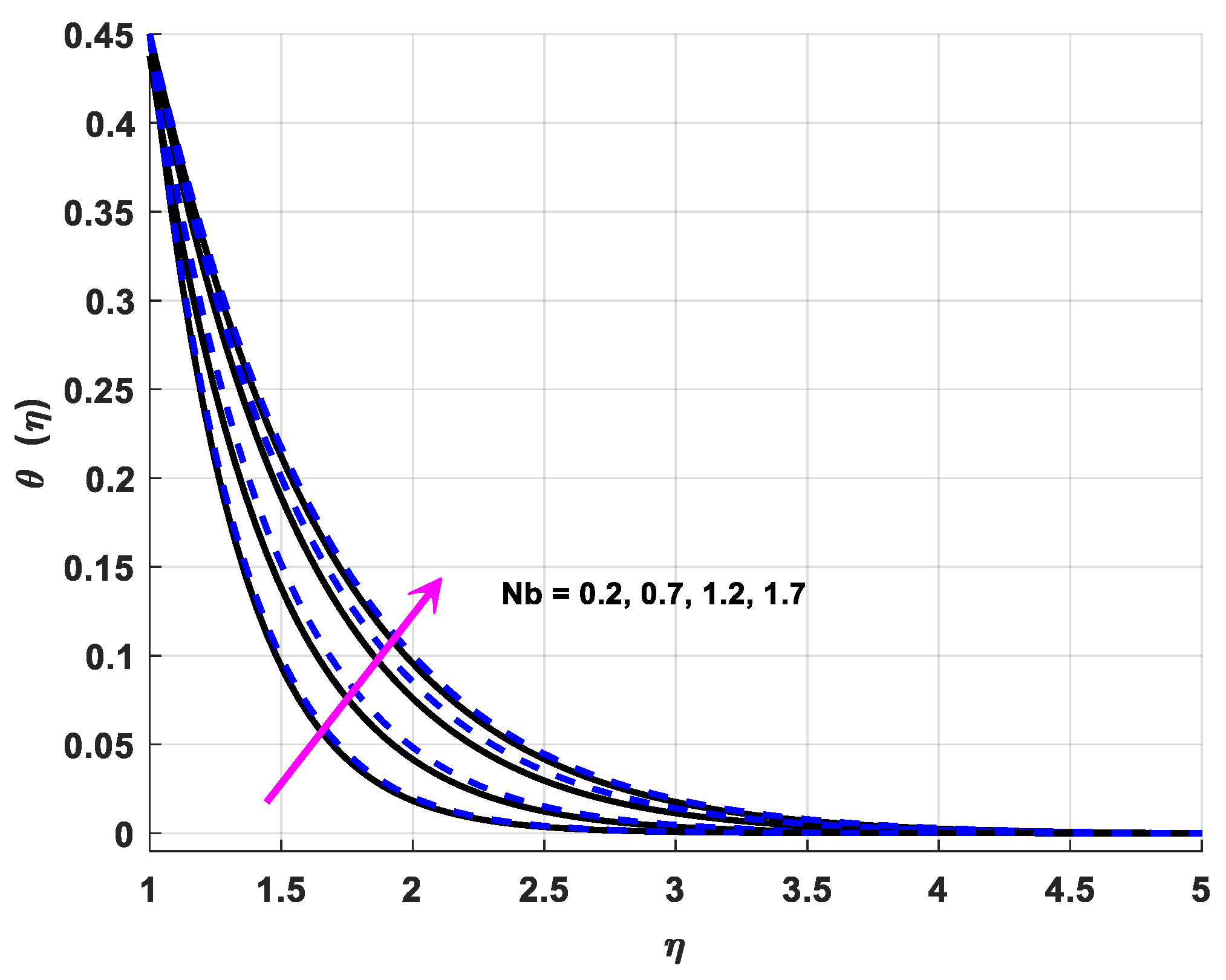
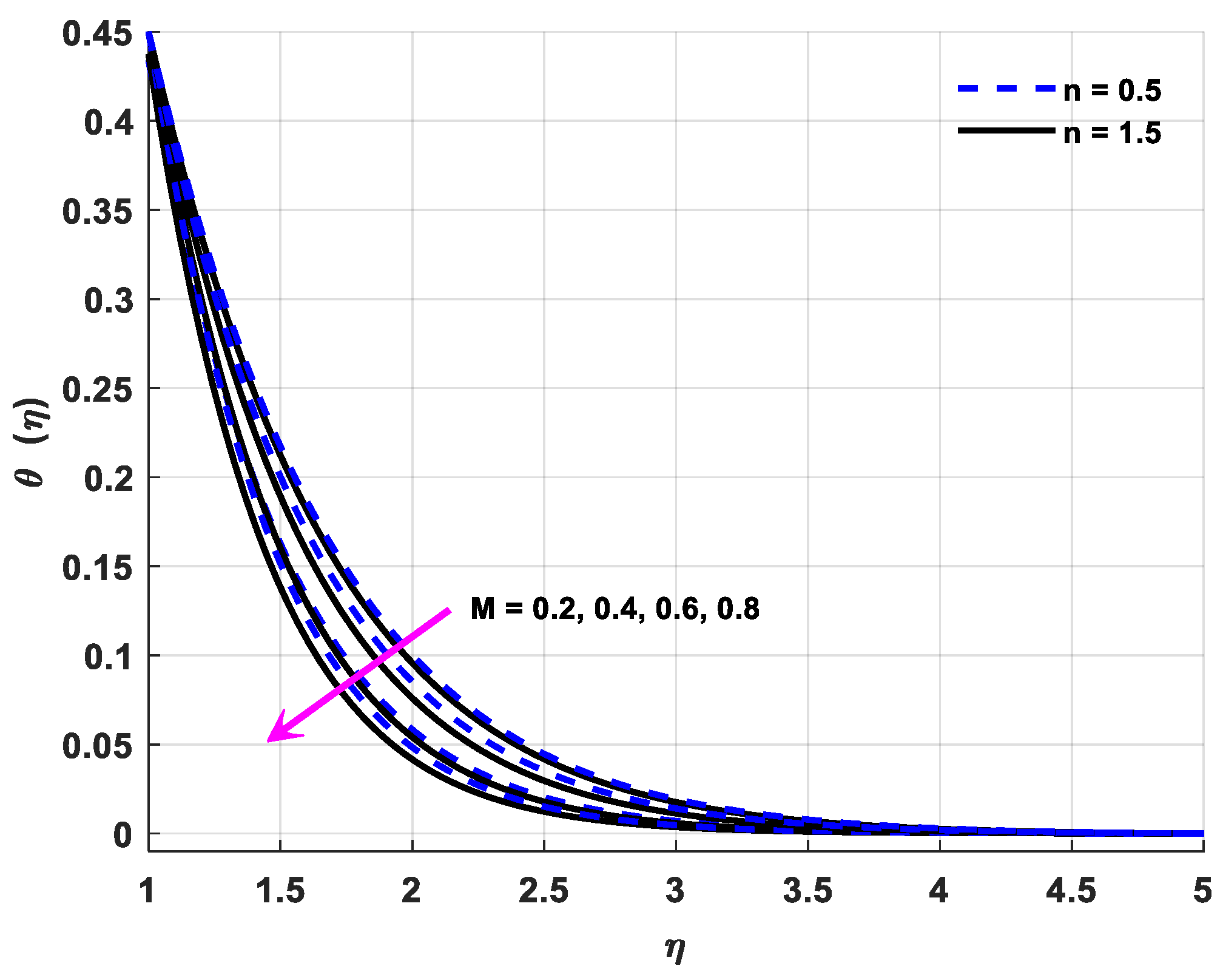
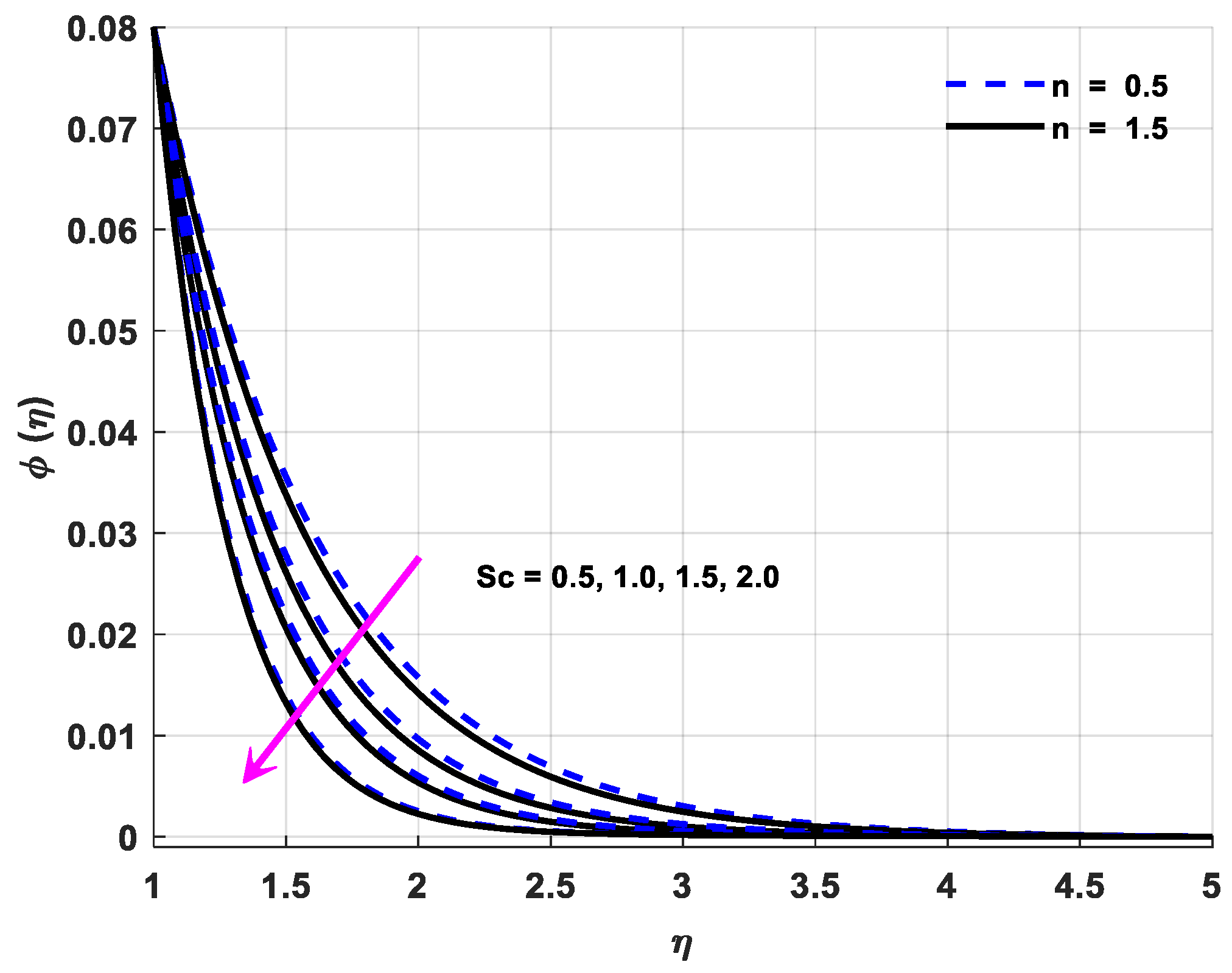
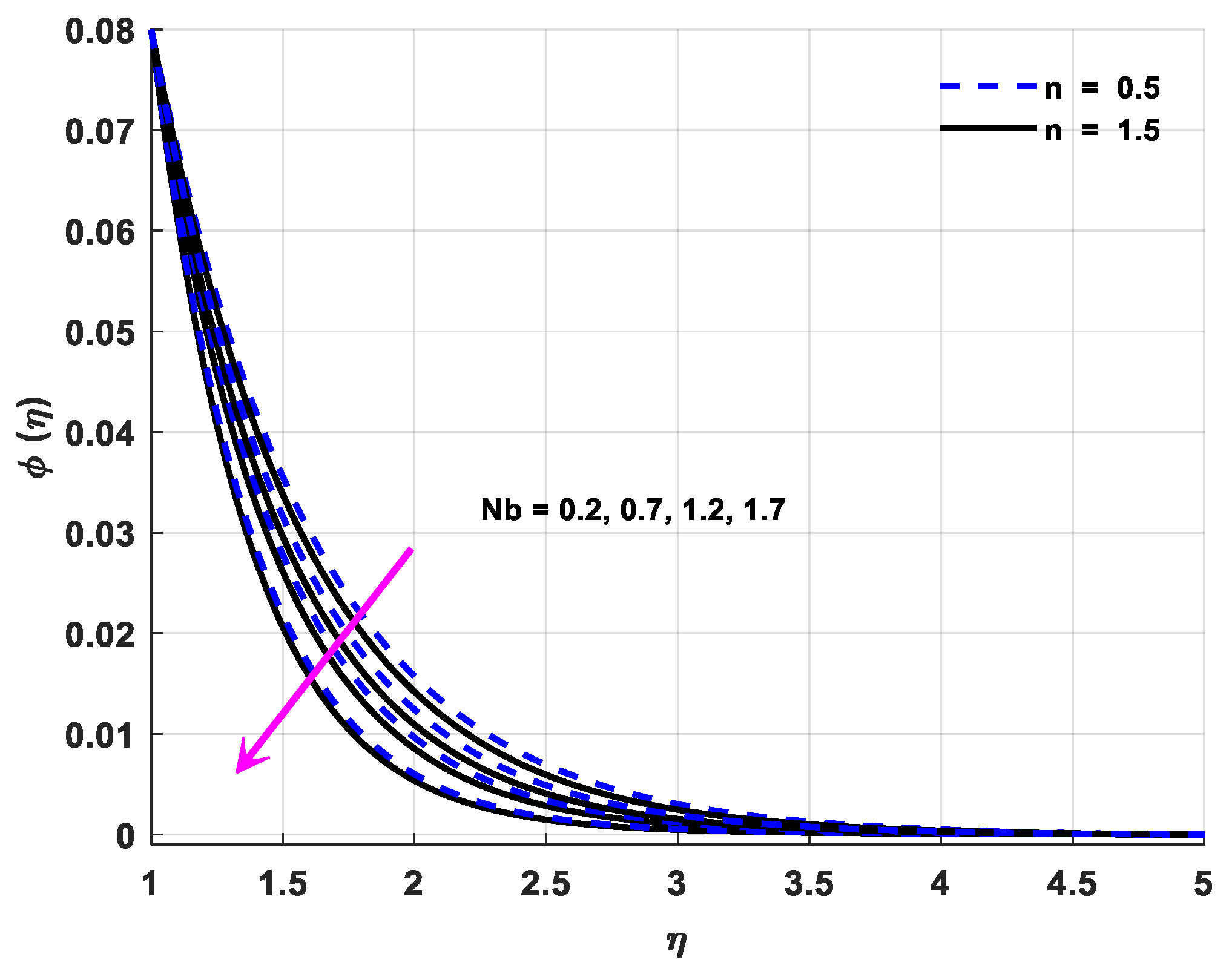
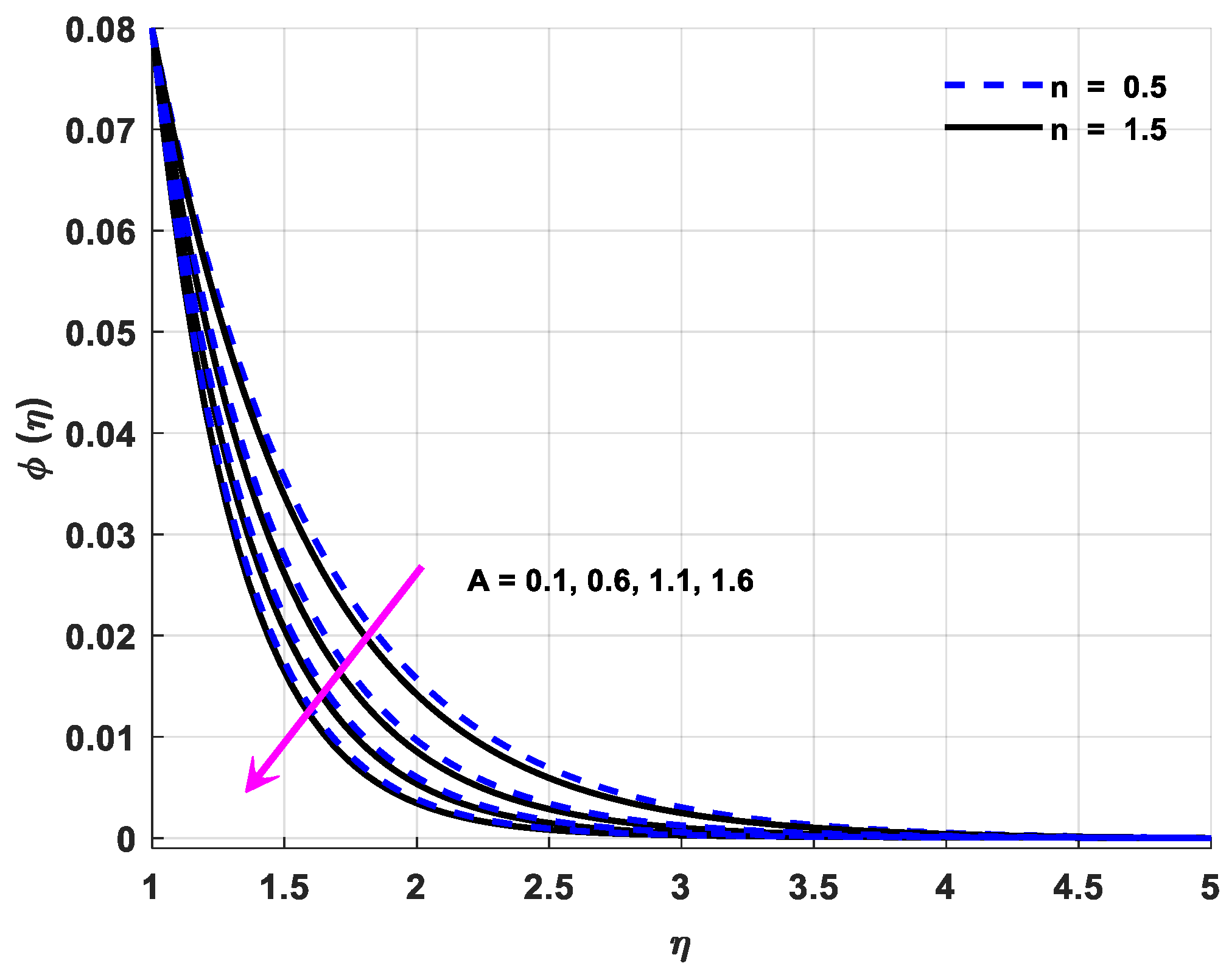
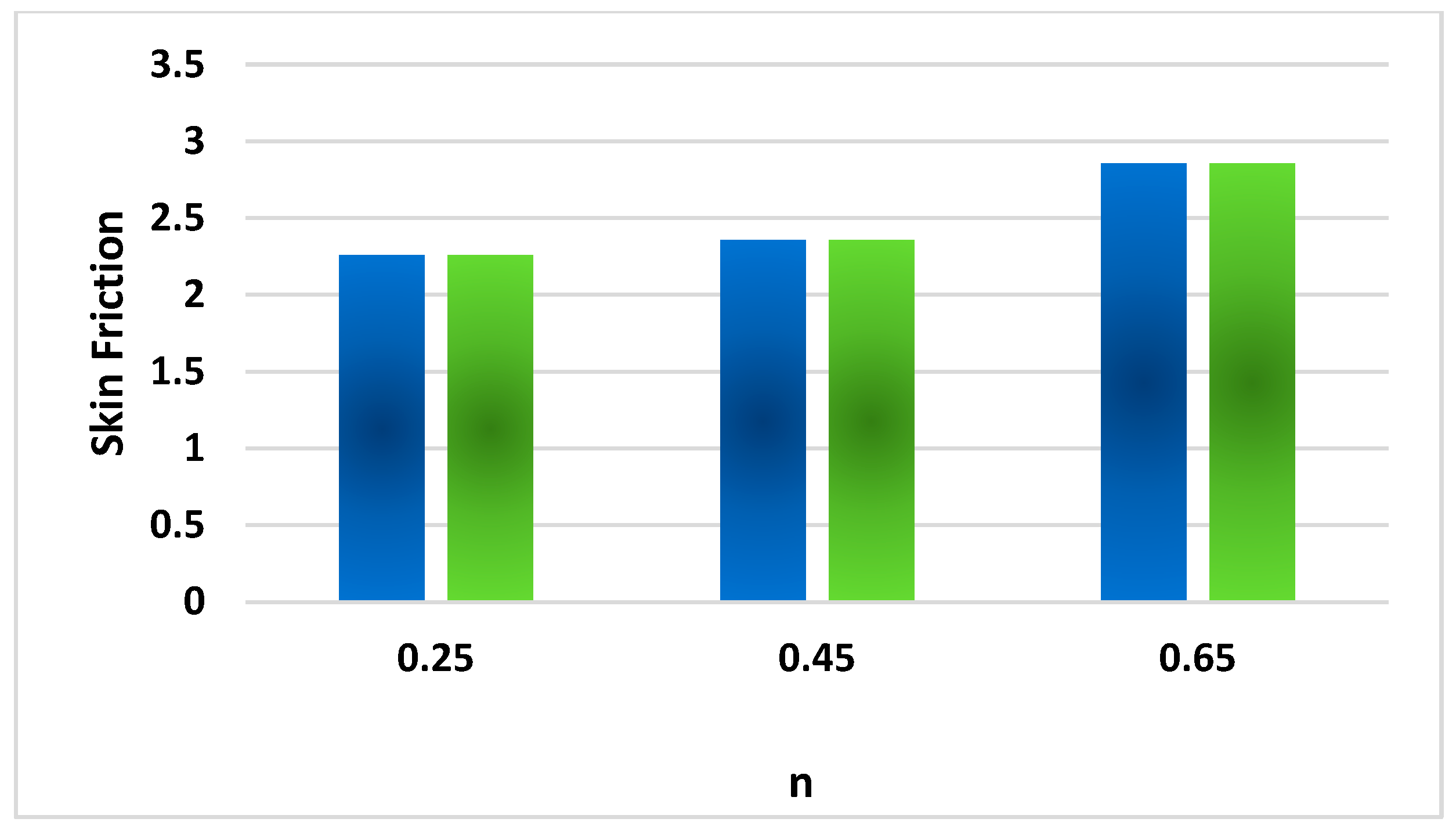
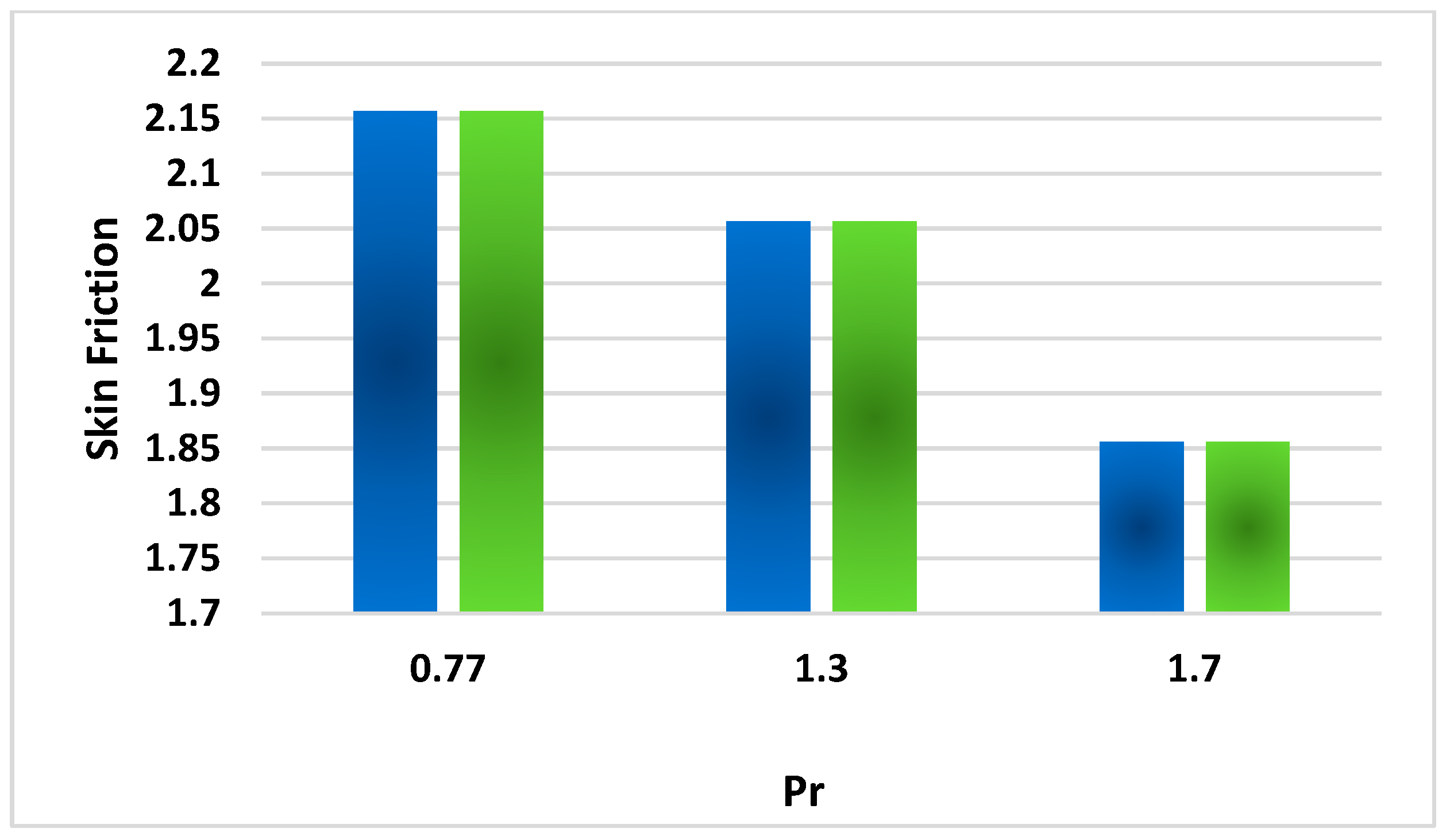
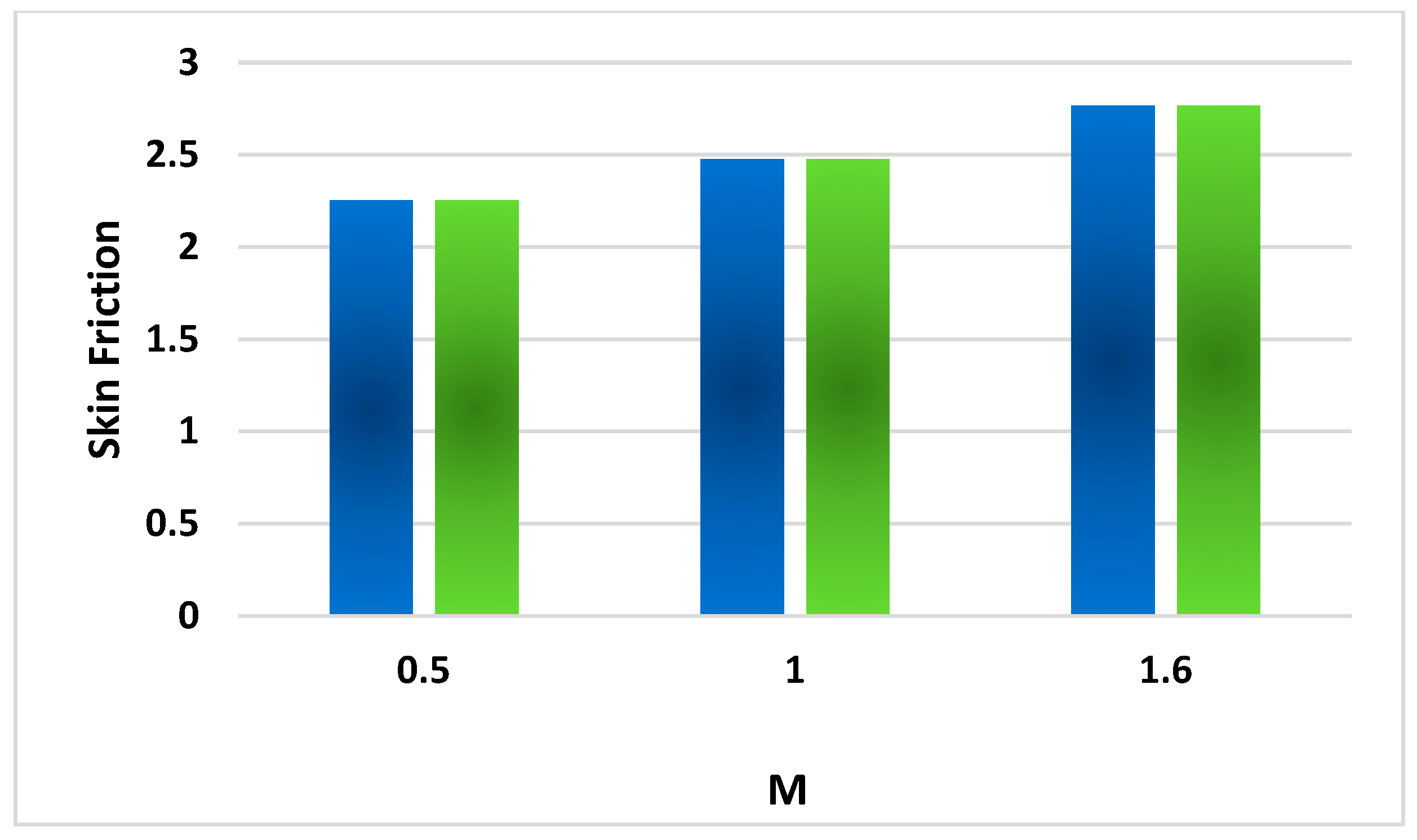
5. Comprehensive Analysis of Outcomes of Attached Parameters
6. Conclusions of Debate
- Infinite shear rate aspect of Carreau nanofluid gives lower velocity.
- Inclined magnetic dipole effect shows lower velocity but high energy.
- Unsteadiness parameter is related to time factor due to this magnitude of velocity decreasing.
- Relaxation time of fluid increasing by the virtue of magnification in We, which diminishes the velocity field.
- A positive variation in magnetic parameters diminishes the surface drag phenomenon.
- Amplification in thermal conductivity magnifies Nb and the temperature field.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Ethical Statement
Nomenclature
| Wall heat transfer coefficient | Schmidt number | ||
| Wall mass transfer coefficient | Density of fluid | ||
| Specific heat | |||
| Ambient concentration | Operative heat capability | ||
| Surface temperature | u, v | Velocity components | |
| Density of fluid | Reaction rate parameter | ||
| Thermophoresis diffusion coefficient | Thermophoresis parameter | ||
| U | Stretching velocity | Rex | Local Reynolds number |
| Thermal diffusivity | I | Identity tensor | |
| Kinematic viscosity | Power law index | ||
| Unsteadiness parameter | A1 | First Rivlin–Ericksen tensor | |
| Brownian diffusion coefficient | Magnetic parameter | ||
| Schmidt number | Magnetic parameter | ||
| Local Weissenberg number | Temperature constant | ||
| Reaction rate parameter | Pr = | Prandtl number | |
| Brownian motion parameter | Activation energy | ||
| Distance along the axial direction | Expansion or contraction strength | ||
| Distance along the radial direction | Dimensionless velocity | ||
| Dimensionless temperature | Radius of cylinder | ||
| Dimensionless concentration | Thermal Biot number | ||
| Concentration Biot number | Time | ||
| We | Local Weissenberg number | V | Velocity field |
| Thermal conductivity parameter | C | Concentration field | |
| Skin friction coefficient | Cauchy stress tensor | ||
| Wall heat transfer coefficient | Pressure | ||
| Wall mass flux | Apparent viscosity | ||
| Wall heat flux | Zero shear rate viscosity | ||
| Wall shear stress | Infinite shear rate viscosity | ||
| Surface temperature | Shear rate | ||
| Brownian motion parameter | Time material constant | ||
| Thermophoresis parameter | Local similarity variable | ||
| Variable thermal conductivity |
References
- Khan, M.; Azam, M.; Alshomrani, A.S. On unsteady heat and mass transfer in Carreau nanofluid flow over expanding or contracting cylinder with convective surface conditions. J. Mol. Liq. 2017, 231, 474–484. [Google Scholar] [CrossRef]
- Ayub, A.; Sabir, Z.; Altamirano, G.C.; Sadat, R.; Ali, M.R. Characteristics of melting heat transport of blood with time-dependent cross-nanofluid model using Keller–Box and BVP4C method. Eng. Comput. 2022, 38, 3705–3719. [Google Scholar] [CrossRef]
- Chen, L.; Abbas, M.A.; Khudair, W.S.; Sun, B. Analytical Solution for the MHD Flow of Non-Newtonian Fluids between Two Coaxial Cylinders. Symmetry 2022, 14, 953. [Google Scholar] [CrossRef]
- Alsaedi, A.; Muhammad, K.; Hayat, T. Numerical study of MHD hybrid nanofluid flow between two coaxial cylinders. Alex. Eng. J. 2022, 61, 8355–8362. [Google Scholar] [CrossRef]
- Wahab, H.A.; Hussain Shah, S.Z.; Ayub, A.; Sabir, Z.; Bilal, M.; Altamirano, G.C. Multiple characteristics of three-dimensional radiative Cross fluid with velocity slip and inclined magnetic field over a stretching sheet. Heat Transf. 2021, 50, 3325–3341. [Google Scholar] [CrossRef]
- Shah, S.Z.; Wahab, H.A.; Ayub, A.; Sabir, Z.; haider, A.; Shah, S.L. Higher order chemical process with heat transport of magnetized cross nanofluid over wedge geometry. Heat Transf. 2021, 50, 3196–3219. [Google Scholar] [CrossRef]
- Lim, Y.J.; Shafie, S.; Mohamad Isa, S.M.; Rawi, N.A.; Mohamad, A.Q. Impact of chemical reaction, thermal radiation and porosity on free convection Carreau fluid flow towards a stretching cylinder. Alex. Eng. J. 2022, 61, 4701–4717. [Google Scholar] [CrossRef]
- Shaw, S.; Samantaray, S.S.; Misra, A.; Nayak, M.K.; Makinde, O.D. Hydromagnetic flow and thermal interpretations of Cross hybrid nanofluid influenced by linear, nonlinear and quadratic thermal radiations for any Prandtl number. Int. Commun. Heat Mass Transf. 2022, 130, 105816. [Google Scholar] [CrossRef]
- Nayak, M.K.; Agbaje, T.M.; Mondal, S.; Sibanda, P.; Makanda, G. Entropy minimized MHD microrotations of Cross nanomaterials with cubic autocatalytic chemical reaction. Heat Transf. 2022, 51, 490–533. [Google Scholar] [CrossRef]
- Aly, A.M.; El-Sapa, S. Effects of Soret and Dufour numbers on MHD thermosolutal convection of a nanofluid in a finned cavity including rotating circular cylinder and cross shapes. Int. Commun. Heat Mass Transf. 2022, 130, 105819. [Google Scholar] [CrossRef]
- Nandi, S.; Kumbhakar, B.; Sarkar, S. MHD stagnation point flow of Fe3O4/Cu/Ag-CH3OH nanofluid along a convectively heated stretching sheet with partial slip and activation energy: Numerical and statistical approach. Int. Commun. Heat Mass Transf. 2022, 130, 105791. [Google Scholar] [CrossRef]
- Zeeshan, A.; Mehmood, O.U.; Mabood, F.; Alzahrani, F. Numerical analysis of hydromagnetic transport of Casson nanofluid over permeable linearly stretched cylinder with Arrhenius activation energy. Int. Commun. Heat Mass Transf. 2022, 130, 105736. [Google Scholar] [CrossRef]
- Alotaibi, H.; Althubiti, S.; Eid, M.R.; Mahny, K. Numerical treatment of MHD flow of Casson nanofluid via convectively heated non-linear extending surface with viscous dissipation and suction/injection effects. Comput. Mater. Contin. 2020, 66, 229–245. [Google Scholar] [CrossRef]
- Khan, M.; Sardar, H. On steady two-dimensional Carreau fluid flow over a wedge in the presence of infinite shear rate viscosity. Results Phys. 2018, 8, 516–523. [Google Scholar] [CrossRef]
- Khan, M.; Sardar, H.; Gulzar, M.M.; Alshomrani, A.S. On multiple solutions of non-Newtonian Carreau fluid flow over an inclined shrinking sheet. Results Phys. 2018, 8, 926–932. [Google Scholar] [CrossRef]
- Ayub, A.; Sabir, Z.; Shah, S.Z.H.; Mahmoud, S.R.; Algarni, A.; Sadat, R.; Ali, M.R. Aspects of infinite shear rate viscosity and heat transport of magnetized Carreau nanofluid. Eur. Phys. J. Plus 2022, 137, 247. [Google Scholar] [CrossRef]
- Sardar, H.; Khan, M. Mixed convection flow and heat transfer mechanism for non-Newtonian Carreau nanofluids under the effect of infinite shear rate viscosity. Phys. Scr. 2020, 95, 035225. [Google Scholar]
- Ali, U.; Rehman, K.U.; Alshomrani, A.S.; Malik, M.Y. Thermal and concentration aspects in Carreau viscosity model via wedge. Case Stud. Therm. Eng. 2018, 12, 126–133. [Google Scholar] [CrossRef]
- Wang, F.; Sajid, T.; Ayub, A.; Sabir, Z.; Bhatti, S.; Shah, N.A.; Sadat, R.; Ali, M.R. Melting and entropy generation of infinite shear rate viscosity Carreau model over Riga plate with erratic thickness: A numerical Keller Box approach. Waves Random Complex Media 2022, 1–25. [Google Scholar] [CrossRef]
- Mosaferi, A.A.; Esmaeili, M.; Rabiee, A.H. Effect of aligned magnetic field on the 2DOF VIV suppression and convective heat transfer characteristics of a circular cylinder. Int. Commun. Heat Mass Transf. 2022, 130, 105807. [Google Scholar] [CrossRef]
- Sierra-Espinosa, F.Z.; Amjad, S.; Carrillo, F.; Soria, J.; Atkinson, C. Turbulent cylinder-stirred flow heat and momentum transfer research in batch operated single-phase square reactor. Int. J. Therm. Sci. 2022, 172, 107325. [Google Scholar] [CrossRef]
- Pennec, Y.; Djafari-Rouhani, B.; Vasseur, J.O.; Khelif, A.; Deymier, P.A. Tunable filtering and demultiplexing in phononic crystals with hollow cylinders. Phys. Rev. E 2004, 69, 046608. [Google Scholar] [CrossRef]
- Keklikcioglu, O.; Ozceyhan, V. Heat transfer augmentation in a tube with conical wire coils using a mixture of ethylene glycol/water as a fluid. Int. J. Therm. Sci. 2022, 171, 107204. [Google Scholar] [CrossRef]
- Waqas, H.; Wakif, A.; Al-Mdallal, Q.; Zaydan, M.; Farooq, U.; Hussain, M. Significance of magnetic field and activation energy on the features of stratified mixed radiative-convective couple-stress nanofluid flows with motile microorganisms. Alex. Eng. J. 2022, 61, 1425–1436. [Google Scholar] [CrossRef]
- Muhammad, T.; Waqas, H.; Manzoor, U.; Farooq, U.; Rizvi, Z.F. On doubly stratified bioconvective transport of Jeffrey nanofluid with gyrotactic motile microorganisms. Alex. Eng. J. 2022, 61, 1571–1583. [Google Scholar] [CrossRef]
- Mohammed, H.A.; Vuthaluru, H.B.; Liu, S. Thermohydraulic and thermodynamics performance of hybrid nanofluids based parabolic trough solar collector equipped with wavy promoters. Renew. Energy 2022, 182, 401–426. [Google Scholar] [CrossRef]
- Song, Y.Q.; Hamid, A.; Sun, T.C.; Khan, M.I.; Qayyum, S.; Kumar, R.N.; Prasannakumarae, B.C.; Khanf, S.U.; Chinram, R. Unsteady mixed convection flow of magneto-Williamson nanofluid due to stretched cylinder with significant non-uniform heat source/sink features. Alex. Eng. J. 2022, 61, 195–206. [Google Scholar] [CrossRef]
- Kumar, L.H.; Kazi, S.N.; Masjuki, H.H.; Zubir, M.N.M. A review of recent advances in green nanofluids and their application in thermal systems. Chem. Eng. J. 2022, 429, 132321. [Google Scholar] [CrossRef]
- Dina, Q.; Shabira, M.S.; Asif, M. A Cubic Autocatalator Chemical Reaction Model with Limit Cycle Analysis and Consistency Preserving Discretization. Match-Commun. Math. Comput. Chem. 2022, 87, 441–462. [Google Scholar] [CrossRef]
- Scholes, C.A. Mass transfer basics and models of membranes containing nanofluids. In Nanofluids and Mass Transfer; Elsevier: Amsterdam, The Netherlands, 2022; pp. 369–384. [Google Scholar]
- Tembhare, S.P.; Barai, D.P.; Bhanvase, B.A. Performance evaluation of nanofluids in solar thermal and solar photovoltaic systems: A comprehensive review. Renew. Sustain. Energy Rev. 2022, 153, 111738. [Google Scholar] [CrossRef]
- Madhukesh, J.K.; Ramesh, G.K.; Roopa, G.S.; Prasannakumara, B.C.; Shah, N.A.; Yook, S.J. 3D Flow of Hybrid Nanomaterial through a Circular Cylinder: Saddle and Nodal Point Aspects. Mathematics 2022, 10, 1185. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, D.; Israr Ur Rehman, M.; Mousa, A.A.; Hamid, A. Numerical simulation of bioconvection radiative flow of Williamson nanofluid past a vertical stretching cylinder with activation energy and swimming microorganisms. Case Stud. Therm. Eng. 2022, 33, 101977. [Google Scholar] [CrossRef]
- Mahdy, A.; Hady, F.M.; Mohamed, R.A.; Abo-zaid, O.A. Activation energy effectiveness in dusty Carreau fluid flow along a stretched cylinder due to non-uniform thermal conductivity property and temperature-dependent heat source/sink. Heat Transf. 2021, 50, 5760–5778. [Google Scholar] [CrossRef]
- Shaheen, N.; Alshehri, H.M.; Ramzan, M.; Shah, Z.; Kumam, P. Soret and Dufour effects on a Casson nanofluid flow past a deformable cylinder with variable characteristics and Arrhenius activation energy. Sci. Rep. 2021, 11, 19282. [Google Scholar] [CrossRef]
- Colak, A.B. Analysis of the effect of arrhenius activation energy and temperature dependent viscosity on non-newtonian maxwell nanofluid bio-convective flow with partial slip by artificial intelligence approach. Chem. Thermodyn. Therm. Anal. 2022, 6, 100039. [Google Scholar] [CrossRef]
- Jamshed, W.; Aziz, A. Entropy Analysis of TiO2-Cu/EG Casson Hybrid Nanofluid via Cattaneo-Christov Heat Flux Model. Appl. Nanosci. 2018, 08, 1–14. [Google Scholar]
- Jamshed, W. Numerical Investigation of MHD Impact on Maxwell Nanofluid. Int. Commun. Heat Mass Transf. 2021, 120, 104973. [Google Scholar] [CrossRef]
- Jamshed, W.; Nisar, K.S. Computational single phase comparative study of Williamson nanofluid in parabolic trough solar collector via Keller box method. Int. J. Energy Res. 2021, 45, 10696–10718. [Google Scholar] [CrossRef]
- Jamshed, W.; Devi, S.U.; Nisar, K.S. Single phase-based study of Ag-Cu/EO Williamson hybrid nanofluid flow over a stretching surface with shape factor. Phys. Scr. 2021, 96, 065202. [Google Scholar] [CrossRef]
- Jamshed, W.; Nisar, K.S.; Ibrahim, R.W.; Shahzad, F.; Eid, M.R. Thermal expansion optimization in solar aircraft using tangent hyperbolic hybrid nanofluid: A solar thermal application. J. Mater. Res. Technol. 2021, 14, 985–1006. [Google Scholar] [CrossRef]
- Jamshed, W.; Nisar, K.S.; Ibrahim, R.W.; Mukhtar, T.; Vijayakumar, V.; Ahmad, F. Computational frame work of Cattaneo-Christov heat flux effects on Engine Oil based Williamson hybrid nanofluids: A thermal case study. Case Stud. Therm. Eng. 2021, 26, 101179. [Google Scholar] [CrossRef]
- Jamshed, W.; Mishra, S.R.; Pattnaik, P.K.; Nisar, K.S.; Devi, S.S.U.; Prakash, M.; Shahzad, F.; Hussain, M.; Vijayakumar, V. Features of entropy optimization on viscous second grade nanofluid streamed with thermal radiation: A Tiwari and Das model. Case Stud. Therm. Eng. 2021, 27, 101291. [Google Scholar] [CrossRef]
- Jamshed, W.; Nasir, N.A.A.M.; Mohamed Isa, S.S.P.; Safdar, R.; Shahzad, F.; Nisar, K.S.; Eid, M.R.; Abdel-Aty, A.H.; Yahia, I.S. Thermal growth in solar water pump using Prandtl–Eyring hybrid nanofluid: A solar energy application. Sci. Rep. 2021, 11, 18704. [Google Scholar] [CrossRef]
- Jamshed, W. Finite element method in thermal characterization and streamline flow analysis of electromagnetic silver-magnesium oxide nanofluid inside grooved enclosure. Int. Commun. Heat Mass Transf. 2021, 130, 105795. [Google Scholar] [CrossRef]
- Jamshed, W.; Eid, M.R.; Hussain, S.M.; Abderrahmane, A.; Safdar, R.; Younis, O.; Pasha, A.A. Physical specifications of MHD mixed convective of Ostwald-de Waele nanofluids in a vented-cavity with inner elliptic cylinder. Int. Commun. Heat Mass Transf. 2022, 134, 106038. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ayub, A.; Sajid, T.; Jamshed, W.; Zamora, W.R.M.; More, L.A.V.; Talledo, L.M.G.; Rodríguez Ortega de Peña, N.I.; Hussain, S.M.; Hafeez, M.B.; Krawczuk, M. Activation Energy and Inclination Magnetic Dipole Influences on Carreau Nanofluid Flowing via Cylindrical Channel with an Infinite Shearing Rate. Appl. Sci. 2022, 12, 8779. https://doi.org/10.3390/app12178779
Ayub A, Sajid T, Jamshed W, Zamora WRM, More LAV, Talledo LMG, Rodríguez Ortega de Peña NI, Hussain SM, Hafeez MB, Krawczuk M. Activation Energy and Inclination Magnetic Dipole Influences on Carreau Nanofluid Flowing via Cylindrical Channel with an Infinite Shearing Rate. Applied Sciences. 2022; 12(17):8779. https://doi.org/10.3390/app12178779
Chicago/Turabian StyleAyub, Assad, Tanveer Sajid, Wasim Jamshed, William Rolando Miranda Zamora, Leandro Alonso Vallejos More, Luz Marina Galván Talledo, Nélida Isabel Rodríguez Ortega de Peña, Syed M. Hussain, Muhammad Bilal Hafeez, and Marek Krawczuk. 2022. "Activation Energy and Inclination Magnetic Dipole Influences on Carreau Nanofluid Flowing via Cylindrical Channel with an Infinite Shearing Rate" Applied Sciences 12, no. 17: 8779. https://doi.org/10.3390/app12178779
APA StyleAyub, A., Sajid, T., Jamshed, W., Zamora, W. R. M., More, L. A. V., Talledo, L. M. G., Rodríguez Ortega de Peña, N. I., Hussain, S. M., Hafeez, M. B., & Krawczuk, M. (2022). Activation Energy and Inclination Magnetic Dipole Influences on Carreau Nanofluid Flowing via Cylindrical Channel with an Infinite Shearing Rate. Applied Sciences, 12(17), 8779. https://doi.org/10.3390/app12178779








