Super-Operator Linear Equations and Their Applications to Quantum Antennas and Quantum Light Scattering
Abstract
:1. Introduction
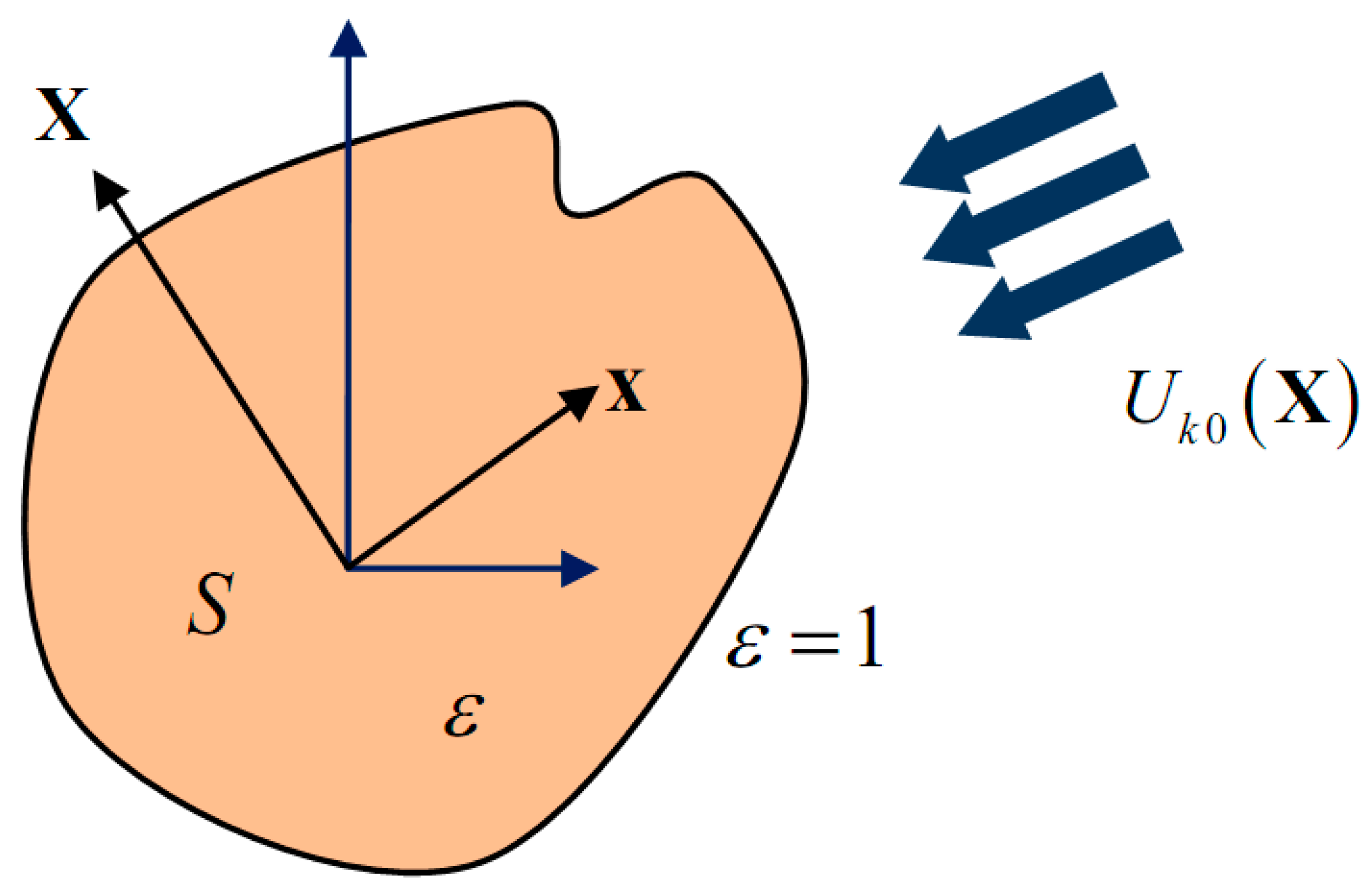
2. Super-Operator Integral Equation for Scattering of Quantum Light by a Dielectric Body
2.1. Preliminaries
2.2. Commutation Relation and Noise Component
2.3. Observable Values
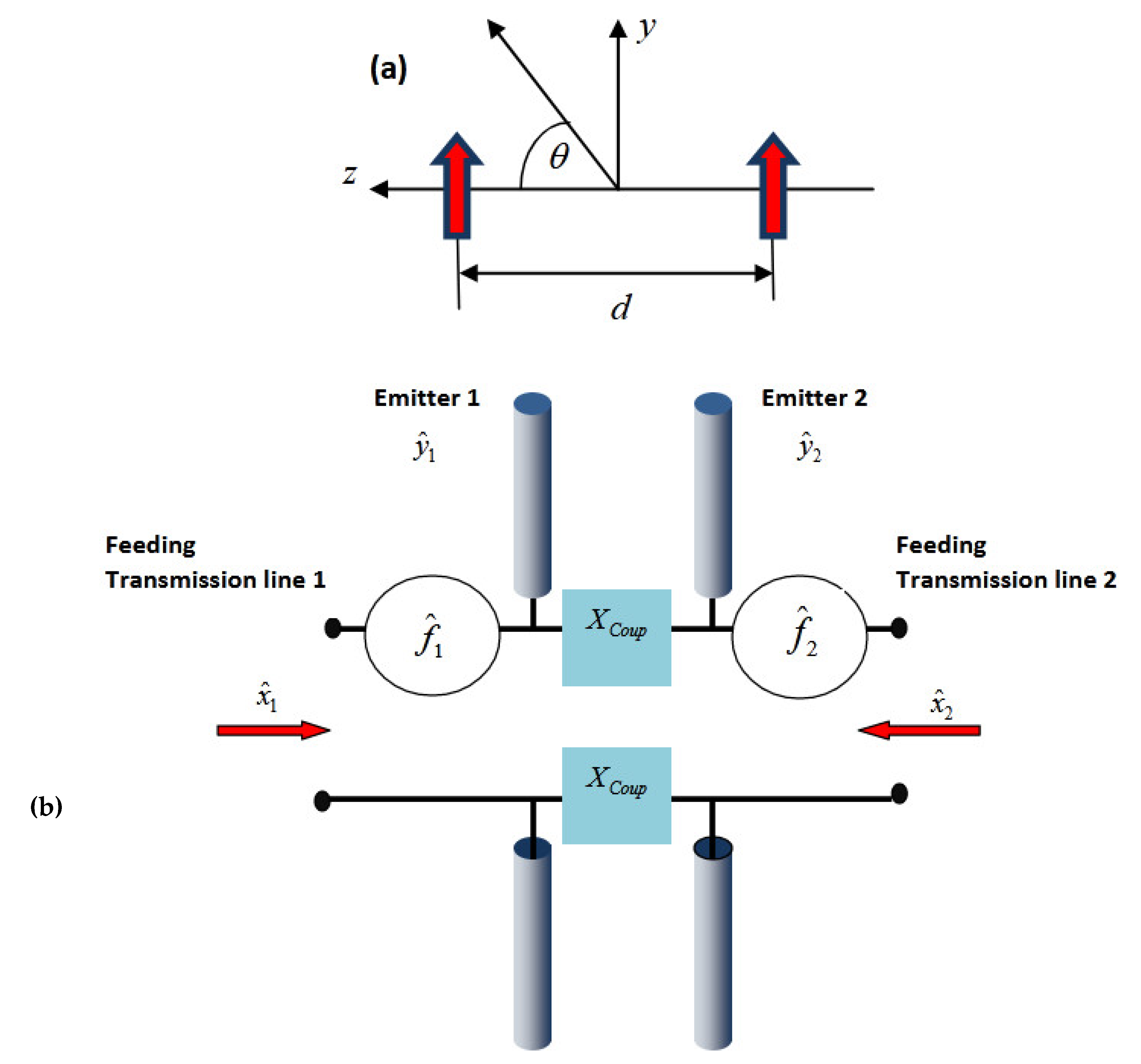
3. Application to the Quantum Two-Element Antenna Array
3.1. Quantum Noise and Commutation Relations
3.2. Transformation of the Statistical Properties of Quantum Light via the Quantum Antenna
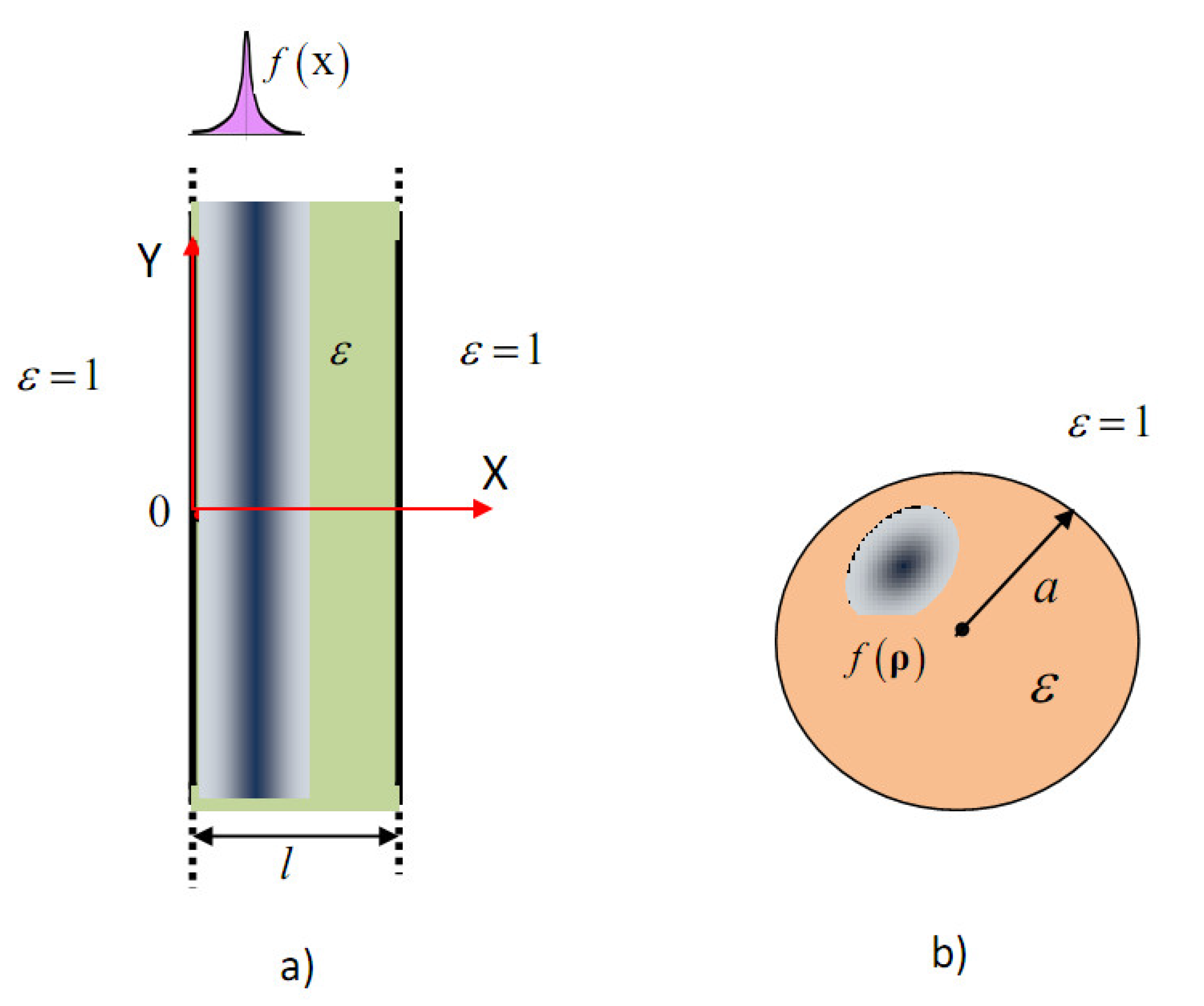
4. Planar Dielectric Layer: Reflection–Transmission
5. Scattering by the Dielectric Cylinder
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
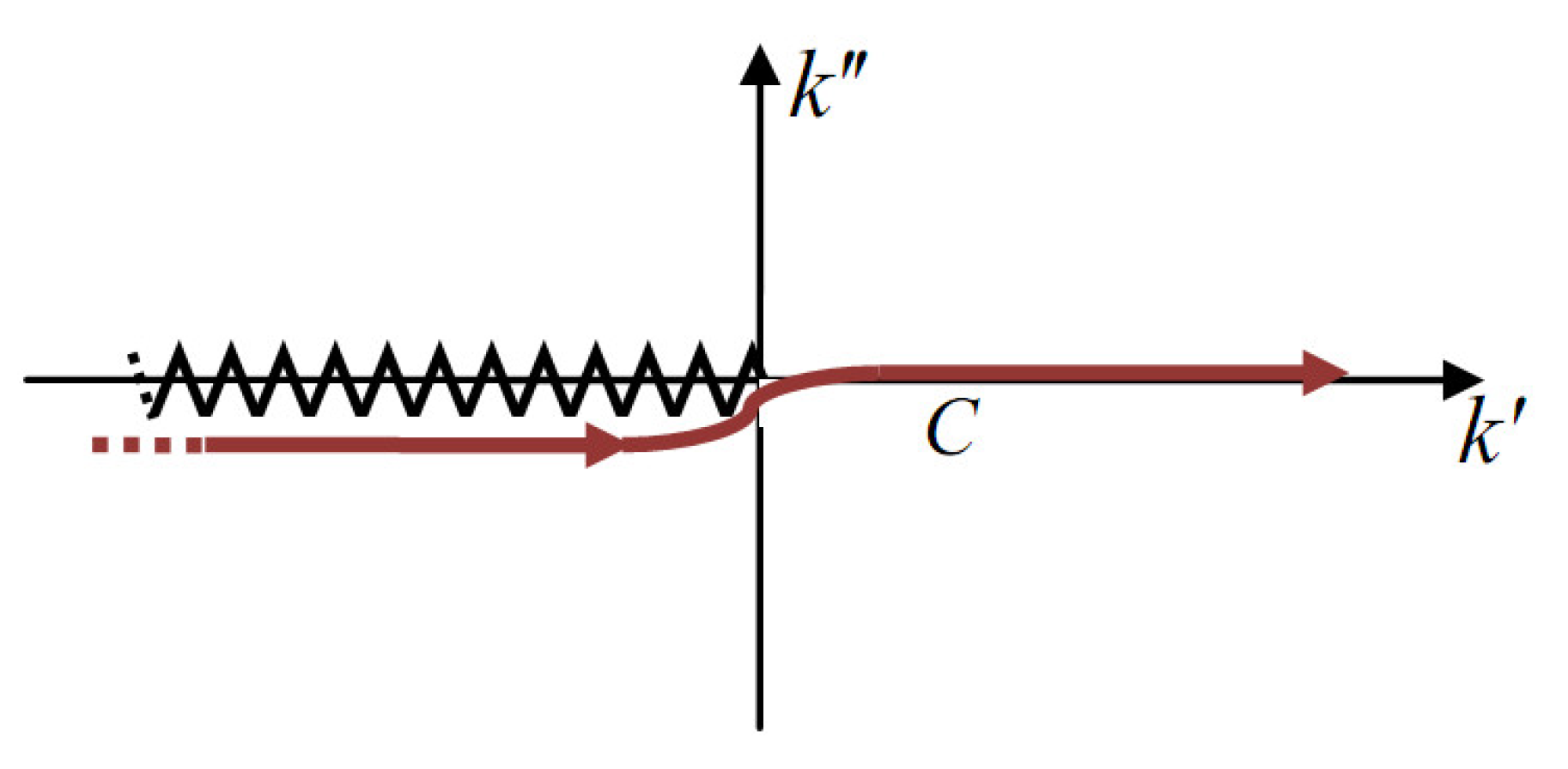
Appendix A. Resolvent Theory for Classical Operators
Appendix B. Quantization of Free Electromagnetic Field on A Cylindrical Basis
Appendix C. Derivation of Equation (8)
Appendix D. Derivation of Equations (52) and (53)
References
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information, 10th ed.; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Blais, A.; Girvin, S.M.; Oliver, W.D. Quantum information processing and quantum optics with circuit quantum electrodynamics. Nat. Phys. 2020, 16, 247–256. [Google Scholar] [CrossRef]
- Kok, P.; Munro, W.J.; Nemoto, K.; Ralph, T.C.; Dowling, J.P.; Milburn, G.J. Linear optical quantum computing with photonic qubits. Rev. Mod. Phys. 2007, 79, 135. [Google Scholar] [CrossRef]
- Zellinger, A. Light for the quantum. Entangled photons and their applications: A very personal perspective. Phys. Scr. 2017, 92, 072501. [Google Scholar] [CrossRef]
- Preskill, J. Quantum computing in the NISQ era and beyond. arXiv 2018, arXiv:1801.00862. [Google Scholar] [CrossRef]
- Clerk, A.; Lehnert, K.W.; Bertet, P.; Petta, J.R.; Nakamura, Y. Hybrid quantum systems with circuit quantum electrodynamics. Nat. Phys. 2020, 16, 257–267. [Google Scholar] [CrossRef]
- Komarov, A.; Slepyan, G. Quantum Antenna as an Open System: Strong Antenna Coupling with Photonic Reservoir. Appl. Sci. 2018, 8, 951. [Google Scholar] [CrossRef]
- Mokhlespour, S.; Haverkort, J.E.M.; Slepyan, G.; Maksimenko, S.; Hoffmann, A. Collective spontaneous emission in coupled quantum dots: Physical mechanism of quantum nanoantenna. Phys. Rev. B 2012, 86, 245322. [Google Scholar] [CrossRef]
- Slepyan, G.Y.; Yerchak, Y.D.; Maksimenko, S.A.; Hoffmann, A.; Bass, F.G. Mixed states in Rabi waves and quantum nanoantennas. Phys. Rev. B 2012, 85, 245134. [Google Scholar] [CrossRef]
- Mikhalychev, A.; Mogilevtsev, D.; Slepyan, G.Y.; Karuseichyk, I.; Buchs, G.; Boiko, D.L.; Boag, A. Synthesis of Quantum Antennas for Shaping Field Correlations. Phys. Rev. Appl. 2018, 9, 024021. [Google Scholar] [CrossRef]
- Slepyan, G.Y.; Boag, A. Quantum Nonreciprocity of Nanoscale Antenna Arrays in Timed Dicke States. Phys. Rev. Lett. 2013, 111, 023602. [Google Scholar] [CrossRef]
- Slepyan, G.Y. Heisenberg uncertainty principle and light squeezing in quantum nanoantennas and electric circuits. J. Nanophoton. 2016, 10, 046005. [Google Scholar] [CrossRef]
- Slepyan, G.Y.; Vlasenko, S.; Mogilevtsev, D. Quantum Antennas. Adv. Quantum Technol. 2020, 3, 1900120. [Google Scholar] [CrossRef]
- Lanzagorta, M. Quantum Radar, Synthesis Lectures on Quantum Computing; Morgan and Claypool Publishers: San Rafael, CA, USA, 2011. [Google Scholar]
- Peshko, I.; Mogilevtsev, D.; Karuseichyk, I.; Mikhalychev, A.; Nizovtsev, A.P.; Slepyan, G.Y.; Boag, A. Quantum noise radar: Superresolution with quantum antennas by accessing spatiotemporal correlations. Opt. Express 2019, 27, 29217. [Google Scholar] [CrossRef] [PubMed]
- Slepyan, G.; Vlasenko, S.; Mogilevtsev, D.; Boag, A. Quantum Radars and Lidars: Concepts, realizations, and perspectives. IEEE Antennas Propag. Mag. 2022, 64, 16–26. [Google Scholar] [CrossRef]
- Dowling, J.P.; Seshadreesan, K.P. Quantum optical technologies for metrology, sensing and imaging. arXiv 2015, arXiv:1412.7578v2. [Google Scholar] [CrossRef]
- Balanis, C.A. Antenna Theory; John Wiley and Sons, Inc.: New York, NY, USA, 1997. [Google Scholar]
- Jackson, J. Classical Electrodynamics; John Wiley & Sons, Inc.: New York, NY, USA, 1962. [Google Scholar]
- Dzsotjan, D.; Rousseaux, B.; Jauslin, H.R.; Colas des Francs, G.; Couteau, C.; Guerin, S. Mode-selective quantization and multimodal effective models for spherically layered systems. Phys. Rev. A 2016, 94, 023818. [Google Scholar] [CrossRef]
- Gutiérrez-Jáuregui, R.; Jáuregui, R. Photons in the presence of parabolic mirrors. Phys. Rev. A 2018, 98, 043808. [Google Scholar] [CrossRef]
- Xiao, Z.; Lanning, R.N.; Zhang, M.; Novikova, I.; Mikhailov, E.E.; Dowling, J.P. Why a hole is like a beam splitter: A general diffraction theory for multimode quantum states of light. Phys. Rev. A 2017, 96, 023829. [Google Scholar] [CrossRef]
- Goldberg, A.Z.; James, D.F.V. Entanglement generation via diffraction. Phys. Rev. A 2019, 100, 042332. [Google Scholar] [CrossRef]
- Leonhardt, U. Quantum physics of simple optical instruments. Rep. Prog. Phys. 2003, 66, 1207–1249. [Google Scholar] [CrossRef]
- Garbacz, R.J. Modal expansions for resonance scattering phenomena. Proc. IEEE 1965, 53, 856–864. [Google Scholar] [CrossRef]
- Garbacz, R.J.; Turpin, R.H. A generalized expansion for radiated and scattered fields. IEEE Trans. Antennas Propag. 1971, 19, 348–358. [Google Scholar] [CrossRef]
- Garbacz, R.J.; Pozar, D.M. Antenna shape synthesis using characteristic modes. IEEE Trans. Antennas Propag. 1982, 30, 340–350. [Google Scholar] [CrossRef]
- Harrington, R.F.; Mautz, J.R. Theory of characteristic modes for conducting bodies. IEEE Trans. Antennas Propag. 1971, 19, 622–628. [Google Scholar] [CrossRef]
- Harrington, R.F.; Mautz, J.R. Computation of characteristic modes for conducting bodies. IEEE Trans. Antennas Propag. 1971, 19, 629–639. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, C.-F. Characteristic Modes: Theory and Applications in Antenna Engineering; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2015. [Google Scholar]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Section 19.1 Fredholm Equations of the Second Kind. In Numerical Recipes: The Art of Scientific Computing, 3rd ed.; Cambridge University Press: New York, NY, USA, 2007. [Google Scholar]
- Chaskalovic, J. Finite Elements Methods for Engineering Sciences; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Strikwerda, J. Finite Difference Schemes and Partial Differential Equations, 2nd ed.; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2004. [Google Scholar]
- Levie, I.; Slepyan, G.Y.; Mogilevtsev, D.; Boag, A. Multimode Quantum Light Scattering: Method of Characteristic Modes. In Proceedings of the International Conference on Microwaves, Communications, Antennas & Electronic Systems, IEEE COMCAS, Tel Aviv, Israel, 1–3 November 2021. [Google Scholar]
- Slepyan, G.Y.; Mogilevtsev, D.; Levie, I.; Boag, A. Modeling of Multimodal Scattering by Conducting Bodies in Quantum Optics: The Method of Characteristic Modes. Phys. Rev. Appl. 2022, 18, 014024. [Google Scholar] [CrossRef]
- Savasta, S.; Di Stefano, O.; Girlanda, R. Light quantization for arbitrary scattering systems. Phys. Rev. B 2002, 65, 043801. [Google Scholar] [CrossRef]
- Na, D.-Y.; Zhu, J.; Chew, W.C.; Teixeira, F.L. Quantum information preserving computational electromagnetic. Phys. Rev. A 2020, 102, 013711. [Google Scholar] [CrossRef]
- Scully, M.O.; Zubairy, M.S. Quantum Optics; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Agarwal, G.S. Quantum Optics; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of N-level systems. J. Math. Phys. 1976, 17, 821. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119. [Google Scholar] [CrossRef]
- Breuer, H.-P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Fabre, C.; Treps, N. Modes and states in quantum optics. Rev. Mod. Phys. 2020, 92, 035005. [Google Scholar] [CrossRef]
- Barnett, S.M.; Jeffers, J.; Gatti, A.; Loudon, R. Quantum optics of lossy beam splitters. Phys. Rev. A 1998, 57, 2134–2145. [Google Scholar] [CrossRef]
- Hanson, G.W. Aspects of quantum electrodynamics compared to the classical case: Similarity and disparity of quantum and classical electromagnetic. IEEE Antennas Propag. Mag. 2020, 62, 16–26. [Google Scholar] [CrossRef]
- Felsen, L.B.; Marcuvitz, N. Radiation and Scattering of Waves; IEEE Press Series on Electromagnetic Waves; Prentice-Hall: Englewood Cliffs, NJ, USA, 1972. [Google Scholar]
- Pozar, D.M. Microwave Engineering, 4th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Peterson, A.F.; Ray, S.L.; Mittra, R. Computational Methods for Electromagnetics; IEEE Press Series on Electromagnetic Wave Theory; Wiley-IEEE Press: Hoboken, NJ, USA, 1997. [Google Scholar]
- Prasad, S.; Scully, M.O.; Martienssen, W. A quantum description of the beam splitter. Opt. Commun. 1987, 62, 139–145. [Google Scholar] [CrossRef]
- Patera, G.; Horoshko, D.B.; Kolobov, M.I. Space-time duality and quantum temporal imaging. Phys. Rev. A 2018, 98, 053815. [Google Scholar] [CrossRef]
- Engheta, N.; Ziolkowski, R.W. Metamaterials: Physics and Engineering Explorations; Wiley Online Library: Hoboken, NJ, USA, 2006. [Google Scholar]
- Biagioni, P.; Huang, J.-S.; Hecht, B. Nanoantennas for visible and infrared radiation. Rep. Prog. Phys. 2012, 75, 024402. [Google Scholar] [CrossRef]
- Novotny, L.; van Hulst, N. Antennas for light. Nat. Photonics 2011, 5, 83. [Google Scholar] [CrossRef]
- Alu, A.; Engheta, N. Input Impedance, Nanocircuit Loading, and Radiation Tuning of Optical Nanoantennas. Phys. Rev. Lett. 2008, 101, 043901. [Google Scholar] [CrossRef]
- Monticone, F.; Argyropoulos, C.; Alù, A. Optical antennas, controlling electromagnetic scattering, radiation, and emission at the nanoscale. IEEE Antennas Propag. Mag. 2017, 59, 43. [Google Scholar] [CrossRef]
- Fredholm, E.I. Sur une classe d’equations fonctionnelles. Acta Math. 1903, 27, 365–390. [Google Scholar] [CrossRef]
- Agranovich, M.S.; Katsenelenbaum, B.Z.; Sivov, A.N.; Voitovich, N.N. Generalized Method of Eigenoscillations in Diffraction Theory; John Wiley & Sons, Inc.: New York, NY, USA, 1999. [Google Scholar]
- Edmunds, D.E.; Evans, W.D. Spectral Theory and Differential Operators; Oxford University Press: Oxford, UK, 1987. [Google Scholar]
- Abramowitz, M.; Stegun, I.A.; Romer, R.H. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Table; Applied Mathematics Series; National Bureau of Standards: Gaithersburg, MD, USA, 1972. [Google Scholar]
- Novotny, L.; Hecht, B. Principles of Nano-Optics; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Slepyan, G.; Boag, A. Super-Operator Linear Equations and Their Applications to Quantum Antennas and Quantum Light Scattering. Appl. Sci. 2022, 12, 8498. https://doi.org/10.3390/app12178498
Slepyan G, Boag A. Super-Operator Linear Equations and Their Applications to Quantum Antennas and Quantum Light Scattering. Applied Sciences. 2022; 12(17):8498. https://doi.org/10.3390/app12178498
Chicago/Turabian StyleSlepyan, Gregory, and Amir Boag. 2022. "Super-Operator Linear Equations and Their Applications to Quantum Antennas and Quantum Light Scattering" Applied Sciences 12, no. 17: 8498. https://doi.org/10.3390/app12178498
APA StyleSlepyan, G., & Boag, A. (2022). Super-Operator Linear Equations and Their Applications to Quantum Antennas and Quantum Light Scattering. Applied Sciences, 12(17), 8498. https://doi.org/10.3390/app12178498





