Development of a Novel Gear-like Disk Resonator Applied in Gyroscope
Abstract
:1. Introduction
2. Design and Analysis
2.1. Structure Design
2.2. Theory
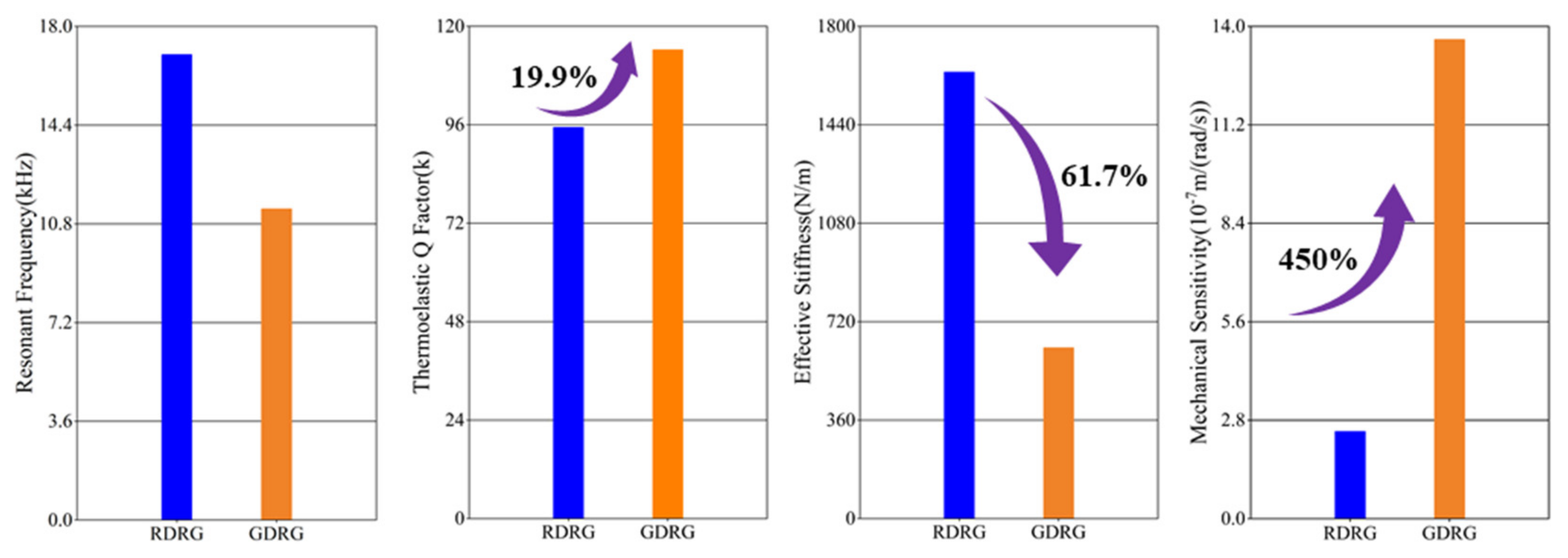
2.3. FEM Simulations
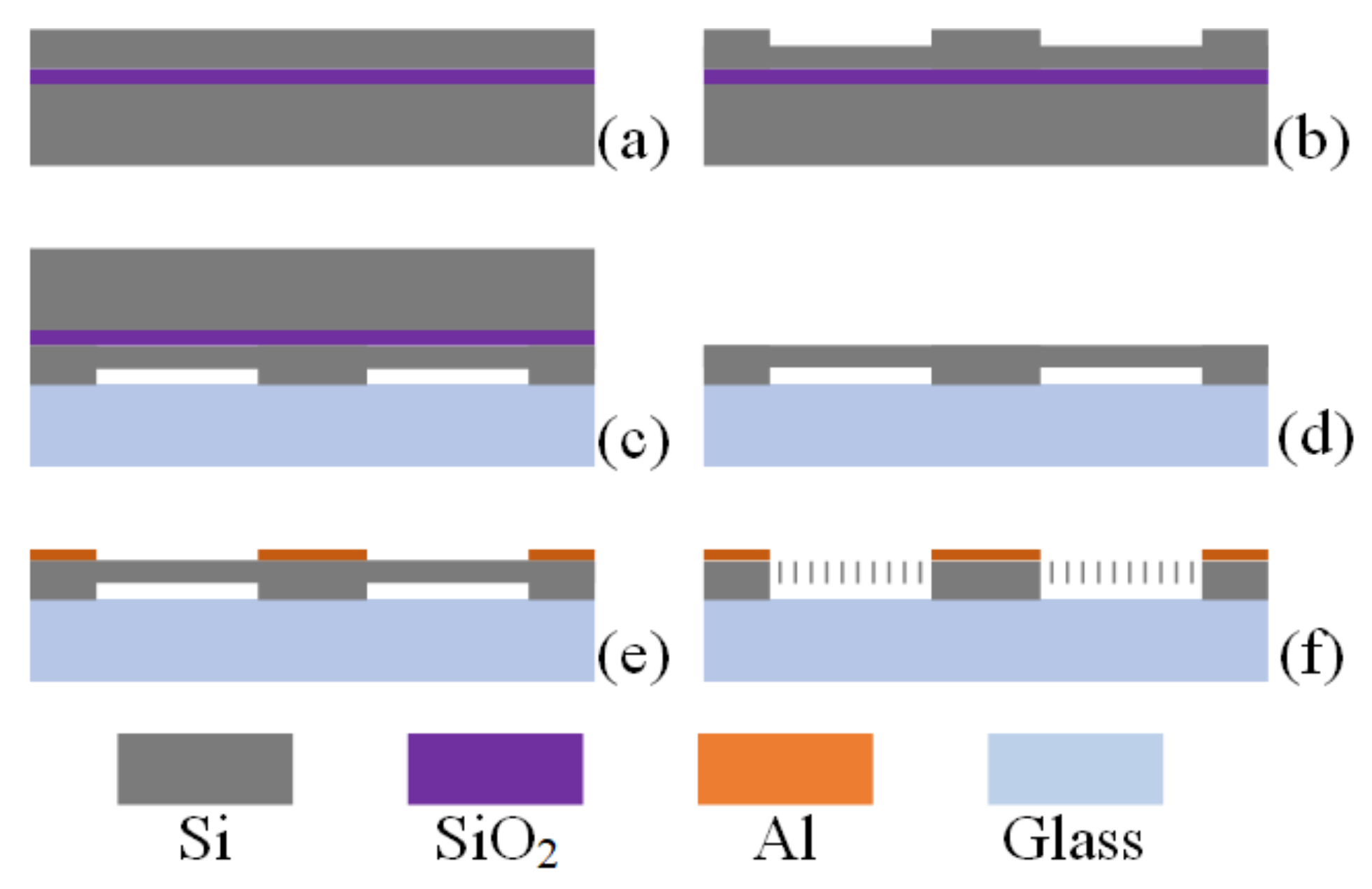
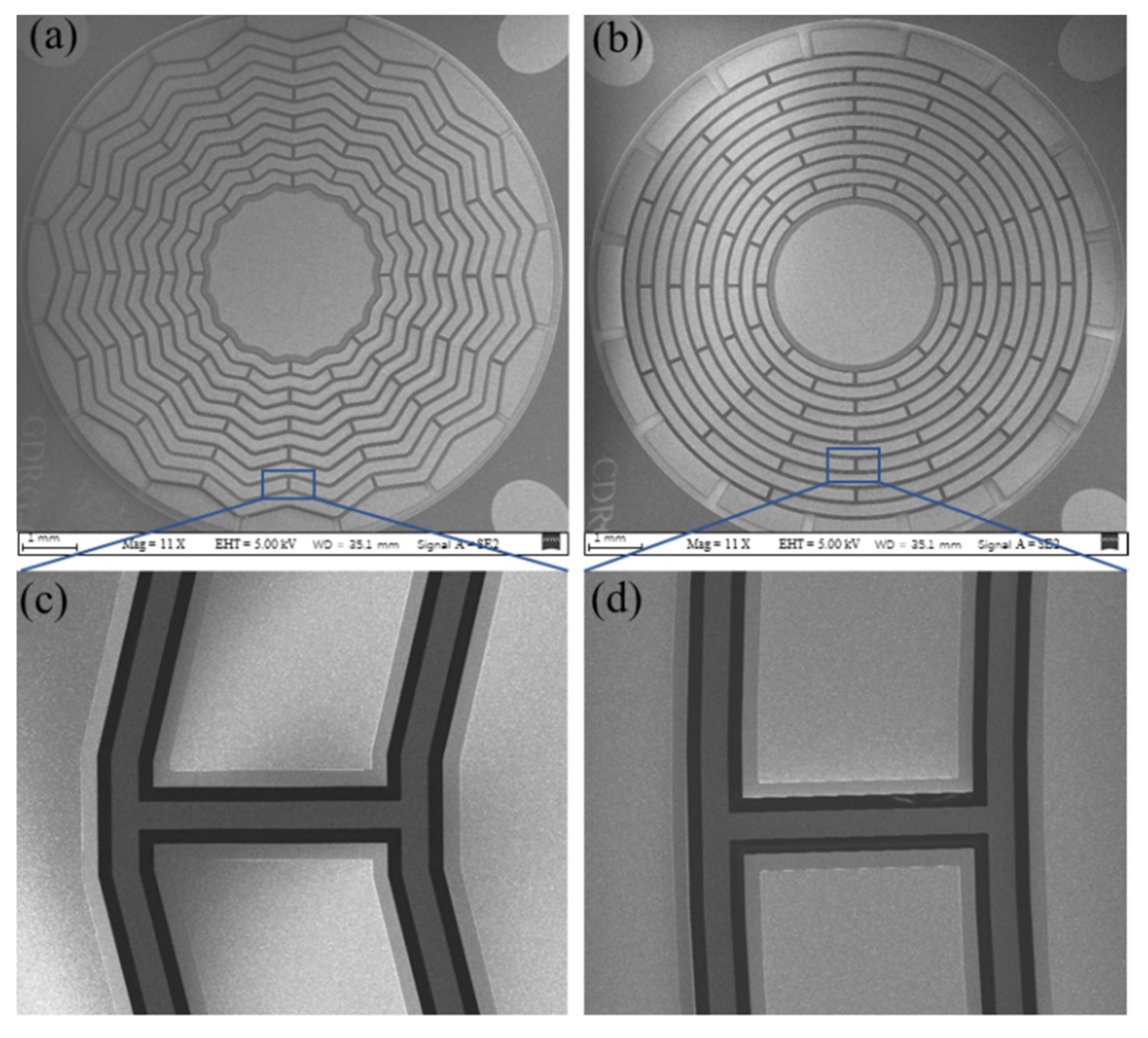
3. Fabrication Processes
4. Resonator Characterization
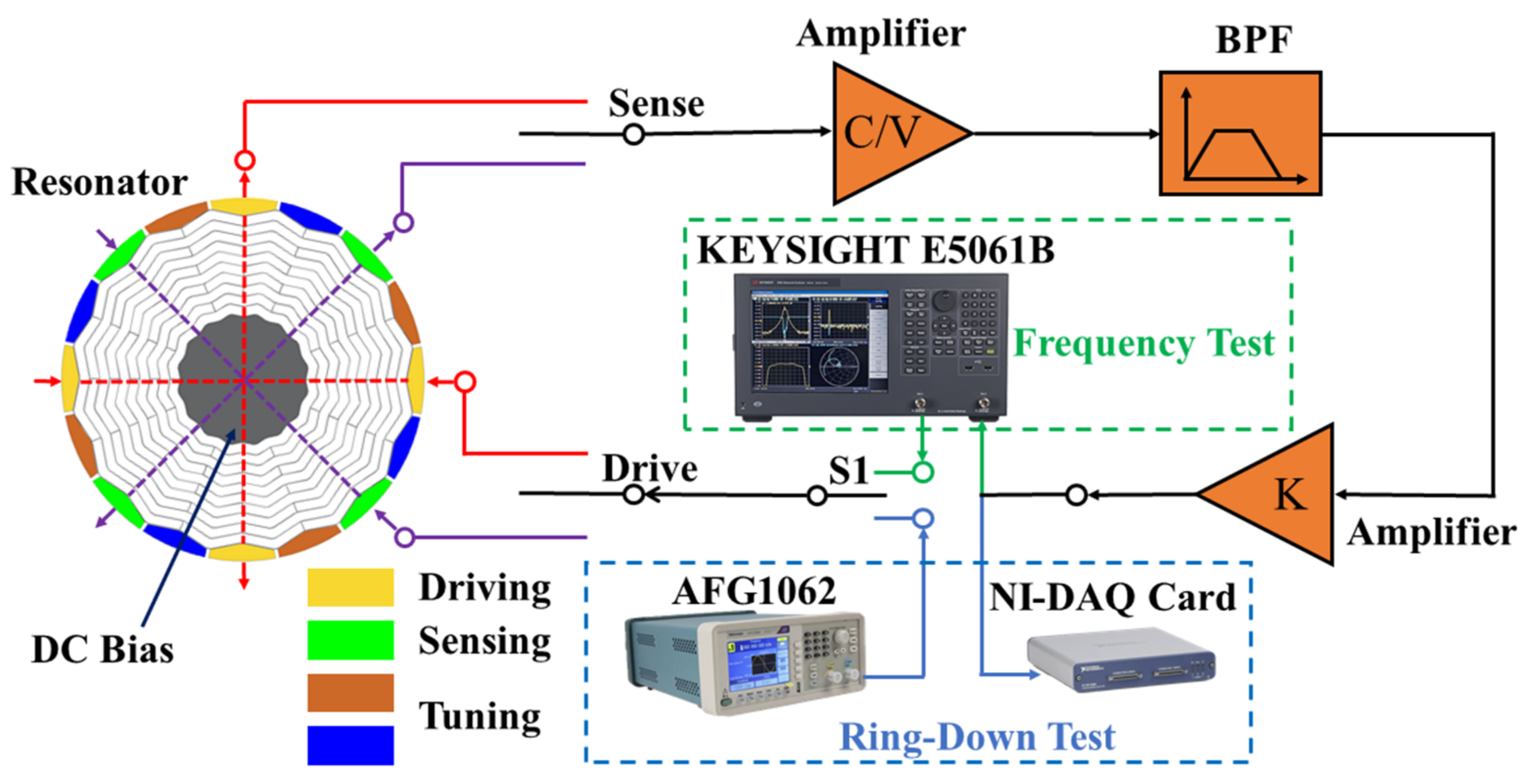
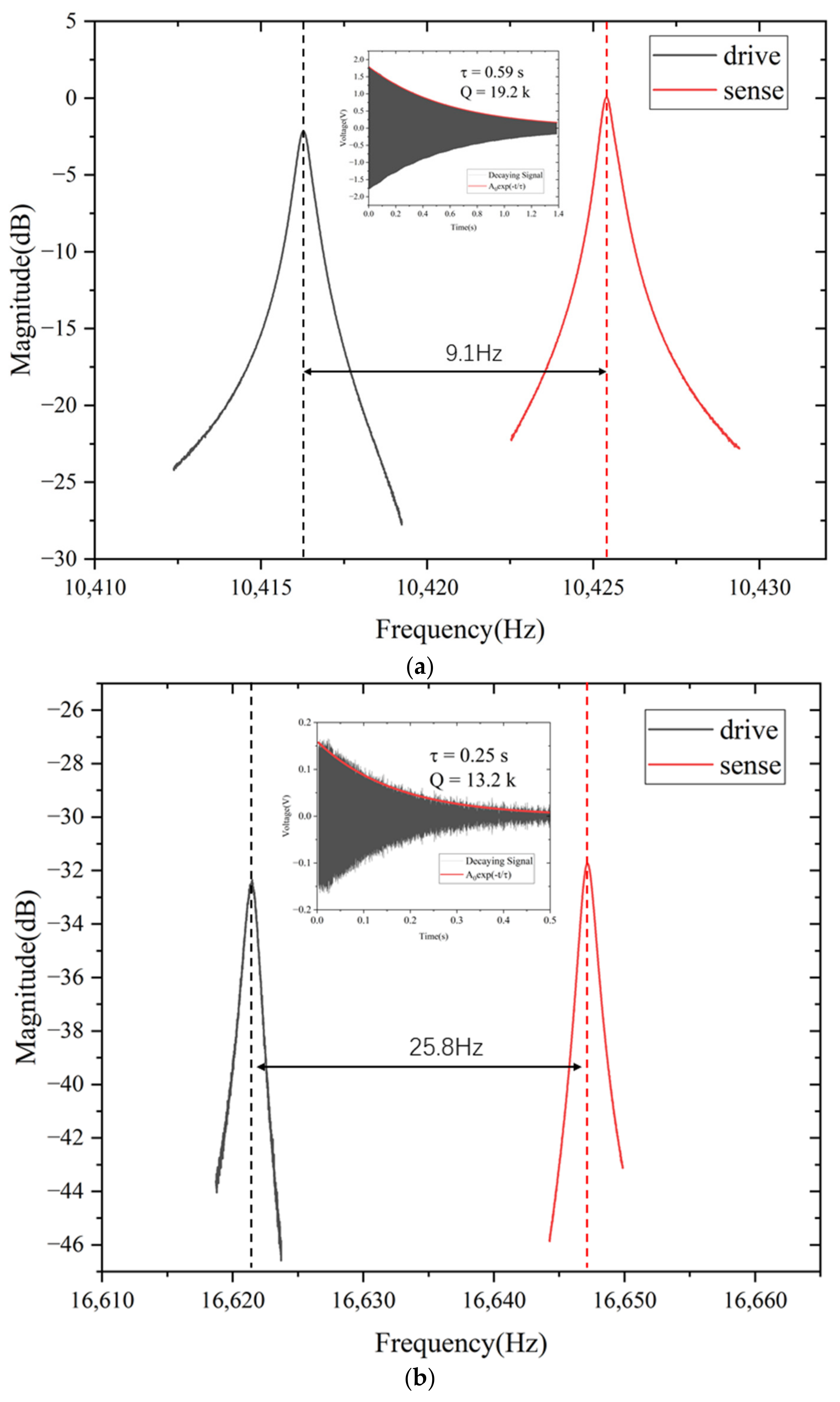
4.1. Frequency Response Test
4.2. Ring-Down Test
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lammel, G. The Future of Mems Sensors in Our Connected World. In Proceedings of the 2015 28th IEEE International Conference on Micro Electro Mechanical Systems (Mems 2015), Estoril, Portugal, 18–22 January 2015; pp. 61–64. [Google Scholar]
- Challoner, A.D.; Howard, H.G.; Liu, J.Y. Boeing disc resonator gyroscope. In Proceedings of the 2014 IEEE/ION Position, Location and Navigation Symposium-PLANS 2014, Monterey, CA, USA, 5–8 May 2014. [Google Scholar]
- Shkel, A.M. Type I and Type II Micromachined Vibratory Gyroscopes. In Proceedings of the 2006 IEEE/Ion. Position, Location and Navigation Symposium, San Diego, CA, USA, 25–27 April 2006;–3; Volume 1–3, pp. 586–593. [Google Scholar]
- Feng, J.; Zhang, W.P.; Gu, L.T.; Liu, Z.Y. Design of a novel gear-like disk resonator gyroscope with high mechanical sensitivity. Microsyst. Technol. 2021, 27, 2715–2722. [Google Scholar] [CrossRef]
- Gerrard, D.D.; Rodriguez, J.; Ortiz, L.C.; Chandorkar, S.A.; Flader, I.B.; Chen, Y.H.; Shin, D.D.; Kenny, T.W. Manipulation of Heat Flux Paths in Thermo-Elastically Damped Resonators for Q Optimization. In Proceedings of the 30th IEEE International Conference on Micro Electro. Mechanical Systems (Mems 2017), Las Vegas, NV, USA, 22–26 January 2017; pp. 1130–1133. [Google Scholar]
- Xia, D.Z.; Huang, L.C.; Xu, L.; Gao, H.Y. Structural Analysis of Disk Resonance Gyroscope. Micromachines 2017, 8, 296. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gerrard, D.D.; Ahn, C.H.; Flader, I.B.; Chen, Y.H.; Ng, E.J.; Yang, Y.S.; Kenny, T.W. Q-Factor Optimization in Disk Resonator Gyroscopes Via Geometric Parameterization. In Proceedings of the 2016 IEEE 29th International Conference on Micro Electro. Mechanical Systems (Mems), Shanghai, China, 24–28 January 2016; pp. 994–997. [Google Scholar]
- Li, Q.S.; Xiao, D.B.; Zhou, X.; Hou, Z.Q.; Xu, Y.; Wu, X.Z. Quality Factor Improvement in the Disk Resonator Gyroscope by Optimizing the Spoke Length Distribution. J. Microelectromech. Syst. 2018, 27, 414–423. [Google Scholar] [CrossRef]
- Li, Q.S.; Xiao, D.B.; Zhou, X.; Xu, Y.; Zhuo, M.; Hou, Z.G.; He, K.X.; Zhang, Y.M.; Wu, X.Z. 0.04 degree-per-hour MEMS disk resonator gyroscope with high-quality factor (510 k) and long decaying time constant (74.9 s). Microsyst. Nanoeng. 2018, 4, 32. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Luo, X.; Xiao, D.; Li, Q.; Hou, Z.; He, K.; Wu, Y.; Wu, X. Investigation on the way of adding lumped masses on disk resonator gyroscope. In Proceedings of the 2017 IEEE Sensors, Glasgow, UK, 29 October–1 November 2017. [Google Scholar]
- Zhou, X.; Xiao, D.; Wu, X.; Wu, Y.; Hou, Z.; He, K.; Li, Q. Stiffness-mass decoupled silicon disk resonator for high resolution gyroscopic application with long decay time constant (8.695 s). Appl. Phys. Lett. 2016, 109, 263501. [Google Scholar] [CrossRef]
- Ko, S.D.; Hamelin, B.; Yang, J.; Ayazi, F. High-Q Monocrystalline Silicon Carbide Disk Resonators Fabricated Using Drie of Thick Sic-on-Insulator Substrates. In Proceedings of the 2018 IEEE Micro Electro. Mechanical Systems (Mems), Belfast, UK, 21–25 January 2018; pp. 6–999. [Google Scholar]
- Giner, J.; Maeda, D.; Ono, K.; Shkel, A.M.; Sekiguchi, T. MEMS Gyroscope with Concentrated Springs Suspensions Demonstrating Single Digit Frequency Split and Temperature Robustness. J. Microelectromech. Syst. 2019, 28, 25–35. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Q.; Wang, P.; Zhang, Y.; Zhou, X.; Yu, L.; Wu, X.; Xiao, D. 0.015 Degree-Per-Hour Honeycomb Disk Resonator Gyroscope. IEEE Sens. J. 2020, 21, 7326–7338. [Google Scholar] [CrossRef]
- Fan, B.; Guo, S.W.; Cheng, M.M.; Yu, L.; Zhou, M.; Hu, W.Y.; Chen, Z.A.; Xu, D.C. A Novel High-Symmetry Cobweb-Like Disk Resonator Gyroscope. IEEE Sens. J. 2019, 19, 10289–10297. [Google Scholar] [CrossRef]
- Efimovskaya, A.; Wang, D.; Lin, Y.-W.; Shkel, A.M. Electrostatic compensation of structural imperfections in dynamically amplified dual-mass gyroscope. Sens. Actuators A Phys. 2018, 275, 99–108. [Google Scholar] [CrossRef]
- Xiao, D.B.; Yu, D.C.; Zhou, X.; Hou, Z.Q.; He, H.H.; Wu, X.Z. Frequency Tuning of a Disk Resonator Gyroscope via Stiffness Perturbation. IEEE Sens. J. 2017, 17, 4725–4734. [Google Scholar] [CrossRef]
- Dong Joon, K.; M’Closkey, R.T. A systematic method for tuning the dynamics of electrostatically actuated vibratory gyros. IEEE Trans. Control Syst. Technol. 2006, 14, 69–81. [Google Scholar] [CrossRef]
- Gallacher, B.J.; Hedley, J.; Burdess, J.S.; Harris, A.J.; Rickard, A.; King, D.O. Electrostatic correction of structural imperfections present in a microring gyroscope. J. Microelectromech. Syst. 2005, 14, 221–234. [Google Scholar] [CrossRef]
- Taheri-Tehrani, P.; Kline, M.; Izyumin, I.; Eminoglu, B.; Yeh, Y.-C.; Yang, Y.; Chen, Y.; Flader, I.; Ng, E.J.; Kenny, T.W.; et al. Epitaxially-encapsulated quad mass gyroscope with nonlinearity compensation. In Proceedings of the 2016 IEEE 29th International Conference on Micro Electro Mechanical Systems (MEMS), Shanghai, China, 24–28 January 2016. [Google Scholar]
- Songqi, H.; Hongjuan, C.; Kun, H.; Zhanqiang, H.; Peng, C.; Dingbang, X.; Xuezhong, W. A method of structural trimming to reduce mode coupling error for micro-gyroscopes. In Proceedings of the 8th Annual IEEE International Conference on Nano/Micro Engineered and Molecular Systems, Suzhou, China, 7–10 April 2013. [Google Scholar]
- Wang, Y.; Asadian, M.H.; Shkel, A.M. Compensation of frequency split by directional lapping in fused quartz micro wineglass resonators. J. Micromech. Microeng. 2018, 28, 095001. [Google Scholar] [CrossRef] [Green Version]
- Behbahani, A.H.; Kim, D.; Stupar, P.; DeNatale, J.; M’Closkey, R.T. Tailored Etch Profiles for Wafer-Level Frequency Tuning of Axisymmetric Resonators. J. Microelectromech. Syst. 2017, 26, 333–343. [Google Scholar] [CrossRef] [Green Version]
- Senkal, D.; Ahamed, M.J.; Trusov, A.A.; Shkel, A.M. Achieving Sub-Hz Frequency Symmetry in Micro-Glassblown Wineglass Resonators. J. Microelectromech. Syst. 2014, 23, 30–38. [Google Scholar] [CrossRef] [Green Version]
- Hsu, T.-R. MEMS and Microsystems: Design, Manufacture, and Nanoscale Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Acar, C.; Shkel, A. MEMS Vibratory Gyroscopes: Structural Approaches to Improve Robustness; Springer Science & Business Media: Berlin, Germany, 2008. [Google Scholar]
- Su, T.-H. Approaches for LOW Noise Sensing in MEMS Disk Gyros; University of California: Berkeley, CA, USA, 2016. [Google Scholar]
- Cho, J.Y. High-Performance Micromachined Vibratory Rate-and Rate-Integrating Gyroscopes. Ph.D. Thesis, University of Michigan, Ann Arbor, MI, USA, 2012. [Google Scholar]
- Ayazi, F.; Najafi, K. A HARPSS polysilicon vibrating ring gyroscope. J. Microelectromech. Syst. 2001, 10, 169–179. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.-O.; Chang, G.-E.; Chou, C.-S.; Chien, W.-T.C.; Chen, P.-C. In-plane free vibration of a single-crystal silicon ring. Int. J. Solids Struct. 2008, 45, 6114–6132. [Google Scholar] [CrossRef] [Green Version]



| Structure Parameters | Value |
|---|---|
| Anchor radius (r) | 1300 μm |
| Outer radius (R) | 3615 μm |
| Short beam length (Lsh) | 80 μm |
| Bending angle (θ) | 130° |
| Ring width (H) | 20 μm |
| Structure thickness (T) | 60 μm |
| Spoke length (L) | 190 μm |
| Ring number (N) | 10 |
| Material Parameters | Value |
|---|---|
| Elastic modulus (E) | 170 GPa |
| Thermal conductivity (κ) | 130 W/(m·K) |
| Coefficient of thermal expansion (αT) | 2.6 × 10−6 1/K |
| Constant pressure heat capacity (Cp) | 700 J/(kg·K) |
| Density (ρ) | 2329 kg/m3 |
| Poisson’s ratio (υ) | 0.28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, L.; Zhang, W.; Feng, J.; Zhang, Z. Development of a Novel Gear-like Disk Resonator Applied in Gyroscope. Appl. Sci. 2022, 12, 7342. https://doi.org/10.3390/app12147342
Gu L, Zhang W, Feng J, Zhang Z. Development of a Novel Gear-like Disk Resonator Applied in Gyroscope. Applied Sciences. 2022; 12(14):7342. https://doi.org/10.3390/app12147342
Chicago/Turabian StyleGu, Liutao, Weiping Zhang, Jun Feng, and Zhihan Zhang. 2022. "Development of a Novel Gear-like Disk Resonator Applied in Gyroscope" Applied Sciences 12, no. 14: 7342. https://doi.org/10.3390/app12147342
APA StyleGu, L., Zhang, W., Feng, J., & Zhang, Z. (2022). Development of a Novel Gear-like Disk Resonator Applied in Gyroscope. Applied Sciences, 12(14), 7342. https://doi.org/10.3390/app12147342







