Robustness for the Starting Point of Two Iterative Methods for Fitting Debye or Cole–Cole Models to a Dielectric Permittivity Spectrum †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Debye Model
2.2. Cole–Cole Model
2.3. Curve Fitting Algorithms
2.3.1. Levenberg–Marquardt Algorithm
2.3.2. Variable Projection Algorithm
2.4. Numerical Simulations
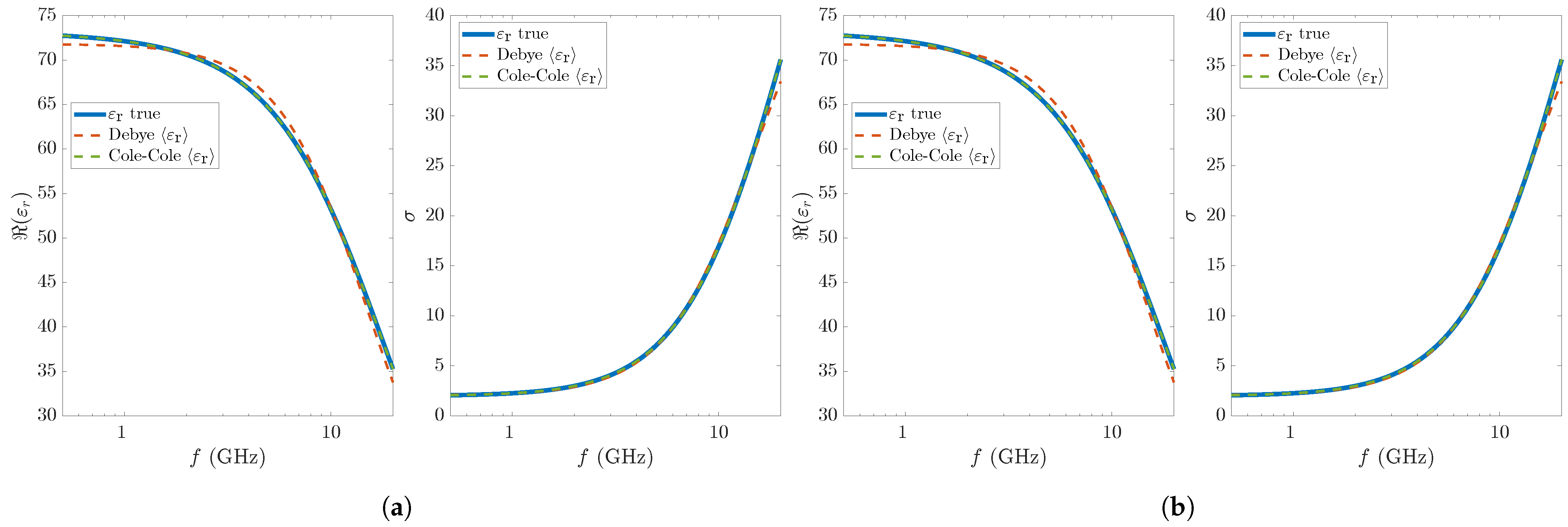
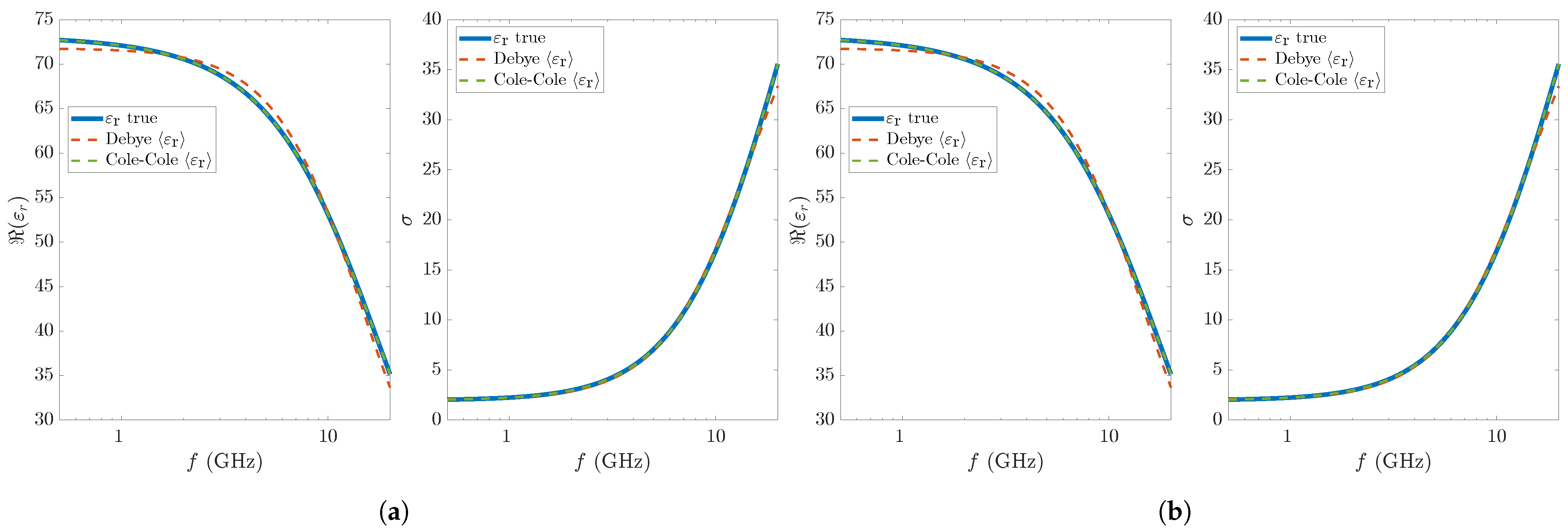
3. Results
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Elwakil, A.S.; Al-Ali, A.A.; Maundy, B.J. Extending the Double-Dispersion Cole–Cole, Cole–Davidson and Havriliak–Negami Electrochemical Impedance Spectroscopy Models. Eur. Biophys. J. 2021, 50, 915–926. [Google Scholar] [CrossRef]
- Naranjo-Hernández, D.; Reina-Tosina, J.; Min, M. Fundamentals, Recent Advances, and Future Challenges in Bioimpedance Devices for Healthcare Applications. J. Sens. 2019, 2019, e9210258. [Google Scholar] [CrossRef] [Green Version]
- Sasaki, K.; Wake, K.; Watanabe, S. Development of Best Fit Cole-Cole Parameters for Measurement Data from Biological Tissues and Organs between 1 MHz and 20 GHz. Radio Sci. 2014, 49, 459–472. [Google Scholar] [CrossRef]
- Fhager, A.; Gustafsson, M.; Nordebo, S. Image Reconstruction in Microwave Tomography Using a Dielectric Debye Model. IEEE Trans. Biomed. Eng. 2012, 59, 156–166. [Google Scholar] [CrossRef] [Green Version]
- Lazebnik, M.; Okoniewski, M.; Booske, J.H.; Hagness, S.C. Highly Accurate Debye Models for Normal and Malignant Breast Tissue Dielectric Properties at Microwave Frequencies. IEEE Microw. Wirel. Compon. Lett. 2007, 17, 822–824. [Google Scholar] [CrossRef]
- Yilmaz, T.; Foster, R.; Hao, Y. Broadband Tissue Mimicking Phantoms and a Patch Resonator for Evaluating Noninvasive Monitoring of Blood Glucose Levels. IEEE Trans. Antennas Propag. 2014, 62, 3064–3075. [Google Scholar] [CrossRef]
- Turgul, V.; Kale, I. On the Accuracy of Complex Permittivity Model of Glucose/Water Solutions for Non-Invasive Microwave Blood Glucose Sensing. In Proceedings of the 2015 E-Health and Bioengineering Conference (EHB), Iasi, Romania, 19–21 November 2015; pp. 1–4. [Google Scholar] [CrossRef]
- Turgul, V.; Kale, I. Characterization of the Complex Permittivity of Glucose/Water Solutions for Noninvasive RF/Microwave Blood Glucose Sensing. In Proceedings of the 2016 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Taipei, Taiwan, 23–26 May 2016; pp. 1–5. [Google Scholar] [CrossRef]
- Turgul, V.; Kale, I. Permittivity Extraction of Glucose Solutions through Artificial Neural Networks and Non-Invasive Microwave Glucose Sensing. Sens. Actuators A Phys. 2018, 277, 65–72. [Google Scholar] [CrossRef] [Green Version]
- Omer, A.E.; Shaker, G.; Safavi-Naeini, S.; Shubair, R.M. EM Measurements of Glucose-Aqueous Solutions. In Proceedings of the 2019 IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting, Atlanta, GA, USA, 7–12 July 2019; pp. 103–104. [Google Scholar] [CrossRef]
- Clegg, J.; Robinson, M.P. A Genetic Algorithm Used to Fit Debye Functions to the Dielectric Properties of Tissues. In Proceedings of the IEEE Congress on Evolutionary Computation, Barcelona, Spain, 18–23 July 2010; pp. 1–8. [Google Scholar] [CrossRef]
- Cruciani, S.; Santis, V.D.; Feliziani, M.; Maradei, F. Cole-Cole vs Debye Models for the Assessment of Electromagnetic Fields inside Biological Tissues Produced by Wideband EMF Sources. In Proceedings of the 2012 Asia-Pacific Symposium on Electromagnetic Compatibility, Singapore, 21–24 May 2012; pp. 685–688. [Google Scholar] [CrossRef]
- Grosse, C. A Program for the Fitting of Debye, Cole–Cole, Cole–Davidson, and Havriliak–Negami Dispersions to Dielectric Data. J. Colloid Interface Sci. 2014, 419, 102–106. [Google Scholar] [CrossRef]
- Grosse, C. A Program for the Fitting of up to Three Havriliak-Negami Dispersions to Dielectric Data. J. Colloid Interface Sci. 2021, 600, 318–323. [Google Scholar] [CrossRef] [PubMed]
- Piuma, F.J.D.; Safar, F.G.E.; Passarella, D.N. Robust Fitting of the Cole-Cole Permittivity Equation. In Proceedings of the 2015 XVI Workshop on Information Processing and Control (RPIC), Cordoba, Argentina, 6–9 October 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Zhang, L.; Liu, P.; Dan, C.; Shi, X.; Dong, X. A Comparative Study on Algorithms for Optimizing Debye Model of Tissue Dielectric Properties. In Proceedings of the 2013 6th International Conference on Biomedical Engineering and Informatics, Hangzhou, China, 16–18 December 2013; pp. 317–321. [Google Scholar] [CrossRef]
- Lin, T. Non-Invasive Glucose Monitoring: A Review of Challenges and Recent Advances. Curr. Trends Biomed. Eng. Biosci. 2017, 6, 555696. [Google Scholar] [CrossRef]
- Costanzo, S.; Cioffi, V. Dielectric Models for the Accurate Design of Wearable Diabetes Sensors. In Proceedings of the 2019 23rd International Conference on Applied Electromagnetics and Communications (ICECOM), Dubrovnik, Croatia, 30 September–2 October 2019; pp. 1–3. [Google Scholar] [CrossRef]
- Venkataraman, J.; Freer, B. Feasibility of Non-Invasive Blood Glucose Monitoring: In-vitro Measurements and Phantom Models. In Proceedings of the 2011 IEEE International Symposium on Antennas and Propagation (APSURSI), Spokane, WA, USA, 3–8 July 2011; pp. 603–606. [Google Scholar] [CrossRef]
- Costanzo, S.; Cioffi, V.; Raffo, A. Complex Permittivity Effect on the Performances of Non-invasive Microwave Blood Glucose Sensing: Enhanced Model and Preliminary Results. In Trends and Advances in Information Systems and Technologies; Rocha, Á., Adeli, H., Reis, L.P., Costanzo, S., Eds.; Advances in Intelligent Systems and Computing; Springer International Publishing: Cham, Switzerland, 2018; pp. 1505–1511. [Google Scholar] [CrossRef]
- Levenberg, K. A Method for the Solution of Certain Non-Linear Problems in Least Squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef] [Green Version]
- Marquardt, D.W. An Algorithm for Least-Squares Estimation of Nonlinear Parameters. J. Soc. Ind. Appl. Math. 1963, 11, 431–441. [Google Scholar] [CrossRef]
- Golub, G.H.; Pereyra, V. The Differentiation of Pseudo-Inverses and Nonlinear Least Squares Problems Whose Variables Separate. SIAM J. Numer. Anal. 1973, 10, 413–432. [Google Scholar] [CrossRef]
- Karacolak, T.; Moreland, E.C.; Topsakal, E. Cole–Cole Model for Glucose-Dependent Dielectric Properties of Blood Plasma for Continuous Glucose Monitoring. Microw. Opt. Technol. Lett. 2013, 55, 1160–1164. [Google Scholar] [CrossRef]
- Dima, R.; Buonanno, G.; Solimene, R. Comparing Two Fitting Algorithms for Determining the Cole–Cole Parameters in Blood Glucose Problems. Eng. Proc. 2021, 11, 45. [Google Scholar] [CrossRef]
- Karacolak, T.; Moreland, E.C.; Topsakal, E. Cole-Cole Model for Glucose-Dependent Dielectric Properties of Blood Plasma for Continuous Glucose Monitoring. In Proceedings of the XXIXth URSI General Assembly, Chicago, IL, USA, 7–16 August 2008. [Google Scholar]
- Debye, P. Polar Molecules; The Chemical Catalog Company, Inc.: New York, NY, USA, 1929. [Google Scholar]
- Cole, K.S.; Cole, R.H. Dispersion and Absorption in Dielectrics I. Alternating Current Characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef] [Green Version]
- Gabriel, S.; Lau, R.W.; Gabriel, C. The Dielectric Properties of Biological Tissues: III. Parametric Models for the Dielectric Spectrum of Tissues. Phys. Med. Biol. 1996, 41, 2271–2293. [Google Scholar] [CrossRef] [Green Version]
- Salahuddin, S.; Porter, E.; Krewer, F.; O’ Halloran, M. Optimised Analytical Models of the Dielectric Properties of Biological Tissue. Med. Eng. Phys. 2017, 43, 103–111. [Google Scholar] [CrossRef] [PubMed]
- Gavin, H.P. The Levenberg-Marquardt Algorithm for Nonlinear Least Squares Curve-Fitting Problems; Duke University: Durham, NC, USA, 2020; p. 19. [Google Scholar]
- Matlab. Optimization Toolbox—User’s Guide; MathWorks: Natick, MA, USA, 2021. [Google Scholar]
- Golub, G.; Pereyra, V. Separable Nonlinear Least Squares: The Variable Projection Method and Its Applications. Inverse Probl. 2003, 19, R1–R26. [Google Scholar] [CrossRef]
- O’Leary, D.P.; Rust, B.W. Variable Projection for Nonlinear Least Squares Problems. Comput. Optim. Appl. 2013, 54, 579–593. [Google Scholar] [CrossRef]
- IDF Diabetes Atlas; Technical Report; International Diabetes Federation: Brussels, Belgium, 2019.
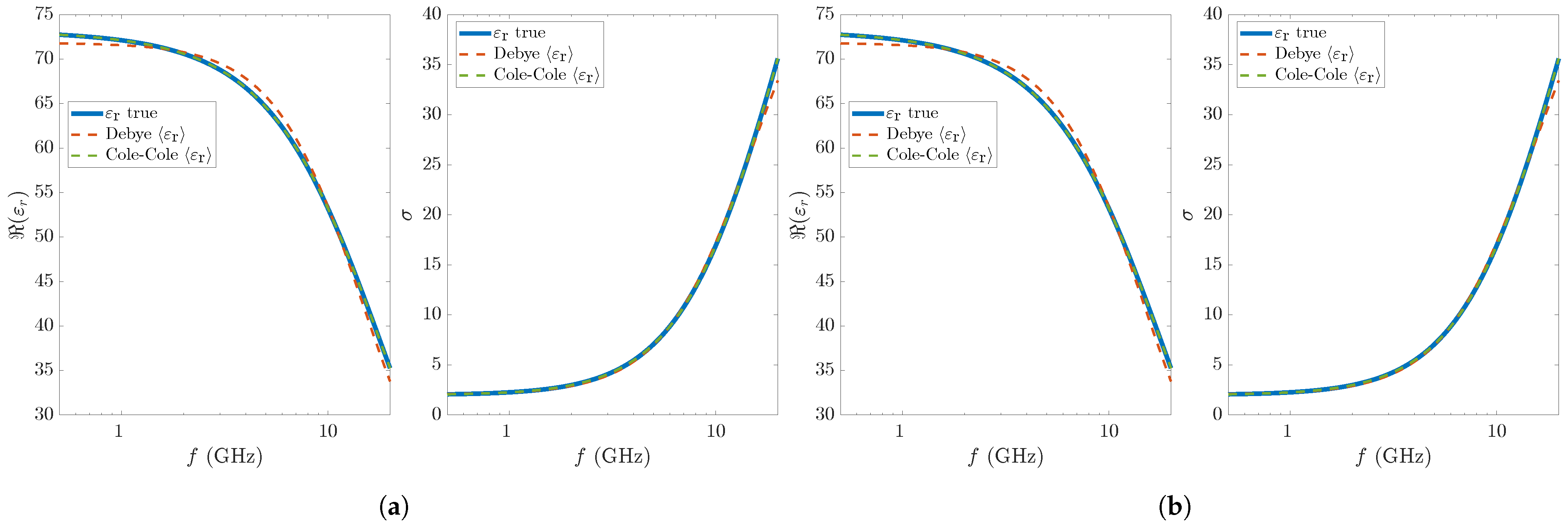
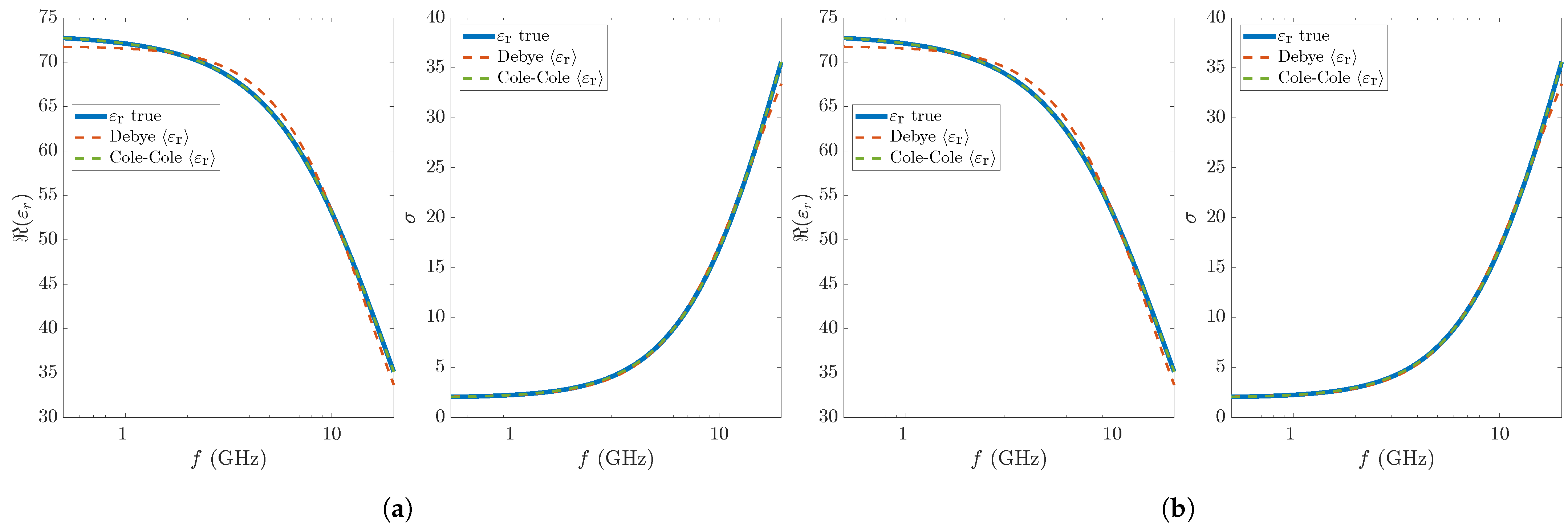
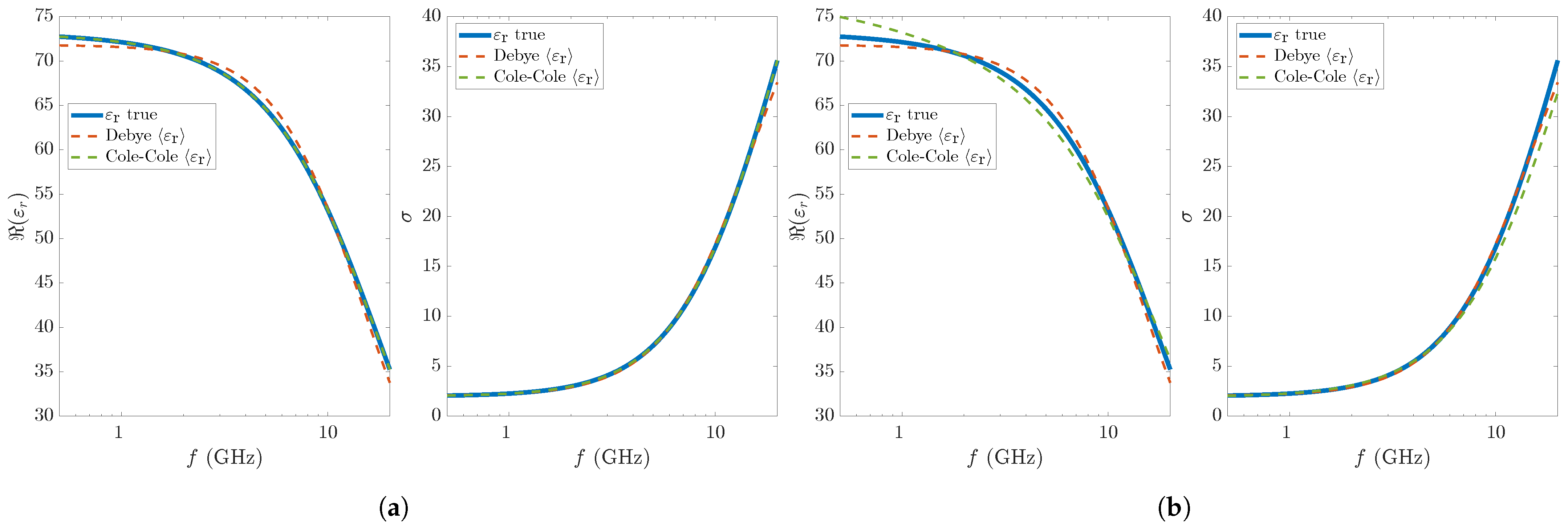
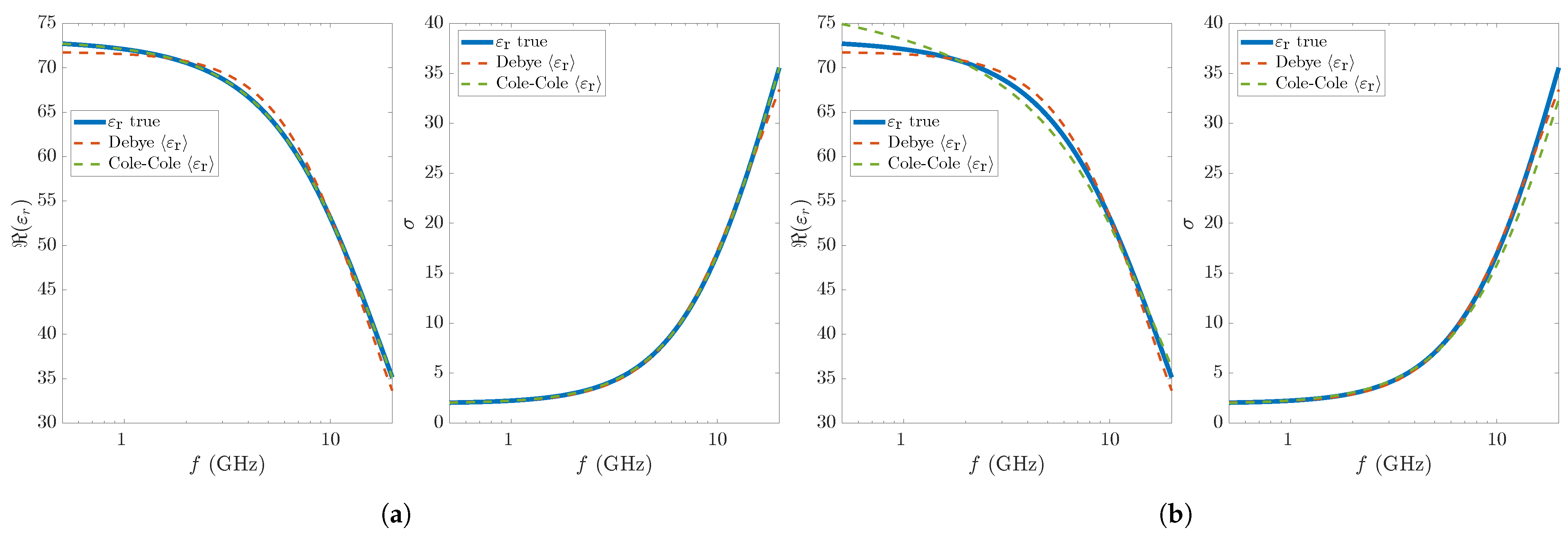
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.3 | 73.3 | 0.1 | 1.99 | - | ||||
| Cole–Cole | LMA | 2.3 | 73.3 | 0.1 | 1.99 | |||
| VPA | 2.3 | 73.3 | 0.1 | 1.99 | ||||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.03 | 1.64 | ||
| VPA | 12.9 | 71.8 | 0 | 2.03 | 1.64 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | ||||||||
| Debye | LMA | 0.000135 | 0 | |||||
| VPA | 0 |
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.31 | 73.3 | 0.1 | 1.97 | - | ||||
| Cole–Cole | LMA | 2.31 | 73.3 | 0.1 | 1.97 | |||
| VPA | 2.31 | 73.3 | 0.1 | 1.97 | ||||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | ||
| VPA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | ||||||||
| Debye | LMA | 0.000136 | 0 | |||||
| VPA | 0 |
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.3 | 73.3 | 0.1 | 1.99 | - | ||||
| Cole–Cole | LMA | 2.3 | 73.3 | 0.1 | 1.99 | |||
| VPA | 2.3 | 73.3 | 0.1 | 1.99 | ||||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.03 | 1.07 | ||
| VPA | 12.9 | 71.8 | 0 | 2.03 | 1.07 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | ||||||||
| Debye | LMA | 0.000139 | 0 | |||||
| VPA | 0 |
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.31 | 73.3 | 0.1 | 1.97 | - | ||||
| Cole–Cole | LMA | 2.31 | 73.3 | 0.1 | 1.97 | |||
| VPA | 2.31 | 73.3 | 0.1 | 1.97 | ||||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | ||
| VPA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | ||||||||
| Debye | LMA | 0.000139 | 0 | |||||
| VPA | 0 |
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.3 | 73.3 | 0.1 | 1.99 | - | ||||
| Cole–Cole | LMA | 2.3 | 73.3 | 0.1 | 1.99 | |||
| VPA | 4.13 | 79.8 | 0.182 | 1.9 | 14.1 | |||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.03 | 1.07 | ||
| VPA | 12.9 | 71.8 | 0 | 2.03 | 1.07 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | 4.64 | 14 | 0.169 | 0.18 | 28.7 | |||
| Debye | LMA | 0.000137 | 0 | |||||
| VPA | 0 |
| (s) | (S/m) | RMSRE | ||||||
|---|---|---|---|---|---|---|---|---|
| 2.31 | 73.3 | 0.1 | 1.97 | - | ||||
| Cole–Cole | LMA | 2.31 | 73.3 | 0.1 | 1.97 | |||
| VPA | 4.12 | 79.7 | 0.181 | 1.88 | 14 | |||
| Debye | LMA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | ||
| VPA | 12.9 | 71.8 | 0 | 2.01 | 1.07 | |||
| s | Cole–Cole | LMA | ||||||
| VPA | 4.59 | 13.9 | 0.168 | 0.18 | 28.6 | |||
| Debye | LMA | 0.000136 | 0 | |||||
| VPA | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dima, R.; Buonanno, G.; Costanzo, S.; Solimene, R. Robustness for the Starting Point of Two Iterative Methods for Fitting Debye or Cole–Cole Models to a Dielectric Permittivity Spectrum. Appl. Sci. 2022, 12, 5698. https://doi.org/10.3390/app12115698
Dima R, Buonanno G, Costanzo S, Solimene R. Robustness for the Starting Point of Two Iterative Methods for Fitting Debye or Cole–Cole Models to a Dielectric Permittivity Spectrum. Applied Sciences. 2022; 12(11):5698. https://doi.org/10.3390/app12115698
Chicago/Turabian StyleDima, Roberto, Giovanni Buonanno, Sandra Costanzo, and Raffaele Solimene. 2022. "Robustness for the Starting Point of Two Iterative Methods for Fitting Debye or Cole–Cole Models to a Dielectric Permittivity Spectrum" Applied Sciences 12, no. 11: 5698. https://doi.org/10.3390/app12115698
APA StyleDima, R., Buonanno, G., Costanzo, S., & Solimene, R. (2022). Robustness for the Starting Point of Two Iterative Methods for Fitting Debye or Cole–Cole Models to a Dielectric Permittivity Spectrum. Applied Sciences, 12(11), 5698. https://doi.org/10.3390/app12115698








