Abstract
Foamed concrete consists of cement matrix and air-foam and has more complicated structure characteristics than normal concrete. However, current research on the elastic modulus of foamed concrete is still limited to empirical equations. In this work, a new theoretical prediction model was proposed for calculating the elastic modulus of foamed concrete. The elastic modulus calculation model for the spherical shell element of foamed concrete is constructed based on the Walsh formula and the assumption of spherical pores. Moreover, the theoretical prediction model is established by introducing the two-layer embedded model for the elastic modulus of foamed concrete. Then, the compressive test is employed to verify the accuracy of the model. The results show that the elastic modulus of foamed concrete decreases with the increase in porosity and matrix Poisson’s ratio, and increases with the increase in the matrix elastic modulus. The research results can improve the mechanical theories of foamed concrete materials and have good engineering application values.
1. Introduction
Foamed concrete is mainly composed of cement matrix and foam in proportion [,,]. The production process of foamed concrete is shown in Figure 1. A porous structure with light bulk density and high strength can be formed after curing through the cast-in-place construction method. In addition, the density and strength properties of foamed concrete can be adjusted according to needs, which is very effective in controlling foundation settlement and reducing structural cracks [,,]. Therefore, foamed concrete has good applicability in practical projects. Foamed concrete was first used in airport roadbed engineering in Japan and was later introduced into China by scholar Chen []. After more than 40 years of engineering practice and unremitting exploration by relevant scholars, foamed concrete has become increasingly extensive, and the research results have been significantly improved.
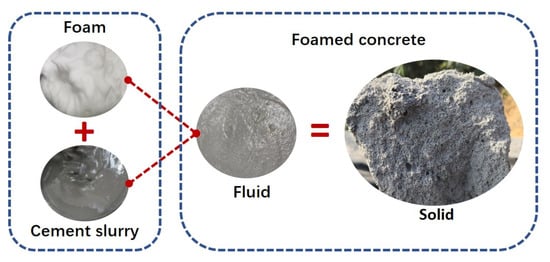
Figure 1.
Production process of foamed concrete.
Foamed concrete has a large number of internal cellular structures, which is different from concrete. These microscopic features [,,] and the properties of matrix materials [,] determine the mechanical properties of foamed concrete, and directly affect the elastic modulus or other parameters, thus affecting its deformation properties. Therefore, scholars have made useful attempts on the mechanical characteristics of foamed concrete. Ramamurthy [,] found that the volume, size, and spacing of pores have a great influence on the compressive strength and density of foamed concrete, and established a compressive strength model of foamed concrete. Their research shows that foamed concrete with uniform distribution of air-void sizes, circular air-voids, and optimal spacing between voids has better mechanical properties. Falliano et al. [,,] studied the dependence of the compressive strength of foamed concrete on dry density, water content, curing conditions, cement type, and foaming agent, and found that compressive strength showed a linear trend with the increase in density. Panesar et al. [] thoroughly studied the influence of the microstructure of foamed concrete on the mechanical parameters, and the study shows that the microstructure is remarkably important to the strength and elastic modulus. Based on these typical research results, therefore, the elastic modulus is the key parameter in which to study the mechanical properties of foamed concrete, which can be used to design roadbed padding and predict the subgrade under load mechanical response of a foamed concrete roadbed in subgrade engineering [,].
Numerical simulation and mechanical tests are effective methods to explore the elastic modulus of foamed concrete. Youssef et al. [] conducted a 3D numerical simulation study on foamed concrete, which showed that the elastic modulus of foamed concrete mainly depends on its internal porosity. When the porosity is at a low level, 3D numerical simulations and mean field schemes provide consistent estimates of the overall stiffness of a biphasic composite material made of spherical pores embedded in a matrix. This study divides foamed concrete into matrix and embedded spherical pores, and preliminarily proves the rationality of this assumption. Therefore, the mechanical analysis of foamed concrete based on a two-layer embedded model is valuable and feasible. According to the study of Jones et al. [], the elastic modulus of foamed concrete is related to the matrix material, and the elastic modulus of foamed concrete using fine sand is higher than that using fly ash as fine aggregate. Their research only qualitatively analyzed the influence of matrix materials on the elastic modulus of foamed concrete, but it is necessary to quantitatively analyze how matrix parameters specifically affect the elastic modulus. Ge et al. [] performed mechanical performance tests on foamed concrete with wet densities of 700 and 800 kg/m3. The test results showed that the elastic modulus meets the requirements of cast-in-place foamed concrete subgrades. They concluded that both air-void content and pore size distribution lead to the significant difference in mechanical performance of mixes. The analysis of the factors affecting the elastic modulus of foamed concrete shows that porosity and matrix material properties have a significant impact on the elastic modulus of foamed concrete.
In addition to the test method, the empirical formula method is also an effective method to determine the elastic modulus. Tan et al. [] conducted a series of uniaxial compression tests of foamed concrete and summarized that the elastic modulus of foamed concrete with densities of 450, 600, and 800 kg/m3 increased with the increase in height-to-diameter (H:D) ratio. This study proposed a prediction model for elastic modulus at different densities and temperatures on the basis of experimental data. Mydin [] recommended a model for the relationship between elastic modulus and porosity of foamed concrete at ambient temperature. Their study confirmed that the mechanical performance model of ordinary strength concrete can predict the reduction in mechanical properties of foamed concrete, and verified the feasibility of using the Balshin equation to calculate the compressive elastic modulus at ambient temperature. Li and Purkiss [] present a critical review of the currently available models for the mechanical behavior of concrete at elevated temperatures, and proposed a stress–strain–temperature model of concrete based on the existing models and test data, which provides a great reference foundation for the prediction of the elastic modulus of foamed concrete.
However, both the test methods and empirical formula methods have limitations. The test methods are time-consuming and can only be used to determine the elastic modulus after foamed concrete is manufactured. The scope of application for the empirical formula is conditional. If the actual situation is different from that of the empirical formula method, the prediction results of the empirical formula may have deviation.
Most of the existing studies on the elastic modulus of foamed concrete remain at the macromechanical level, mainly including mechanical tests [,,,] and empirical formula analysis [,,]. However, theoretical research on foamed concrete from the perspective of mesomechanics is lacking. Thus, this paper considers the influence of the internal pore structure characteristics and matrix material properties of foamed concrete on the overall elastic modulus. Moreover, the Walsh formula and spherical pore assumption are introduced to construct an elastic modulus calculation model of the spherical shell element of foamed concrete. Combined with the two-layer embedded model, an inclusion theory model of the elastic modulus of foamed concrete is established. Then, the influence law of the elastic parameters of foamed concrete is thoroughly explored in accordance with the laboratory tests and the existing theoretical models to verify the theory. The research results can improve the mechanical theories of foamed concrete materials and have good engineering application values.
2. Micromechanical Analysis of Foamed Concrete Elements
2.1. Walsh Formula
Foamed concrete is a typical porous medium material, and the content of internal air bubbles is closely related to the macroscopic deformation parameters of foamed concrete. Therefore, this study introduces the Walsh formula [] for the theoretical analysis of the elastic modulus of foamed concrete elements from the perspective of mesomechanics. In accordance with the reciprocity theorem of work, Walsh deduces the relationship between the volume flexibility of the pore matrix in the material and the volume flexibility of the solid matrix, as given in Equation (1) []:
where V is the unit volume of the porous medium, δ is the volume compliance of the overall unit, Vc is the pore volume, δs is the volume compliance of the soil particle matrix, and ΔVc is the variation in pore volume under the action of volume stress Δσ.
Cao et al. [] transformed the derivation of bulk flexibility into the solution of elastic modulus by introducing the intermediate parameter of bulk modulus. They obtained the relationship between the elastic modulus of the element matrix and the equivalent elastic modulus of spherical shell elements on the basis of the Walsh formula, as shown by Equation (2) []:
where E2 is the equivalent elastic modulus of the spherical shell element and E1 is the matrix elastic modulus. The schematic of the spherical shell section of the medium element is shown in Figure 2.
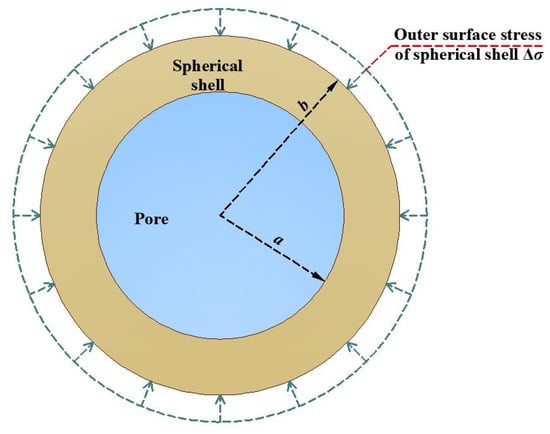
Figure 2.
Schematic of the spherical shell section of the medium element.
2.2. Spherical Pore Assumption
In accordance with the elastic mechanics calculation formula for uniformly distributed pressure inside and outside the spherical shell, the following assumptions are made. (1) The representative material unit is a spherical shell, and its inner and outer diameters are a and b, respectively. The inner sphere represents the pore part of the material, and the spherical shell represents the skeleton of the material. (2) The spherical shell, that is, the material skeleton, is a linear elastic body. (3) The pores in the material are independent of one another. The schematic of the spherical shell of a foamed concrete unit is shown in Figure 3. In consideration of the volume stress on the outer surface, the radial displacement of the spherical shell can be obtained by elastic mechanics, as shown by Equation (3) []:
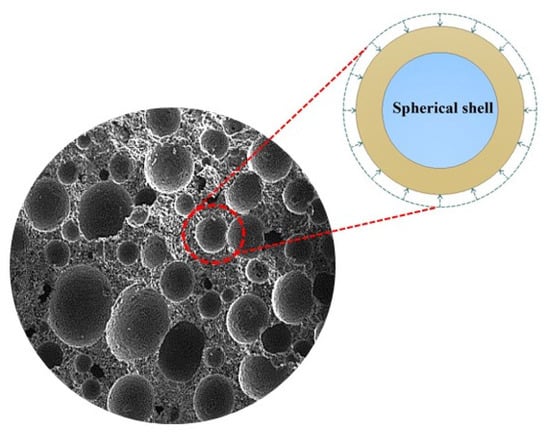
Figure 3.
Schematic of the spherical shell of a foamed concrete unit.
2.3. Spherical Shell Elastic Modulus of Foamed Concrete
Owing to the existence of a large number of closed air bubbles in foamed concrete, mechanical analysis of foamed concrete microelements can be conducted on the basis of the assumption of spherical pores. First, a foamed concrete unit containing spherical pores is selected, which can be regarded as a spherical shell with inner and outer diameters a and b, respectively. When the internal pore connectivity is poor, it is assumed that no pore water pressure exists. The inner diameter variation for the spherical shell has the following relationship with volume variation, as shown by Equations (4)–(6).
When Equation (6) is substituted into Equation (2), the calculation formula of the elastic modulus of foamed concrete element with respect to porosity can be obtained, as shown by Equations (7) and (8).
The initial porosity of foamed concrete can be measured experimentally. Nevertheless, in the actual compression process, the porosity changes with the pore deformation of foamed concrete. Therefore, this study analyzes it from the perspective of stress. The derivation process is shown in Equations (9) and (10).
Equation (10) is substituted into Equation (6) to obtain Equation (11).
Based on Equation (11), Equation (12) can be obtained:
Equation (12) is substituted into Equation (2), and the calculation formula of the spherical shell elastic modulus of foamed concrete is obtained in Equation (13).
3. Two-Layer Embedded Model Analysis of Foamed Concrete
3.1. Two-Layer Embedded Model
Foamed concrete is a composite material, which mainly includes fine aggregate and a large number of dense circular holes. Owing to the random distribution of internal holes, its stress condition is extremely complicated. For simplicity, a two-layer embedded model in the porous material theory is proposed. The basic principle of the model is that foamed concrete is regarded as a two-phase composite material, and the overall elastic modulus is obtained through the homogenization process. From a microscopic analysis, the holes in foamed concrete can be regarded as spherical shells, so its mesoscopic structure can be regarded as the form of a matrix unit wrapping a spherical shell unit. The schematic of the calculation model is shown in Figure 4. From the figure, foamed concrete conforms to the two-layer embedded wrapping model [,,] on the mesostructure.
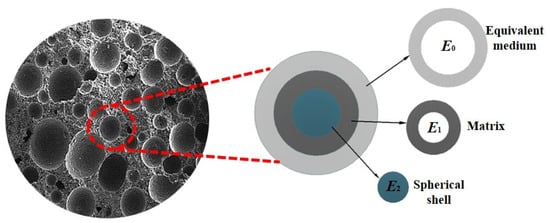
Figure 4.
Two-layer embedded model of foamed concrete.
3.2. Prediction Model for the Elastic Modulus of Foamed Concrete
Li et al. [] proposed an analytical solution for elastic theory for the two-layer embedded model. Accordingly, this study performs the theoretical analysis of the mesomechanics of foamed concrete by introducing the model. The prediction model for the elastic modulus of foamed concrete is shown in Figure 5, and the derivation process is shown in Equations (14)–(18) [].
where u is the displacement; u1a and u2a are the displacements of matrix, spherical shell at boundary r = a, respectively; u0b and u1b are the displacements of equivalent medium, matrix at boundary r = b, respectively; u0c is the displacement of equivalent medium at boundary r = c; and v0, v1, and v2 are the Poisson’s ratio of the equivalent medium, foamed concrete matrix, and spherical shell, respectively. On the basis of the theory of elasticity, Li [] further proposed formulas for the effective elastic modulus E0 of a composite material in Equations (19)–(21) [].
where f is the volume percentage of inclusions. The volume ratio of the spherical shell inclusion can be regarded as the bubble porosity for foamed concrete, as shown in Equation (22). E1 and v1 can be obtained through the performance test of foamed concrete matrix material. For the convenience of analysis, it is assumed that v2 = v1, but v0 is still unknown. Therefore, the 3D problem should be simplified to a 2D problem. The relationship between the size of the spherical shell and the volume ratio is shown in Equation (23).
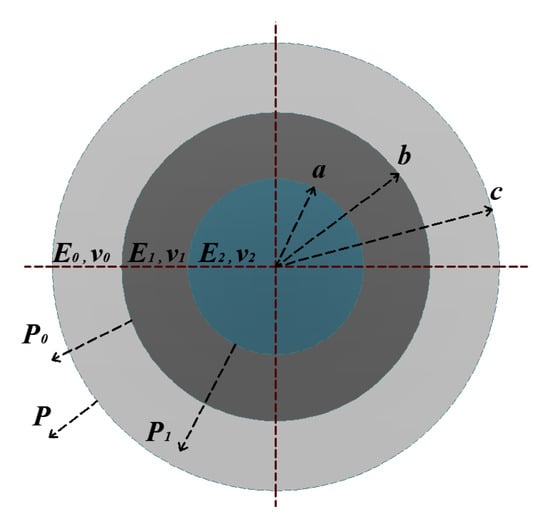
Figure 5.
Prediction model for the elastic modulus of foamed concrete.
With reference to the formula for calculating the shear modulus of single-inclusion composites proposed by Christensen et al. [], the effective elastic modulus E0 of foamed concrete material can be completely determined, which is also based on the two-layer embedded model. The calculation formula for the effective shear modulus μ0 can be derived, as shown in Equations (24)–(29) [].
where μ0, μ1, and μ2 represent the shear elastic moduli of the equivalent medium, matrix, and spherical shell, respectively. In accordance with the principle of elastic mechanics, the relationship between elastic modulus, shear modulus, and Poisson’s ratio is shown in Equation (30).
The elastic modulus prediction model of foamed concrete can be obtained by solving Equations (19), (24) and (30) simultaneously.
3.3. Calculation Process for the Elastic Modulus of Foamed Concrete
Foamed concrete is a two-layer embedded single-inclusion composite material. The main idea of its calculation follows. The inclusion spherical shell is placed into foamed concrete matrix material (assuming its elastic characteristic is F, which is determined by the material properties, such as elastic modulus E and Poisson’s ratio v, and assuming its elastic characteristic be S). Then, the formula above is used to complete the homogenization process to obtain the macroscopic properties of foamed concrete (setting its elastic characteristics as F-SM). The calculation diagram is shown in Figure 6.
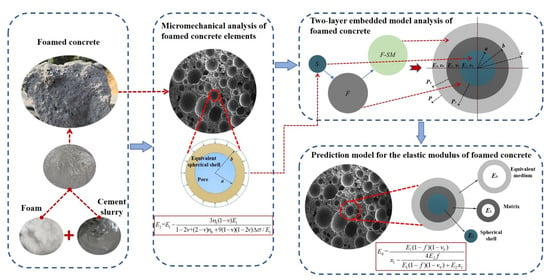
Figure 6.
Schematic of the calculation of a single inclusion in foamed concrete.
From the mesomechanical analysis process described above, the calculation process for the elastic modulus of foamed concrete under normal conditions can be determined when combined with the basic physical performance parameters of foamed concrete, as shown in Figure 7.
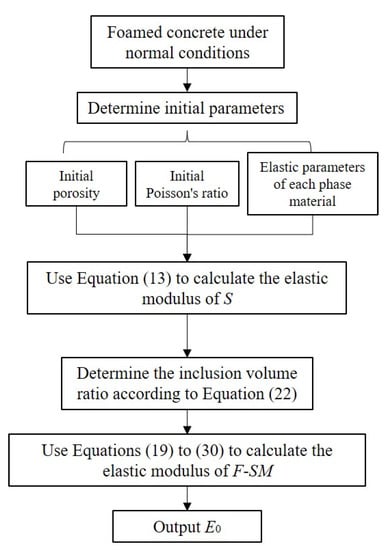
Figure 7.
Flowchart of the calculation for the elastic modulus of foamed concrete.
4. Model Validation
This section mainly verifies the inclusion theoretical model of foamed concrete constructed above on the basis of the comparison of laboratory tests and existing research literature.
4.1. Comparison with Test Results
In this test, an instron1346 universal testing machine produced by the Instron company of Britain is used to carry out the indoor compression test of foamed concrete test block. The raw materials of foamed concrete are 42.5-grade ordinary Portland cement, class F fly ash, and QW-100-type foaming agent. The size of the test block is set to 100 mm × 100 mm × 100 mm. The curing conditions are implemented in a sealed plastic bag for constant-temperature curing, and the curing temperature is 20 ± 2 °C. The test process is shown in Figure 8.

Figure 8.
Schematic of test.
In the test, a controlled strain rate of 50 mm/min is used to load the foamed concrete test block, and the stress–strain curve is immediately generated using the universal testing machine. Loading is conducted until the foamed concrete specimen fails, and the maximum failure load is recorded. The elastic modulus of the foamed concrete test block can be obtained by measuring the slope of the straight-line segment on the stress–strain curve.
In accordance with the compressive strength test results of foamed concrete cubes, the test data of the test blocks are listed in Table 1.

Table 1.
Test value of the compressive strength of foamed concrete test blocks.
The porosity of foamed concrete and the mechanical constants of the matrix material are mainly involved in the analysis and calculation of this model. The former is mainly obtained by measuring the bubble rate in indoor tests, and the relevant parameters of the latter are listed in Table 2.

Table 2.
Values of the relevant parameters of matrix materials.
From the experimental–theoretical comparison results given in Figure 9, the elastic modulus of foamed concrete calculated using the theoretical model reported in this paper is in good agreement with the laboratory test data. The overall error is within a reasonable range. In addition, the distribution law of the elastic modulus of foamed concrete with bulk density indicates that a certain linear quantitative relationship exists between its elastic modulus and its density, which can be described by an empirical formula.
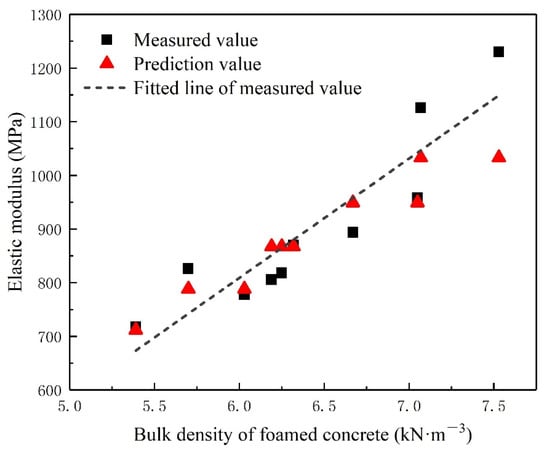
Figure 9.
Comparison of elastic modulus test and theory of foamed concrete.
4.2. Comparison with Existing Research
Tan et al. [] conducted a study on the stress–strain characteristics of foamed concrete with different densities; they provided a simple description formula of elastic modulus based on laboratory tests, as shown by Equation (31) []:
Therefore, on the basis of the compressive strength test of foamed concrete, the relevant parameters of the indoor test block are substituted into the theoretical model described in this paper for comparative analysis with Equation (31). The results are shown in Figure 10.
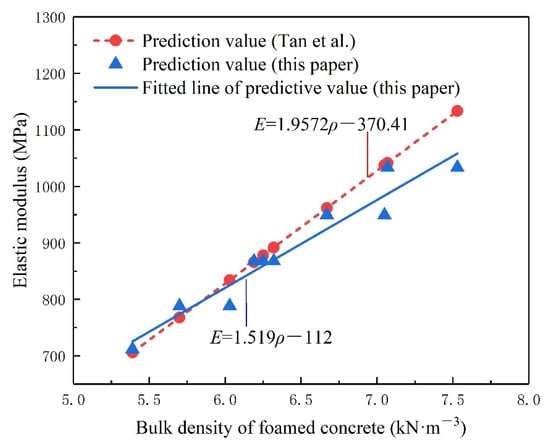
Figure 10.
Comparison between the elastic modulus theory of foamed concrete and existing research (Tan et al. []).
From the comparison of the calculation results shown in Figure 10, the model described in this paper is relatively close to the calculation results of the existing theoretical results, and the data have a high degree of agreement. Moreover, in accordance with the results of this model, the linear description of the elastic modulus on the density of foamed concrete can be obtained, as shown in Equation (32). Comparison of the linear distribution laws of the two indicates that the overall slope deviation is not large.
McCormick et al. [] conducted a series of mechanical tests on foamed porous concrete materials, and they obtained the relationship between the elastic modulus, wet bulk density, and compressive strength of foamed concrete based on a large number of test data, such as Equation (33); Jones et al. [] gave the relationship between the elastic modulus and compressive strength of foamed concrete based on experimental studies, as shown in Equation (34).
Referring to the test data of each group of test blocks in Table 1, the relevant parameters are substituted into the theoretical model of this paper for comparative analysis with Equation (33); the calculation results are shown in Figure 11. Equation (34) is compared with the model in this paper, and the calculation results are shown in Figure 12. In order to more intuitively reflect the rationality of the model described in this paper, the calculated values of McCormick’s, Jones’s, Tan’s, and the model in this paper are compared with the experimental values, and the results are shown in Figure 13.

Figure 11.
Comparison of elastic modulus theory of foamed concrete and existing research (McCormick []).
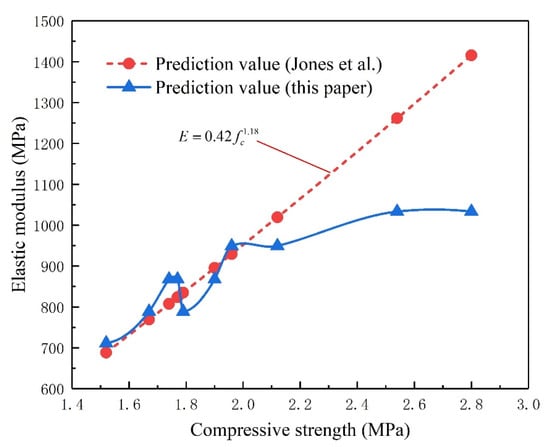
Figure 12.
Comparison of elastic modulus theory of foamed concrete with existing research (Jones et al. []).
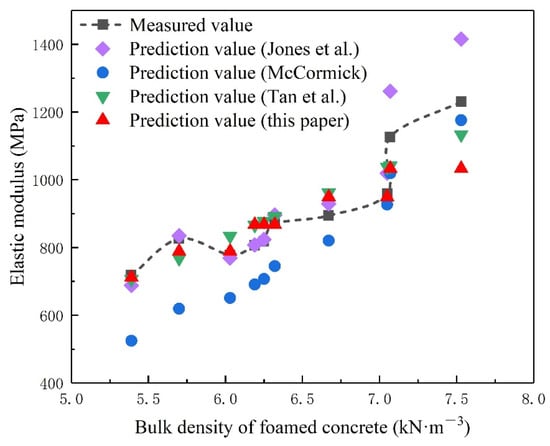
Figure 13.
Comprehensive comparison of calculation results of elastic modulus of foamed concrete (Jones et al. [], McCormick [] and Tan et al. []).
It can be seen from Figure 11 that the proposed model and McCormick’s model have a certain similarity in the calculation results. It can be seen from Figure 12 that when the compressive strength is in the range 1.5–2.0 MPa, the calculation results of the proposed model and Jones’s model are relatively close. When the compressive strength of foamed concrete is higher than 2.0 MPa, the difference between the two calculated values becomes increasingly larger.
According to the comparison of elastic modulus values shown in Figure 13, it can be seen that compared with the research results of McCormick, Jones, and Tan, the calculation results of the elastic modulus prediction model of foam concrete proposed in this paper are closer to the experimental data, indicating that the accuracy of this model is better than other models. Therefore, the theoretical model of the elastic modulus of foamed concrete based on the Walsh formula has good rationality.
5. Parameter Sensitivity Analysis
In accordance with the relevant parameters of foamed concrete used in the laboratory test, parameter sensitivity analysis is further performed for the theoretical model of a foamed concrete inclusion. The basic parameters of the model are shown in Table 2. From the previous analysis, the elastic modulus of foamed concrete is closely related to the density, and the material density is related to the porosity and the elastic parameters of the matrix material. Therefore, the influence of these main parameters on the elastic modulus of foamed concrete is explored in depth.
5.1. Porosity of Foamed Concrete
The elastic modulus results of foamed concrete with porosity 0.59, 0.62, 0.65, 0.68, and 0.71 are calculated. Figure 14 presents the relationship between the elastic modulus and porosity of foamed concrete. As shown in Figure 14, compared with foamed concrete with porosity 0.59, the elastic modulus of foamed concrete with porosity 0.62, 0.65, 0.68, and 0.71 decreased by 8.1%, 16%, 23.7%, and 31.1%, respectively. This shows that the calculation results of this model are negatively correlated with porosity; the higher the porosity of foamed concrete, the lower the elastic modulus. The main reason is that porosity is directly related to the overall stiffness of the test block, and an excessively high porosity greatly reduces the overall stiffness of foamed concrete.
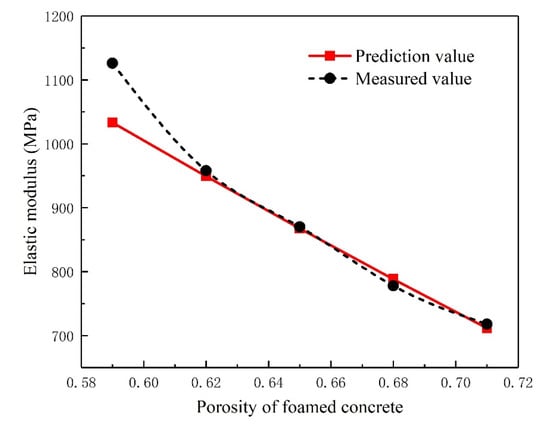
Figure 14.
Variation in elastic modulus of foamed concrete with porosity.
This law can also be obtained from the laboratory test data, but the difference is that the test data do not show a strict linear correlation with porosity. Accordingly, in practice, the relationship between the elastic modulus and the porosity of foamed concrete is nonlinear, but on the whole, the model is in good agreement with the experimental data in the porosity range 0.62–0.71. This model can be used to explore the effect of porosity on the elastic modulus of foamed concrete.
5.2. Poisson’s Ratio of Foamed Concrete Matrix
The elastic modulus of foamed concrete with Poisson’s ratio of matrix material in the range 0.1~0.28 were calculated, and the corresponding curves drawn, as shown in Figure 15. It can be seen from Figure 15 that when the matrix Poisson’s ratio increases from 0.1 to 0.28, the elastic modulus of foamed concrete with porosity 0.59, 0.62, 0.65, 0.68, and 0.71 decreased by 18.7%, 19.7%, 20.6%, 21.5%, and 22.4%, respectively. This shows that the elastic modulus decreases continuously with the increase in Poisson’s ratio. This is mainly because the material is not prone to lateral deformation when Poisson’s ratio is small; on the contrary, when the Poisson’s ratio is large, the material is prone to lateral deformation, and the stiffness of the material is large. Therefore, the elastic modulus is negatively correlated with the matrix Poisson’s ratio.
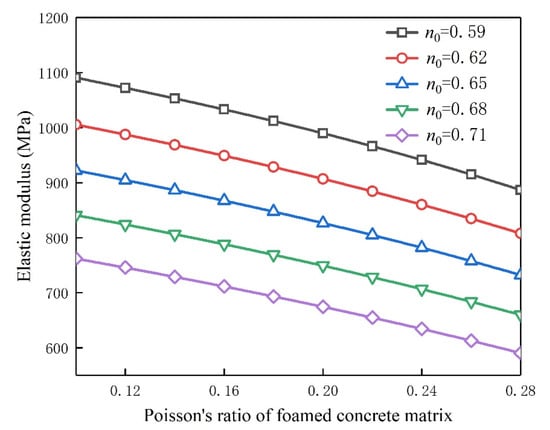
Figure 15.
Variation in elastic modulus of foamed concrete with Poisson’s ratio of matrix.
5.3. Elastic Modulus of Foamed Concrete Matrix
The elastic modulus of foamed concrete with the elastic modulus of matrix material in the range 1450~3450 MPa was calculated; Figure 16 shows the relationship between the elastic modulus of foamed concrete and the elastic modulus of the matrix. When the elastic modulus of the matrix increases from 1450 to 3450 MPa, the elastic modulus of foamed concrete with porosity 0.59, 0.62, 0.65, 0.68, and 0.71 increases by 118%, 116%, 113%, 111%, and 108%, respectively. This shows that there is a positive correlation between the elastic modulus of foamed concrete and that of the matrix material.
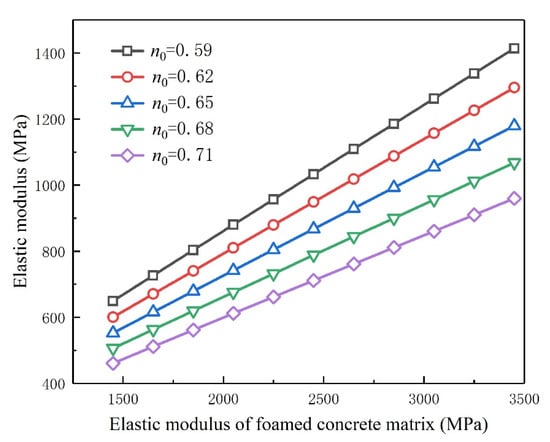
Figure 16.
Variation law of the elastic modulus of foamed concrete with the elastic modulus of matrix.
In addition, comparison of the curve slopes under different porosity calculation conditions shows that the smaller the porosity, the higher the curve slope. That is, the elastic modulus of foamed concrete has a high correlation with the elastic properties of the matrix in the case of low porosity. The performance of foamed concrete in the case of high porosity is not only related to the matrix but is also restricted by the pore state.
Quantitative analysis based on theory shows that for foamed concrete, a two-phase medium material composed of matrix material and bubble pores, its elastic properties are controlled by two main factors: material porosity and matrix material properties. Adjusting the level of these two factors can effectively change the elastic properties of foamed concrete.
6. Conclusions
In this paper, an elastic modulus prediction model of foamed concrete is established based on the derivation of the Walsh formula and inclusion theory. The research can be mainly summarized as the following points:
- (1)
- According to the internal structure characteristics of foamed concrete, the calculation formula is established for elastic modulus of spherical shell by introducing the Walsh formula and spherical pore hypothesis. Moreover, the elastic modulus prediction model of foamed concrete is constructed based on the two-layer embedded model in inclusion theory.
- (2)
- The uniaxial compression test of foamed concrete is carried out, and the proposed model is compared with the test data and the existing theoretical models. The verification results show that the elastic modulus values calculated using the proposed model are consistent with the experimental data. The values are also in good agreement with the distribution law of the calculation results calculated by using other theoretical models.
- (3)
- The effects of porosity, Poisson’s ratio of matrix, and elastic modulus of matrix on the elastic modulus of foamed concrete are analyzed by the proposed model. The results show that the elastic modulus of foamed concrete decreases with the increase in porosity and matrix Poisson’s ratio, and increases with the increase in matrix elastic modulus.
Author Contributions
Conceptualization, F.L. and J.H.; methodology, F.L.; validation, F.L. and J.H.; formal analysis, Z.Z. and M.L.; investigation, J.Z.; data curation, F.L. and J.Z.; writing—original draft preparation, F.L.; writing—review and editing, J.H.; supervision, Z.Z. and M.L.; project administration, Z.Z.; funding acquisition, Z.Z. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the National Natural Science Foundation of China, grant number 50908234, and Hunan Provincial Natural Science Foundation of China, grant number 2020JJ4743.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Data are contained within the article.
Conflicts of Interest
The authors declare no conflict of interest.
Abbreviations
| Main symbols and notations used in the paper | |
| Symbol | Description |
| a | inner diameter of spherical shell |
| b | outer diameter of spherical shell |
| c | outer diameter of equivalent medium |
| E | elastic modulus |
| E0 | elastic modulus of equivalent medium |
| E1 | elastic modulus of the matrix |
| E2 | elastic modulus of the spherical shell element |
| f | volume percentage of inclusions |
| fc | compressive strength of foamed concrete |
| n | porosity |
| n0 | initial porosity of foamed concrete |
| P | radial effective stress at the outer diameter of the equivalent medium |
| P0 | radial effective stress at the outer diameter of spherical shell |
| P1 | radial effective stress at the inner radius of spherical shell |
| ua | radial displacement of the spherical shell |
| u0b | displacement of equivalent medium at boundary r = b |
| u0c | displacement of equivalent medium at boundary r = c |
| u1a | displacement of matrix at boundary r = a |
| u1b | displacement of matrix at boundary r = b |
| u2a | displacement of spherical shell at boundary r = a |
| V | volume of the porous medium |
| Vc | pore volume |
| W | wet bulk density of foamed concrete |
| δ | volume compliance of the overall unit |
| δs | volume compliance of the matrix |
| ΔVc | change in pore volume |
| Δa | change of inner diameter of spherical shell |
| Δσ | the action of volume stress |
| ρ | density of foamed concrete |
| ρs | density of the matrix |
| υ | Poisson’s ratio |
| υ0 | Poisson’s ratio of foamed concrete |
| υ1 | Poisson’s ratio of the matrix |
| υ2 | Poisson’s ratio of the spherical shell |
References
- Othuman, M.A.; Wang, Y.C. Elevated-temperature thermal properties of lightweight foamed concrete. Constr. Build. Mater. 2011, 25, 705–716. [Google Scholar] [CrossRef]
- Kearsley, E.P.; Wainwright, P.J. The effect of porosity on the strength of foamed concrete. Cem. Concr. Res. 2002, 32, 233–239. [Google Scholar] [CrossRef]
- Kearsley, E.P.; Wainwright, P.J. The effect of high fly ash content on the compressive strength of foamed concrete. Cem. Concr. Res. 2001, 31, 105–112. [Google Scholar] [CrossRef]
- Zhou, Z.; Zhang, J.J.; Gong, C.J. Automatic detection method of tunnel lining multi-defects via an enhanced You Only Look Once network. Comput.-Aided Civil Infrastruct. Eng. 2022, 37, 762–780. [Google Scholar] [CrossRef]
- Huang, J.J.; Su, Q.; Zhao, W.H.; Li, T.; Zhang, X.X. Experimental study on use of lightweight foam concrete as subgrade bed filler of ballastless track. Constr. Build. Mater. 2017, 149, 911–920. [Google Scholar] [CrossRef]
- Kim, T.H.; Kim, T.H.; Kang, G.C. Performance evaluation of road embankment constructed using lightweight soils on an unimproved soft soil layer. Eng. Geol. 2013, 160, 34–43. [Google Scholar] [CrossRef]
- Chen, Z.P.; Wang, J.B.; Liu, J.F.; Zhao, W.H. Experimental study on dynamic engineering characteristics of foam lightweight soil. Highway 2019, 64, 77–80. (In Chinese) [Google Scholar]
- Jiang, C.; Wu, Y.F.; Jiang, J.F. Effect of aggregate size onstress-strain behavior of concrete confined by fiber composites. Compos. Struct. 2017, 168, 851–862. [Google Scholar] [CrossRef]
- Hilal, A.A.; Thom, N.H.; Dawson, A.R. The Use of Additives to Enhance Properties of Pre-Formed Foamed Concrete. Int. J. Eng. Technol. 2015, 7, 286–293. [Google Scholar] [CrossRef] [Green Version]
- Hilal, A.A.; Thom, N.H.; Dawson, A.R. On void structure and strength of foamed concrete made without/with additives. Constr. Build. Mater. 2015, 85, 157–164. [Google Scholar] [CrossRef]
- Amran, Y.H.M.; Farzadnia, N.; Ali, A.A.A. Properties and applications of foamed concrete; a review. Constr. Build. Mater. 2015, 101, 990–1005. [Google Scholar] [CrossRef]
- Liu, C.; Luo, J.; Li, Q.; Gao, S.; Su, D.; Zhang, J.; Chen, S. Calcination of green highbelite sulphoaluminate cement (GHSC) and performance optimizations of GHSC-based foamed concrete. Mater. Des. 2019, 182, 107986. [Google Scholar] [CrossRef]
- Ramamurthy, K.; Nambiar, E.K.K.; Ranjani, G.I.S. A classification of studies on properties of foam concrete. Cem. Concr. Compos. 2009, 31, 388–396. [Google Scholar] [CrossRef]
- Ranjani, G.I.S.; Ramamurthy, K. Behaviour of foam concrete under sulphate environments. Cem. Concr. Compos. 2012, 34, 825–834. [Google Scholar] [CrossRef]
- Falliano, D.; De Domenico, D.; Ricciardi, G.; Gugliandolo, E. Experimental investigation on the compressive strength of foamed concrete: Effect of curing conditions, cement type, foaming agent and dry density. Constr. Build. Mater. 2018, 165, 735–749. [Google Scholar] [CrossRef]
- Falliano, D.; De Domenico, D.; Ricciardi, G.; Gugliandolo, E. 3D-printable lightweight foamed concrete and comparison with classical foamed concrete in terms of fresh state properties and mechanical strength. Constr. Build. Mater. 2020, 254, 119271. [Google Scholar] [CrossRef]
- Falliano, D.; De Domenico, D.; Ricciardi, G.; Gugliandolo, E. Compressive and flexural strength of fiber-reinforced foamed concrete: Effect of fiber content, curing conditions and dry density. Constr. Build. Mater. 2019, 198, 479–493. [Google Scholar] [CrossRef]
- Panesar, D.K. Cellular concrete properties and the effect of synthetic and protein foaming agents. Constr. Build. Mater. 2013, 44, 575–584. [Google Scholar] [CrossRef]
- She, W.; Du, Y.; Zhao, G.; Feng, P.; Zhang, Y.; Cao, X. Influence of coarse fly ash on the performance of foam concrete and its application in high-speed railway roadbeds. Constr. Build. Mater. 2018, 170, 153–166. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, D.; Liang, Y.; Qu, K.; Lu, K.; Chen, S.; Kong, M. Study on engineering properties of foam concrete containing waste seashell. Constr. Build. Mater. 2020, 260, 119896. [Google Scholar] [CrossRef]
- Ben Youssef, M.; Lavergne, F.; Sab, K.; Miled, K.; Neji, J. Upscaling the elastic stiffness of foam concrete as a three-phase composite material. Cem. Concr. Res. 2018, 110, 13–23. [Google Scholar] [CrossRef] [Green Version]
- Jones, M.R.; McCarthy, A. Preliminary views on the potential of foamed concrete as a structural material. Mag. Constr. Res. 2005, 57, 21–32. [Google Scholar] [CrossRef]
- Ge, Z.; Guan, H.Q.; Sun, R.J.; Zhang, H.Z.; Wang, W.L.; Qi, H. Use of green calcium sulphoaluminate cement to prepare foamed concrete for road embankment: A feasibility study. Constr. Build. Mater. 2020, 237, 117791. [Google Scholar] [CrossRef]
- Tan, X.J.; Chen, W.Z.; Yang, D.S.; Liu, H.Y.; Chan, A.H.C. Experimental and theoretical studies on effect of height-to-diameter ratios on failure forms and mechanical characteristics of foamed concrete. J. Mater. Civ. Eng. 2019, 31, 04018341. [Google Scholar] [CrossRef]
- Mydin, M.A.O.; Wang, Y.C. Mechanical properties of foamed concrete exposed to high temperatures. Constr. Build. Mater. 2012, 26, 638–654. [Google Scholar] [CrossRef]
- Li, L.; Purkiss, J. Stress-strain constitutive equations of concrete material at elevated temperatures. Fire Saf. J. 2005, 40, 669–686. [Google Scholar] [CrossRef]
- Shi, X.N.; Huang, J.J.; Su, Q. Experimental and numerical analyses of lightweight foamed concrete as filler for widening embankment. Constr. Build. Mater. 2020, 250, 118897. [Google Scholar] [CrossRef]
- Horpibulsuk, S.; Suddeepong, A.; Chinkulkijniwat, A.; Liu, M.D. Strength and compressibility of lightweight cemented clays. Appl. Clay. Sci. 2012, 69, 11–21. [Google Scholar] [CrossRef] [Green Version]
- Cong, M.; Bing, C. Properties of a foamed concrete with soil as filler. Constr. Build. Mater. 2015, 76, 61–69. [Google Scholar] [CrossRef]
- Nambiar, E.K.K.; Ramamurthy, K. Air-void characterisation of foam concrete. Cem. Concr. Res. 2007, 37, 221–230. [Google Scholar] [CrossRef]
- McCormick, F.C. Rational proportioning of preformed foam cellular concrete. ACI J. Proc. 1967, 64, 104–110. [Google Scholar]
- Saint-Jalmes, A.; Peugeot, M.L.; Ferraz, H.; Langevin, D. Differences between protein and surfactant foams: Microscopic properties, stability and coarsening. Colloids Surf. A Physicochem. Eng. Asp. 2005, 263, 219–225. [Google Scholar] [CrossRef]
- Raj, A.; Sathyan, D.; Mini, K.M. Physical and functional characteristics of foam concrete: A review. Constr. Build. Mater. 2019, 221, 787–799. [Google Scholar] [CrossRef]
- Walsh, J.B. The effect of cracks on the compressibility of rock. J. Geophys. Res. 1965, 70, 381–389. [Google Scholar] [CrossRef]
- Cao, W.G.; Li, P.; Xu, X.; He, M. An analytical method of ground foundation settlement influenced by modulus change and thre e-dimensional stress. J. Hunan Univ. (Nat. Sci.) 2018, 45, 142–148. (In Chinese) [Google Scholar]
- Yang, H.; Zhou, Z.; Wang, X.C.; Zhang, Q.F. Elastic modulus calculation model of a soil-rock mixture at normal or freezing temperature based on micromechanics approach. Adv. Mater. Sci. Eng. 2015, 2015, 576080. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Z.; Yang, H.; Xing, K.; Gao, W.Y. Prediction models of the shear modulus of normal or frozen soil-rock mixtures. Geomech. Eng. 2018, 15, 783–791. [Google Scholar] [CrossRef]
- Zhou, Z.; Liu, Z.Z.; Yang, H.; Gao, W.Y.; Zhang, C.C. Freeze-thaw damage mechanism of elastic modulus of soil-rock mixtures at different confining pressures. J. Cent. South Univ. 2020, 27, 554–565. [Google Scholar] [CrossRef]
- Li, G.; Li, Y.; Metcalf, J.B.; Pang, S.S. Elastic modulus prediction of asphalt concrete. J. Mater. Civ. Eng. 1999, 11, 236–241. [Google Scholar] [CrossRef]
- Christensen, R.M.; Lo, K.H. Solutions for effective shear properties in three phase sphere and cylinder models. J. Mech. Phys. Solids 1979, 27, 315–330. [Google Scholar] [CrossRef]
- Tan, X.J.; Chen, W.Z.; Liu, H.Y.; Chan, A.H.C. Stress-Strain Characteristics of Foamed Concrete Subjected to Large Deformation under Uniaxial and Triaxial Compressive Loading. J. Mater. Civ. Eng. 2018, 30, 04018095. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).