Inversion of Sound Speed and Thickness of High-Speed Sediment Using Interference Structure in Shadow Zone
Abstract
:1. Introduction
2. Theory and Analysis
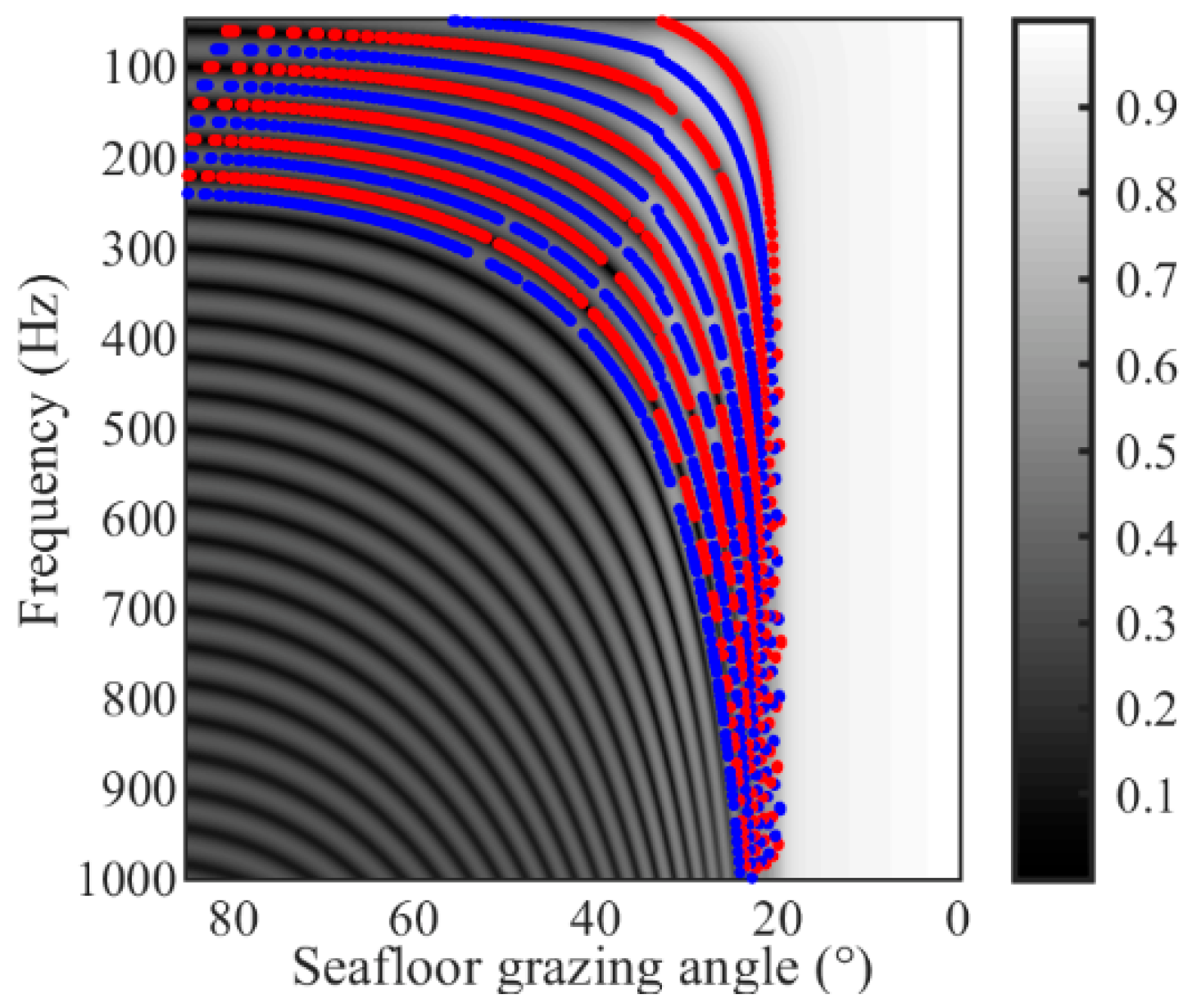
2.1. Interference Structure of Ocean Bottom Reflection Coefficient in Angle-Frequency Domain
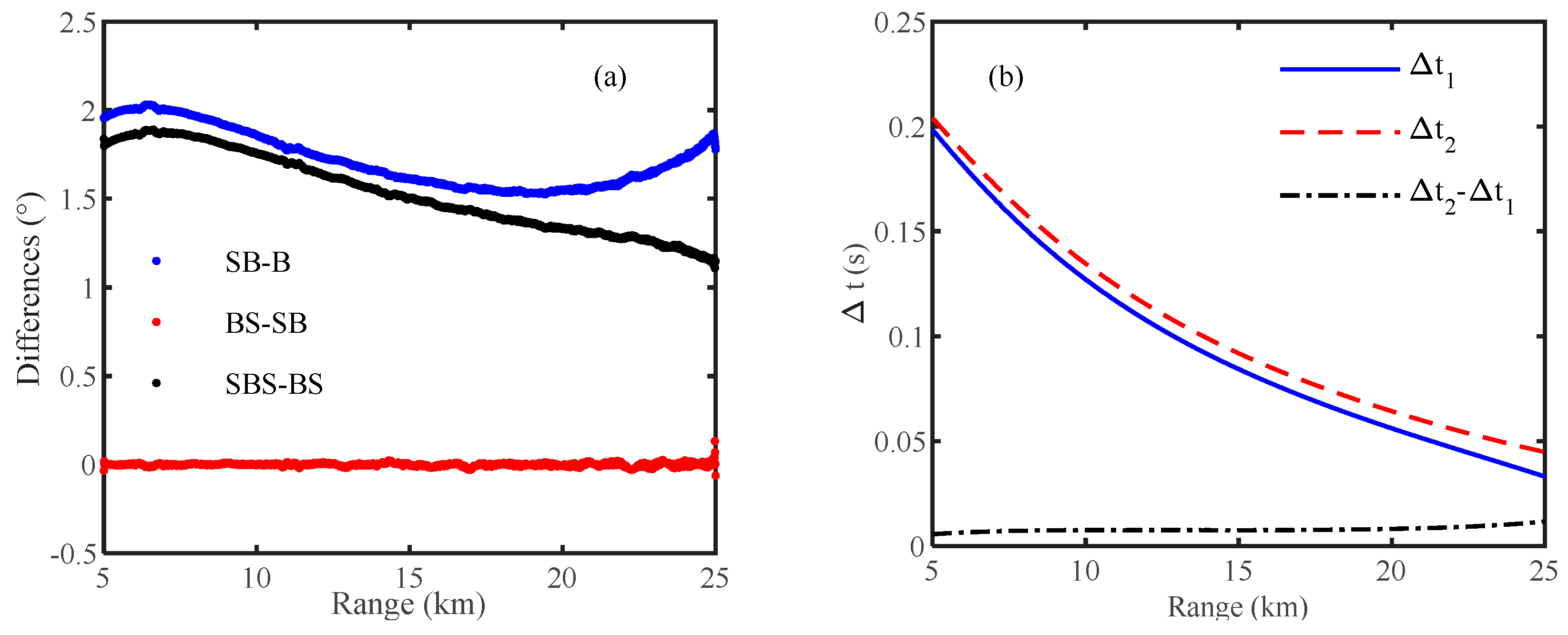
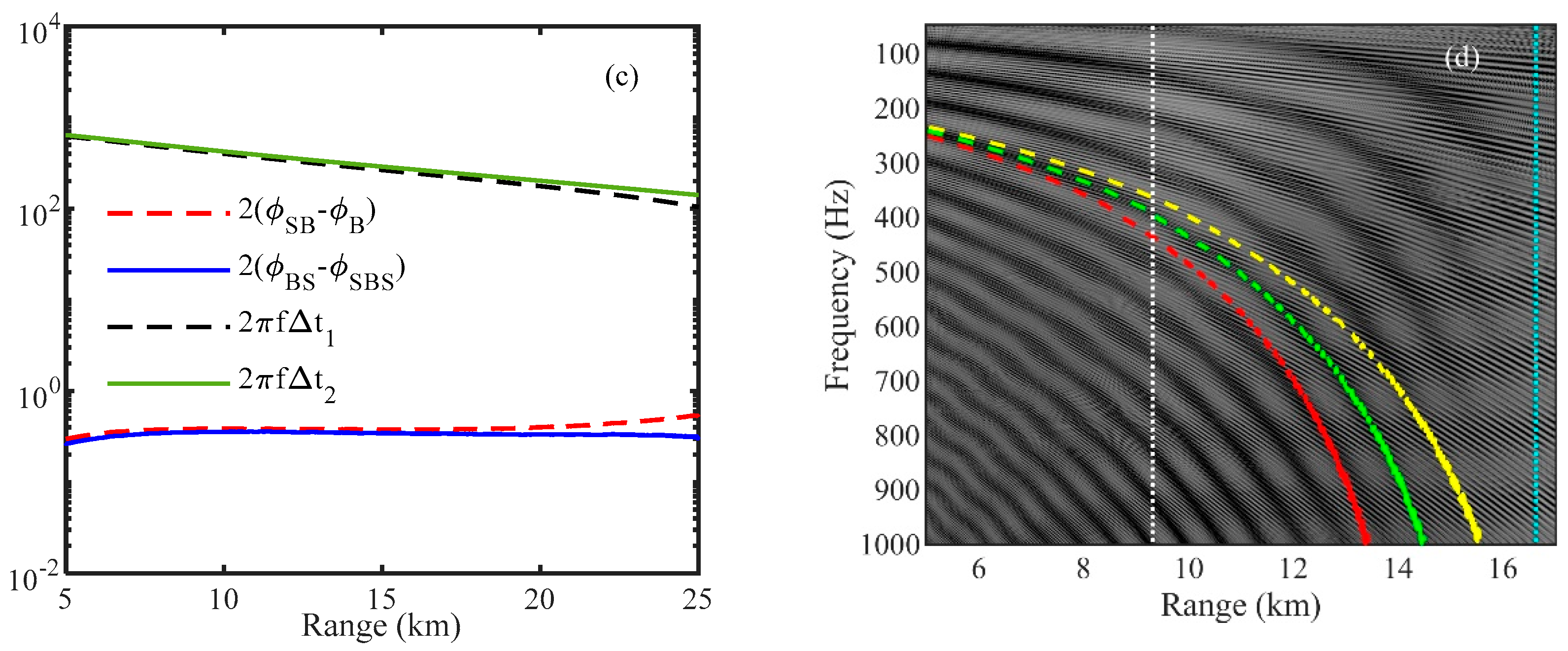
2.2. Range-Frequency Interference Structure of Shadow Zone
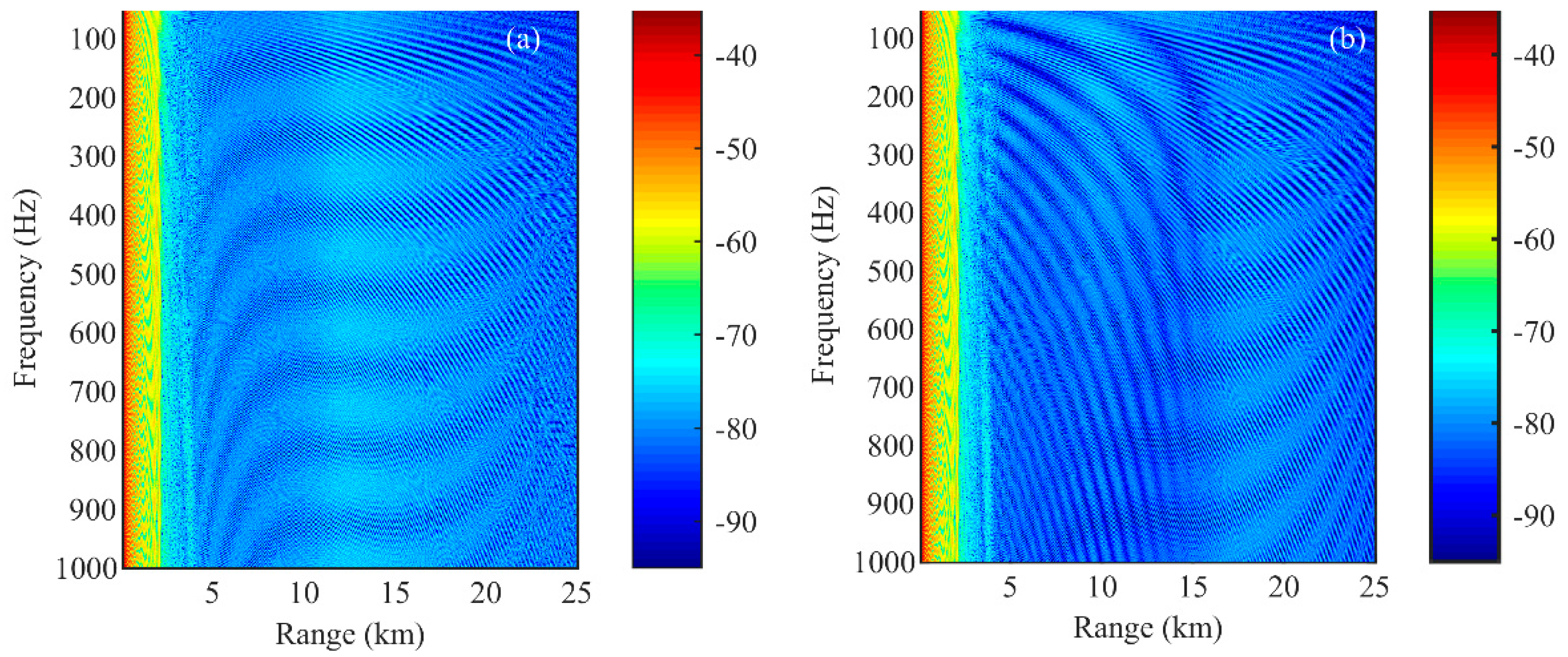
3. Applications
3.1. Sensitivity Analysis
3.2. Comparison of Inversion Results of Different Cost Functions
3.3. Interference Fringe Extraction
3.4. Geoacoustic Parameter Inversion
3.4.1. Inversion of Sound Speed and Thickness of Sediment by Grid Search Method
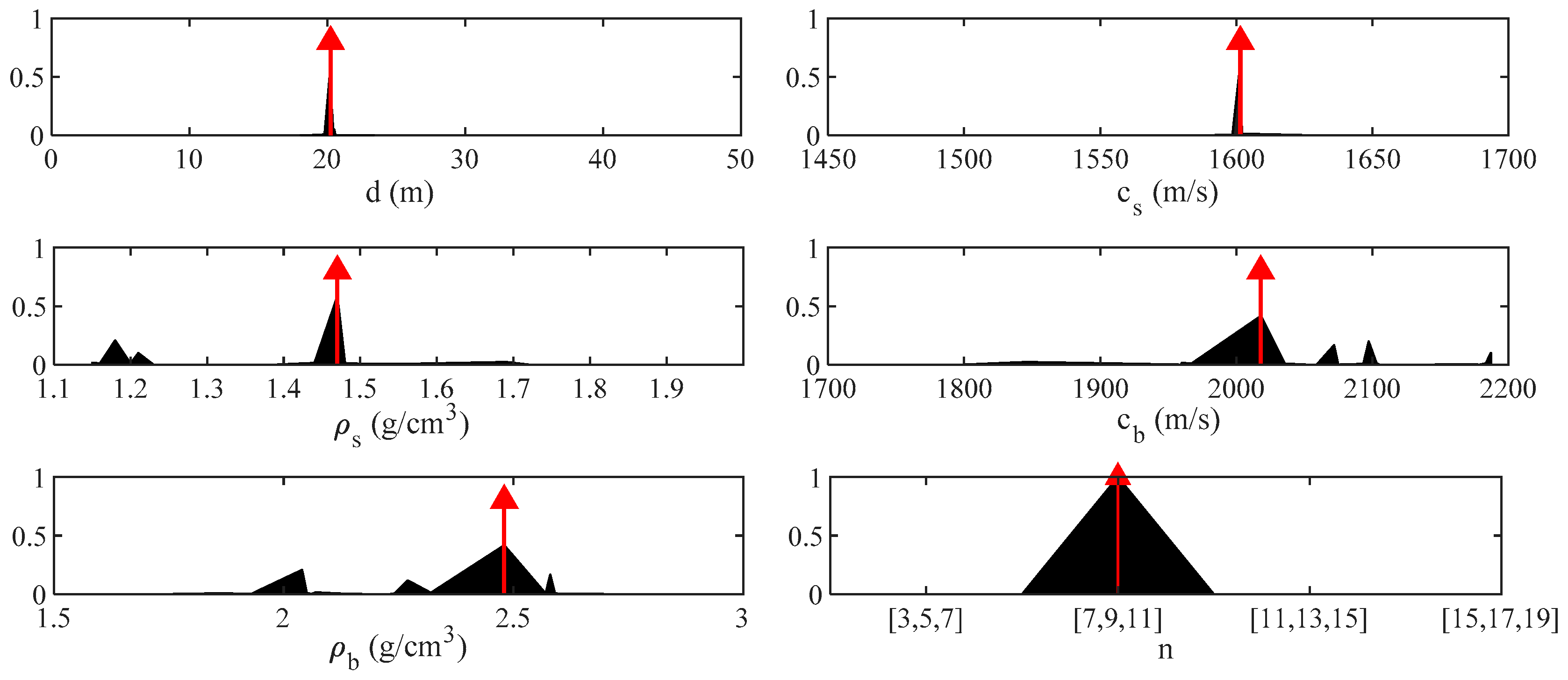
3.4.2. Inversion of Geoacoustic Parameters by Genetic Algorithm
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chapman, D.M. What are we inverting for? In Inverse Problems in Underwater Acoustics; Springer: New York, NY, USA, 2001; pp. 1–14. [Google Scholar] [CrossRef]
- Neumann, P.; Muncill, G. Using the Adaptive Simulated Annealing Algorithm to Estimate Ocean-Bottom Geoacoustic Properties from Measured and Synthetic Transmission Loss Data. IEEE J. Ocean. Eng. 2004, 29, 13–28. [Google Scholar] [CrossRef]
- Wan, L.; Zhou, J.-X.; Rogers, P.; Simmen, J.; Livingston, E.S.; Li, F.-H. Seabed Geoacoustic Inversion from Long-Range Broadband Sound Propagation in the Yellow Sea. AIP Conf. Proc. 2010, 1272, 270–277. [Google Scholar] [CrossRef]
- Wu, S.-L.; Li, Z.-L.; Qin, J.-X. Geoacoustic Inversion for Bottom Parameters in the Deep-Water Area of the South China Sea. Chin. Phys. Lett. 2015, 32, 124301. [Google Scholar] [CrossRef]
- Yang, K.; Xu, L.; Yang, Q.; Li, G. Two-Step Inversion of Geoacoustic Parameters with Bottom Reverberation and Transmission Loss in the Deep Ocean. Acoust. Aust. 2018, 46, 131–142. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, R.; Yan, J.; Li, F.; Liu, J. Geoacoustic Inversion by Matched-Field Processing Combined with Vertical Reflection Coefficients and Vertical Correlation. IEEE J. Ocean. Eng. 2004, 29, 973–979. [Google Scholar] [CrossRef]
- Zhou, J.-X.; Zhang, X.-Z.; Rogers, P.; Simmen, J.; Dahl, P.; Jin, G.; Peng, Z. Reverberation Vertical Coherence and Sea-Bottom Geoacoustic Inversion in Shallow Water. IEEE J. Ocean. Eng. 2004, 29, 988–999. [Google Scholar] [CrossRef]
- Barclay, D.R.; Bevans, D.A.; Buckingham, M.J. Estimation of the Geoacoustic Properties of the New England Mud Patch from the Vertical Coherence of the Ambient Noise in the Water Column. IEEE J. Ocean. Eng. 2019, 45, 51–59. [Google Scholar] [CrossRef]
- Chapman, N.R. Perspectives on Geoacoustic Inversion of Ocean Bottom Reflectivity Data. J. Mar. Sci. Eng. 2016, 4, 61. [Google Scholar] [CrossRef] [Green Version]
- Yang, K.; Xiao, P.; Duan, R.; Ma, Y. Bayesian Inversion for Geoacoustic Parameters from Ocean Bottom Reflection Loss. J. Comput. Acoust. 2017, 25, 1750019. [Google Scholar] [CrossRef]
- Li, H.; Guo, X.-Y.; Ma, L. Estimating structure and geoacoustic parameters of sub-bottom by using spatial characteristics of ocean ambient noise in shallow water. Acta Phys. Sin. 2019, 68, 20190824, (In Chinese with English abstract). [Google Scholar] [CrossRef]
- Belcourt, J.; Holland, C.W.; Dosso, S.E.; Dettmer, J.; Goff, J.A. Depth-Dependent Geoacoustic Inferences with Dispersion at the New England Mud Patch via Reflection Coefficient Inversion. IEEE J. Ocean. Eng. 2019, 45, 69–91. [Google Scholar] [CrossRef]
- Li, Z.; Li, F. Geoacoustic inversion for sediments in the South China Sea based on a hybrid inversion scheme. Chin. J. Oceanol. Limnol. 2010, 28, 990–995. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, R. Hybrid geoacoustic inversion scheme with an equivalent seabed model. J. Acoust. Soc. Am. 2012, 131, 3487. [Google Scholar] [CrossRef]
- Li, M.Z.; Li, Z.L.; Li, Q.Q. Geoacoustic inversion for bottom parameters in a thermocline environment in the northern area of the South China Sea. Acta Acust. 2020, 39, 14. [Google Scholar]
- Aunsri, N.; Michalopoulou, Z.-H. Sequential filtering for dispersion tracking and sediment sound speed inversion. J. Acoust. Soc. Am. 2014, 136, 2665–2674. [Google Scholar] [CrossRef]
- Bonnel, J.; Dosso, S.E.; Eleftherakis, D.; Chapman, N.R. Trans-Dimensional Inversion of Modal Dispersion Data on the New England Mud Patch. IEEE J. Ocean. Eng. 2019, 45, 116–130. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Yang, K.; Ma, Y.; Yang, Q.; Huang, C. Synchrosqueezing transform for geoacoustic inversion with air-gun source in the East China Sea. Appl. Acoust. 2020, 169, 107460. [Google Scholar] [CrossRef]
- Jiang, Y.-M.; Dosso, S.E.; Bonnel, J.; Wilson, P.S.; Knobles, D.P. Passive Acoustic Glider for Seabed Characterization at the New England Mud Patch. IEEE J. Ocean. Eng. 2021, 1–12. [Google Scholar] [CrossRef]
- Weng, J.B.; Li, F.H.; Guo, Y.G. The sound field frequency-range interference patterns in deep water: Theory and experiment. Acta Acust. 2015, 41, 330–342, (In Chinese with English abstract). [Google Scholar] [CrossRef]
- Weng, J.B.; Li, F.H.; Guo, Y.G. Geoacoustic inversion based on near-field frequency-range interference pattern in deep water. Acta Acust. 2015, 40, 207–215, (In Chinese with English abstract). [Google Scholar] [CrossRef]
- Wang, P.; Song, W. Matched-field geoacoustic inversion using propagation invariant in a range-dependent waveguide. J. Acoust. Soc. Am. 2020, 147, EL491–EL497. [Google Scholar] [CrossRef]
- Brekhovskikh, B.M. Waves in Layered Media: Applied Mathematics and Mechanics; Academic Press: Cambridge, MA, USA, 1980. [Google Scholar]
- Otsu, N. A threshold selection method from gray-level histograms. IEEE Trans. Syst. Man Cybern. 1979, 9, 62–66. [Google Scholar] [CrossRef] [Green Version]
- Fritsch, F.N.; Carlson, R.E. Monotone Piecewise Cubic Interpolation. SIAM J. Numer. Anal. 1980, 17, 238–246. [Google Scholar] [CrossRef]
- Huang, N.E.; Shen, Z.; Long, S.R.; Wu, M.C.; Shih, H.H.; Zheng, Q.; Yen, N.-C.; Tung, C.C.; Liu, H.H. The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 1998, 454, 903–995. [Google Scholar] [CrossRef]
- Hamilton, E.L.; Bachman, R.T. Sound velocity and related properties of marine sediments. J. Acoust. Soc. Am. 1982, 72, 1891–1904. [Google Scholar] [CrossRef]
- Gingras, D.F. Inversion for geometric and geoacoustic parameters in shallow water: Experimental results. J. Acoust. Soc. Am. 1995, 97, 3589–3598. [Google Scholar] [CrossRef] [Green Version]
- Gerstoft, P. Inversion of seismoacoustic data using genetic algorithms and a posteriori probability distributions. J. Acoust. Soc. Am. 1994, 95, 770–782. [Google Scholar] [CrossRef] [Green Version]

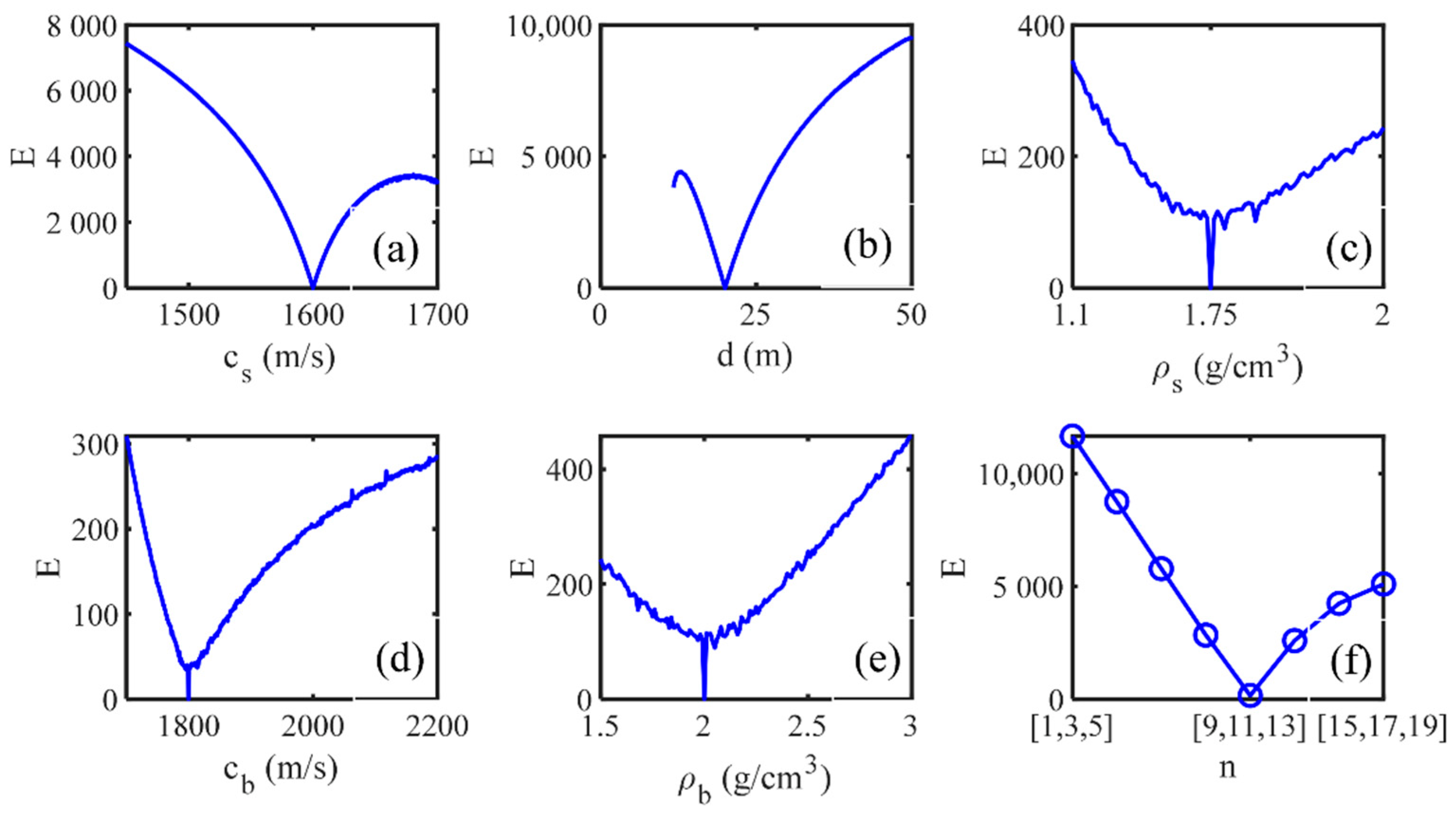
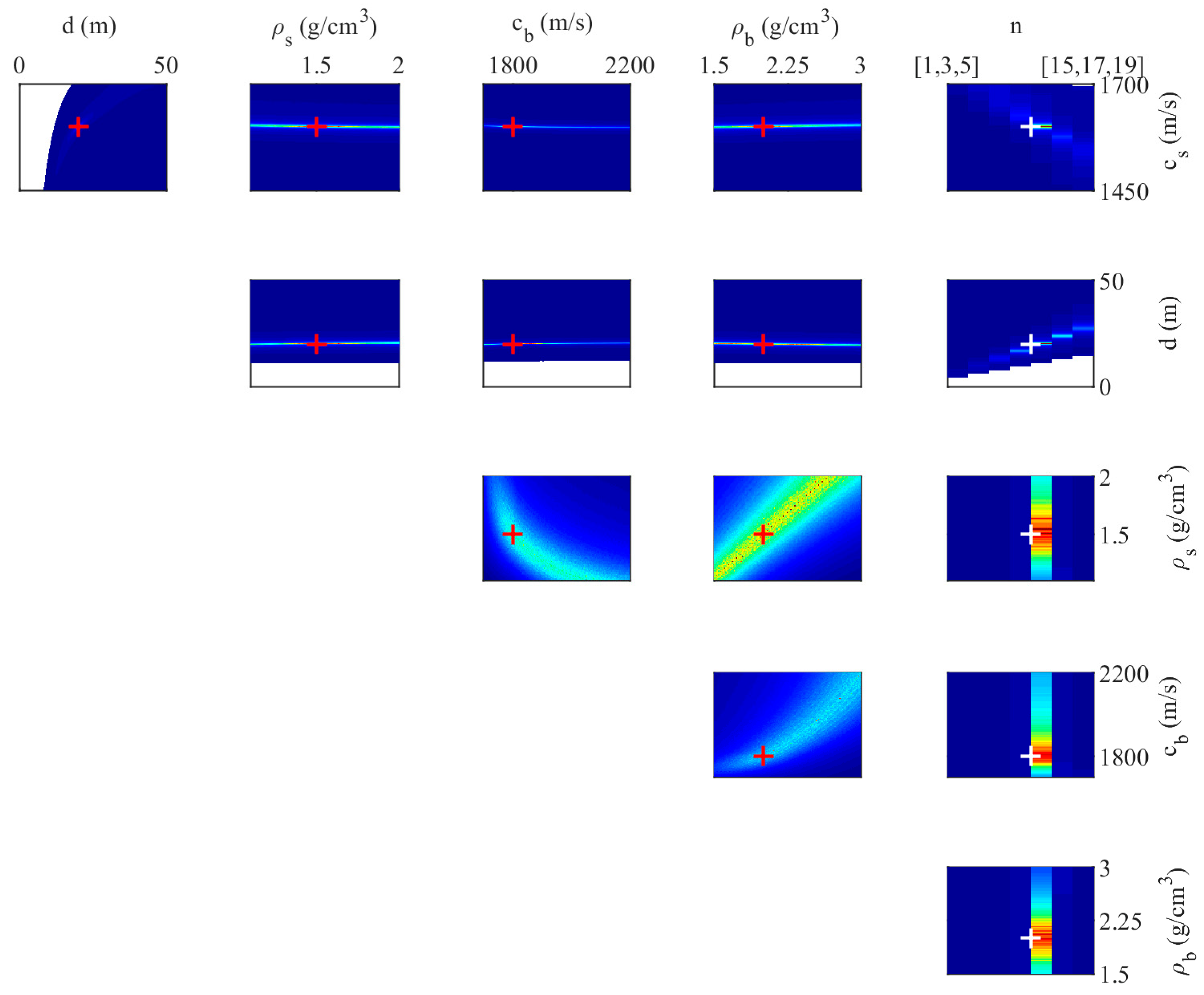

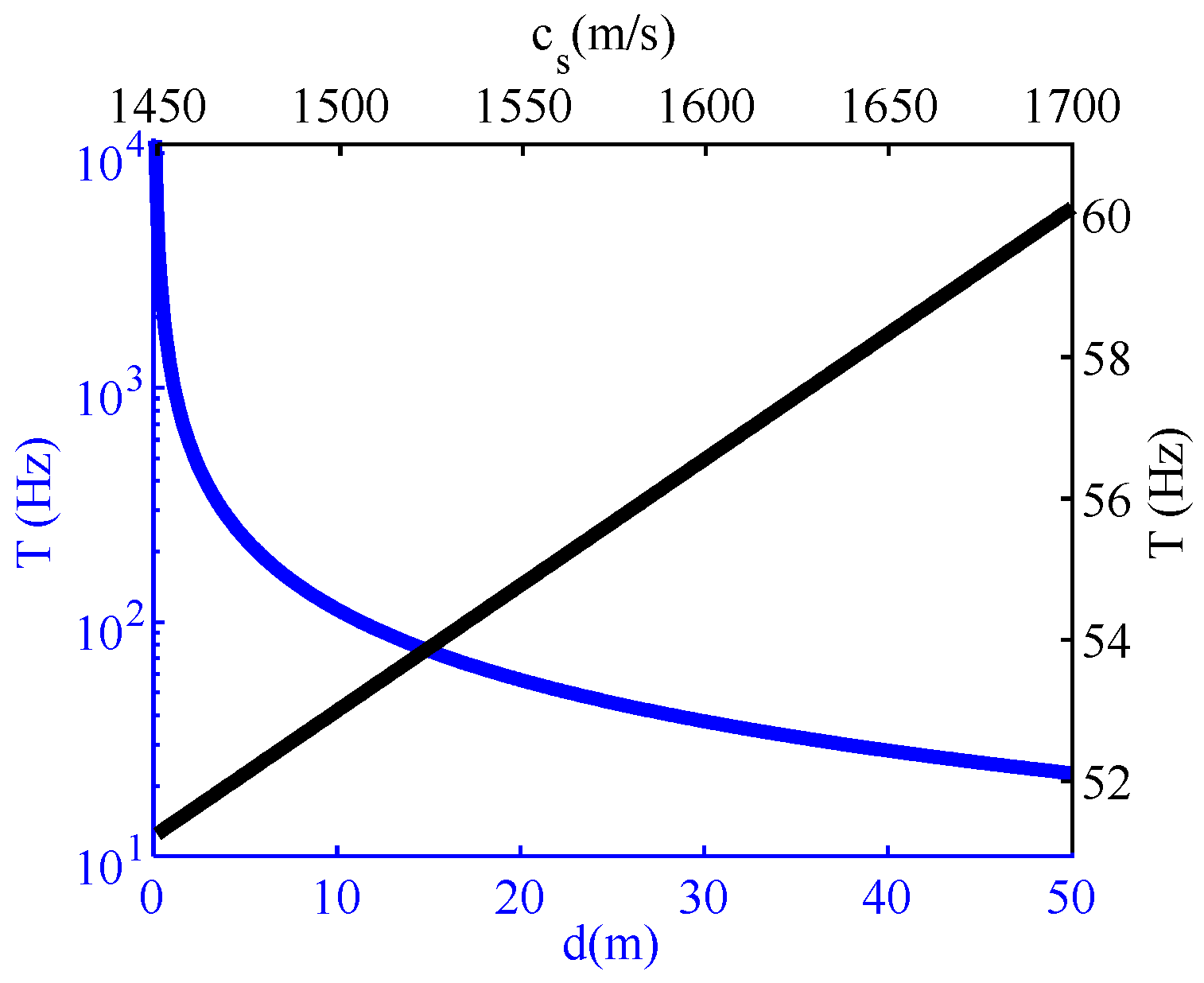
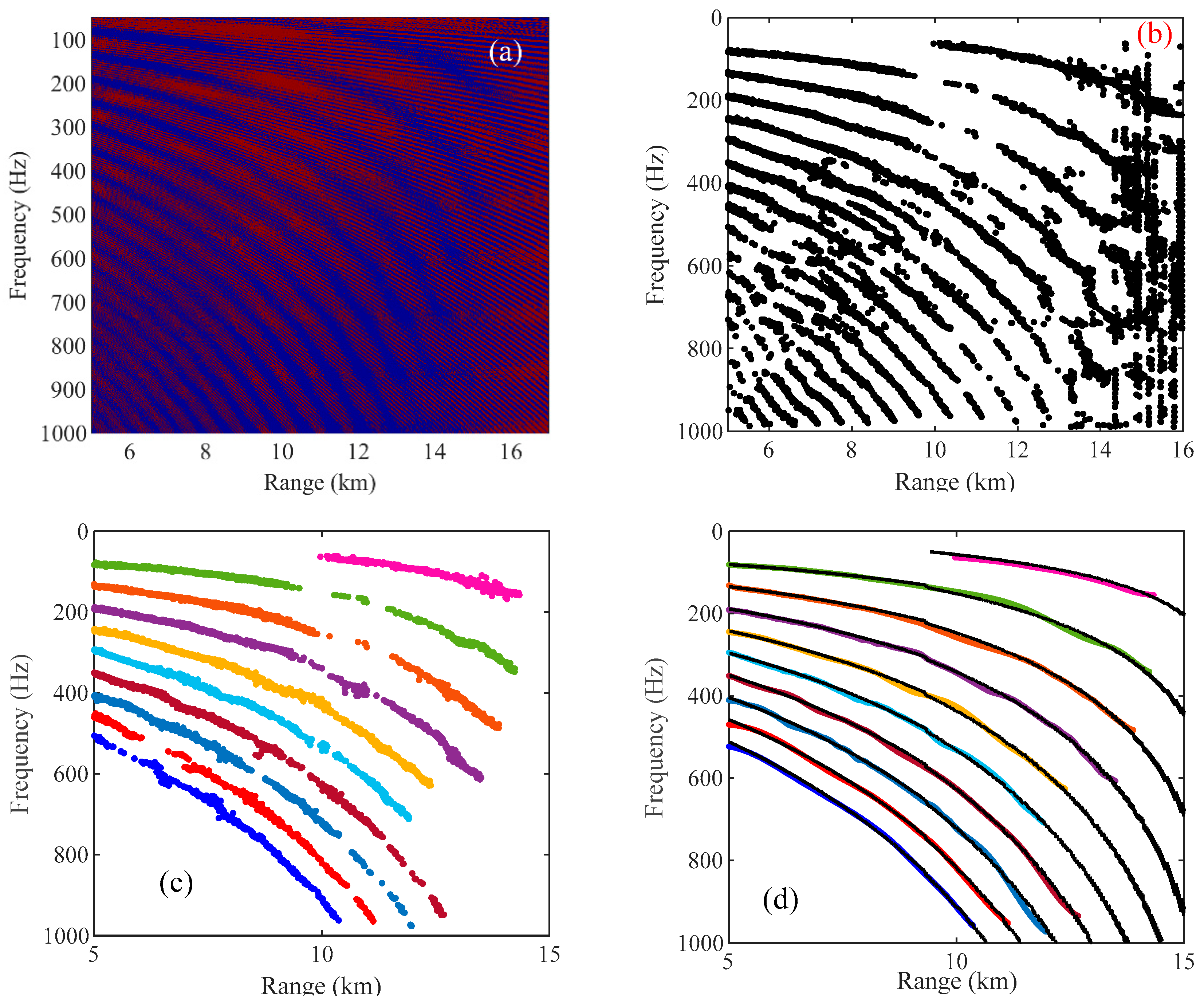

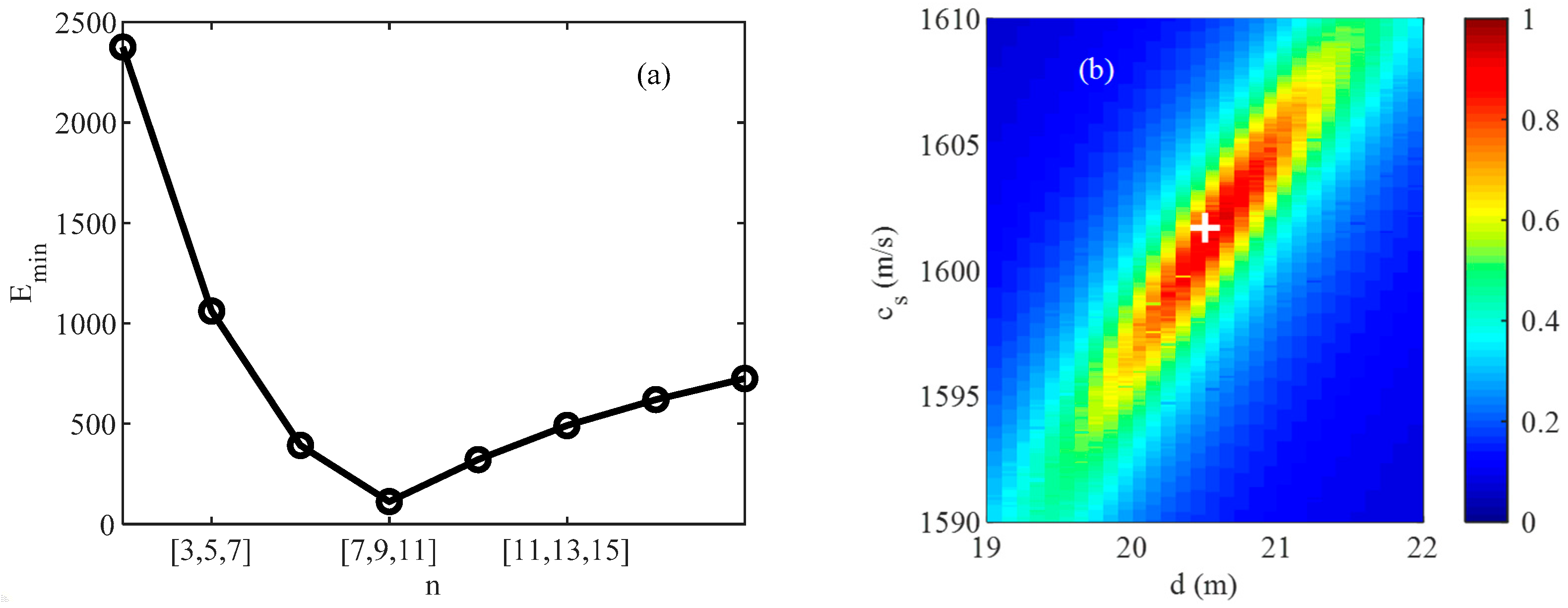
| Ocean Bottom Modal | Sound Speed (m/s) | Density (g/cm3) | Attenuation Coefficient (dB/λ) | Thickness (m) | |
|---|---|---|---|---|---|
| Semi-infinite ocean bottom | 1700.00 | 1.80 | 0.50 | - | |
| Layered ocean bottom | sediment | 1600.00 | 1.50 | 0.20 | 20.0 |
| basement | 1800.00 | 2.00 | 1.00 | - | |
| Parameter | Range | True Value |
|---|---|---|
| (m/s) | 1450 to 1700 | 1600.00 |
| (m) | 0 to 50 | 20.0 |
| (g/cm3) | 1.1 to 2.0 | 1.50 |
| (m/s) | 1700 to 2200 | 1800.00 |
| (g/cm3) | 1.5 to 3.0 | 2.0 |
| [1,3,5] to [15,17,19] | [9,11,13] |
| Inversion Parameters | Optimization Range | Optimization Result | True Values | Error |
|---|---|---|---|---|
| (m) | 0 to 50 | 20.23 | 20.0 | 1.15% |
| (m/s) | 1450 to 1700 | 1601.52 | 1600.00 | 0.095% |
| (g/cm3) | 1.1 to 2.0 | 1.47 | 1.50 | 4.50% |
| (m/s) | 1700 to 2200 | 2018.18 | 1800.00 | 12.12% |
| (g/cm3) | 1.5 to 3.0 | 2.48 | 2.00 | 24.00% |
| [1,3,5] to [15,17,19] | [7,9,11] | [7,9,11] | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Hu, C.; Zhao, M. Inversion of Sound Speed and Thickness of High-Speed Sediment Using Interference Structure in Shadow Zone. Appl. Sci. 2022, 12, 5077. https://doi.org/10.3390/app12105077
Li Z, Hu C, Zhao M. Inversion of Sound Speed and Thickness of High-Speed Sediment Using Interference Structure in Shadow Zone. Applied Sciences. 2022; 12(10):5077. https://doi.org/10.3390/app12105077
Chicago/Turabian StyleLi, Zhanglong, Changqing Hu, and Mei Zhao. 2022. "Inversion of Sound Speed and Thickness of High-Speed Sediment Using Interference Structure in Shadow Zone" Applied Sciences 12, no. 10: 5077. https://doi.org/10.3390/app12105077
APA StyleLi, Z., Hu, C., & Zhao, M. (2022). Inversion of Sound Speed and Thickness of High-Speed Sediment Using Interference Structure in Shadow Zone. Applied Sciences, 12(10), 5077. https://doi.org/10.3390/app12105077






