Measurement of Absolute Acoustic Nonlinearity Parameter Using Laser-Ultrasonic Detection
Abstract
1. Introduction
2. Brief Description of Theoretical Background
2.1. Absolute Acoustic Nonlinearity Parameter (β)
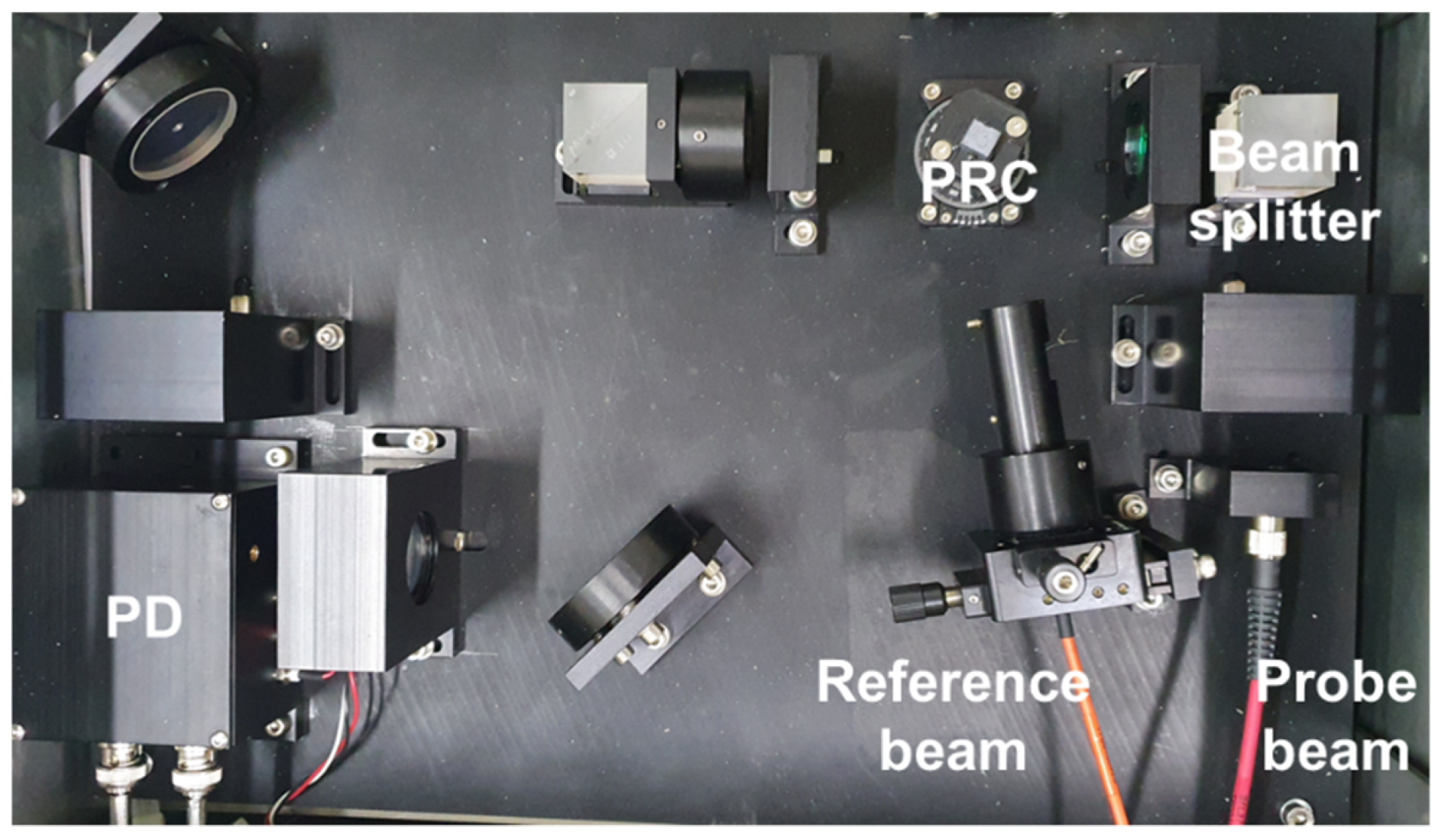
2.2. Laser-Ultrasonic Detection Using Photorefractive Interferometer
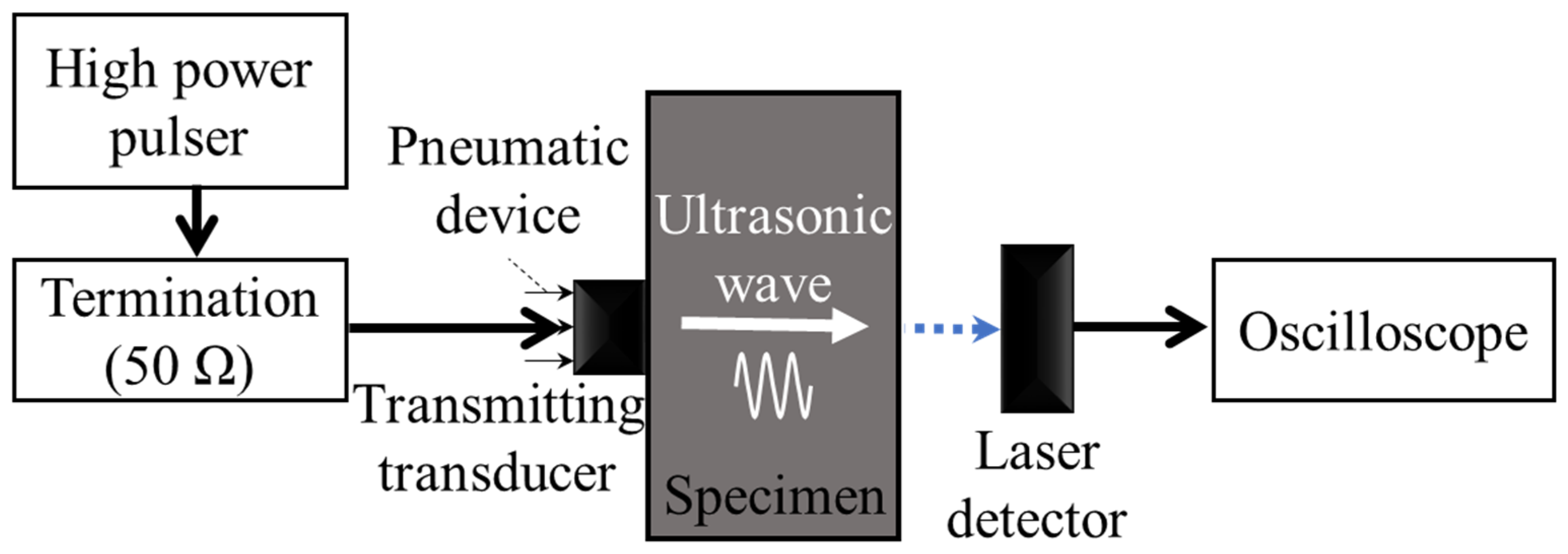
3. Experimental Setup
3.1. Test Specimens
3.2. Ultrasonic Measurement Using Laser Detection
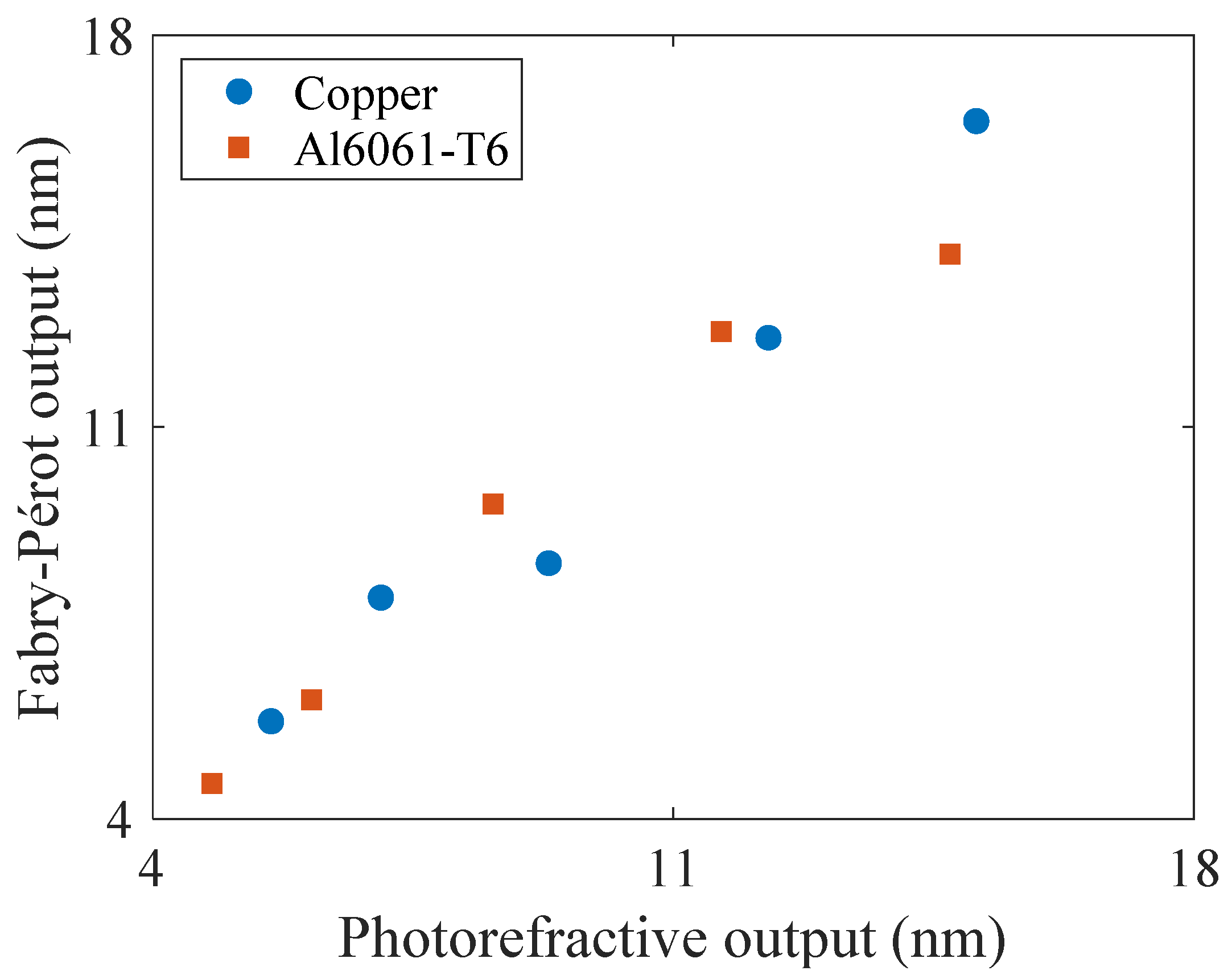
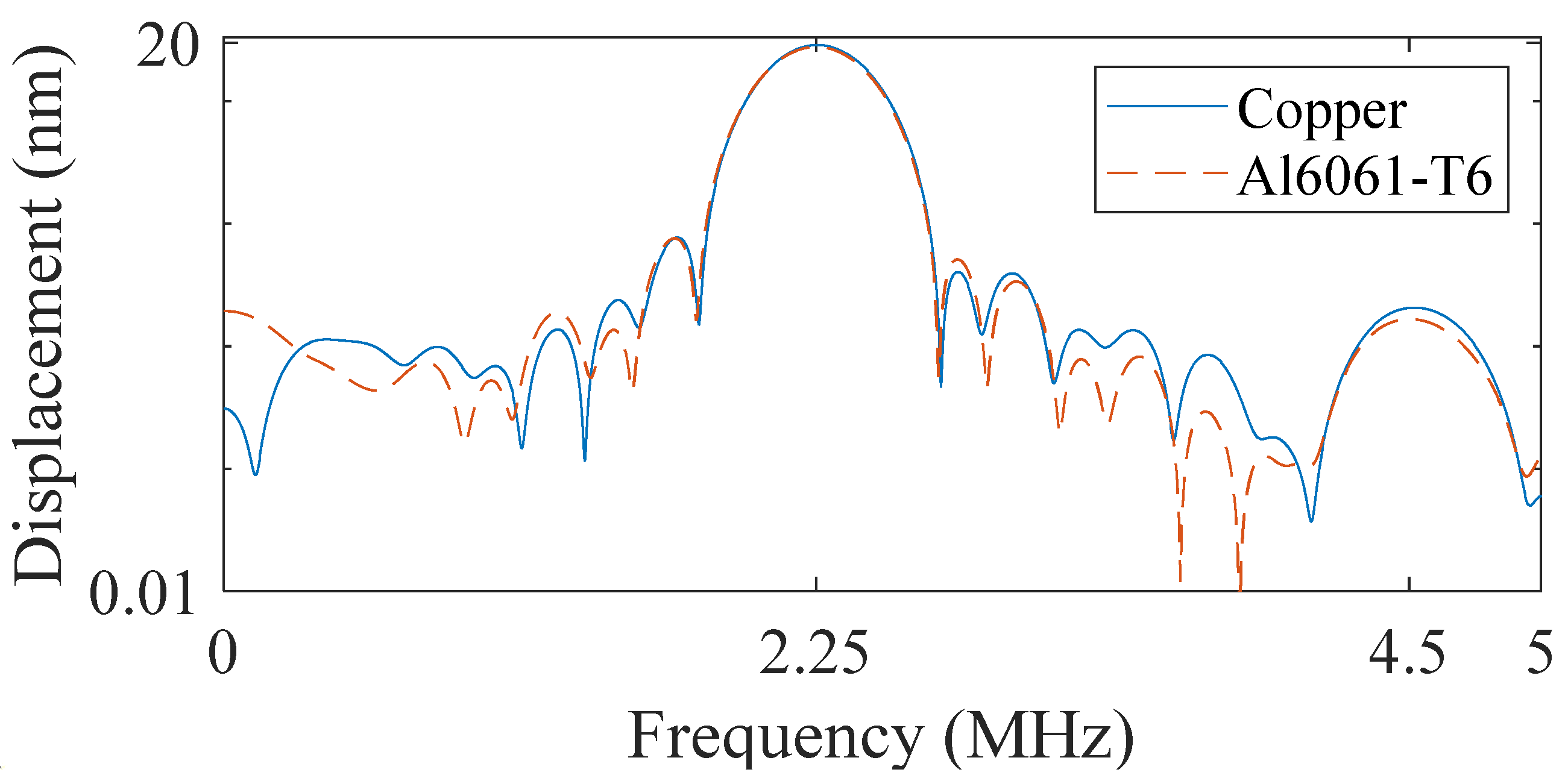
4. Experimental Results
4.1. Laser-Ultrasonic Detection Results
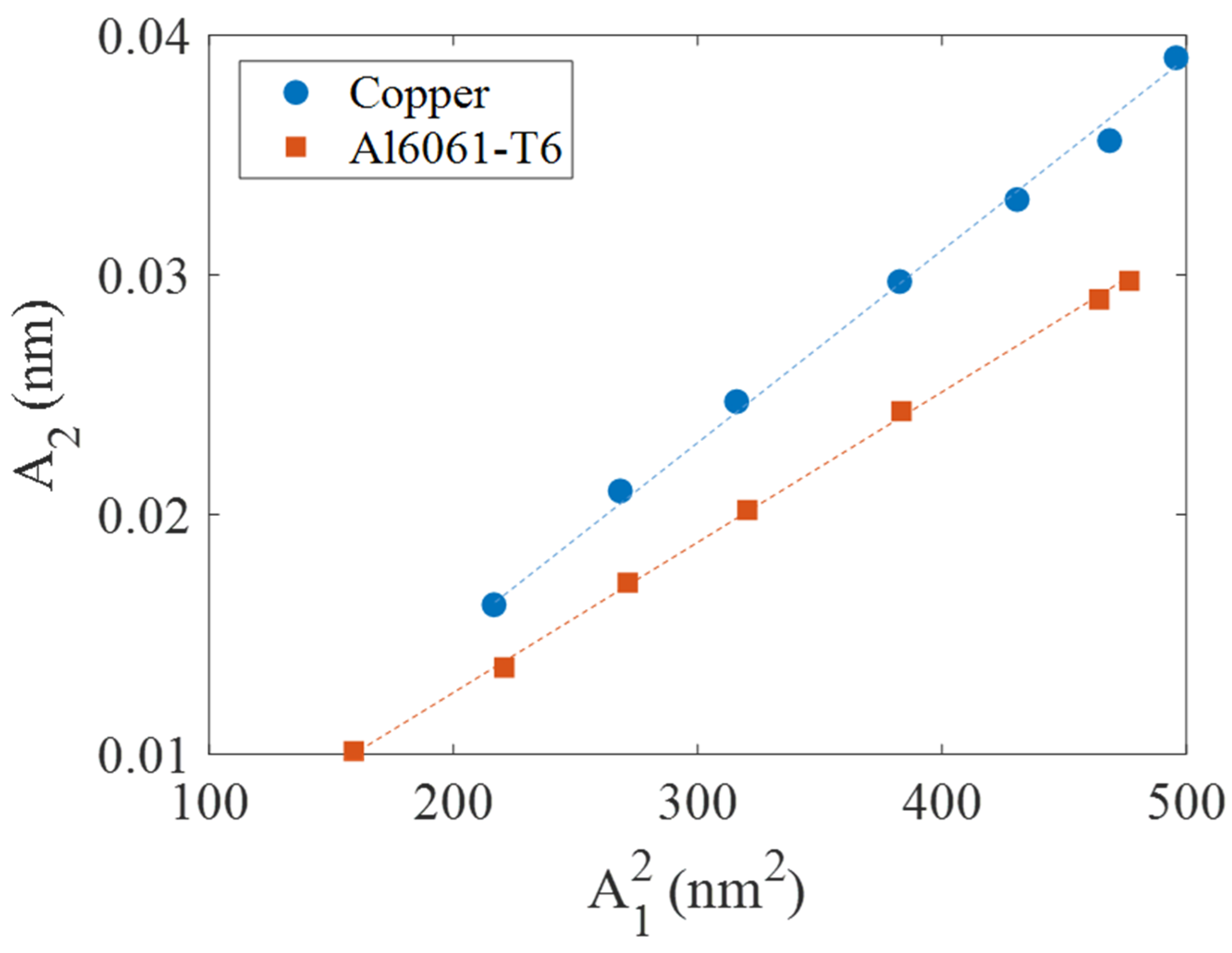
4.2. Validations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Jhang, K.Y. Nonlinear ultrasonic techniques for nondestructive assessment of micro damage in material: A review. Int. J. Precis. Eng. Manuf. 2009, 10, 139. [Google Scholar] [CrossRef]
- Kim, J.; Ha, H.-P.; Kim, K.-M.; Jhang, K.-Y. Analysis of the Influence of Surface Roughness on Measurement of Ultrasonic Nonlinearity Parameter Using Contact-Type Transducer. Appl. Sci. 2020, 10, 8661. [Google Scholar] [CrossRef]
- Park, J.; Kim, M.; Chi, B.; Jang, C. Correlation of metallurgical analysis & higher harmonic ultrasound response for long term isothermally aged and crept FM steel for USC TPP turbine rotors. NDT&E Int. 2013, 54, 159–165. [Google Scholar]
- Li, M.; Lomonosov, A.M.; Shen, Z.; Seo, H.; Jhang, K.-Y.; Gusev, V.E.; Ni, C. Monitoring of thermal aging of aluminum alloy via nonlinear propagation of acoustic pulses generated and detected by lasers. Appl. Sci. 2019, 9, 1191. [Google Scholar] [CrossRef]
- Cantrell, J.H. Dependence of microelastic-plastic nonlinearity of martensitic stainless steel on fatigue damage accumulation. J. Appl. Phys. 2006, 100, 063508. [Google Scholar] [CrossRef]
- Balasubramaniam, K.; Valluri, J.S.; Prakash, R.V. Creep damage characterization using a low amplitude nonlinear ultrasonic technique. Mater. Charact. 2011, 62, 275–286. [Google Scholar] [CrossRef]
- Liu, M.H.; Kim, J.Y.; Jacobs, L.; Qu, J.M. Experimental study of nonlinear Rayleigh wave propagation in shot-peened aluminum plates-Feasibility of measuring residual stress. NDT&E Int. 2011, 44, 67–74. [Google Scholar]
- Zeitvogel, D.T.; Matlack, K.H.; Kim, J.Y.; Jacobs, L.J.; Singh, P.M.; Qu, J.M. Characterization of stress corrosion cracking in carbon steel using nonlinear Rayleigh surface waves. NDT&E Int. 2014, 62, 144–152. [Google Scholar]
- Jhang, K.-Y.; Choi, S.; Kim, J. Measurement of Nonlinear Ultrasonic Parameters from Higher Harmonics. In Measurement of Nonlinear Ultrasonic Characteristics; Springer: Berlin/Heidelberg, Germany, 2020; pp. 9–60. [Google Scholar]
- Doerr, C.; Kim, J.-Y.; Singh, P.; Wall, J.J.; Jacobs, L. Evaluation of sensitization in stainless steel 304 and 304L using nonlinear Rayleigh waves. NDT&E Int. 2017, 88, 17–23. [Google Scholar]
- Cantrell, J.H.; Yost, W.T. Effect of precipitate coherency strains on acoustic harmonic generation. Int. J. Appl. Phys. 1997, 81, 2957–2962. [Google Scholar] [CrossRef]
- Cantrell, J.H.; Yost, W.T. Determination of precipitate nucleation and growth rates from ultrasonic harmonic generation. Appl. Phys. Lett. 2000, 77, 1952–1954. [Google Scholar] [CrossRef]
- Cantrell, J.H.; Zhang, X.-G. Nonlinear acoustic response from precipitate-matrix misfit in a dislocation network. Int. J. Appl. Phys. 1998, 84, 5469–5472. [Google Scholar] [CrossRef]
- Park, S.-H.; Kim, J.; Jhang, K.-Y. Relative measurement of the acoustic nonlinearity parameter using laser detection of an ultrasonic wave. Int. J. Precis. Eng. Manuf. 2017, 18, 1347–1352. [Google Scholar] [CrossRef]
- Hong, X.; Liu, Y.; Lin, X.; Luo, Z.; He, Z. Nonlinear ultrasonic detection method for delamination damage of lined anti-corrosion pipes using PZT transducers. Appl. Sci. 2018, 8, 2240. [Google Scholar] [CrossRef]
- Kim, J.; Jhang, K.-Y.; Kim, C. Dependence of nonlinear ultrasonic characteristic on second-phase precipitation in heat-treated Al 6061-T6 alloy. Ultrasonics 2018, 82, 84–90. [Google Scholar] [CrossRef]
- Jhang, K.Y. Applications of nonlinear ultrasonics to the NDE of material degradation. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2000, 47, 540–548. [Google Scholar] [CrossRef]
- Choi, S.; Lee, P.; Jhang, K.Y. A Pulse Inversion-Based Nonlinear Ultrasonic Technique using a Single-Cycle Longitudinal Wave for Evaluating Localized Material Degradation in Plates. Int. J. Precis. Eng. Manuf. 2019, 20, 549–558. [Google Scholar] [CrossRef]
- Dace, G.E.; Thompson, R.B.; Brasche, L.J.; Rehbein, D.K.; Buck, O. Nonlinear acoustics, a technique to determine microstructural changes in materials. In Review of Progress in Quantitative Nondestructive Evaluation; Springer: Boston, MA, USA, 1991; pp. 1685–1692. [Google Scholar]
- Kim, J.; Song, D.-G.; Jhang, K.-Y. Absolute measurement and relative measurement of ultrasonic nonlinear parameters. Res. Nondestruct. Eval. 2017, 28, 211–225. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.-G.; Kong, B.; Kim, K.-M.; Jang, C.; Kang, S.-S.; Jhang, K.-Y. Applicability of nonlinear ultrasonic technique to evaluation of thermally aged CF8M cast stainless steel. Nucl. Eng. Technol. 2020, 52, 621–625. [Google Scholar] [CrossRef]
- Kim, J.; Kim, C.-S.; Kim, K.-C.; Jhang, K.-Y.; International, E. Evaluation of yield strength by ultrasonic reconstruction of quadratic nonlinear Stress–Strain curve. NDT&E Int. 2020, 112, 102242. [Google Scholar]
- Choi, S.; Seo, H.; Jhang, K.Y. Noncontact Evaluation of Acoustic Nonlinearity of a Laser-Generated Surface Wave in a Plastically Deformed Aluminum Alloy. Res. Nondestruct. Eval. 2015, 26, 13–22. [Google Scholar] [CrossRef]
- Dace, G.E.; Thompson, R.B.; Buck, O. Measurement of the acoustic harmonic generation for materials characterization using contact transducers. Rev. Prog. Quant. Nondestruct. Eval. 1992, 11, 2069–2076. [Google Scholar]
- Yost, W.T.; Cantrell, J.H. Absolute ultrasonic displacement amplitude measurements with a submersible electrostatic acoustic transducer. Rev. Sci. Instrum. 1992, 63, 4182–4188. [Google Scholar] [CrossRef]
- Hurley, D.; Yost, W.; Boltz, E.; Fortunko, C. A Comparison of Three Techniques to Determine the Nonlinear Ultrasonic Parameter β. In Review of Progress in Quantitative Nondestructive Evaluation; Springer: Boston, MA, USA, 1997; pp. 1383–1390. [Google Scholar]
- Zhou, X.L.; Yu, Q.X. Wide-Range Displacement Sensor Based on Fiber-Optic Fabry–Perot Interferometer for Subnanometer Measurement. IEEE Sens. J. 2011, 11, 1602–1606. [Google Scholar] [CrossRef]
- Park, S.-H.; Hong, J.-Y.; Ha, T.; Choi, S.; Jhang, K.-Y. Deep Learning-Based Ultrasonic Testing to Evaluate the Porosity of Additively Manufactured Parts with Rough Surfaces. Metals 2021, 11, 290. [Google Scholar] [CrossRef]
- Delaye, P.; Blouin, A.; Drolet, D.; de Montmorillon, L.-A.; Roosen, G.; Monchalin, J.-P. Detection of ultrasonic motion of a scattering surface by photorefractive InP: Fe under an applied dc field. JOSA B 1997, 14, 1723–1734. [Google Scholar] [CrossRef]
- Blouin, A.; Delaye, P.; Drolet, D.; Monchalin, J.P. Optical detection of ultrasound by two-wave mixing in photorefractive semiconductor crystals under applied field. In Review of Progress in Quantitative Nondestructive Evaluation; Springer: Boston, MA, USA, 1997; pp. 571–577. [Google Scholar]
- Pouet, B.F.; Ing, R.; Krishnaswamy, S.; Royer, D. Heterodyne interferometer with two-wave mixing in photorefractive crystals for ultrasound detection on rough surfaces. Appl. Phys. Lett. 1996, 69, 3782–3784. [Google Scholar] [CrossRef]
- Park, S.-H.; Choi, S.; Jhang, K.-Y. Porosity Evaluation of Additively Manufactured Components Using Deep Learning-based Ultrasonic Nondestructive Testing. Int. J. Precis. Eng. Manuf.-Green Tech. 2021, 1–13. [Google Scholar] [CrossRef]
- Harris, F.J. On the use of windows for harmonic analysis with the discrete Fourier transform. Proc. IEEE 1978, 66, 51–83. [Google Scholar] [CrossRef]
- Park, S.-H.; Jhang, K.-Y.; Yoon, H.-S.; Sohn, H. Porosity Evaluation of Additive Manufactured Parts: Ultrasonic Testing and Eddy Current Testing. J Korean Soc Nondestruct Test. 2021, 41, 1–10. [Google Scholar] [CrossRef]
- Cantrell, J.H. Crystalline-Structure and Symmetry Dependence of Acoustic Nonlinearity Parameters. J. Appl. Phys. 1994, 76, 3372–3380. [Google Scholar] [CrossRef]
- Yost, W.T.; Cantrell, J.H.; Breazeale, M.A. Ultrasonic nonlinearity parameters and third-order elastic constants of copper between 300 and 3 °K. J. Appl. Phys. 1981, 52, 126–128. [Google Scholar] [CrossRef]
- Li, P.; Yost, W.T.; Cantrell, J.H.; Salama, K. Dependence of acoustic nonlinearity parameter on second phase precipitates of aluminum alloys. In Proceedings of the IEEE 1985 Ultrasonics Symposium, San Francisco, CA, USA, 16–18 October 1985; pp. 1113–1115. [Google Scholar]



| Specimen | Copper | Al6061-T6 |
|---|---|---|
| Thickness (mm) | 20 | 20 |
| Density (kg/m3) | 8960 | 2700 |
| Longitudinal wave velocity (m/s) | 4750 | 6300 |
| Acoustic impedance (106 kg/m2s) | 42.6 | 17.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, S.-H.; Kim, J.; Song, D.-G.; Choi, S.; Jhang, K.-Y. Measurement of Absolute Acoustic Nonlinearity Parameter Using Laser-Ultrasonic Detection. Appl. Sci. 2021, 11, 4175. https://doi.org/10.3390/app11094175
Park S-H, Kim J, Song D-G, Choi S, Jhang K-Y. Measurement of Absolute Acoustic Nonlinearity Parameter Using Laser-Ultrasonic Detection. Applied Sciences. 2021; 11(9):4175. https://doi.org/10.3390/app11094175
Chicago/Turabian StylePark, Seong-Hyun, Jongbeom Kim, Dong-Gi Song, Sungho Choi, and Kyung-Young Jhang. 2021. "Measurement of Absolute Acoustic Nonlinearity Parameter Using Laser-Ultrasonic Detection" Applied Sciences 11, no. 9: 4175. https://doi.org/10.3390/app11094175
APA StylePark, S.-H., Kim, J., Song, D.-G., Choi, S., & Jhang, K.-Y. (2021). Measurement of Absolute Acoustic Nonlinearity Parameter Using Laser-Ultrasonic Detection. Applied Sciences, 11(9), 4175. https://doi.org/10.3390/app11094175







