Principles of Different X-ray Phase-Contrast Imaging: A Review
Abstract
1. Introduction
2. Methods
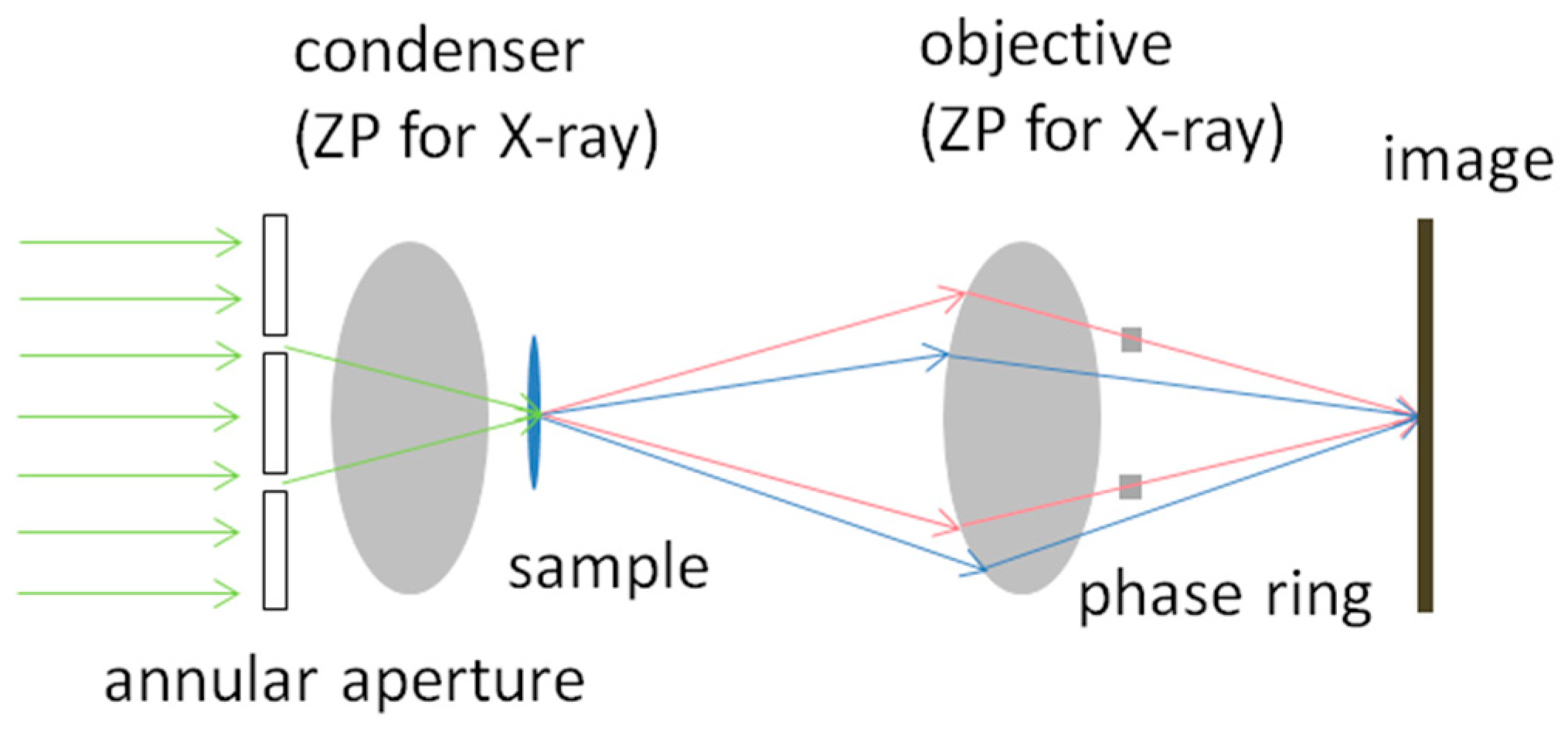
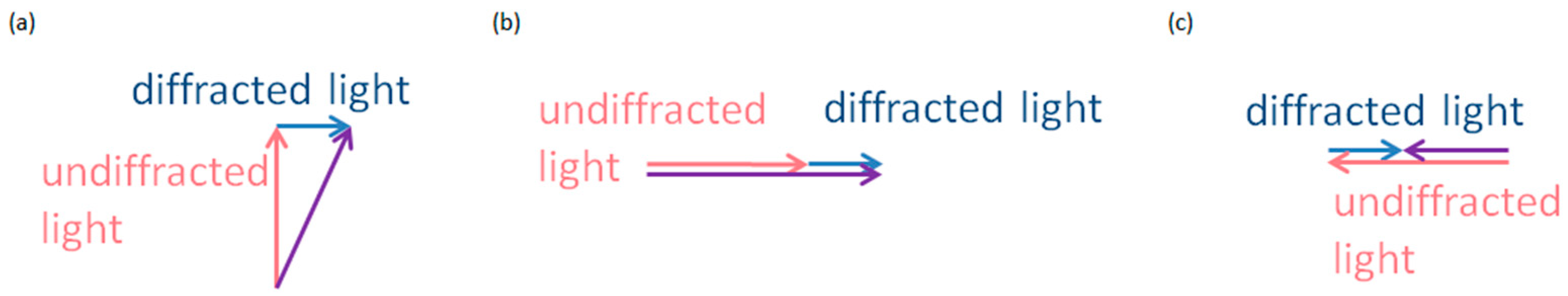
2.1. Zernike’s X-ray Microscopy
2.2. X-ray Holography
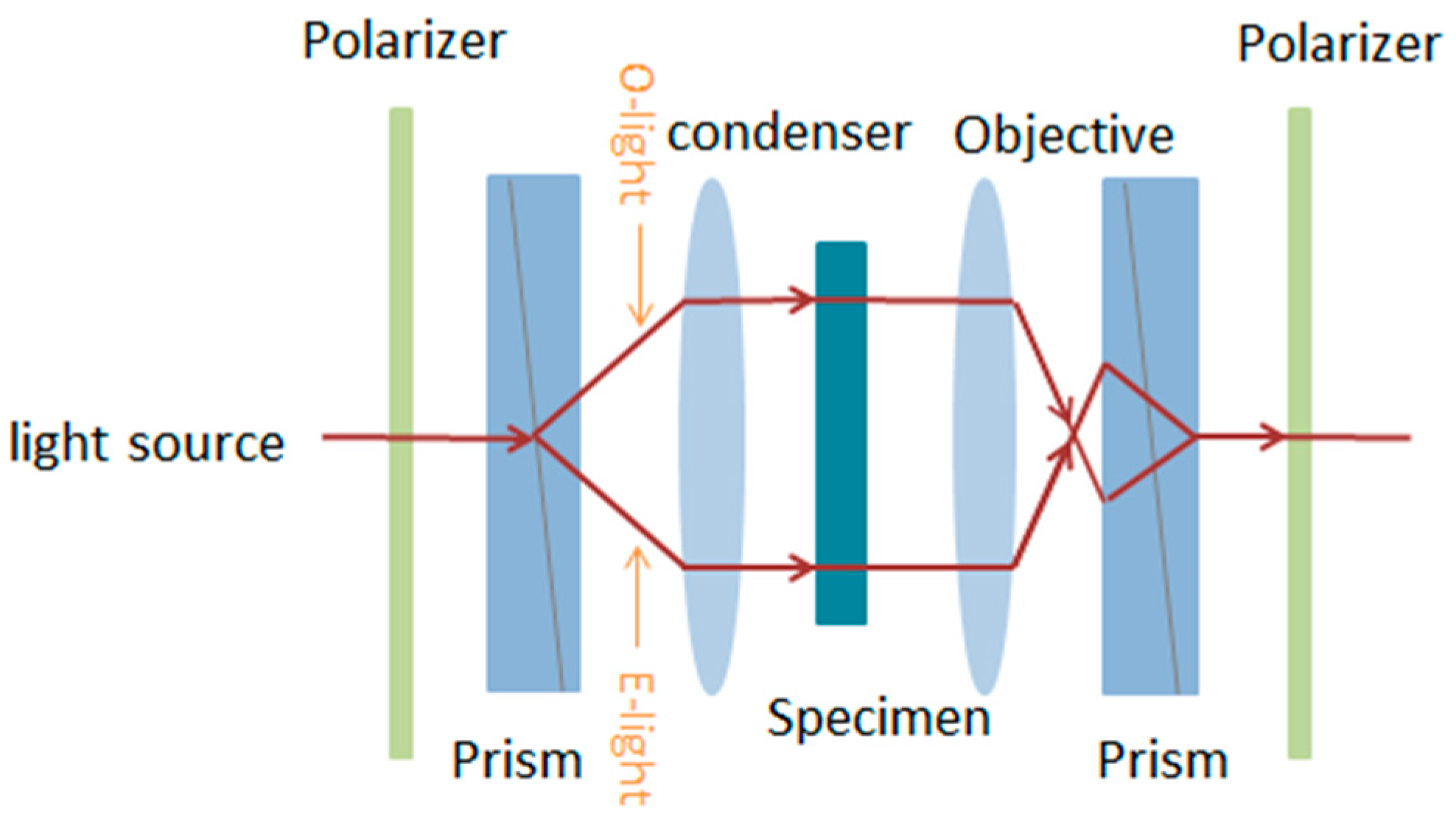
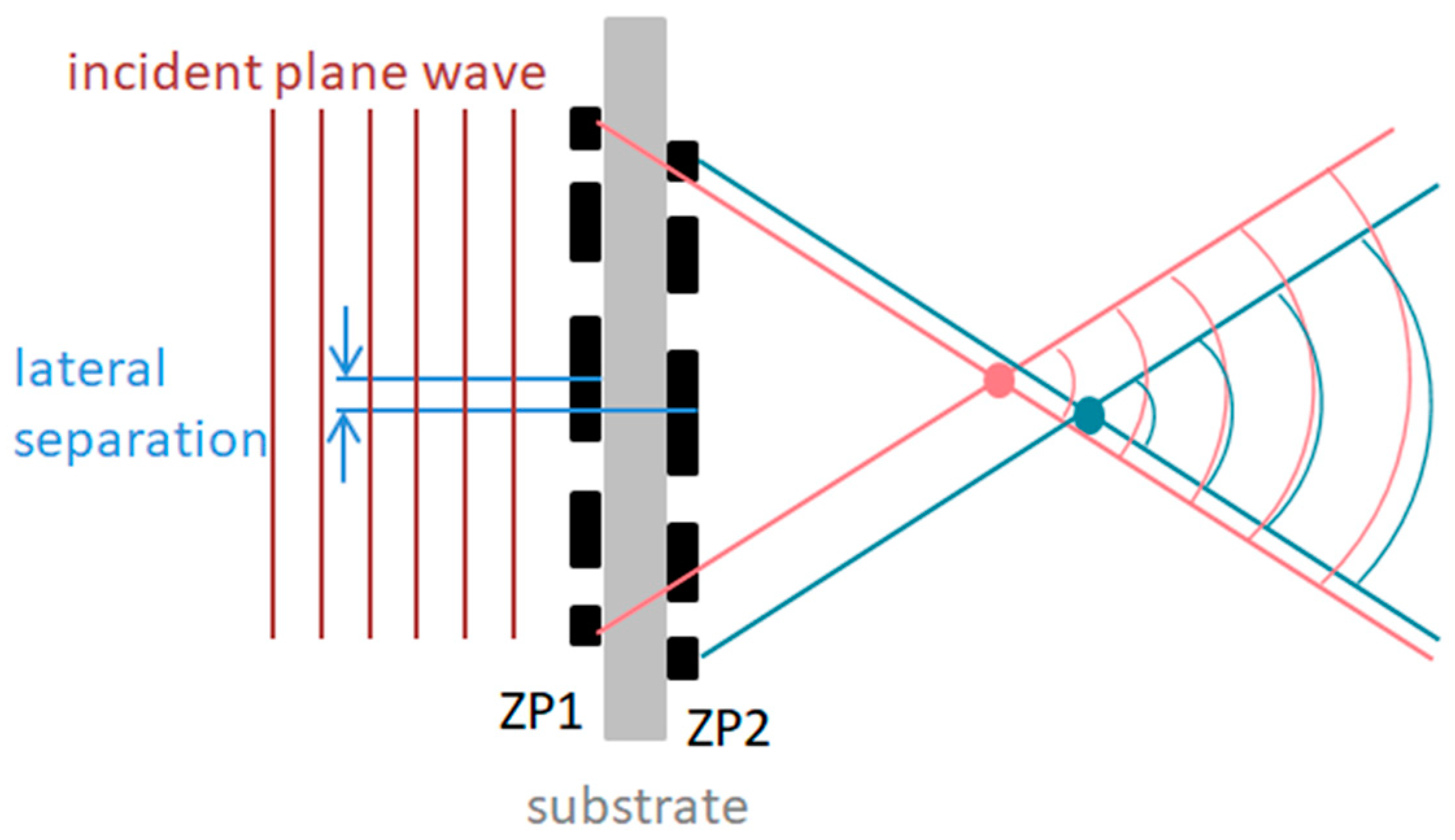
2.3. Differential X-ray Microscopy
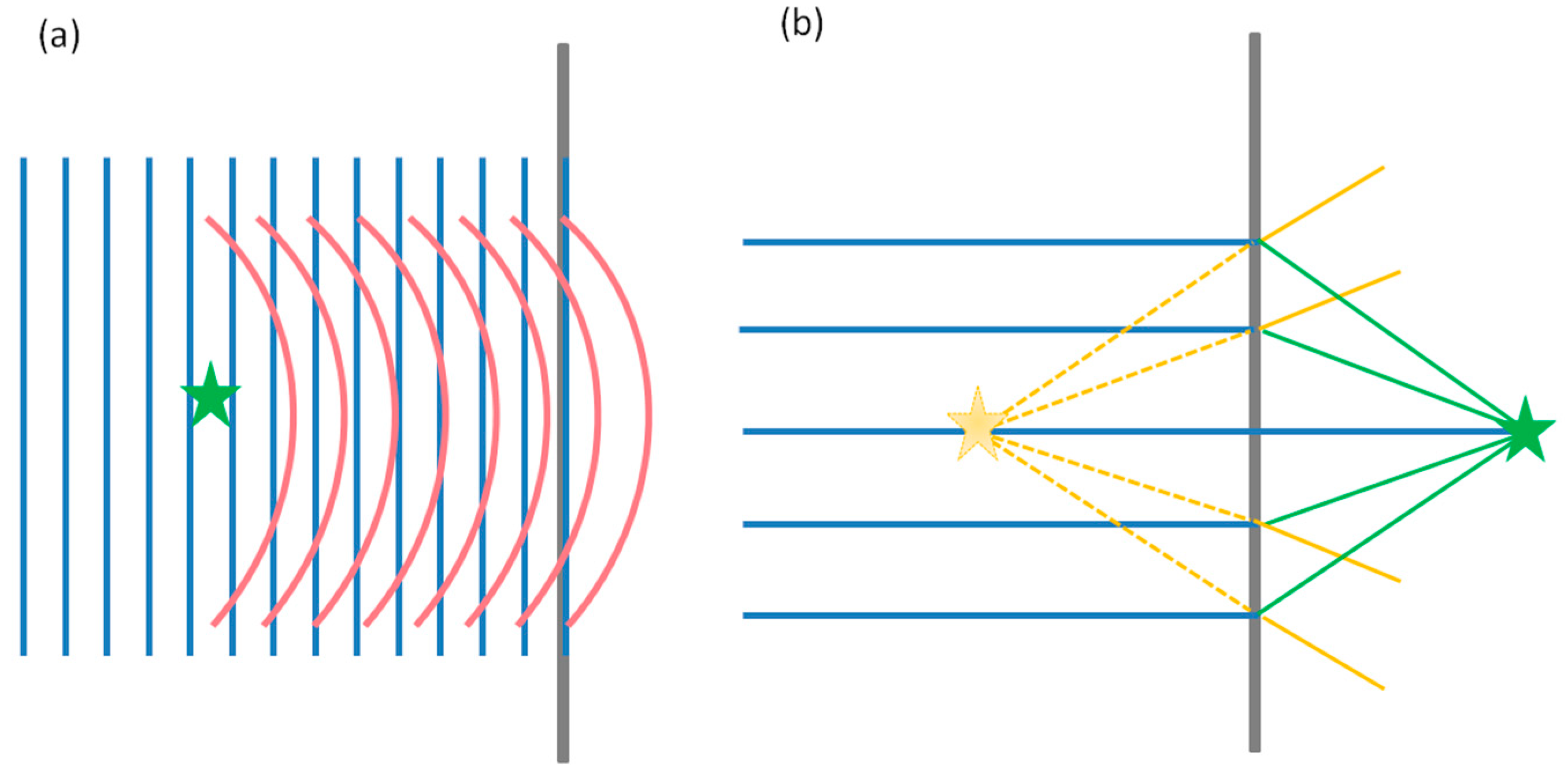
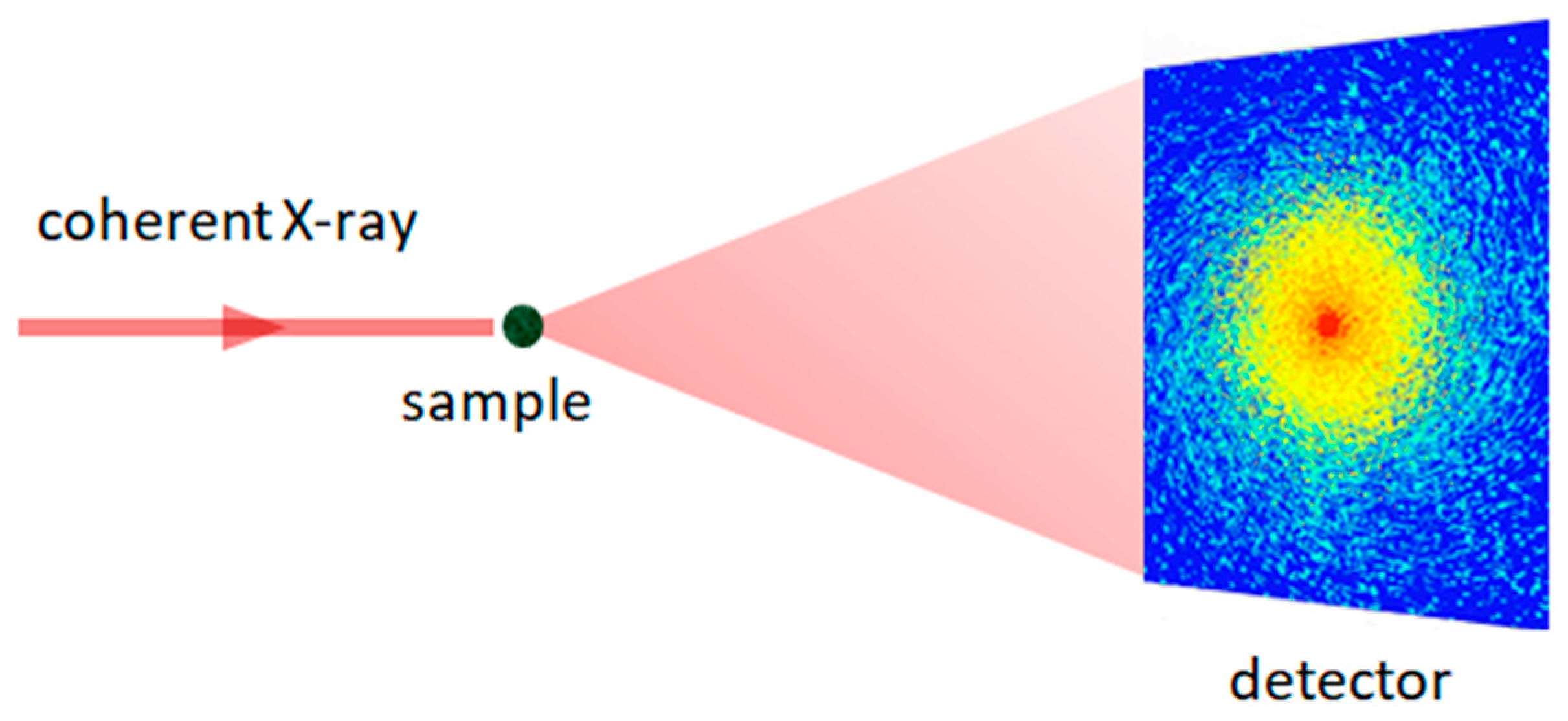
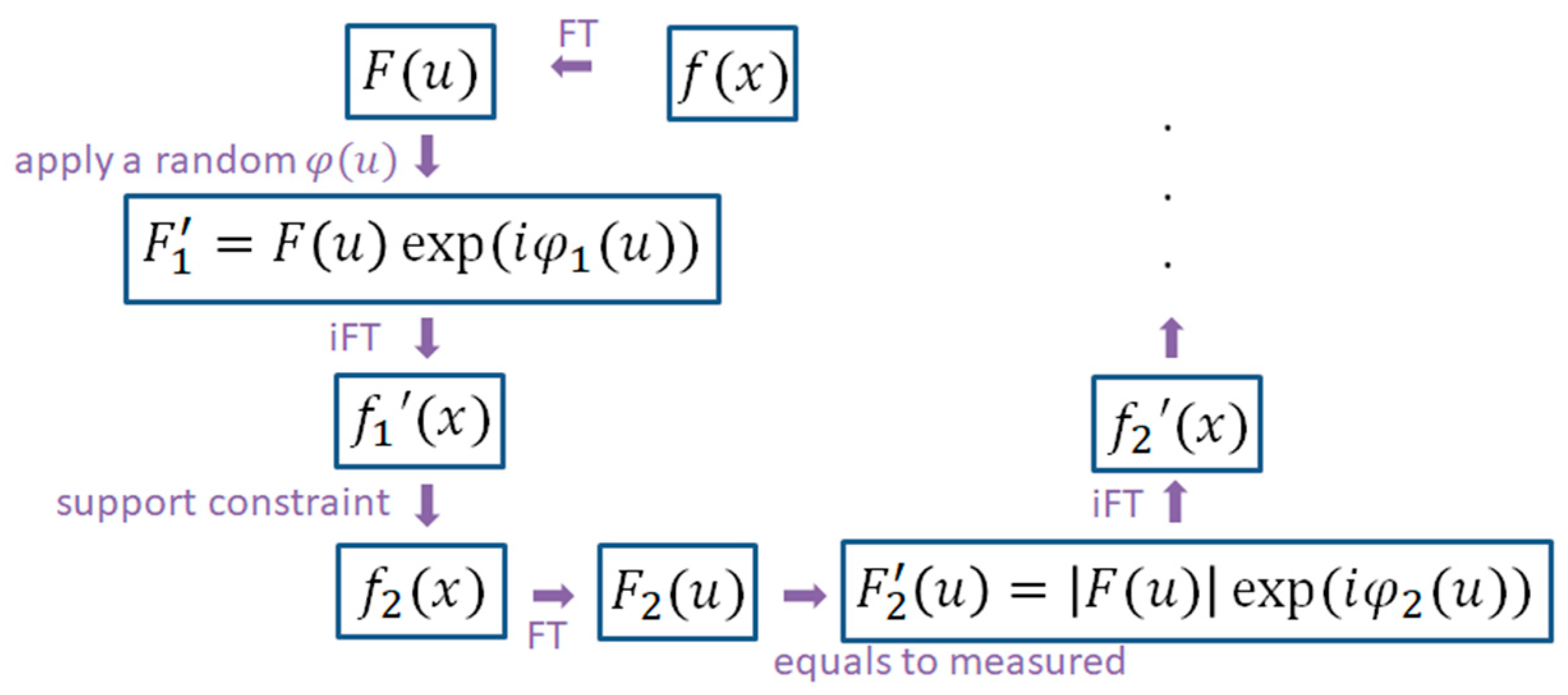
2.4. Coherent Diffraction Imaging
2.5. Crystal Interferometer
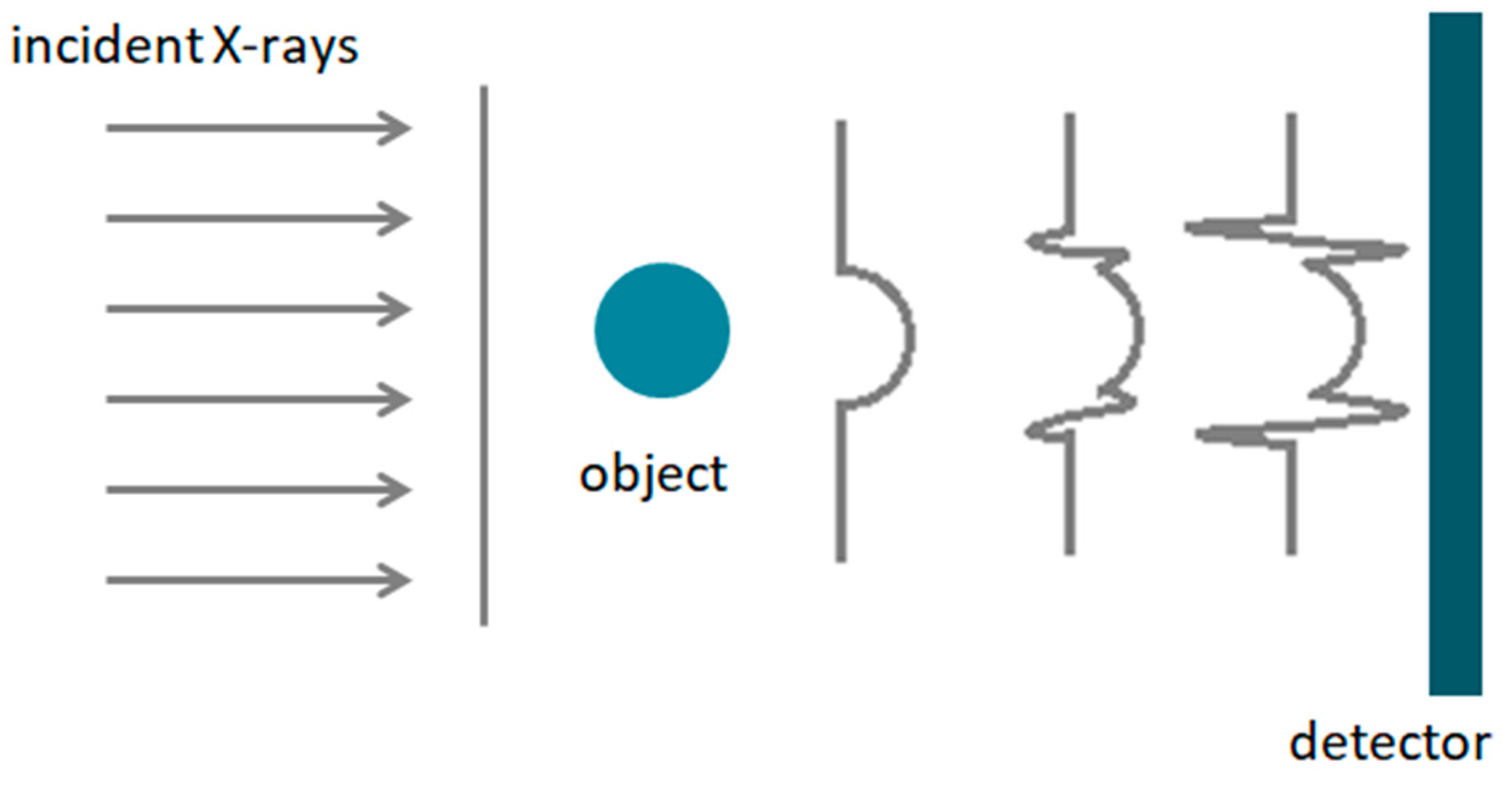
2.6. Grating Interferometer
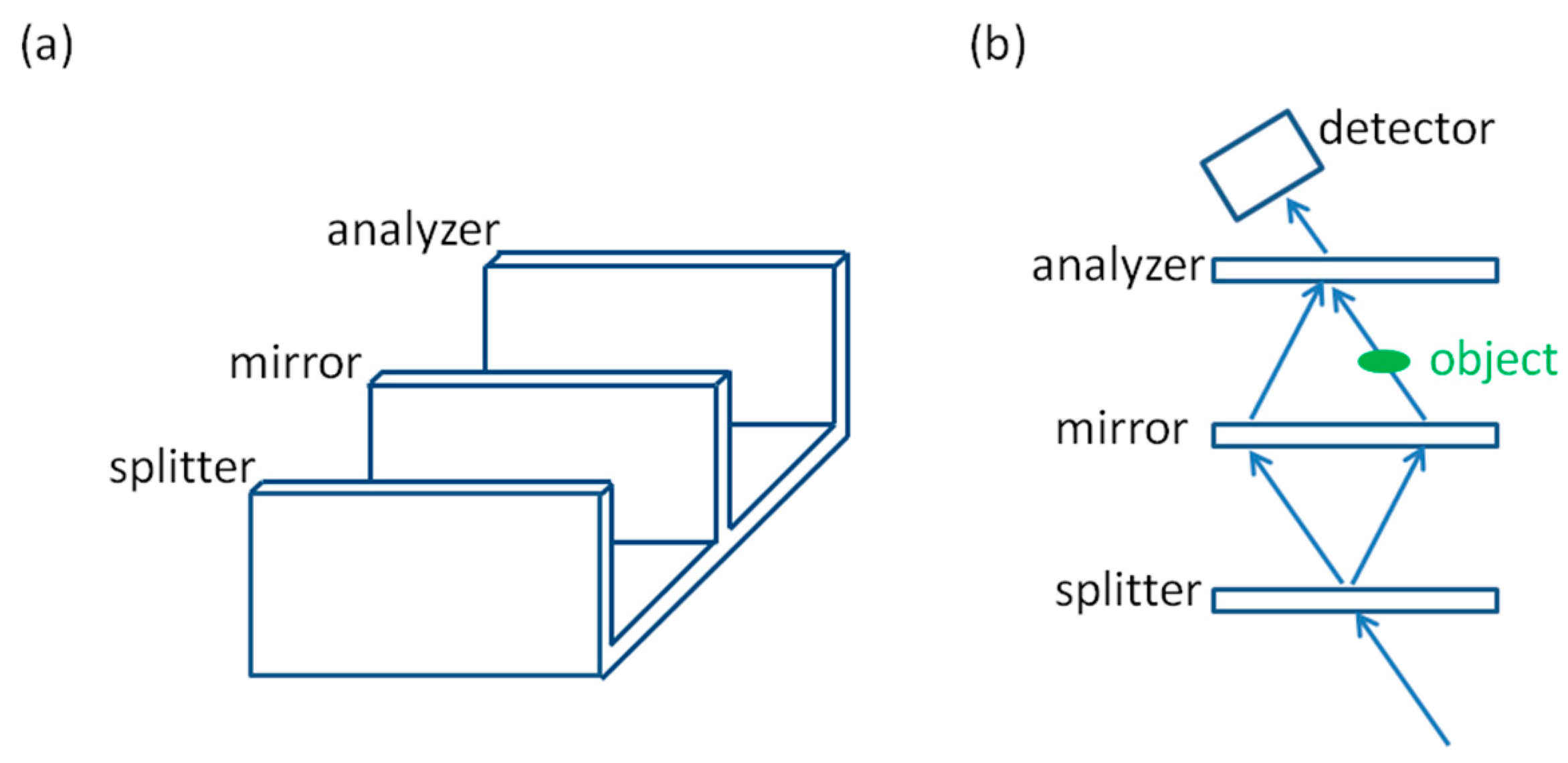
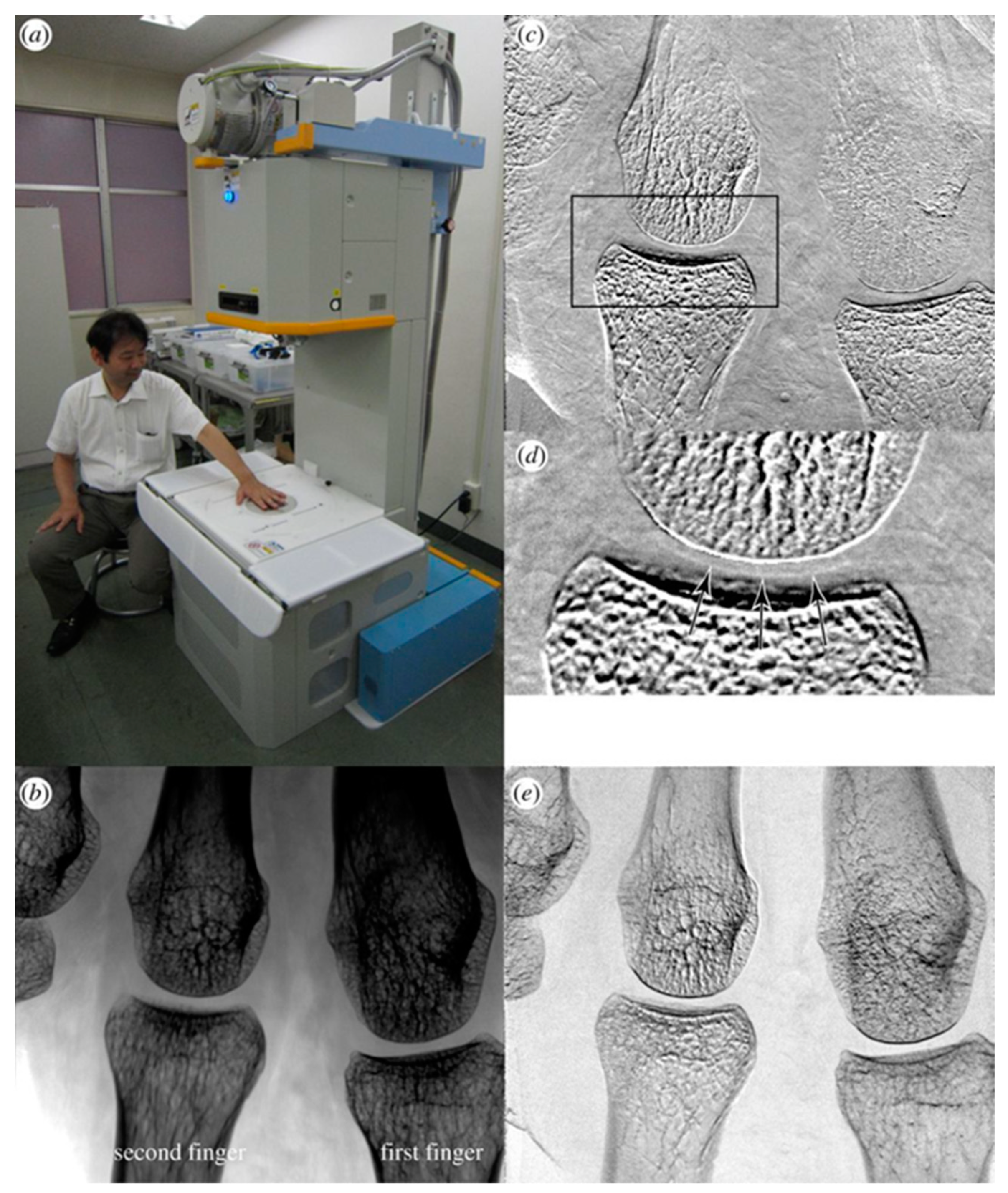
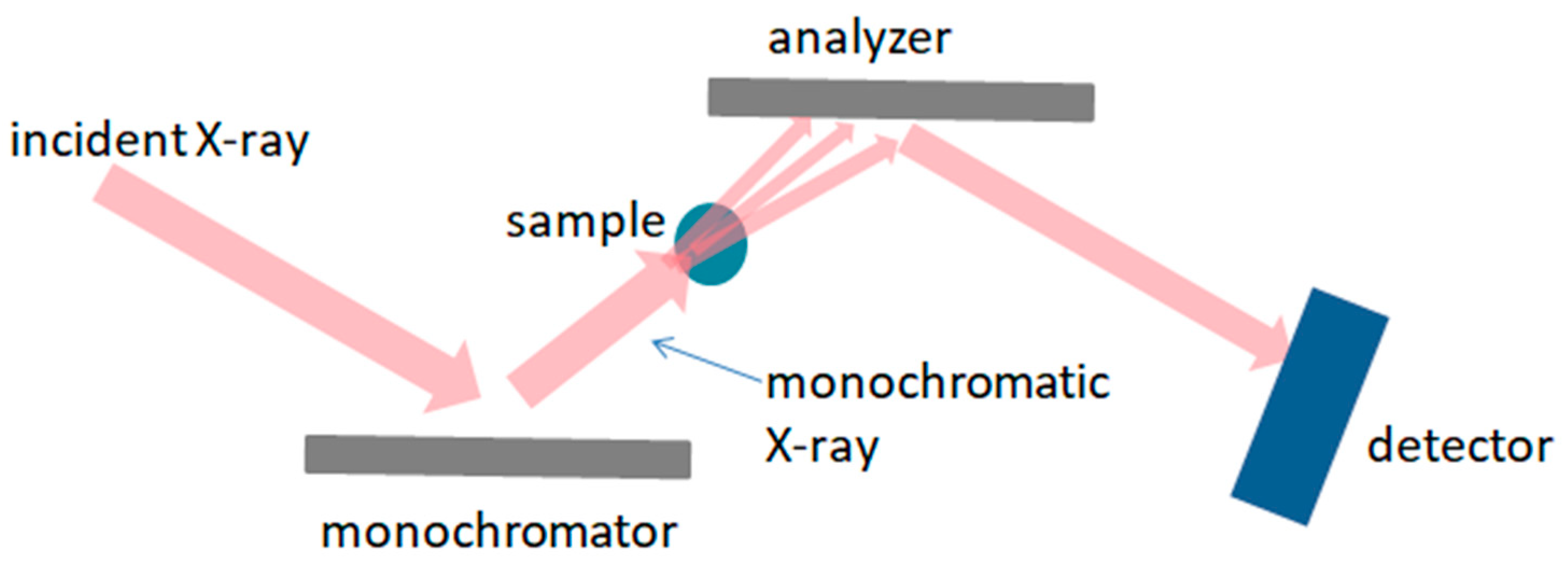
2.7. Diffraction-Enhanced Imaging (DEI)
2.8. Propagation-Based Imaging
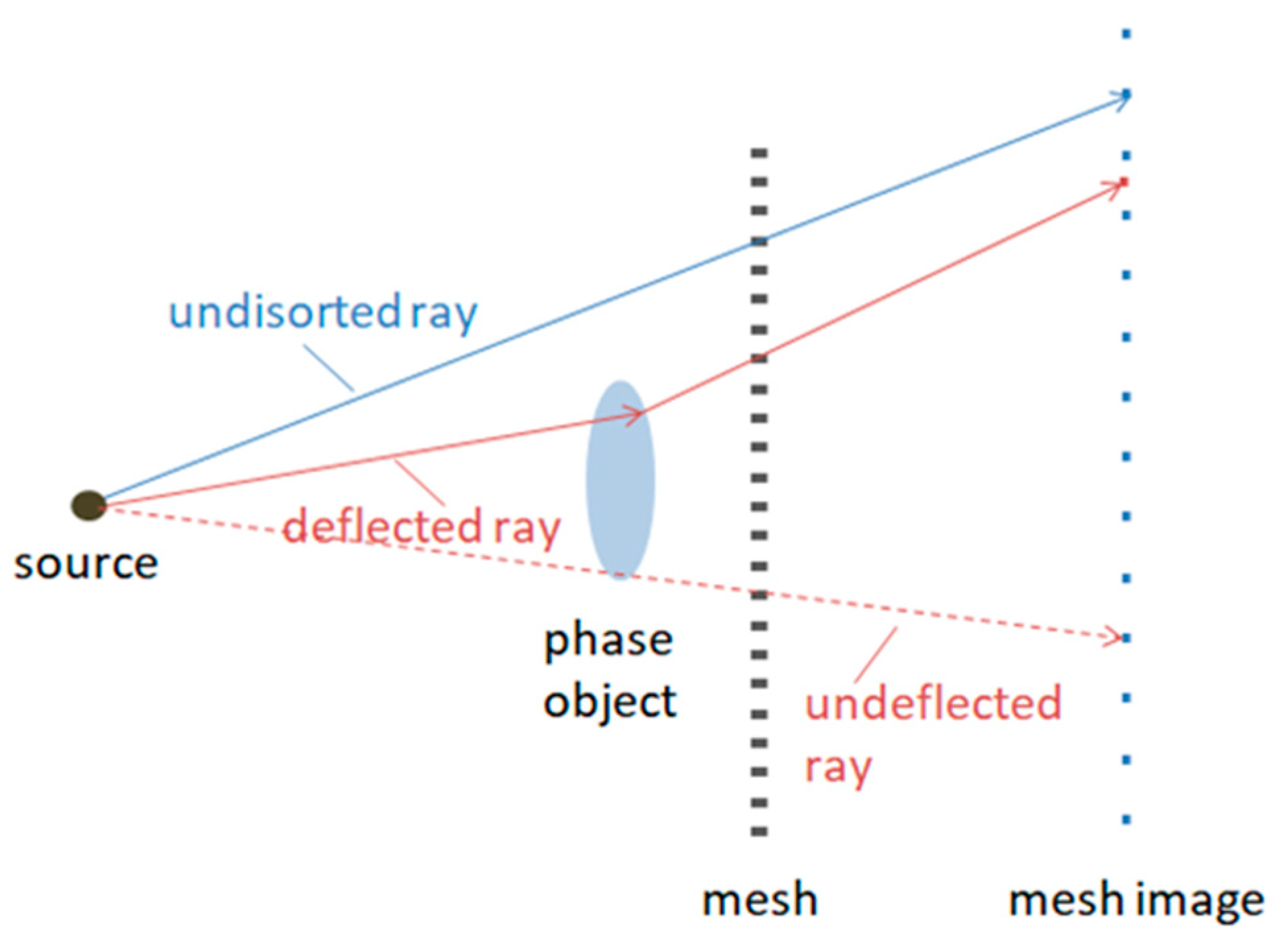
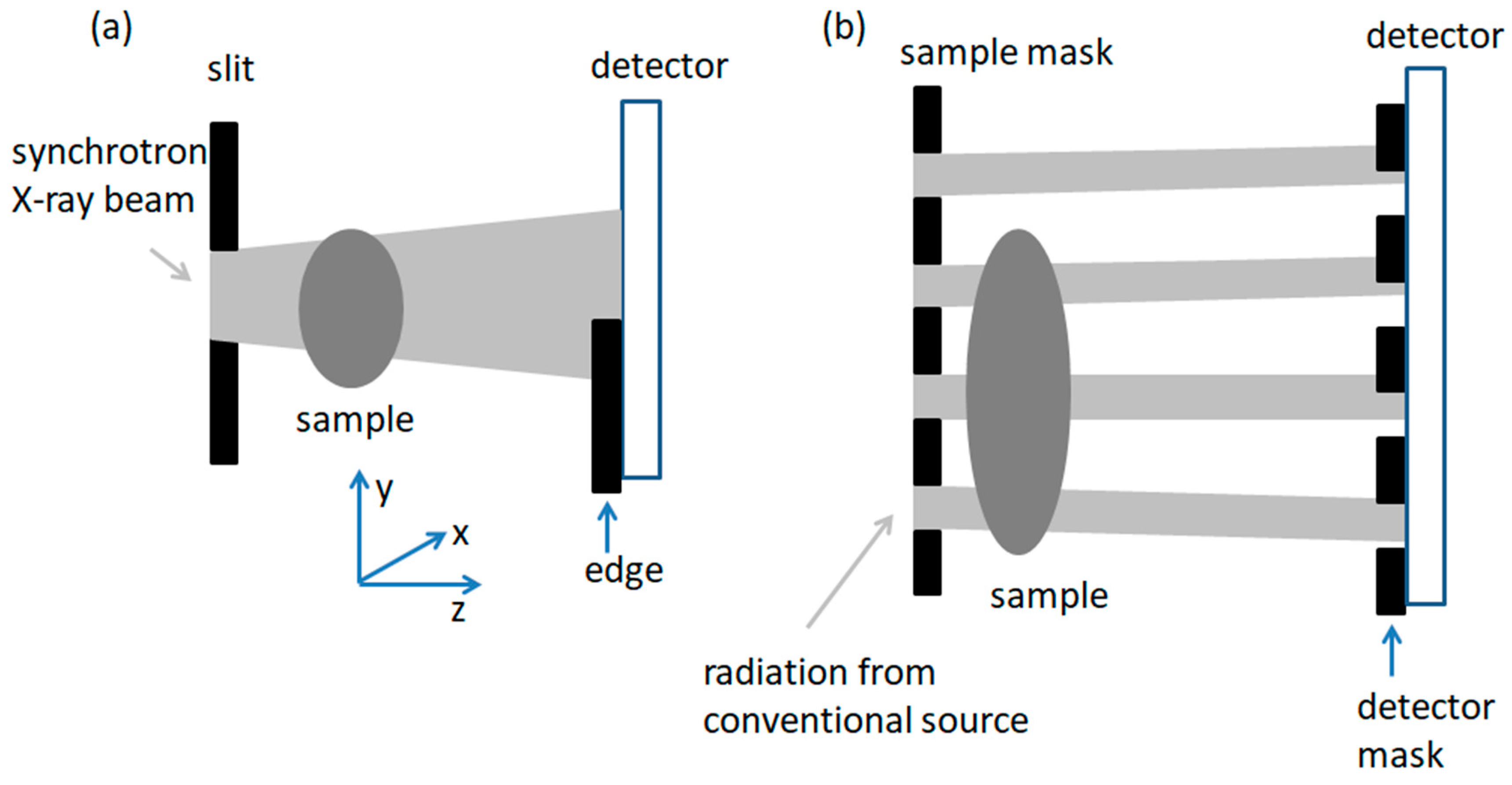
2.9. Edge Illumination (EI)
3. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Momose, A. Recent Advances in X-ray phase imaging. Jpn. J. Appl. Phys. 2005, 44, 6355–6367. [Google Scholar] [CrossRef]
- Momose, A. Development toward high-resolution X-ray phase imaging. QJM Int. J. Med. 2017, 66, 155–166. [Google Scholar] [CrossRef]
- Zernike, F. Phase contrast, a new method for the microscopic observation of transparent objects part II. Physica 1942, 9, 974–986. [Google Scholar] [CrossRef]
- Schmahl, G.; Rudolph, D.; Schneider, G.; Guttmann, P.; Niemann, B. Phase contrast X-ray microscopy studies. Optik 1994, 97, 181–182. [Google Scholar]
- Gabor, D. A new microscopic principle. Nat. Cell Biol. 1948, 161, 777–778. [Google Scholar] [CrossRef]
- Allen, R.D.; David, G.B.; Nomarski, G. The Zeiss-Nomarski differential interference equipment for transmitted-light microscopy. Z. Wiss. Mikrosk. Mikrosk. Tech. 1969, 69, 193–221. [Google Scholar]
- Wilhein, T.; Kaulich, B.; Di Fabrizio, E.; Romanato, F.; Cabrini, S.; Susini, J. Differential interference contrast X-ray microscopy with submicron resolution. Appl. Phys. Lett. 2001, 78, 2082–2084. [Google Scholar] [CrossRef]
- Sayre, D. Some implications of a theorem due to Shannon. Acta Crystallogr. 1952, 5, 843. [Google Scholar] [CrossRef]
- Bonse, U.; Hart, M. An X-ray interferometer. Appl. Phys. Lett. 1965, 6, 155–156. [Google Scholar] [CrossRef]
- Momose, A.; Kawamoto, S.; Koyama, I.; Hamaishi, Y.; Takai, K.; Suzuki, Y. Demonstration of X-Ray talbot interferometry. Jpn. J. Appl. Phys. 2003, 42, L866–L868. [Google Scholar] [CrossRef]
- Weitkamp, T.; Diaz, A.; David, C.; Pfeiffer, F.; Stampanoni, M.; Cloetens, P.; Ziegler, E. X-ray phase imaging with a grating interferometer. Opt. Express 2005, 13, 6296–6304. [Google Scholar] [CrossRef]
- Chapman, D.; Thomlinson, W.; E Johnston, R.; Washburn, D.; Pisano, E.; Gmür, N.; Zhong, Z.; Menk, R.; Arfelli, F.; Sayers, D. Diffraction enhanced X-ray imaging. Phys. Med. Biol. 1997, 42, 2015–2025. [Google Scholar] [CrossRef]
- Snigirev, A.; Snigireva, I.; Kohn, V.G.; Kuznetsov, S.; A Schelokov, I. On the possibilities of x-ray phase contrast microimaging by coherent high-energy synchrotron radiation. Rev. Sci. Instrum. 1995, 66, 5486–5492. [Google Scholar] [CrossRef]
- Zhou, S.-A.; Brahme, A. Development of phase-contrast X-ray imaging techniques and potential medical applications. Phys. Med. 2008, 24, 129–148. [Google Scholar] [CrossRef]
- Keyriläinen, J.; Bravin, A.; Fernandez, M.; Tenhunen, M.; Virkkunen, P.; Suortti, P. Phase-contrast X-ray imaging of breast. Acta Radiol. 2010, 51, 866–884. [Google Scholar] [CrossRef]
- Diemoz, P.C.; Bravin, A.; Coan, P. Theoretical comparison of three X-ray phase-contrast imaging techniques: Propagation-based imaging, analyzer-based imaging and grating interferometry. Opt. Express 2012, 20, 2789–2805. [Google Scholar] [CrossRef]
- Bravin, A.; Coan, P.; Suortti, P. X-ray phase-contrast imaging: From pre-clinical applications towards clinics. Phys. Med. Biol. 2012, 58, R1–R35. [Google Scholar] [CrossRef] [PubMed]
- Kagoshima, Y.; Yokoyama, Y.; Niimi, T.; Koyama, T.; Tsusaka, Y.; Matsui, J.; Takai, K. Hard X-ray phase-contrast microscope for observing transparent specimens. J. Phys. Colloq. 2003, 104, 49–52. [Google Scholar] [CrossRef]
- Tkachuk, A.; Duewer, F.; Cui, H.; Feser, M.; Wang, S.; Yun, W. X-ray computed tomography in Zernike phase contrast mode at 8 keV with 50-nm resolution using Cu rotating anode X-ray source. Z. Krist. Cryst. Mater. 2007, 222, 650–655. [Google Scholar] [CrossRef]
- Holzner, C.; Feser, M.; Vogt, S.; Hornberger, B.; Baines, S.B.; Jacobsen, C. Zernike phase contrast in scanning microscopy with X-rays. Nat. Phys. 2010, 6, 883–887. [Google Scholar] [CrossRef]
- Youn, H.S.; Jung, S.W. Hard X-ray microscopy with Zernike phase contrast. J. Microsc. 2006, 223, 53–56. [Google Scholar] [CrossRef] [PubMed]
- Vartiainen, I.; Mokso, R.; Stampanoni, M.; David, C. Halo suppression in full-field x-ray Zernike phase contrast microscopy. Opt. Lett. 2014, 39, 1601–1604. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.-T.; Chen, T.-Y.; Yi, J.; Chu, Y.S.; Lee, W.-K.; Wang, C.-L.; Kempson, I.M.; Hwu, Y.; Gajdosik, V.; Margaritondo, G. Hard X-ray Zernike microscopy reaches 30 nm resolution. Opt. Lett. 2011, 36, 1269–1271. [Google Scholar] [CrossRef]
- Bradley, R.S.; Robinson, I.K.; Yusuf, M. 3D X-Ray Nanotomography of cells grown on electrospun scaffolds. Macromol. Biosci. 2016, 17, 1600236. [Google Scholar] [CrossRef]
- Falch, K.V.; Casari, D.; Detlefs, C.; Snigireva, A.; Honkimäki, V.; Di Michiel, M.; Snigireva, I.; Mathiesen, R.H. In situ hard X-ray transmission microscopy for material science. J. Mater. Sci. 2017, 52, 3497–3507. [Google Scholar] [CrossRef]
- Su, Z.; De Andrade, V.; Cretu, S.; Yin, Y.; Wojcik, M.; Franco, A.; Demortiere, A. X-ray Nanocomputed tomography in Zernike phase contrast for studying 3D morphology of Li-O2 battery elec-trode. ACS Appl. Energy Mater. 2020. [Google Scholar] [CrossRef]
- Longo, E.; Sancey, L.; Flenner, S.; Kubec, A.; Bonnin, A.; David, C.; Muller, M.; Greving, I. X-ray Zernike phase contrast tomography: 3D ROI visualization of mm-sized mice organ tissues down to sub-cellular components. Biomed. Opt. Express 2020, 11, 5506–5517. [Google Scholar] [CrossRef]
- Park, J.Y.; Kim, Y.; Lee, S.; Lim, J. Zernike phase-contrast full-field transmission X-ray nanotomography for 400 micrometre-sized samples. J. Synchrotron Radiat. 2020, 27, 1696–1702. [Google Scholar] [CrossRef]
- Suzuki, Y. Two-beam X-ray interferometer using prism optics. Jpn. J. Appl. Phys. 2002, 41, L1019–L1021. [Google Scholar] [CrossRef]
- Chapman, H.N.; Nugent, K.A. Coherent lensless X-ray imaging. Nat. Photon. 2010, 4, 833–839. [Google Scholar] [CrossRef]
- Podorov, S.; Pavlov, K.M.; Paganin, D.M.J.O.E. A non-iterative reconstruction method for direct and unambiguous coherent dif-fractive imaging. Opt. Express 2007, 15, 9954–9962. [Google Scholar] [CrossRef]
- Guizar-Sicairos, M.; Fienup, J.R. Holography with extended reference by autocorrelation linear differential operation. Opt. Express 2007, 15, 17592–17612. [Google Scholar] [CrossRef] [PubMed]
- Gauthier, D.; Guizar-Sicairos, M.; Ge, X.; Boutu, W.; Carré, B.; Fienup, J.R.; Merdji, H. Single-shot femtosecond X-ray holography using extended references. Phys. Rev. Lett. 2010, 105, 093901. [Google Scholar] [CrossRef] [PubMed]
- Pfau, B.; Eisebitt, S. X-ray holography. Synchrotron light sources and free-electron. Lasers 2020, 5, 1295–1335. [Google Scholar]
- Zhu, D.; Guizar-Sicairos, M.; Wu, B.; Scherz, A.; Acremann, Y.; Tyliszczak, T.; Fischer, P.; Friedenberger, N.; Ollefs, K.J.; Farle, M.; et al. High-resolution X-ray lensless imaging by differential holographic encoding. Phys. Rev. Lett. 2010, 105, 043901. [Google Scholar] [CrossRef]
- Krenkel, M.; Bartels, M.; Salditt, T. Transport of intensity phase reconstruction to solve the twin image problem in holographic X-ray imaging. Opt. Express 2013, 21, 2220–2235. [Google Scholar] [CrossRef] [PubMed]
- Geilhufe, J.; Pfau, B.; Schneider, M.; Büttner, F.; Günther, C.M.; Werner, S.; Schaffert, S.; Guehrs, E.; Frömmel, S.; Kläui, M.; et al. Monolithic focused reference beam X-ray holography. Nat. Commun. 2014, 5, 3008. [Google Scholar] [CrossRef] [PubMed]
- Gorkhover, T.; Ulmer, A.; Ferguson, K.; Bucher, M.; Maia, F.R.N.C.; Bielecki, J.; Ekeberg, T.; Hantke, M.F.; Daurer, B.J.; Nettelblad, C.; et al. Femtosecond X-ray Fourier holography imaging of free-flying nanoparticles. Nat. Photon. 2018, 12, 150–153. [Google Scholar] [CrossRef]
- Vidas, L.; Günther, C.M.; Miller, T.A.; Pfau, B.; Perez-Salinas, D.; Martínez, E.; Schneider, M.; Guehrs, E.; Gargiani, P.; Valvidares, M.; et al. Imaging nanometer phase coexistence at defects during the insulator—Metal phase transformation in VO2 thin films by resonant soft X-ray holography. Nano Lett. 2018, 18, 3449–3453. [Google Scholar] [CrossRef]
- Burgos-Parra, E.; Bukin, N.; Sani, S.; Figueroa, A.I.; Beutier, G.; Dupraz, M.; Chung, S.; Dürrenfeld, P.; Le, Q.T.; Mohseni, S.M.; et al. Investigation of magnetic droplet solitons using x-ray holography with extended references. Sci. Rep. 2018, 8, 11533. [Google Scholar] [CrossRef]
- Blukis, R.; Pfau, B.; Günther, C.M.; Hessing, P.; Eisebitt, S.; Einsle, J.; Harrison, R.J. Nanoscale imaging of high-field magnetic hysteresis in meteoritic metal using X-Ray holography. Geochem. Geophys. Geosyst. 2020, 21, 009044. [Google Scholar] [CrossRef]
- Geilhufe, J.; Pfau, B.; Günther, C.M.; Schneider, M.; Eisebitt, S. Achieving diffraction-limited resolution in soft-X-ray Fourier-transform holography. Ultramicroscopy 2020, 214, 113005. [Google Scholar] [CrossRef] [PubMed]
- Turnbull, L.A.; Birch, M.T.; Laurenson, A.; Bukin, N.; Burgos-Parra, E.O.; Popescu, H.; Wilson, M.N.; Stefančič, A.; Balakrishnan, G.; Ogrin, F.Y.; et al. Tilted X-ray holography of magnetic bubbles in MnNiGa lamellae. ACS Nano 2021, 15, 387–395. [Google Scholar] [CrossRef]
- Tegze, M.; Faigel, G. X-ray holography with atomic resolution. Nat. Cell Biol. 1996, 380, 49–51. [Google Scholar] [CrossRef]
- Happo, N.; Hada, T.; Kubota, A.; Ebisu, Y.; Hosokawa, S.; Kimura, K.; Tajiri, H.; Matsushita, T.; Hayashi, K. Improvement of graphite crystal analyzer for light elements on X-ray fluorescence holography measurement. Jpn. J. Appl. Phys. 2018, 57, 058006. [Google Scholar] [CrossRef]
- Kudo, K.; Ioka, S.; Happo, N.; Ota, H.; Ebisu, Y.; Kimura, K.; Hada, T.; Kimuea, T.; Tajiri, H.; Hosokawa, S.; et al. Impact of local atomic fluctuations on superconductivity of Pr-substituted CaFe2As2 studied by X-ray fluores-cence holography. J. Phys. Soc. Jpn. 2019, 88, 063704. [Google Scholar] [CrossRef]
- Stellhorn, J.R.; Hosokawa, S.; Happo, N.; Tajiri, H.; Matsushita, T.; Kaminaga, K.; Fukumura, T.; Hasegawa, T.; Hayashi, K. A valence-selective X-ray fluorescence holography study of an yttrium oxide thin film. J. Appl. Crystallogr. 2017, 50, 1583–1589. [Google Scholar] [CrossRef]
- Hosokawa, S.; Stellhorn, J.R.; Matsushita, T.; Happo, N.; Kimura, K.; Hayashi, K.; Ebisu, Y.; Ozaki, T.; Ikemoto, H.; Setoyama, H.; et al. Impurity position and lattice distortion in a Mn-doped Bi2Te3 topological insulator investigated by X-ray fluorescence holography and X-ray absorption fine structure. Phys. Rev. B 2017, 96, 214207. [Google Scholar] [CrossRef]
- Hayashi, K.; Korecki, P.J.J.O. X-ray fluorescence holography: Principles, apparatus; applications. J. Phys. Soc. Jpn. 2018, 87, 061003. [Google Scholar] [CrossRef]
- Kaulich, B.; Wilhein, T.; Di Fabrizio, E.; Romanato, F.; Altissimo, M.; Cabrini, S.; Fayard, B.; Susini, J. Differential interference contrast X-ray microscopy with twin zone plates. J. Opt. Soc. Am. A 2002, 19, 797–806. [Google Scholar] [CrossRef] [PubMed]
- Di Fabrizio, E.; Kaulich, B.; Wilhein, T.; Susini, J. Differential interference contrast for X-ray microscopy: Fabrication and characterization of twin zone plate optics. Surf. Rev. Lett. 2002, 9, 243–248. [Google Scholar] [CrossRef]
- Vogt, U.; Lindblom, M.; Jansson, P.A.; Tuohimaa, T.T.; Holmberg, A.; Hertz, H.M.; Wieland, M.; Wilhein, T. Single-optical-element soft-X-ray interferometry with a laser-plasma X-ray source. Opt. Lett. 2005, 30, 2167–2169. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.; Sakdinawat, A.; Fischer, P.; Anderson, E.; Attwood, D. Single-element objective lens for soft X-ray differential interference contrast microscopy. Opt. Lett. 2006, 31, 1564–1566. [Google Scholar] [CrossRef] [PubMed]
- Bertilson, M.C.; Von Hofsten, O.; Lindblom, M.; Wilhein, T.; Hertz, H.M.; Vogt, U. Compact high-resolution differential interference contrast soft X-ray microscopy. Appl. Phys. Lett. 2008, 92, 064104. [Google Scholar] [CrossRef]
- Cui, X.; Lew, M.; Yang, C. Quantitative differential interference contrast microscopy based on structured-aperture interference. Appl. Phys. Lett. 2008, 93, 091113. [Google Scholar] [CrossRef]
- Xie, C.; Zhu, X.; Li, H.; Shi, L.; Hua, Y.; Liu, M. Toward two-dimensional nanometer resolution hard X-ray differential-interference-contrast imaging using modified photon sieves. Opt. Lett. 2012, 37, 749–751. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Xie, C.J.O. Differential-interference-contrast digital in-line holography microscopy based on a single-optical-element. Opt. Lett. 2015, 40, 5015–5018. [Google Scholar] [CrossRef]
- Matsuda, K.; Lopez, J.C.A.; Rehman, S.; Misawa, M.; Suzuki, Y.; Takeuchi, A.; Yasumoto, M.; Hibino, K.; Roy, M.; Hanayama, R.; et al. Differential interference contrast microscopy for cells using hard X-ray holography. Appl. Opt. 2018, 57, 4795–4801. [Google Scholar] [CrossRef] [PubMed]
- Miao, J.; Charalambous, P.; Kirz, J.; Sayre, D. Extending the methodology of X-ray crystallography to allow imaging of micrometre-sized non-crystalline speci-mens. Nature 1999, 400, 342–344. [Google Scholar] [CrossRef]
- Miao, J.; Sandberg, R.L.; Song, C. Coherent X-ray diffraction imaging. IEEE J. Sel. Top. Quantum Electron. 2012, 18, 399–410. [Google Scholar] [CrossRef]
- Gerchberg, R.W.; Saxton, W.O. A practical algorithm for the determination of phase from image and diffraction plane pictures. Optik 1972, 35, 237–246. [Google Scholar]
- Nam, D.; Park, J.; Gallagher-Jones, M.; Kim, S.; Kim, S.; Kohmura, Y.; Naitow, H.; Kunishima, N.; Yoshida, T.; Ishikawa, T.; et al. Imaging fully hydrated whole cells by coherent X-ray diffraction microscopy. Phys. Rev. Lett. 2013, 110, 098103. [Google Scholar] [CrossRef] [PubMed]
- Takahashi, Y.; Suzuki, A.; Zettsu, N.; Oroguchi, T.; Takayama, Y.; Sekiguchi, Y.; Kobayashi, A.; Yamamoto, M.; Nakasako, M. Coherent diffraction imaging analysis of shape-controlled nanoparticles with focused hard X-ray free-electron laser pulses. Nano Lett. 2013, 13, 6028–6032. [Google Scholar] [CrossRef]
- Kourousias, G.; Bozzini, B.; Gianoncelli, A.; Jones, M.W.M.; Junker, M.; Van Riessen, G.; Kiskinova, M. Shedding light on electrodeposition dynamics tracked in situ via soft X-ray coherent diffraction imaging. Nano Res. 2016, 9, 2046–2056. [Google Scholar] [CrossRef]
- Glownia, J.M.; Natan, A.; Cryan, J.P.; Hartsock, R.; Kozina, M.; Minitti, M.P.; Nelson, S.; Robinson, J.; Sato, T.; Van Driel, T.; et al. Self-referenced coherent diffraction X-ray movie of Ångstrom- and Femtosecond-scale atomic motion. Phys. Rev. Lett. 2016, 117, 153003. [Google Scholar] [CrossRef]
- Cha, W.; Ulvestad, A.; Allain, M.; Chamard, V.; Harder, R.; Leake, S.J.; Maser, J.; Fuoss, P.H.; Hruszkewycz, S.O. Three dimensional variable-wavelength X-ray bragg coherent diffraction imaging. Phys. Rev. Lett. 2016, 117, 225501. [Google Scholar] [CrossRef] [PubMed]
- Faulkner, H.M.L.; Rodenburg, J.M. Movable aperture lensless transmission microscopy: A novel phase retrieval algorithm. Phys. Rev. Lett. 2004, 93, 023903. [Google Scholar] [CrossRef]
- Rodenburg, J.M.; Faulkner, H.M.L. A phase retrieval algorithm for shifting illumination. Appl. Phys. Lett. 2004, 85, 4795–4797. [Google Scholar] [CrossRef]
- Pfeiffer, F. X-ray ptychography. Nat. Photon. 2017, 12, 9–17. [Google Scholar] [CrossRef]
- Shi, X.; Burdet, N.; Chen, B.; Xiong, G.; Streubel, R.; Harder, R.; Robinson, I.K. X-ray ptychography on low-dimensional hard-condensed matter materials. Appl. Phys. Rev. 2019, 6, 011306. [Google Scholar] [CrossRef]
- Hill, M.O.; Calvo-Almazan, I.; Allain, M.; Holt, M.V.; Ulvestad, A.; Treu, J.; Koblmueller, G.; Huang, C.; Huang, X.; Yan, H.; et al. Measuring three-dimensional strain and structural defects in a single InGaAs nanowire using coherent X-ray multiangle bragg projection ptychography. Nano Lett. 2018, 18, 811–819. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Zhu, X.; West, M.M.; Tyliszczak, T.; Shiu, H.-W.; Shapiro, D.; Berejnov, V.; Susac, D.; Stumper, J.; Hitchcock, A.P. High-resolution imaging of polymer electrolyte membrane fuel cell cathode layers by soft X-ray spectro-ptychography. J. Phys. Chem. C 2018, 122, 11709–11719. [Google Scholar] [CrossRef]
- Hirose, M.; Ishiguro, N.; Shimomura, K.; Nguyen, D.-N.; Matsui, H.; Dam, H.C.; Tada, M.; Takahashi, Y. Oxygen-diffusion-driven oxidation behavior and tracking areas visualized by X-ray spectro-ptychography with unsupervised learning. Commun. Chem. 2019, 2, 50. [Google Scholar] [CrossRef]
- Bossers, K.W.; Valadian, R.; Zanoni, S.; Smeets, R.; Friederichs, N.; Garrevoet, J.; Meirer, F.; Weckhuysen, B.M. Correlated X-ray ptychography and fluorescence nano-tomography on the fragmentation behavior of an individual catalyst particle during the early stages of olefin polymerization. J. Am. Chem. Soc. 2020, 142, 3691–3695. [Google Scholar] [CrossRef]
- Polo, C.C.; Pereira, L.; Mazzafera, P.; Flores-Borges, D.N.A.; Mayer, J.L.S.; Guizar-Sicairos, M.; Holler, M.; Barsi-Andreeta, M.; Westfahl, H.; Meneau, F. Correlations between lignin content and structural robustness in plants revealed by X-ray ptychography. Sci. Rep. 2020, 10, 6023. [Google Scholar] [CrossRef]
- Lo, Y.H.; Zhou, J.; Rana, A.; Morrill, D.; Gentry, C.; Enders, B.; Yu, Y.-S.; Sun, C.-Y.; Shapiro, D.A.; Falcone, R.W.; et al. X-ray linear dichroic ptychography. Proc. Natl. Acad. Sci. USA 2021, 118, 118. [Google Scholar] [CrossRef] [PubMed]
- Yao, Y.; Jiang, Y.; Klug, J.A.; Wojcik, M.; Maxey, E.R.; Sirica, N.S.; Roehrig, C.; Cai, Z.; Vogt, S.; Lai, B.; et al. Multi-beam X-ray ptychography for high-throughput coherent diffraction imaging. Sci. Rep. 2020, 10, 1–8. [Google Scholar] [CrossRef]
- Momose, A.; Fukuda, J. Phase-contrast radiographs of nonstained rat cerebellar specimen. Med. Phys. 1995, 22, 375–379. [Google Scholar] [CrossRef] [PubMed]
- Takeda, T.; Momose, A.; Wu, J.; Yu, Q.; Zeniya, T.; Lwin, T.-T.; Yoneyama, A.; Itai, Y. Vessel imaging by interferometric phase-contrast X-ray technique. Circulation 2002, 105, 1708–1712. [Google Scholar] [CrossRef] [PubMed]
- Yoneyama, A.; Takeda, T.; Tsuchiya, Y.; Wu, J.; Koizumi, A.; Hyodo, K.; Itai, Y. A phase-contrast X-ray imaging system—With a 60 × 30 mm field of view—Based on a skew-symmetric two-crystal X-ray interferometer. Nucl. Instrum. Methods Phys. Res. Sect. A 2004, 523, 217–222. [Google Scholar] [CrossRef]
- Takeda, T.; Yoneyama, A.; Wu, J.; Lwin, T.T.; Tsuchiya, Y.; Hyodo, K. In-vivo imaging of cancer implanted in nude mice by two-crystal interferometer-based phase-contrast X-ray computed tomography. Jpn. J. Appl. Phys. 2004, 43, L1144–L1146. [Google Scholar] [CrossRef]
- Takeda, T.; Yoneyama, A.; Wu, J.; Momose, A.; Hyodo, K. In vivo physiological saline-infused hepatic vessel imaging using a two-crystal-interferometer-based phase-contrast X-ray technique. J. Synchrotron Radiat. 2012, 19, 252–256. [Google Scholar] [CrossRef] [PubMed]
- Yoneyama, A.; Hyodo, K.; Takeda, T. Feasibility test of Zeff imaging using X-ray interferometry. Appl. Phys. Lett. 2013, 103, 204108. [Google Scholar] [CrossRef]
- Mkrtchyan, V.P.; Gasparyan, L.G.; Dadalyan, T.K.; Balyan, M.K.; Kuyumchyan, A.V. Measurement of the refractive index of nematic liquid crystals 5CB by means of X-ray interferometry. In Proceedings of the SPIE—The International Society for Optical Engineering, Bellingham, WA, USA, 1–7 August 2014; p. 920714. [Google Scholar]
- Lwin, T.-T.; Yoneyama, A.; Hara, A.; Ohbu, M.; Maruyama, H.; Taguchi, M.; Esashi, S.; Matsushima, T.; Terazaki, K.; Hyodo, K.; et al. Spontaneous brain tumor imaging of aged rat by crystal X-ray interferometer-based phase-contrast X-ray CT. Acta Radiol. Open 2016, 5, 2058460115626958. [Google Scholar] [CrossRef]
- Yoneyama, A.; Hyodo, K.; Baba, R.; Takeya, S.; Takeda, T. Feasibility study of phase-contrast X-ray laminography using X-ray interferometry. J. Synchrotron Radiat. 2018, 25, 1841–1846. [Google Scholar] [CrossRef]
- Momose, A. Phase-contrast X-ray imaging based on interferometry. J. Synchroton Radiat. 2002, 9, 136–142. [Google Scholar] [CrossRef]
- Lider, V.V. X-ray crystal interferometers. Phys. Uspekhi 2014, 57, 1099–1117. [Google Scholar] [CrossRef]
- Pfeiffer, F.; Herzen, J.; Willner, M.; Chabior, M.; Auweter, S.; Reiser, M.; Bamberg, F. Grating-based X-ray phase contrast for biomedical imaging applications. Z. Med. Phys. 2013, 23, 176–185. [Google Scholar] [CrossRef]
- Pfeiffer, F.; Weitkamp, T.; Bunk, O.; David, C. Phase retrieval and differential phase-contrast imaging with low-brilliance X-ray sources. Nat. Phys. 2006, 2, 258–261. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Kido, K.; Kiyohara, J.; Makifuchi, C.; Ito, T.; Nagatsuka, S.; Honda, C.; Noda, D.; Hattori, T.; et al. X-ray phase imaging: From synchrotron to hospital. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2014, 372, 20130023. [Google Scholar] [CrossRef][Green Version]
- Itoh, H.; Nagai, K.; Sato, G.; Yamaguchi, K.; Nakamura, T.; Kondoh, T.; Ouchi, C.; Teshima, T.; Setomoto, Y.; Den, T. Two-dimensional grating-based X-ray phase-contrast imaging using Fourier transform phase retrieval. Opt. Express 2011, 19, 3339–3346. [Google Scholar] [CrossRef]
- Ge, Y.; Li, K.; Garrett, J.; Chen, G.-H. Grating based X-ray differential phase contrast imaging without mechanical phase stepping. Opt. Express 2014, 22, 14246–14252. [Google Scholar] [CrossRef]
- Birnbacher, L.; Willner, M.; Velroyen, A.; Marschner, M.; Hipp, A.; Meiser, J.; Koch, F.; Schröter, T.; Kunka, D.; Mohr, J.; et al. Experimental realisation of high-sensitivity laboratory X-ray grating-based phase-contrast computed tomography. Sci. Rep. 2016, 6, 1–8. [Google Scholar] [CrossRef]
- Yashiro, W.; Noda, D.; Kajiwara, K. Sub-10-ms X-ray tomography using a grating interferometer. Appl. Phys. Express 2017, 10, 52501. [Google Scholar] [CrossRef]
- Gradl, R.; Morgan, K.S.; Dierolf, M.; Jud, C.; Hehn, L.; Gunther, B.; Moller, W.; Kutschke, D.; Yang, L.; Stoeger, T.; et al. Dynamic in vivo chest X-ray dark-field imaging in mice. IEEE Trans. Med. Imaging 2018, 38, 649–656. [Google Scholar] [CrossRef] [PubMed]
- Arboleda, C.; Wang, Z.; Jefimovs, K.; Koehler, T.; Van Stevendaal, U.; Kuhn, N.; David, B.; Prevrhal, S.; Lång, K.; Forte, S.; et al. Towards clinical grating-interferometry mammography. Eur. Radiol. 2020, 30, 1419–1425. [Google Scholar] [CrossRef]
- Wen, H.H.; Bennett, E.E.; Kopace, R.; Stein, A.F.; Pai, V. Single-shot X-ray differential phase-contrast and diffraction imaging using two-dimensional transmission gratings. Opt. Lett. 2010, 35, 1932–1934. [Google Scholar] [CrossRef] [PubMed]
- Tahir, S.; Bashir, S.; Macdonald, C.; Petruccelli, J.C. Mesh-based phase contrast Fourier transform imaging. Opt. Commun. 2017, 389, 103–109. [Google Scholar] [CrossRef]
- Morgan, K.S.; Paganin, D.M.; Siu, K.K.W. X-ray phase imaging with a paper analyzer. Appl. Phys. Lett. 2012, 100, 124102. [Google Scholar] [CrossRef]
- Pisano, E.D.; Johnston, R.E.; Chapman, D.; Geradts, J.; Iacocca, M.V.; Livasy, C.A.; Washburn, D.B.; Sayers, D.E.; Zhong, Z.; Kiss, M.Z.; et al. Human breast cancer specimens: Diffraction-enhanced imaging with histologic correlation—Improved con-spicuity of lesion detail compared with digital radiography. Radiology 2000, 214, 895–901. [Google Scholar] [CrossRef] [PubMed]
- Dilmanian, A.F.; Zhong, Z.; Ren, B.; Wu, X.Y.; Chapman, L.D.; Orion, I.; Thomlinson, W.C. Computed tomography of X-ray index of refraction using the diffraction enhanced imaging method. Phys. Med. Biol. 2000, 45, 933–946. [Google Scholar] [CrossRef]
- Mollenhauer, J.; Aurich, M.; Zhong, Z.; Muehleman, C.; Cole, A.; Hasnah, M.; Oltulu, O.; Kuettner, K.; Margulis, A.; Chapman, L. Diffraction-enhanced X-ray imaging of articular cartilage. Osteoarthr. Cartil. 2002, 10, 163–171. [Google Scholar] [CrossRef]
- Oltulu, O.; Zhong, Z.; Hasnah, M.; Wernick, M.N.; Chapman, D. Extraction of extinction, refraction and absorption properties in diffraction enhanced imaging. J. Phys. D Appl. Phys. 2003, 36, 2152–2156. [Google Scholar] [CrossRef]
- Kelly, M.E.; Coupal, D.J.; Beavis, R.C.; Schultke, E.; Romanchuk, K.; Juurlink, B.H.J.; Zhong, Z.; Chapman, L.D. Diffraction-enhanced imaging of a porcine eye. Can. J. Ophthalmol. 2007, 42, 731–733. [Google Scholar] [CrossRef] [PubMed]
- Kao, T.; Connor, D.; A Dilmanian, F.; Faulconer, L.; Liu, T.; Parham, C.; Pisano, E.D.; Zhong, Z. Characterization of diffraction-enhanced imaging contrast in breast cancer. Phys. Med. Biol. 2009, 54, 3247–3256. [Google Scholar] [CrossRef]
- Muehleman, C.; Li, J.; Connor, D.; Parham, C.; Pisano, E.; Zhong, Z. Diffraction-enhanced imaging of musculoskeletal tissues using a conventional X-ray tube. Acad. Radiol. 2009, 16, 918–923. [Google Scholar] [CrossRef]
- Faulconer, L.; Parham, C.; Connor, D.M.; Zhong, Z.; Kim, E.; Zeng, D.; Livasy, C.; Cole, E.; Kuzmiak, C.; Koomen, M.; et al. Radiologist evaluation of an X-ray tube-based diffraction-enhanced imaging prototype using full-thickness breast specimens. Acad. Radiol. 2009, 16, 1329–1337. [Google Scholar] [CrossRef]
- Nesch, I.; Fogarty, D.P.; Tzvetkov, T.; Reinhart, B.; Walus, A.C.; Khelashvili, G.; Muehleman, C.; Chapman, D. The design and application of an in-laboratory diffraction-enhanced X-ray imaging instrument. Rev. Sci. Instrum. 2009, 80, 93702. [Google Scholar] [CrossRef]
- Parham, C.; Zhong, Z.; Connor, D.M.; Chapman, L.D.; Pisano, E.D. Design and implementation of a compact low-dose diffraction enhanced medical imaging system. Acad. Radiol. 2009, 16, 911–917. [Google Scholar] [CrossRef]
- Zhou, W.; Majidi, K.; Brankov, J.G. Analyzer-based phase-contrast imaging system using a micro focus X-ray source. Rev. Sci. Instrum. 2014, 85, 85114. [Google Scholar] [CrossRef] [PubMed]
- Ando, M.; Nakao, Y.; Jin, G.; Sugiyama, H.; Sunaguchi, N.; Sung, Y.; Suzuki, Y.; Sun, Y.; Tanimoto, M.; Kawashima, K.; et al. Improving contrast and spatial resolution in crystal analyzer-based x-ray dark-field imaging: Theoretical con-siderations and experimental demonstration. Med. Phys. 2020, 47, 5505–5513. [Google Scholar] [CrossRef]
- Li, J.; Zhong, Z.; Lidtke, R.; Kuettner, K.E.; Peterfy, C.; Aliyeva, E.; Muehleman, C. Radiography of soft tissue of the foot and ankle with diffraction enhanced imaging. J. Anat. 2003, 202, 463–470. [Google Scholar] [CrossRef]
- Majumdar, S.; Issever, A.S.; Burghardt, A.; Lotz, J.; Arfelli, F.; Rigon, L.; Heitner, G.; Menk, R.-H. Diffraction enhanced imaging of articular cartilage and comparison with micro-computed tomography of the underlying bone structure. Eur. Radiol. 2004, 14, 1440–1448. [Google Scholar] [CrossRef]
- Fernández, M.; Keyriläinen, J.; Serimaa, R.; Torkkeli, M.; Karjalainen-Lindsberg, M.L.; Leidenius, M.; von Smitten, K.; Tenhunen, M.; Fiedler, S.; Bravin, A.; et al. Human breast cancer in vitro: Matching histo-pathology with small-angle X-ray scattering and diffraction enhanced X-ray imaging. Phys. Med. Biol. 2005, 50, 2991. [Google Scholar] [CrossRef] [PubMed]
- Antunes, A.; Honnicke, M.; Safatle, A.; Cusatis, C.; Barros, P.M.; Morelhao, S.L. Diffraction enhanced X-ray imaging of mammals crystalline lens. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2005, 238, 28–31. [Google Scholar] [CrossRef]
- Yin, H.; Gao, X.; Luo, S.; Liu, B.; Gao, X.; Zhu, P.; Shu, H. Diffraction enhanced X-ray imaging for observing guinea pig cochlea. In Proceedings of the 2005 IEEE Engineering in Medicine and Biology 27th Annual Conference; Orlando, FL, USA, 31 October–3 November 2005, Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2005; Volume 6, pp. 5699–5701. [Google Scholar]
- Bravin, A.; Keyriläinen, J.; Fernandez, M.; Fiedler, S.; Nemoz, C.; Karjalainen-Lindsberg, M.-L.; Tenhunen, M.; Virkkunen, P.; Leidenius, M.; Von Smitten, K.; et al. High-resolution CT by diffraction-enhanced X-ray imaging: Mapping of breast tissue samples and comparison with their histo-pathology. Phys. Med. Biol. 2007, 52, 2197–2211. [Google Scholar] [CrossRef]
- Fogarty, D.P.; Reinhart, B.; Tzvetkov, T.; Nesch, I.; Williams, C. In-laboratory diffraction-enhanced X-ray imaging of an equine hoof. J. Equine Veter. Sci. 2011, 31, 365–369. [Google Scholar] [CrossRef]
- Cooper, D.M.; Bewer, B.; Wiebe, S.; Wysokinski, T.W.; Chapman, D. Diffraction enhanced X-ray imaging of the distal radius: A novel approach for visualization of trabecular bone architecture. Can. Assoc. Radiol. J. 2011, 62, 251–255. [Google Scholar] [CrossRef]
- Mizuno, K.; Kobayashi, T.; Fujiki, F.; Okamoto, H.; Furuya, Y.; Hirano, K. Visualization of hydrides in titanium by means of diffraction-enhanced X-ray imaging. J. Alloy. Compd. 2005, 402, 109–114. [Google Scholar] [CrossRef]
- Mizuno, K.; Furuya, Y.; Hirano, K.; Okamoto, H. Hydrogen diffusion in titanium-hydride observed by the diffraction-enhanced X-ray imaging method. Phys. Status Solidi 2007, 204, 2734–2739. [Google Scholar] [CrossRef]
- Lang, S.; Zanette, I.; Dominietto, M.; Langer, M.; Rack, A.; Schulz, G.; Le Duc, G.; David, C.; Mohr, J.; Pfeiffer, F.; et al. Experimental comparison of grating- and propagation-based hard X-ray phase tomography of soft tissue. J. Appl. Phys. 2014, 116, 154903. [Google Scholar] [CrossRef]
- Preissner, M.; Murrie, R.P.; Pinar, I.; Werdiger, F.; Carnibella, R.P.; Zosky, G.R.; Fouras, A.; Dubsky, S. High resolution propagation-based imaging system for in vivo dynamic computed tomography of lungs in small animals. Phys. Med. Biol. 2018, 63, 08NT03. [Google Scholar] [CrossRef] [PubMed]
- Kalasová, D.; Zikmund, T.; Pína, L.; Takeda, Y.; Horváth, M.; Omote, K.; Kaiser, J. Characterization of a laboratory-based X-ray computed nanotomography system for propagation-based meth-od of phase contrast imaging. IEEE Proc. 2019, 69, 1170–1178. [Google Scholar]
- Longo, R.; Arfelli, F.; Bonazza, D.; Bottigli, U.; Brombal, L.; Contillo, A.; Cova, M.A.; Delogu, P.; Di Lillo, F.; Di Trapani, V.; et al. Advancements towards the implementation of clinical phase-contrast breast computed tomography at Elettra. J. Synchrotron Radiat. 2019, 26, 1343–1353. [Google Scholar] [CrossRef] [PubMed]
- Bidola, P.; Morgan, K.; Willner, M.; Fehringer, A.; Allner, S.; Prade, F.; Pfeiffer, F.; Achterhold, K. Application of sensitive, high-resolution imaging at a commercial lab-based X-ray micro-CT system using propagation-based phase retrieval. J. Microsc. 2017, 266, 211–220. [Google Scholar] [CrossRef]
- Baran, P.; Pacile, S.; Nesterets, I.Y.; Mayo, S.C.; Dullin, C.; Dreossi, D.; Arfelli, F.; Thompson, D.; Lockie, D.; McCormack, M.; et al. Optimization of propagation-based X-ray phase-contrast tomography for breast cancer imaging. Phys. Med. Biol. 2017, 62, 2315–2332. [Google Scholar] [CrossRef]
- Carrel, M.; Beltran, M.A.; Morales, V.L.; Derlon, N.; Morgenroth, E.; Kaufmann, R.; Holzner, M. Biofilm imaging in porous media by laboratory X-ray tomography: Combining a non-destructive contrast agent with propagation-based phase-contrast imaging tools. PLoS ONE 2017, 12, e0180374. [Google Scholar] [CrossRef] [PubMed]
- Töpperwien, M.; Gradl, R.; Keppeler, D.; Vassholz, M.; Meyer, A.; Hessler, R.; Achterhold, K.; Gleich, B.; Dierolf, M.; Pfeiffer, F.; et al. Propagation-based phase-contrast X-ray tomography of cochlea using a compact synchrotron source. Sci. Rep. 2018, 8, 1–12. [Google Scholar] [CrossRef]
- Krenkel, M.; Töpperwien, M.; Dullin, C.; Alves, F.; Salditt, T. Propagation-based phase-contrast tomography for high-resolution lung imaging with laboratory sources. AIP Adv. 2016, 6, 035007. [Google Scholar] [CrossRef]
- Olivo, A.; Arfelli, F.; Cantatore, G.; Longo, R.; Menk, R.H.; Pani, S.; Prest, M.; Poropat, P.; Rigon, L.; Tromba, G.; et al. An innovative digital imaging set-up allowing a low-dose approach to phase contrast applications in the medi-cal field. Med. Phys. 2001, 28, 1610–1619. [Google Scholar] [CrossRef]
- Olivo, A.; Diemoz, P.C.; Bravin, A. Amplification of the phase contrast signal at very high X-ray energies. Opt. Lett. 2012, 37, 915–917. [Google Scholar] [CrossRef]
- Diemoz, P.C.; Endrizzi, M.; Zapata, C.E.; Pešić, Z.D.; Rau, C.; Bravin, A.; Robinson, I.K.; Olivo, A. X-ray phase-contrast imaging with nanoradian angular resolution. Phys. Rev. Lett. 2013, 110, 138105. [Google Scholar] [CrossRef]
- Diemoz, P.C.; Hagen, C.K.; Endrizzi, M.; Olivo, A. Sensitivity of laboratory based implementations of edge illumination X-ray phase-contrast imaging. Appl. Phys. Lett. 2013, 103, 244104. [Google Scholar] [CrossRef]
- Diemoz, P.C.; Vittoria, F.A.; Olivo, A. Spatial resolution of edge illumination X-ray phase-contrast imaging. Opt. Express 2014, 22, 15514–15529. [Google Scholar] [CrossRef] [PubMed]
- Zamir, A.; Hagen, C.; Diemoz, P.C.; Endrizzi, M.; Vittoria, F.; Chen, Y.; Anastasio, M.A.; Olivo, A. Recent advances in edge illumination X-ray phase-contrast tomography. J. Med. Imaging 2017, 4, 040901. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Olivo, A.; Speller, R. A coded-aperture technique allowing x-ray phase contrast imaging with conventional sources. Appl. Phys. Lett. 2007, 91, 074106. [Google Scholar] [CrossRef]
- Marenzana, M.; Hagen, C.K.; Borges, P.D.N.; Endrizzi, M.; Szafraniec, M.B.; Ignatyev, K.; Olivo, A. Visualization of small lesions in rat cartilage by means of laboratory-based X-ray phase contrast imaging. Phys. Med. Biol. 2012, 57, 8173–8184. [Google Scholar] [CrossRef]
- Hagen, C.K.; Diemoz, P.C.; Endrizzi, M.; Rigon, L.; Dreossi, D.; Arfelli, F.; Lopez, F.C.M.; Longo, R.; Olivo, A. Theory and preliminary experimental verification of quantitative edge illumination X-ray phase contrast tomog-raphy. Opt. Express 2014, 22, 7989–8000. [Google Scholar] [CrossRef]
- Modregger, P.; Cremona, T.P.; Benarafa, C.; Schittny, J.C.; Olivo, A.; Endrizzi, M. Small angle X-ray scattering with edge-illumination. Sci. Rep. 2016, 6, 30940. [Google Scholar] [CrossRef]
- Havariyoun, G.; Vittoria, A.F.; Hagen, C.K.; Basta, D.; Kallon, G.K.; Endrizzi, M.; Massimi, L.; Munro, P.R.T.; Hawker, S.; Smit, B.; et al. A compact system for intraoperative specimen imaging based on edge illumination X-ray phase contrast. Phys. Med. Biol. 2019, 64, 235005. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, S.; He, C.; Hao, X.; Kuang, C.; Liu, X. Principles of Different X-ray Phase-Contrast Imaging: A Review. Appl. Sci. 2021, 11, 2971. https://doi.org/10.3390/app11072971
Tao S, He C, Hao X, Kuang C, Liu X. Principles of Different X-ray Phase-Contrast Imaging: A Review. Applied Sciences. 2021; 11(7):2971. https://doi.org/10.3390/app11072971
Chicago/Turabian StyleTao, Siwei, Congxiao He, Xiang Hao, Cuifang Kuang, and Xu Liu. 2021. "Principles of Different X-ray Phase-Contrast Imaging: A Review" Applied Sciences 11, no. 7: 2971. https://doi.org/10.3390/app11072971
APA StyleTao, S., He, C., Hao, X., Kuang, C., & Liu, X. (2021). Principles of Different X-ray Phase-Contrast Imaging: A Review. Applied Sciences, 11(7), 2971. https://doi.org/10.3390/app11072971



