Efficiency of Machine Sanding of Wood
Abstract
1. Introduction
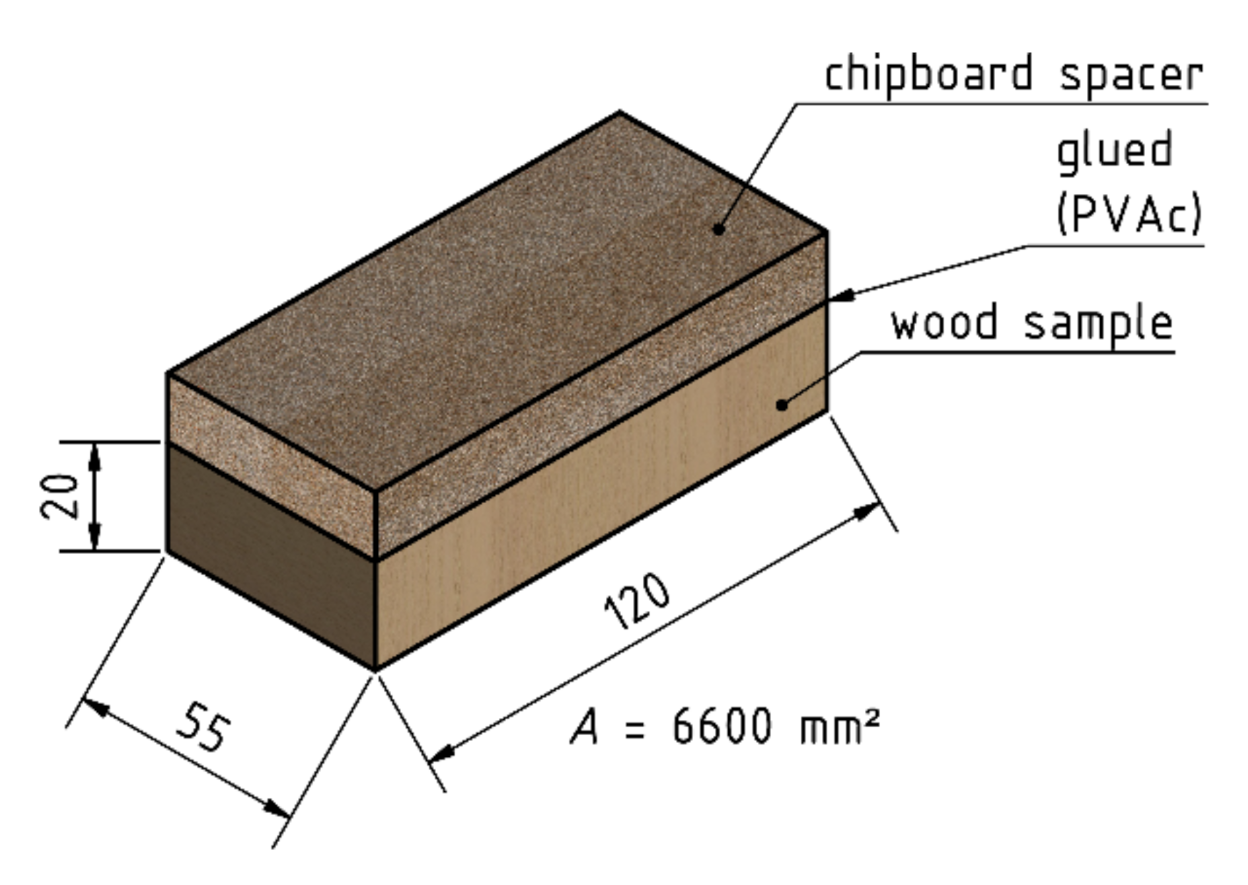
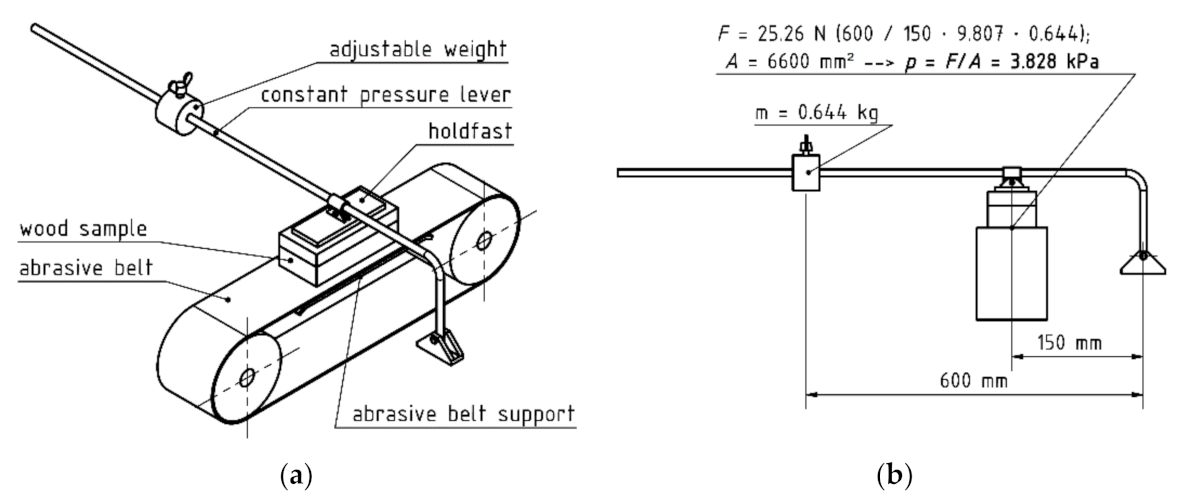
2. Materials and Methods
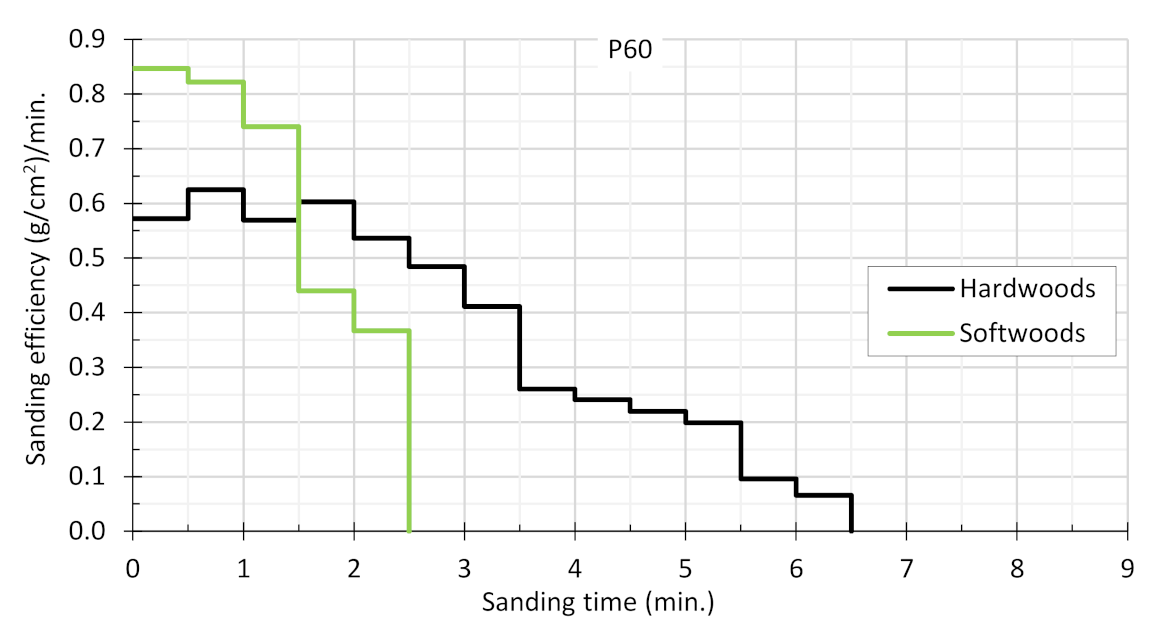
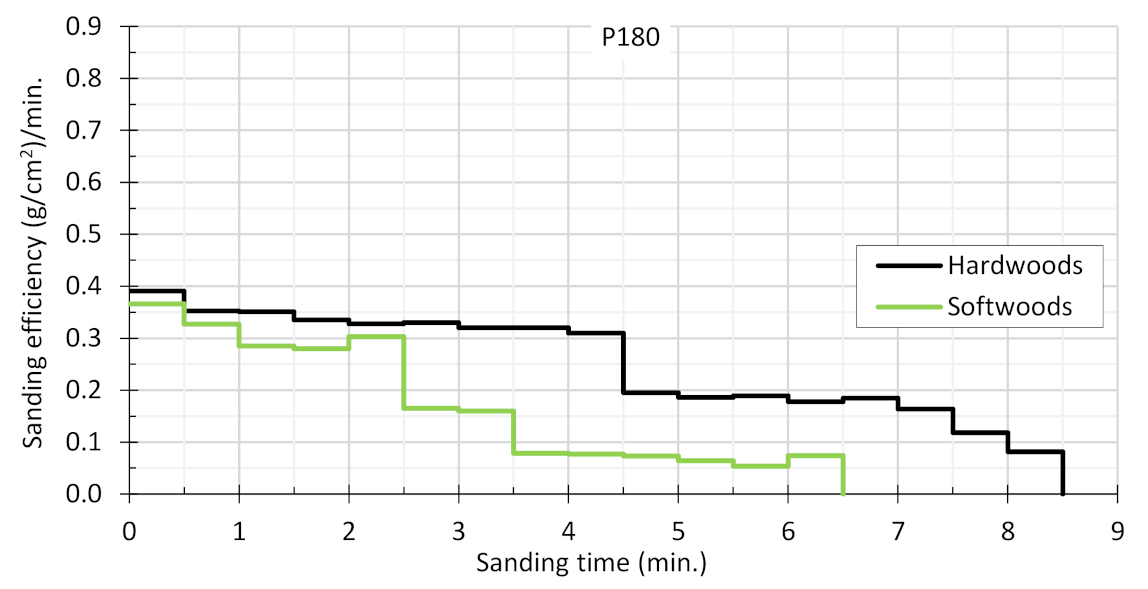
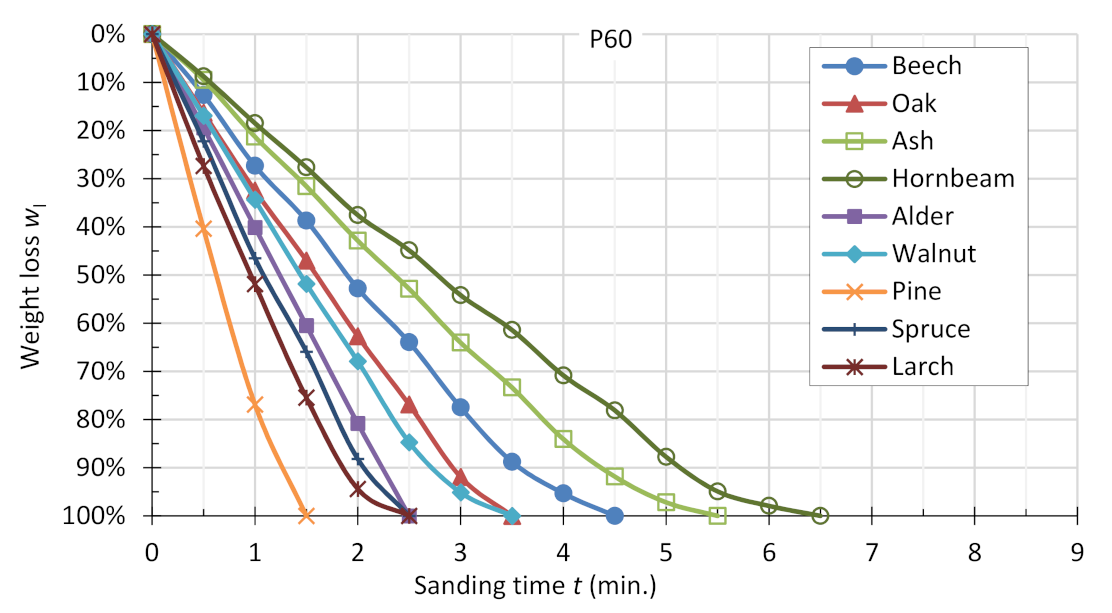
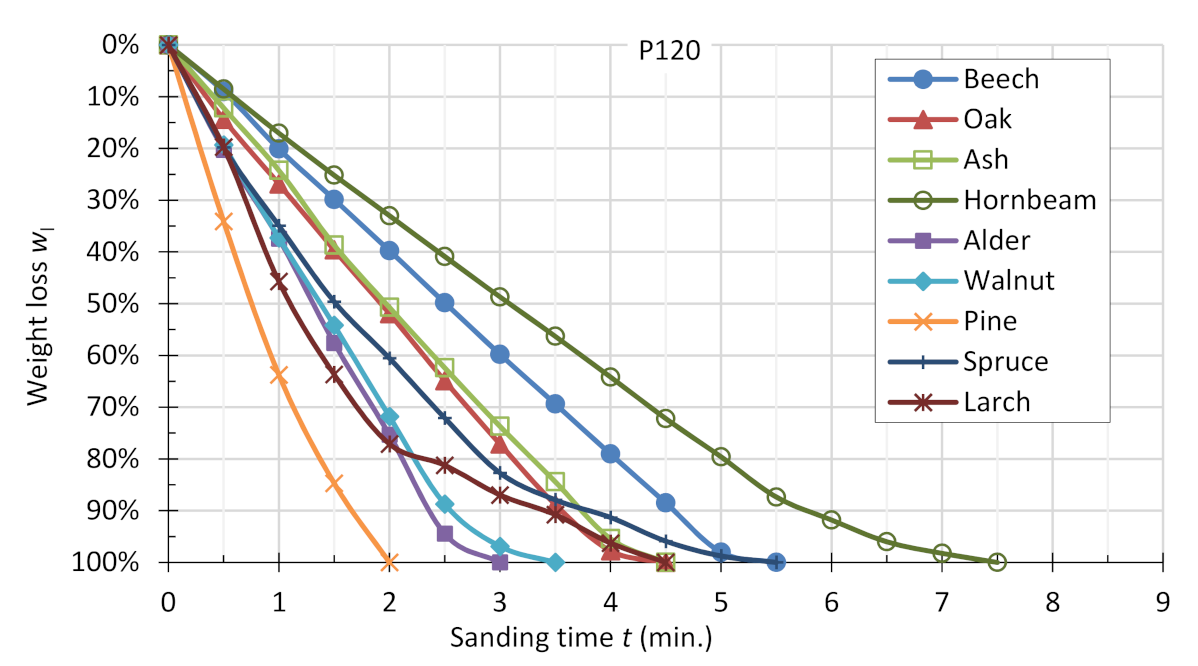
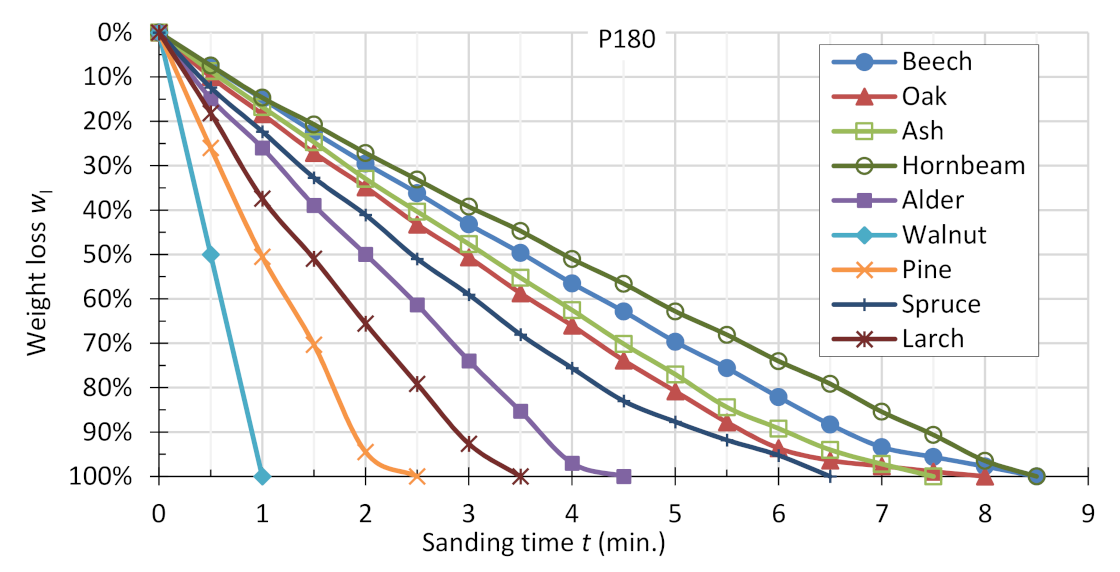
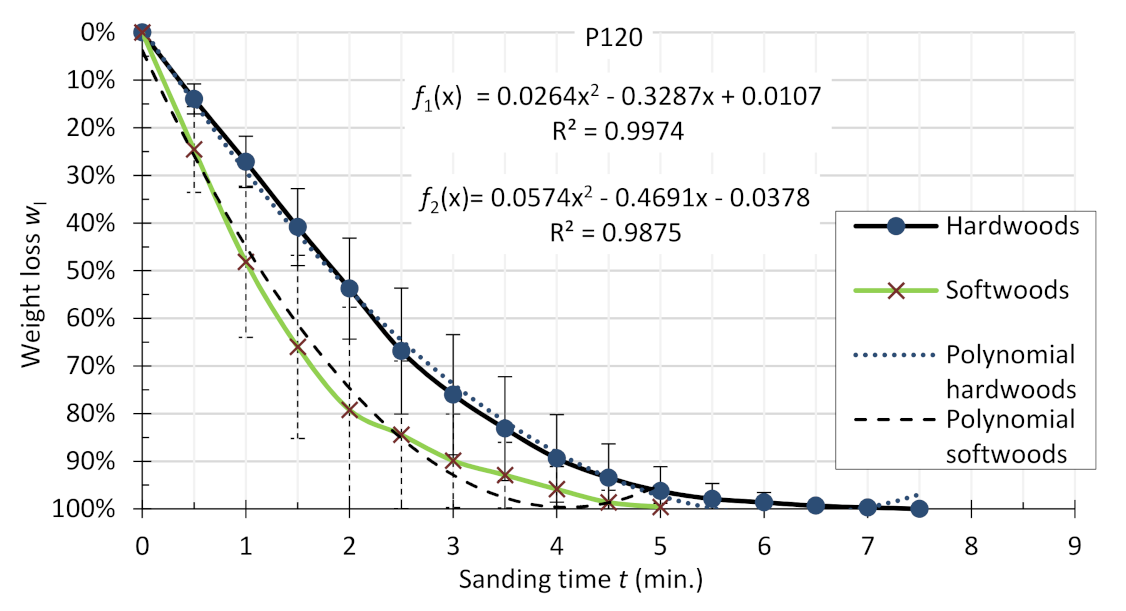
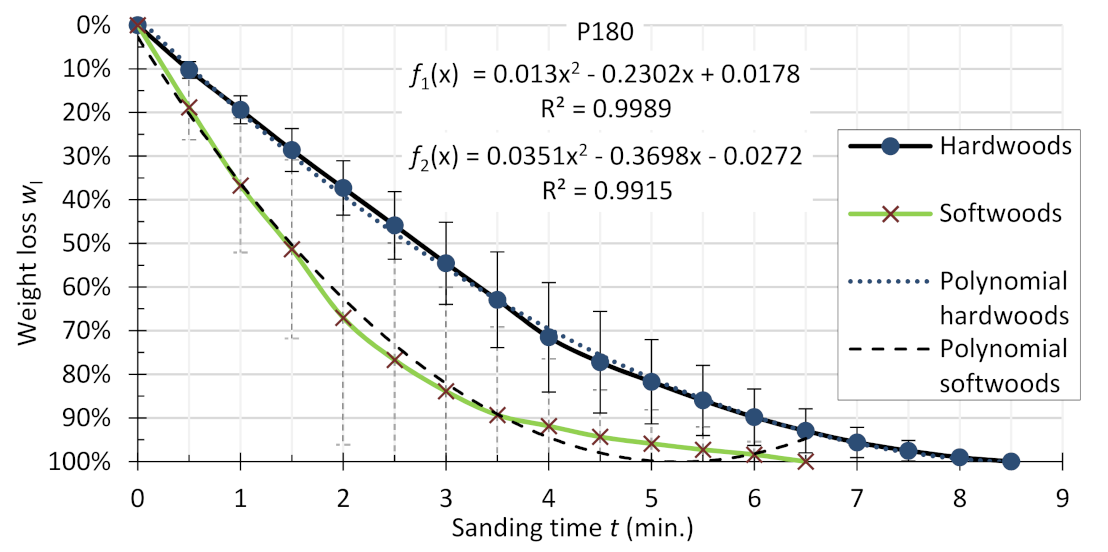
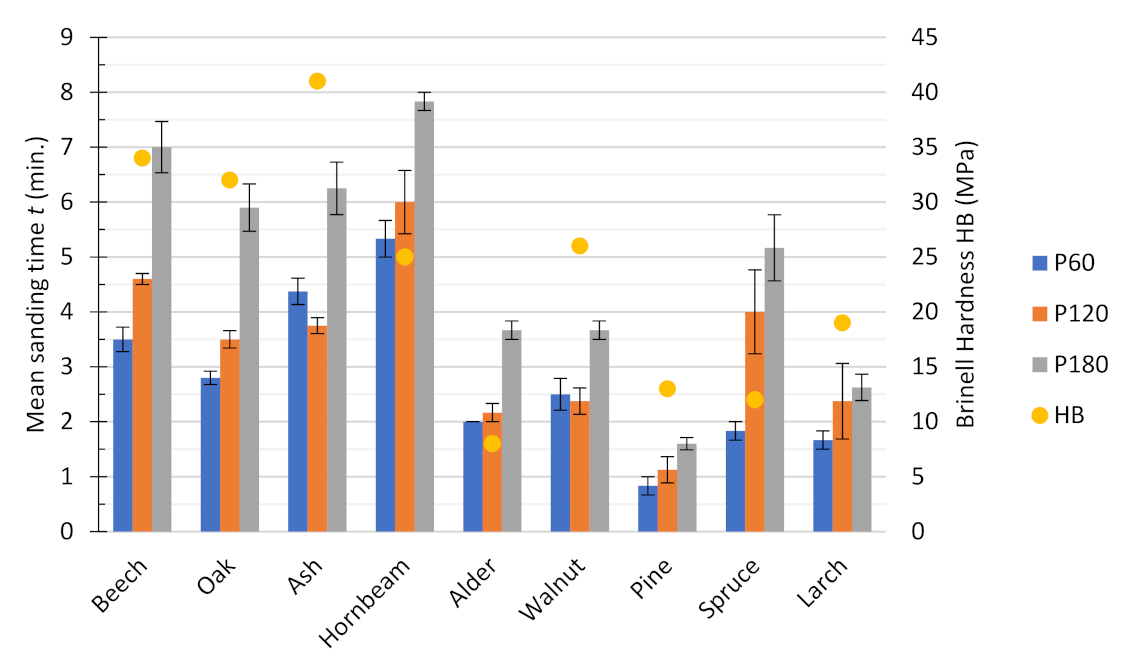
3. Results
4. Discussion
5. Conclusions
- The spread of sanding efficiency values for softwood is much larger than in hardwood species. This may be due to the uneven blunting of the belts due to the specific tribological properties of the softwood (resin content).
- The type of wood is the factor that determines the wood removal rate and thus the sanding efficiency in the initial blunting phase of the sanding belts. In the first phase of blunting of the sanding belts, the sanding processes of hardwood and softwood are significantly different. In the second phase of blunting, sanding belts with higher grit numbers (P120 and P180) behave similarly while sanding hardwood and softwood. Wood type is, therefore, another factor apart from the previously known ones (pressure force, belt speed, wood density, and the grit number of the sanding belt), which should be taken into account when designing the sanding processes of solid wood elements.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rogoziński, T.; Hlásková, L.; Wieruszewski, M.; Očkajová, A. Particle-Size Distribution of Dust Created during Sanding the Modified Ash Wood. Ann. WULS SGGW For. Wood Tech. 2015, 90, 162–166. [Google Scholar]
- Antov, P.; Brezin, V. Инженерна екология/Engineering Ecology; Publishing house—University of Forestry: Sofia, Bulgaria, 2015; ISBN 978-954-332-135-3. [Google Scholar]
- Očkajová, A.; Kučerka, M.; Krišťák, L.; Igaz, R. Granulometric Analysis of Sanding Dust from Selected Wood Species. Bioresources 2018, 13, 7481–7495. [Google Scholar] [CrossRef]
- Antov, P.; Neykov, N.; Savov, V. Effect of Occupational Safety and Health Risk Management on the Rate of Work–Related Accidents in the Bulgarian Furniture Industry. Wood Des. Technol. 2018, 7, 1–9. [Google Scholar]
- Pędzik, M.; Stuper-Szablewska, K.; Sydor, M.; Rogoziński, T. Influence of Grit Size and Wood Species on the Granularity of Dust Particles during Sanding. Appl. Sci. 2020, 10, 8165. [Google Scholar] [CrossRef]
- Očkajová, A.; Kučerka, M.; Kminiak, R.; Krišťák, Ľ.; Igaz, R.; Réh, R. Occupational Exposure to Dust Produced When Milling Thermally Modified Wood. Int. J. Environ. Res. Public Health 2020, 17, 1478. [Google Scholar] [CrossRef] [PubMed]
- Pahlitzsch, G. Internationaler Stand der Forschung auf dem Gebiet des Schleifens von Holz. Holz Roh- Werkst. 1970, 28, 329–343. [Google Scholar] [CrossRef]
- Csanády, E.; Magoss, E. Mechanics of Wood Machining, 3rd ed.; Springer: Cham, Switzerland, 2013; ISBN 3-642-29955-5. [Google Scholar]
- Vlasev, V.; Kovatchev, G.; Atanasov, V. Mechanism for Belt Sanding Machines with a Fixed Bearing of the Sanding Belt and Eccentric Tension. In Proceedings of the Implementation of Wood Science in Woodworking Sector, Zagreb, Croatia, 12–13 December 2019; pp. 221–224. [Google Scholar]
- Pahlitzsch, G.; Dziobek, K. Über das Wesen der Abstumpfung von Schleifbändern beim Bandschleifen von Holz. Holz Roh- Werkst. 1961, 19, 136–149. [Google Scholar] [CrossRef]
- Taylor, J.B.; Carrano, A.L.; Lemaster, R.L. Quantification of Process Parameters in a Wood Sanding Operation. For. Prod. J. 1999, 49, 41–46. [Google Scholar]
- Očkajová, A.; Sikliena, M. The Influence of Chosen Factors of Wood Sanding upon the Efficiency of Sand Belt. Drevarsky Vyskum/Wood Res. 2000, 45, 33–38. [Google Scholar]
- Carrano, A.L.; Taylor, J.B.; Lemaster, R. Parametric Characterization of Peripheral Sanding. For. Prod. J. 2002, 52, 44–50. [Google Scholar]
- Sinn, G.; Gindl, M.; Reiterer, A.; Stanzl-Tschegg, S. Changes in the Surface Properties of Wood Due to Sanding. Holzforschung 2004, 58, 246–251. [Google Scholar] [CrossRef]
- Gurau, L.; Mansfield-Williams, H.; Irle, M. Processing Roughness of Sanded Wood Surfaces. Holz Roh- Werkst. 2005, 63, 43–52. [Google Scholar] [CrossRef]
- Porankiewicz, B.; Banski, A.; Wieloch, G. Specific Resistance and Specific Intensity of Belt Sanding of Wood. Bioresources 2010, 5, 1626–1660. [Google Scholar]
- Ratnasingam, J.; Reid, H.F.; Perkins, M.C. The Abrasive Sanding of Rubberwood (Hevea Brasiliensis): An Industrial Perspective. Holz Roh- Werkst. 2002, 60, 191–196. [Google Scholar] [CrossRef]
- Burdurlu, E.; Usta, I.; Ulupinar, M.; Aksu, B.; Erarslan, T.Ç. The Effect of the Number of Blades and the Grain Size of Abrasives in Planing and Sanding on the Surface Roughness of European Black Pine and Lombardy Poplar. Turk. J. Agric. For. 2005, 29, 315–321. [Google Scholar] [CrossRef]
- Malkoçogǧlu, A.; Özdemir, T. The Machining Properties of Some Hardwoods and Softwoods Naturally Grown in Eastern Black Sea Region of Turkey. J. Mater. Process. Technol. 2006, 173, 315–320. [Google Scholar] [CrossRef]
- Aslan, S.; Coşkun, H.; Kiliç, M. The Effect of the Cutting Direction, Number of Blades and Grain Size of the Abrasives on Surface Roughness of Taurus Cedar (Cedrus Libani A. Rich.) Woods. Build. Environ. 2008, 43, 696–701. [Google Scholar] [CrossRef]
- Očkajová, A.; Kučerka, M.; Krišťák, L.; Ružiak, I.; Gaff, M. Efficiency of Sanding Belts for Beech and Oak Sanding. Bioresources 2016, 11. [Google Scholar] [CrossRef]
- Wieloch, G.; Sikliena, M. Wpływ Wybranych Parametrów Na Wydajność Ubytkową Procesu Szlifowania Drewna/Influence of selected parameters on the loss efficiency of the wood sanding process. Drewno 2004, 47, 121–130. [Google Scholar]
- Saloni, D.E.; Lemaster, R.L.; Jackson, S.D. Abrasive Machining Process Characterization on Material Removal Rate, Final Surface Texture, and Power Consumption for Wood. For. Prod. J. 2005, 55, 35–41. [Google Scholar]
- Błaszczyk, A.; Turek, S. Teoria Mnogości/Set Theory; Wydawnictwo Naukowe PWN: Warsaw, Poland, 2007; ISBN 978-83-01-15232-1. [Google Scholar]
- Miao, T.; Li, L. Study on Influencing Factors of Sanding Efficiency of Abrasive Belts in Wood Materials Sanding. Wood Res. 2014, 59, 835–842. [Google Scholar]
- Thorpe, A.; Brown, R.C. Factors Influencing the Production of Dust During the Hand Sanding of Wood. Am. Ind. Hyg. 1995, 56, 236–242. [Google Scholar] [CrossRef]
- Chand, N.; Fahim, M. Tribology of Natural Fiber Polymer Composites; Woodhead Publishing; CRC Press: Boca Raton, FL, USA, 2008; ISBN 978-1-84569-393-0. [Google Scholar]
- Proper and Improper Forcing; Perspectives in Mathematical Logic, 2nd ed.; Springer: Berlin/Heidleberg, Germany; New York, NY, USA, 1997; Volume 5, ISBN 978-3-540-51700-9.
- Woodin, W.H. The Axiom of Determinacy, Forcing Axioms, and the Nonstationary Ideal, 2nd ed.; De Gruyter: Berlin/Heidleberg, Germany; New York, NY, USA, 2010; ISBN 978-3-11-021317-1. [Google Scholar]
- Sydor, M.; Pinkowski, G.; Jasińska, A. The Brinell Method for Determining Hardness of Wood Flooring Materials. Forests 2020, 11, 878. [Google Scholar] [CrossRef]
- Sin, H.; Saka, N.; Suh, N.P. Abrasive Wear Mechanisms and the Grit Size Effect. Wear 1979, 55, 163–190. [Google Scholar] [CrossRef]
- Ohtani, T.; Yakou, T.; Kitayama, S. Conditions and Origin of the Critical Grain Size Effect on the Abrasive Wear of Woods. Mokuzai Gakkaishi/J. Jpn. Wood Res. Soc. 1996, 42, 1057–1063. [Google Scholar]
- Xu, M.; Li, L.; Wang, M.; Luo, B. Effects of Surface Roughness and Wood Grain on the Friction Coefficient of Wooden Materials for Wood–Wood Frictional Pair. Tribol. Trans. 2014, 57, 871–878. [Google Scholar] [CrossRef]


| Type of Wood | Density | Number of Samples in Set | ||
|---|---|---|---|---|
| kg/m−3 | Sandpaper P60 | Sandpaper P120 | Sandpaper P180 | |
| Beech (Fagus sylvatica L.) | 686.6 | 5 | 5 | 5 |
| Oak (Quercus robur L) | 686.4 | 5 | 5 | 5 |
| Ash (Fraxinus excelsior L.) | 621.3 | 4 | 4 | 4 |
| Hornbeam (Carpinus betulus L.) | 753.6 | 3 | 3 | 3 |
| Alder (Alnus glutinosa (L.) Gaertn.) | 446.3 | 3 | 3 | 3 |
| Walnut (Juglans nigra L.) | 641.0 | 3 | 4 | 3 |
| Pine (Pinus sylvestris L.) | 545.7 | 3 | 4 | 5 |
| Spruce (Picea abies (L.) H.Karst.) | 453.2 | 3 | 3 | 3 |
| Larch (Larix decidua Mill.) | 420.1 | 3 | 4 | 4 |
| Type | EKA 1000 F (Ekamant) | ||
|---|---|---|---|
| ISO/FEPA Grit designation | P 60 (medium) | P 120 (fine) | P 180 (very fine) |
| abrasive material | aluminum oxide, av. particle size 269 µm | aluminum oxide, av. particle size 120 µm | aluminum oxide, av. particle size 82 µm |
| Backing | F weight paper | ||
| adhesive | resin | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sydor, M.; Mirski, R.; Stuper-Szablewska, K.; Rogoziński, T. Efficiency of Machine Sanding of Wood. Appl. Sci. 2021, 11, 2860. https://doi.org/10.3390/app11062860
Sydor M, Mirski R, Stuper-Szablewska K, Rogoziński T. Efficiency of Machine Sanding of Wood. Applied Sciences. 2021; 11(6):2860. https://doi.org/10.3390/app11062860
Chicago/Turabian StyleSydor, Maciej, Radosław Mirski, Kinga Stuper-Szablewska, and Tomasz Rogoziński. 2021. "Efficiency of Machine Sanding of Wood" Applied Sciences 11, no. 6: 2860. https://doi.org/10.3390/app11062860
APA StyleSydor, M., Mirski, R., Stuper-Szablewska, K., & Rogoziński, T. (2021). Efficiency of Machine Sanding of Wood. Applied Sciences, 11(6), 2860. https://doi.org/10.3390/app11062860







