Dirac Equation-Based Formulation for the Quantum Conductivity in 2D-Nanomaterials
Abstract
1. Introduction
2. Dirac Equation-Based Model
3. Current: The Classical Drude Limit
4. Full Quantum-Mechanical Calculation
5. Graphene
6. Results
6.1. Graphene Conductivity under a Uniform Bias Potential
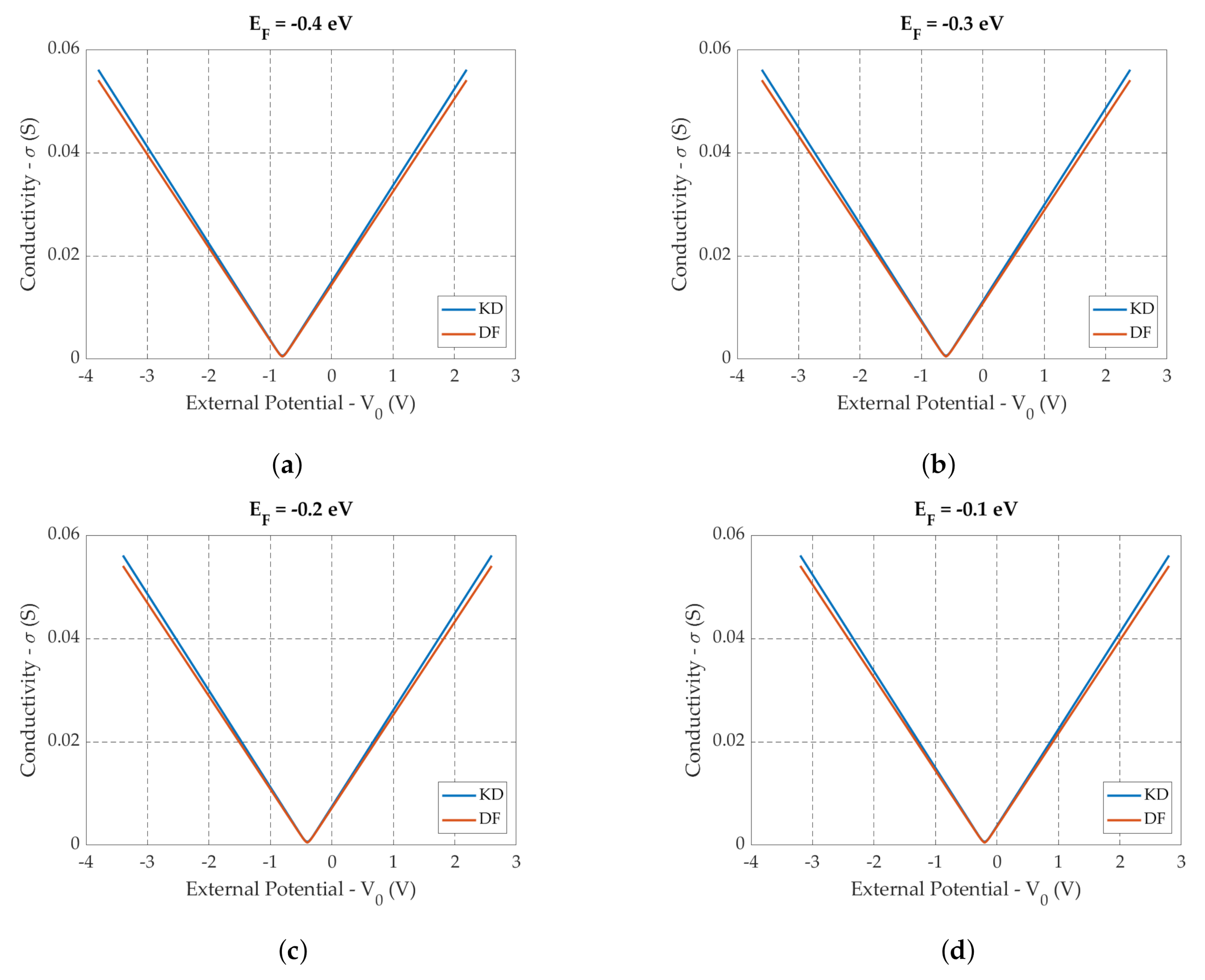
6.1.1. Conductivity in Function of the Chemical Potential
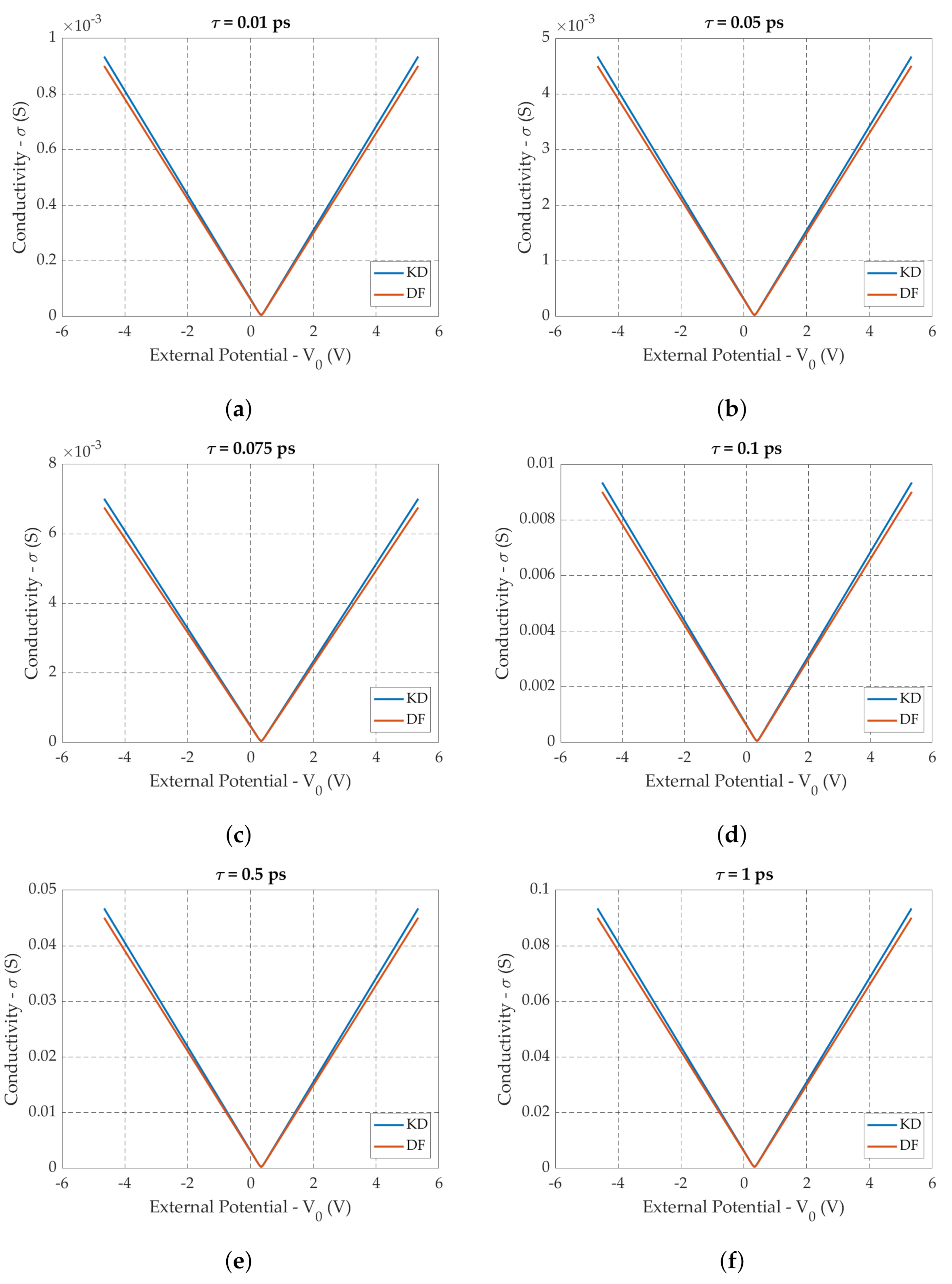
6.1.2. Conductivity in Function of the Scattering Time
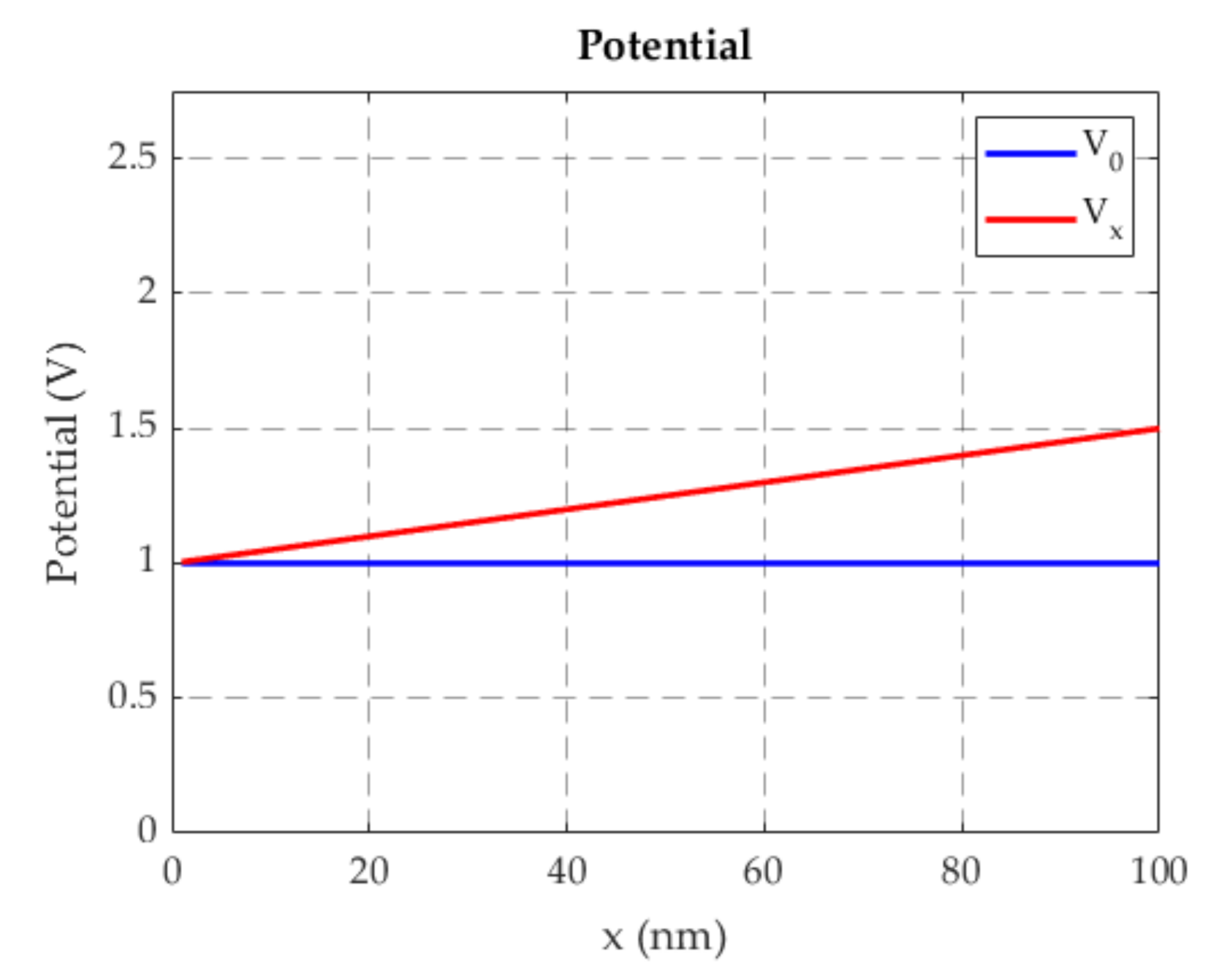
6.2. Graphene Conductivity by Applying a Longitudinal Electric Field
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. arXiv 2007, arXiv:cond-mat/0702595. [Google Scholar] [CrossRef]
- Morozov, S.V. Giant intrinsic carrier mobilities in graphene and its bilayer. Phys. Rev. Lett. 2008, 100, 602–616. [Google Scholar] [CrossRef]
- Blake, P.; Hill, E.W.; Castro Neto, A.H.; Novoselov, K.S.; Jiang, D.; Yang, R.; Booth, T.J.; Geim, A.K. Making graphene visible. Appl. Phys. Lett. 2007, 91, 063124. [Google Scholar] [CrossRef]
- Son, Y.W.; Cohen, M.L.; Louie, S.G. Energy gaps in graphene nanoribbons. Phys. Rev. Lett. 2006, 97, 216803-4. [Google Scholar] [CrossRef] [PubMed]
- Pierantoni, L.; Mencarelli, D.; Bozzi, M.; Moro, R.; Moscato, S.; Perregrini, L.; Micciulla, F.; Cataldo, A.; Bellucci, S. Broadband Microwave Attenuator Based on Few Layer Graphene Flakes. IEEE Trans. Microw. Theory Tech. 2015, 63, 2491–2497. [Google Scholar] [CrossRef]
- Rozzi, T.; Mencarelli, D.; Pierantoni, L. Towards a Unified Approach to Electromagnetic Fields and Quantum Currents From Dirac Spinors. IEEE Trans. Microw. Theory Tech. 2011, 59, 2587–2594. [Google Scholar] [CrossRef]
- Koppens, F.H.L.; Chang, D.E.; de Abajo, F.J.G. Graphene Plasmonics: A Platform for Strong Light–Matter Interactions. Nano Lett. 2011, 11, 3370. [Google Scholar] [CrossRef] [PubMed]
- Ju, L.; Geng, B.; Hornh, J.; Girit, C.; Martin, M.; Hao, Z.; Betchel, H.A.; Liang, X.; Zettl, A.; Shen, Y.R.; et al. Graphene plasmonics for tunable terahertz metamaterials. Nat. Nanotechnol. 2011, 6, 630. [Google Scholar] [CrossRef]
- Echtermeyer, T.; Britnell, L.; Jasnos, P.K.; Lombardo, A.; Gorbachev, R.V.; Grigorenko, A.N.; Geim, A.K.; Ferrari, A.C.; Novoselov, K.S. Strong plasmonic enhancement of photovoltage in graphene. Nat. Commun. 2011, 2, 458. [Google Scholar] [CrossRef]
- Christensen, J.; Manjavacas, A.; Thongrattanasiri, S.; Koppens, F.H.; García de Abajo, F.J. Graphene Plasmon Waveguiding and Hybridization in Individual and Paired Nanoribbons. ACS Nano 2011, 6, 431. [Google Scholar] [CrossRef] [PubMed]
- Smirnova, D.A.; Noskov, R.E.; Smirnov, L.A.; Kivshar, Y.S. Dissipative plasmon solitons in graphene nanodisk arrays. Phys. Rev. B 2015, 91, 075409. [Google Scholar] [CrossRef]
- Diaconescu, B.; Pohl, K.; Vattuone, L.; Savio, L.; Hofmann, P.; Silkin, V.M.; Pitarke, J.M.; Chulkov, E.V.; Echenique, P.M.; Farías, D.; et al. Low-energy acoustic plasmons at metal surfaces. Nature 2007, 448, 57. [Google Scholar] [CrossRef]
- Hartmann, R.R.; Kono, J.; Portnoi, M. Terahertz science and technology of carbon nanomaterials. Nanotechnology 2014, 25, 322001. [Google Scholar] [CrossRef] [PubMed]
- Low, T.; Avouris, P. Graphene Plasmonics for Terahertz to Mid-Infrared Applications. ACS Nano 2014, 8, 1086. [Google Scholar] [CrossRef] [PubMed]
- Grigorenko, A.N.; Polini, M.; Novoselov, K. Graphene plasmonics. Nat. Photonics 2012, 6, 749. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Fal’ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192. [Google Scholar] [CrossRef] [PubMed]
- García de Abajo, F.J. Graphene Plasmonics: Challenges and Opportunities. ACS Photonics 2014, 1, 135. [Google Scholar] [CrossRef]
- Pisarra, M.; Sindona, A.; Riccardi, P.; Silkin, V.; Pitarke, J. Acoustic plasmons in extrinsic free-standing graphene. New J. Phys. 2014, 16, 083003. [Google Scholar] [CrossRef]
- Farina, M.; Ye, T.; Lanzani, G.; Di Donato, A.; Venanzoni, G.; Mencarelli, D.; Pietrangelo, T.; Morini, A.; Keivanidis, P.E. Fast ultrahigh-density writing of low-conductivity patterns on semiconducting polymers. Nat. Commun. 2013, 4, 1. [Google Scholar] [CrossRef][Green Version]
- Correas-Serrano, D.; Gomez-Diaz, J.S.; Alvarez-Melcon, A. On the Influence of Spatial Dispersion on the Performance of Graphene-Based Plasmonic Devices. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 345. [Google Scholar] [CrossRef]
- Lovat, G.; Hanson, G.W.; Araneo, R.; Burghignoli, P. Semiclassical spatially dispersive intraband conductivity tensor and quantum capacitance of graphene. Phys. Rev. B 2013, 87, 115429. [Google Scholar] [CrossRef]
- Correas-Serrano, D.; Gomez-Diaz, J.S.; Perruisseau-Carrier, J.; Alvarez-Melcon, A. Spatially Dispersive Graphene Single and Parallel Plate Waveguides: Analysis and Circuit Model. IEEE Trans. Microw. Theory Tech. 2013, 61, 4333. [Google Scholar] [CrossRef]
- Louie, S.G.; Cohen, M.L. (Eds.) Chapter 6 electron transport. In Conceptual Foundations of Materials: A Standard Model for Ground- and Excited-State Properties; Contemporary Concepts of Condensed Matter Science; Elsevier: Amsterdam, The Netherlands, 2006; Volume 2, pp. 165–218. [Google Scholar]
- Gusynin, V.; Sharapov, S.; Carbotte, J. Magneto-optical conductivity in graphene. J. Phys. Condens. Matter 2007, 19, 026222. [Google Scholar] [CrossRef]
- Gusynin, V.; Sharapov, S.; Carbotte, J. Sum rules for the optical and Hall conductivity in graphene. Phys. Rev. B 2007, 75, 165407. [Google Scholar] [CrossRef]
- Hanson, G.W. Dyadic Green’s functions and guided surface waves for a surface conductivity model of graphene. J. Appl. Phys. 2008, 103, 064302. [Google Scholar] [CrossRef]
- Liang, L.H.; Wei, Y.G.; Li, B. Size-dependent interface phonon transmission and thermal conductivity of nanolaminates. J. Appl. Phys. 2008, 103, 084314. [Google Scholar] [CrossRef]
- Mencarelli, D.; Pierantoni, L.; di Donato, A.; Farina, M. Microwave characterization of anisotropic graphene by applying the Duality theorem. J. Comput. Electron. 2015, 14, 214. [Google Scholar] [CrossRef]
- He, X.Y.; Li, R. Comparison of Graphene-Based Transverse Magnetic and Electric Surface Plasmon Modes. IEEE J. Sel. Top. Quantum Electron. 2014, 20, 62. [Google Scholar]
- Gradshteyn, I.; Ryzhik, I. Table of Integrals, Series, and Products, 7th ed.; Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Durkan, C. Current at the Nanoscale; College Press: Joplin, MO, USA, 2007. [Google Scholar]
- Hanson, G.W. Dyadic Green’s Functions for an Anisotropic, Non-Local Model of Biased Graphene. IEEE Trans. Antennas Propag. 2008, 56, 747. [Google Scholar] [CrossRef]
- Mencarelli, D.; Bellucci, S.; Sindona, A.; Pierantoni, L. Spatial dispersion effects upon local excitation of extrinsic plasmons in graphene micro-discs. J. Phys. D Appl. Phys. 2015, 48, 1–9. [Google Scholar] [CrossRef][Green Version]
- Lancaster, P. Theory of Matrices; Academic Press: Cambridge, MA, USA, 1969. [Google Scholar]
- Hanson, G.W. Quasi-transverse electromagnetic modes supported by a graphene parallel-plate waveguide. J. Appl. Phys. 2008, 104, 084314. [Google Scholar] [CrossRef]
- Mencarelli, D.; Rozzi, T.; Pierantoni, L. Coherent Carrier Transport and Scattering by Lattice Defects in Single- and Multi-Branch Carbon Nanoribbons. Phys. Rev. B Condens. Matter Mater. 2008, 77, 195435-1–195435-11. [Google Scholar] [CrossRef]
- Passi, V.; Gahoi, A.; Lemme, M.C. Enhanced asymmetry in monolayer graphene geometric diodes. In Proceedings of the 2017 Silicon Nanoelectronics Workshop (SNW), Kyoto, Japan, 4–5 June 2017. [Google Scholar]

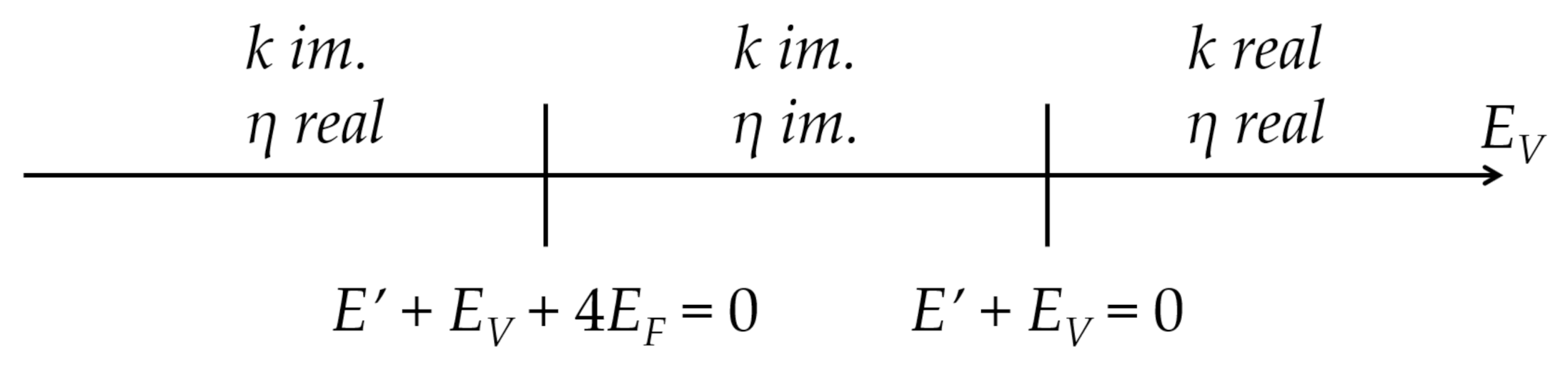


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pierantoni, L.; Pelagalli, N.; Mencarelli, D.; Di Donato, A.; Orlandini, M.; Pagliuca, J.; Rozzi, T. Dirac Equation-Based Formulation for the Quantum Conductivity in 2D-Nanomaterials. Appl. Sci. 2021, 11, 2398. https://doi.org/10.3390/app11052398
Pierantoni L, Pelagalli N, Mencarelli D, Di Donato A, Orlandini M, Pagliuca J, Rozzi T. Dirac Equation-Based Formulation for the Quantum Conductivity in 2D-Nanomaterials. Applied Sciences. 2021; 11(5):2398. https://doi.org/10.3390/app11052398
Chicago/Turabian StylePierantoni, Luca, Nicola Pelagalli, Davide Mencarelli, Andrea Di Donato, Matteo Orlandini, Jacopo Pagliuca, and Tullio Rozzi. 2021. "Dirac Equation-Based Formulation for the Quantum Conductivity in 2D-Nanomaterials" Applied Sciences 11, no. 5: 2398. https://doi.org/10.3390/app11052398
APA StylePierantoni, L., Pelagalli, N., Mencarelli, D., Di Donato, A., Orlandini, M., Pagliuca, J., & Rozzi, T. (2021). Dirac Equation-Based Formulation for the Quantum Conductivity in 2D-Nanomaterials. Applied Sciences, 11(5), 2398. https://doi.org/10.3390/app11052398







