Experimental Study on the Water Selective Dense Membrane under an Absolute Pressure Difference with Simplified Test Method
Abstract
:1. Introduction
2. Materials and Methods
3. Membrane Performance Measurements
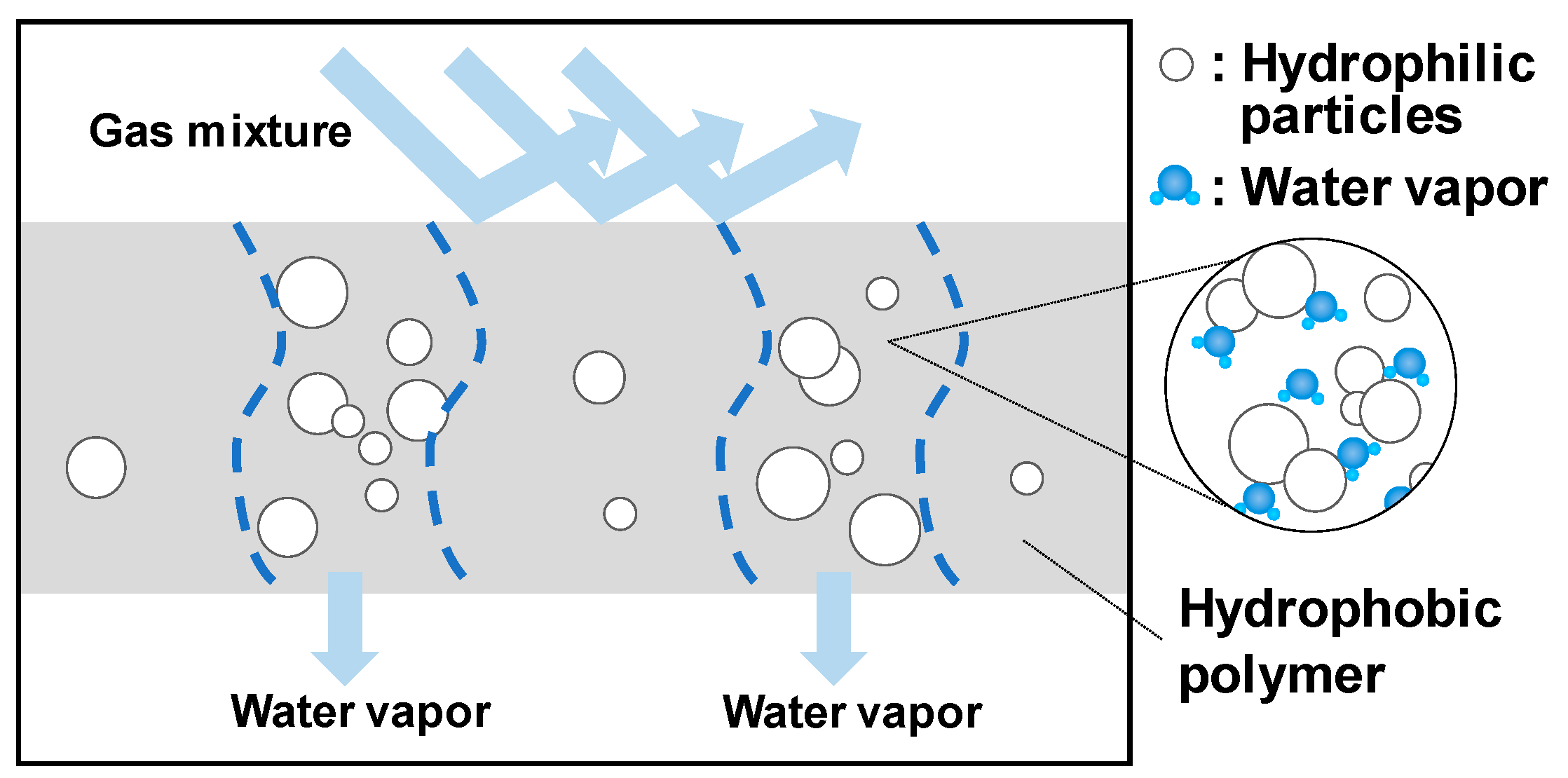
3.1. Test Methods for Water Vapor Permeability
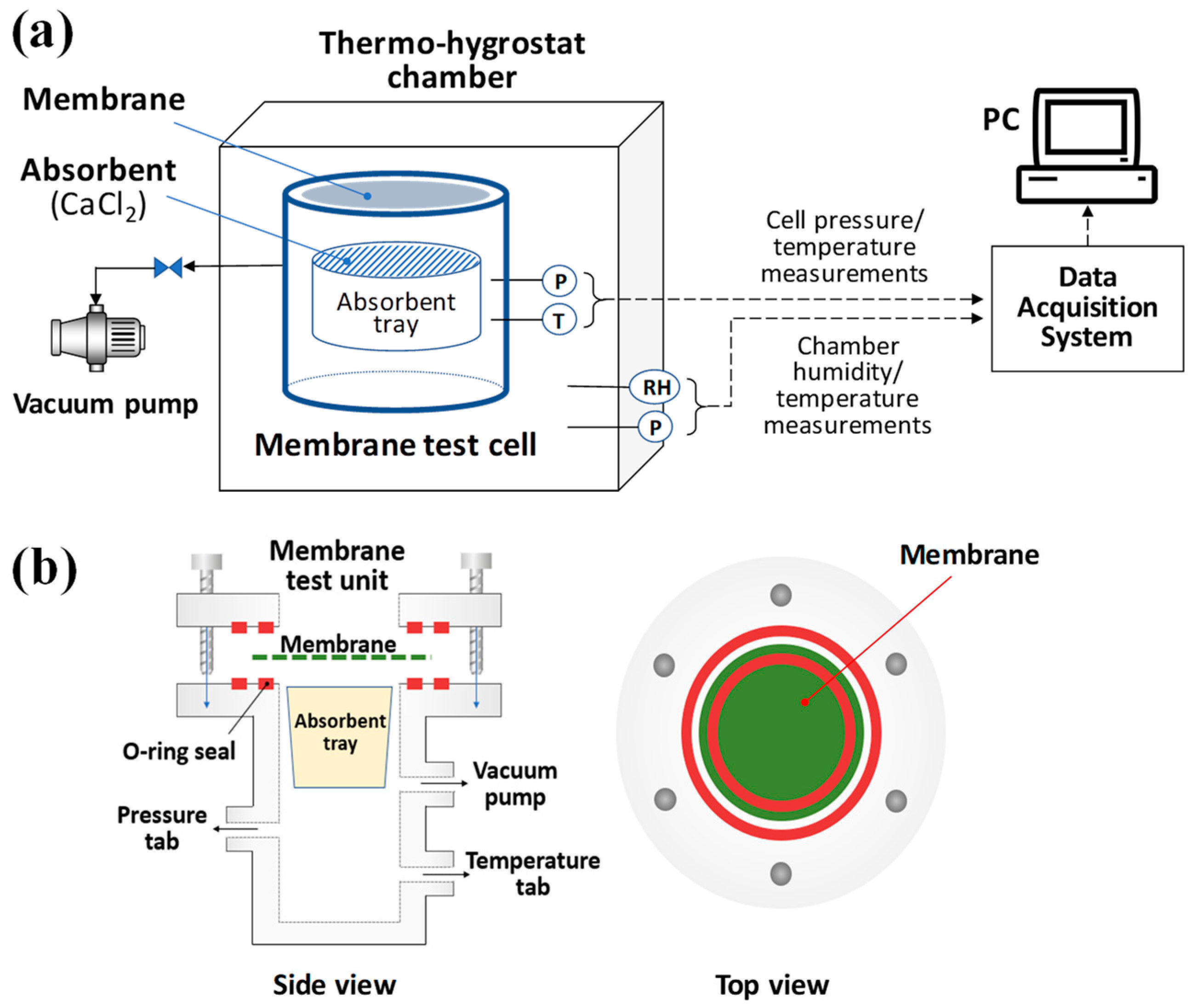
3.2. Experimental Setup
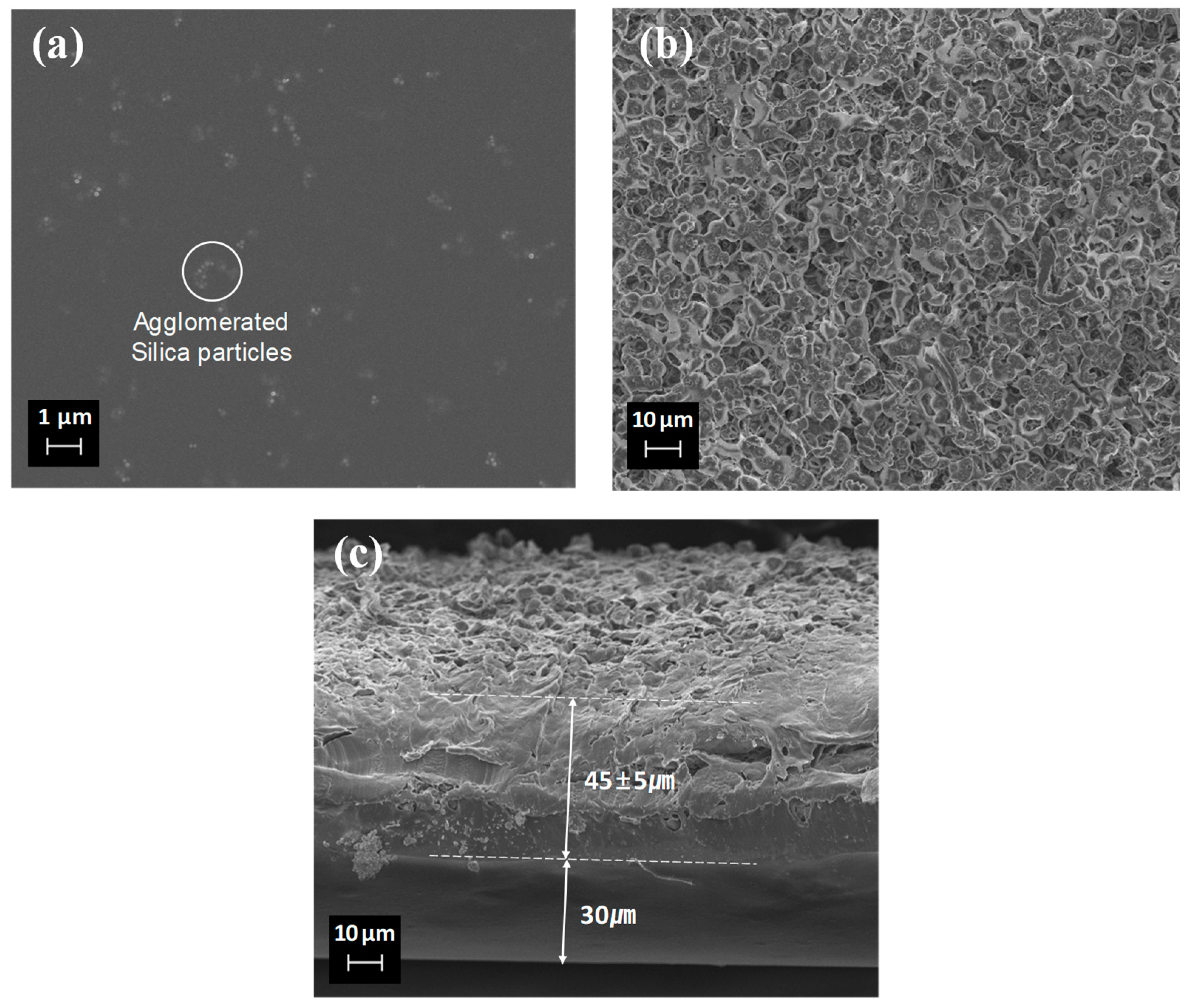
3.3. Dense Membrane Sample
3.4. Test Condition and Cases
3.5. Membrane Performance Calculation
3.5.1. H2O Permeance
3.5.2. N2 Permeance
3.5.3. Selectivity
3.6. Uncertainty of Measurements
3.6.1. Experimental Uncertainties
3.6.2. Measurement and Calculation Uncertainties
4. Results and Discussion
4.1. Experiment Repeatability
4.2. Membrane Performance
4.2.1. Effect of Absolute Pressure Difference
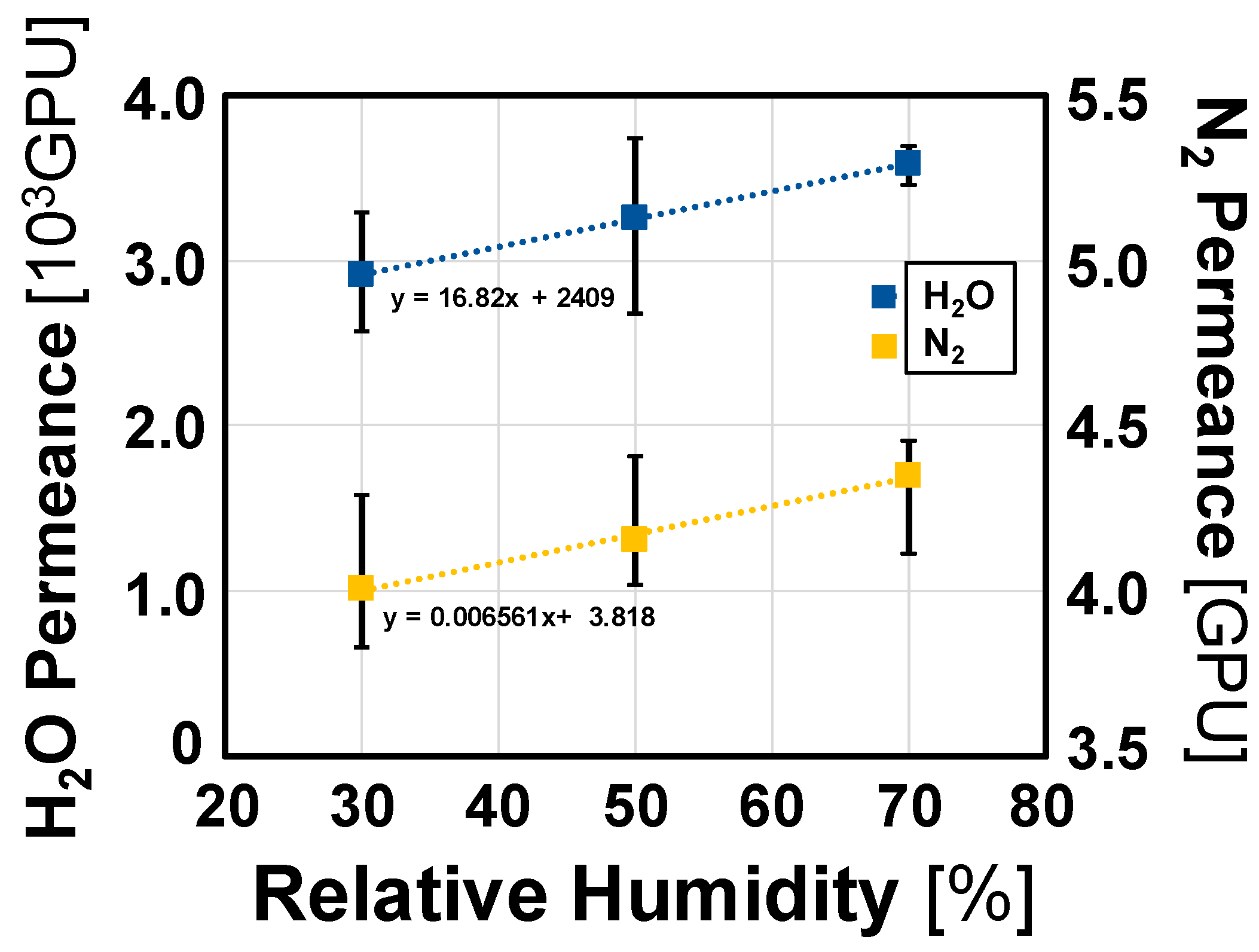
4.2.2. Effect of Relative Humidity
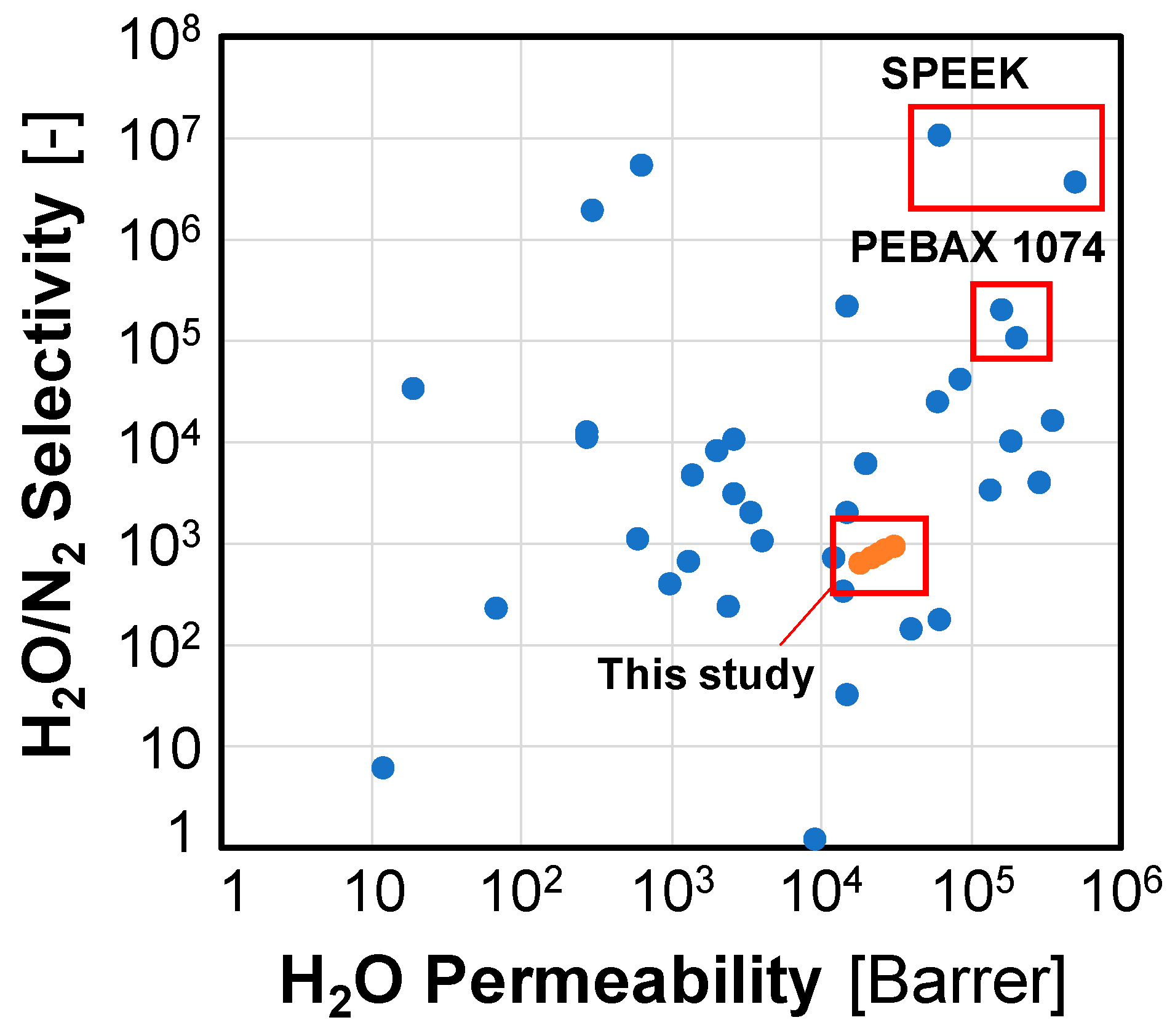
4.3. Comparison with Previous Studies
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| area of membrane (m2) | |
| width of masked edge (m) | |
| distance between the absorbent and membrane (m) | |
| diffusion coefficient (-) | |
| mass flux of H2O (g/m2s) | |
| mass flux of N2 (g/m2s) | |
| mass variation of CaCl2 (g) | |
| mass variation of N2 (g) | |
| molar mass of H2O (g/mol) | |
| molar mass of N2 (g/mol) | |
| pressure of test cell (kPa) | |
| atmospheric pressure (kPa) | |
| water saturation pressure (kPa) | |
| water vapor pressure (kPa) | |
| pressure difference (kPa) | |
| corrected water vapor permeance (mol/m2sPa) | |
| water vapor permeance (mol/m2sPa) | |
| nitrogen permeance (mol/m2sPa) | |
| resistance due to still air (mol/m2sPa) | |
| ideal gas constant of H2O (kJ/kgK) | |
| ideal gas constant of N2 (kJ/kgK) | |
| parameter by four times the test area divided by the perimeter (m) | |
| time variation (s) | |
| temperature (°C) | |
| volume of the cell (m3) | |
| selectivity (-) | |
| specimen thickness (m) | |
| error (%) |
References
- International Energy Agency. Energy Technology Perspectives 2017; IEA: Paris, France, 2017. [Google Scholar]
- U.S. Department of Energy; Building Technologies Office. Energy Savings Potential and RD & D Opportunities for Non-Vapor-Compression HVAC Technologies; Office of Energy and Renewable Energy: Washington, DC, USA, 2014.
- Lim, H.; Choi, S.; Cho, Y.; Kim, S.; Kim, M. Comparative thermodynamic analysis of membrane-based vacuum air dehumidification systems. Appl. Therm. Eng. 2020, 179, 115676. [Google Scholar] [CrossRef]
- Qu, M.; Abdelaziz, O.; Gao, Z.; Yin, H. Isothermal membrane-based air dehumidification: A comprehensive review. Renew. Sustain. Energy Rev. 2018, 82, 4060–4069. [Google Scholar] [CrossRef]
- Woods, J. Membrane processes for heating, ventilation, and air conditioning. Renew. Sustain. Energy Rev. 2014, 33, 290–304. [Google Scholar] [CrossRef]
- Labban, O.; Chen, T.; Ghoniem, A.F.; Lienhard, J.H.; Norford, L.K. Next-generation HVAC: Prospects for and limitations of desiccant and membrane-based dehumidification and cooling. Appl. Energy 2017, 200, 330–346. [Google Scholar] [CrossRef] [Green Version]
- Bui, D.T.; Ja, M.K.; Gordon, J.M.; Ng, K.C.; Chua, K.J. A thermodynamic perspective to study energy performance of vacuum-based membrane dehumidification. Energy 2017, 132, 106–115. [Google Scholar] [CrossRef] [Green Version]
- Baig, M.I.; Ingole, P.G.; Kil Choi, W.; Park, S.R.; Kang, E.C.; Lee, H.K. Development of carboxylated TiO2 incorporated thin film nanocomposite hollow fiber membranes for flue gas dehydration. J. Membr. Sci. 2016, 514, 622–635. [Google Scholar] [CrossRef]
- Freeman, B.D. Basis of Permeability/Selectivity Tradeoff Relations in Polymeric Gas Separation Membranes. Macromolecules 1999, 32, 375–380. [Google Scholar] [CrossRef]
- Robeson, L.M. The upper bound revisited. J. Membr. Sci. 2008, 320, 390–400. [Google Scholar] [CrossRef]
- Baker, R.W.; Wijmans, J.G.; Huang, Y. Permeability, permeance and selectivity: A preferred way of reporting pervaporation performance data. J. Membr. Sci. 2010, 348, 346–352. [Google Scholar] [CrossRef]
- ASTM International. Standard Test Methods for Water Vapor Transmission of Materials, E 96/E 96M; ASTM International: West Conshohocken, PA, USA, 2016. [Google Scholar]
- Stern, S.A.; Gareis, P.J.; Sinclair, T.F.; Mohr, P.H. Performance of a versatile variable-volume permeability cell. Comparison of gas permeability measurements by the variable-volume and variable-pressure methods. J. Appl. Polym. Sci. 1963, 7, 2035–2051. [Google Scholar] [CrossRef]
- Siegel, R.D.; Coughlin, R.W. Errors in diffusivity as deduced from permeation experiments using the time-lag technique. J. Appl. Polym. Sci. 1970, 14, 3145–3149. [Google Scholar] [CrossRef]
- AlMahmoud, O.; Choi, T.-Y.; Kim, H.-S.; Seo, Y.-S.; Yoon, S.H. A molecular dynamics study on the effect of modified silica surface on water vapor diffusion in the silica–polyurethane nanocomposite membrane. MRS Commun. 2020, 10, 492–499. [Google Scholar] [CrossRef]
- Massman, W. A review of the molecular diffusivities of H2O, CO2, CH4, CO, O3, SO2, NH3, N2O, NO, and NO2 in air, O2 and N2 near STP. Atmos. Environ. 1998, 32, 1111–1127. [Google Scholar] [CrossRef]
- Thuan, B.D.; Yonghui, W.; Jon, C.K.; Choon, N.K. Evaluating water vapor permeance measurement techniques for highly permeable membranes. Comput. Mater. Contin. 2015, 47, 89–105. [Google Scholar] [CrossRef]
- Figliola, R.S.; E Beasley, D. Theory and design for mechanical measurements. Meas. Sci. Technol. 1996, 7. [Google Scholar] [CrossRef] [Green Version]
- Metz, S.; Van De Ven, W.; Potreck, J.; Mulder, M.; Wessling, M. Transport of water vapor and inert gas mixtures through highly selective and highly permeable polymer membranes. J. Membr. Sci. 2005, 251, 29–41. [Google Scholar] [CrossRef]
- Sijbesma, H.; Nymeijer, K.; Van Marwijk, R.; Heijboer, R.; Potreck, J.; Wessling, M. Flue gas dehydration using polymer membranes. J. Membr. Sci. 2008, 313, 263–276. [Google Scholar] [CrossRef]
- Shin, Y.; Liu, W.; Schwenzer, B.; Manandhar, S.; Chase-Woods, D.; Engelhard, M.H.; Devanathan, R.; Fifield, L.S.; Bennett, W.D.; Ginovska, B.; et al. Graphene oxide membranes with high permeability and selectivity for dehumidification of air. Carbon 2016, 106, 164–170. [Google Scholar] [CrossRef] [Green Version]
- Scovazzo, P. Testing and evaluation of room temperature ionic liquid (RTIL) membranes for gas dehumidification. J. Membr. Sci. 2010, 355, 7–17. [Google Scholar] [CrossRef]
- Sato, S.; Suzuki, M.; Kanehashi, S.; Nagai, K. Permeability, diffusivity, and solubility of benzene vapor and water vapor in high free volume silicon- or fluorine-containing polymer membranes. J. Membr. Sci. 2010, 360, 352–362. [Google Scholar] [CrossRef]


| Variables | Test A | Test B | Test C | Test D |
|---|---|---|---|---|
| Initial absorbent tray mass (g) | 77.31 | 79.92 | 89.19 | 75.78 |
| Distance from membrane to absorbent (mm) | 5 | 5 | 5 | 5 |
| Relative humidity (%) | 50 | 50 | 50 | 50 |
| Temperature (°C) | 30 | 30 | 30 | 30 |
| Pressure difference, (kPa) | −42.22 | −41.98 | −42.25 | 162.46 |
| Variables | Scales/Types | Error |
|---|---|---|
| Humidity (RH) | 3–95% RH | ±2% FS (full-scale) |
| Temperature (T) | T-type thermocouple | ±0.5 °C |
| Pressure (p) | 0–200 kPa, abs | ±0.1% FS |
| Mass (m) | 0–600 g, d = 0.01 g | ±0.1 g |
| Test A | Test B | |||
|---|---|---|---|---|
| Time (h) | p(abs) (kPa) | Absorbent Mass (g) | p(abs) (kPa) | Absorbent Mass (g) |
| 0 | 42.22 | 77.31 | 41.98 | 79.92 |
| 12 | 58.78 | - | 57.36 | - |
| 24 | 69.02 | - | 67.68 | - |
| 36 | 76.76 | - | 75.19 | - |
| 48 | 82.48 | 80.60 | 80.97 | 83.21 |
| Time (h) | Test C | Test D | ||
|---|---|---|---|---|
| p(abs) (kPa) | Absorbent Mass (g) | p(abs) (kPa) | Absorbent Mass (g) | |
| 0 | 42.25 | 89.19 | 162.46 | 75.78 |
| 12 | 58.24 | - | 153.40 | - |
| 24 | 68.95 | - | 144.95 | - |
| 36 | 76.58 | - | 138.03 | - |
| 48 | 82.43 | 92.38 | 132.22 | 77.76 |
| Performance Factor | Test C | Test D | Cup |
|---|---|---|---|
| Permeance (H2O) (GPU) | 3.56 × 103 | 2.08 × 103 | 1.68 × 103 |
| Permeance (N2) (GPU) | 4.40 | 2.52 | - |
| Selectivity (-) | 8.08 × 102 | - | - |
| Test Method | RH (%) | H2O Permeability (Barrer) | N2 Permeability (Barrer) | Selectivity (-) |
|---|---|---|---|---|
| Cup method | 70 | 11,390 | - | - |
| Reverse permeation of N2 | 50 | 15,610 | 18.93 | - |
| Test result | 30 | 21,820 | 30.08 | 725.6 |
| 50 | 24,430 | 31.16 | 783.8 | |
| 70 | 26,870 | 32.04 | 838.5 | |
| Extrapolation | 0 | 18,070 | 28.64 | 631.0 |
| 100 | 30,680 | 33.56 | 914.4 |
| Material | H2O Permeability (Barrer) | N2 Permeability (Barrer) | Selectivity (H2O/N2) | Base Condition | Ref. |
|---|---|---|---|---|---|
| Ethyl cellulose | 20,000 | 3.3 | 6061 | Temperature: 30 °C Data from the reference literature were used and extrapolated to a vapor activity of 0 | [19] |
| Cellulose acetate (CA) | 60,000 | 2.5 | 24,000 | ||
| Natural rubber | 2600 | 0.87 | 2999 | ||
| 1000PEO56PBT44 | 85,500 | 2.11 | 40,500 | ||
| Polyacrylonitrile | 300 | 0.00016 | 1,875,000 | ||
| Polyamide 6 (Nylon 6) | 275 | 0.025 | 11,000 | ||
| Polycarbonate | 1400 | 0.3 | 4667 | ||
| Polydimethylsiloxane | 40,000 | 279.72 | 143 | ||
| Polyethersulfone | 2620 | 0.25 | 10,480 | ||
| Polyethylene | 12 | 2 | 6 | ||
| Polyimide (Kapton) | 640 | 0.00012 | 5,333,333 | ||
| Polyphenyleneoxide | 4060 | 3.8 | 1068 | ||
| Polypropylene | 68 | 0.3 | 227 | ||
| Polystyrene | 970 | 2.5 | 388 | ||
| Polysulfone | 2000 | 0.25 | 8000 | ||
| Polyvinylalcohol | 19 | 0.00057 | 33,333 | ||
| Polyvinylchloride | 275 | 0.02 | 12,500 | ||
| Sulfonated polyetheretherketon (SPEEK) | 61,000 | 0.006 | 10,166,667 | ||
| Sulfonated polyethersulofone (SPES) | 15,000 | 0.07 | 214,286 | ||
| Sulfonated polyetheretherketon (SPEEK) | 490,000 | 0.14 | 3,500,000 | Temperature: 30 ℃, RH: 93%, Feed side pressure: 2.5 bar, Mixed-gas measurement | [20] |
| PEBAX® 1074 (polyeth block amide + poly) | 200,000 | 1.92 | 104,166.67 | ||
| PEBAX® 1074 (polyeth block amide + poly) | 160,000 | 0.8 | 200,000 | Single gas ratio (Ideal) | [21] |
| Stabilized liquid polyethylene glycol | 15,000 | 7.5 | 2000 | ||
| NaA zeolite/Ni sheet | 60,900 | 342.13 | 178 | ||
| Graphene oxide | 182,000 | 18.2 | 10,000 | Temperature: 43.4 ℃, H2O mole fraction: 0.02, Mixed-gas measurement | |
| Stabilized liquid Triethylene glycol | 3360 | 1.68 | 2000 | - | [22] |
| (emim) (Tf2N) | 283,000 | 73.64 | 3843 | Single gas ratio (Ideal) | |
| (N(4)111) (Tf2N) | 133,000 | 40.43 | 3290 | ||
| (emim) (BF4) | 354,000 | 21.72 | 16,300 | ||
| PDMS | 15,000 | 468.75 | 32 | Temperature: 35 °C Nitrogen permeability data at 1 atm, Constant-volume variable-pressure, Infinite-dilution data | [23] |
| PTMSMMA | 2400 | 10 | 240 | ||
| PTMSP | 9200 | 7666.67 | 1.2 | ||
| 6FDA-mPD | 600 | 0.55 | 1100 | ||
| 6FDA-MPD | 1300 | 2 | 650 | ||
| 6FDA-TMPD | 12,000 | 16.9 | 710 | ||
| 6FDA-TeMPD | 14,000 | 42.42 | 330 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, J.; Kim, H.-S.; Ku, D.; Lim, J.; Jung, M.; Kim, S.; Jeong, S.; Kim, M.; Seo, Y.-S. Experimental Study on the Water Selective Dense Membrane under an Absolute Pressure Difference with Simplified Test Method. Appl. Sci. 2021, 11, 1710. https://doi.org/10.3390/app11041710
Lee J, Kim H-S, Ku D, Lim J, Jung M, Kim S, Jeong S, Kim M, Seo Y-S. Experimental Study on the Water Selective Dense Membrane under an Absolute Pressure Difference with Simplified Test Method. Applied Sciences. 2021; 11(4):1710. https://doi.org/10.3390/app11041710
Chicago/Turabian StyleLee, Jinwook, Hyo-Sun Kim, Donik Ku, Jihun Lim, Minkyu Jung, Soyeon Kim, Sanghun Jeong, Minsung Kim, and Young-Soo Seo. 2021. "Experimental Study on the Water Selective Dense Membrane under an Absolute Pressure Difference with Simplified Test Method" Applied Sciences 11, no. 4: 1710. https://doi.org/10.3390/app11041710
APA StyleLee, J., Kim, H.-S., Ku, D., Lim, J., Jung, M., Kim, S., Jeong, S., Kim, M., & Seo, Y.-S. (2021). Experimental Study on the Water Selective Dense Membrane under an Absolute Pressure Difference with Simplified Test Method. Applied Sciences, 11(4), 1710. https://doi.org/10.3390/app11041710





