Effect of Compressive Strain Rate on Auxetic Foam
Abstract
1. Introduction
2. Methods
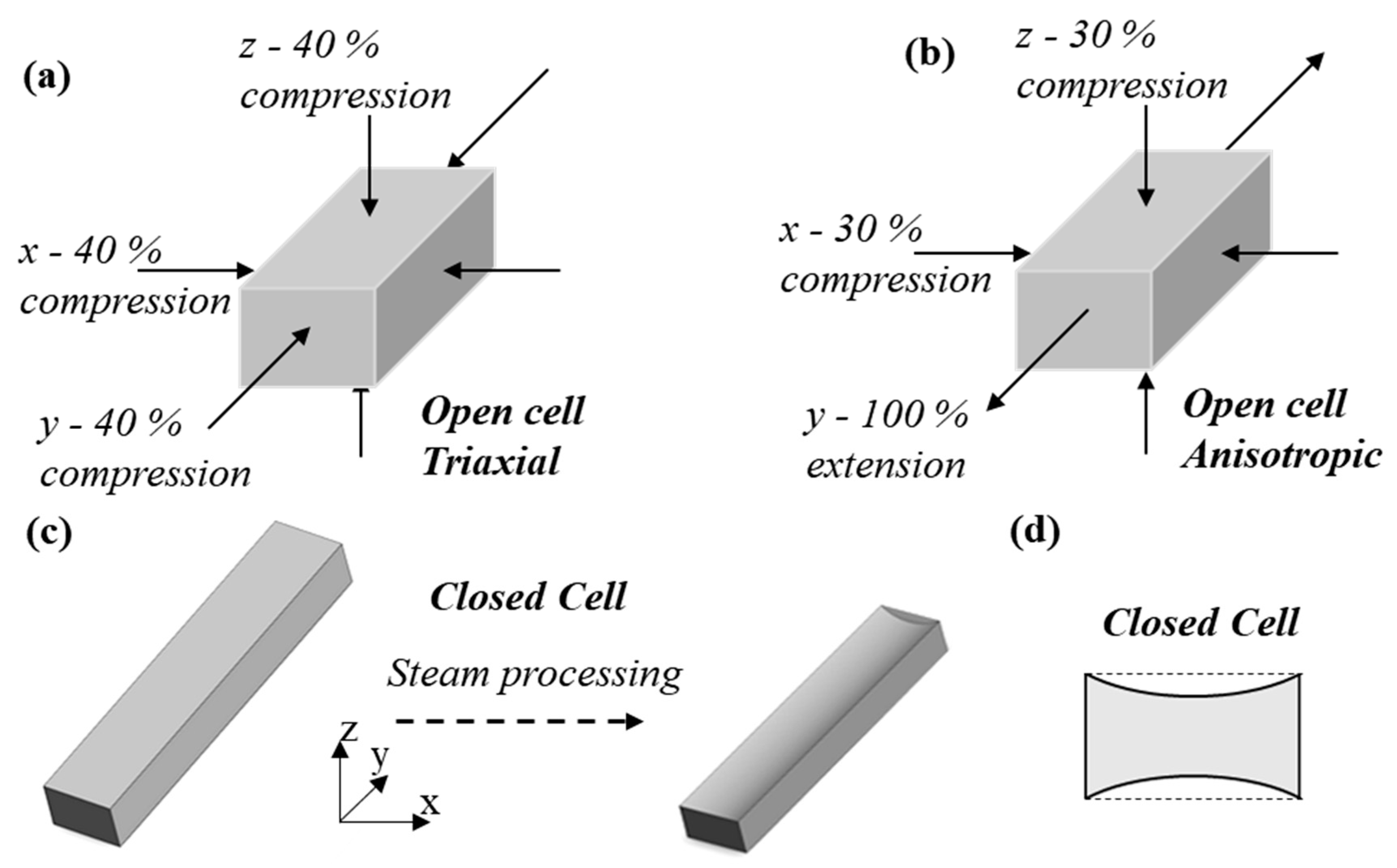
2.1. Fabrication: Open Cell Foams
2.2. Fabrication: Closed Cell Foams
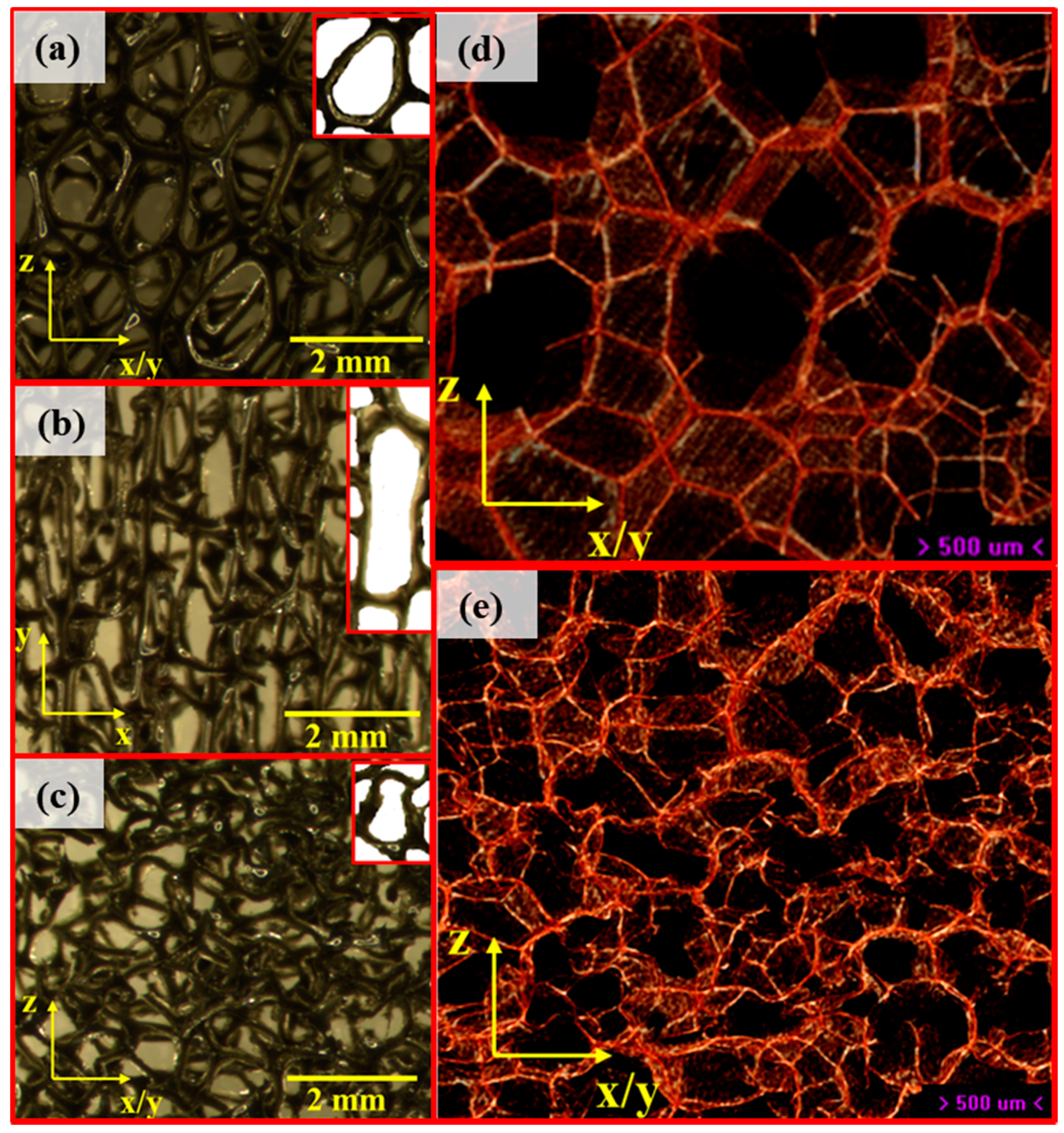
2.3. Cell Structures
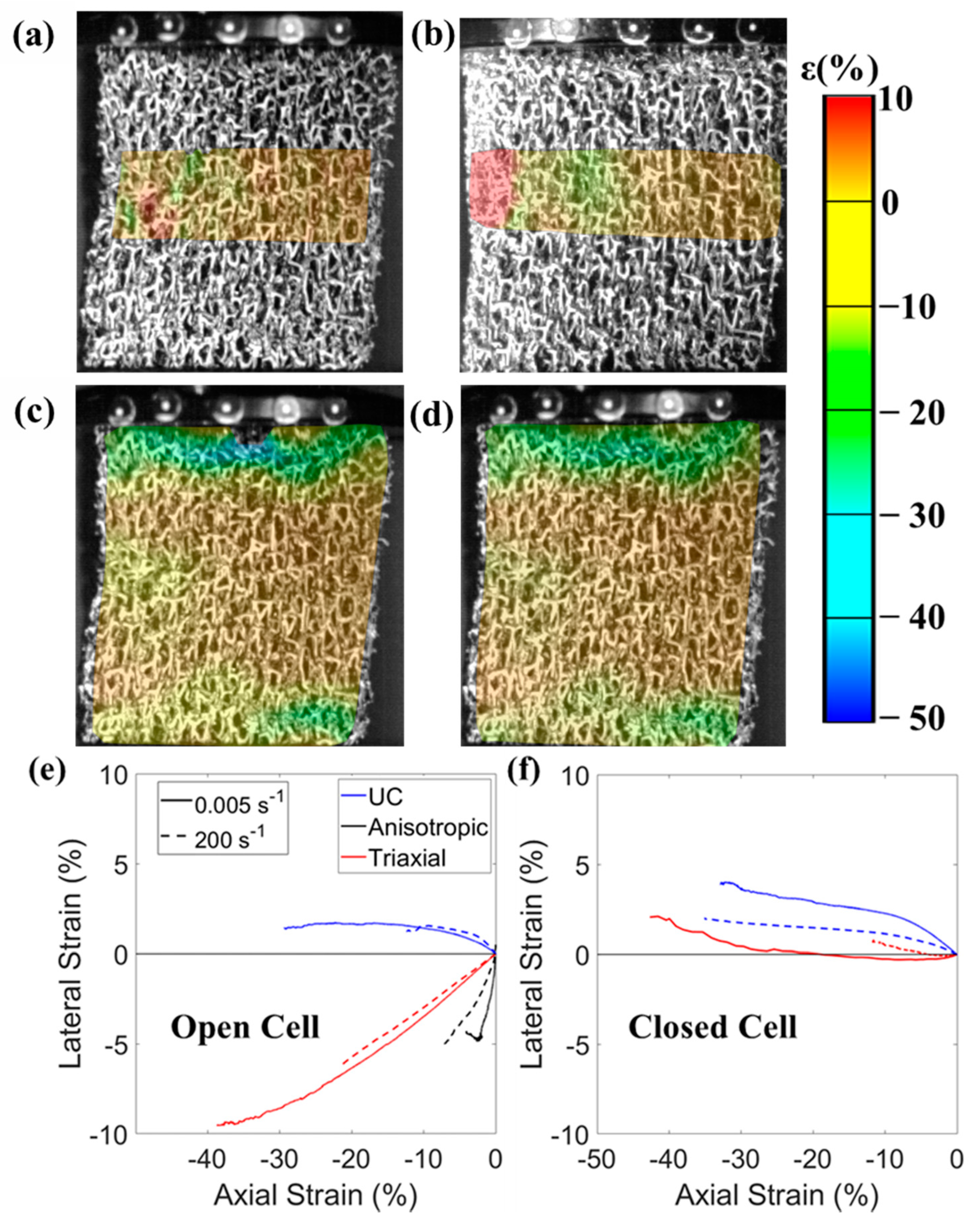
2.4. Compression Testing
3. Results
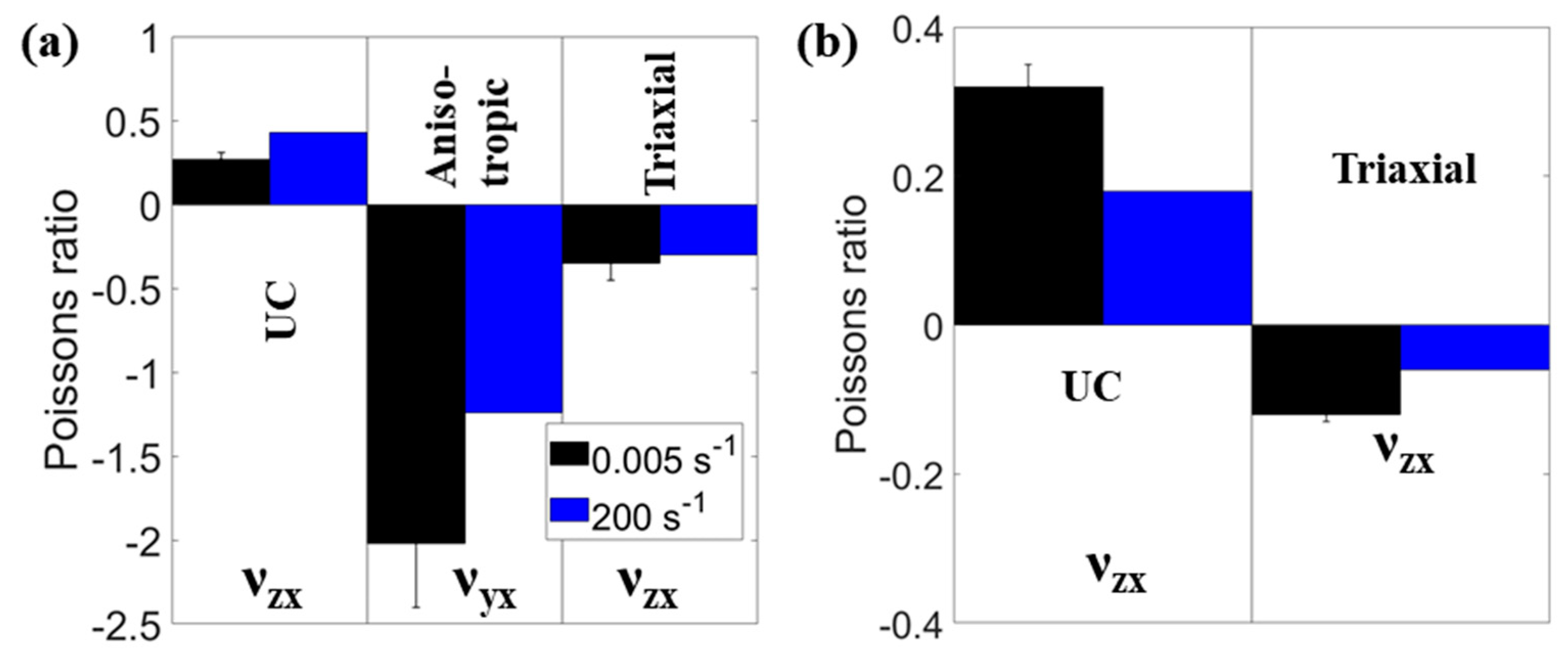
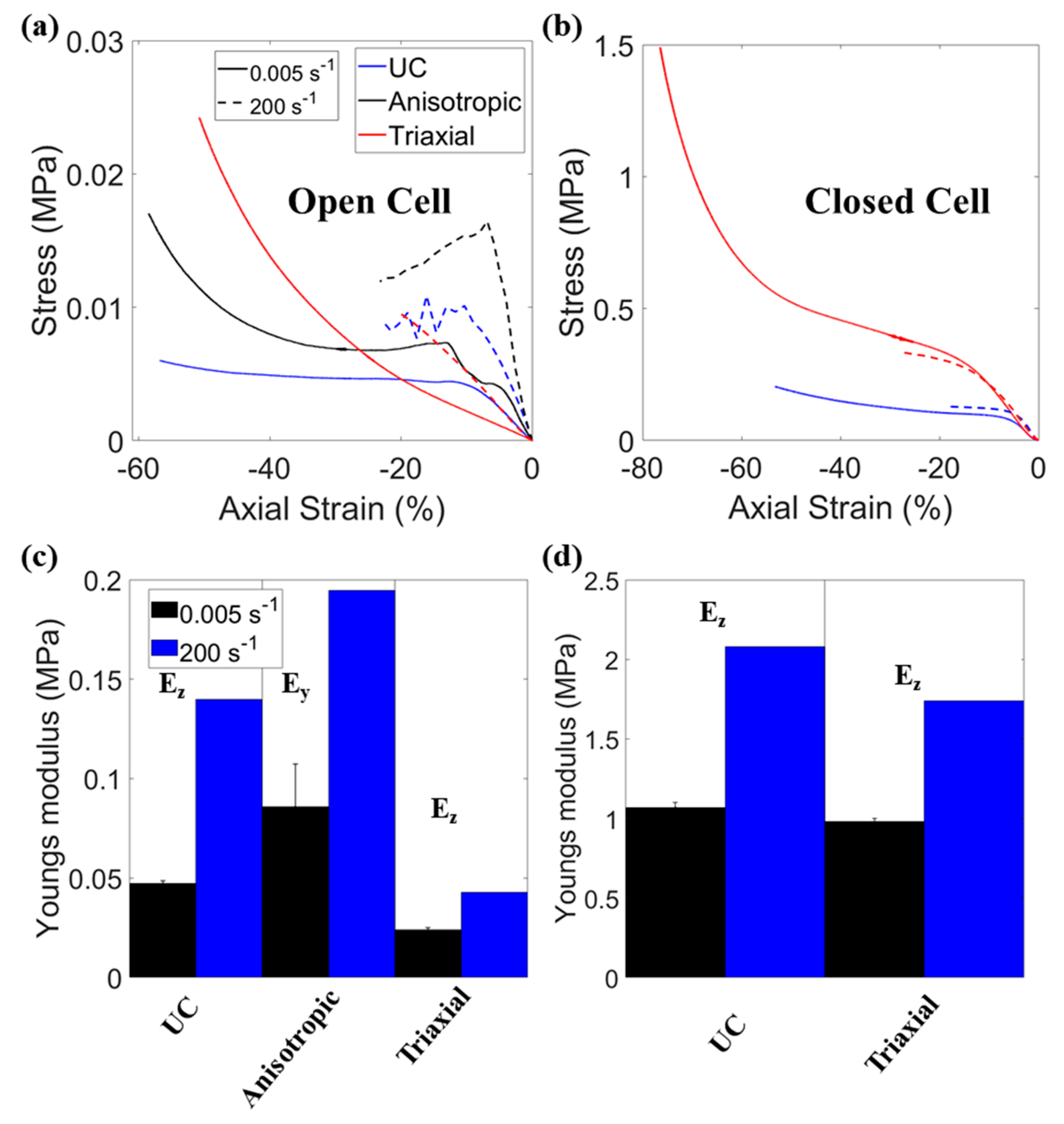
3.1. Poisson’s Ratio
3.2. Young’s Moduli
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Payne, T.; Mitchell, S.; Halkon, B.; Bibb, R. A systematic approach to the characterisation of human impact injury scenarios in sport. BMJ Open Sport Exerc. Med. 2016, 2, e000017. [Google Scholar] [CrossRef] [PubMed]
- Michel, F.I.; Schmitt, K.U.; Liechti, B.; Stämpfli, R.; Brühwiler, P. Functionality of back protectors in snow sports concerning safety requirements. Procedia Eng. 2010, 2, 2869–2874. [Google Scholar] [CrossRef]
- Schmitt, K.-U.; Liechti, B.; Michel, F.I.; Stämpfli, R.; Brühwiler, P.A. Are current back protectors suitable to prevent spinal injury in recreational snowboarders? Br. J. Sports Med. 2010, 44, 822–826. [Google Scholar] [CrossRef] [PubMed]
- Duncan, O.; Shepherd, T.; Moroney, C.; Foster, L.; Venkatraman, P.D.; Winwood, K.; Allen, T.; Alderson, A. Review of auxetic materials for sports applications: Expanding options in comfort and protection. Appl. Sci. 2018, 8, 941. [Google Scholar] [CrossRef]
- Hrysomallis, C.; Morrison, W.; He, J. Assessing the shock absorption of thigh pads. J. Sci. Med. 1999, 2, 49. [Google Scholar] [CrossRef]
- Ankrah, S.; Mills, N.J. Performance of football shin guards for direct stud impacts. Sports Eng. 2003, 6, 207–219. [Google Scholar] [CrossRef]
- Wardiningsih, W.; Troynikov, O.; Nawaz, N.; Watson, C. Influence of wearing impact protective garment on thermophysiological comfort of the wearer. Procedia Eng. 2014, 72, 551–556. [Google Scholar] [CrossRef]
- Webster, J. The Perception of Comfort and Fit of Personal Protective Equipment in Sport. Ph.D. Thesis, Loughborough University, Loughborough, UK, 2010. [Google Scholar]
- Nicotra, M.; Moncalero, M.; Messori, M.; Fabbri, E.; Fiorini, M.; Colonna, M. Thermo-mechanical and impact properties of polymeric foams used for snow sports protective equipment. Procedia Eng. 2014, 72, 678–683. [Google Scholar] [CrossRef]
- Dotti, F.; Ferri, A.; Moncalero, M.; Colonna, M. Thermo-physiological comfort of soft-shell back protectors under controlled environmental conditions. Appl. Ergon. 2016, 56, 144–152. [Google Scholar] [CrossRef]
- Finch, C.F.; McIntosh, A.S.; McCrory, P. What do under 15 year old schoolboy rugby union players think about protective headgear? Br. J. Sports Med. 2001, 35, 89–94. [Google Scholar] [CrossRef]
- Quinn, E.M.; Bradley, C.P. Injury prevention in camogie: Current trends in the use of protective equipment. Eur. J. Sport Sci. 2012, 12, 185–192. [Google Scholar] [CrossRef]
- Webster, J.M.; Roberts, J. Incorporating subjective end-user perceptions in the design process: A study of leg guard comfort in cricket. Proc. Inst. Mech. Eng. Part P J. Sport. Eng. Technol. 2009, 223, 49–62. [Google Scholar] [CrossRef]
- Webster, J.M.; Roberts, J.R. Comfort of cricket leg guards: A study of strap contact pressure. Procedia Eng. 2010, 2, 3385–3390. [Google Scholar] [CrossRef][Green Version]
- Hughes, A.; Carré, P.M.; Driscoll, H. Perceptions and Attitudes Towards Shoulder Padding and Shoulder Injury in Rugby Union. medRxiv 2020, 1–12. [Google Scholar] [CrossRef]
- Brisbine, B.R.; Steele, J.R.; Phillips, E.J.; McGhee, D.E. Use and perception of breast protective equipment by female contact football players. J. Sci. Med. Sport. 2020, 23, 820–825. [Google Scholar] [CrossRef]
- Palmer, R.M.; Green, P.C. Energy Absorbing Material. US Patent US 7381460B2, 3 June 2008. [Google Scholar]
- Plant, D.J. Energy Absorbing System. US Patent US 2012/0021167A1, 26 January 2012. [Google Scholar]
- Wyner, D.M.; Fox, R.B.; Cafaro, T.; Thorn, S.; Newsham, A.; Foster, D. Flexible Cushioning Pads, Items Incorporating Such Pads, and Methods of Making and Using. US Patent US 9,770,642B2, 26 September 2017. [Google Scholar]
- Cossa, K.N. Basic concepts on rheology and application of shear-thickening fluids in protective gear. SN Appl. Sci. 2019, 1, 1284. [Google Scholar] [CrossRef]
- Bhagavathula, K.B.; Azar, A.; Ouellet, S.; Satapathy, S.; Dennison, C.R.; Hogan, J.D. High Rate Compressive Behaviour of a Dilatant Polymeric Foam. J. Dyn. Behav. Mater. 2018, 4, 573–585. [Google Scholar] [CrossRef]
- Kaewpradit, P.; Kongchoo, A.; Chonlathan, P.; Lehman, N.; Kalkornsurapranee, E. Impact Absorbing Kneepad Prepared from Natural Rubber. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Bangkok, Thailand, 16–18 December 2019; Volume 773, p. 012063. [Google Scholar]
- Signetti, S.; Nicotra, M.; Colonna, M.; Pugno, N.M. Modeling and simulation of the impact behavior of soft polymeric-foam-based back protectors for winter sports. J. Sci. Med. Sport 2019, 22, S65–S70. [Google Scholar] [CrossRef]
- Standardization, E.C. for. EN 1621-2 2003 Motorradfahrer Schutzkleidung Teil 2 Rückenprotektoren. 2003. Available online: https://shop.bsigroup.com/ProductDetail/?pid=000000000030109684 (accessed on 26 January 2021).
- Wei, W.; Evin, M.; Bailly, N.; Llari, M.; Laporte, J.D.; Arnoux, P.J. Spinal injury analysis for typical snowboarding backward falls. Scand. J. Med. Sci. Sport 2019, 29, 450–459. [Google Scholar] [CrossRef]
- Michel, F.I.; Schmitt, K.U.; Greenwald, R.M.; Russell, K.; Simpson, F.I.; Schulz, D.; Langran, M. White Paper: Functionality and efficacy of wrist protectors in snowboarding-towards a harmonized international standard. Sports Eng. 2013, 16, 197–210. [Google Scholar] [CrossRef]
- Mcintosh, A.S. Biomechanical considerations in the design of equipment to prevent sports injury. Proc. Inst. Mech. Eng. Part P J. Sport. Eng. Technol. 2011, 226, 193–199. [Google Scholar] [CrossRef]
- Payne, T.; Mitchell, S.; Halkon, B.; Bibb, R.; Waters, M. Development of a synthetic human thigh impact surrogate for sports personal protective equipment testing. Proc. Inst. Mech. Eng. Part P J. Sport. Eng. Technol. 2016, 230, 5–16. [Google Scholar] [CrossRef]
- Whyte, T.; Stuart, C.A.; Mallory, A.; Ghajari, M.; Plant, D.J.; Siegmund, G.P.; Cripton, P.A. A Review of Impact Testing Methods for Headgear in Sports: Considerations for Improved Prevention of Head Injury Through Research and Standards. J. Biomech. Eng. 2019, 141, 070803. [Google Scholar] [CrossRef] [PubMed]
- Novak, N.; Vesenjak, M.; Ren, Z. Auxetic cellular materials—A review. Stroj. Vestnik/J. Mech. Eng. 2016, 62, 485–493. [Google Scholar] [CrossRef]
- Evans, K.E.; Alderson, A. Auxetic materials: Functional materials and structures from lateral thinking! Adv. Mater. 2000, 12, 617–628. [Google Scholar] [CrossRef]
- Scarpa, F.; Giacomin, J.; Zhang, Y.; Pastorino, P. Mechanical performance of auxetic polyurethane foam for antivibration glove applications. Cell. Polym. 2005, 24, 253–268. [Google Scholar] [CrossRef]
- Scarpa, F.; Ciffo, L.G.; Yates, J.R. Dynamic properties of high structural integrity auxetic open cell foam. Smart Mater. Struct. 2003, 13, 49–56. [Google Scholar] [CrossRef]
- Duncan, O.; Foster, L.; Senior, T.; Alderson, A.; Allen, T. Quasi-static characterisation and impact testing of auxetic foam for sports safety applications. Smart Mater. Struct. 2016, 25, 054014. [Google Scholar] [CrossRef]
- Foster, L.; Peketi, P.; Allen, T.; Senior, T.; Duncan, O.; Alderson, A. Application of Auxetic Foam in Sports Helmets. Appl. Sci. 2018, 8, 354. [Google Scholar] [CrossRef]
- Allen, T.; Shepherd, J.; Hewage, T.A.M.; Senior, T.; Foster, L.; Alderson, A. Low-kinetic energy impact response of auxetic and conventional open-cell polyurethane foams. Phys. Status Solidi Basic Res. 2015, 252, 1631–1639. [Google Scholar] [CrossRef]
- Ge, C. A comparative study between felted and triaxial compressed polymer foams on cushion performance. J. Cell. Plast. 2013, 49, 521–533. [Google Scholar] [CrossRef]
- Lisiecki, J.; Błazejewicz, T.; Kłysz, S.; Gmurczyk, G.; Reymer, P.; Mikułowski, G. Tests of polyurethane foams with negative Poisson’s ratio. Phys. Status Solidi Basic Res. 2013, 250, 1988–1995. [Google Scholar] [CrossRef]
- Evans, K.E. The design of doubly curved sandwich panels with honeycomb cores. Compos. Struct. 1991, 17, 95–111. [Google Scholar] [CrossRef]
- Cross, T.M.; Hoffer, K.W.; Jones, D.P.; Kirschner, P.B.; Meschter, J.C. Auxetic Structures and Footwear with Soles Having Auxetic Structures. U.S. Patent No. 9,402,439, 19 March 2015. [Google Scholar]
- Alderson, K.L.; Fitzgerald, A.; Evans, K.E. The strain dependent indentation resilience of auxetic microporous polyethylene. J. Mater. Sci. 2000, 35, 4039–4047. [Google Scholar] [CrossRef]
- Chan, N.; Evans, K.E. Indentation resilience of conventional and auxetic foams. J. Cell. Plast. 1998, 34, 231–260. [Google Scholar] [CrossRef]
- Lakes, R.S.; Elms, K. Indentability of conventional and negative Poisson’s ratio foams. J. Compos. Mater. 1993, 27, 1193–1202. [Google Scholar] [CrossRef]
- Alderson, K.L.; Pickles, A.P.; Neale, P.J.; Evans, K.E. Auxetic polyethylene: The effect of a negative Poisson’s ratio on hardness. Acta Metall. Mater. 1994, 42, 2261–2266. [Google Scholar] [CrossRef]
- Argatov, I.I.; Sabina, F.J. Small-scale indentation of an elastic coated half-space: The effect of compliant substrate. Int. J. Eng. Sci. 2016, 104, 87–96. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Goodier, J.N. Theory of Elasticity, 3rd ed.; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Press Syndicate of the University of Cambridge: Cambridge, UK, 1997; pp. 67, 176–183, 259–264, 286, 301. 498p. [Google Scholar]
- Mills, N.J.; Fitzgerald, C.; Gilchrist, A.; Verdejo, R. Polymer foams for personal protection: Cushions, shoes and helmets. Compos. Sci. Technol. 2003, 63, 2389–2400. [Google Scholar] [CrossRef]
- Mills, N.J.; Zhu, H.X. The high strain compression of closed-cell polymer foams. J. Mech. Phys. Solids 1999, 47, 669–695. [Google Scholar] [CrossRef]
- Plant, D.; Leevers, P. Injection moldable rate stiffening re-entrant cell arrays for wearable impact protection. Polym. Eng. Sci. 2020, 60, 1546–1555. [Google Scholar] [CrossRef]
- Nakonieczna, P.; Wierzbicki, Ł.; Śladowska, B.; Leonowicz, M.; Lisiecki, J. Composites with impact absorption ability based on shear thickening fluids and auxetic foams. Compos. Theory Pract. 2017, 2, 67–72. [Google Scholar]
- Pastorino, P.; Scarpa, F.; Patsias, S.; Yates, J.R.; Haake, S.J.; Ruzzene, M. Strain rate dependence of stiffness and Poisson’s ratio of auxetic open cell PU foams. Phys. Status Solidi Basic Res. 2007, 244, 955–965. [Google Scholar] [CrossRef]
- Critchley, R.; Corni, I.; Wharton, J.A.; Walsh, F.C.; Wood, R.J.K.; Stokes, K.R. A review of the manufacture, mechanical properties and potential applications of auxetic foams. Phys. Status Solidi Basic Res. 2013, 250, 1963–1982. [Google Scholar] [CrossRef]
- Lakes, R.S. Foam Structures with a Negative Poisson’s Ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef] [PubMed]
- Duncan, O.; Allen, T.; Foster, L.; Senior, T.; Alderson, A. Fabrication, characterisation and modelling of uniform and gradient auxetic foam sheets. Acta Mater. 2017, 126, 426–437. [Google Scholar] [CrossRef]
- Chan, N.; Evans, K.E. Fabrication methods for auxetic foams. J. Mater. Sci. 1997, 32, 5945–5953. [Google Scholar] [CrossRef]
- Alderson, A.; Davies, P.J.; Alderson, K.I.M.L.; Smart, G.M. The Effects of Processing on the Topology and Mechanical Properties of Negative Poisson’s Ratio Foams. In Proceedings of the IMECE2005 2005 ASME International Mechanical Engineering Congress and Exposition IMECE200, Orlando, FL, USA, 5–11 November 2005; pp. 1–8. [Google Scholar]
- Duncan, O.; Clegg, F.; Essa, A.; Bell, A.M.T.; Foster, L.; Allen, T.; Alderson, A. Effects of Heat Exposure and Volumetric Compression on Poisson’s Ratios, Young’s Moduli, and Polymeric Composition During Thermo-Mechanical Conversion of Auxetic Open Cell Polyurethane Foam. Phys. Status Solidi 2019, 256, 1800393. [Google Scholar] [CrossRef]
- Choi, J.B.; Lakes, R.S. Nonlinear Analysis of the Poisson’s Ratio of Negative Poisson’s Ratio Foams. J. Compos. Mater. 1994, 29, 113–128. [Google Scholar] [CrossRef]
- Chan, N.; Evans, K.E. The mechanical properties of conventional and auxetic foams. Part I: Compression and tension. J. Cell. Plast. 1999, 35, 130–165. [Google Scholar]
- Martz, E.O.; Lee, T.; Lakes, R.S.; Goel, V.K.; Park, J.B. Re-entrant transformation methods in closed cell foams. Cell. Polym. 1996, 15, 229–249. [Google Scholar]
- Duncan, O.; Allen, T.; Birch, A.; Foster, L.; Hart, J.; Alderson, A. Effect of steam conversion on the cellular structure, Young’s modulus and negative Poisson’s ratio of closed cell foam. Smart Mater. Struct. 2020, 30, 015031. [Google Scholar] [CrossRef]
- Fan, D.; Li, M.; Qiu, J.; Xing, H.; Jiang, Z.; Tang, T. Novel Method for Preparing Auxetic Foam from Closed-Cell Polymer Foam Based on the Steam Penetration and Condensation Process. ACS Appl. Mater. Interfaces. 2018, 10, 22669–22677. [Google Scholar] [CrossRef] [PubMed]
- European Committee for Standardization. BS EN 13061:2009 Protective Clothing—Shin Guards for Association Football Players—Requirements and Test Methods. BS EN: 13061:2009. 2009. Available online: https://www.en-standard.eu/bs-en-13061-2009-protective-clothing.-shin-guards-for-association-football-players.-requirements-and-test-methods/ (accessed on 26 January 2021).
- European Committee for Standardization. BS 6183-3:2000-Protective Equipment for Cricketers. 2000. Available online: https://shop.bsigroup.com/ProductDetail/?pid=000000000019995423 (accessed on 26 January 2021).
- Ankrah, S.; Mills, N.J. Analysis of ankle protection in Association football. Sports Eng. 2004, 7, 41–52. [Google Scholar] [CrossRef]
- Critchley, R.; Smy, V.; Corni, I.; Wharton, J.A.; Walsh, F.C.; Robert, J.K.; Wood, R.J.; Stokes, K.R. Experimental and computation assessment of thermomechanical effects during auxetic foam fabrication. Sci. Rep. 2020, 10, 1–14. [Google Scholar] [CrossRef]
- Allen, T.; Hewage, T.; Newton-Mann, C.; Wang, W.; Duncan, O.; Alderson, A. Fabrication of Auxetic Foam Sheets for Sports Applications. Phys. Status Solidi Basic Res. 2017, 254, 1700596. [Google Scholar] [CrossRef]
- McDonald, S.A.; Dedreuil-Monet, G.; Yao, Y.T.; Alderson, A.; Withers, P.J. In situ 3D X-ray microtomography study comparing auxetic and non-auxetic polymeric foams under tension. Phys. Status Solidi Basic Res. 2011, 248, 45–51. [Google Scholar] [CrossRef]
- Pierron, F. Identification of Poisson’s ratios of standard and auxetic low-density polymeric foams from full-field measurements. J. Strain Anal. Eng. Des. 2010, 45, 233–253. [Google Scholar] [CrossRef]
- Duncan, O.; Alderson, A.; Allen, T. Fabrication, characterisation and analytical modelling of gradient auxetic closed cell foams. Smart Mater. Struct. 2021. [Google Scholar] [CrossRef]
- Lane, B.; Sherratt, P.; Hu, X.; Harland, A. Measurement of strain and strain rate during the impact of tennis ball cores. Appl. Sci. 2018, 8, 371. [Google Scholar] [CrossRef]
- Šleichrt, J.; Fíla, T.; Koudelka, P.; Adorna, M.; Falta, J.; Zlámal, P.; Glinz, J.; Neuhäuserová, M.; Doktor, T.; Mauko, A.; et al. Dynamic penetration of cellular solids: Experimental investigation using Hopkinson bar and computed tomography. Mater. Sci. Eng. A 2021, 800, 140096. [Google Scholar] [CrossRef]
- Reu, P.L.; Miller, T.J. The application of high-speed digital image correlation. J. Strain Anal. Eng. Des. 2008, 43, 673–688. [Google Scholar] [CrossRef]
- Allen, T.; Duncan, O.; Foster, L.; Senior, T.; Zampieri, D.; Edeh, V.; Alderson, A. Auxetic foam for snow-sport safety devices. Snow Sport. Trauma Saf. Proc. Int. Soc. Ski. Saf. 2016, 21. [Google Scholar] [CrossRef]
- Boba, K.; Bianchi, M.; McCombe, G.; Gatt, R.; Griffin, A.C.; Richardson, R.M.; Scarpa, F.; Hamerton, I.; Grima, J.N. Blocked shape memory effect in negative Poisson’s ratio polymer metamaterials. ACS Appl. Mater. Interfaces 2016, 8, 20319–20328. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Lu, W.; Scarpa, F.; Barton, D.; Lakes, R.S.; Zhu, Y.; Lang, Z.; Peng, H.X. Large stiffness thermoformed open cell foams with auxeticity. Appl. Mater. Today 2020, 20, 100775. [Google Scholar] [CrossRef]
- Bianchi, M.; Scarpa, F.; Smith, C.W. Shape memory behaviour in auxetic foams: Mechanical properties. Acta Mater. 2010, 58, 858–865. [Google Scholar] [CrossRef]
- Duncan, O.; Foster, L.; Senior, T.; Allen, T.; Alderson, A. A Comparison of Novel and Conventional Fabrication Methods for Auxetic Foams for Sports Safety Applications. Procedia Eng. 2016, 147, 384–389. [Google Scholar] [CrossRef]
- Pierron, F.; McDonald, S.A.; Hollis, D.; Fu, J.; Withers, P.J.; Alderson, A. Comparison of the mechanical behaviour of standard and auxetic foams by x-ray computed tomography and digital volume correlation. Strain 2013, 49, 467–482. [Google Scholar] [CrossRef]
- Bailly, N.; Petit, Y.; Desrosier, J.-M.; Laperriere, O.; Langlois, S.; Wagnac, E. Strain rate dependant behaviour of Vinyl Nitrile helmet foam in compression and combined compression and shear. Appl. Sci. 2020, 10, 8286. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duncan, O.; Bailly, N.; Allen, T.; Petit, Y.; Wagnac, E.; Alderson, A. Effect of Compressive Strain Rate on Auxetic Foam. Appl. Sci. 2021, 11, 1207. https://doi.org/10.3390/app11031207
Duncan O, Bailly N, Allen T, Petit Y, Wagnac E, Alderson A. Effect of Compressive Strain Rate on Auxetic Foam. Applied Sciences. 2021; 11(3):1207. https://doi.org/10.3390/app11031207
Chicago/Turabian StyleDuncan, Olly, Nicolas Bailly, Tom Allen, Yvan Petit, Eric Wagnac, and Andrew Alderson. 2021. "Effect of Compressive Strain Rate on Auxetic Foam" Applied Sciences 11, no. 3: 1207. https://doi.org/10.3390/app11031207
APA StyleDuncan, O., Bailly, N., Allen, T., Petit, Y., Wagnac, E., & Alderson, A. (2021). Effect of Compressive Strain Rate on Auxetic Foam. Applied Sciences, 11(3), 1207. https://doi.org/10.3390/app11031207





