A Fluid–Solid–Heat Coupling Analysis for Water-Lubricated Rubber Stern Bearing Considering the Deflection of Propeller Shaft
Abstract
1. Introduction
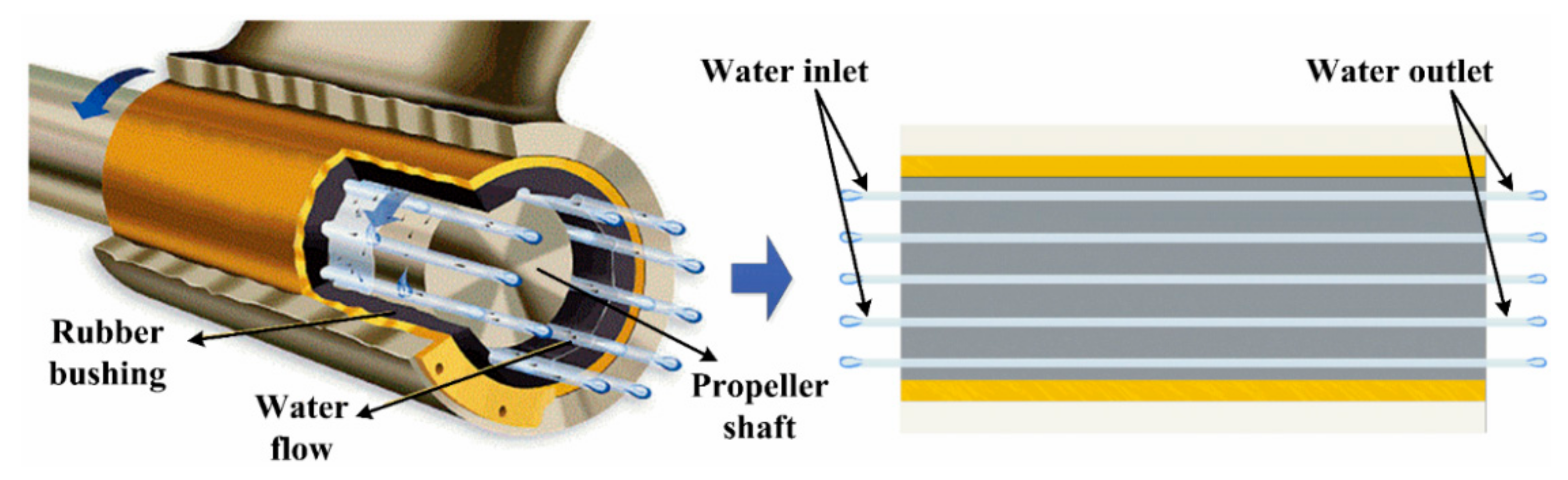
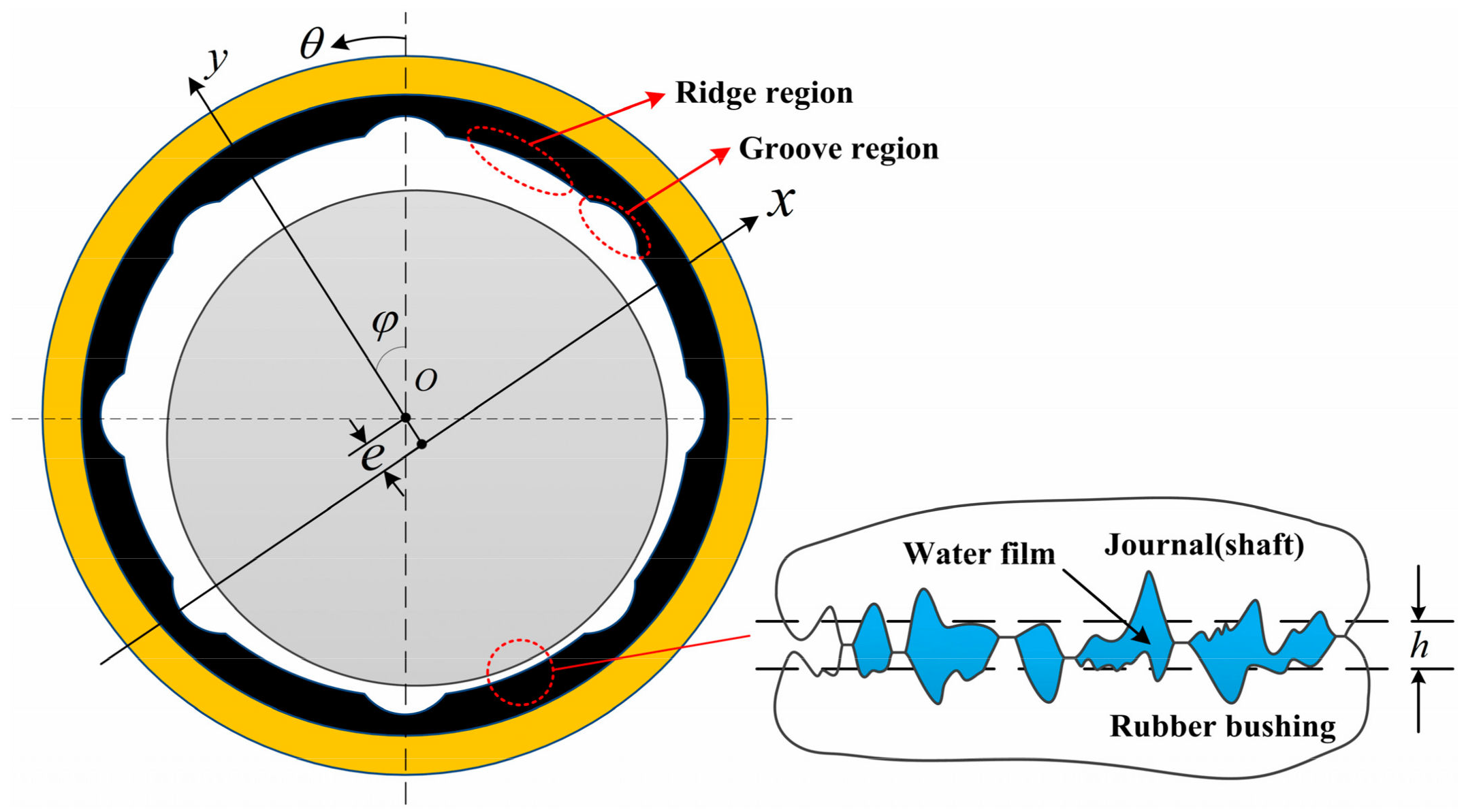
2. Numerical Models
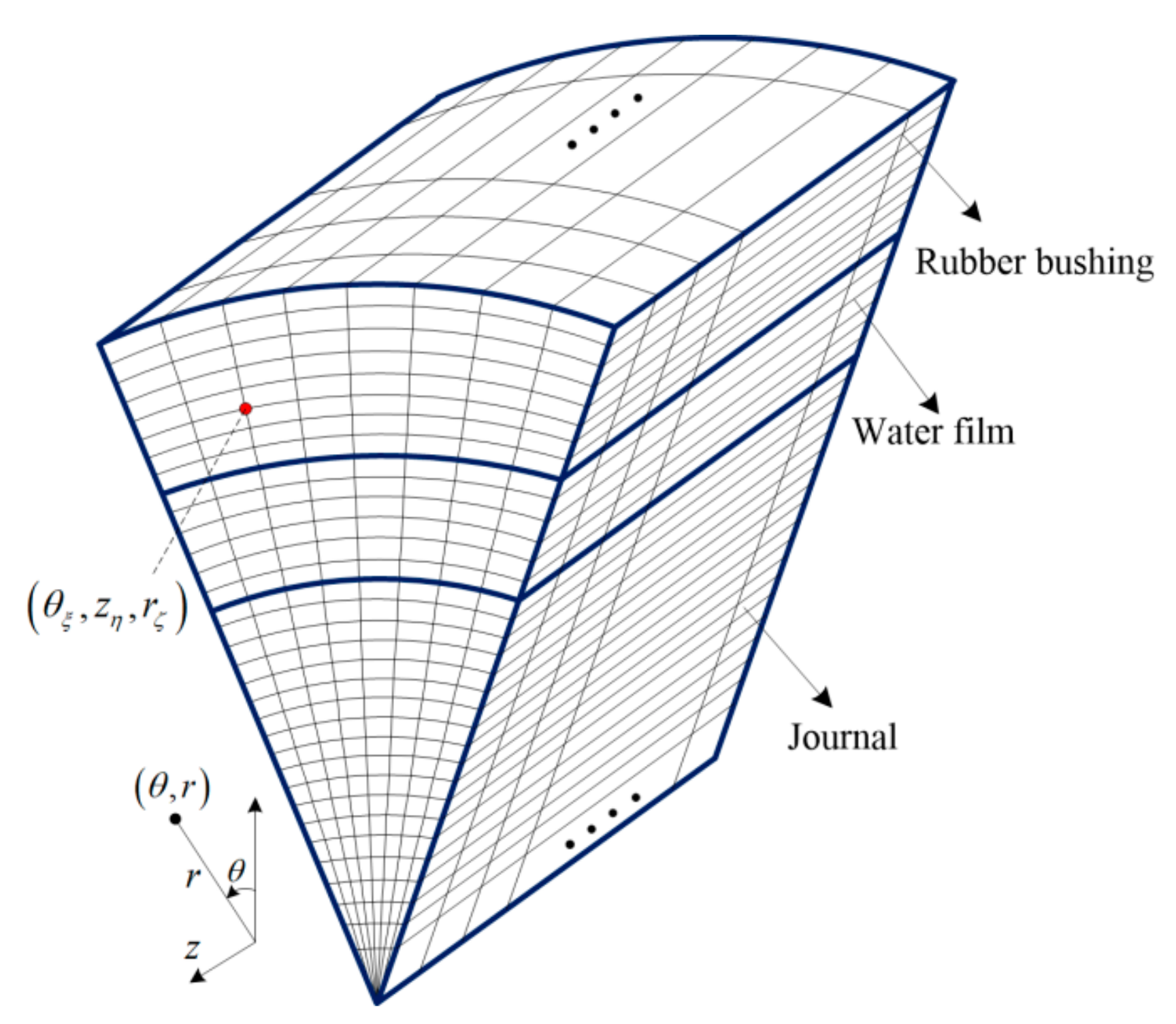
2.1. Governing Equation
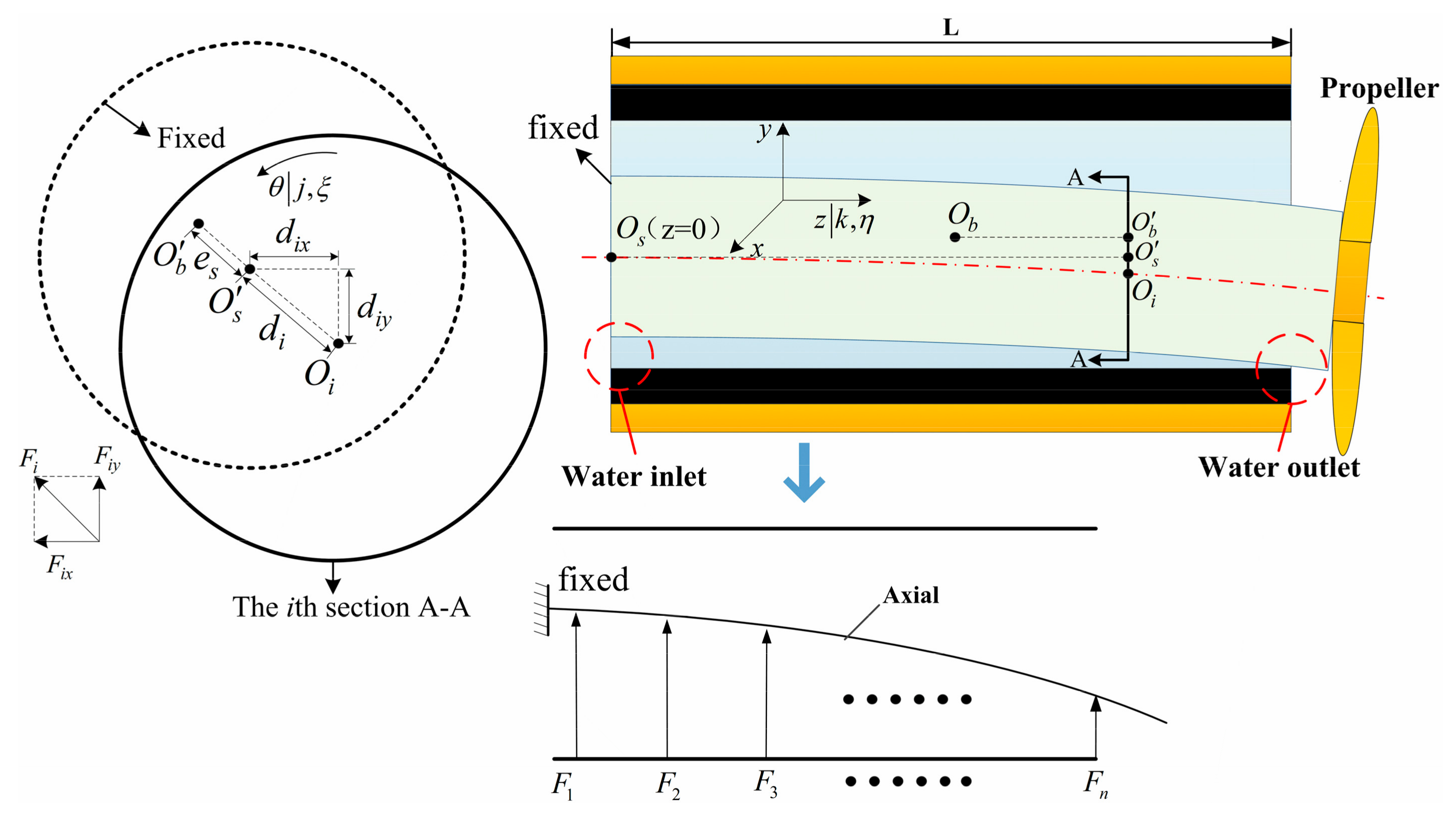
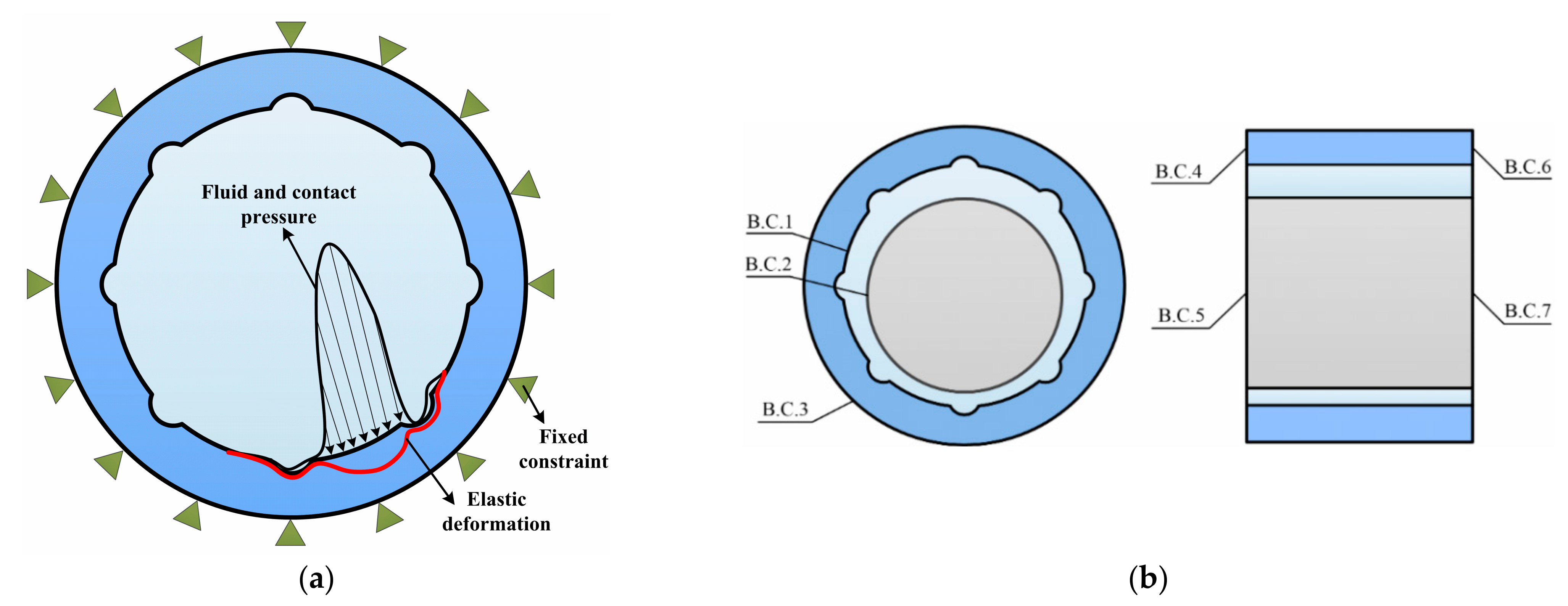
2.2. Boundary Condition
- (1)
- Internal boundary conditions: B.C.1 and B.C.2 represent the bearing–water and water–journal interfaces; which can be evaluated by the following Equation (22).
- (2)
- External boundary conditions: B.C.3 through B.C.7 are the external boundaries for the system and they must be specified. In the present thermal model, the convective boundary is applied to describe the thermal behavior at B.C.3–B.C.7, which is given by:
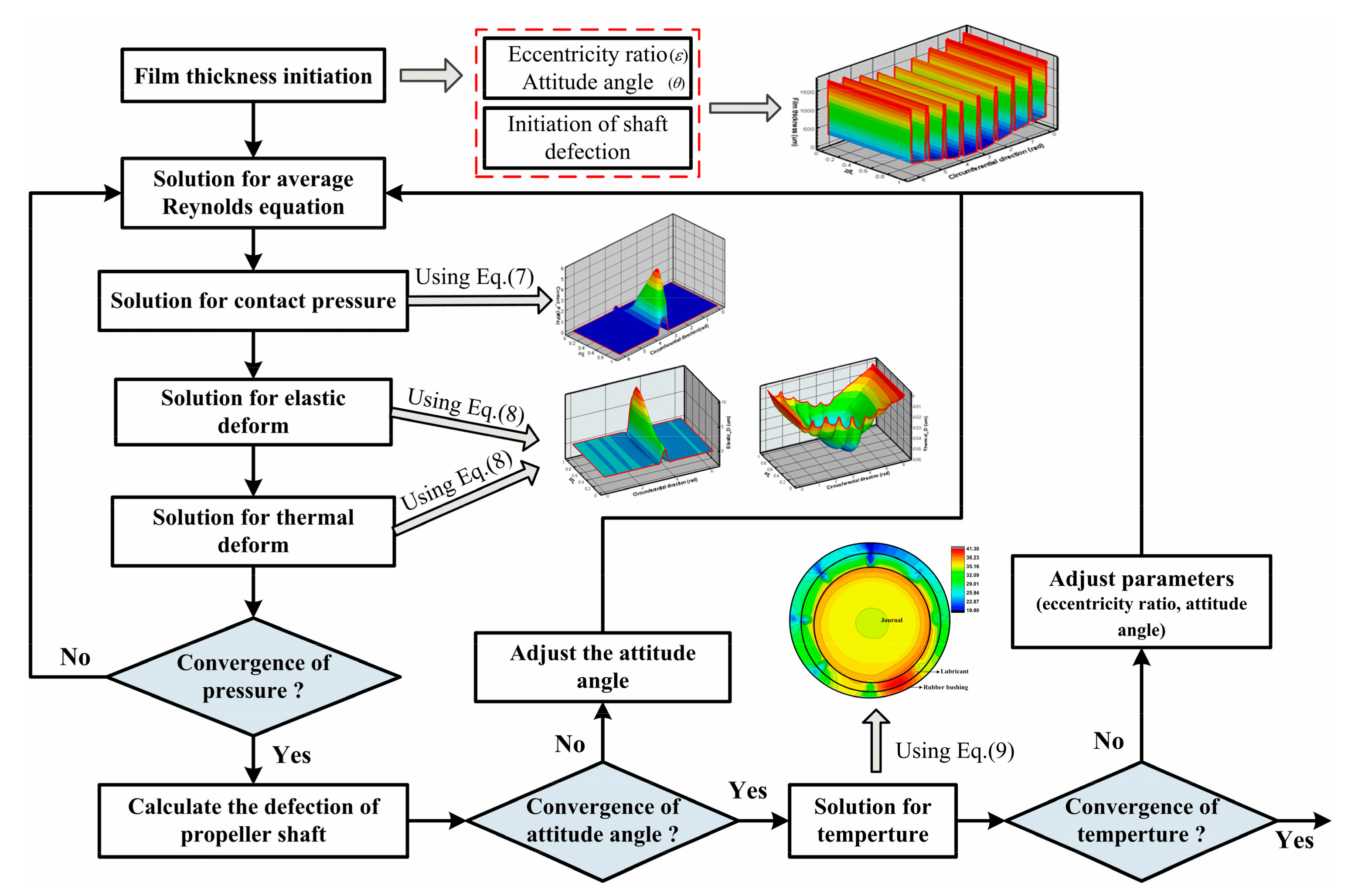
2.3. Numerical Algorithm
3. Results and Discussion
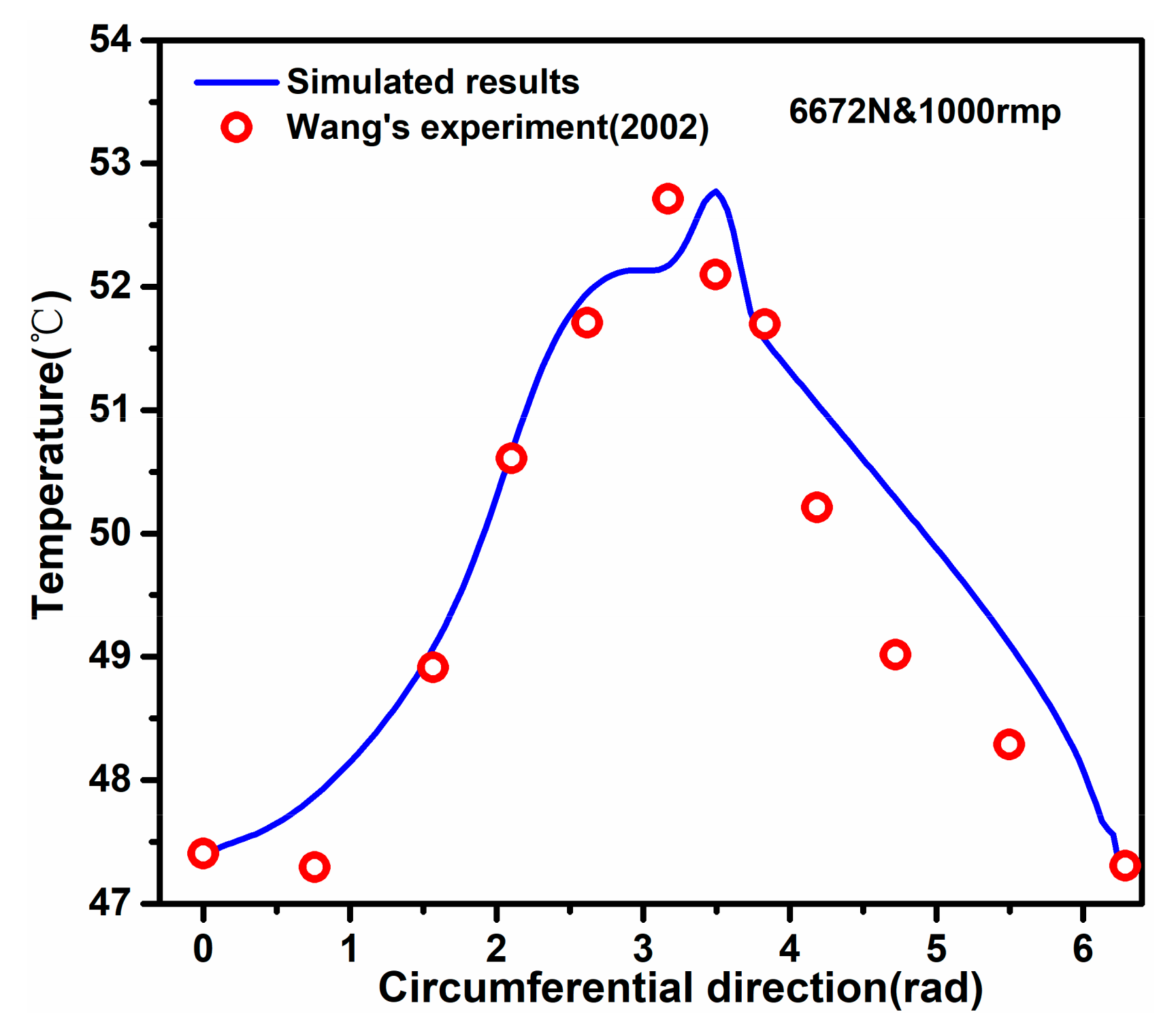
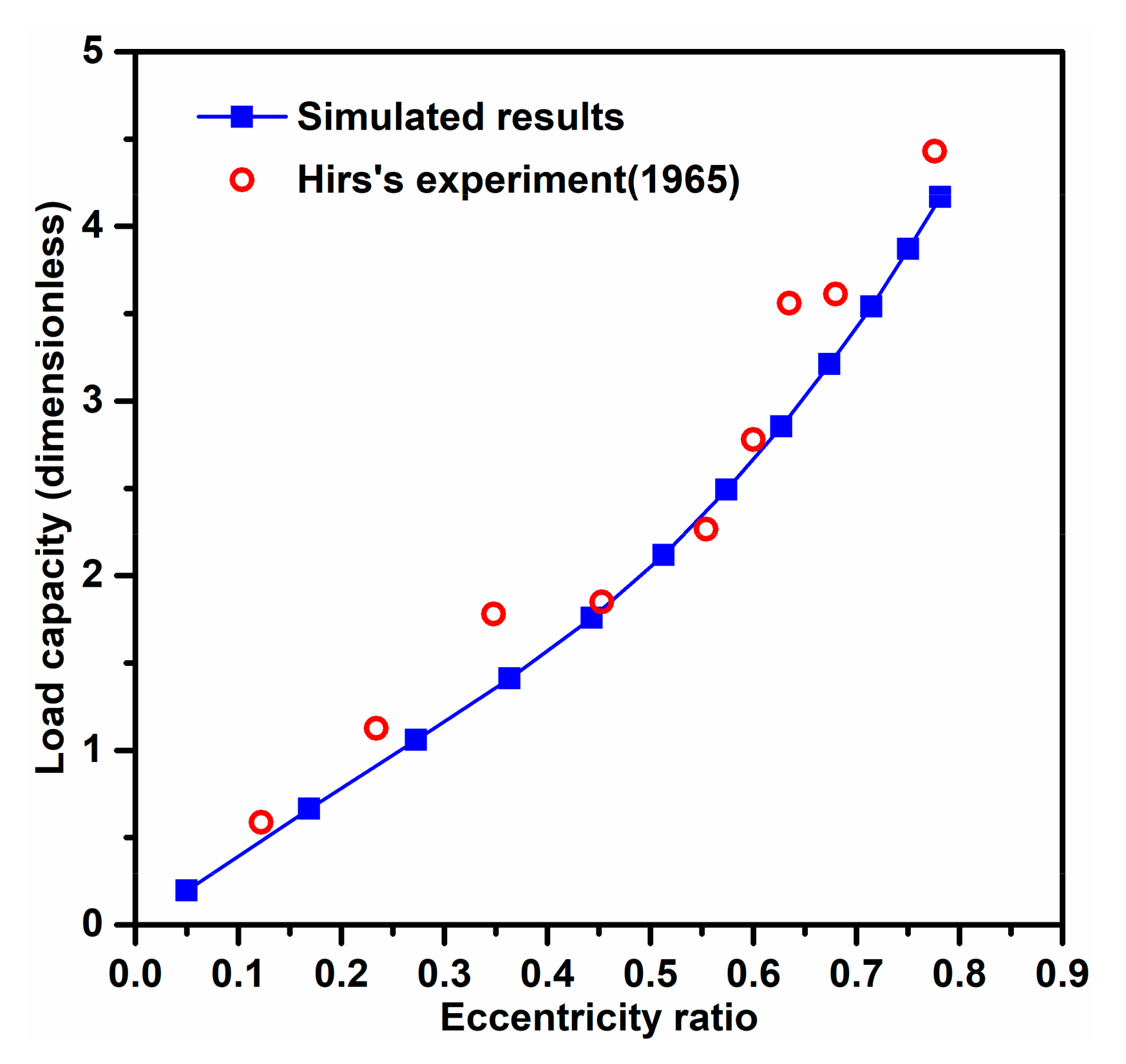
3.1. Model Validation
3.2. The Effects of Deflection of Propeller Shaft on Pressure and Deform Distribution
3.3. Thermal Analysis of the JWR System
3.4. Comparisons of FSH Coupling Performance
4. Conclusions
- (1)
- The temperature distribution and the load capacity obtained by the proposed model agree well with the experimental data given in the literature, thus validating that the presented model can be used to predict the FSH coupling performances of hydrodynamic bearings.
- (2)
- The asymmetric tribological performance distributions along the axial direction occur due to the propeller shaft deflection, including the hydrodynamic force, asperity contact, and temperature. The maximum asperity contact and temperature rise of the misaligned shaft are obviously larger than those of the aligned shaft. Furthermore, the temperature yielded by the water outlet is larger than that yielded by the water inlet for both the misaligned and aligned shaft cases.
- (3)
- Owing to the WLRSB having a larger length-to-diameter ratio compared with the general journal bearing, the misalignment caused by the deflection of the propeller shaft affects the FSH performance of the JWR system significantly, especially under a relatively heavy load condition. Furthermore, the aligned shaft case was found to be a proper substitute for a misaligned shaft case when the load capacity is smaller than 4 kN for the current simulation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| RB | Bearing radius, mm |
| L | Bearing width, mm |
| ph | Hydrodynamic pressure, MPa |
| ω | Angular velocity, rad/s |
| h | Lubrication gap, μm |
| φ | Flow/shear/contact factor |
| σ | Surface roughness, μm |
| ρ | Water density, kg/m3 |
| η | Water viscosity, Pa·s |
| hg | Groove depth, μm |
| δ | Deformation, μm |
| h0 | Average geometric gap, mm |
| C | Radial clearance, mm |
| ϕ | Attitude angle, rad |
| ε | Eccentricity ratio |
| e | Eccentricity |
| d | Shaft deflection, μm |
| F | Force, N |
| M | Self-weight of propeller, kg |
| E | Elastic modulus, MPa |
| I | Inertia moment, mm4 |
| D | Shaft diameter, mm |
| es | Eccentricity of end face |
| pc | Contact pressure, MPa |
| HB | Bearing hardness, Pa |
| β | Asperity curvature radius, μm |
| Ds | Asperity density |
| v | Velocity, m/s |
| GRE | Influence coefficient of elastic |
| GRT | Influence coefficient of thermal |
| V | Volume, mm3 |
| A | Area, mm2 |
| αJ | Coefficient of thermal expansion |
| νΒ | Bearing Poisson ratio |
| νJ | Journal Poisson ratio |
| Hd | Dimensionless lubrication gap |
| β | Asperity curvature, μm |
| δΒΕ | Elastic deformation, μm |
| δΤ0 | Thermal deformation, μm |
| GBE | Elastic influence coefficient |
| GBT | Thermal influence coefficient |
| ΔΤ | Temperature rise, °C |
| T | Temperature, °C |
| CP | Specific heat capacity, J/(kg·K) |
| Tc | Temperature of water inlet, °C |
| T∞ | Ambient temperature, °C |
| hh | Heat transfer coefficient, W/m·K |
| Rg | Groove radius, mm |
| Wg | Groove width, mm |
| ng | Groove number |
| Φ | Heat source, J |
| μc | Boundary friction coefficient |
| Ff | Friction force, N |
| k | Thermal conductivity, W/m·K |
| u | Linear velocity of journal, m/s |
| Subscripts: | |
| R, W, J | Rubber, water, journal |
| j, k | Circumference, axial node |
| θ, z, r | Circumference, axial, radial direction |
| x, y | Tangential, normal |
| s | Shear |
| h, c | Hydrodynamic, contact |
| E, T | Elastic, thermal |
| g | Groove |
References
- Roy, L.; Orndorff, J.R. Water-lubricated rubber bearings, history and new developments. Nav. Eng. J. 1985, 97, 39–52. [Google Scholar]
- Szeri, A.Z. Composite-film hydrodynamic bearings. Int. J. Eng. Sci. 2010, 48, 1622–1632. [Google Scholar] [CrossRef]
- Prakash, J.; Sinha, P. Lubrication theory for micropolar fluids and its application to a journal bearing. Int. J. Eng. Sci. 1975, 13, 217–232. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of Fluid Film Lubrication; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Zhang, Y.; Li, X.; Dong, C. A semianalytical approach to nonlinear fluid film forces of a hydrodynamic journal bearing with two axial grooves. Appl. Math. Model. 2019, 65, 318–332. [Google Scholar] [CrossRef]
- Litwin, W. Influence of local bush wear on water lubricated sliding bearing load carrying capacity. Tribol. Int. 2016, 103, 352–358. [Google Scholar] [CrossRef]
- Lin, J. The influences of longitudinal surface roughness on sub-critical and super-critical limit cycles of short journal bearings. Appl. Math. Model. 2014, 38, 392–402. [Google Scholar] [CrossRef]
- He, T.; Zou, D.; Lu, X. Mixed-lubrication analysis of marine stern tube bearing considering bending deformation of stern shaft and cavitation. Tribol. Int. 2014, 73, 108–116. [Google Scholar] [CrossRef]
- Kraker, A.D.; Ostayen, R.A.J.; Rixen, D.J. Calculation of Stribeck curves for (water) lubricated journal bearings. Tribol. Int. 2007, 40, 459–469. [Google Scholar] [CrossRef]
- Chan, C.; Han, Y.; Wang, Z. Exploration on a fast EHL computing technology for analyzing journal bearings with engineered surface textures. Tribol. Trans. 2014, 57, 206–215. [Google Scholar] [CrossRef]
- Han, Y.; Chan, C.; Wang, Z. Effects of shaft axial motion and misalignment on the lubrication performance of journal bearings via a fast mixed EHL computing technology. Tribol. Trans. 2015, 58, 247–259. [Google Scholar] [CrossRef]
- Lv, F.; Rao, Z.; Na, T. Mixed-lubrication analysis of thin polymer film overplayed metallic marine stern bearing considering wall slip and journal misalignment. Tribol. Int. 2017, 109, 390–397. [Google Scholar] [CrossRef]
- Pu, W.; Wang, J.; Zhang, Y. A Theoretical analysis of the mixed elastohydrodynamic lubrication in elliptical contacts with an arbitrary entrainment angle. J. Tribol. Trans. ASME 2014, 136, 041505. [Google Scholar] [CrossRef]
- Majumdar, B.C.; Saha, A.K. Temperature distribution in oil journal bearings. Wear 1974, 28, 259–266. [Google Scholar] [CrossRef]
- Bouyer, J.; Fillon, M. An experimental analysis of misalignment effects on hydrodynamic plain journal bearing performances. J. Tribol. Trans. ASME 2002, 124, 313–319. [Google Scholar] [CrossRef]
- Pierre, I.; Bouyer, J.; Fillon, M. Thermohydrodynamic behaviour of misaligned plain journal bearings: Theoretical and experimental approaches. ASLE Trans. 2004, 47, 594–604. [Google Scholar]
- Mitsui, J.; Hori, Y.; Tanaka, M. An experimental investigation on the temperature distribution in circular journal bearings. J. Tribol. Trans. ASME 1986, 53, 621–627. [Google Scholar] [CrossRef]
- Fitzgerald, M.K.; Neal, B. Temperature distributions and heat transfer in journal bearings. J. Tribol. Trans. ASME 1992, 114, 122–130. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C.; Wang, Q. A mixed-TEHD analysis and experiment of journal bearings under severe operating conditions. Tribol. Int. 2002, 35, 395–407. [Google Scholar] [CrossRef]
- Yadav, J.S.; Kapur, V.K. On the viscosity variation with temperature and pressure in thrust bearing. Int. J. Eng. Sci. 1981, 19, 269–277. [Google Scholar] [CrossRef]
- Sahu, M.; Sarangi, M.; Majumdar, B.C. Thermohydrodynamic analysis of herringbone-grooved journal bearings. Tribol. Int. 2006, 39, 1395–1404. [Google Scholar] [CrossRef]
- Sun, J.; Deng, M.; Fu, Y. Thermohydrodynamic lubrication analysis of misaligned plain journal bearing with rough surface. J. Tribol. Trans. ASME 2010, 132, 011704–011708. [Google Scholar] [CrossRef]
- Mishra, P.C. Mathematical modeling of stability in rough elliptic bore misaligned journal bearing considering thermal and non-Newtonian effects. Appl. Math. Model. 2013, 37, 5896–5912. [Google Scholar] [CrossRef]
- Lin, X.; Jiang, S.; Zhang, C. Thermohydrodynamic analysis of high speed water-lubricated spiral groove thrust bearing considering effects of cavitation, inertia and turbulence. Tribol. Int. 2018, 119, 645–658. [Google Scholar] [CrossRef]
- Hoon, K.S.; Won, L.S.; Sangmok, H. Numerical investigation of thermal characteristics of spray cooling with minimum quantity lubrication in milling process. Appl. Math. Model. 2018, 65, 137–147. [Google Scholar]
- Pu, W.; Wang, J.; Zhu, D. Friction and flash temperature prediction of mixed lubrication in elliptical contacts with arbitrary velocity vector. Tribol. Int. 1999, 99, 38–46. [Google Scholar] [CrossRef]
- Li, Y.; Ji, Z.; Yang, L. Thermal-fluid-structure coupling analysis for valve plate friction pair of axial piston pump in electrohydrostatic actuator (EHA) of aircraft. Appl. Math. Model. 2017, 47, 839–858. [Google Scholar] [CrossRef]
- Feng, H.; Jiang, S.; Ji, A. Investigations of the static and dynamic characteristics of water-lubricated hydrodynamic journal bearing considering turbulent, thermohydrodynamic and misaligned effects. Tribol. Int. 2019, 130, 245–260. [Google Scholar] [CrossRef]
- Wodtke, M.; Litwin, W. Water-lubricated stern tube bearing-experimental and theoretical investigations of thermal effects. Tribol. Int. 2021, 153, 106608. [Google Scholar] [CrossRef]
- Litwin, W.; Olszewski, A.; Wodtke, M. Influence of shaft misalignment on water lubricated turbine sliding bearings with various bush modules of elasticity. Key Eng. Mater. 2011, 490, 128–134. [Google Scholar] [CrossRef]
- Mallya, R.; Shenoy, S.B.; Pai, R. Steady state characteristics of misaligned multiple axial groove water-lubricated journal bearing. J. Eng. Tribol. 2014, 229, 712–722. [Google Scholar] [CrossRef]
- Wu, C.; Zheng, L. An average reynolds equation for partial film lubrication with a contact factor. J. Tribol. Trans. ASME 1989, 111, 83–90. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef]
- Xiang, G.; Han, Y.; He, T. Wear and fluid-solid-thermal transient coupled model for journal bearings. Appl. Math. Model. 2020, 85, 19–45. [Google Scholar] [CrossRef]
- Hirs, G.G. The load capacity and stability characteristics of hydrodynamic grooved journal bearings. ASLE Trans. 1965, 8, 296–305. [Google Scholar] [CrossRef]
- Zhou, G.; Wang, J.; Han, Y. An experimental study on film pressure circumferential distribution of water-lubricated rubber bearings with multiple grooves. ASLE Trans. 2016, 60, 385–391. [Google Scholar] [CrossRef]

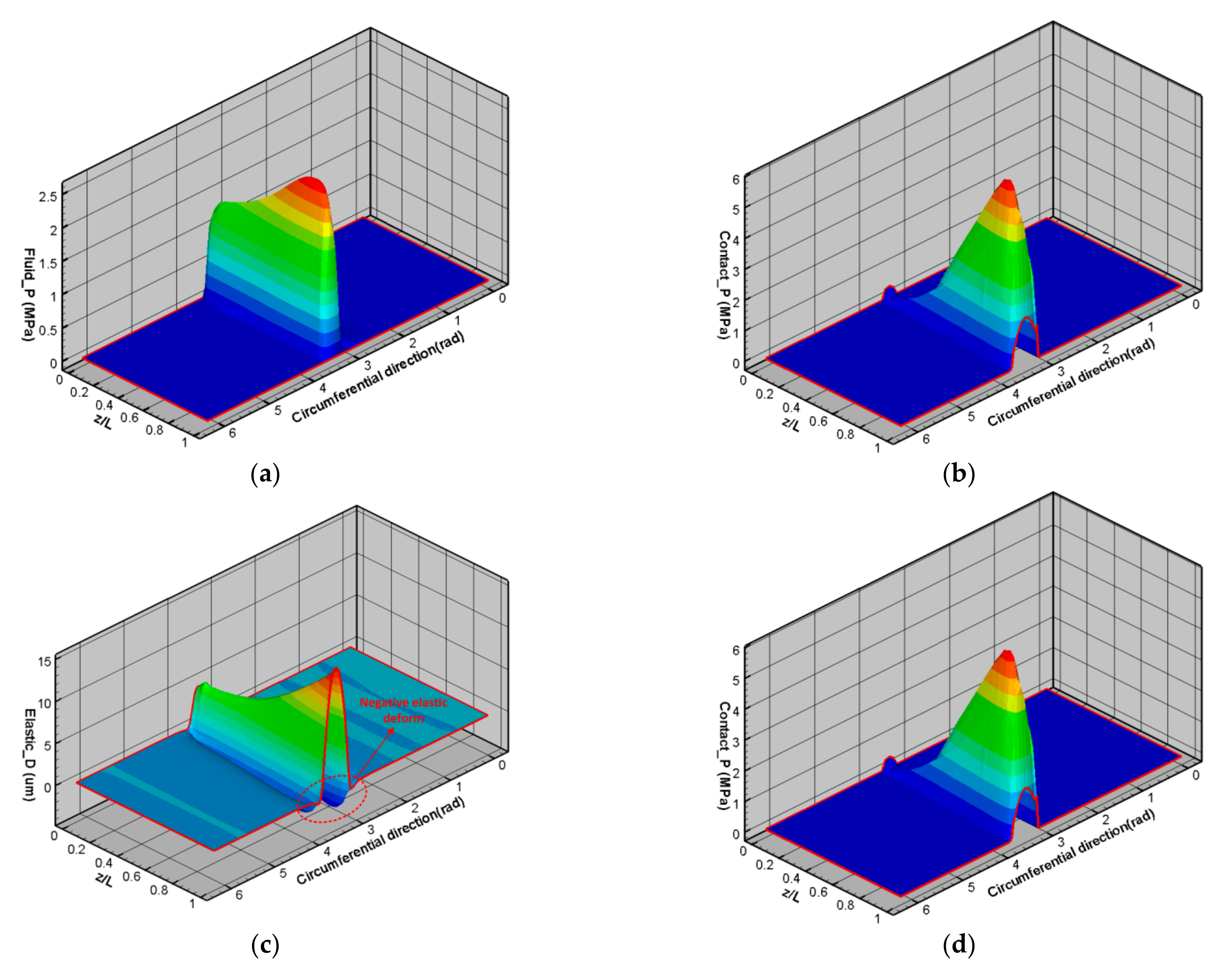
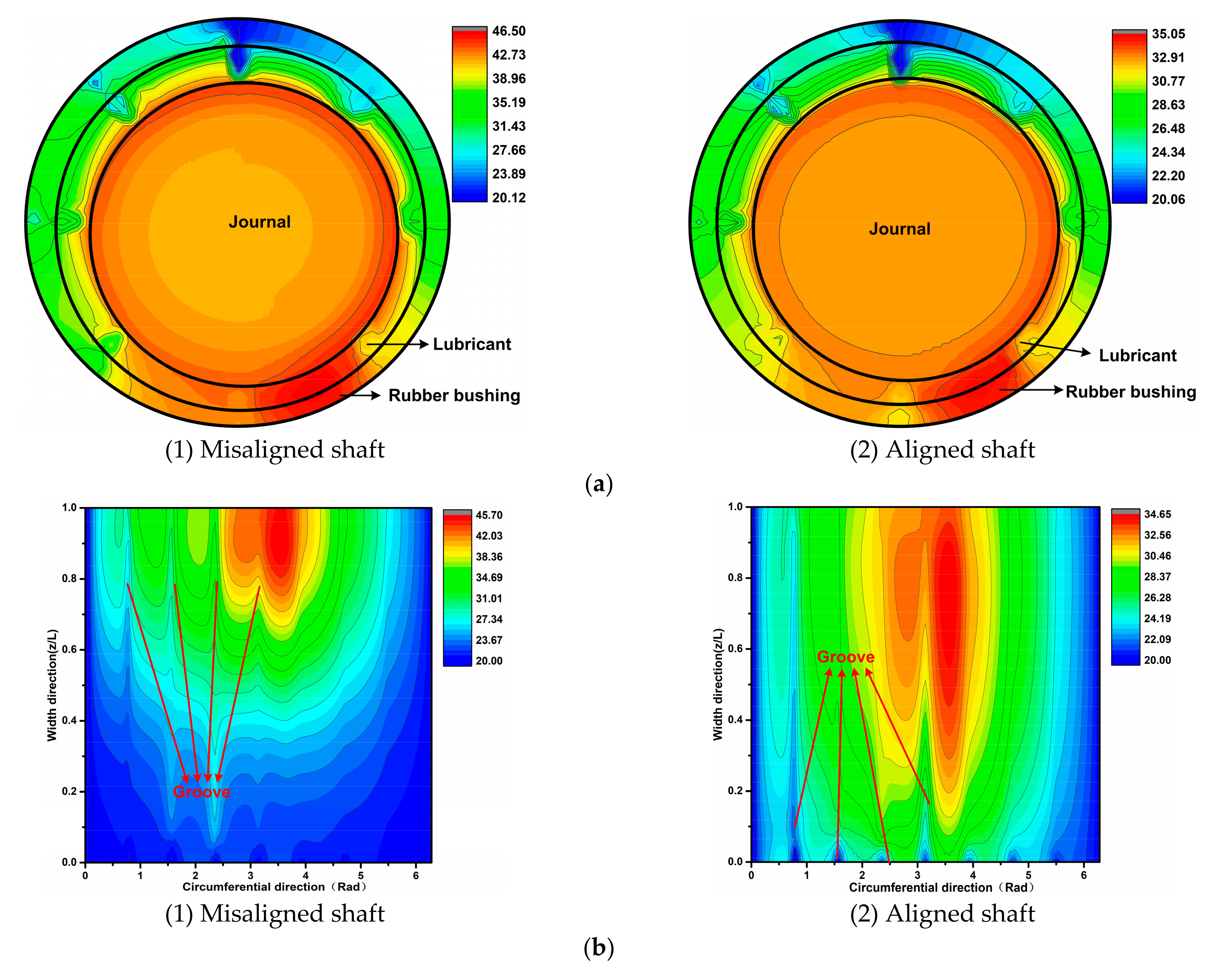

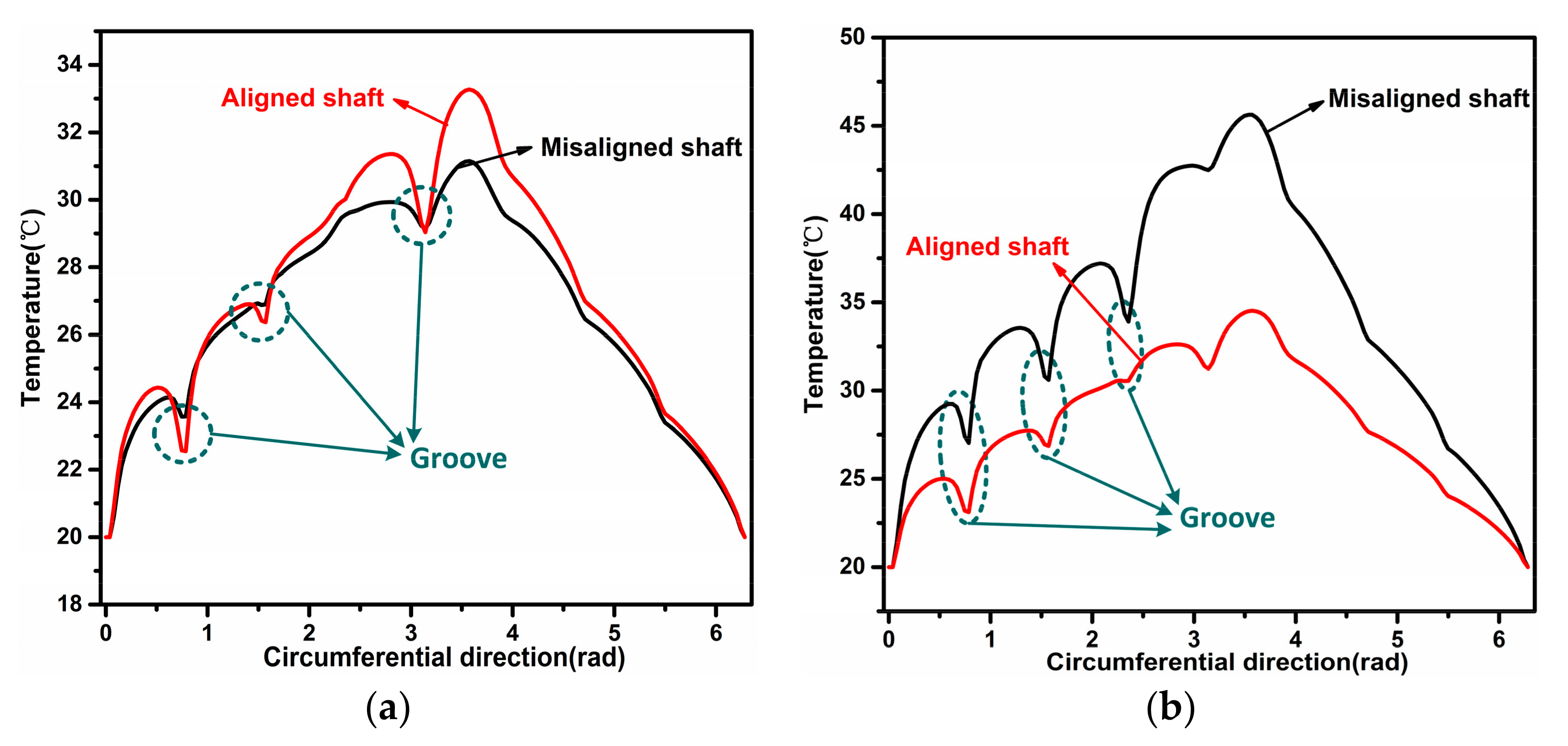


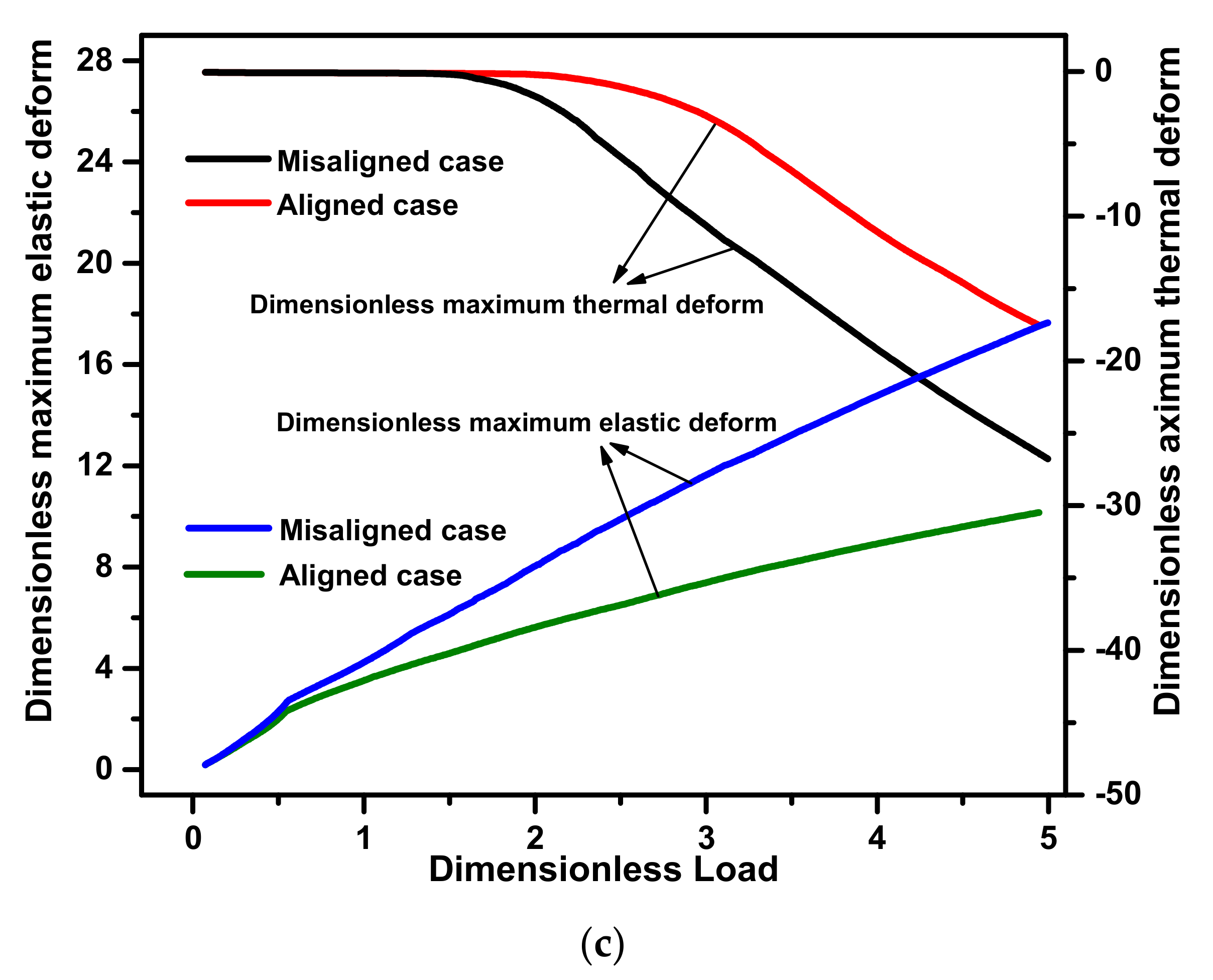

| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Inner radius/R1 | 45 mm | External force/M | 10 kN |
| Outer radius/R2 | 50 mm | Bearing density/ρR | 1500 kg/m3 |
| Clearance/C | 0.15 mm | Rubber thermal conductivities/kR | 0.288 W/(m·K) |
| Bearing width/L | 160 mm | Rubber specific heat/CPR | 1700 J/(kg·K) |
| Groove radius/Rg | 2.5 mm | Rubber thermal expansion/TR | 121 µm/(m·K) |
| Groove width/Wg | 6 mm | Water viscosity/η | 8.944 × 10−4 Pa·s |
| Groove number/ng | 8 | Water density/ρW | 1000 kg/m3 |
| Journal elastic modulus/EJ | 210 GPa | Water thermal conductivities/kW | 0.61 W/(m·K) |
| Journal Poisson ratio/νJ | 0.3 | Water specific heat/CPW | 4200 J/(kg·K) |
| Journal density/ρJ | 7800 kg/m3 | Heat transfer coefficient/hh | 80 W(m2·K) |
| Journal thermal conductivities/kJ | 50 W/(m·K) | Inlet temperature/Tc | 20 °C |
| Journal specific heat/CPJ | 460 J/(m·K) | Ambient temperature/T∞ | 20 °C |
| Journal thermal expansion/TJ | 11.9 µm/(m·K) | Friction coefficient/μC | 0.15 |
| Bearing elastic modulus/ER | 7.85 × 108 Pa | Journal roughness/σJ | 0.5 µm |
| Bearing Poisson ratio/νR | 0.47 | Rubber roughness/σR | 0.6 µm |
| Bearing hardness/HB | 5 × 107 Pa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, Y.; Tang, T.; Xiang, G.; Jia, H. A Fluid–Solid–Heat Coupling Analysis for Water-Lubricated Rubber Stern Bearing Considering the Deflection of Propeller Shaft. Appl. Sci. 2021, 11, 1170. https://doi.org/10.3390/app11031170
Han Y, Tang T, Xiang G, Jia H. A Fluid–Solid–Heat Coupling Analysis for Water-Lubricated Rubber Stern Bearing Considering the Deflection of Propeller Shaft. Applied Sciences. 2021; 11(3):1170. https://doi.org/10.3390/app11031170
Chicago/Turabian StyleHan, Yanfeng, Ting Tang, Guo Xiang, and Hang Jia. 2021. "A Fluid–Solid–Heat Coupling Analysis for Water-Lubricated Rubber Stern Bearing Considering the Deflection of Propeller Shaft" Applied Sciences 11, no. 3: 1170. https://doi.org/10.3390/app11031170
APA StyleHan, Y., Tang, T., Xiang, G., & Jia, H. (2021). A Fluid–Solid–Heat Coupling Analysis for Water-Lubricated Rubber Stern Bearing Considering the Deflection of Propeller Shaft. Applied Sciences, 11(3), 1170. https://doi.org/10.3390/app11031170






