Implementation of AHP Methodology for the Evaluation and Selection Process of a Reverse Engineering Scanning System
Abstract
1. Introduction
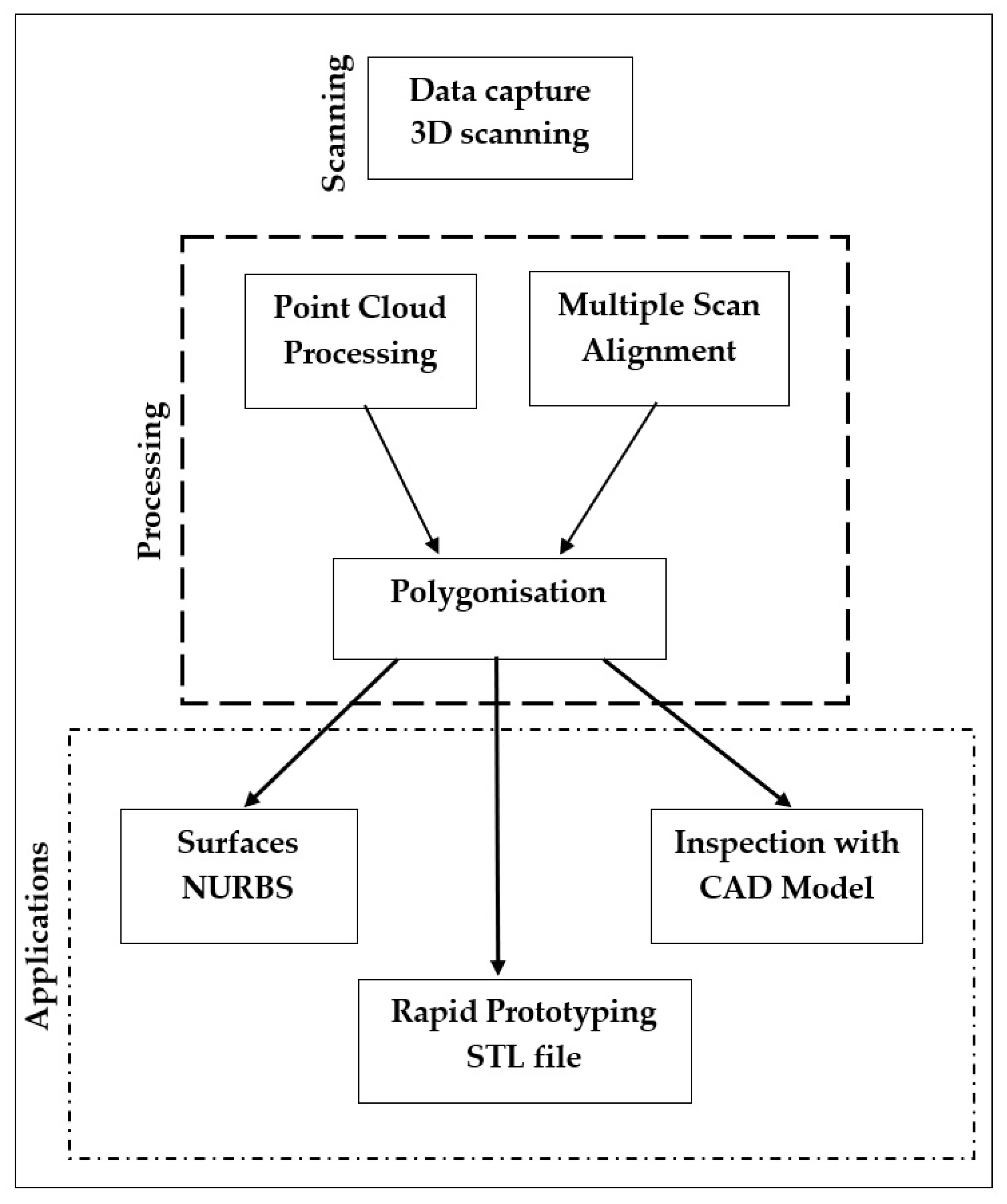
2. Materials and Methods
- MetraSCAN 70 (a1);
- MetraSCAN 70-R (a2);
- HandyPROBE (a3);
- Nikon XC65Dx(a4);
- Nikon LC60Dx (a5);
- Nikon LC15Dx (a6);
- Metronor DUO (a7);
- ATOS Triple Scan (a8).
2.1. Pairwise Comparison of Expert Evaluation Criteria
- Degree of education f1;
- Practical experience with RE systems f2;
- Theoretical knowledge in the field of quality inspection f3;
- Economic knowledge f4.
2.2. Pairwise Comparison of Experts According to Individual Criteria
3. Results
4. Discussion
| Algorithm 1. MATLAB program |
| [x,xn] = ahp(A), Where x is a proprietary vector of matrix A, xn is a proprietary vector of matrix A (x/sum x) and alfa is a level of consistence function [x,xn] = ahp(A,alfa) % Check that the matrix is entered correctly for i = 1:size(A,1) for j = 1:size(A,1) if i~= j if A(j,i) == A(i,j) & A(j,i) ~= 1; str=[‘The matrix is misspelled: element a_’… ,num2str(i),num2str(j),’ ‘,’=‘,’ ‘… ,’a_’,num2str(j),num2str(i)]; disp(str) return; elseif A(j,i) ~= 1/A(i,j) str = [‘The matrix is misspelled: element a_’… ,num2str(i),num2str(j),’ ‘,’a’,’ ‘… ,’a_’,num2str(j),num2str(i),’ ‘,… ‘ not in the desired shape a_ij = 1/a_ji’]; disp(str) return; end end end % Check of matrix A consistency. if eigs(A,1)<= size(A,1) + alfa*(1.7699*size(A) − 4.3513) % Calculation of eigenvector of matrix (x) and the eigenvalue vector % matrix (xn) according formula (A − lambda*J)x = 0 x = (A − eigs(A,1)*eye(size(A,1))); x = [1;inv(x(2:size(A),2:size(A)))*(−A(2:size(A),1))]; xn = x/sum(x); else str = [‘Matrix on level alfa=‘,num2str(alfa),’ ‘,’ is not consistent’ ]; disp(str) end |
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dúbravčík, M.; Kender, S. Application of Reverse Engineering Techniques in Mechanics System Services. Procedia Eng. 2012, 48, 96–104. [Google Scholar] [CrossRef]
- Buonamici, F.; Carfagni, M.; Furferi, R.; Governi, L.; Lapini, A.; Volpe, Y. Reverse engineering of mechanical parts: A template-based approach. J. Comput. Des. Eng. 2017, 5, 145–159. [Google Scholar] [CrossRef]
- Moon, S.; Ko, K. A point projection approach for improving the accuracy of the multilevel B-spline approximation. J. Comput. Des. Eng. 2017, 5, 173–179. [Google Scholar] [CrossRef]
- Rysiński, J.; Wrobel, I. Diagnostics of machine parts by means of reverse engineering procedures. Adv. Mech. Eng. 2015, 7, 1687814015584543. [Google Scholar] [CrossRef]
- Docchio, F.; Sansoni, G.; Trebeschi, M. Inspection, 3D modelling, and rapid prototyping of cultural heritage by means of a 3D optical digitiser. Opt. Methods Arts Archaeol. 2005, 5857, 58570D. [Google Scholar] [CrossRef]
- Pandilov, Z.; Betim, S.; Dejan, S. Reverse engineering an effective tool for design and development of mechanical parts. Acta Tech. Corviniensis–Bull. Eng. 2018, 11, 113–118. [Google Scholar]
- Atanasova-Pacemska, T.P.; Lapevski, M.; Timovski, R. Analytical hierarchical process (ahp) method application in the process of selection and evaluation. In Proceedings of the UNITECH—International Scientific Conference, Gabrovo, Bulgaria, 21–22 November 2014; Volume 14. [Google Scholar]
- Buonamici, F.; Carfagni, M.; Furferi, R.; Governi, L.; Lapini, A.; Volpe, Y. Reverse engineering modeling methods and tools: A survey. Comput. Des. Appl. 2017, 15, 443–464. [Google Scholar] [CrossRef]
- Eldad, E. Reversing: Secrets of Reverse Engineering; Wiley Publishing, Inc.: Indianapolis, ID, USA, 2015; ISBN 0-7645-7481-7. [Google Scholar]
- Thompson, W.; Owen, J.; Germain, H.D.S.; Stark, S.; Henderson, T. Feature-based reverse engineering of mechanical parts. IEEE Trans. Robot. Autom. 1999, 15, 57–66. [Google Scholar] [CrossRef][Green Version]
- Kumar, A.; Jain, P.; Pathak, P. Conception of Part Reconstruction: Integration of Non-Contact Scanning and Rapid Prototyping. In Proceedings of the Conference AM-2014, Bengaluru, India, 1–2 September 2014. [Google Scholar]
- Prochazkova, J.; Procházka, D.; Landa, J. Sharp Feature Detection as a Useful Tool in Smart Manufacturing. ISPRS Int. J. Geo-Inf. 2020, 9, 422. [Google Scholar] [CrossRef]
- Miądlicki, K.; Jasiewicz, M.; Gołaszewski, M.; Królikowski, M.; Powałka, B. Remanufacturing System with Chatter Suppression for CNC Turning. Sensors 2020, 20, 5070. [Google Scholar] [CrossRef]
- Raut, L.; Barai, G.; Shete, S. Design and development of a component by reverse engineering. Int. J. Res. Eng.Technol. 2015, 4, 539–546. [Google Scholar]
- Ivana, R.; Zuzana, M. The Analysis of AHP method and its potential use in logistics. Acta Montanistica Slovaca 2009, 14, 103–112. [Google Scholar]
- Gospodarek, M.; Rybarczyk, P.; Szulczyński, B.; Gębicki, J. Comparative Evaluation of Selected Biological Methods for the Removal of Hydrophilic and Hydrophobic Odorous VOCs from Air. Processes 2019, 7, 187. [Google Scholar] [CrossRef]
- Ruiz-Ramos, J.; Marino, A.; Boardman, C.; Suarez, J. Continuous Forest Monitoring Using Cumulative Sums of Sentinel-1 Timeseries. Remote Sens. 2020, 12, 3061. [Google Scholar] [CrossRef]
- Koczkodaj, W.W.; Szybowski, J.; Wajch, E. Inconsistency indicator maps on groups for pairwise comparisons. Int. J. Approx. Reason. 2016, 69, 81–90. [Google Scholar] [CrossRef]
- Leal, J.E. AHP-express: A simplified version of the analytical hierarchy process method. MethodsX 2019, 7, 100748. [Google Scholar] [CrossRef] [PubMed]
- Freerk, A.L. Multi-Criteria Decision Analysis via Ratio and Difference Judgement; Applied Optimization; Springer: Boston, MA, USA, 1999. [Google Scholar] [CrossRef]
- Viana, V.R.; Ipma-B, P. Using the Analytic Hierarchy Process (AHP) to Select and Prioritize Projects in a Portfolio; PMI Global Congress: Washington, DC, USA, 2010; pp. 1–12. [Google Scholar]
- Podvezko, V.; Mitkus, S.; Trinkūniene, E. Complex evaluation of contracts for construction. J. Civ. Eng. Manag. 2010, 16, 287–297. [Google Scholar] [CrossRef]
- Kim, S.; Kim, B. A Decision-Making Model for Adopting Al-Generated News Articles: Preliminary Results. Sustainability 2020, 12, 7418. [Google Scholar] [CrossRef]
- Kristbaum, J.P.; Ciarallo, F.W. Strategic Decision Facilitation: Supporting Critical Assumptions of the Human in Empirical Modeling of Pairwise Value Comparisons. Systems 2020, 8, 30. [Google Scholar] [CrossRef]
- Derrek, P.H.; Neda, J.; Sarah, E.; Medland, P.M. Thompson, Chapter Nine-Continuous Inflation Analysis: A Threshold-Free Method to Estimate Genetic Overlap and Boost Power in Imaging Genetics; Adrian, V., Dalca, N., Batmanghelich, K., Li, S., Mert, R.S., Eds.; Imaging Genetics; Academic Press: Cambridge, MA, USA, 2018; pp. 147–162. ISBN 9780128139684. [Google Scholar] [CrossRef]
- Nordstokke, D.; Stelnicki, A.M. Pairwise Comparisons. In Encyclopedia of Quality of Life and Well Being Research; Michalos, A.C., Ed.; Springer: Dordrecht, The Netherlands, 2014. [Google Scholar] [CrossRef]
- Sébastien, R.; Laroche, F. Durupt Alexandre, Bernard Alain, Knowledge Based Reverse Engineering Methodology. In Proceedings of the ASME 2012 11th Biennial Conference on Engineering Systems Design and Analysis, ESDA 2012, Nantes, France, 2–4 July 2012. [Google Scholar] [CrossRef]
- Alexander, M. Manažérstvo Kvality: História, Koncepty, Metódy, 1st ed.; Epos: 2006; p. 752. ISBN 80-8057-656-4. Available online: https://books.google.com.hk/books/about/Mana%C5%BE%C3%A9rstvo_kvality.html?id=85u2AAAACAAJ&redir_esc=y (accessed on 14 December 2021).
- Creath, K.; Wyant, J.C. Moiré and Fringe Projection Techniques. In Optical Shop Testing, 2nd ed.; Malacara, D., Ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1992; pp. 653–685. [Google Scholar]
- Zalai, K.; Kalafutová, E.; Šnircová, J. Financial and Economic Analysis of the Company; Sprint: Bratislava, Slovensko, 2002; p. 305. ISBN 80-88848-94-6. [Google Scholar]
- Várady, T.; Martin, R.R.; Cox, J. Reverse engineering of geometric models—An introduction. Comput. Des. 1997, 29, 255–268. [Google Scholar] [CrossRef]
- Svetlík, J.; Demeč, P. Methods of Identifying the Workspace of Modular Serial Kinematic Structures. Appl. Mech. Mater. 2013, 309, 75–79. [Google Scholar] [CrossRef]
- Alonso, J.A. Lamata Teresa, Consistency in the analytic hierarchy process: A new approach. Int. J. Uncertain. Fuzziness Knowl.-Based Syst. 2006, 14, 445–459. Available online: http://hera.ugr.es/doi/16515833.pdf (accessed on 10 November 2021).
- Raja, V.; Fernandes, K.J. Fernandes, Reverse Engineering—An Industrial Perspective; Springer: London, UK, 2008; ISBN 978-1-84628-855-5. [Google Scholar] [CrossRef]
- Park, J.; DeSouza, G.N. 3-D Modeling of Real-World Objects Using Range and Intensity Images. In Machine Learning and Robot Perception; Springer: Berlin/Heidelberg, Germany, 2005; pp. 203–264. [Google Scholar] [CrossRef]
- Rocchini, C.; Cignoni, P.; Montani, C.; Pingi, P.; Scopigno, R. A low cost 3D scanner based on structured light. Comput. Graph. Forum 2001, 20, 299–308. [Google Scholar] [CrossRef]
- Boehler, W.; Marbs, A. The potential of non-contact close range laser scanners for cultural heritage recording. Int. Arch. Photogramm. Remote Sens. Spat. Inf.Sci. 2002, 34, 430–436. [Google Scholar]
- Beniak, J.; Križan, P.; Matus, M.; Svatek, M. Ecological PLA plastic used for FDM rapid prototyping technology. In Proceedings of the International Multidisciplinary Scientific GeoConference Surveying Geology and Mining Ecology Management, SGEM, Albena, Bulgaria, 18–24 June 2015; Volume 1, pp. 117–123. [Google Scholar] [CrossRef]
- Svetlik, J.; Demeč, P. Methods of Identifying the Workspace of Modular Serial Kinematic Structures. In Proceedings of the Applied Mechanics and Materials: CECOL 2012: 3rd Central European Conference on Logistics, Trnava, Slovakia, 28–30 November 2012; Volume 309, pp. 75–79, ISBN 978-303785636-9. [Google Scholar]

| Parameter | MetraSCAN 70 | MetraSCAN 70R | HandyPROBE |
|---|---|---|---|
| Price (EUR) | 67,350 | 130,000 | 38,500 |
| Weight (kg) | 1.85 kg | 1.85 kg | 0.45 kg |
| Dimensions (mm) | 282 × 250 × 282 | 204 × 159 × 97 | |
| Measuring speed (pts/s) | 36,000 | 30 | |
| Volumetric accuracy (mm) | 0.075 | 0.022 | |
| Spacing distance (mm) | 152 | N/A | |
| Depth/width FOV (Field of View) (mm) | 50/2 × 70 | N/A | |
| Operating humidity range (%) | 10–90% | 10–90% | |
| Working temperature (°C) | 15–40 | 15–40 °C | |
| Parameter | XC65Dx | LC60Dx | LC15Dx |
|---|---|---|---|
| Price (EUR) | 97,130 | 65,110 | 64,850 |
| Weight (kg) | 0.44 | 0.39 | 0.37 |
| Dimensions (mm) | 155 × 86 × 142 | N/A | 100 × 104 × 58 |
| Measuring speed (pts/s) | 75,000 | 75,000 | 70,000 |
| Accuracy (mm) | 0.012 | 0.009 | 0.006 |
| Spacing distance (mm) | 75 | 95 | 60 |
| Depth/width FOV (mm) | 3 × 65/3 × 65 | 60/- | 15/- |
| Operating humidity range (%) | 10–90% | 10–90% | 10–90% |
| Working temperature (°C) | 10–40 °C | 10–40 °C | 10–40 °C |
| Parameter | Metronor DUO | ATOS III Triple Scan |
|---|---|---|
| Price (EUR) | 41,700 | 98,540 |
| Weight (kg) | 0.52 | 7.5 |
| Dimensions (mm) | 500 × 200 × 30 | 155 × 86 × 142 |
| Measuring speed (pts/s) | 35 | 75,000 |
| Accuracy (mm) | 0.025 | 0.01 |
| Spacing distance (mm) | 1500–15,000 | 490–2000 |
| Depth FOV (Field of View) (mm) | 230 | |
| Width FOV (Field of View) (mm) | 250 × 250 | |
| Working temperature (°C) | 10–45 °C | 5–40 °C |
| Operating humidity range (%) | <90% | 10–90% |
| Criterion | f1 | f2 | f3 | f4 |
|---|---|---|---|---|
| f1 | 1 | 1/9 | 1/8 | 1/5 |
| f2 | 9 | 1 | 3 | 5 |
| f3 | 8 | 1/3 | 1 | 3 |
| f4 | 5 | 1/5 | 1/3 | 1 |
| Kr. | f1 | f2 | f3 | f4 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| a1 | a2 | a3 | a4 | a1 | a2 | a3 | a4 | a1 | a2 | a3 | a4 | a1 | a2 | a3 | a4 | |
| a1 | 1 | 3 | 1 | 3 | 1 | 2 | 9 | 6 | 1 | 3 | 9 | 5 | 1 | 2 | 1/7 | 3 |
| a2 | 1/3 | 1 | 1/4 | 1/3 | 1/2 | 1 | 9 | 6 | 1/3 | 1 | 9 | 4 | 1/2 | 1 | 1/8 | 2 |
| a3 | 1 | 4 | 1 | 4 | 1/9 | 1/9 | 1 | 1/4 | 1/9 | 1/9 | 1 | 1/5 | 7 | 8 | 1 | 8 |
| a4 | 1/3 | 3 | 1/4 | 1 | 1/6 | 1/6 | 4 | 1 | 1/5 | 1/4 | 5 | 1 | 1/3 | 1/2 | 1/8 | 1 |
| Criterion | Weight | Quality Manager | Technician | Economist | Engineer |
|---|---|---|---|---|---|
| Education degree | 0.0383 | 0.3535 | 0.0855 | 0.4103 | 0.1507 |
| Practical experiences with RE systems | 0.5635 | 0.5083 | 0.3612 | 0.0376 | 0.0928 |
| Theoretical knowledge from quality inspection | 0.2736 | 0.5525 | 0.298 | 0.0362 | 0.1133 |
| Economic knowledge | 0.1246 | 0.1458 | 0.0892 | 0.7067 | 0.0583 |
| Weighted sum | 0.4693 | 0.2995 | 0.1349 | 0.0963 |
| Expert | Weight | f1 | f2 | f3 | f4 | f5 | f6 | f7 |
|---|---|---|---|---|---|---|---|---|
| Quality manager | 0.4693 | 0.0228 | 0.4286 | 0.1083 | 0.1293 | 0.1582 | 0.1170 | 0.0358 |
| Technician | 0.2995 | 0.0232 | 0.4391 | 0.1958 | 0.0718 | 0.0597 | 0.1299 | 0.0805 |
| Economist | 0.1349 | 0.3375 | 0.2646 | 0.0352 | 0.0667 | 0.0887 | 0.1499 | 0.0574 |
| Engineer | 0.0963 | 0.0245 | 0.4574 | 0.1734 | 0.0943 | 0.0806 | 0.1170 | 0.0528 |
| Weighted sum: | 0.0655 | 0.4124 | 0.1309 | 0.1003 | 0.1118 | 0.1253 | 0.0537 |
| Criterion | Weight | a1 | a2 | a3 | a4 | a5 | a6 | a7 | a8 |
|---|---|---|---|---|---|---|---|---|---|
| Price (f1) | 0.0655 | 0.0894 | 0.0204 | 0.2930 | 0.0497 | 0.1252 | 0.1252 | 0.2473 | 0.0497 |
| Accuracy (f2) | 0.4124 | 0.0266 | 0.0198 | 0.0526 | 0.1292 | 0.2216 | 0.3488 | 0.0640 | 0.1375 |
| Portability (f3) | 0.1309 | 0.2288 | 0.0326 | 0.3073 | 0.0310 | 0.0310 | 0.0310 | 0.3073 | 0.0310 |
| Sensed area (f4) | 0.1003 | 0.1163 | 0.1163 | 0.0156 | 0.2009 | 0.0910 | 0.0584 | 0.0157 | 0.3859 |
| Depth of field (f5) | 0.1118 | 0.0339 | 0.0339 | 0.2928 | 0.0516 | 0.0699 | 0.0204 | 0.2784 | 0.2190 |
| Sensing rate (f6) | 0.1253 | 0.1305 | 0.1174 | 0.0175 | 0.1714 | 0.0800 | 0.0567 | 0.0165 | 0.4100 |
| Possibility to move with sensed object & ease of implement. (f7) | 0.0537 | 0.2082 | 0.2082 | 0.2082 | 0.0334 | 0.0334 | 0.0334 | 0.2416 | 0.0334 |
| Weighted sum: | 0.0898 | 0.0551 | 0.1288 | 0.1098 | 0.1324 | 0.1731 | 0.1306 | 0.1804 | |
| Order: | 7 | 8 | 5 | 6 | 3 | 2 | 4 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Beniak, J.; Šooš, Ľ.; Križan, P.; Matúš, M. Implementation of AHP Methodology for the Evaluation and Selection Process of a Reverse Engineering Scanning System. Appl. Sci. 2021, 11, 12050. https://doi.org/10.3390/app112412050
Beniak J, Šooš Ľ, Križan P, Matúš M. Implementation of AHP Methodology for the Evaluation and Selection Process of a Reverse Engineering Scanning System. Applied Sciences. 2021; 11(24):12050. https://doi.org/10.3390/app112412050
Chicago/Turabian StyleBeniak, Juraj, Ľubomír Šooš, Peter Križan, and Miloš Matúš. 2021. "Implementation of AHP Methodology for the Evaluation and Selection Process of a Reverse Engineering Scanning System" Applied Sciences 11, no. 24: 12050. https://doi.org/10.3390/app112412050
APA StyleBeniak, J., Šooš, Ľ., Križan, P., & Matúš, M. (2021). Implementation of AHP Methodology for the Evaluation and Selection Process of a Reverse Engineering Scanning System. Applied Sciences, 11(24), 12050. https://doi.org/10.3390/app112412050







