Assessment and Evaluation of Force–Velocity Variables in Flywheel Squats: Validity and Reliability of Force Plates, a Linear Encoder Sensor, and a Rotary Encoder Sensor
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Design
2.2. Participants
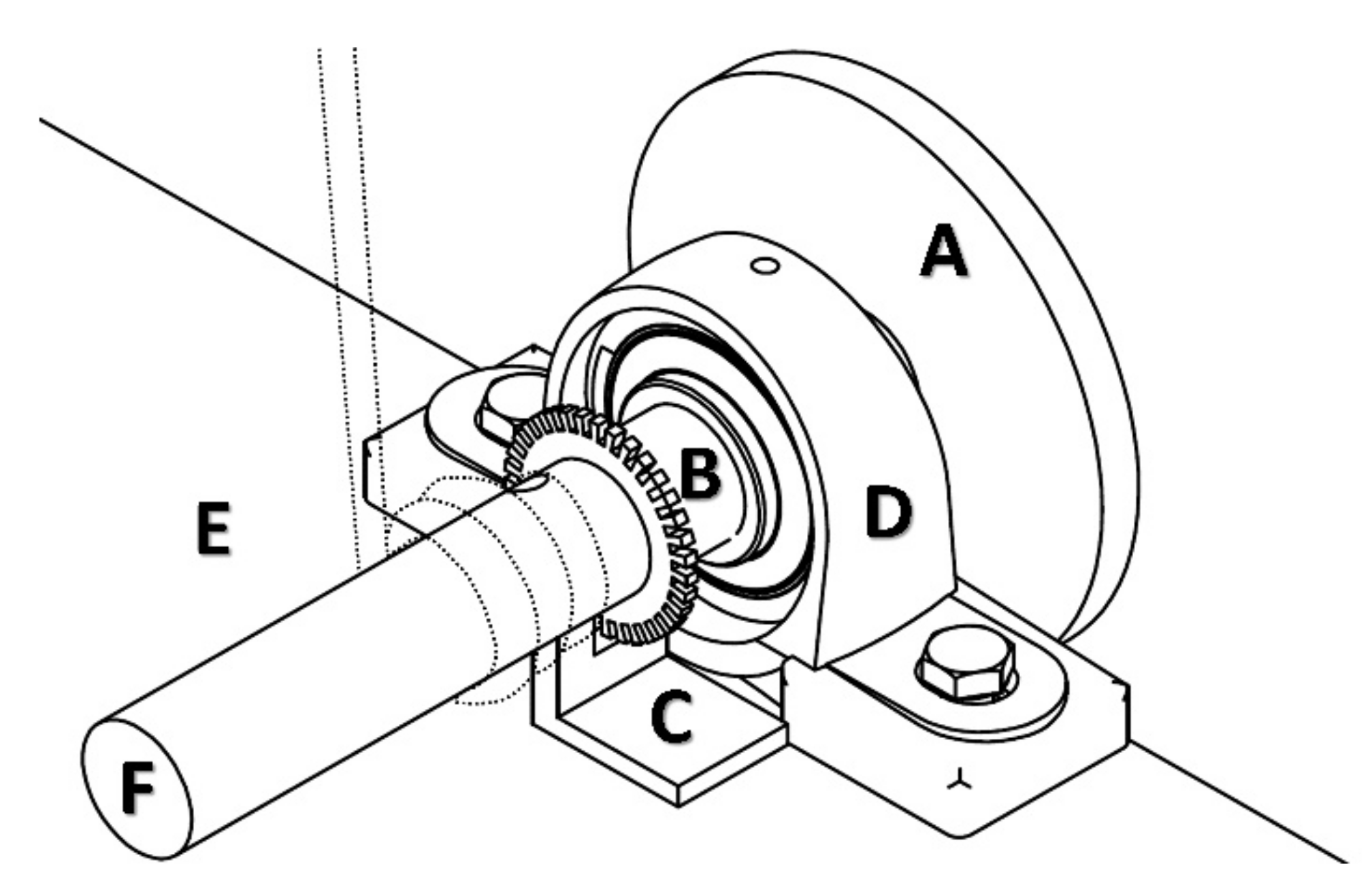
2.3. Testing Procedures
2.4. Data Processing
2.5. Statistical Analyses
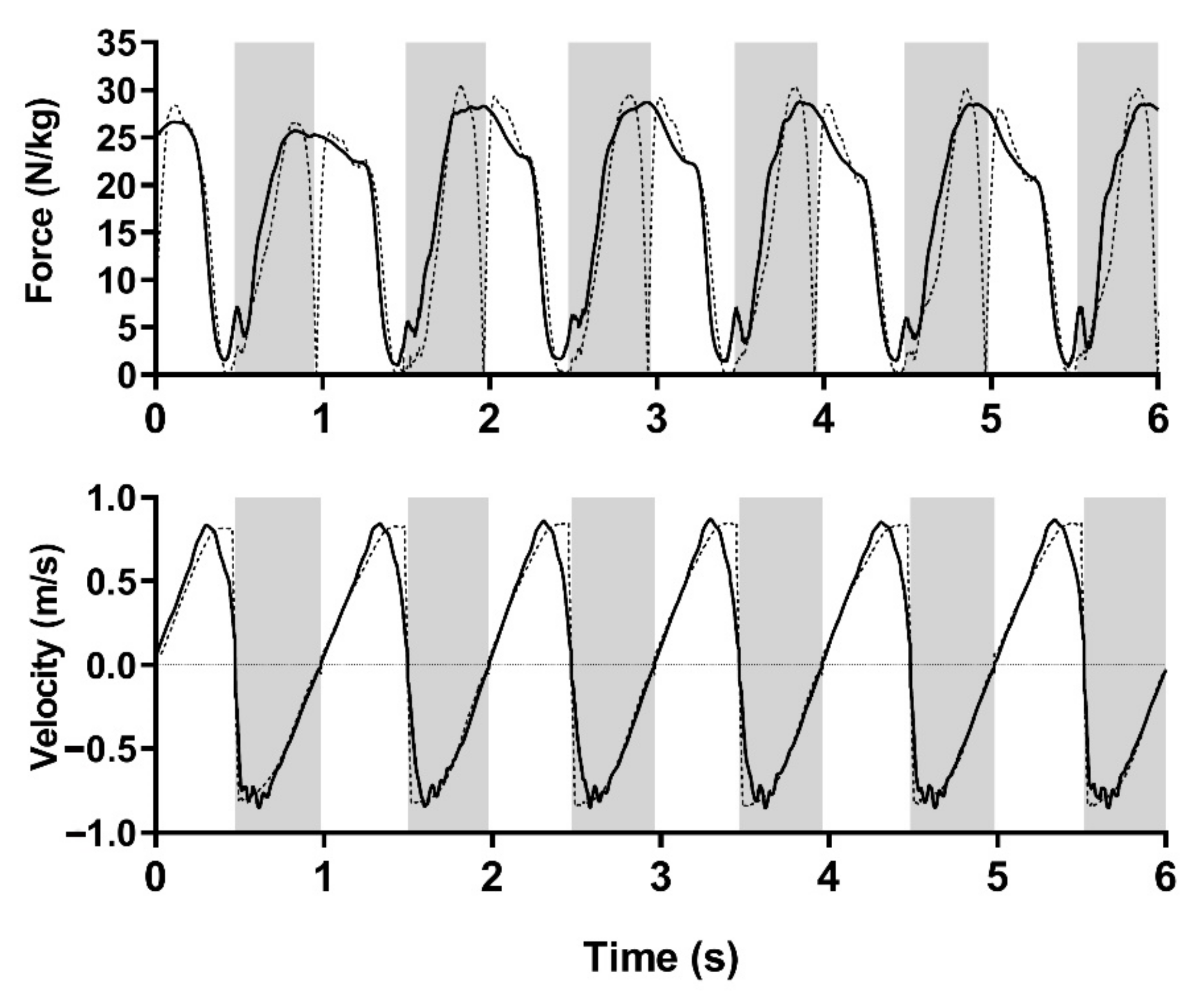
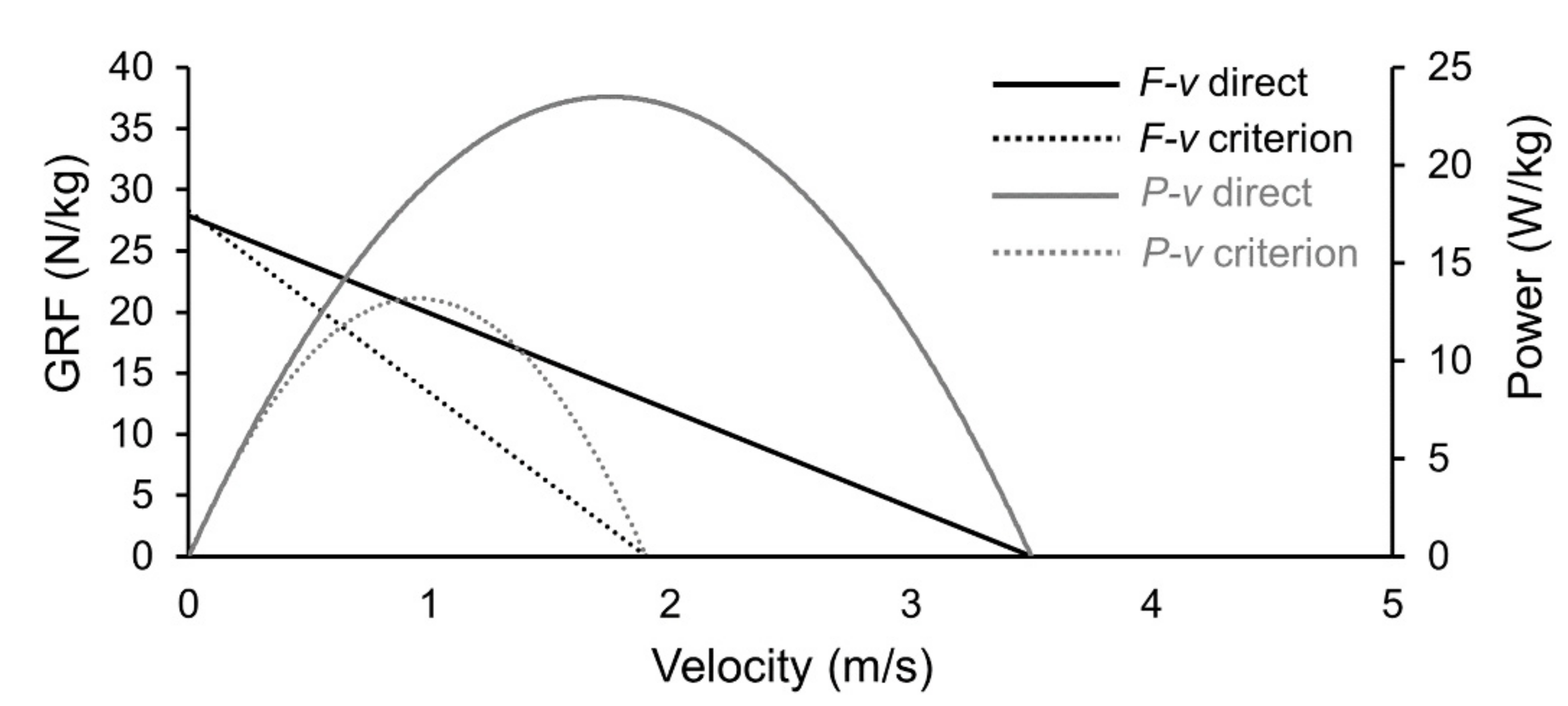
3. Results
3.1. Intra-Session Reliability
3.2. Concurrent Validity
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Mean | Peak | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variable | Inertia (kg∙m2) | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | ||||||
| Bias Avg (LoA) | p | ICC2.k (95% CI) | CV (%) | Bias Avg (LoA) | p | ICC2.k (95% CI) | CV (%) | ||||||
| Concentric | |||||||||||||
| GRF [N/Kg] | 0.025 | 18.83 (1.51) | 18.85 (1.48) | −0.02 (−0.24; 0.22) | 0.898 | 0.923 (0.864; 0.956) | 4.3 | 25.2 (2.64) | 25.15 (2.8) | 0.05 (−0.35; 0.44) | 0.814 | 0.931 (0.879; 0.961) | 5.5 |
| 0.075 | 20.38 (1.90) | 20.64 (1.98) | −0.26 (−0.53; 0.01) | 0.057 | 0.944 (0.895; 0.97) | 4.2 | 26.01 (3.4) | 26.41 (3.35) | −0.41 (−0.82; 0.01) | 0.055 | 0.955 (0.915; 0.976) | 5.2 | |
| 0.225 | 22.43 (2.80) | 23.06 (2.9) | −0.63 (−0.94; −0.32) | <0.001 | 0.952 (0.873; 0.978) | 4.7 | 29.01 (4.04) | 29.59 (3.95) | −0.58 (−1.25; 0.09) | 0.088 | 0.906 (0.831; 0.947) | 7.9 | |
| 0.25 | 23.18 (2.95) | 23.68 (3.02) | −0.50 (−0.84; −0.15) | 0.006 | 0.957 (0.909; 0.978) | 4.8 | 30.13 (4.14) | 30.38 (4.12) | −0.25 (−0.73; 0.22) | 0.286 | 0.963 (0.932; 0.98) | 5.1 | |
| v [m/s] | 0.025 | 0.97 (0.14) | 0.96 (0.14) | 0.01 (−0.01; 0.03) | 0.485 | 0.951 (0.911; 0.973) | 6.3 | 1.37 (0.20) | 1.39 (0.20) | −0.02 (−0.03; 0.00) | 0.111 | 0.97 (0.947; 0.983) | 4.8 |
| 0.075 | 0.72 (0.11) | 0.73 (0.11) | −0.00 (−0.02; 0.01) | 0.796 | 0.964 (0.93; 0.981) | 5.8 | 1.11 (0.17) | 1.12 (0.17) | −0.01 (−0.03; 0) | 0.059 | 0.982 (0.965; 0.99) | 4 | |
| 0.225 | 0.48 (0.08) | 0.49 (0.08) | −0.01 (−0.02; −0.00) | <0.000 | 0.989 (0.952; 0.996) | 2.9 | 0.77 (0.15) | 0.78 (0.15) | −0.01 (−0.02; −0.00) | 0.010 | 0.99 (0.98; 0.995) | 3.6 | |
| 0.25 | 0.47 (0.08) | 0.47 (0.07) | −0.00 (−0.01; 0.01) | 0.728 | 0.976 (0.954; 0.987) | 5 | 0.74 (0.14) | 0.76 (0.14) | −0.01 (−0.02; −0.01) | 0.001 | 0.991 (0.977; 0.996) | 3 | |
| Eccentric | |||||||||||||
| GRF [N/Kg] | 0.025 | 16.84 (1.31) | 16.73 (1.21) | 0.11 (−0.07; 0.29) | 0.214 | 0.933 (0.882; 0.962) | 3.7 | 28.69 (3.54) | 28.01 (3.82) | 0.68 (0.05; 1.31) | 0.034 | 0.892 (0.807; 0.939) | 7.9 |
| 0.075 | 19.09 (1.8) | 19.43 (1.87) | −0.34 (−0.61; −0.07) | 0.017 | 0.93 (0.861; 0.963) | 4.6 | 29.19 (3.88) | 29.87 (4.46) | −0.68 (−1.19; −0.18) | 0.009 | 0.954 (0.905; 0.976) | 5.6 | |
| 0.225 | 22.76 (2.69) | 23.32 (2.84) | −0.56 (−0.89; −0.24) | 0.001 | 0.948 (0.881; 0.974) | 4.8 | 33.70 (5.19) | 34.49 (5.04) | −0.79 (−1.77; 0.19) | 0.11 | 0.874 (0.776; 0.93) | 9.9 | |
| 0.25 | 23.61 (3.07) | 24.07 (2.86) | −0.46 (−0.82; −0.09) | 0.015 | 0.953 (0.906; 0.976) | 5 | 34.53 (5.39) | 35.92 (5.54) | −1.39 (−2.10; −0.67) | <0.000 | 0.937 (0.832; 0.971) | 6.7 | |
| v [m/s] | 0.025 | −0.97 (0.13) | −0.97 (0.13) | 0.00 (−0.01; 0.02) | 0.872 | 0.962 (0.933; 0.979) | 5.1 | −1.36 (0.20) | −1.37 (0.21) | −0.01 (−0.03; 0.02) | 0.511 | 0.95 (0.913; 0.972) | 6.6 |
| 0.075 | −0.76 (0.11) | −0.76 (0.12) | −0.01 (−0.02; 0.00) | 0.148 | 0.981 (0.964; 0.989) | 4.2 | −1.09 (0.18) | −1.11 (0.18) | −0.01 (−0.03; 0.00) | 0.078 | 0.985 (0.971; 0.992) | 4 | |
| 0.225 | −0.51 (0.09) | −0.51 (0.09) | −0.01 (−0.013; 0.0) | 0.041 | 0.985 (0.973; 0.992) | 4.1 | −0.76 (0.16) | −0.77 (0.16) | −0.01 (−0.02; −0.00) | 0.009 | 0.991 (0.982; 0.995) | 3.6 | |
| 0.25 | −0.48 (0.08) | −0.49 (0.09) | −0.01 (−0.01; −0.00) | 0.022 | 0.988 (0.976; 0.994) | 3.7 | −0.73 (0.15) | −0.74 (0.15) | −0.01 (−0.02; −0.00) | 0.002 | 0.992 (0.982; 0.996) | 3.3 | |
Appendix B
| Mean | Peak | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variable | Inertia (kg∙m2) | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | ||||||
| Bias (LoA) | p | ICC2.k (95% CI) | CV (%) | Bias (LoA) | p | ICC2.k (95% CI) | CV (%) | ||||||
| Concentric | |||||||||||||
| GRF [N/Kg] | 0.025 | 14.62 (1.00) | 14.67 (1.02) | −0.05 (−0.20; 0.10) | 0.52 | 0.923 (0.865; 0.956) | 3.7 | 25.09 (2.73) | 25.00 (3.00) | 0.09 (−0.48; 0.66) | 0.763 | 0.86 (0.754; 0.92) | 8.1 |
| 0.075 | 17.14 (1.53) | 17.42 (1.51) | −0.28 (−0.53; −0.02) | 0.032 | 0.906 (0.826; 0.948) | 5 | 23.87 (3.15) | 24.32 (2.88) | −0.46 (−0.92; 0.01) | 0.055 | 0.923 (0.858; 0.958) | 6.5 | |
| 0.225 | 20.21 (2.32) | 20.87 (2.49) | −0.66 (−0.92; −0.40) | <0.001 | 0.945 (0.806; 0.977) | 4.5 | 26.25 (3.53) | 27.03 (3.60) | −0.78 (−1.12; −0.44) | <0.001 | 0.961 (0.877; 0.983) | 4.4 | |
| 0.25 | 20.98 (2.76) | 21.44 (2.59) | −0.46 (−0.76; −0.16) | 0.004 | 0.957 (0.907; 0.978) | 4.8 | 27.22 (3.97) | 27.79 (3.80) | −0.57 (−1.01; −0.14) | 0.011 | 0.96 (0.919; 0.979) | 5.2 | |
| v [m/s] | 0.025 | 0.85 (0.12) | 0.86 (0.13) | −0.01 (−0.03; 0.01) | 0.499 | 0.915 (0.85; 0.952) | 8.1 | 1.41 (0.20) | 1.45 (0.23) | −0.04 (−0.06; −0.01) | 0.005 | 0.944 (0.888; 0.97) | 6.5 |
| 0.075 | 0.67 (0.11) | 0.68 (0.11) | −0.00 (−0.02; 0.01) | 0.623 | 0.964 (0.935; 0.979) | 6.1 | 1.17 (0.18) | 1.17 (0.18) | −0.00 (−0.02; 0.02) | 0.831 | 0.972 (0.95; 0.985) | 5.1 | |
| 0.225 | 0.44 (0.08) | 0.44 (0.08) | −0.00 (−0.01; 0.00) | 0.242 | 0.983 (0.971; 0.991) | 4.5 | 0.82 (0.15) | 0.81 (0.15) | 0.01 (−0.01; 0.02) | 0.515 | 0.974 (0.953; 0.985) | 6 | |
| 0.25 | 0.42 (0.08) | 0.43 (0.08) | −0.00 (−0.01; 0.00) | 0.355 | 0.983 (0.97; 0.99) | 4.7 | 0.78 (0.15) | 0.79 (0.16) | −0.01 (−0.03; 0) | 0.044 | 0.977 (0.957; 0.987) | 5.7 | |
| Eccentric | |||||||||||||
| GRF [N/Kg] | 0.025 | 14.14 (0.96) | 14.07 (0.97) | 0.07 (−0.12; 0.26) | 0.451 | 0.87 (0.771; 0.926) | 4.7 | 25.82 (3.12) | 25.66 (3.38) | 0.17 (−0.49; 0.82) | 0.615 | 0.85 (0.738; 0.914) | 9.2 |
| 0.075 | 15.66 (1.68) | 16.16 (1.59) | −0.50 (−0.81; −0.19) | 0.002 | 0.869 (0.72; 0.934) | 6.5 | 24.47 (3.36) | 25.15 (3.31) | −0.67 (−1.16; −0.19) | 0.008 | 0.925 (0.852; 0.961) | 6.7 | |
| 0.225 | 18.74 (2.23) | 19.64 (2.31) | −0.90 (−1.19; −0.62) | <0.001 | 0.916 (0.552; 0.97) | 5 | 27.43 (3.74) | 28.89 (4.01) | −1.46 (−1.94; −0.97) | <0.001 | 0.918 (0.618; 0.969) | 6 | |
| 0.25 | 19.72 (2.60) | 20.41 (2.47) | −0.69 (−1.01; −0.37) | <0.001 | 0.938 (0.809; 0.973) | 5.3 | 28.75 (4.22) | 29.82 (4.10) | −1.07 (−1.60; −0.53) | <0.001 | 0.935 (0.829; 0.97) | 6.2 | |
| v [m/s] | 0.025 | −0.86 (0.14) | −0.88 (0.14) | −0.01 (−0.03; 0.01) | 0.225 | 0.936 (0.887; 0.963) | 7.9 | −1.61 (0.23) | −1.65 (0.26) | −0.04 (−0.08; −0.01) | 0.028 | 0.915 (0.848; 0.952) | 8.2 |
| 0.075 | −0.66 (0.13) | −0.67 (0.13) | −0.01 (−0.03; −0.00) | 0.027 | 0.97 (0.945; 0.984) | 6.2 | −1.36 (0.22) | −1.39 (0.22) | −0.03 (−0.06; 0.00) | 0.069 | 0.933 (0.882; 0.962) | 7.8 | |
| 0.225 | −0.42 (0.08) | −0.43 (0.08) | −0.01 (−0.02; −0.01) | <0.001 | 0.972 (0.933; 0.986) | 5.7 | −0.91 (0.17) | −0.92 (0.16) | −0.01 (−0.04; 0.01) | 0.249 | 0.94 (0.895; 0.965) | 8.7 | |
| 0.25 | −0.41 (0.08) | −0.42 (0.08) | −0.02 (−0.02; −0.01) | <0.001 | 0.979 (0.904; 0.992) | 4.6 | −0.86 (0.15) | −0.86 (0.16) | −0.04 (−0.05; −0.02) | <0.001 | 0.945 (0.86; 0.974) | 7.3 | |
References
- Beato, M.; Mcerlain-naylor, S.A.; Halperin, I.; Iacono, A. Dello Current Evidence and Practical Applications of Flywheel Eccentric Overload Exercises as Postactivation Potentiation Protocols: A Brief Review Current Evidence and Practical Applications of Flywheel Eccentric Overload Exercises as Postactivation Potentiat. Int. J. Sports Physiol. Perform. 2020, 15, 154–161. [Google Scholar] [CrossRef]
- Beato, M.; Stiff, A.; Coratella, G. Effects of Postactivation Potentiation After an Eccentric Overload Bout on Countermovement Jump and Lower-Limb Muscle Strength. J. Strength Cond. Res. 2021, 35, 1825–1832. [Google Scholar] [CrossRef]
- Coratella, G.; Chemello, A.; Schena, F. Muscle damage and repeated bout effect induced by enhanced eccentric squats. J. Sports Med. Phys. Fitness 2016, 56, 1540–1546. [Google Scholar] [PubMed]
- González, J.R.; de Keijzer, K.; Bishop, C.; Beato, M. Effects of flywheel training on strength-related variables in female populations. A systematic review. Res. Sports Med. 2021, 1–18, published online ahead of print, 5 January 2021. [Google Scholar] [CrossRef]
- Nuñez Sanchez, F.J.; Villarreal, E.S. Does flywheel paradigm training improve muscle volume and force? A meta-analysis. J. Strength Cond. Res. 2017, 31, 3177–3186. [Google Scholar] [CrossRef]
- Maroto-Izquierdo, S.; García-López, D.; Fernandez-Gonzalo, R.; Moreira, O.C.; González-Gallego, J.; Paz, J.A. Skeletal muscle functional and structural adaptations after eccentric overload flywheel resistance training: A systematic review and meta-analysis. J. Sci. Med. Sport 2017, 20, 943–951. [Google Scholar] [CrossRef] [PubMed]
- Petré, H.; Wernstål, F.; Mattsson, C.M. Effects of Flywheel Training on Strength-Related Variables: A Meta-analysis. Sports Med. 2018, 4, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Raya-González, J.; Castillo, D.; Beato, M. The Flywheel Paradigm in Team Sports. Strength Cond. J. 2020, 43, 12–22. [Google Scholar] [CrossRef]
- Beato, M.; Fleming, A.; Coates, A.; Dello Iacono, A. Validity and reliability of a flywheel squat test in sport. J. Sports Sci. 2021, 39, 482–488. [Google Scholar] [CrossRef]
- Cabanillas, R.; Serna, J.; Muñoz-Arroyave, V.; Ramos, J.A.E. Effect of eccentric overload through isoinertial technology in basketball players. Rev. Bras. Cineantropometria Desempenho Hum. 2020, 22, e59831. [Google Scholar] [CrossRef]
- Coratella, G.; Beato, M.; Cè, E.; Scurati, R.; Milanese, C.; Schena, F.; Esposito, F. Effects of in-season enhanced negative work-based vs traditional weight training on change of direction and hamstrings-to-quadriceps ratio in soccer players. Biol. Sport 2019, 36, 241–248. [Google Scholar] [CrossRef]
- Gual, G.; Fort-Vanmeerhaeghe, A.; Romero-Rodriguez, D.; Tesch, P.A. Effects of in-season inertial resistance training with eccentric overload in a sports population at risk for patellar tendinopathy. J. Strength Cond. Res. 2015, 30, 1834–1842. [Google Scholar] [CrossRef] [PubMed]
- Romero-rodriguez, D.; Gual, G.; Tesch, P.A. Efficacy of an inertial resistance training paradigm in the treatment of patellar tendinopathy in athletes: A case-series study. Phys. Ther. Sport 2010, 12, 43–48. [Google Scholar] [CrossRef] [PubMed]
- Tesch, P.A.; Fernandez-Gonzalo, R.; Lundberg, T.R. Clinical applications of iso-inertial, eccentric-overload (YoYoTM) resistance exercise. Front. Physiol. 2017, 8, 241. [Google Scholar] [CrossRef]
- Agustín, R.M.S.; Sánchez-Barbadora, M.; García-Vidal, J.A. Validity of an inertial system for measuring velocity, force, and power during hamstring exercises performed on a flywheel resistance training device. PeerJ 2020, 8, e10169. [Google Scholar] [CrossRef] [PubMed]
- McErlain-Naylor, S.A.; Beato, M. Concentric and eccentric inertia–velocity and inertia–power relationships in the flywheel squat. J. Sports Sci. 2020, 39, 1136–1143. [Google Scholar] [CrossRef] [PubMed]
- Spudić, D.; Smajla, D.; Šarabon, N. Validity and reliability of force–velocity outcome parameters in flywheel squats. J. Biomech. 2020, 107, 109824. [Google Scholar] [CrossRef] [PubMed]
- Zivkovic, M.Z.; Djuric, S.; Cuk, I.; Suzovic, D.; Jaric, S. Muscle Force-Velocity Relationships Observed in Four Different Functional Tests. J. Hum. Kinet. 2017, 56, 39–49. [Google Scholar] [CrossRef]
- Jiménez-Reyes, P.; Samozino, P.; Cuadrado-Peñafiel, V.; Conceição, F.; González-Badillo, J.J.; Morin, J.B. Effect of countermovement on power–force–velocity profile. Eur. J. Appl. Physiol. 2014, 114, 2281–2288. [Google Scholar] [CrossRef]
- Bobbert, M.F. Why is the force-velocity relationship in leg press tasks quasi-linear rather than hyperbolic? Why is the force-velocity relationship in leg press tasks quasi-linear rather than hyperbolic? J. Appl. Physiol. 2012, 112, 1975–1983. [Google Scholar] [CrossRef] [PubMed]
- Jiménez-Reyes, P.; Samozino, P.; Morin, J.-B. Optimized training for jumping performance using the force-velocity imbalance: Individual adaptation kinetics. PLoS ONE 2019, 14, e0216681. [Google Scholar]
- Jaric, S.; Markovic, G. Body mass maximizes power output in human jumping: A strength-independent optimum loading behavior. Eur. J. Appl. Physiol. 2013, 113, 2913–2923. [Google Scholar] [CrossRef]
- Samozino, P.; Morin, J.B.; Hintzy, F.; Belli, A. A simple method for measuring force, velocity and power output during squat jump. J. Biomech. 2008, 41, 2940–2945. [Google Scholar] [CrossRef]
- Samozino, P.; Morin, J.-B.; Hintzy, F.; Belli, A. Jumping ability: A theoretical integrative approach. J. Theor. Biol. 2010, 264, 11–18. [Google Scholar] [CrossRef] [PubMed]
- Markovic, G.; Jaric, S. Positive and negative loading and mechanical output in maximum vertical jumping. Med. Sci. Sports Exerc. 2007, 39, 1757–1764. [Google Scholar] [CrossRef] [PubMed]
- Cormie, P.; McGuigan, M.; Newton, R. Developing Maximal Neuromuscular Power. Sports Med. 2011, 41, 125–146. [Google Scholar] [CrossRef] [PubMed]
- Raya-González, J.; Prat-Luri, A.; López-Valenciano, A.; Sabido, R.; Luis Hernández-Davó, J. Effects of flywheel resistance training on sport actions. A systematic review and meta-analysis. J. Hum. Kinet. 2021, 77, 191–204. [Google Scholar] [CrossRef] [PubMed]
- Coratella, G.; Beato, M.; Milanese, C.; Longo, S.; Limonta, E.; Rampichini, S.; Ce, E.; Bisconti, A.V.; Schena, F.; Esposito, F. Specific adaptations in performance and muscle architecture after weighted jumpsquat vs. body mass squat jump training in recreational soccer players. J. Strength Cond. Res. 2018, 32, 921–929. [Google Scholar] [CrossRef]
- Vázquez-Guerrero, J.; Moras, G.; Baeza, J.; Rodríguez-Jiménez, S. Force outputs during squats performed using a rotational inertia device under stable versus unstable conditions with different loads. PLoS ONE 2016, 11, e0154346. [Google Scholar] [CrossRef][Green Version]
- Carroll, K.M.; Wagle, J.P.; Sato, K.; Christopher, B.; Taber, N.Y.; Bingham, G.E.; Stone, M.H. Characterising overload in inertial flywheel devices for use in exercise training. Sports Biomech. 2018, 18, 390–401. [Google Scholar] [CrossRef] [PubMed]
- Spudić, D.; Kambič, T.; Cvitkovič, R.; Pori, P. Reproducibility and criterion validity of data derived from a flywheel resistance exercise system. Isokinet. Exerc. Sci. 2020, 28, 129–137. [Google Scholar] [CrossRef]
- Sabido, R.; Hernández-Davó, J.L.; Pereyra-Gerber, G. Influence of Different Inertial Loads on Basic Training Variables During the Flywheel Squat Exercise. Int. J. Sports Physiol. Perform. 2018, 13, 482–489. [Google Scholar] [CrossRef] [PubMed]
- López, A.M.; Fonseca, F.; Ramirez-Campillo, R.; Nuñez, F.J.; Nakamura, F.Y. The use of real-time monitoring during flywheel resistance training programs: How can we measure the eccentric overload? A systematic review and meta-analysis. Biol. Sport 2021, 38, 639–652. [Google Scholar] [CrossRef]
- Weakley, J.; Fernández-Valdés, B.; Thomas, L.; Ramirez-Lopez, C.; Jones, B. Criterion validity of force and power outputs for a commonly used flywheel resistance training device and bluetooth app. J. Strength Cond. Res. 2019, 33, 1180–1184. [Google Scholar] [CrossRef] [PubMed]
- Sarabon, N.; Rosker, J.; Fruhmann, H.; Burggraf, S.; Loefler, S.; Kern, H. Reliability of maximal voluntary contraction related parameters measured by a novel portable isometric knee dynamometer. Phys. Med. Rehabil. Kurortmed. 2013, 23, 22–27. [Google Scholar] [CrossRef]
- Hagströmer, M.; Oja, P.; Sjöström, M. The International Physical Activity Questionnaire (IPAQ): A study of concurrent and construct validity. Public Health Nutr. 2006, 9, 755–762. [Google Scholar] [CrossRef] [PubMed]
- Sabido, R.; Hernández-davó, J.L.; Capdepon, L.; Tous-fajardo, J. How Are Mechanical, Physiological, and Perceptual Variables Affected by the Rest Interval Between Sets During a Flywheel Resistance Session? Front. Physiol. 2020, 11, 1–8. [Google Scholar] [CrossRef]
- Harris, N.K.; Cronin, J.; Taylor, K.; Boris, J.; Sheppard, J. Understanding Position Transducer Technology for Strength and Conditioning Practitioners. Strength Cond. J. 2010, 32, 66–79. [Google Scholar] [CrossRef]
- Samozino, P.; Edouard, P.; Sangnier, S.; Brughelli, M.; Gimenez, P.; Morin, J.B. Force-velocity profile: Imbalance determination and effect on lower limb ballistic performance. Int. J. Sports Med. 2014, 35, 505–510. [Google Scholar] [CrossRef]
- Bland, M.; Altman, D. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1986, 8, 307–310. [Google Scholar] [CrossRef]
- Koo, T.K.; Li, M.Y. A Guideline of Selecting and Reporting Intraclass Correlation Coefficients for Reliability Research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef] [PubMed]
- Hopkins, W.G. Measures of Reliability in Sports Medicine and Science. Sports Med. 2000, 30, 1–15. [Google Scholar] [CrossRef]
- Cormack, S.; Newton, R.; McGuigan, M.; Doyle, T. Reliability of measures obtained during single and repeated countermovement jumps. Int. J. Sports Physiol. Perform. 2008, 3, 131–144. [Google Scholar] [CrossRef]
- Hinkle, D.E.; Wiersma, W.; Jurs, S.G. Applied Statistics for the Behavioral Sciences, 5th ed.; Houghton Mifflin: Boston, MA, USA, 2003. [Google Scholar]
- Demsar, J.; Curk, T.; Erjavec, A.; Gorup, C.; Hocevar, T.; Milutinovic, M.; Mozina, M.; Polajnar, M.; Toplak, M.; Staric, A.; et al. Orange: Data Mining Toolbox in Python. J. Mach. Learn. Res. 2013, 14, 2349–2353. [Google Scholar]
- Bakdash, J.Z.; Marusich, L.R. Repeated measures correlation. Front. Psychol. 2017, 8, 1–13. [Google Scholar] [CrossRef]
- Bobbert, M.F.; Van Soest, A.J. Effects of muscle strengthening on vertical jump height: A simulation study. Med. Sci. Sports Exerc. 1994, 26, 1012–1020. [Google Scholar] [CrossRef]
- Bollinger, L.; Brantley, J.; Tarlton, J.; Baker, P.; Seay, R.; Abel, M. Construct validity, test-retest reliability, and repeatability of performance variables using a flywheel resistance training device. J. Strength Cond. Res. 2020, 34, 3149–3156. [Google Scholar] [CrossRef]
- García-Ramos, A.; Feriche, B.; Pérez-Castilla, A.; Padial, P.; Jaric, S. Assessment of leg muscles mechanical capacities: Which jump, loading, and variable type provide the most reliable outcomes? Eur. J. Sport Sci. 2017, 17, 690–698. [Google Scholar] [CrossRef]
- Smajla, D.; Spudić, D.; Šarabon, N. Influence of Load and Phase of Contraction on Lateral Symmetries in Flywheel Squats. Symmetry 2021, 13, 111. [Google Scholar] [CrossRef]
- Alcazar, J.; Csapo, R.; Ara, I.; Alegre, L.M. On the shape of the force-velocity relationship in skeletal muscles: The linear, the hyperbolic, and the double-hyperbolic. Front. Physiol. 2019, 10, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Hansen, K.T.; Cronin, J.B.; Newton, M.J. The reliability of linear position transducer and force plate measurement of explosive force-time variables during a loaded jump squat in elite athletes. J. Strength Cond. Res. 2011, 25, 1447–1456. [Google Scholar] [CrossRef] [PubMed]
- García-Ramos, A.; Pérez-Castilla, A.; Morales-Artacho, A.J.; Almeida, F.; Padial, P.; Bonitch-Góngora, J.; Fuente, B.D.; Feriche, B. Force-velocity relationship in the countermovement jump exercise assessed by different measurement methods. J. Hum. Kinet. 2019, 67, 37–47. [Google Scholar] [CrossRef] [PubMed]
- Giroux, C.; Rabita, G.; Chollet, D.; Guilhem, G. What is the Best Method for Assessing Lower Limb Force- Velocity Relationship? Int. J. Sports Med. 2015, 36, 143–149. [Google Scholar] [CrossRef] [PubMed]
- García-Ramos, A.; Jaric, S.; Pérez-Castilla, A.; Padial, P.; Feriche, B. Reliability and magnitude of mechanical variables assessed from unconstrained and constrained loaded countermovement jumps. Sport Biomech. 2017, 16, 514–526. [Google Scholar] [CrossRef] [PubMed]
- García-Ramos, A.; Štirn, I.; Padial, P.; Argüelles-Cienfuegos, J.; De la Fuente, B.; Strojnik, V.; Feriche, B. Predicting vertical jump height from bar velocity. J. Sports Sci. Med. 2015, 14, 256–262. [Google Scholar]
- Courel-Ibáñez, J.; Martínez-Cava, A.; Morán-Navarro, R.; Escribano-Peñas, P.; Chavarren-Cabrero, J.; González-Badillo, J.J.; Pallarés, J.G. Reproducibility and Repeatability of Five Different Technologies for Bar Velocity Measurement in Resistance Training. Ann. Biomed. Eng. 2019, 47, 1523–1538. [Google Scholar] [CrossRef] [PubMed]
- Garcia-Ramos, A.; Jaric, S. Two-point method: A quick and fatigue-free procedure for assessment of muscle mechanical capacities and the 1 repetition maximum. Strength Cond. J. 2018, 40, 54–66. [Google Scholar] [CrossRef]
- Lake, J.P.; Lauder, M.A.; Smith, N.A. Barbell kinematics should not be used to estimate power output applied to the barbell-and-body system center of mass during lower-body resistance exercise. J. Strength Cond. Res. 2012, 26, 1302–1307. [Google Scholar] [CrossRef]
- García-Ramos, A.; Stirn, I.; Strojnik, V.; Padial, P.; De la Fuente, B.; Argüelles-Cienfuegos, J.; Feriche, B. Comparison of the force-, velocity-, and power-time curves recorded with a force plate and a linear velocity transducer. Sports Biomech. 2016, 15, 329–341. [Google Scholar] [CrossRef] [PubMed]
- Spudić, D.; Smajla, D.; Burnard, M.D.; Šarabon, N. Muscle Activation Sequence in Flywheel Squats. Int. J. Environ. Res. Public Health 2021, 18, 3168. [Google Scholar] [CrossRef] [PubMed]
- Knudson, D. Letter to the editor regarding ‘the correlation of force-velocity-power relationship of a whole-body movement with 20 m and 60 m sprint’. Sports Biomech. 2021, 1–5. [Google Scholar] [CrossRef] [PubMed]
- Samozino, P.; Rejc, E.; Di Prampero, P.E.; Belli, A.; Morin, J.B. Optimal force-velocity profile in ballistic movements-Altius: Citius or Fortius? Med. Sci. Sports Exerc. 2012, 44, 313–322. [Google Scholar] [CrossRef] [PubMed]

| N | Age (Years) | Mass (kg) | Height (cm) | Body Mass Index (kg/m2) | Training History (Years) | IPAQ Score (MET/min/Week) | Knee Extension Strength (Nm/kg) | |
|---|---|---|---|---|---|---|---|---|
| Male | 24 | 23.8 (2.8) | 79.3 (12.2) | 181.3 (6.3) | 24.3 (3.0) | 12.1 (3.6) | 3366.8 (1743.3) | 3.44 (0.62) |
| Female | 26 | 22.2 (2.9) | 63.0 (9.1) | 168.4 (5.6) | 22.4 (2.6) | 10.5 (3.5) | 3352.8 (2093.0) | 2.88 (0.48) |
| All | 50 | 23.0 (2.9) | 71.1 (13.4) | 174.8 (8.8) | 23.4 (3.0) | 11.3 (3.6) | 3359.8 (1907.7) | 3.12 (0.61) |
| Criterion (Force Plates and Linear Encoder) | Direct (Rotary Encoder) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variable | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | Set 1 Avg (SD) | Set 2 Avg (SD) | Set 1–Set 2 | ||||||
| Bias (95% LoA) | p | ICC2.1 (95% CI) | CV (%) | Bias (95% LoA) | p | ICC2.1 (95% CI) | CV (%) | |||||
| F0 [Nm·kg−1] | 27.341 (6.389) | 27.937 (4.938) | −0.596 (−1.495; 0.303) | 0.189 | 0.916 (0.852; 0.952) | 11.3 | 26.809 (4.657) | 27.977 (4.801) | −1.169 (−1.866; −0.471) | 0.002 | 0.921 (0.823; 0.961) | 8.6 |
| V0 [m·s−1] | 2.041 (0.522) | 1.948 (0.417) | 0.093 (−0.013; 0.199) | 0.084 | 0.809 (0.664; 0.891) | 18.6 | 4.047 (1.861) | 3.514 (1.573) | 0.534 (−0.03; 1.098) | 0.063 | 0.65 (0.303; 0.848) | 39.5 |
| Pmax [W·kg−1] | 13.343 (2.112) | 13.299 (2.252) | 0.043 (−0.374; 0.461) | 0.835 | 0.874 (0.778; 0.929) | 10.9 | 25.757 (8.951) | 23.5 (7.548) | 2.257 (−0.54; 5.054) | 0.111 | 0.613 (0.235; 0.828) | 28.5 |
| Slope [N·s·m−1·kg−1] | −15.102 (8.412) | −15.252 (5.003) | 0.15 (−1.462; 1.762) | 0.852 | 0.801 (0.649; 0.887) | 27.3 | −8.165 (4.15) | −9.326 (3.743) | 1.16 (0.116; 2.204) | 0.03 | 0.738 (0.524; 0.855) | 30.1 |
| Variables | Mean | Peak | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Inertia (kg∙m2) | Criterion (m/s) | Direct (m/s) | Bias (95% LoA) | p | r (95% CI) | TE | TEs | Criterion (m/s) | Direct (m/s) | Bias (95% LoA) | p | r (95% CI) | TE | TEs |
| Concentric | ||||||||||||||
| 0.025 | 0.87 (0.13) | 0.96 (0.15) | −0.09 (−0.12; −0.07) | <0.001 | 0.864 (0.771; 0.920) | 0.012 | 0.094 | 1.45 (0.22) | 1.39 (0.2) | 0.06 (0.03; 0.09) | <0.001 | 0.900 (0.829; 0.942) | 0.016 | 0.070 |
| 0.075 | 0.68 (0.1) | 0.73 (0.12) | −0.05 (−0.07; −0.04) | <0.001 | 0.884 (0.803; 0.933) | 0.009 | 0.063 | 1.17 (0.18) | 1.12 (0.17) | 0.05 (0.03; 0.07) | <0.001 | 0.917 (0.858; 0.952) | 0.012 | 0.065 |
| 0.225 | 0.45 (0.07) | 0.49 (0.09) | −0.04 (−0.05; −0.03) | <0.001 | 0.887 (0.808; 0.935) | 0.006 | 0.077 | 0.82 (0.15) | 0.78 (0.15) | 0.03 (0.02; 0.05) | <0.001 | 0.948 (0.910; 0.970) | 0.008 | 0.053 |
| 0.25 | 0.43 (0.08) | 0.47 (0.08) | −0.04 (−0.06; −0.02) | <0.001 | 0.904 (0.836; 0.945) | 0.006 | 0.079 | 0.8 (0.15) | 0.76 (0.14) | 0.04 (0.02; 0.06) | <0.001 | 0.937 (0.981; 0.964) | 0.009 | 0.058 |
| Eccentric | ||||||||||||||
| 0.025 | −0.87 (0.14) | −0.97 (0.12) | −0.1 (−0.13; −0.07) | <0.001 | 0.797 (0.585; 0.931) | 0.017 | 0.122 | −1.61 (0.25) | −1.35 (0.19) | 0.26 (0.21; 0.30) | <0.001 | 0.852 (0.732; 0.921) | 0.022 | 0.087 |
| 0.075 | −0.66 (0.12) | −0.76 (0.12) | −0.101 (−0.13; −0.08) | <0.001 | 0.79 (0.629; 0.886) | 0.013 | 0.105 | −1.36 (0.21) | −1.10 (0.19) | 0.26 (0.23; 0.30) | <0.001 | 0.853 (0.733; 0.921) | 0.018 | 0.085 |
| 0.225 | −0.43 (0.08) | −0.50 (0.08) | −0.074 (−0.09; −0.06) | <0.001 | 0.887 (0.792; 0.94) | 0.006 | 0.073 | −0.89 (0.15) | −0.74 (0.15) | 0.15 (0.12; 0.17) | <0.001 | 0.853 (0.732; 0.921) | 0.013 | 0.086 |
| 0.25 | −0.41 (0.08) | −0.48 (0.08) | −0.063 (−0.08; −0.05) | <0.001 | 0.874 (0.77; 0.933) | 0.007 | 0.085 | −0.87 (0.15) | −0.72 (0.15) | 0.15 (0.13; 0.18) | <0.001 | 0.899 (0.812; 0.946) | 0.011 | 0.073 |
| Variables | Mean | Peak | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Inertia (kg∙m2) | Criterion (N/kg) | Direct (N/kg) | Bias (95% LoA) | p | r (95% CI) | TE | TEs | SF (95% CI) | Criterion (N/kg) | Direct (N/kg) | Bias (95% LoA) | p | r (95% CI) | TE | TEs | SF (95% CI) |
| Concentric | ||||||||||||||||
| 0.025 | 14.61 (0.86) | 18.79 (1.21) | −4.18 (−4.39; −3.96) | <0.001 | 0.855 (0.736; 0.922) | 0.105 | 0.122 | −0.2 (−0.24; −0.15) | 25.06 (2.36) | 25.14 2.48 | −0.08 (−0.59; 0.43) | 0.759 | 0.795 (0.637; 0.889) | 0.252 | 0.102 | 0.98 (0.91; 1.1) |
| 0.075 | 17.46 (1.6) | 20.65 (2.01) | −3.19 (−3.42; −2.97) | <0.001 | 0.953 (0.911; 0.976) | 0.111 | 0.069 | −0.74 (−0.84; −0.64) | 24.50 (2.99) | 26.49 3.45 | −1.97 (−2.48; −1.50) | <0.001 | 0.902 (0.818; 0.948) | 0.242 | 0.081 | 0.29 (0.16; 0.42) |
| 0.225 | 20.57 (2.44) | 22.64 (2.82) | −2.06 (−2.34; −1.79) | <0.001 | 0.959 (0.922; 0.979) | 0.136 | 0.056 | −1.44 (−1.68; −1.21) | 26.67 (3.47) | 29.25 3.89 | −2.58 (−3.14; −2.02) | <0.001 | 0.899 (0.814; 0.947) | 0.276 | 0.08 | −1.0 (−1.33; −0.67) |
| 0.25 | 20.98 (2.31) | 22.98 (2.64) | −2.0 (−2.26; −1.74) | <0.001 | 0.959 (0.922; 0.979) | 0.126 | 0.054 | −1.61 (−1.91; −1.32) | 27.27 (3.43) | 29.72 3.75 | −2.45 (−2.86; −2.05) | <0.001 | 0.945 (0.896; 0.971) | 0.199 | 0.058 | −1.12 (−1.43; −0.82) |
| Eccentric | ||||||||||||||||
| 0.025 | 14.05 (0.84) | 16.61 (1.0) | −2.56 (−2.82; −2.30) | <0.001 | 0.75 (0.513; 0.904) | 0.129 | 0.154 | 0.04 (−0.04; 0.12) | 25.65 (2.72) | 28.40 (2.96) | −2.76 (−3.58; −1.93) | <0.001 | 0.712 (0.463; 0.879) | 0.407 | 0.015 | 0.67 (0.56; 0.79) |
| 0.075 | 16.21 (1.68) | 19.53 (1.88) | −3.32 (−3.60; −3.04) | <0.001 | 0.891 (0.798; 0.943) | 0.14 | 0.084 | −1.02 (−1.19; −0.86) | 25.26 (3.43) | 29.93 (4.57) | −4.67 (−5.52; −3.82) | <0.001 | 0.827 (0.69; 0.907) | 0.42 | 0.123 | −0.34 (−0.58; −0.1) |
| 0.225 | 19.45 (2.25) | 22.98 (2.72) | −3.54 (−3.87; −3.20) | <0.001 | 0.936 (0.878; 0.967) | 0.165 | 0.073 | −3.2 (−3.53; −2.87) | 28.38 (3.71) | 33.98 (4.74) | −5.60 (−6.43; −4.77) | <0.001 | 0.85 (0.728; 0.92) | 0.409 | 0.11 | −2.45 (−3.1; −1.81) |
| 0.25 | 20.02 (2.17) | 23.45 (2.492) | −3.43 (−3.77; −3.09) | <0.001 | 0.914 (0.838; 0.955) | 0.167 | 0.077 | −3.35 (−3.76; −2.95) | 29.15 (3.47) | 34.88 (4.78) | −5.73 (−6.59; −4.87) | <0.001 | 0.846 (0.722; 0.918) | 0.424 | 0.122 | −2.83 (−3.48; −2.17) |
| Variable | Criterion | Direct | Bias (95% LoA) | p | r (95% CI) | TE | TEs |
|---|---|---|---|---|---|---|---|
| F0 (Nm·kg−1) | 28.31 (4.76) | 27.98 (4.80) | 0.333 (−0.148; 0.814) | 0.171 | 0.943 (0.898; 0.968) | 0.23 | 0.05 |
| V0 (m·s−1) | 1.90 (0.40) | 3.51 (1.57) | −1.611 (−1.987; −1.236) | <0.001 | 0.827 (0.707; 0.901) | 0.18 | 0.46 |
| Pmax (W·kg−1) | 13.20 (2.23) | 23.50 (7.55) | −10.298 (−12.188; −8.409) | <0.001 | 0.737 (0.526; 0.883) | 0.93 | 0.42 |
| Slope (N·s·m−1·kg−1) | −15.73 (4.86) | −9.33 (3.74) | −6.401 (−7.133; −5.669) | <0.001 | 0.867 (0.771; 0.925) | 0.36 | 0.07 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Spudić, D.; Cvitkovič, R.; Šarabon, N. Assessment and Evaluation of Force–Velocity Variables in Flywheel Squats: Validity and Reliability of Force Plates, a Linear Encoder Sensor, and a Rotary Encoder Sensor. Appl. Sci. 2021, 11, 10541. https://doi.org/10.3390/app112210541
Spudić D, Cvitkovič R, Šarabon N. Assessment and Evaluation of Force–Velocity Variables in Flywheel Squats: Validity and Reliability of Force Plates, a Linear Encoder Sensor, and a Rotary Encoder Sensor. Applied Sciences. 2021; 11(22):10541. https://doi.org/10.3390/app112210541
Chicago/Turabian StyleSpudić, Darjan, Robert Cvitkovič, and Nejc Šarabon. 2021. "Assessment and Evaluation of Force–Velocity Variables in Flywheel Squats: Validity and Reliability of Force Plates, a Linear Encoder Sensor, and a Rotary Encoder Sensor" Applied Sciences 11, no. 22: 10541. https://doi.org/10.3390/app112210541
APA StyleSpudić, D., Cvitkovič, R., & Šarabon, N. (2021). Assessment and Evaluation of Force–Velocity Variables in Flywheel Squats: Validity and Reliability of Force Plates, a Linear Encoder Sensor, and a Rotary Encoder Sensor. Applied Sciences, 11(22), 10541. https://doi.org/10.3390/app112210541







