Optimization of Metal–Ceramic Functionally Graded Plates Using the Simulated Annealing Algorithm
Abstract
1. Introduction
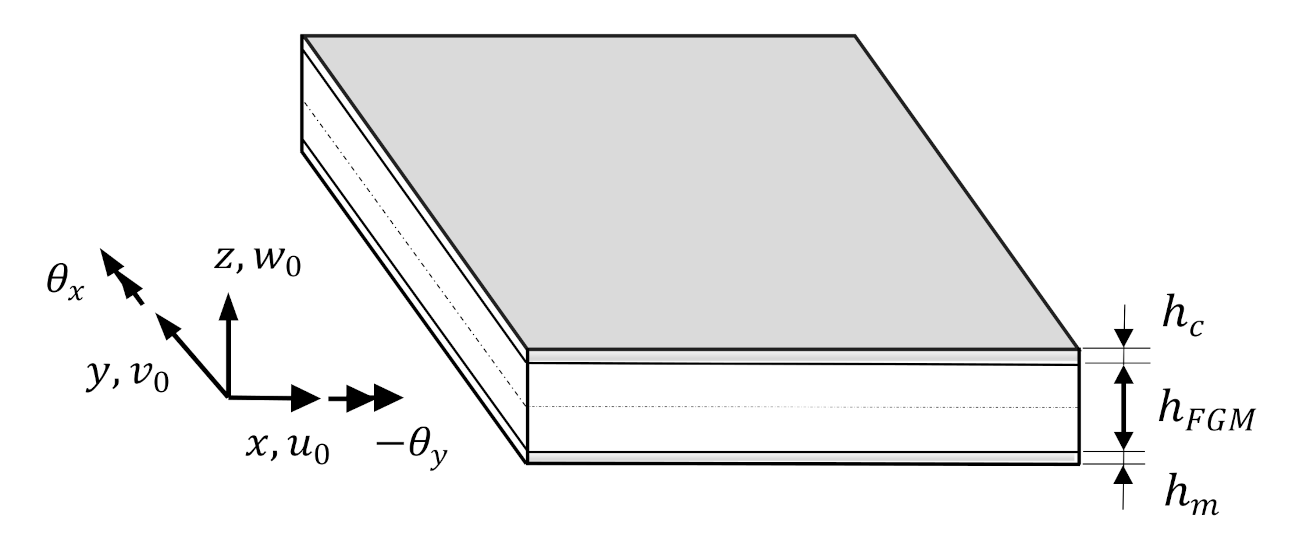
2. Constitutive Relations of the Functionally Graded Material (FGM)
3. Finite Element Model
4. Optimization with Simulated Annealing
5. Numerical Applications
5.1. Design Optimization Studies with a Simply Supported FGM Plate Subjected to Mechanical Loading
5.2. Design Optimization Studies with a Square FGM Plate with Circular Hole Subjected to Thermal Loading
5.3. Design Optimization Studies with a Square FGM Plate with Circular Hole Subjected to Mechanical Loading
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jha, D.K.; Kant, T.; Singh, R.K. A critical review of recent research on functionally graded plates. Compos. Struct. 2013, 96, 833–849. [Google Scholar] [CrossRef]
- Swaminathan, K.; Naveenkumar, D.T.; Zenkour, A.M.; Carrera, E. Stress, vibration and buckling analyses of FGM plates—A state-of-the-art review. Compos. Struct. 2015, 120, 10–31. [Google Scholar] [CrossRef]
- Thai, H.T.; Kim, S.E. A review of theories for the modeling and analysis of functionally graded plates and shells. Compos. Struct. 2015, 128, 70–86. [Google Scholar] [CrossRef]
- Gupta, A.; Talha, M. Recent development in modeling and analysis of functionally graded materials and structures. Prog. Aerosp. Sci. 2015, 79, 1–14. [Google Scholar] [CrossRef]
- Nikbakht, S.; Kamarian, S.; Shakeri, M. A review on optimization of composite structures Part II: Functionally graded materials. Compos. Struct. 2019, 214, 83–102. [Google Scholar] [CrossRef]
- Cho, J.R.; Shin, S.W. Material composition optimization for heat-resisting FGMs by artificial neural network. Compos. Part A: Appl. Sci. Manuf. 2004, 35, 585–594. [Google Scholar] [CrossRef]
- Chen, B.; Tong, L. Thermomechanically coupled sensitivity analysis and design optimization of functionally graded materials. Comput. Methods Appl. Mech. Eng. 2005, 194, 1891–1911. [Google Scholar] [CrossRef]
- Goupee, A.J.; Vel, S.S. Multi-objective optimization of functionally graded materials with temperature-dependent material properties. Mater. Des. 2007, 28, 1861–1879. [Google Scholar] [CrossRef]
- Goupee, A.J.; Vel, S.S. Two-dimensional optimization of material composition of functionally graded materials using meshless analyses and a genetic algorithm. Comput. Methods Appl. Mech. Eng. 2006, 195, 5926–5948. [Google Scholar] [CrossRef]
- Xia, Q.; Wang, M.Y. Simultaneous optimization of the material properties and the topology of functionally graded structures. Comput. Aided Des. 2008, 40, 660–675. [Google Scholar] [CrossRef]
- Icardi, U.; Ferrero, L. Optimization of sandwich panels with functionally graded core and faces. Compos. Sci. Technol. 2009, 69, 575–585. [Google Scholar] [CrossRef]
- Na, K.S.; Kim, J.H. Volume fraction optimization of functionally graded composite panels for stress reduction and critical temperature. Finite Elem. Anal. Des. 2009, 45, 845–851. [Google Scholar] [CrossRef]
- Na, K.S.; Kim, J.H. Optimization of volume fractions for functionally graded panels considering stress and critical temperature. Compos. Struct. 2009, 89, 509–516. [Google Scholar] [CrossRef]
- Na, K.S.; Kim, J.H. Volume fraction optimization for step-formed functionally graded plates considering stress and critical temperature. Compos. Struct. 2010, 92, 1283–1290. [Google Scholar] [CrossRef]
- Mozafari, H.; Ayob, A.; Kamali, F. Optimization of functional graded plates for buckling load by using imperialist competitive algorithm. Procedia Technol. 2012, 1, 144–152. [Google Scholar] [CrossRef][Green Version]
- Kou, X.Y.; Parks, G.T.; Tan, S.T. Optimal design of functionally graded materials using a procedural model and particle swarm optimization. Comput. Aided Des. 2012, 44, 300–310. [Google Scholar] [CrossRef]
- Noh, Y.J.; Kang, Y.J.; Youn, S.J.; Cho, J.R.; Lim, O.K. Reliability-based design optimization of volume fraction distribution in functionally graded composites. Comput. Mater. Sci. 2013, 69, 435–442. [Google Scholar] [CrossRef]
- Vatanabe, S.L.; Paulino, G.H.; Silva, E.C.N. Design of functionally graded piezocomposites using topology optimization and homogenization—Toward effective energy harvesting materials. Comput. Methods Appl. Mech. Eng. 2013, 266, 205–218. [Google Scholar] [CrossRef]
- Ashjari, M.; Khoshravan, M.R. Mass optimization of functionally graded plate for mechanical loading in the presence of deflection and stress constraints. Compos. Struct. 2014, 110, 118–132. [Google Scholar] [CrossRef]
- Loja, M.A.R. On the use of particle swarm optimization to maximize bending stiffness of functionally graded structures. J. Symb. Comput. 2014, 61, 12–30. [Google Scholar] [CrossRef]
- Taheri, A.H.; Hassani, B. Simultaneous isogeometrical shape and material design of functionally graded structures for optimal eigenfrequencies. Comput. Methods Appl. Mech. Eng. 2014, 277, 46–80. [Google Scholar] [CrossRef]
- Taheri, A.H.; Hassani, B.; Moghaddam, N.Z. Thermo-elastic optimization of material distribution of functionally graded structures by an isogeometrical approach. Int. J. Solids Struct. 2014, 51, 416–429. [Google Scholar] [CrossRef]
- Shi, J.X.; Shimoda, M. Interface shape optimization of designing functionally graded sandwich structures. Compos. Struct. 2015, 125, 88–95. [Google Scholar] [CrossRef]
- Wang, Z.W.; Zhang, Q.; Xia, L.Z.; Wu, J.T.; Liu, P.Q. Stress Analysis and Parameter Optimization of an FGM Pressure Vessel Subjected to Thermo-Mechanical Loadings. Procedia Eng. 2015, 130, 374–389. [Google Scholar] [CrossRef]
- Roque, C.M.C.; Martins, P.A.L.S. Differential evolution for optimization of functionally graded beams. Compos. Struct. 2015, 133, 1191–1197. [Google Scholar] [CrossRef]
- Roque, C.M.C.; Martins, P.A.L.S.; Ferreira, A.J.M.; Jorge, R.M.N. Differential evolution for free vibration optimization of functionally graded nano beams. Compos. Struct. 2016, 156, 29–34. [Google Scholar] [CrossRef]
- Tsiatas, G.C.; Charalampakis, A.E. Optimizing the natural frequencies of axially functionally graded beams and arches. Compos. Struct. 2017, 160, 256–266. [Google Scholar] [CrossRef]
- Shabana, Y.M.; Elsawaf, A.; Khalaf, H.; Khalil, Y. Stresses minimization in functionally graded cylinders using particle swarm optimization technique. Int. J. Press. Vessel. Pip. 2017, 154, 1–10. [Google Scholar] [CrossRef]
- Lieu, Q.X.; Lee, J. Modeling and optimization of functionally graded plates under thermo-mechanical load using isogeometric analysis and adaptive hybrid evolutionary firefly algorithm. Compos. Struct. 2017, 179, 89–106. [Google Scholar] [CrossRef]
- Lieu, Q.X.; Lee, J.; Lee, D.; Lee, S.; Kim, D.; Lee, J. Shape and size optimization of functionally graded sandwich plates using isogeometric analysis and adaptive hybrid evolutionary firefly algorithm. Thin Walled Struct. 2018, 124, 588–604. [Google Scholar] [CrossRef]
- Jafari, S.; Hojjati, M.H.; Fathi, A. Classical and modern optimization methods in minimum weight design of elastic rotating disk with variable thickness and density. Int. J. Press. Vessel. Pip. 2012, 92, 41–47. [Google Scholar] [CrossRef]
- Khorsand, M.; Tang, Y. Design functionally graded rotating disks under thermoelastic loads: Weight optimization. Int. J. Press. Vessel. Pip. 2018, 161, 33–40. [Google Scholar] [CrossRef]
- Moita, J.S.; Araújo, A.L.; Correia, V.F.; Soares, C.M.M.; Herskovits, J. Material distribution and sizing optimization of functionally graded plate-shell structures. Compos. Part B Eng. 2018, 142, 263–272. [Google Scholar] [CrossRef]
- Vel, S.S.; Pelletier, J.L. Multi-objective optimization of functionally graded thick shells for thermal loading. Compos. Struct. 2007, 81, 386–400. [Google Scholar] [CrossRef]
- Wang, C.; Koh, J.M.; Yu, T.; Xie, N.G.; Cheong, K.H. Material and shape optimization of bi-directional functionally graded plates by GIGA and an improved multi-objective particle swarm optimization algorithm. Comput. Methods Appl. Mech. Eng. 2020, 366, 113017. [Google Scholar] [CrossRef]
- Ashjari, M.; Khoshravan, M.R. Multi-objective optimization of a functionally graded sandwich panel under mechanical loading in the presence of stress constraint. J. Mech. Behav. Mater. 2017, 26, 79–93. [Google Scholar] [CrossRef]
- Correia, V.M.F.; Madeira, J.F.A.; Araújo, A.L.; Soares, C.M.M. Multiobjective optimization of ceramic-metal functionally graded plates using a higher order model. Compos. Struct. 2018, 183, 146–160. [Google Scholar] [CrossRef]
- Correia, V.M.F.; Madeira, J.F.A.; Araújo, A.; Soares, C.M.M. Multiobjective optimization of functionally graded material plates with thermo-mechanical loading. Compos. Struct. 2019, 207, 845–857. [Google Scholar] [CrossRef]
- Moleiro, F.; Madeira, J.F.A.; Carrera, E.; Reddy, J.N. Design optimization of functionally graded plates under thermo-mechanical loadings to minimize stress, deformation and mass. Compos. Struct. 2020, 245, 112360. [Google Scholar] [CrossRef]
- Morales-Castañeda, B.; Zaldivar, D.; Cuevas, E.; Maciel-Castillo, O.; Aranguren, I.; Fausto, F. An improved Simulated Annealing algorithm based on ancient metallurgy techniques. Appl. Soft Comput. 2019, 84, 105761. [Google Scholar] [CrossRef]
- Reddy, J.N. Mechanics of Laminated Composite Plates-Theory and Applications; CRC Press: Boca Raton, FL, USA, 1997. [Google Scholar]
- Reddy, J.N.; Chin, C.D. Thermomechanical analysis of functionally graded cylinders and plates. J. Therm. Stress. 1998, 21, 593–626. [Google Scholar] [CrossRef]
- Shen, H.S.; Wang, Z.X. Assessment of Voigt and Mori–Tanaka models for vibration analysis of functionally graded plates. Compos. Struct. 2012, 94, 2197–2208. [Google Scholar] [CrossRef]
- Correia, V.M.F.; Gomes, M.A.A.; Suleman, A.; Soares, C.M.M.; Soares, C.M. Modelling and design of adaptive composite structures. Comput. Methods Appl. Mech. Eng. 2000, 185, 325–346. [Google Scholar] [CrossRef]
- Kirkpatrick, S.; Gellat, C.D.; Vecchi, M.P. Optimization by Simulated Annealing. Science 1983, 220, 671–680. [Google Scholar] [CrossRef] [PubMed]
- Corana, A.; Marchesi, M.; Martini, C.; Ridella, S. Minimizing multimodal functions of continuous variables with the Simulated Annealing algorithm. ACM Trans. Math. Softw. 1987, 13, 262–280. [Google Scholar] [CrossRef]
- Henderson, D.; Jacobson, S.H.; Johnson, A.W. The Theory and Practice of Simulated Annealing. In International Series in Operations Research & Management Science; Glover, F., Kochenberger, G.A., Eds.; Springer: Boston, MA, USA, 2003; Volume 57. [Google Scholar] [CrossRef]
- Goffe, W.L.; Ferrier, G.D.; Rogers, J. Global optimization of statistical functions with simulated annealing. J. Econ. 1994, 60, 65–99. [Google Scholar] [CrossRef]
- Custódio, A.L.; Madeira, J.F.A. GLODS: Global and Local Optimization using Direct Search. J. Glob. Optim. 2014, 62, 1–28. [Google Scholar] [CrossRef]

| Materials | Properties | P0 | P−1 | P1 | P2 | P3 |
|---|---|---|---|---|---|---|
| Aluminum | E (Pa) | 349.55 × 109 | 0 | −3.853 × 10−4 | 4.027 × 10−7 | −1.673 × 10−10 |
| oxide | ν | 0.26 | 0 | 0 | 0 | 0 |
| (Al2O3) | ρ (kg/m3) | 3800 | 0 | 0 | 0 | 0 |
| α (1/K) | 6.8269 × 10−6 | 0 | 1.838 × 10−4 | 0 | 0 | |
| κ (W/m K) | −14.087 | −1123.6 | −6.227 × 10−3 | 0 | 0 | |
| Silicon Nitride | E (Pa) | 348.43 × 109 | 0 | −3.070 × 10−4 | 2.160 × 10−7 | −8.946 × 10−11 |
| (Si3N4) | ν | 0.24 | 0 | 0 | 0 | 0 |
| ρ (kg/m3) | 2370 | 0 | 0 | 0 | 0 | |
| α (1/K) | 5.8723 × 10−6 | 0 | 9.095 × 10−4 | 0 | 0 | |
| κ (W/m K) | 113.723 | 0 | −1.032 × 10−3 | 5.466 × 10−7 | −7.876 × 10−11 | |
| Titanium alloy | E (Pa) | 122.56 × 109 | 0 | −4.586 × 10−4 | 0 | 0 |
| (Ti-66Al-4V) | ν | 0.2884 | 0 | 1.121 × 10−4 | 0 | 0 |
| ρ (kg/m3) | 4429 | 0 | 0 | 0 | 0 | |
| α (1/K) | 7.5788 × 10−6 | 0 | 6.638 × 10−4 | −3.147 × 10−7 | 0 | |
| κ (W/m K) | 1.0 | 0 | 1.704 × 10−2 | 0 | 0 | |
| Stainless steel | E (Pa) | 201.04 × 109 | 0 | 3.079 × 10−4 | −6.534 × 10−7 | 0 |
| (SS304) | ν | 0.3262 | 0 | −2.002 × 10−4 | 3.797 × 10−7 | 0 |
| ρ (kg/m3) | 8166 | 0 | 0 | 0 | 0 | |
| α (1/K) | 12.330 × 10−6 | 0 | 8.086 × 10−4 | 0 | 0 | |
| κ (W/m K) | 15.379 | 0 | −1.264 × 10−3 | 2.092 × 10−6 | −7.223 × 10−10 |
| XT | XC | S | |
|---|---|---|---|
| Aluminum Oxide (Al2O3) | 210 | 2200 | 330 |
| Silicon Nitride (Si3N4) | 360 | 689 | 207.8 |
| Stainless Steel (SUS304) | 215 | 215 | 124 |
| Titanium alloy (Ti6Al4V) | 880 | 880 | 550 |
| Design Variables | Tsai-Hill Criteria | Mass (kg) | Cost (USD) | ||
|---|---|---|---|---|---|
| p | |||||
| Objective: min Mass | |||||
| Simulated Annealing (SA) | 0.2 | 37.8 | 0.999 | 31.5 | 1279 |
| Correia et al. [38] | 0.2 | 37.8 | 0.999 | 31.5 | 1279.24 |
| Objective: min Cost | |||||
| SA | 10 | 20.2 | 0.993 | 38.59 | 227 |
| Correia et al. [38] | 10 | 20.2 | 0.993 | 38.59 | 226.65 |
| Design Variables | Tsai-Hill Criteria | Mass (kg) | Cost (USD) | ||
|---|---|---|---|---|---|
| p | |||||
| Objective: min Mass | |||||
| SA | 0.2 | 34.2 | 0.999 | 38.69 | 886 |
| Correia et al. [38] | 0.2 | 34.2 | 0.9991 | 38.69 | 885.75 |
| Objective: min Cost | |||||
| SA | 2.9 | 53.2 | 0.999 | 93.72 | 752 |
| Correia et al. [38] | 2.9 | 53.2 | 1.0 | 93.72 | 752.32 |
| Design Variables | Mass (kg) | Cost (USD) | |||
|---|---|---|---|---|---|
| (mm) | |||||
| SA | 3.4 | 59.1 | 8000 | 101.20 | 1076.50 |
| Correia et al. [37] | 3.4 | 59.1 | 8000 | 101.20 | 1076.50 |
| Moita et al. [33] | 3.8 | 59.5 | 8000 | 103.74 | - |
| Design Variables | (10−3 m) | Mass (kg) | Cost (USD) | ||||
|---|---|---|---|---|---|---|---|
| (mm) | p | ||||||
| SUS304/Si3N4 | |||||||
| objective: min. Mass | 7.3 | 0 | 2.1331 | 2015 | 3.95 | 197 | S1 |
| objective: min. Cost | 15.7 | 10 | 1.0457 | 2010 | 27.37 | 161 | S2 |
| SUS304/Al2O3 | |||||||
| objective: min. Mass | 9.3 | 0 | 1.5913 | 2003 | 8.06 | 221 | S3 |
| objective: min. Cost | 15.8 | 10 | 1.7380 | 2003 | 28.01 | 131 | S4 |
| Ti6Al4V/Si3N4 | |||||||
| objective: min. Mass | 7.3 | 0 | 2.1331 | 2014 | 3.95 | 197 | S5 |
| objective: min. Cost | 7.3 | 0 | 2.1331 | 2014 | 3.95 | 197 | S6 |
| Ti6Al4V/Al2O3 | |||||||
| objective: min. Mass | 9.3 | 0 | 1.5573 | 2014 | 8.06 | 221 | S7 |
| objective: min. Cost | 9.3 | 0 | 1.5573 | 2014 | 8.06 | 221 | S8 |
| Design Variables | p | (10−3 m) | Mass (kg) | Cost (USD) | |||||
|---|---|---|---|---|---|---|---|---|---|
| (mm) | (mm) | (mm) | |||||||
| SUS304/Si3N4 | |||||||||
| objective: min. Mass | 0 | 6.3 | 1.0 | 0 | 2.2277 | 2014 | 3.95 | 197 | S1′ |
| objective: min. Cost | 5.7 | 5.9 | 0.5 | 0 | 1.706 | 2004 | 14.08 | 205 | S2′ |
| SUS304/Al2O3 | |||||||||
| objective: min. Mass | 0 | 9.3 | 0 | 0 | 1.5631 | 2014 | 8.06 | 221 | S3′ |
| objective: min. Cost | 0 | 15.8 | 0 | 10 | 1.7380 | 2003 | 28.01 | 131 | S4′ |
| Ti6Al4V/Si3N4 | |||||||||
| objective: min. Mass | 0 | 7.3 | 0 | 0 | 2.1952 | 2002 | 3.95 | 197 | S5′ |
| objective: min. Cost | 0 | 7.3 | 0 | 0 | 2.1952 | 2002 | 3.95 | 197 | S6′ |
| Ti6Al4V/Al2O3 | |||||||||
| objective: min. Mass | 0 | 9.3 | 0 | 0 | 1.5997 | 2003 | 8.06 | 221 | S7′ |
| objective: min. Cost | 0 | 8.2 | 1.1 | 0 | 1.6054 | 2014 | 8.06 | 221 | S8′ |
| Design Variables | δmax (×10−3 mm) | Elastic Strain Energy (J/m2) | Mass (kg) | Cost (USD) |
|---|---|---|---|---|
| 1.8741 | 33.094 | 5.41 | 270.4 | |
| Ti6Al4V/Si3N4 and SUS304/Si3N4 | ||||
| 1.8912 | 3286 | 8.67 | 237.6 | |
| SU304/Al2O3 and Ti6Al4V/Al2O3 |
| Design Variables | Tsai-Hill Criteria | Elastic Strain Energy (J/m2) | Mass (kg) | Cost (USD) |
|---|---|---|---|---|
| 0.10368 | 47.66 | 7.17 | 298.7 | |
| Ti6Al4V/Si3N4 | ||||
| 0.10425 | 47.88 | 9.01 | 261.5 | |
| Ti6Al4V/Al2O3 | ||||
| 0.27673 | 39.10 | 12.41 | 211.0 | |
| SUS304/Al2O3 | ||||
| 0.27738 | 399 | 10.37 | 296.1 | |
| SUS304/Si3N4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Franco Correia, V.; Moita, J.S.; Moleiro, F.; Soares, C.M.M. Optimization of Metal–Ceramic Functionally Graded Plates Using the Simulated Annealing Algorithm. Appl. Sci. 2021, 11, 729. https://doi.org/10.3390/app11020729
Franco Correia V, Moita JS, Moleiro F, Soares CMM. Optimization of Metal–Ceramic Functionally Graded Plates Using the Simulated Annealing Algorithm. Applied Sciences. 2021; 11(2):729. https://doi.org/10.3390/app11020729
Chicago/Turabian StyleFranco Correia, Victor, José S. Moita, Filipa Moleiro, and Cristóvão M. Mota Soares. 2021. "Optimization of Metal–Ceramic Functionally Graded Plates Using the Simulated Annealing Algorithm" Applied Sciences 11, no. 2: 729. https://doi.org/10.3390/app11020729
APA StyleFranco Correia, V., Moita, J. S., Moleiro, F., & Soares, C. M. M. (2021). Optimization of Metal–Ceramic Functionally Graded Plates Using the Simulated Annealing Algorithm. Applied Sciences, 11(2), 729. https://doi.org/10.3390/app11020729






