3D Knee Loading during Stationary Cycling: A Comprehensive Model Development and Reliability Analysis
Abstract
:1. Introduction
2. Materials and Methods
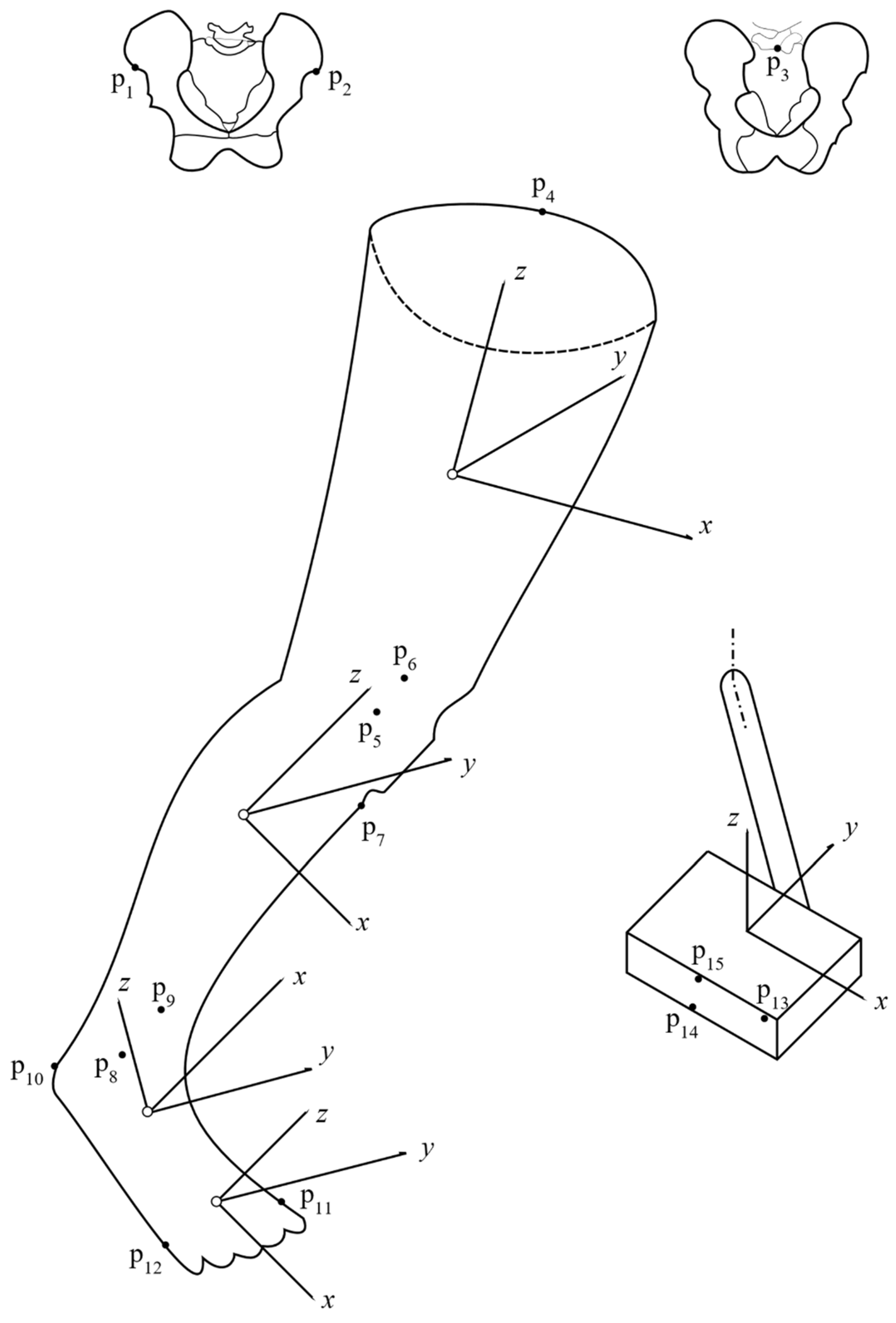
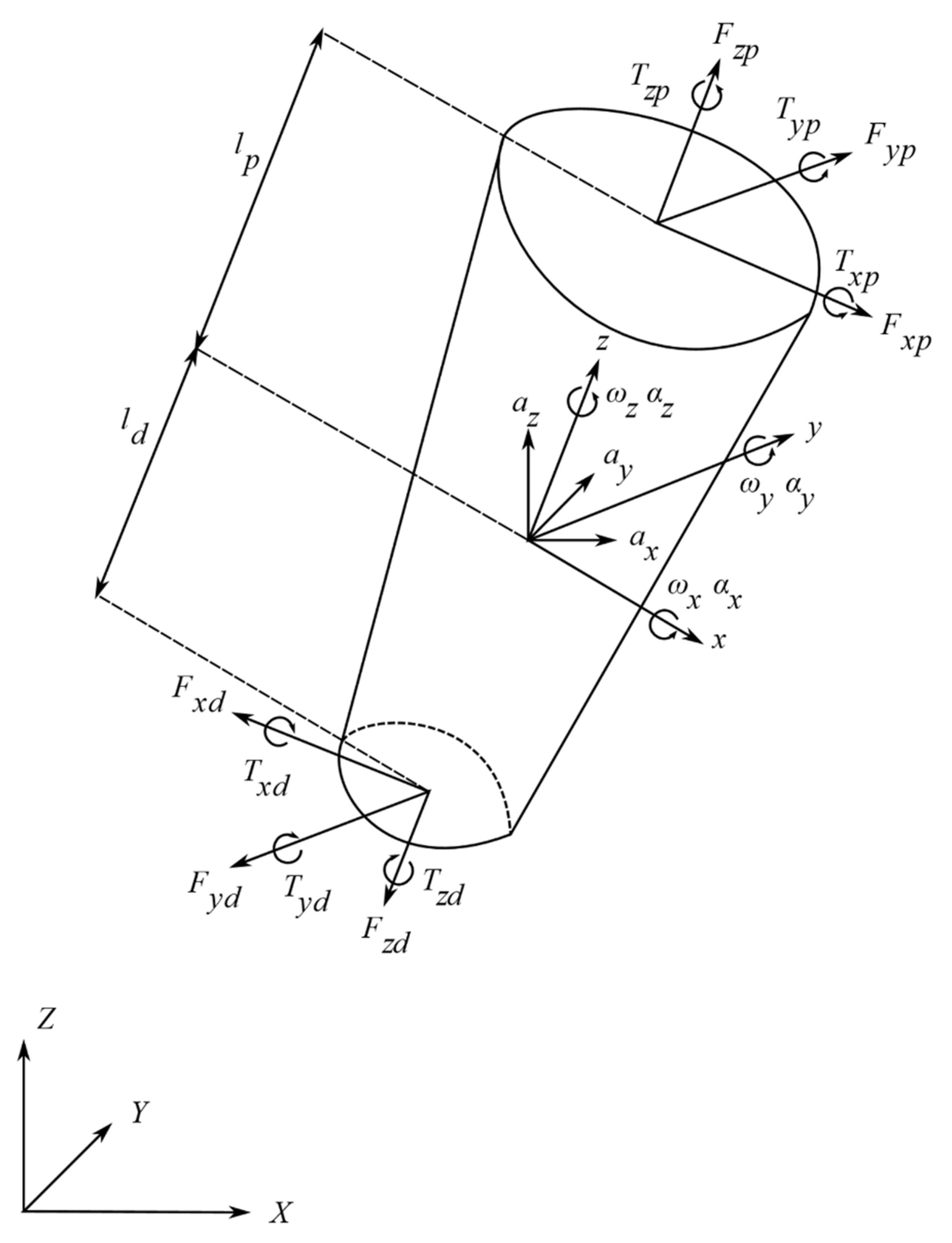
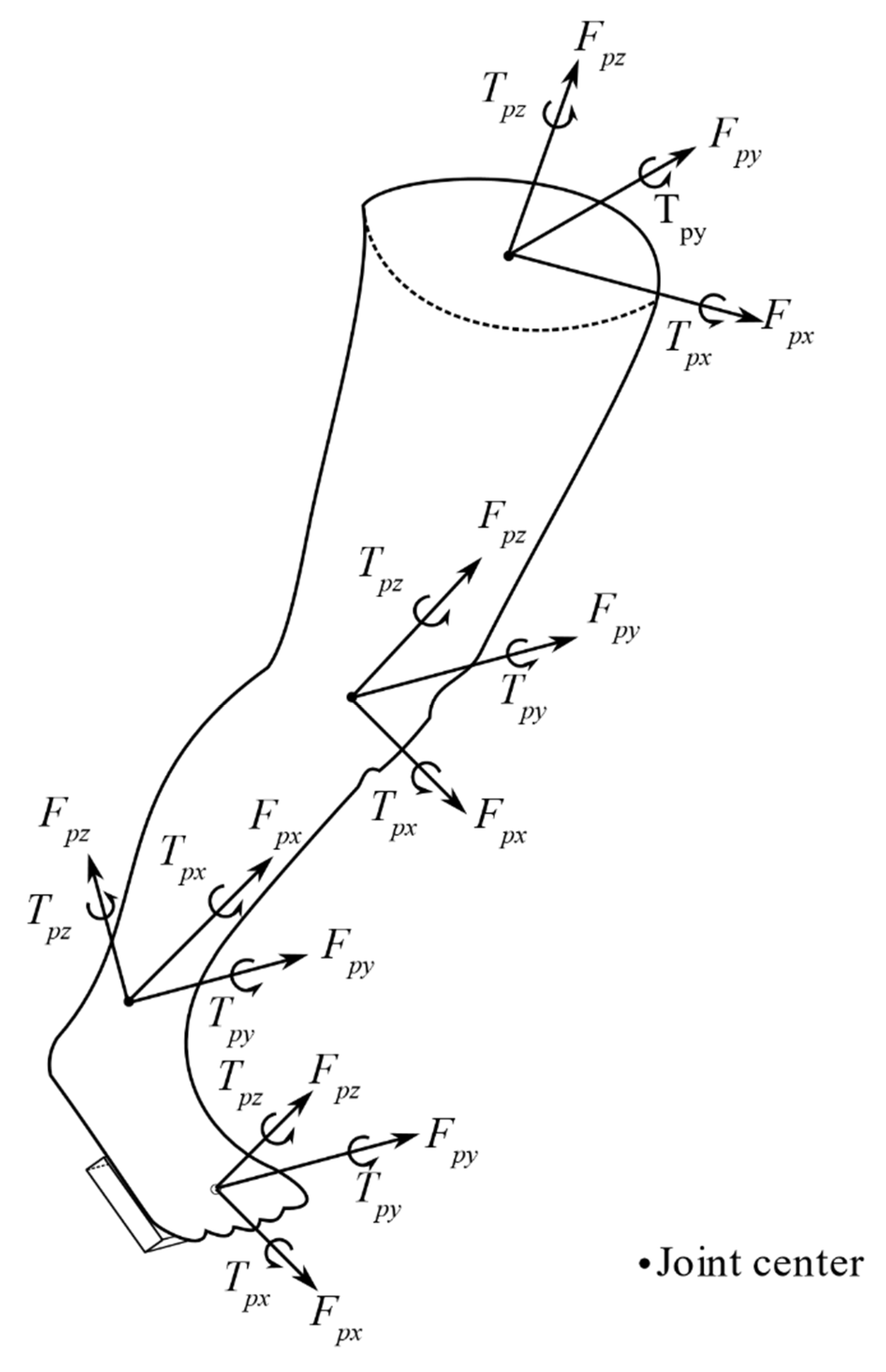
2.1. Analytic Model
2.1.1. Static Calibration
2.1.2. Trial Measurements
2.2. Protocol
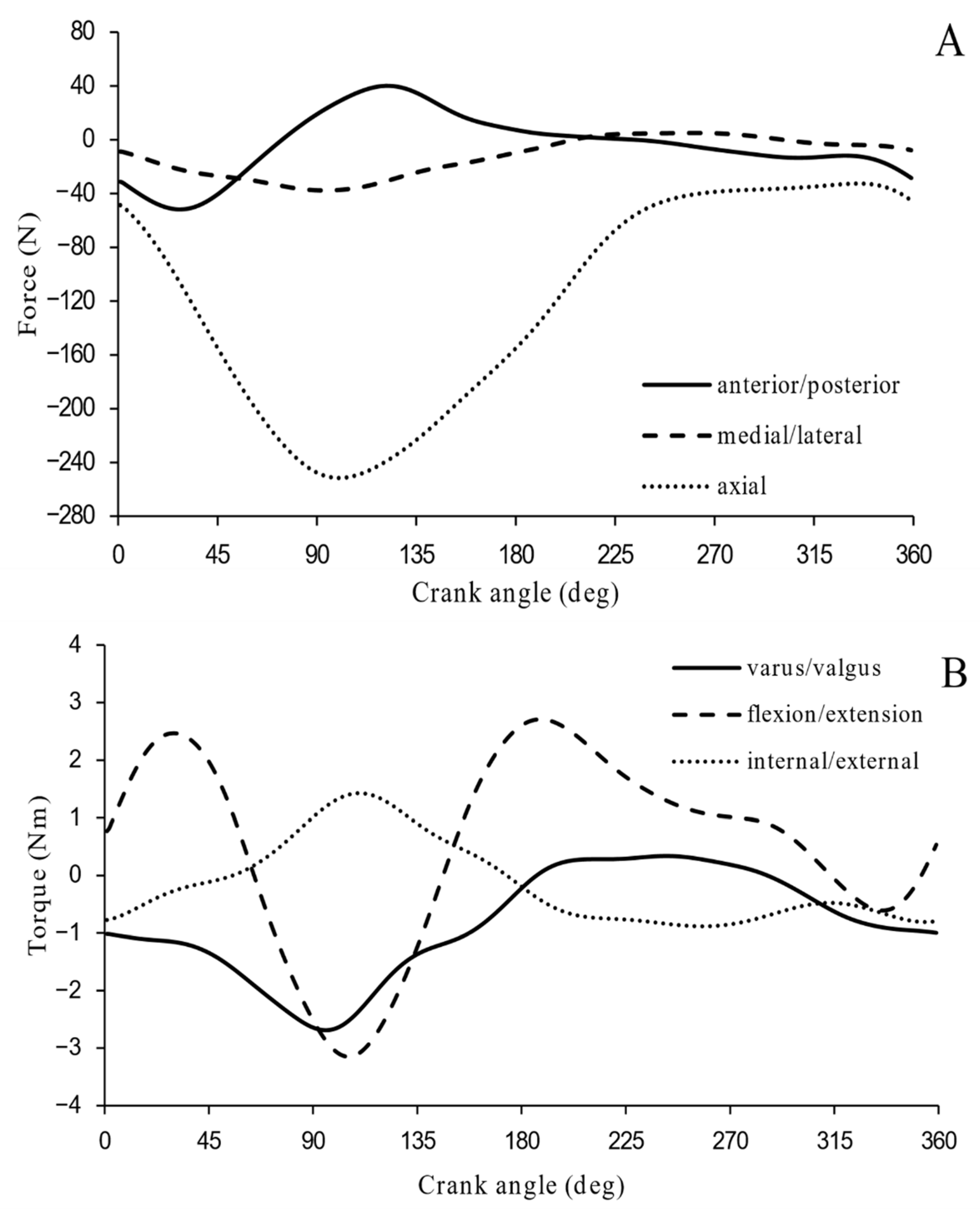
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- De Bernardo, N.; Barrios, C.; Vera, P.; Laíz, C.; Hadala, M. Incidence and risk for traumatic and overuse injuries in top-level road cyclists. J. Sports Sci. 2012, 30, 1047–1053. [Google Scholar] [CrossRef] [PubMed]
- Swart, J.; Holliday, W. Cycling Biomechanics Optimization—The (R) Evolution of Bicycle Fitting. Curr. Sports Med. Rep. 2019. [Google Scholar] [CrossRef] [PubMed]
- Salacinski, A.J.; Krohn, K.; Sco, F.L.; Megan, L.H.; Ireland, K.; Marchei, G. The effects of group cycling on gait and pain-related disability in individuals with mild-to-moderate knee osteoarthritis: A randomized controlled trial. J. Orthop. Sports Phys. Ther. 2012. [Google Scholar] [CrossRef]
- Bertucci, W.; Grappe, F.; Girard, A.; Betik, A.; Rouillon, J.D. Effects on the crank torque profile when changing pedalling cadence in level ground and uphill road cycling. J. Biomech. 2005, 38, 1003–1010. [Google Scholar] [CrossRef] [PubMed]
- Fonda, B.; Sarabon, N. Biomechanics of Cycling (Literature review). Sport Sci. Rev. 2010, 19, 187–210. [Google Scholar] [CrossRef]
- Callaghan, M.J. Lower body problems and injury in cycling. J. Bodyw. Mov. Ther. 2005, 9, 226–236. [Google Scholar] [CrossRef]
- Bini, R.; Flores-Bini, A. Potential factors associated with knee pain in cyclists: A systematic review. Open Access J. Sports Med. 2018. [Google Scholar] [CrossRef] [Green Version]
- Faria, E.W.; Parker, D.L.; Faria, I.E. The science of cycling: Factors affecting performance—Part 2. Sports Med. 2005, 35, 313–337. [Google Scholar] [CrossRef]
- Asplund, C.; St Pierre, P. Knee pain and bicycling: Fitting concepts for clinicians. Phys. Sportsmed. 2004. [Google Scholar] [CrossRef] [Green Version]
- Fang, Y.; Fitzhugh, E.C.; Crouter, S.E.; Gardner, J.K.; Zhang, S. Effects of workloads and cadences on frontal plane knee biomechanics in cycling. Med. Sci. Sports Exerc. 2016, 48, 260–266. [Google Scholar] [CrossRef]
- Ruby, P.; Hull, M.L.; Hawkins, D. Three-dimensional knee joint loading during seated cycling. J. Biomech. 1992, 25, 41–53. [Google Scholar] [CrossRef]
- Gregersen, C.S.; Hull, M.L. Non-driving intersegmental knee moments in cycling computed using a model that includes three-dimensional kinematics of the shank/foot and the effect of simplifying assumptions. J. Biomech. 2003, 36, 803–813. [Google Scholar] [CrossRef]
- Vigotsky, A.D.; Zelik, K.E.; Lake, J.; Hinrichs, R.N. Mechanical misconceptions: Have we lost the “mechanics” in “sports biomechanics”? J. Biomech. 2019, 93, 1–5. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Winter, D.A. Biomechanics and Motor Control of Human Movement; John Wiley & Sons: Hoboken, NJ, USA, 2009; ISBN 9780470549148. [Google Scholar]
- Vaughan, C.L.; Davis, B.L.; O’Connor, J.C. DYNAMICS OF HUMAN GAIT; Kiboho Publishers: Cape Town, South Africa, 1999; ISBN 0-620-23558-6. [Google Scholar]
- Yeadon, M.R.; Morlock, M. The appropriate use of regression equations for the estimation of segmental inertia parameters. J. Biomech. 1989. [Google Scholar] [CrossRef]
- Vaeck, J.; Bouillid, A.; Bouzigon, R.; Pinot, J.; Grappe, F. Accuracy of the new Elite Drivo ergometer. In Proceedings of the Science & Cycling Congress, Düsseldorf, Germany, 28–29 June 2017. [Google Scholar]
- Manal, K.; McClay, I.; Richards, J.; Galinat, B.; Stanhope, S. Knee moment profiles during walking: Errors due to soft tissue movement of the shank and the influence of the reference coordinate system. Gait Posture 2002, 15, 10–17. [Google Scholar] [CrossRef]
- Bini, R.R.; Diefenthaeler, F.; Mota, C.B. Fatigue effects on the coordinative pattern during cycling: Kinetics and kinematics evaluation. J. Electromyogr. Kinesiol. 2010. [Google Scholar] [CrossRef]
- Costa-Santos, C.; Bernardes, J.; Ayres-De-Campos, D.; Costa, A.; Costa, C. The limits of agreement and the intraclass correlation coefficient may be inconsistent in the interpretation of agreement. J. Clin. Epidemiol. 2011, 64, 264–269. [Google Scholar] [CrossRef]
- Ruby, P.; Hull, M.L. Response of intersegmental knee loads to foot/pedal platform degrees of freedom in cycling. J. Biomech. 1993, 26, 1327–1340. [Google Scholar] [CrossRef]
- Bini, R.R.; Hume, P.A. Effects of workload and pedalling cadence on knee forces in competitive cyclists. Sports Biomech. 2013, 12, 93–107. [Google Scholar] [CrossRef]
- Thompson, R.L.; Gardner, J.K.; Zhang, S.; Reinbolt, J.A. Lower-limb joint reaction forces and moments during modified cycling in healthy controls and individuals with knee osteoarthritis. Clin. Biomech. 2020. [Google Scholar] [CrossRef]
- Delp, S.L.; Anderson, F.C.; Arnold, A.S.; Loan, P.; Habib, A.; John, C.T.; Guendelman, E.; Thelen, D.G. OpenSim: Open-source software to create and analyze dynamic simulations of movement. IEEE Trans. Biomed. Eng. 2007. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Seth, A.; Sherman, M.; Reinbolt, J.A.; Delp, S.L. OpenSim: A musculoskeletal modeling and simulation framework for in silico investigations and exchange. Procedia Iutam 2011, 2, 212–232. [Google Scholar] [CrossRef] [PubMed]

| Variable | Mean Difference ± SD | Cronbach’s Alpha | ICC | p-Value |
|---|---|---|---|---|
| Tx—inversion/eversion torque | ||||
| max (Nm) | 0.1 ± 0.1 | 0.958 | 0.963 | 0.639 |
| min (Nm) | 0.0 ± 0.0 | 0.991 | 0.985 | 0.052 |
| max (deg) | 10.1 ± 14.2 | 0.846 | 0.818 | 0.187 |
| min (deg) | 12.6 ± 13.6 | 0.923 | 0.880 | 0.058 |
| Tz—internal/external rotation torque | ||||
| max (Nm) | 0.3 ± 0.2 | 0.891 | 0.906 | 0.973 |
| min (Nm) | 0.3 ± 0.3 | 0.700 | 0.735 | 0.938 |
| max (deg) | 5.4 ± 8.5 | 0.843 | 0.817 | 0.171 |
| min (deg) | 20.3 ± 32.3 | 0.791 | 0.776 | 0.271 |
| Fx—tangential force | ||||
| max (N) | 5.5 ± 3.0 | 0.971 | 0.975 | 0.802 |
| min (N) | 4.4 ± 4.0 | 0.987 | 0.989 | 0.789 |
| max (deg) | 23.7 ± 28.5 | 0.781 | 0.784 | 0.393 |
| min (deg) | 4.1 ± 2.2 | 0.997 | 0.937 | 0.010 |
| Fy—transverse force | ||||
| max (N) | 4.0 ± 2.8 | −0.370 | −0.460 | 0.980 |
| min (N) | 4.1 ± 2.2 | 0.978 | 0.964 | 0.055 |
| max (deg) | 19.9 ± 19.9 | 0.907 | 0.865 | 0.098 |
| min (deg) | 3.1 ± 2.6 | 0.945 | 0.937 | 0.202 |
| Fz—normal force | ||||
| max (N) | 10.7 ± 9.0 | 0.884 | 0.896 | 0.697 |
| min (N) | 23.8 ± 16.9 | 0.949 | 0.868 | 0.010 |
| max (deg) | 13.1 ± 12.4 | 0.831 | 0.831 | 0.358 |
| min (deg) | 7.0 ± 5.7 | 0.872 | 0.860 | 0.239 |
| Variable | Mean Difference ± SD | Cronbach’s Alpha | ICC | p-Value |
|---|---|---|---|---|
| Tx—varus/valgus torque | ||||
| max (Nm) | 0.1 ± 0.1 | 0.962 | 0.951 | 0.132 |
| min (Nm) | 0.3 ± 0.2 | 0.975 | 0.970 | 0.174 |
| max (deg) | 25.0 ± 19.6 | 0.714 | 0.740 | 0.629 |
| min (deg) | 4.9 ± 4.1 | 0.966 | 0.934 | 0.073 |
| Ty—flexion/extension torque | ||||
| max (Nm) | 0.8 ± 0.3 | 0.979 | 0.981 | 0.535 |
| min (Nm) | 1.3 ± 0.9 | 0.959 | 0.955 | 0.238 |
| max (deg) | 37.0 ± 32.9 | 0.850 | 0.875 | 0.811 |
| min (deg) | 6.6 ± 13.0 | 0.953 | 0.957 | 0.501 |
| Tz—internal/external rotation torque | ||||
| max (Nm) | 0.23 ± 0.2 | 0.927 | 0.934 | 0.633 |
| min (Nm) | 0.3 ± 0.2 | 0.861 | 0.881 | 0.945 |
| max (deg) | 7.7 ± 8.2 | 0.829 | 0.759 | 0.095 |
| min (deg) | 10.7 ± 9.7 | 0.878 | 0.893 | 0.866 |
| Fx—anterior/posterior force | ||||
| max (N) | 18.1 ± 12.2 | 0.966 | 0.950 | 0.079 |
| min (N) | 8.3 ± 8.7 | 0.982 | 0.978 | 0.161 |
| max (deg) | 10.4 ± 13.8 | 0.941 | 0.827 | 0.012 |
| min (deg) | 2.3 ± 2.2 | 0.980 | 0.978 | 0.245 |
| Fy—medial/lateral force | ||||
| max (N) | 4.1 ± 1.9 | −1.139 | −1.628 | 0.903 |
| min (N) | 3.2 ± 2.3 | 0.962 | 0.957 | 0.202 |
| max (deg) | 22.4 ± 17.5 | 0.753 | 0.781 | 0.740 |
| min (deg) | 5.4 ± 2.9 | 0.932 | 0.941 | 0.912 |
| Fz—axial force | ||||
| max (N) | 9 ± 4.1 | 0.987 | 0.929 | 0.001 |
| min (N) | 15.9 ± 7.2 | 0.994 | 0.965 | 0.001 |
| max (deg) | 11.1 ± 11.7 | 0.914 | 0.913 | 0.346 |
| min (deg) | 7.9 ± 7.1 | 0.949 | 0.920 | 0.061 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kozlovič, P.; Šarabon, N.; Fonda, B. 3D Knee Loading during Stationary Cycling: A Comprehensive Model Development and Reliability Analysis. Appl. Sci. 2021, 11, 528. https://doi.org/10.3390/app11020528
Kozlovič P, Šarabon N, Fonda B. 3D Knee Loading during Stationary Cycling: A Comprehensive Model Development and Reliability Analysis. Applied Sciences. 2021; 11(2):528. https://doi.org/10.3390/app11020528
Chicago/Turabian StyleKozlovič, Peter, Nejc Šarabon, and Borut Fonda. 2021. "3D Knee Loading during Stationary Cycling: A Comprehensive Model Development and Reliability Analysis" Applied Sciences 11, no. 2: 528. https://doi.org/10.3390/app11020528
APA StyleKozlovič, P., Šarabon, N., & Fonda, B. (2021). 3D Knee Loading during Stationary Cycling: A Comprehensive Model Development and Reliability Analysis. Applied Sciences, 11(2), 528. https://doi.org/10.3390/app11020528







