Simulation Verification of the Contact Parameter Influence on the Forces’ Course of Cereal Grain Impact against a Stiff Surface
Abstract
1. Introduction
2. Materials and Methods
2.1. Computer Simulation
2.2. Mathematical Contact Model
- m1, m2—particle mass (kg);
- S1, S2—contact force (N);
- t—time (s);
- v1, v2—velocity (m s−1).
- c1, c2—damping parameter (kg s−1);
- k1, k2—contact stiffness (N m−1);
- x1, x2—overlap (m).
- tk—contact time (s).
- − Poisson parameter—0.2 (-);
- − Kirchhoff’s module—9.0 (MPa);
- − Particle friction parameter—0.25 (-);
- − Damping parameter—1.0 (Ns·m−1);
- − Grain diameter—5.0 (mm);
- − Grain velocity—2.0 (m·s−1);
- − Grain density—765 (kg·m−3).
- − Sensor diameter—10.0 (mm);
- − Disc thickness—2.0 (mm).
- − Constant contact stiffness—k1 = 1 × 104 (N·m−1), k2 = 1 × 105 (N·m−1),
- − Force sensor resonance frequency—f = 20, 40, and 200 kHz.
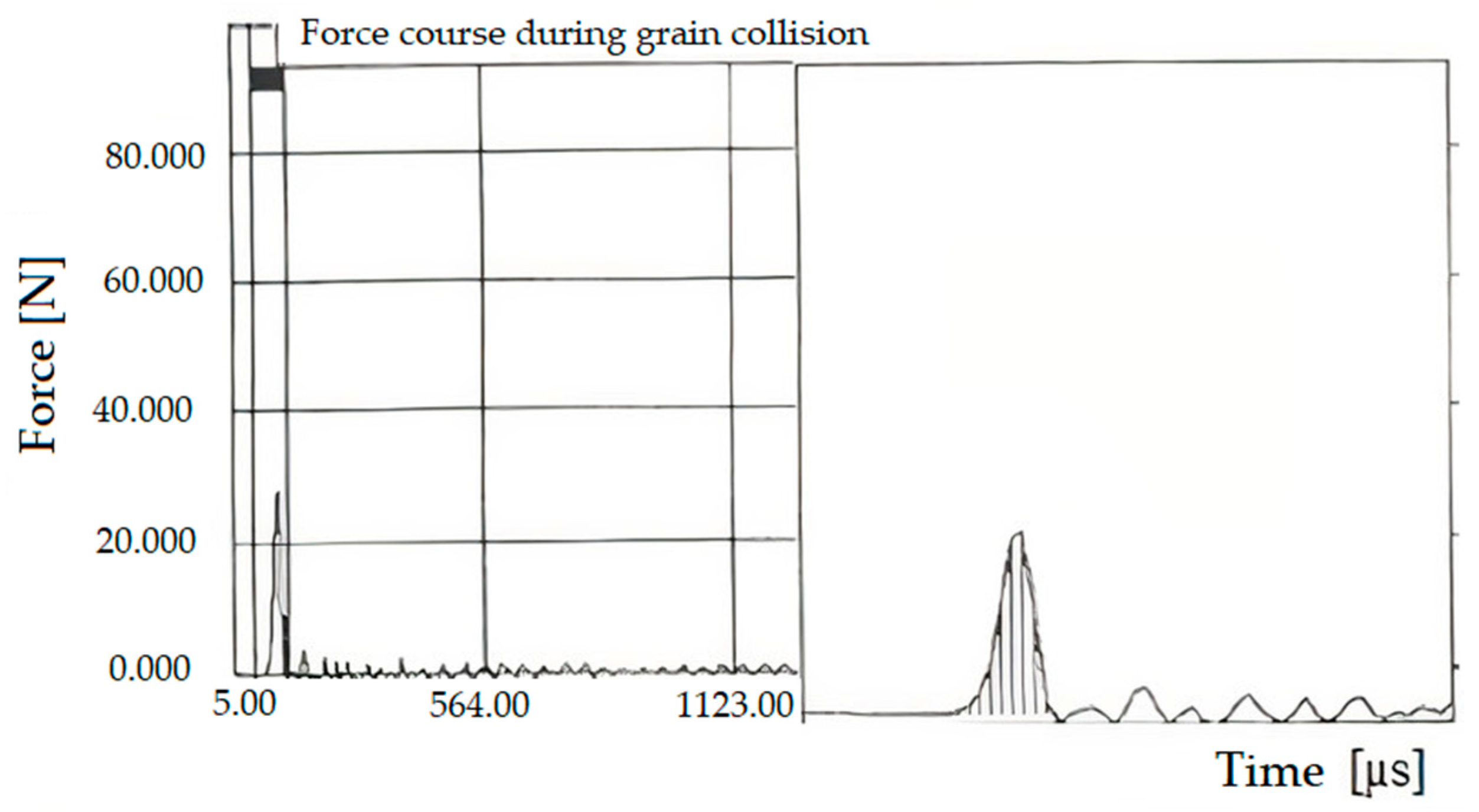
3. Results
4. Conclusions
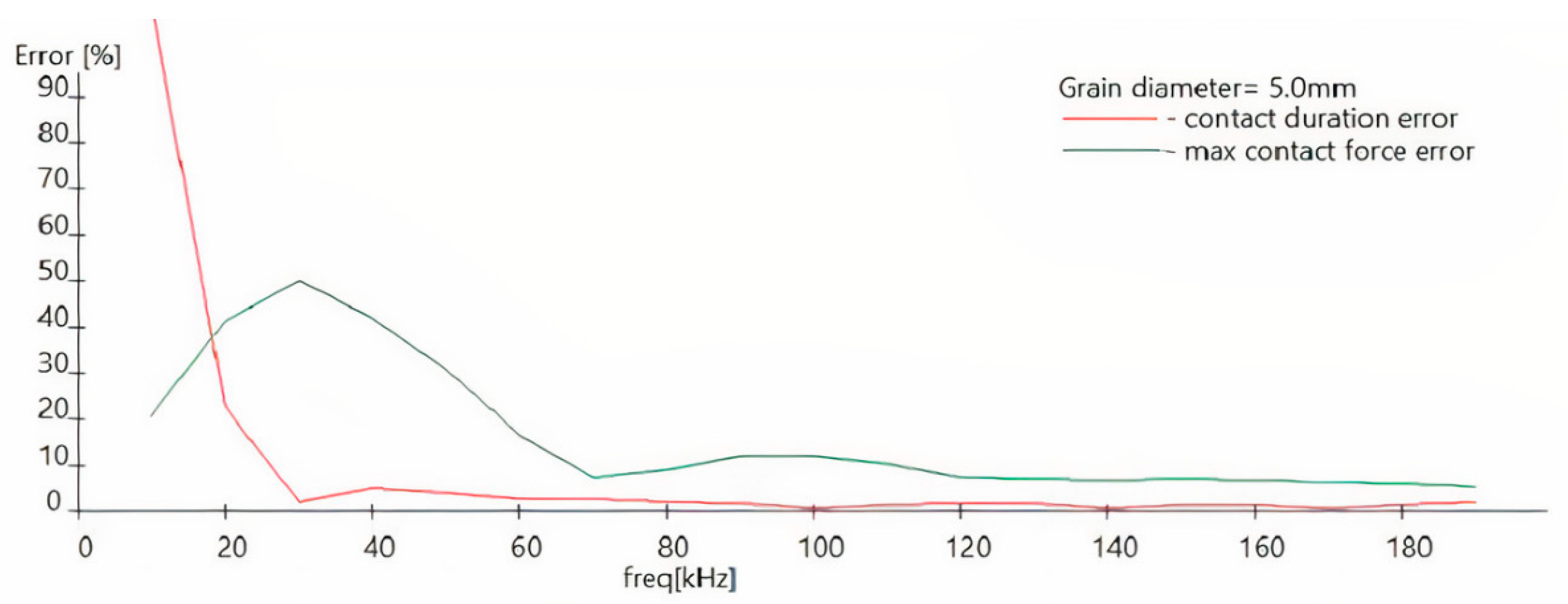
- The key influence on the precision of impact force course modelling was the frequency of the free vibrations (resonance frequency) of the force sensor and the grain size.
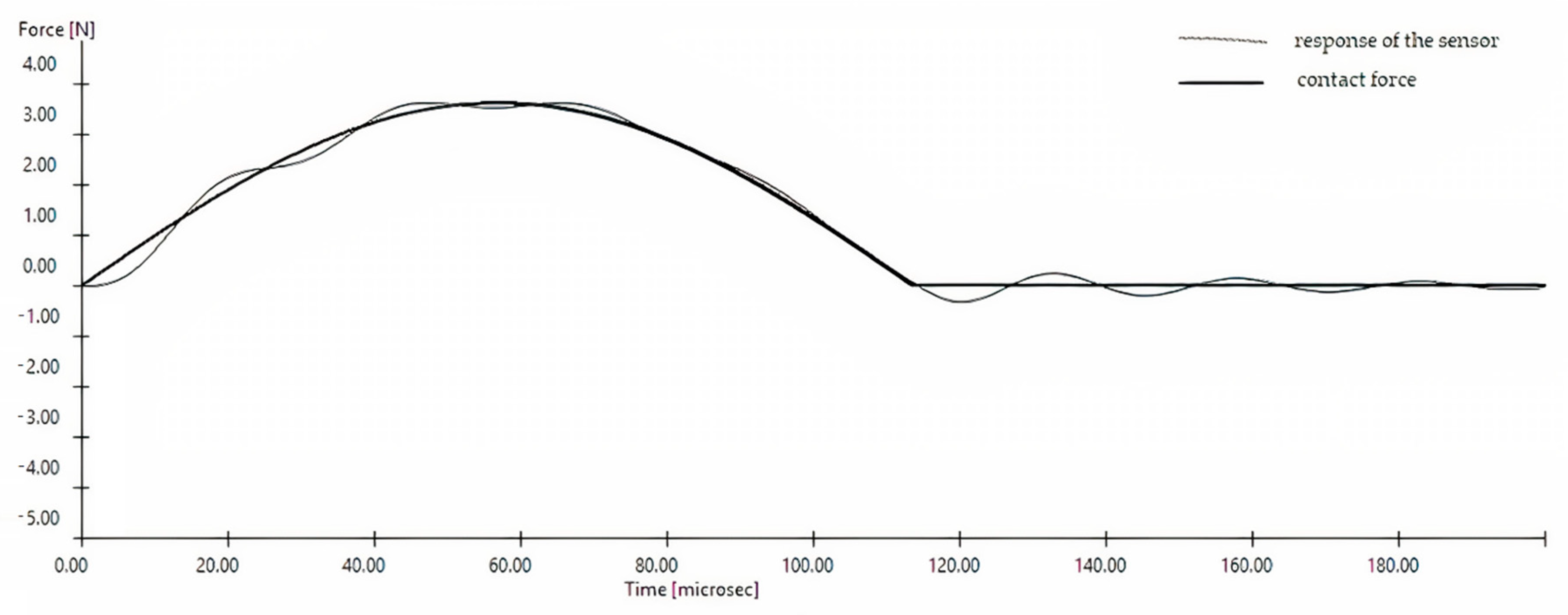
- If the duration of the contact force pulse was longer than half of the sensor’s own vibration period, the measurement signal coincided significantly with the contact force course. This occurred for large and soft grains with a higher moisture content.
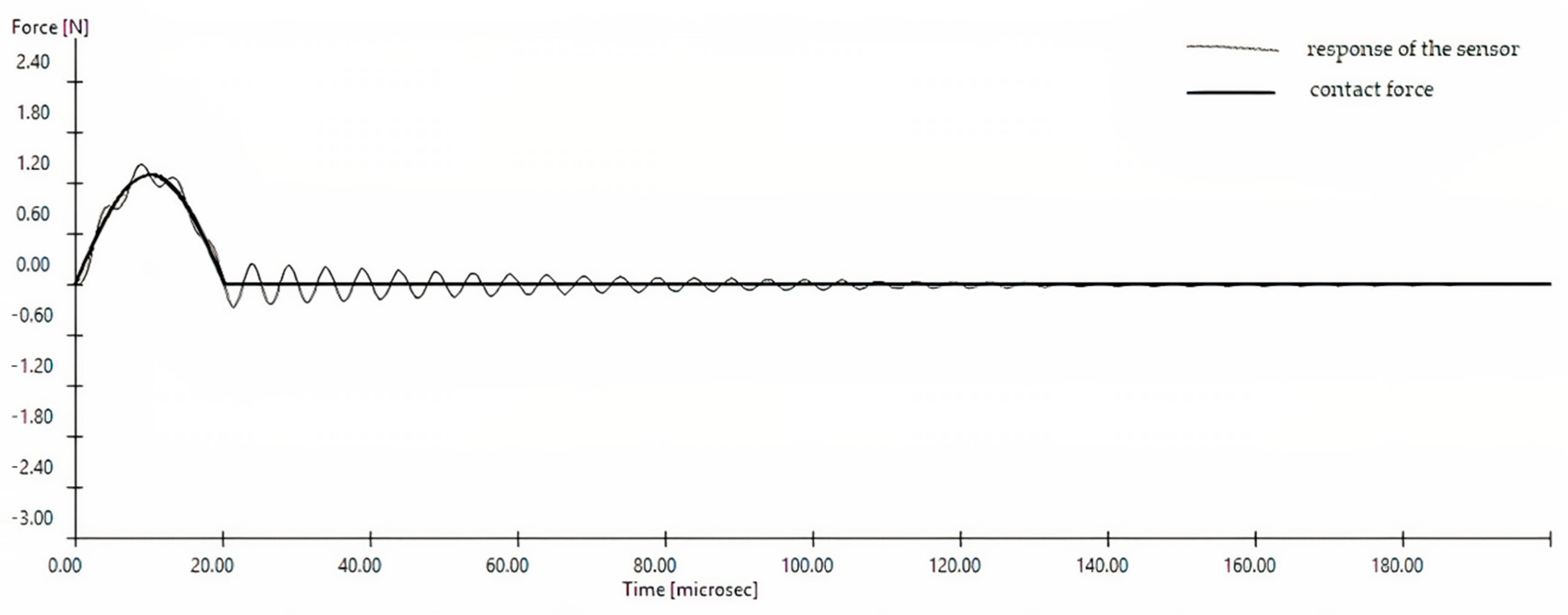
- If the duration of the contact force pulse was shorter than half of the sensor’s own vibration period, the results of the contact force measurement were not reliable because the first cycle of the measurement signal lasted longer than the actual contact time. Moreover, with hard fine grain, the signal itself was always close to half a sinusoid of a period corresponding to the resonance vibration period of the force sensor, regardless of the shape of the contact force pulse resulting from the shape of the grain mechanical properties.
- For large grains (e.g., maize, pea, and Pisum arvense) with a diameter greater than 5.0 mm, the modelling of the impact force by the sensor response signal with free vibrations of approximately 40 kHz could be considered adequate.
- For fine grains (e.g., rape) with a diameter of less than 2.0 mm, the exact modelling could only be obtained by a sensor with a free vibration frequency exceeding 200 kHz. Piezoelectric force sensors available for sale have a free vibration frequency of 70 kHz, which does not allow the credible impact force value to be measured for fine grain e.g., rape and mustard.
- To reliably measure the course of the impact force, impact force sensors of a special design with high resonance frequency should be used. The technological capabilities of manufacturers of such sensors allow resonance frequencies of up to 200 kHz.
- The most crucial factors influencing the measurement accuracy of the grain impact force course were the grain dimensions (diameter) and the force sensor resonance frequency.
- In DEM calculations, where the contact force course is of key importance, the empirical research results of the force courses for fine grain should be approached carefully, by only relying on theoretical models (rheological) of the force courses.
Author Contributions
Funding
Conflicts of Interest
References
- Anand, A.; Curtis, J.S.; Wassgren, C.R.; Hancock, B.C.; Ketterhagen, W.R. Predicting discharge dynamics of wet cohesive particles from a rectangular hopper using the discrete element method (DEM). Chem. Eng. Sci. 2009, 64, 5268–5275. [Google Scholar] [CrossRef]
- Raji, A.O.; Favier, J.F. Model for deformation in agricultural and food particulate materials under bulk compressive loading using discrete element method. II: Compression of oilseeds. J. Food Eng. 2004, 64, 373–380. [Google Scholar] [CrossRef]
- Sakaguchi, E.; Kawakami, S.; Tobita, F. Simulation of Flowing Phenomena of Grains by Distinct Element Method; Report No. 94-G-025; Ageng’ 94: Milano, Italy, 1994; pp. 1–8. [Google Scholar]
- Cundall, P.A.; Strack, O.D. A discrete element model for granular assemblies. Geotehnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Malone, K.F.; Xu, B.H. Determination of contact parameters for discrete element method simulations of granular systems. Particuology 2008, 6, 521–528. [Google Scholar] [CrossRef]
- Tijskens, E.; Ramon, H.; Baerdemaeker, J. Discrete element modelling for process simulation in agriculture. J. Sound Vib. 2003, 266, 493–514. [Google Scholar] [CrossRef]
- Mishra, B.; Murty, C. On the determination of contact parameters for realistic DEM simulations of ball mills. Powder Technol. 2001, 115, 290–297. [Google Scholar] [CrossRef]
- Fraczek, J. Method of measuring the force of internal friction of biological bulk materials. Zesz. Probl. Adv. Agric. Sci. 1998, 423, 93–101. (In Polish) [Google Scholar]
- Youngdahl, C.J.; Sanders, P.G.; Eastman, J.; Weertman, J.R. Compressive yield strengths of nanocrystalline Cu and Pd. Scr. Mater. 1997, 37, 809–813. [Google Scholar] [CrossRef]
- Jaeger, H.M.; Shinbrot, T.; Umbanhowar, P.B. Does the granular matter? Proc. Natl. Acad. Sci. USA 2000, 97, 12959–12960. [Google Scholar] [CrossRef]
- Kustermann, M. Stossartige Belastung von Maiskörner. Grundl. Landtech. 1987, 37, 121–131. [Google Scholar]
- Musson, R.W.; Carlson, W. Finite element study of the effect of material properties on reaction forces produced by solitary wave propagation in granular chains. Granul. Matter 2019, 18, 22. [Google Scholar] [CrossRef]
- Arslan, S. A Grain Flow Model to Simulate Grain Yield Sensor Response. Sensors 2008, 8, 952–962. [Google Scholar] [CrossRef] [PubMed]
- Reinke, R.; Dankowicz, H.; Phelan, J.; Kang, W. A dynamic grain flow model for a mass flow yield sensor on a combine. Precis. Agric. 2011, 12, 732–749. [Google Scholar] [CrossRef]
- Kęska, W. Research on the course of forces at the collision of seeds of some cultivated plants with the working surfaces of agricultural machines. Work. Ind. Inst. Agric. Mach. 1996, 41, 17–21. (In Polish) [Google Scholar]
- Horabik, J.; Molenda, M. Parameters and contact models for DEM simulations of agricultural granular materials: A review. Biosyst. Eng. 2016, 147, 206–225. [Google Scholar] [CrossRef]
- Wojtkowski, M.; Pecen, J.; Horabik, J.; Molenda, M. Rapeseed impact against a flat surface: Physical testing and DEM simulation with two contact models. Powder Technol. 2010, 198, 61–68. [Google Scholar] [CrossRef]
- Feder, S. Examination of the coefficient of grain velocity restitution in the separation process. Work. Ind. Inst. Agric. Mach. 1996, 41, 27–33. (In Polish) [Google Scholar]
- Michalak, D. Examination of mechanical properties of cereal grains. Work. Ind. Inst. Agric. Mach. 1996, 41, 22–26. (In Polish) [Google Scholar]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Munjiza, A. The Combined Finite-Discrete Element Method; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2004. [Google Scholar]
- Kruggel-Emden, H.; Simsek, E.; Rickelt, S.; Wirtz, S.; Scherer, V. Review and extension of normal force models for the Discrete Element Method. Powder Technol. 2007, 171, 157–173. [Google Scholar] [CrossRef]
- Thornton, C.; Ning, Z. A theoretical model for the stick/ bounce behaviour of adhesive, elasticeplastic spheres. Powder Technol. 1998, 99, 154–162. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Goodier, J.N. Theory of Elasticity; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- Weir, G.; Tallon, S. The coefficient of restitution for normal incident, low velocity particle impacts. Chem. Eng. Sci. 2005, 60, 3637–3647. [Google Scholar] [CrossRef]
- Chen, Z.; Wassgren, C.; Veikle, E.; Ambrose, R.K. Determination of material and interaction properties of maize and wheat kernels for DEM simulation. Biosyst. Eng. 2020, 195, 208–226. [Google Scholar] [CrossRef]
- Marinack, M.C., Jr.; Jasti, V.K.; Choi, Y.E.; Higgs, C.F.; Higgs, C.F. Couette grain flow experiments: The effects of the coefficient of restitution, global solid fraction, and materials. Powder Technol. 2011, 211, 144–155. [Google Scholar] [CrossRef]
- Chwaleba, A.; Ponięski, M.; Siedlecki, A. Electrical Metrology; WNT: Warszawa, Poland, 1998. (In Polish) [Google Scholar]
- Miłek, M. Measurements of Non-Electrical Quantities by Electrical Methods; Academic textbook ed.; Zielona Góra University of Technology: Zielona Góra, Poland, 1998. (In Polish) [Google Scholar]
- Romer, E. Industrial Measurement; PWN: Warszawa, Poland, 1978. (In Polish) [Google Scholar]
- Altintas, Y.; Eynian, M.; Onozuka, H. Identification of dynamic cutting force coefficients and chatter stability with process damping. CIRP Ann. Manuf. Technol. 2008, 57, 371–374. [Google Scholar] [CrossRef]
- Hagel, R.; Zakrzewski, J. Dynamic Measuremen; WNT: Warszawa, Poland, 1984. (In Polish) [Google Scholar]
- Szumielewicz, B.; Słomski, B.; Styburski, W. Electronic Measurements in Technolog; WNT: Warszawa, Poland, 1982. (In Polish) [Google Scholar]
- Kuwabara, G.; Kono, K. Restitution Coefficient in a Collision between Two Spheres. Jpn J. Appl. Phys. 1987, 26, 1230–1233. [Google Scholar] [CrossRef]
- Alizadeh, E.; Bertrand, F.; Chaouki, J. Development of a granular normal contact force model based on a non-Newtonian liquid filled dashpot. Powder Technol. 2013, 237, 202–212. [Google Scholar] [CrossRef]
- Hu, S.; Xinglin, G. A dissipative contact force model for impact analysis in multibody dynamics. Multibody Syst. Dyn. 2015, 35, 131–151. [Google Scholar] [CrossRef]
- Hu, Z.-Y.; Hua, W.; Zhang, L.; Deng, L.-B.; Wang, X.-F.; Liu, G.-H.; Hao, W.J.; Wang, H.Z. Seed struc-ture characteristics to form ultrahigh oil content in rapeseed. PLoS ONE 2013, 8, e62099. [Google Scholar]
- Hu, G.; Hu, Z.; Jian, B.; Liu, L.; Wan, H. On the determination of the damping coef-ficient of non-linear springdashpot system to model Hertz contact for simulation by discrete ele-ment method. J. Comput. 2011, 6, 984–988. [Google Scholar] [CrossRef]
- Van Zeebroeck, M.; Tijskens, E.; Van Liedekerke, P.; Deli, V.; De Baerdemaeker, J.; Ramon, H. Determination of the dynamical behaviour of biologicalmaterials during impact using apendulumdevice. J. Soundandvibration 2003, 266, 456–480. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kęska, W.; Marcinkiewicz, J.; Gierz, Ł.; Staszak, Ż.; Selech, J.; Koszela, K. Simulation Verification of the Contact Parameter Influence on the Forces’ Course of Cereal Grain Impact against a Stiff Surface. Appl. Sci. 2021, 11, 466. https://doi.org/10.3390/app11020466
Kęska W, Marcinkiewicz J, Gierz Ł, Staszak Ż, Selech J, Koszela K. Simulation Verification of the Contact Parameter Influence on the Forces’ Course of Cereal Grain Impact against a Stiff Surface. Applied Sciences. 2021; 11(2):466. https://doi.org/10.3390/app11020466
Chicago/Turabian StyleKęska, Włodzimierz, Jacek Marcinkiewicz, Łukasz Gierz, Żaneta Staszak, Jarosław Selech, and Krzysztof Koszela. 2021. "Simulation Verification of the Contact Parameter Influence on the Forces’ Course of Cereal Grain Impact against a Stiff Surface" Applied Sciences 11, no. 2: 466. https://doi.org/10.3390/app11020466
APA StyleKęska, W., Marcinkiewicz, J., Gierz, Ł., Staszak, Ż., Selech, J., & Koszela, K. (2021). Simulation Verification of the Contact Parameter Influence on the Forces’ Course of Cereal Grain Impact against a Stiff Surface. Applied Sciences, 11(2), 466. https://doi.org/10.3390/app11020466






