A Water/Ion Separation Device: Theoretical and Numerical Investigation
Abstract
:Featured Application
Abstract
1. Introduction
2. Materials and Methods
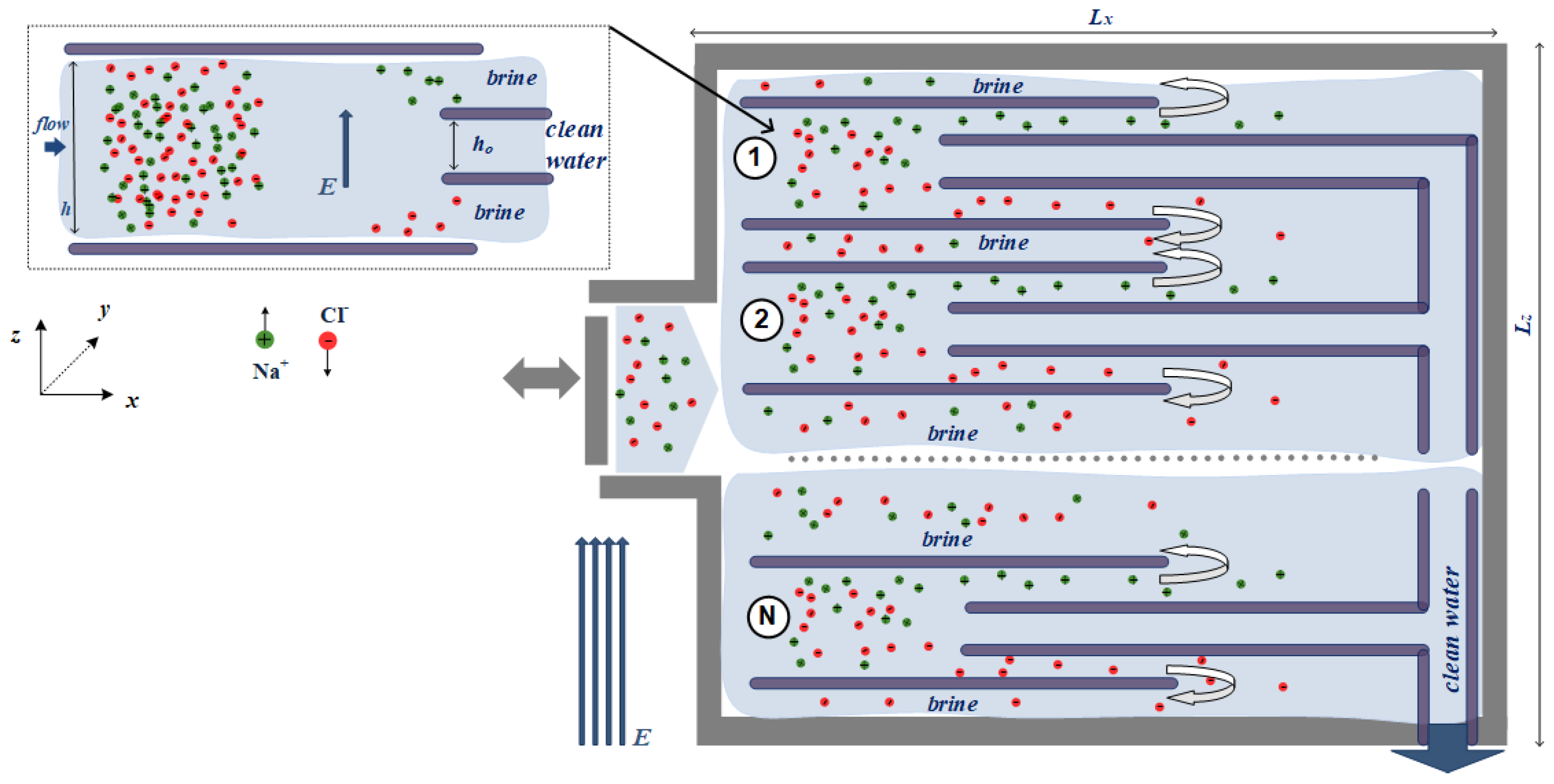
2.1. System Model
2.2. Mathematical Formulation
3. Results and Discussion
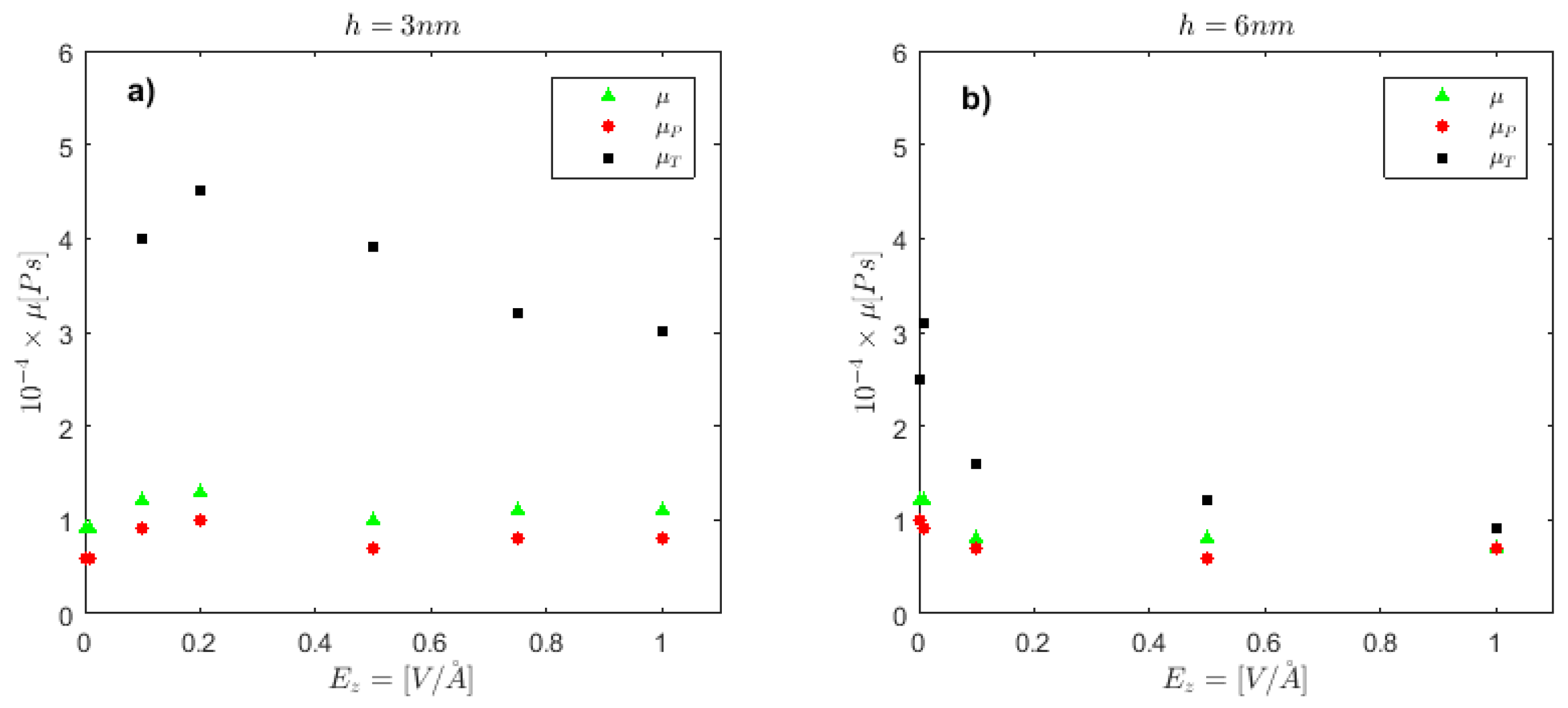
3.1. Shear Viscosity Calculations
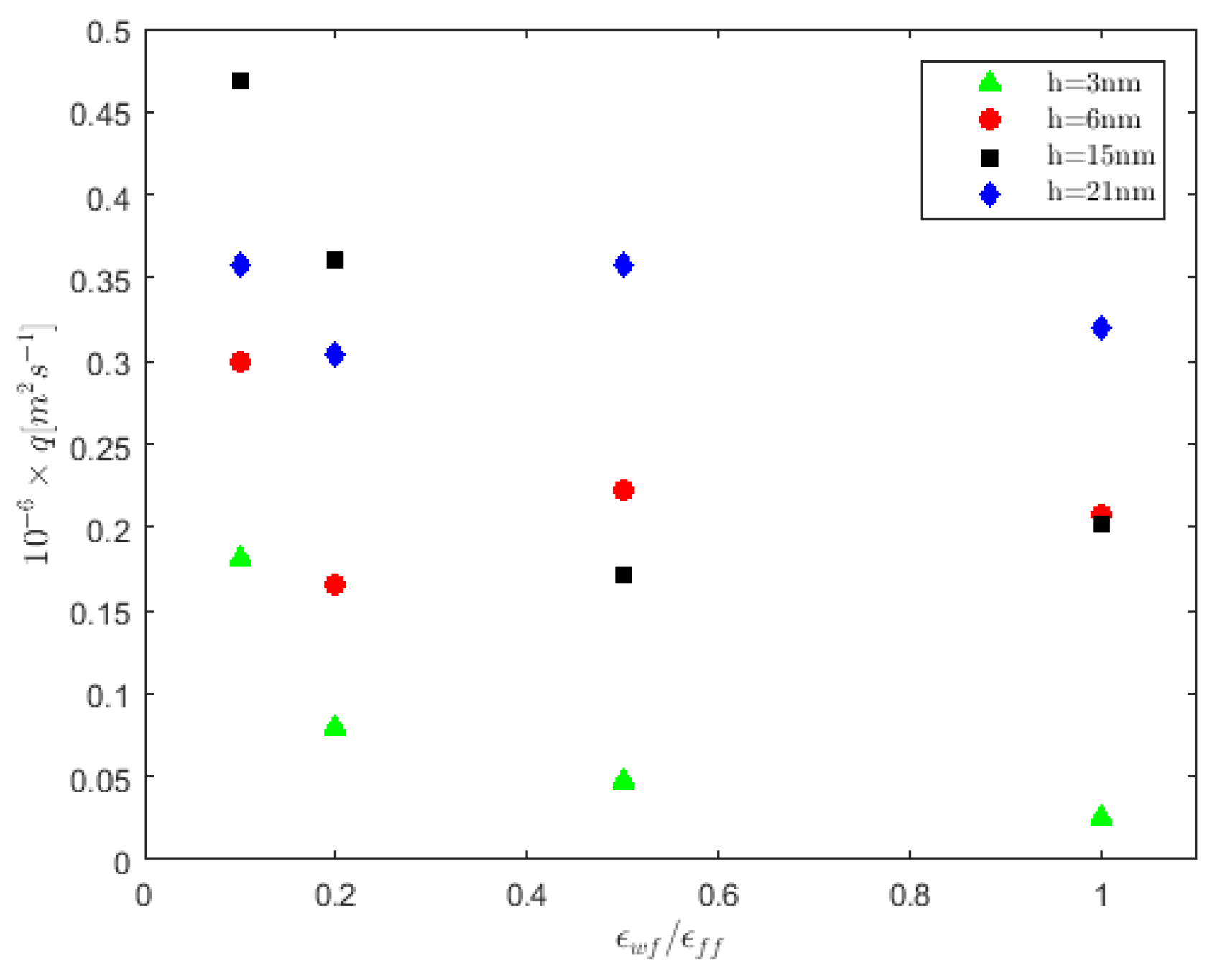
3.2. Volumetric Flow Rate
3.3. Configuration Issues
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, J.H.; Gao, L.; Hou, D.; Wang, P.; Zhou, Y.; Ding, Q.; Xiong, C. Insights on the ion migration throughout the nano-channel of ettringite under an external electric field: Structure, dynamics, and mechanisms. Constr. Build. Mater. 2020, 262, 120074. [Google Scholar] [CrossRef]
- Wen, Q.; Jia, P.; Cao, L.; Li, J.; Quan, D.; Wang, L.; Zhang, Y.; Lu, D.; Jiang, L.; Guo, W. Electric-Field-Induced Ionic Sieving at Planar Graphene Oxide Heterojunctions for Miniaturized Water Desalination. Adv. Mater. 2020, 32, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Xie, Q.; Alibakhshi, M.A.; Jiao, S.; Xu, Z.; Hempel, M.; Kong, J.; Park, H.G.; Duan, C. Fast water transport in graphene nanofluidic channels. Nat. Nanotechnol. 2018, 13, 238–245. [Google Scholar] [CrossRef] [PubMed]
- Faucher, S.; Aluru, N.; Bazant, M.Z.; Blankschtein, D.; Brozena, A.H.; Cumings, J.; Pedro De Souza, J.; Elimelech, M.; Epsztein, R.; Fourkas, J.T.; et al. Critical Knowledge Gaps in Mass Transport through Single-Digit Nanopores: A Review and Perspective. J. Phys. Chem. C 2019, 123, 21309–21326. [Google Scholar] [CrossRef]
- Zhong, J.; Alibakhshi, M.A.; Xie, Q.; Riordon, J.; Xu, Y.; Duan, C.; Sinton, D. Exploring Anomalous Fluid Behavior at the Nanoscale: Direct Visualization and Quantification via Nanofluidic Devices. Acc. Chem. Res. 2020, 53, 347–357. [Google Scholar] [CrossRef]
- Shahbabaei, M.; Kim, D. Advances in nanofluidics for water purification and filtration: Molecular dynamics (MD) perspective. Environ. Sci. Nano 2021, 8, 2120–2151. [Google Scholar] [CrossRef]
- Liu, P.Y.; Yan, T.T.; Shi, L.Y.; Park, H.S.; Chen, X.S.; Zhao, Z.G.; Zhang, D.S. Graphene-based materials for capacitive deionization. J. Mater. Chem. A. 2017, 5, 13907–13943. [Google Scholar] [CrossRef]
- Zunita, M. Graphene Oxide-Based Nanofiltration for Hg Removal from Wastewater: A Mini Review. Membranes 2021, 11, 269. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; He, Y.; Rosentsvit, L.; Suss, M.E.; Zhang, X.; Gao, T.; Liang, P. Flow-Electrode Capacitive Deionization: A Review and New Perspectives. Water Res. 2021, 200, 117222. [Google Scholar] [CrossRef]
- Bartzis, V.; Sarris, I.E. Time Evolution Study of the Electric Field Distribution and Charge Density Due to Ion Movement in Salty Water. Water 2021, 13, 2185. [Google Scholar] [CrossRef]
- Bartzis, V.; Sarris, I.E. A Theoretical Model for Salt Ion Drift Due to Electric Field Suitable to Seawater Desalination. Desalination 2020, 473, 114163. [Google Scholar] [CrossRef]
- Lodi, M.B.; Fanari, F.; Fanti, A.; Desogus, F.; Getaneh, W.; Mazzarella, G.; Valera, P. Preliminary Study and Numerical Investigation of an Electrostatic Unit for the Removal of Fluoride from Thermal Water of Ethiopian Rift Valley. IEEE J. Multiscale Multiphys. Comput. Tech. 2020, 5, 72–82. [Google Scholar] [CrossRef]
- Li, J.; Chen, D.; Ye, J.; Zhang, L.; Zhou, T.; Zhou, Y. Direct Numerical Simulation of Seawater Desalination Based on Ion Concentration Polarization. Micromachines 2019, 10, 562. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Patel, S.K.; Patel, S.K.; Ritt, C.L.; Deshmukh, A.; Wang, Z.; Qin, M.; Qin, M.; Epsztein, R.; Elimelech, M.; Elimelech, M. The relative insignificance of advanced materials in enhancing the energy efficiency of desalination technologies. Energy Environ. Sci. 2020, 13, 1694–1710. [Google Scholar] [CrossRef] [Green Version]
- Le, V.T.; Almomani, F.; Vasseghian, Y.; Vilas–Boas, J.A.; Dragoi, E.N. Graphene-based nanomaterial for desalination of water: A systematic review and meta-analysis. Food Chem. Toxicol. 2021, 148, 1–8. [Google Scholar] [CrossRef]
- Hardiagon, A.; Murail, S.; Huang, L.B.; Van Der Lee, A.; Sterpone, F.; Barboiu, M.; Baaden, M. Molecular dynamics simulations reveal statistics and microscopic mechanisms of water permeation in membrane-embedded artificial water channel nanoconstructs. J. Chem. Phys. 2021, 154, 184102. [Google Scholar] [CrossRef]
- Jofre, M.; Jofre, L.; Jofre-Roca, L. On the Wireless Microwave Sensing of Bacterial Membrane Potential in Microfluidic-Actuated Platforms. Sensors 2021, 21, 3420. [Google Scholar] [CrossRef]
- Murail, S.; Vasiliu, T.; Neamtu, A.; Barboiu, M.; Sterpone, F.; Baaden, M. Water permeation across artificial I-quartet membrane channels: From structure to disorder. Faraday Discuss. 2018, 209, 125–148. [Google Scholar] [CrossRef]
- Qiu, H.; Guo, W. Electromelting of confined monolayer ice. Phys. Rev. Lett. 2013, 110. [Google Scholar] [CrossRef]
- Sofos, F.; Karakasidis, T.E.; Spetsiotis, D. Molecular dynamics simulations of ion separation in nano-channel water flows using an electric field. Mol. Simul. 2019, 45, 1395–1402. [Google Scholar] [CrossRef]
- Lu, R.; Leaist, D.G. Mutual Diffusion in Solutions of Alkali Metal Halides. J. Chem. Soc. Faraday Trans. 1998, 94, 111–114. [Google Scholar] [CrossRef]
- Kilic, M.S.; Bazant, M.Z.; Ajdari, A. Steric Effects in the Dynamics of Electrolytes at Large Applied Voltages. I. Double-Layer Charging. Phys. Rev. E 2007, 75, 021502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kilic, M.S.; Bazant, M.Z.; Ajdari, A. Steric Effects in the Dynamics of Electrolytes at Large Applied Voltages. II. Modified Poisson-Nernst-Planck Equations. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2007, 75, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Ali Abdol, M.; Sadeghzadeh, S.; Jalaly, M.; Mahdi Khatibi, M. On the desalination performance of multi-layer graphene membranes; A molecular dynamics study. Comput. Mater. Sci. 2021, 191, 110335. [Google Scholar] [CrossRef]
- Golchoobi, A.; Tasharrofi, S.; Taghdisian, H. Functionalized nanoporous graphene membrane for water desalination; Effect of feed salinity on permeability and salt rejection, a molecular dynamics study. Comput. Mater. Sci. 2020, 172, 109399. [Google Scholar] [CrossRef]
- Hu, H.; Bao, L.; Priezjev, N.V.; Luo, K. Identifying two regimes of slip of simple fluids over smooth surfaces with weak and strong wall-fluid interaction energies. J. Chem. Phys. 2017, 146, 34701. [Google Scholar] [CrossRef] [PubMed]
- Sadeghpour, A.; Zeng, Z.; Ji, H.; Ebrahimi, N.D.; Bertozzi, A.L.; Ju, Y.S. Water vapor capturing using an array of traveling liquid beads for desalination and water treatment. Sci. Adv. 2019, 5, eaav7662. [Google Scholar] [CrossRef] [Green Version]
- Ruan, Y.; Nadim, A.; Duvvoori, L.; Chugunova, M. Liquid Films Falling Down a Vertical Fiber: Modeling, Simulations and Experiments. Fluids 2021, 6, 281. [Google Scholar] [CrossRef]
- Mohammad, A.W.; Teow, Y.H.; Ang, W.L.; Chung, Y.T.; Oatley-Radcliffe, D.L.; Hilal, N. Nanofiltration membranes review: Recent advances and future prospects. Desalination 2015, 356, 226–254. [Google Scholar] [CrossRef]
- Sofos, F.; Karakasidis, T.; Sarris, I.E. Molecular dynamics simulations of ion drift in nanochannel water flow. Nanomaterials 2020, 10, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids, 2nd ed.; Oxford University Press: Oxford, UK, 2017; ISBN 9780198803195. [Google Scholar]
- Jorgensen, W.L.; Chandrasekhar, J.; Madura, J.D.; Impey, R.W.; Klein, M.L. Comparison of simple potential functions for simulating liquid water. J. Chem. Phys. 1983, 79, 926–935. [Google Scholar] [CrossRef]
- Prasad, V.; Kannam, S.K.; Hartkamp, R.; Sathian, S.P. Water desalination using graphene nanopores: Influence of the water models used in simulations. Phys. Chem. Chem. Phys. 2018, 20, 16005–16011. [Google Scholar] [CrossRef] [Green Version]
- Giannakopoulos, A.E.; Sofos, F.; Karakasidis, T.E.; Liakopoulos, A. Unified Description of Size Effects of Transport Properties of Liquids Flowing in Nanochannels. Int. J. Heat Mass Transf. 2012, 55, 5087–5092. [Google Scholar] [CrossRef]
- Liakopoulos, A.; Sofos, F.; Karakasidis, T.E. Friction Factor in Nanochannel Flows. Microfluid. Nanofluidics 2016, 20, 1–7. [Google Scholar] [CrossRef]
- Sparreboom, W.; van Den Berg, A.; Eijkel, J.C.T. Transport in Nanofluidic Systems: A Review of Theory and Applications. New J. Phys. 2010, 12, 015004. [Google Scholar] [CrossRef]
- Ritos, K.; Mattia, D.; Calabrò, F.; Reese, J.M. Flow enhancement in nanotubes of different materials and lengths. J. Chem. Phys. 2014, 140, 014702. [Google Scholar] [CrossRef] [Green Version]
- Thomas, J.A.; Mcgaughey, A.J.H. Reassessing Fast Water Transport in CNT.pdf. Nano Lett. 2008, 8, 2788–2793. [Google Scholar] [CrossRef]
- Sofos, F.; Karakasidis, T.E.; Liakopoulos, A. How wall properties control diffusion in grooved nanochannels: A molecular dynamics study. Heat Mass Transf. und Stoffuebertragung 2013, 49, 1081–1088. [Google Scholar] [CrossRef]
- Banerjee, P.; Bagchi, B. Ions’ Motion in Water. J. Chem. Phys. 2019, 150, 190901. [Google Scholar] [CrossRef]
- Zong, D.; Hu, H.; Duan, Y.; Sun, Y. Viscosity of Water under Electric Field: Anisotropy Induced by Redistribution of Hydrogen Bonds. J. Phys. Chem. B 2016, 120, 4818–4827. [Google Scholar] [CrossRef] [PubMed]
- Kimura, H.; Sugiyama, T.; Takahashi, S.; Tsuchida, A. Viscosity change in aqueous hectorite suspension activated by DC electric field. Rheol. Acta 2013, 52, 139–144. [Google Scholar] [CrossRef]
- Sofos, F.; Karakasidis, T.E.; Liakopoulos, A. Fluid flow at the nanoscale: How fluid properties deviate from the bulk. Nanosci. Nanotechnol. Lett. 2013, 5, 457–460. [Google Scholar] [CrossRef]

| (lt/day) | ||||
|---|---|---|---|---|
| 395,257 | 2.53 × 10−6 | 1 | 7.33 × 10−4 | 1.54 × 10−7 |
| 293,255 | 3.41 × 10−6 | 1 | 9.89 × 10−4 | 2.79 × 10−7 |
| 227,273 | 4.40 × 10−6 | 1 | 1.28 × 10−3 | 4.66 × 10−7 |
| 180,733 | 5.53 × 10−6 | 1 | 1.61 × 10−3 | 7.37 × 10−7 |
| 80,000 | 1.25 × 10−5 | 1 | 3.63 × 10−3 | 3.76 × 10−6 |
| 8000 | 1.25 × 10−4 | 1 | 3.64 × 10−2 | 3.76 × 10−4 |
| 800 | 1.25 × 10−3 | 1 | 3.64 × 10−1 | 3.76 × 10−2 |
| 80 | 1.25 × 10−2 | 1 | 3.64 | 3.76 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sofos, F. A Water/Ion Separation Device: Theoretical and Numerical Investigation. Appl. Sci. 2021, 11, 8548. https://doi.org/10.3390/app11188548
Sofos F. A Water/Ion Separation Device: Theoretical and Numerical Investigation. Applied Sciences. 2021; 11(18):8548. https://doi.org/10.3390/app11188548
Chicago/Turabian StyleSofos, Filippos. 2021. "A Water/Ion Separation Device: Theoretical and Numerical Investigation" Applied Sciences 11, no. 18: 8548. https://doi.org/10.3390/app11188548
APA StyleSofos, F. (2021). A Water/Ion Separation Device: Theoretical and Numerical Investigation. Applied Sciences, 11(18), 8548. https://doi.org/10.3390/app11188548






