Probabilistic Analysis as a Method for Ground Freezing Depth Estimation
Abstract
:1. Introduction
2. Material and Methodology
2.1. Measurements of Soil Temperature
2.2. Methods of Probabilistic Analysis
2.3. Estimation of Gumbel Distribution Parameters
2.3.1. Least Square Estimation
2.3.2. Maximum Likelihood Method
2.3.3. Method of Moments
2.3.4. Lieblein Method—BLUE
3. Results
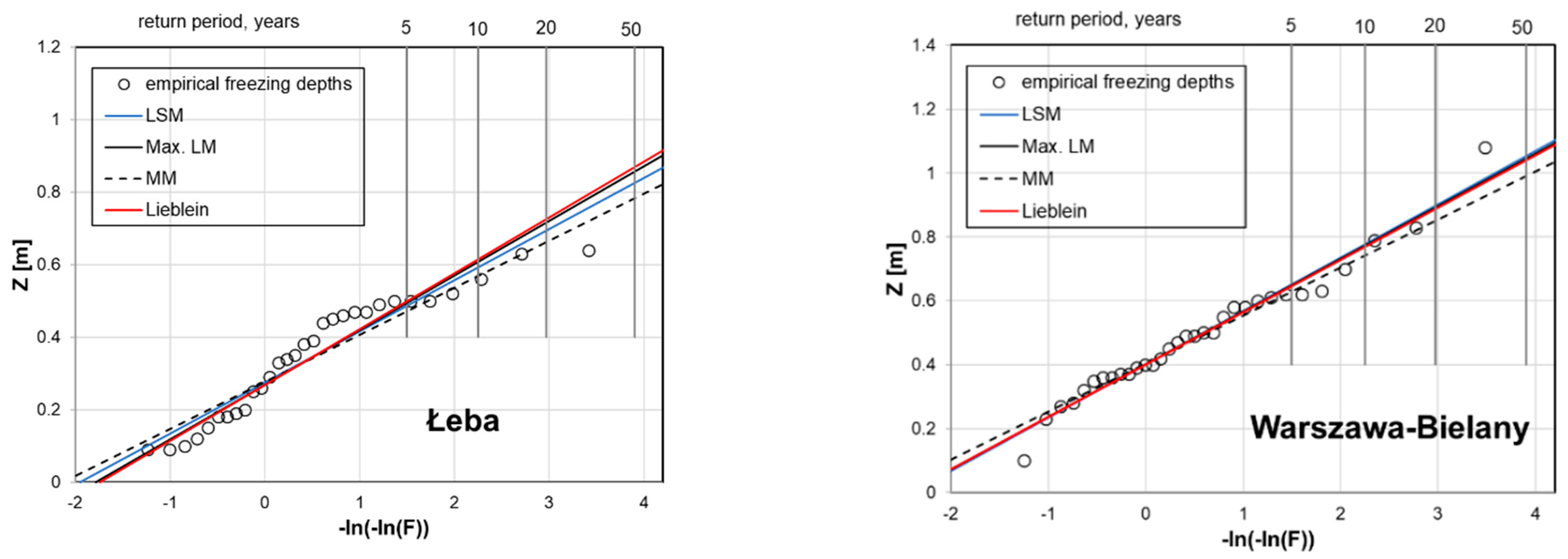
3.1. Estimation Models
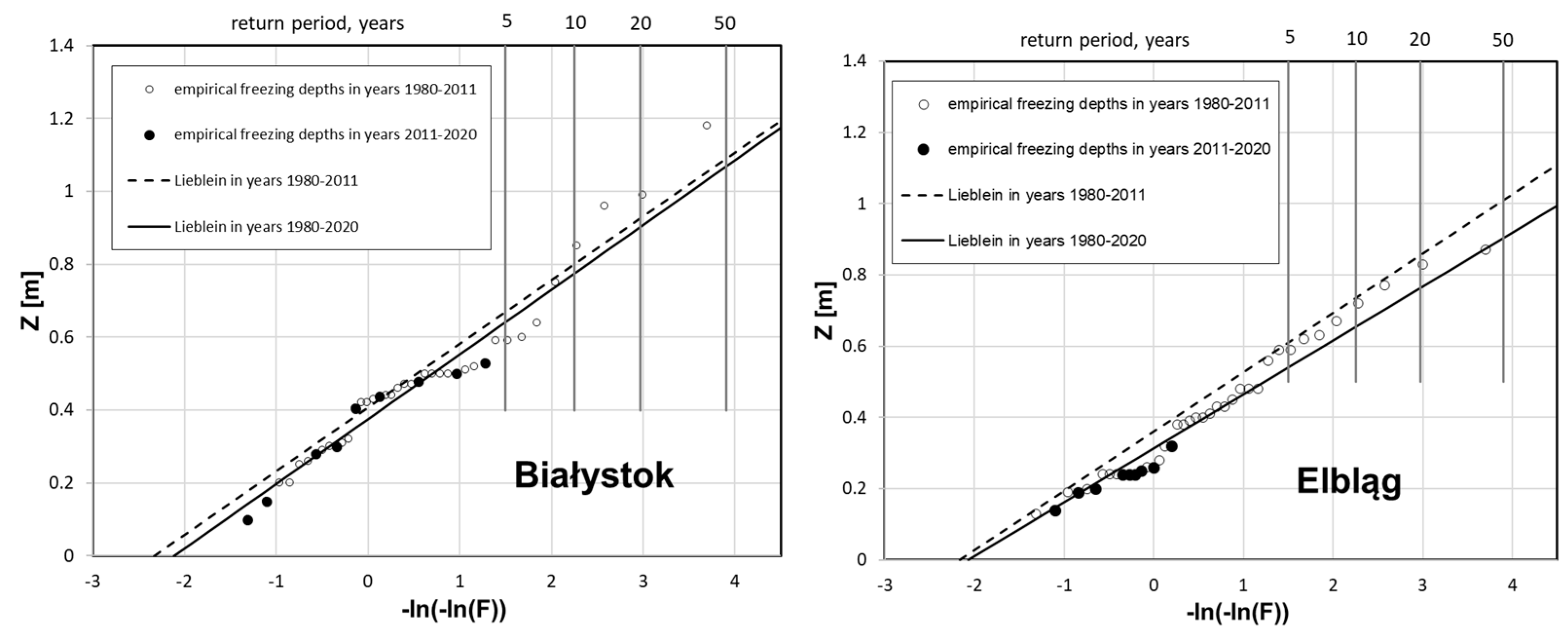
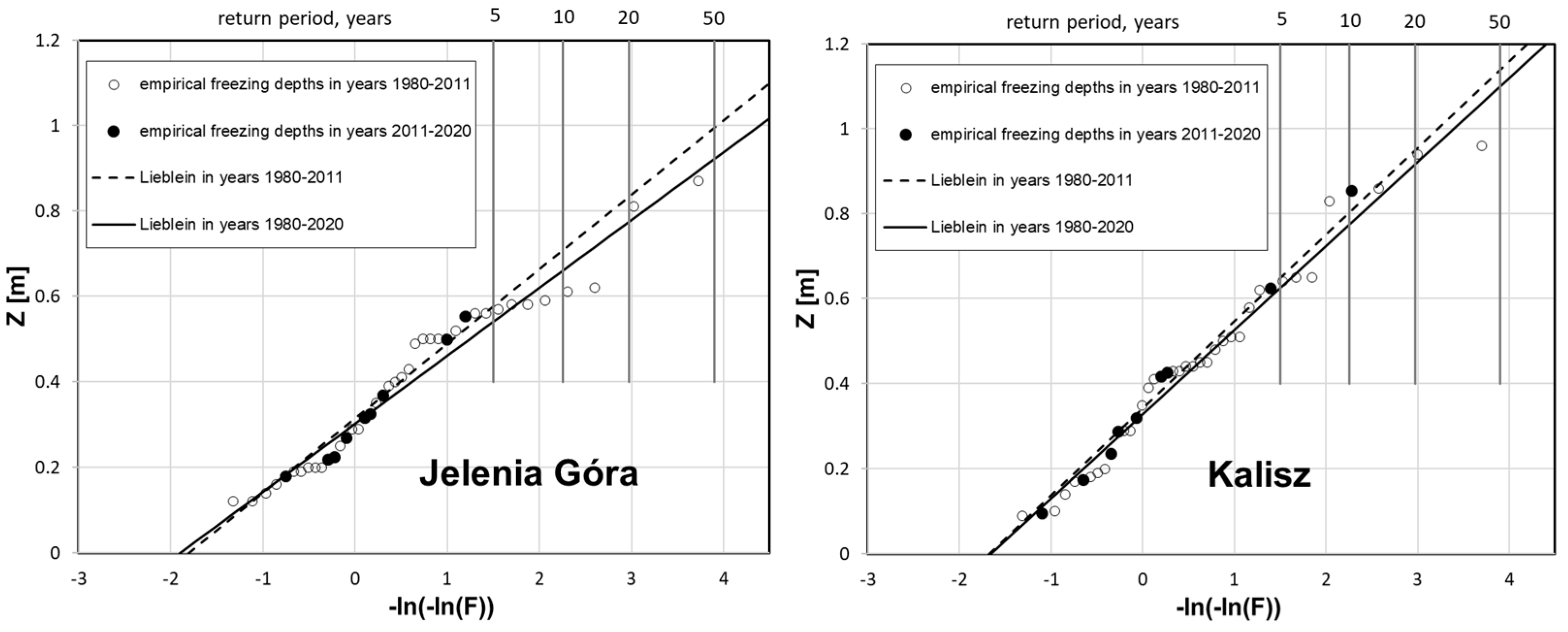
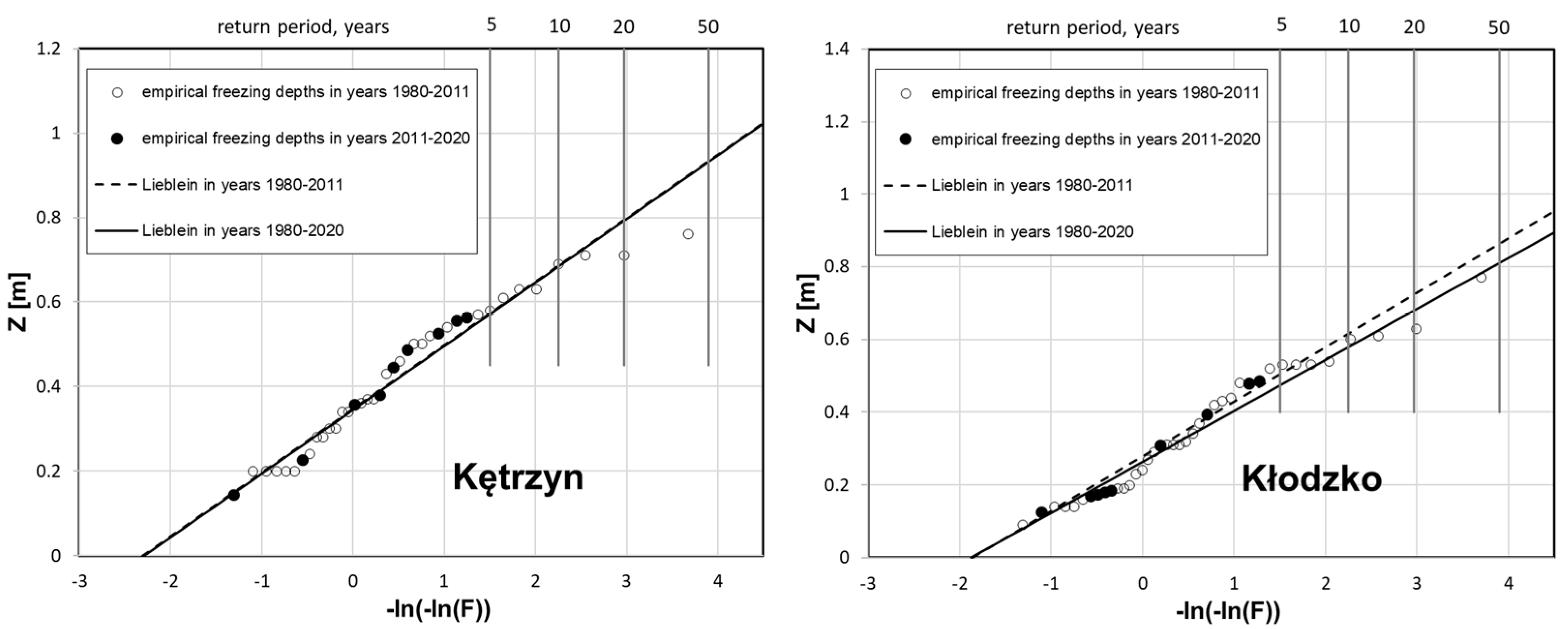
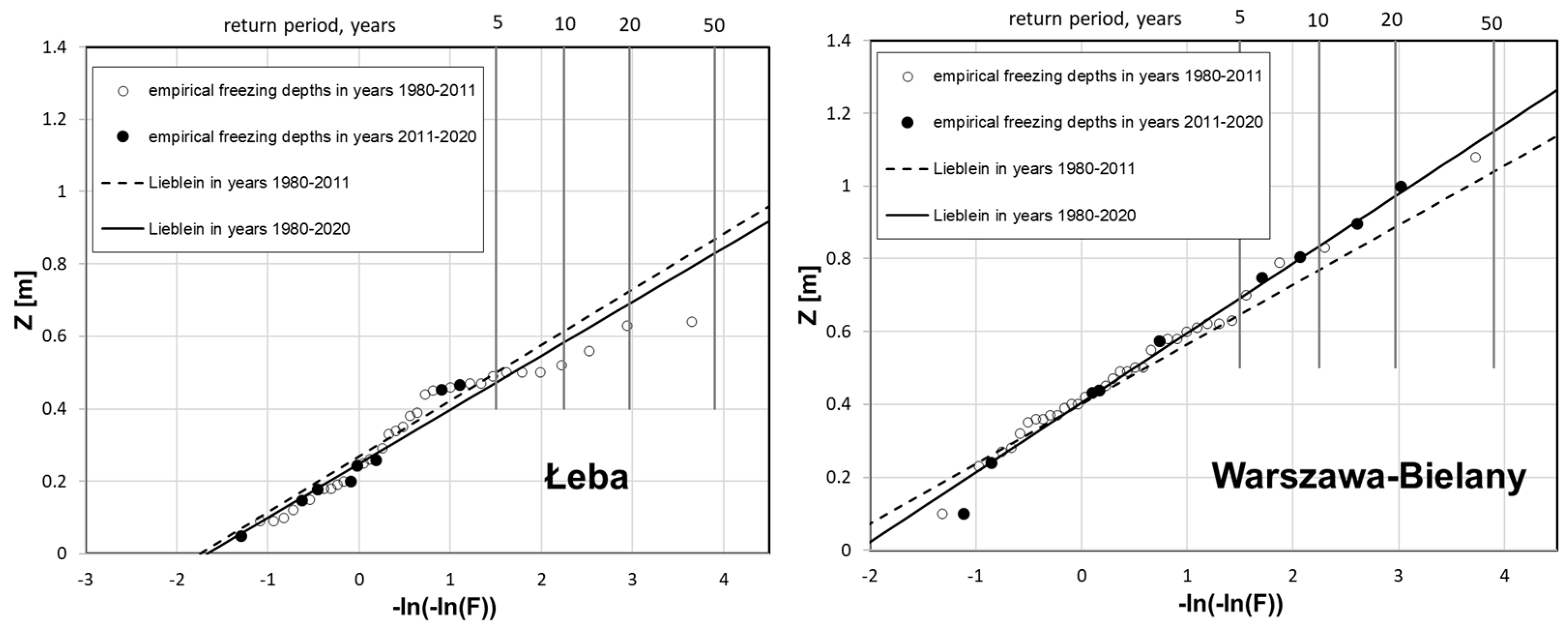
3.2. Validation of Chosen Model of Estimation (Lieblein)
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gontaszewska, A. Thermophisical Properties in Soils in the Freezing Aspects; Uniwersytet Zielonogórski: Zielona Góra, Poland, 2010. (In Polish) [Google Scholar]
- Konrad, J.-M.; Shen, M. 2-D frost action modeling using the segregation potential of soils. Cold Reg. Sci. Technol. 1996, 24, 263–278. [Google Scholar] [CrossRef]
- Rajaei, P.; Baladi, G.Y. Frost Depth: General Prediction Model, Transportation Research Record. J. Transp. Res. Board 2015, 2510, 74–80. [Google Scholar] [CrossRef]
- Venäläinen, A.; Tuomenvirta, H.; Heikinheimo, M.; Kellomäki, S.; Peltola, H.; Strandman, H.; Väisänen, H. Impact of climate change on soil frost under snow cover in a forested landscape. Clim. Res. 2001, 17, 63–72. [Google Scholar] [CrossRef] [Green Version]
- Arctic and Subarctic Construction Calculation Methods for Determination of Depths of freeze and Thaw in Soils; Technical Manual: TM5-852-6/AFM; Departments of the Army and the Air Force: Washington, DC, USA, 1966.
- Aldrich, H.P., Jr.; Paynter, H.M. Analytical Studies of Freezing and Thawing of Soils; First Interim Report; U.S. Army Corps of Engineeris, Airfields Branch, Engineering Division, Military Construction, ACFEL TR42: Boston, MA, USA, 1953. [Google Scholar]
- Kersten, M.S. Thermal properties of soils. In Proceedings of the 30th Annual Meeting of the Highway Research Board, Highway Research Board Special Report: Frost Action in Soils: A Symposium, Washington, DC, USA, 9–12 January 1951; Volume 2, pp. 161–166. [Google Scholar]
- Edwards, A.C.; Cresser, M.S. Freezing and its Effects on Chemical and Biological Properties of Soil. Adv. Soil Sci. 1992, 18, 59–79. [Google Scholar]
- Wiłun, Z.; Piasecki, A.; Kowalewski, Z. Freezing of soils (Przemarzanie gruntów). In Informator Instytutu Technicznego Wojsk Lotniczych; Seria A, Nr 5/1962: Warsaw, Poland, 1962. (In Polish) [Google Scholar]
- Zavarina, M.V. Methods of Computing Maximum Soil Freezing Depth; Translated from Voyeykov Main Geophysical Observatory (Trudy GGO). Sov. Hydrol. Sel. Papers 1969, 2, 131–139. [Google Scholar]
- Nixon, J.F. Discrete ice lens theory for frost heave in soils. Can. Geotech. J. 1991, 28, 843–859. [Google Scholar] [CrossRef]
- Tarnawski, V.R.; Wagner, B. On the prediction of hydraulic conductivity of frozen soils. Can. Geotech. J. 1996, 33, 176–180. [Google Scholar] [CrossRef]
- Vermette, S.; Christopher, S. Using the rate of accumulated freezing and thawing degree days as a surrogate for determining freezing depth in a temperate forest soil. Middle States Geogr. 2008, 41, 68–73. [Google Scholar]
- Goodrich, L.E. The Influence of Snow Cover on the Ground Thermal Regime. Can. Geotech. J. 1982, 19, 421–432. [Google Scholar] [CrossRef] [Green Version]
- Grodecki, W. Analysis of Certain Real-World Ground Freezing Factors. Ph.D. Thesis, Politechnika Warszawska, Warszawa, Poland, 1971. (In Polish). [Google Scholar]
- Gontaszewska, A. Comparison of calculated and observed depth of frost penetration in west Poland. In Faculty of Civil and Environmental Engineering, Institute of Structural Engineers; ASCE: New York, NY, USA, 2003. [Google Scholar]
- Steurer, P.M. Probability distributions used in 100-year return period of air-freezing index. J. Cold Reg. Eng. 1996, 10, 25–35. [Google Scholar] [CrossRef]
- Mageau, D.W.; Morgenstern, N.R. Observations on moisture migration in frozen soils. Can. Geotech. J. 1980, 17, 54–60. [Google Scholar] [CrossRef]
- Żurański, J.A.; Sobolewski, A. About measurements of the soil temperature and forecasting of the depth of soil freezing. Inżynieria Bud. 2013, 3, 141–145. [Google Scholar]
- Janiszewski, F. Instruction for Meteorological Stations; IMGW, Wydawnictwa Geologiczne: Warszawa, Poland, 1988. (In Polish) [Google Scholar]
- World Meteorological Organization. Guide to Meteorological Instruments and Methods of Observation, WMO-No. 8; WMO: Geneva, Switzerland, 2018. [Google Scholar]
- Gumbel, E.J. Statistics of Extremes; Columbia University Press: New York, NY, USA, 1958. [Google Scholar]
- Żurański, J.A.; Sobolewski, A. Snow Loads in Poland (Obciążenie Sniegiem w Polsce); Instytut Techniki Budowlanej, Monografie: Warszawa, Poland, 2009. [Google Scholar]
- Smith, M.G.; Rager, R.E. Protective Layer Design in Landfill Covers based on Frost Penetration. J. Geotech. Geoenviron. Eng. 2002, 128, 794–799. [Google Scholar] [CrossRef]
- Lieblein, J. Efficient Methods of Extreme-Value Methodology; Technical Report, Institute for Applied Technology, National Bureau of Standards: WaWashington, DC, USA, 1976. [Google Scholar]
- Żurawski, J.A.; Godlewski, T. Seasonal Ground Freezing in Poland; Instytut Techniki Budowlanej, Monografie: Warszawa, Poland, 2017. [Google Scholar]
- Jakubek, M. Statistica (in Polish). Politechnika Krakowska, Poland. 2017. Available online: https://www.l5.pk.edu.pl/~mj/lib/exe/fetch.php?media=pl:dydaktyka:matematyka2:skrypt_statystyka.pdf (accessed on 5 April 2017).
- Kozłowski, E. Mathematical Statistics. Lecture II: Point Estimation; Politechnika Lubelska: Lublin, Poland, 2020; Available online: http://www.kozlowski.pollub.pl/wyklady/SM/sm_wyklad2.pdf (accessed on 1 February 2020).
- Plucińska, A.; Pluciński, E. Introduction to the Probability and Mathematical Statistics Account; Wydawnictwa Politechniki Warszawskiej: Warsaw, Poland, 1974. (In Polish) [Google Scholar]
- Kordecki, W. Probability Account and Mathematical Statistics: Definitions, Theorems, Formulae; Oficyna Wydawnicza GiS: Wrocław, Poland, 2003. (In Polish) [Google Scholar]
- Pekasiewicz, D. Selected compliance tests for extreme statistics distributions and their use in financial analysis. Pr. Nauk. Uniw. Ekon. Wrocławiu 2014, 371, 268–276. [Google Scholar] [CrossRef]
- Xu, X.Z.; Wang, J.C.; Zhang, L.X. Physics of Frozen Soil; Science Press: Beijing, China, 2001. [Google Scholar]
- Yu, F.; Guo, P.; Yuanming, L.; Stolle, D. Frost heave and thaw consolidation modelling. Part 1: A water flux function for frost heaving. Can. Geotech. J. 2020, 57, 1581–1594. [Google Scholar] [CrossRef]
- Ickiewicz, I.; Pogorzelski, J.A. Influence of selected factors on the freezing depts in soils. Inżynieria Bud. 1987, 10–11, 338–342. [Google Scholar]

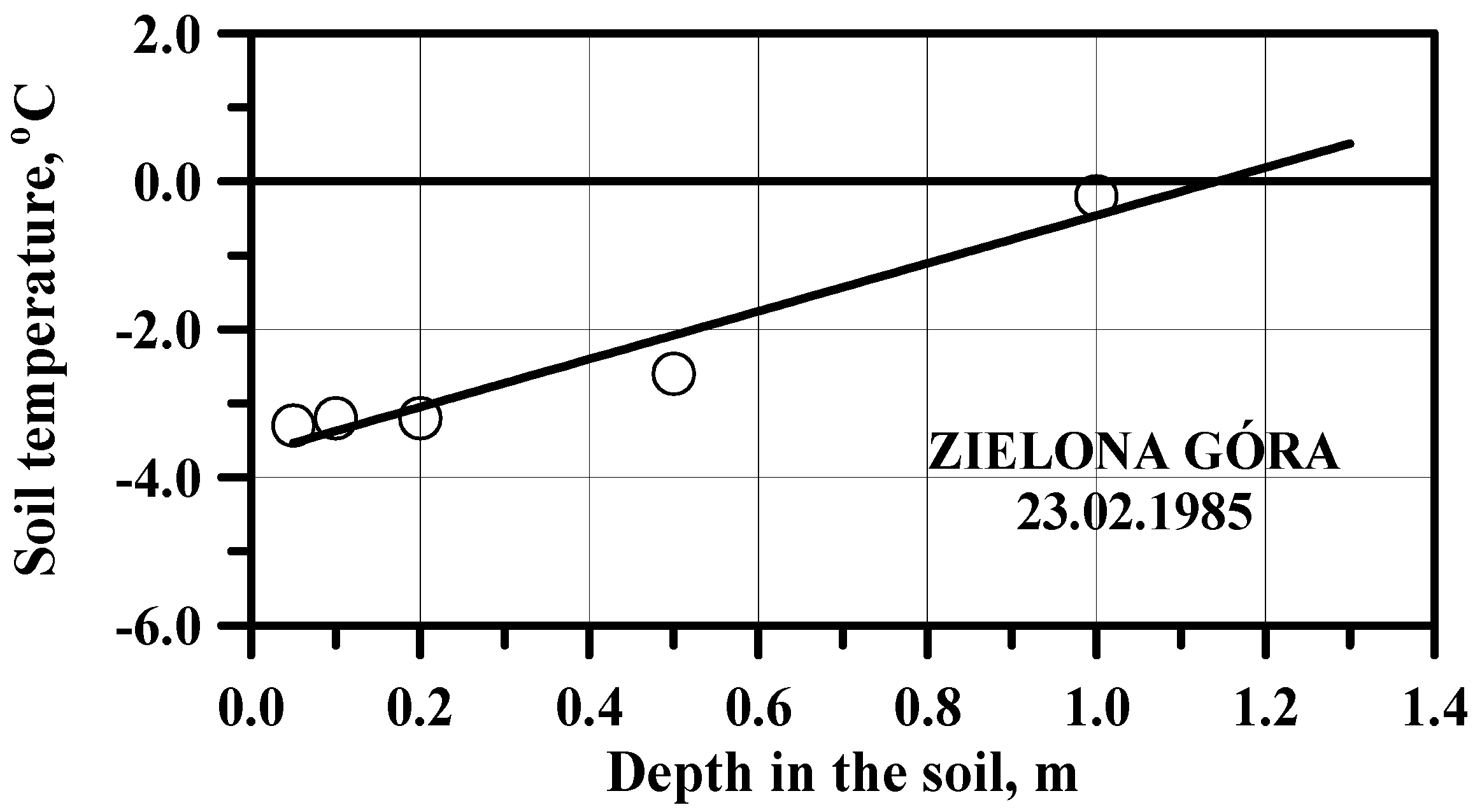
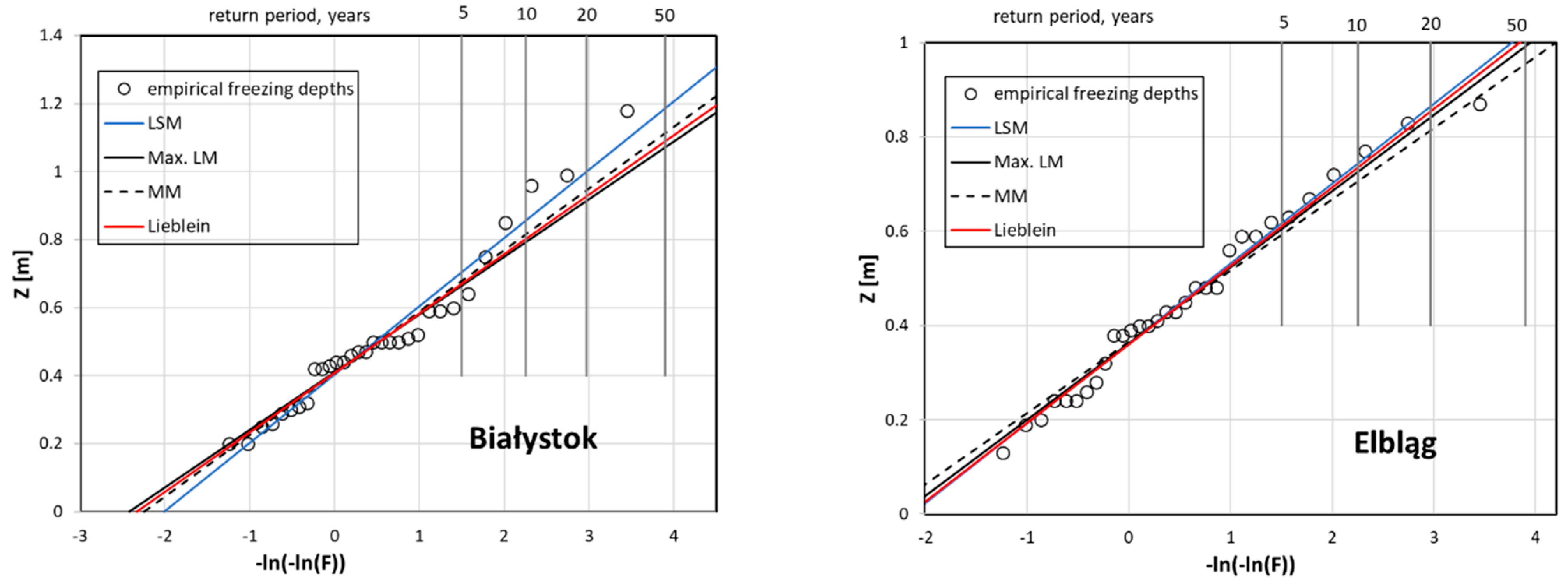
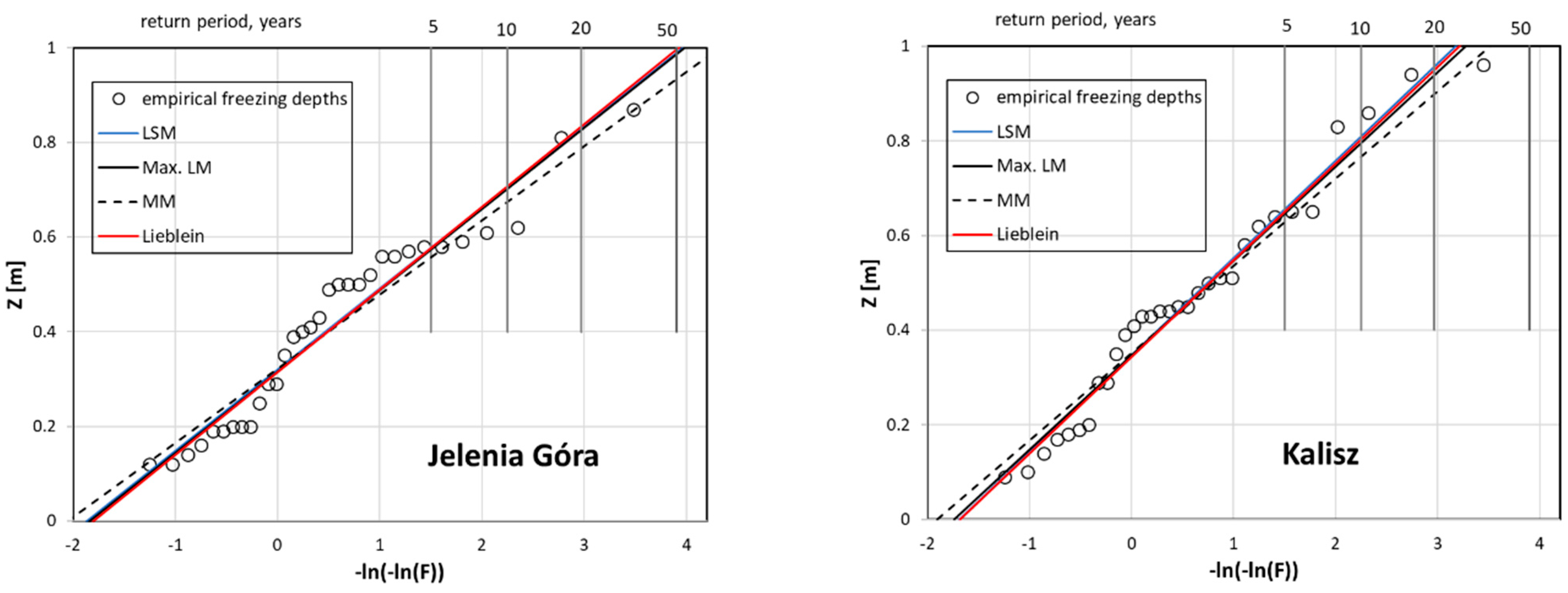
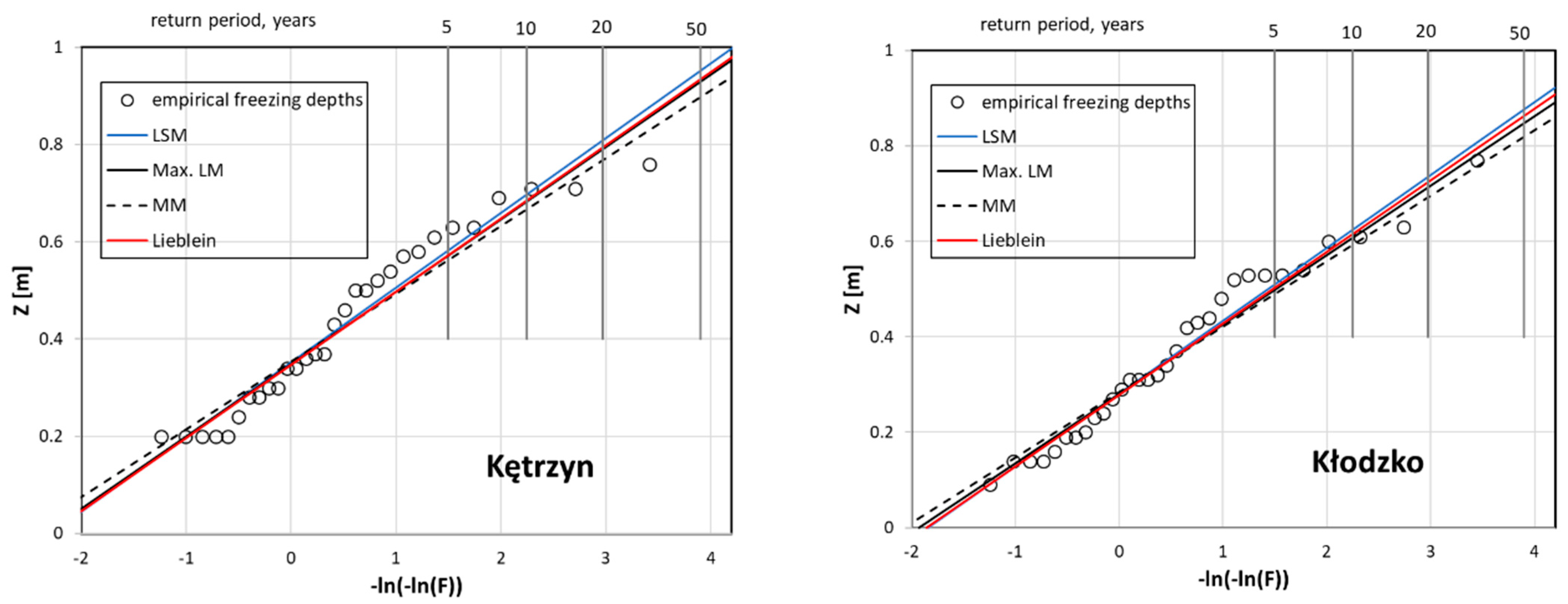
| No. | Localization | Gumbel Distribution Parameters for Chosen Estimation Models: | Correlation Coefficient R2 for Lieblein | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| LSM | Max. LM | MM | Lieblein | |||||||
| α | u | α | u | α | u | α | U | |||
| 1. | Białystok | 4.980 | 0.404 | 5.895 | 0.410 | 5.515 | 0.407 | 5.717 | 0.408 | 0.980 |
| 2. | Bielsko-Biała | 8.958 | 0.212 | 10.479 | 0.214 | 10.019 | 0.214 | 10.133 | 0.213 | 0.984 |
| 3. | Chojnice | 4.460 | 0.400 | 5.334 | 0.407 | 5.005 | 0.405 | 5.130 | 0.404 | 0.990 |
| 4. | Elbląg | 5.902 | 0.363 | 6.182 | 0.362 | 6.617 | 0.366 | 5.999 | 0.360 | 0.992 |
| 5. | Gorzów | 4.664 | 0.302 | 5.647 | 0.307 | 5.150 | 0.305 | 5.555 | 0.306 | 0.977 |
| 6. | Jelenia Góra | 5.831 | 0.320 | 5.826 | 0.316 | 6.385 | 0.322 | 5.733 | 0.315 | 0.971 |
| 7. | Kalisz | 4.864 | 0.347 | 5.014 | 0.346 | 5.419 | 0.351 | 4.894 | 0.343 | 0.985 |
| 8. | Katowice | 7.291 | 0.201 | 8.759 | 0.204 | 8.199 | 0.204 | 8.584 | 0.203 | 0.986 |
| 9. | Kętrzyn | 6.506 | 0.352 | 6.726 | 0.349 | 7.178 | 0.354 | 6.643 | 0.347 | 0.973 |
| 10. | Kielce | 4.645 | 0.399 | 5.351 | 0.404 | 5.157 | 0.402 | 5.191 | 0.402 | 0.979 |
| 11. | Kłodzko | 6.541 | 0.281 | 6.865 | 0.280 | 7.284 | 0.284 | 6.663 | 0.278 | 0.985 |
| 12. | Koło | 5.973 | 0.336 | 5.809 | 0.334 | 6.658 | 0.339 | 5.687 | 0.332 | 0.983 |
| 13. | Koszalin | 6.065 | 0.313 | 6.669 | 0.316 | 6.817 | 0.317 | 6.449 | 0.313 | 0.991 |
| 14. | Kraków-Balice | 6.670 | 0.261 | 6.721 | 0.258 | 7.328 | 0.263 | 6.565 | 0.256 | 0.972 |
| 15. | Łeba | 7.097 | 0.275 | 6.643 | 0.268 | 7.709 | 0.276 | 6.498 | 0.268 | 0.958 |
| 16. | Legnica | 4.592 | 0.327 | 4.952 | 0.328 | 5.136 | 0.332 | 4.790 | 0.325 | 0.987 |
| 17. | Lesko | 7.026 | 0.234 | 8.079 | 0.237 | 7.875 | 0.237 | 7.897 | 0.235 | 0.986 |
| 18. | Leszno | 5.208 | 0.350 | 5.459 | 0.349 | 5.826 | 0.354 | 5.294 | 0.347 | 0.992 |
| 19. | Lublin | 5.682 | 0.333 | 6.244 | 0.335 | 6.364 | 0.337 | 6.099 | 0.332 | 0.991 |
| 20. | Łódź | 4.699 | 0.386 | 4.905 | 0.386 | 5.284 | 0.391 | 4.736 | 0.382 | 0.992 |
| 21. | Mikołajki | 6.024 | 0.311 | 6.656 | 0.312 | 6.718 | 0.315 | 6.510 | 0.311 | 0.986 |
| 22. | Nowy Sącz | 8.851 | 0.202 | 8.788 | 0.199 | 9.776 | 0.203 | 8.526 | 0.198 | 0.977 |
| 23. | Opole | 5.006 | 0.324 | 5.689 | 0.327 | 5.607 | 0.328 | 5.475 | 0.325 | 0.988 |
| 24. | Resko | 5.888 | 0.245 | 6.623 | 0.247 | 6.539 | 0.248 | 6.537 | 0.246 | 0.982 |
| 25. | Rzeszów | 8.658 | 0.351 | 7.514 | 0.344 | 9.415 | 0.351 | 7.498 | 0.344 | 0.959 |
| 26. | Sandomierz | 5.560 | 0.434 | 6.095 | 0.436 | 6.141 | 0.436 | 6.014 | 0.434 | 0.974 |
| 27. | Słubice | 5.857 | 0.298 | 6.476 | 0.300 | 6.544 | 0.301 | 6.290 | 0.297 | 0.991 |
| 28. | Suwałki | 6.276 | 0.442 | 6.343 | 0.441 | 6.969 | 0.445 | 6.236 | 0.439 | 0.982 |
| 29. | Świnoujście | 5.232 | 0.433 | 5.322 | 0.432 | 5.860 | 0.437 | 5.205 | 0.429 | 0.988 |
| 30. | Szczecin | 4.253 | 0.282 | 4.616 | 0.279 | 4.622 | 0.284 | 4.633 | 0.280 | 0.961 |
| 31. | Tarnów | 6.189 | 0.322 | 6.494 | 0.321 | 6.884 | 0.325 | 6.323 | 0.319 | 0.984 |
| 32. | Toruń | 4.528 | 0.446 | 5.095 | 0.450 | 5.079 | 0.450 | 4.978 | 0.447 | 0.989 |
| 33. | Warszawa | 5.986 | 0.401 | 6.066 | 0.402 | 6.647 | 0.404 | 6.109 | 0.401 | 0.985 |
| 34. | Włodawa | 3.726 | 0.507 | 4.476 | 0.515 | 4.188 | 0.513 | 4.334 | 0.512 | 0.992 |
| 35. | Zakopane | 5.450 | 0.378 | 5.050 | 0.372 | 6.008 | 0.380 | 4.998 | 0.370 | 0.975 |
| 36. | Zielona Góra | 3.553 | 0.421 | 3.589 | 0.416 | 3.915 | 0.425 | 3.516 | 0.413 | 0.972 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Godlewski, T.; Wodzyński, Ł.; Wszędyrówny-Nast, M. Probabilistic Analysis as a Method for Ground Freezing Depth Estimation. Appl. Sci. 2021, 11, 8194. https://doi.org/10.3390/app11178194
Godlewski T, Wodzyński Ł, Wszędyrówny-Nast M. Probabilistic Analysis as a Method for Ground Freezing Depth Estimation. Applied Sciences. 2021; 11(17):8194. https://doi.org/10.3390/app11178194
Chicago/Turabian StyleGodlewski, Tomasz, Łukasz Wodzyński, and Małgorzata Wszędyrówny-Nast. 2021. "Probabilistic Analysis as a Method for Ground Freezing Depth Estimation" Applied Sciences 11, no. 17: 8194. https://doi.org/10.3390/app11178194
APA StyleGodlewski, T., Wodzyński, Ł., & Wszędyrówny-Nast, M. (2021). Probabilistic Analysis as a Method for Ground Freezing Depth Estimation. Applied Sciences, 11(17), 8194. https://doi.org/10.3390/app11178194






