Abstract
European Union legislation requires organizations to assess their processes in the context of risk management. The main task of risk management is to manage all risks that can significantly affect the outcome of processes. The article is focused on risk evaluation in pressure leaching at elevated temperature using the method Fault Tree Analysis (FTA). The effectivity of pyrite and arsenic pyrite decomposition by oxidative pressure leaching is influenced by the duration of the process, by the temperature, concentration of the leaching solution and by a density of the slurry. It was found that, under equitable conditions, the arsenic pyrite decomposes more intensely than pyrite. Under laboratory conditions, leaching is performed in an autoclave. Due to the aggressive environment, increased pressure and temperature, process failure is possible. Its probability was calculated by FTA. It has been found that the probability of the top event in the examined process was disproportionately high (0.057) and represents an invitation to take corrective actions. The Monte Carlo method was used for the simulation of the effect the probability of basic events on the probability of the top event—the failure of the leaching process.
1. Introduction
Currently, it is very important to ensure the continuous improvement of all processes in the industry. It is this improvement of processes in organizations that should ensure the constant competitiveness of organizations in the market. The process of oxidative leaching in autoclaves is a complicated process that can be influenced by several factors. Even one factor can cause an error in the system, or process failure, where it is then necessary to subsequently look for the causes of the failure.
The FTA method is used to evaluate the causes that lead to system failure. The FTA is a quantitative evaluation technique that identifies an event as the top event and systematically arranges all the causes of the error in a top-down structure that looks like a tree in order to calculate the probability of occurrence of the top event []. According to Luo W. et al. FTA computes a large class of system reliability properties and measures based on the fault tree that model’s failure propagation in a system []. Risk assessment methods are often used in industrial processes to avoid risks and reduce losses [,].
The aim of the work is to study the application of the FTA method in the process of assessing and minimizing the risk of total failure of the leaching process, which can lead to damage to health or even endanger the lives of operating personnel in addition to material losses. By identifying the riskiest branch, attention will be focused on eliminating significant sources of dangers and threats. The results obtained have great potential for use in future safety research as well as in the hydrometallurgical leaching process. They prove that the application of the FTA method is suitable for improving not only the analyzed leaching process, but also seems to be suitable for other hydrometallurgical technologies that work with aggressive substances, often at high temperature and pressure.
2. Characteristics of the Leaching Process in the Context of Risk Assessment
The technology of oxidation leaching in autoclaves is a complicated process. Depending of leaching conditions several processes may run simultaneously in the closed autoclave, e.g., sorption of the gaseous oxygen in the solution, mass transport from the fluid volume to solid particles, diffusion of reagents through the boundary surface layers on the surface of solid particles, diffusion of the products of chemical reactions in the solution. Oxidation of sulfides runs by means of dissolved oxygen in a liquid phase whereby sorption of oxygen by leaching solution plays an important role [,,].
Decomposition of the real flotation sulfide concentrate is a goal of the process.
Processes of dissolution, oxidation, diffusion, precipitation, and hydrolysis can be described by the following reactions:
The flotation sulfide concentrate from Pezinok (Slovak Republic) was used for the experimental work. Chemical composition of the concentrate is listed in Table 1. As regards the mineralogical composition, it contained pyrite, arsenic pyrite, tailings, silica, and shale.

Table 1.
Chemical composition of the concentrate (wt. %).
Rotating autoclave LAMPART of 4 l volume was used for leaching; oxygen was introduced through a pressure reducer. The concentrate was mixed with a relevant volume of the solution of NaOH a slurry of different density with the ratio L:S (liquid/solid) between 5:1 and 20:1 was formed. The concentration of the leaching solution NaOH varied from 3.55 to 7.1 M and applied temperatures ranged between 120 and 185 °C. After the temperature reached the required values, the reaction time 90–300 min was measured. All experiments were carried out at a constant initial pressure of oxygen 0.9 MPa which was sufficient for the course of oxidizing reactions in the system. Mikloš and Fröhlich proposed that in the equipment used for pressure leaching the highest efficiencies of the parallel decomposition of both sulfides was achieved at the following conditions: concentration of the leaching solution–3.55 M NaOH, the temperature of 185 °C, the initial pressure of O2–0.9 MPa, time–180 min [].
Due to the aggressive environment, increased pressure and temperature, the failure of the leaching process is possible. Its probability was calculated by Fault Tree Analysis (FTA).
The term “failure” (fault = top event in FTA terminology) means the premature termination of leaching, the result of which is unusable and implies material and financial loss or damage to health.
The goals of the work are:
- To calculate the probability of the top event-the failure (early termination) of the leaching process using the FTA.
- To model the impact of the dynamic of the probability of base events on the top event using the Monte Carlo method.
- Propose an effective improvement of the leaching process in order to effectively reduce the probability of the top event.
3. Fault Tree Analysis (FTA) Analysis
The gradual development of civilization brings together, along with many technical facilities, the possibility of significant damage to the natural environment and one of the greatest possible threats to it is chemical failures and disasters []. Occupational risk assessment is one of the employer’s core duties but there are no practical implementing provisions for the use of hazard identification and risk assessment methods [,,,].
As mentioned above, leaching in the autoclave is accompanied by the risk of material damage and in the worst case an injury to the health of the operator. This fact is influenced by risk factors such as the aggressive environment due to an alkaline slurry reaction, high temperature, and pressure, electrical installation, the risk of injury from handling by heavy autoclave. They together create an indirect risk of a failure, for example, if the operator is injured, the autoclave will be out of service with great probability. These sources of risk, in view of their indirect action, are not considered in the construction of FTA [,].
Many authors, for example Girmanová et al., propose to construct a cause-and-effect diagram, also called Ishikawa’s diagram by its creator, before constructing a fault tree. It allows for “clarification” of the problem and is often serviceable as a basis for the graphic design of a “tree”. The aim is to identify the sources that contribute to the analysis problem and the relationships between them [,,].
The diagram is a visualization tool for categorizing the potential causes of the problem—the top event to identify its basic or root causes. The design of the diagram looks much like a skeleton of a fish. Fishbone diagrams are typically worked right to left, with each large “bone”, or “ribs”, of the fish branching out to include smaller bones containing more detail. The head and backbone of the fish list the top event—the problem or issue to be studied. Five “causes” that contribute to the problem (Materials, Machines, Manpower, Methods, Measurement, and Environment) create the first bones (ribs) of the fish []. Other, more specific levels of bones (horizontal, vertical, horizontal...) can be added to the “ribs” until the cause of the problem can be divided into subcategories. The practical number of levels is usually four to five. The lowest level is a basic event. When the diagram is complete, we provide an overview of the possible causes (not symptoms) of the solved problem. It is important to explore more times repeating subcategories to find a link between them or eliminate unnecessary duplication. Correctly designed diagram significantly facilitates the design of fault tree analysis.
As can be seen in Figure 1, the ribs have been modified according to actual problems of the leaching process (Material-tested concentrate, Machine—autoclave, Methods, Environment, Measurement methods, and Appraisers). The whole diagram was not used to construct the FTA; it did not address the problem of the appraiser, which is rather a matter of human resources. The influence of the environment was also not considered. It does not have a statistically significant influence on the probability of the process failure in according to the results of the observation (the autoclave is in a heated room, and it is isolated from plants that would negative (for example dustiness) affect its operation. Problems with negligible probability of occurrence have also not been considered.
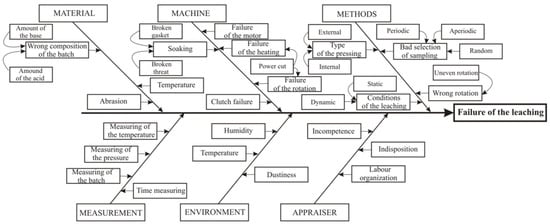
Figure 1.
Ishikawa diagram.
Failure Tree Analysis (FTA) is a qualitative and quantitative analysis of the failure in the shape of a tree []. It defines the relationship between a top event—a failure—and basic events (roots), which are the faults of the lowest elements of the system or external influences. Logical links, that is, branch of the tree of failures, are made up of gates using the logical symbols (AND) and (OR). The gate has one output event and several input events. The “AND” gate uses a logical sum. It represents a combination of independent events. That is, the probability of any input event to an AND gate is unaffected by any other input event to the same gate. It is “favorable”—an adverse event at the output P(G) occurs only if all events at the input (Ai) occur at the same time. The probability of an output event at this type of gate is calculated using Equation (3).
The “OR” gate is “unfavorable”—an adverse event at the output P(G) occurs when either of event occurs at an input (Ai). The probability of an event at the output of this type of gate is calculated using Equation (4). From the knowledge of the probability of occurrence of a base event or the intensity of its occurrence, it is possible to estimate the probability or intensity of the occurrence of a top event. The “tree” creation process and subsequent calculation are documented in STN EN 61025: 2007 [].
The fault tree is in a shape that can be understood, analyzed and modified if necessary to simplify the identification of the failure. From the highest level of the failure—the top event—it is possible to proceed at tree level to a base event. In this way, it is possible to investigate any dependencies in the system as well as its subsystems [,]. In analyzing this method, a systematic approach is necessary, as it is necessary to capture the functional linkages between the elements of the monitored system. The FTA method going from the top down through the tree makes it easy to recognize the causal dependence of the top event. FTA is particularly suited for the analysis of complex systems that consist of functionally dependent subsystems serving to fulfil a defined function. It is particularly suited to cases where the process of analysis requires many different specialists. FTA method was first used in 1962 by Bell Telephone Laboratories in the development of intercontinental ballistic missiles (Minuteman Intercontinental Ballistic Missile Launch Control System) and was improved by Boeing in 1966. It started to be used in the nuclear power industry since 1975. Its use in this industry experienced a significant increase after an accident in Three Mile Island in 1979. It is an important part of reliability engineering.
It is most used in nuclear technology, in complex systems such as aircraft, chemical operations, and complex information systems []. It allows easy understanding of the system and its functional links, as well as its impact factors. If these trees are extensive, it is advisable to use the computing technique, since the process of verifying the tree’s faults in the usual way would be difficult. Evolved from the works of Rajkumar et al. and Plura the aim of qualitative or logical analysis is to find all reasonably possible combinations of factors operating conditions, environmental conditions, human errors and faults of elements of which could lead to the top event [,]. A manual solution in a qualitative analysis of the fault tree is currently done only in the case of simple trees (usually a few tens of basic events), otherwise special software products must be used. If the parameters of confidence (probability of developing) of basic events are known, it is possible to make a quantitative fault tree analysis. The goal of this procedure is:
- The systematic identification of all possible combinations of (basic) causes leading to the top event;
- Easy definition of the top event probability in the system or its arbitrary part;
- Creating a model (causal dependence) for examining the security of the monitored system to know the input/output interactions;
- Modelling for simulation of safety, reliability, etc.;
- Provide a transparent analytical listing of logical operations existing in the tracking system;
- Display of the monitored system in the form of a graphical model in which quantitative and qualitative data are recorded;
- Recognition of individual risk factors in the risk analysis process.
The FTA proceeds in steps. Clemens and Vesely et al. present the main principles of creation of FTA in their works. The specific sequence of steps is not unified; the sequence below is the minimum that each analysis must contain [,,,]:
- Definition of the analyzed system, purpose and extent of the analysis, and basic assumptions that have been adopted. Prerequisites should include the conditions under which the monitored system is operated (normal operation, maintenance, special operations...). The amount of information required is subject to the purpose of the analysis. Knowledge of one person is often inadequate, and professional groups need to be set up to obtain sufficient information. In this step, it is useful to use statistical methods and quality tools (e.g., process map, Failure Mode and Effect Analysis FMEA, flowchart, regulatory diagrams, trend analysis, Pareto and Ishikawa diagram).
- Definition of a top event-specifying an undesirable event means defining the onset or existence of dangerous conditions, the inability of the system to perform the required functions. The top event must be defined clearly and unambiguously.
- Construction of the failure tree—the tree is a graphical representation consisting of individual elements that are bound by logical operations describing the observed process. It is necessary to distinguish between conditional and unconditional states in the process of the construction.
- Evaluation of tree failure can be quantitative (numeric) or qualitative (logical) [,,,,].
In the case of quantitative FTA, the direct calculation method, the minimal critical cut method and the simulation method (e.g., Monte Carlo) are most used. The probability of each basic event listed in Table 2. The probability was evaluated using data, collected over 9 years. During this period 1011 leaching experiments were performed. Some data, e.g., probability of event A was evaluated based on even longer observations. Electrical current provides the heating and movement (rotation) of the autoclave. From the point of view of the failures associated with the current, basic events considered were included in the gate G1. The fault tree with the individual basic events and gates is shown in Figure 2. Factor A-total (electric) power fault (the probability of the event was based on the long-term observation (factor J in Table 1) [].

Table 2.
The probability of individual basic events.
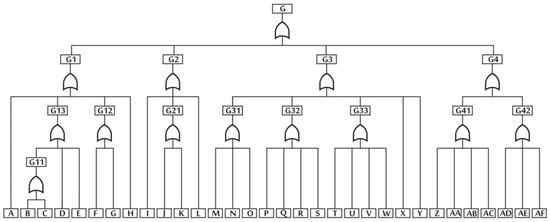
Figure 2.
The fault tree.
The heating of the autoclave vessel (the inner, removable part of the autoclave in which it is the slurry) is solved by Kanthal spirals. Their malfunctioning may result in factor B—mechanical damage (stroke, improper handling) or in factor C—electro-technical damage C (bad contact, most often after deformation of Kanthal spirals due to heat). The base events B and C are connected in the gate G11. The voltage of the electric current supplied to the spirals is manually controlled by a circular rheostat. The probability of its failure, caused by the most frequent the over-firing (blowing) of the resistance wire, is factor D. As with other electric heaters, there is a certain inertia in the heating spirals. The result is “slow” regulation, i.e., the impossibility of accurately setting the temperature when using rheostat control. Based on our experience, appraiser set the rheostat to a voltage that corresponds to the desired temperature. However, the temperature increase does not have to stop at the intended level. The increasing of the temperature can continue to value that degrade experimental intentions. This increase depends on several factors (ambient temperature, spiral state, their resistance depending on the working temperature), more closely the factor H in Table 1 []. The probability of “inertia” problems expresses factor E. The overall probability of a heating failure is expressed by the gate G13, which includes the probabilities of the gate G11 and factors D and E. The rotary movement of the autoclave is provided by an asynchronous electric motor coupled to an autoclave by a mechanical clutch with belt transmissions. The basic events associated with the electric drive are connected to the gate G12, namely the probability of the electric failure of the motor F and the probability of overload G (the necessary replacement of the fuses). The pressure in the autoclave can be controlled (if there is no external pressure) by the temperature. Due to the above-mentioned “inertia” (as a factor E), it is sometimes difficult to achieve a stable temperature and pressure match in autoclave in real time. The probability of this disagreement is a factor H. Gate G2 quantifies the probability of the failure of rotation motion transmission between the motor and the autoclave. The most frequent failures (factor I) are unfastened component or increased friction (due to dust, corrosive environment) may occur in the mechanical clutch. In case of a clutch with belt drive, the failure can result in belt slipping (factor J) or in belt breakage (factor K). The common probability of a belt failure is determined by the gate G21. If the cover of heater spirals is insufficiently fixed on the autoclave, it may be loosened with the probability of L (often associated with damage to the spiral). Gas soaking, and in the worst case soaking of liquid content (generally aggressive) of the autoclave vessel results in the termination of the test. This factor is solved in the G3 gate. The places of the soaking can be in the throat of the vessel between “body” and screwed cover–the “head” (gate G31), in the input of the gas (gate G32) and in the place of the connection of the manometer (gate G33). The last two are placed on the cover. The gate G31 also includes the probability of the soaking due to a poor or inappropriate seal (factor M) or the absence of the seal (forgotten or poorly inserted, factor N). The cause of the overflow may also be a weakly tightened thread connection of the container with its cover with probability O. The same basic events can also occur at the input of (generally inert) gases. Gases allow to create a protective or desired atmosphere in the autoclave and, to a certain extent, to regulate the internal pressure. The gas input closes the valve, screwed onto the cover of the vessel. Factor P represents the probability of selecting an improper sealing of the screwed connection, factor Q probability of the absence of the sealing and factor R inadequate tightening of the threaded (screwed) connection between the valve and the cover. Factor S is the probability of imperfect valve closure. The manometer threaded (screwed) to the cover of the vessel of the autoclave serves for the measurement of its internal pressure. Factor T represents the probability of selecting an improper sealing of the screwed connection, factor U probability of the absence of the sealing and factor V inadequate tightening of the threaded (screwed) connection between the manometer and the cover. The probability of using a manometer with a too small range is the factor W is also covered by gate G33. If the pressure in the vessel of the autoclave exceeds its upper limit, the destruction of the manometer with the following consequences may be possible. Temperature measurement in the autoclave allows the dead-ended tube firmly coupled to the cover reaching the autoclave vessel. The analysis of the failures for temperature measurement is given in gate G4. The leached slurry is placed in a vessel that is inserted into the autoclave. Its purpose is to avoid the contact of the aggressive slurry with the body of the autoclave. The probability of the leakage of the slurry out of the working vessel due to an inappropriate setting of the autoclave is the factor X and the effect of overloading the vessel is the factor Y. The G4 gate combines the probability of failure of the autoclave measuring devices. Uncontrollable and potentially a serious accident can occur due to the extreme pressure and temperature increase in the vessel (intense overflow or “explosion”) if the measuring devices fail. The temperature of the slurry, its pressure and the reaction time are measured during autoclave operation. The temperature is measured by a thermocouple whose thermoelectric voltage measures a suitable electronic thermometer. The probability of failure of a thermometer device indicates the gate G41. The gate G41 joints the probability of failure of the electronic thermometer (factor Z), the probability of breakage (mechanical damage) of the used thermocouple (factor AA), damage to the ceramic cover of the thermocouple (factor AB), and the short-circuit (unwanted contact between thermocouple wires out of the isolation) of the thermocouple (or connecting wires) AC. Gate G42 indicates the probability of failure of the manometer due to the jamming of the mechanical parts with the probability of AD or damage due to the aggressive leaching media AE. The most recent underlying cause is an incorrect indication of the start time of the experiment and its end (or its absence) with the probability of AF.
All the gates considered are “OR”. The probability of gate G1 is 0.02622, G2 gate: 0.00748, G3 gate: 0.01460 and Gate G4: 0.00936. The riskiest G1 gate appears to characterize the device for rotation of the autoclave vessel. The probability of the top event is 0.05653, i.e., 5.7%.
Thus, the probability of failure of the leaching process—the top event—is relatively high, so roughly every 17th leaching is terminated prematurely. This results in time, material and consequently economic losses, possibly (e.g., leakage of the slurry with high temperature and usually with a basic reaction) can also lead to damage to the health of the appraiser. Therefore, it is advisable to reduce the probability of a top event. The “tree of failures” makes it easy for us to find a “critical cut”, which is to take the direction of eliminating the causes of the failure. In our case, they are branches that connect in the G1 gate.
4. Monte Carlo Method
The Monte Carlo method has a wide use of simulation of experiments, through counting of some integrals, to solving differential equations. The basic idea of the method is very simple: we want to determine the mean value of a quantity that is the result of random action []. A computerized model is created and after enough overrun simulations, data can be processed by standard statistical methods, such as determining the mean, median and the standard deviation. The method is named after Monte Carlo, known for its casinos and especially roulette. The term was first used by physicists in 1940 to build an American atomic bomb.
Besides the mathematical problems, the Monte Carlo method is used to solve economic problems, particularly in insurance and finance. The method helps to solve deterministic and probabilistic tasks by many times repeated random experiments on the input data sample. The method was dealt with by many authors, the works of Knežo, Raychaudhuri, Kuselman et al., Petrík and Blaško, and Sienkowski were used in the paper [,,,].
The impact of a certain dynamics of the probability of occurrence of basic events was simulated by Monte Carlo. We assumed that they varied within ± 10% of the value in Table 2 according to the triangular distribution. The calculation was performed using QUANTUM XL software with 10,000 simulations. In this case, the probability of an unsuccessful (the failure-early termination) leaching process would increase to 15.7%.
The most hazardous branches of the "tree" are branches who relate to gates G1 and G3. The relationship between the probability of failure in these branches and the probability of top event is shown in Figure 3. The theoretical possibility of lowering the probability of a top event could be a change in a type of gates from “OR” to less risky “AND”. It is practically difficult to achieve it without intervention in the technology or autoclave construction.
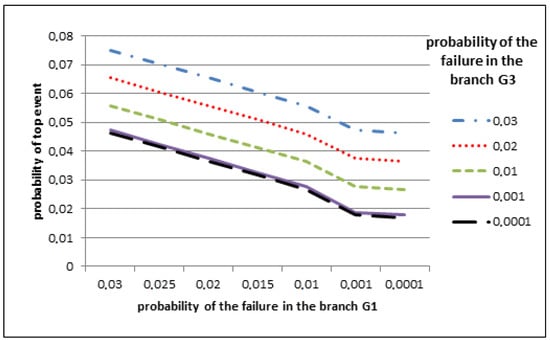
Figure 3.
The relationship between the probability of failure in branches G1 and G3 and the probability of the top event.
Outputs from FTA but ETA (event tree analysis) analysis are important inputs to the risk assessment process by other methods, such as L-matrix analysis, hazard and operability analysis (HAZOP), fault tree analysis (FTA), bow-tie analysis and fault mode effect analysis [,], or Failure Mode and Effects Analysis (FMEA). The legislation of the Slovak Republic, based on the directives of the European Union, Council Directive 89/391/EEC on the introduction of measures to encourage improvements in the safety and health of workers at work and Council Directive 89/392/EEC machinery directive, shows organizations to manage their risks. The FTA used in solving the problem presented in the article is also suitable for this purpose.
5. Conclusions
The probability of the top event-early termination of the leaching process in autoclave calculated by FTA is 5.7%. The most hazardous is the branch connected with gate G1, which includes electrical and mechanical components providing rotation of the autoclave. If the variability of the probability of basic events increases by 10%, the probability of the top event increase to 15.7% according to the Monte Carlo method. Decreasing of the top event probability is practically difficult to achieve it without intervention in the technology or autoclave construction.
The results obtained have great potential for use in future safety research as well as in the hydrometallurgical leaching process. They prove that the application of the FTA method is suitable for improving not only the analyzed leaching process, but also seems to be suitable for other hydrometallurgical technologies that work with aggressive substances, often at high temperature and pressure.
The presented example of the use of FTA in the production process in which there is a possibility of damage to the health of employees as well as material and financial losses contributes to meeting the requirements of European Union legislation, which shows organizations to manage risks.
Author Contributions
Management and validation, writing and final review, S.M. and J.K.; application of statistical, mathematical techniques, J.P. and P.B.; research and verification, M.Š.; development of methodology, S.M. and J.P.; data collection, P.B., M.B. (Michaela Balážiková), M.B. (Martin Bezákand). All authors have read and agreed to the published version of the manuscript.
Funding
This contribution is the result of the implementation of the following projects: KEGA No. 019TUKE-4/2020 “Application-oriented education in ISO 9001:2015 requirements implementation”.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Acknowledgments
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Tavakoli, M.; Nafar, M. Modification of the FFTA method for calculating and analyzing the human reliability of maintenance groups in power transmission grids. Int. J. Syst. Assur. Eng. Manag. 2021, 1–14. [Google Scholar] [CrossRef]
- Luo, W.; Wei, O.; Wan, H. SATMCS: An Efficient SAT-Based Algorithm and Its Improvements for Computing Minimal Cut Sets. IEEE Trans. Reliab. 2021, 70, 575–589. [Google Scholar] [CrossRef]
- Zhu, H.-L.; Liu, S.-S.; Qu, Y.-Y.; Han, X.-X.; He, W.; Cao, Y. A new risk assessment method based on belief rule base and fault tree analysis. Proc. Inst. Mech. Eng. Part O J. Risk Reliab. 2021, 235, 1748006X2110114. [Google Scholar] [CrossRef]
- Hudák, R.; Šarik, M.; Dadej, R.; Živčák, J.; Harachová, D. Material and Thermal analysis of Laser Sinterted Products. Acta Mech. Autom. 2013, 7, 15–19. [Google Scholar] [CrossRef]
- Rusanen, L.; Aromaa, J.; Forsen, O. Pressure Oxidation of Pyrite-Arsenopyrite Refractory Gold Concentrate. Physicochem. Probl. Miner. Process. 2013, 49. [Google Scholar] [CrossRef]
- Correia, M.N.; Carvalho, J.; Monhemius, A. Study of the autoclave leaching of a tetrahedrite concentrate. Miner. Eng. 1993, 6, 1117–1125. [Google Scholar] [CrossRef]
- Koslides, T.; Ciminelli, V. Pressure oxidation of arsenopyrite and pyrite in alkaline solutions. Hydrometallurgy 1992, 30, 87–106. [Google Scholar] [CrossRef]
- Mikloš, V.; Fröhlich, L. Decomposition of Pyrite and Arsenopyrite by Pressure Oxidation of Tetrahedrite Raw Materials. Metalurgija 2002, 41, 29–32. [Google Scholar]
- Żarczyński, A. Environmental Hazard by Major Accidents in the Polish Chemical Industry. Zagrożenie środowiska Na Terenie Polski Ze Strony Poważnych Awarii w Zakładach Przemysłu Chemicznego. Chem. Rev. 2015, 1, 45–51. [Google Scholar] [CrossRef]
- Dobrzyńska, E. Ocena ryzyka związanego z występowaniem substancji chemicznych na stanowiskach pracy. Metody bezpomiarowe w bazie CHEMPYŁ. Przemysł Chem. 2018, 1, 155–160. [Google Scholar] [CrossRef]
- Shafiee, M.; Enjema, E.; Kolios, A. An Integrated FTA-FMEA Model for Risk Analysis of Engineering Systems: A Case Study of Subsea Blowout Preventers. Appl. Sci. 2019, 9, 1192. [Google Scholar] [CrossRef]
- Dado, M.; Hnilica, R.; Kotus, M.; Kotek, L. Use of virtual reality in machinery safety education. In Proceedings of the ICERI Proceedings, 10th International Conference of Education, Research and Innovation (ICERI2017), Seville, Spain, 16–18 November 2017; Proceedings Paper; WOS: 000429975302127. IATED-INT Association Technology Education & Development: Valencia, Spain, 2017. ISBN 978-84-697-6957-7. [Google Scholar]
- Novakova, R.; Paulikova, A.; Cekanova, K. More Wood, Better Management, Increasing Effectiveness: Starting Points and Perspective. In Proceedings of the Scientific Papers, Prague, Czech Republic, 24–26 May 2017; Dudik, R., Ed.; Česká Zemědělská Univerzita v Praze, Lesnická Fakulta: Prague, Czech Republic; WoodEMA Instituce: Zagreb, Croatia, 2017; ISBN 978-80-213-2761-0. [Google Scholar]
- Gabriska, D. Evaluation of the Level of Reliability in Hazardous Technological Processes. Appl. Sci. 2020, 11, 134. [Google Scholar] [CrossRef]
- Takahashi, M.; Anang, Y.; Watanabe, Y. A Safety Analysis Method for Control Software in Coordination with FMEA and FTA. Information. 2021, 12, 79. [Google Scholar] [CrossRef]
- Girmanová, L.; Mikloš, V.; Palfy, P.; Petrík, J.; Sütőová, A.; Šolc, M. Nástroje a Metódy Manažérstva Kvality; ELFA, s.r.o.: Košice, Slovakia, 2009; ISBN 978-80-553-0144-0. (In Slovak) [Google Scholar]
- Tureková, I.; Tomková, V.; Bagalová, T. Work at Height in Safety Work Plan Management. Adv. Mater. Res. 2014, 919, 523–526. [Google Scholar] [CrossRef]
- Halfarova, P.; Ustyugova, T.; Vykydal, D. The application of Fuzzy FMEA. In Proceedings of the 22nd International Conference on Metallurgy and Materials (METAL), Brno, Czech Republic, 15–17 May 2013; Technical University of Ostrava VSB: Ostrava, Czech Republic, 2013; pp. 1909–1913. [Google Scholar]
- Markulik, S.; Nagyova, A.; Turisova, R.; Villinsky, T. Improving Quality in the Process of Hot Rolling of Steel Sheets. Appl. Sci. 2021, 11, 5451. [Google Scholar] [CrossRef]
- Bujna, M.; Dostál, P. Assessment of Selected Equipment by Method FTA. Acta Univ. Agric. Silvic. Mendel. Brun. 2017, 65, 1655–1661. [Google Scholar] [CrossRef]
- IEC 61025. Fault Tree Analysis (FTA); International Electrotechnical Commission IEC: Geneva, Switzerland, 2006. [Google Scholar]
- Husar, J.; Knapcikova, L.; Balog, M. Implementation of Material Flow Simulation as a Learning Tool. In Advances in Design, Simulation and Manufacturing; Lecture Notes in Mechanical, Engineering; Ivanov, V., Rong, Y., Trojanowska, J., Venus, J., Liaposhchenko, O., Zajac, J., Pavlenko, I., Edl, M., Perakovic, D., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 33–41. ISBN 978-3-319-93586-7. [Google Scholar]
- Toth, T.; Hudak, R.; Zivcak, J. Dimensional verification and quality control of implants by additive manufacturing. Qual. Innov. Prosper. 2015, 19. [Google Scholar] [CrossRef][Green Version]
- Monkova, K.; Hric, S.; Knapčikova, L.; Vagaska, A.; Matiskova, D. Application of simulation for product quality enhancement. In Proceedings of the International Conference on Informatics, Management Engineering and Industrial Application (IMEIA), Phuket, Thailand, 24–25 April 2016; DEStech Transactions on Engineering and Technology Research: Lancaster, PA, USA, 2016; pp. 216–220. [Google Scholar]
- Patil, R.B.; Waghmode, L.Y.; Chikali, P.B.; Mulla, T.S. An Overview of Fault Tree Analysis (FTA) Method for Reliability Analysis & Life Cycle Cost (LCC) Management. In Proceedings of the Second International Conference on Emerging Trends in Engineering (SICETE), Nagpur, India, 16–18 December 2009; p. 6. [Google Scholar]
- Plura, J. Plánování a Neustálé Zlepšování Jakosti; Computer Press: Praha, Czech Republic, 2001; ISBN 978-80-7226-543-5. (In Czech) [Google Scholar]
- Clemens, P.L. Fault Tree Analysis; Technology Sverdrup: Pittsburg, CA, USA, 1993; 96p, Available online: http://rischioatmosfereesplosive.studiomarigo.it/profiles/marigo2/images/file/1736612536.pdf (accessed on 22 July 2021).
- Vesely, W.; Stamatelatos, M.; Dugan, J.; Fragola, J.; Minarick, J.; Railsback, J. FTA Handbook with Aerospace Applications; NASA Langley Research Center: Washington, DC, USA, 2002. Available online: http://www.hq.nasa.gov/office/codeq/doctree/fthb.pdf (accessed on 22 July 2021).
- Živčák, J.; Šarik, M.; Hudák, R. FEA simulation of thermal processes during the direct metal laser sintering of Ti64 titanium powder. Measurement 2016, 94, 893–901. [Google Scholar] [CrossRef]
- Pačaiová, H.; Andrejiová, M.; Balažiková, M.; Tomašková, M.; Gazda, T.; Chomová, K.; Hijj, J.; Salaj, L. Methodology for Complex Efficiency Evaluation of Machinery Safety Measures in a Production Organization. Appl. Sci. 2021, 11, 453. [Google Scholar] [CrossRef]
- Blaško, P.; Petrík, J. FTA analysis of the failure in the horizontal fluidity test. Met. Mater. Eng. 2015, 21, 229–240. [Google Scholar] [CrossRef]
- Liu, J.; Yang, H.; Wei, D.; Song, X. Time Distribution Simulation of Household Power Load Based on Travel Chains and Monte Carlo–A Study of Beijing in Summer. Sustainability 2021, 13, 6651. [Google Scholar] [CrossRef]
- Knežo, D. O Metóde Monte Carlo a Možnostiach jej Aplikácií. Transf. Inovácií 2012, 24, 178–181. Available online: https://www.sjf.tuke.sk/transferinovacii/pages/archiv/transfer/24-2012/pdf/178-181.pdf (accessed on 22 July 2021). (In Slovak).
- Raychaudhuri, S. Introduction to Monte Carlo simulation. In Proceedings of the 2008 Winter Simulation Conference, Miami, FL, USA, 7–10 December 2008; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2008; pp. 91–100. [Google Scholar]
- Kuselman, I.; Pennecchi, F.; Epstein, M.; Fajgelj, A.; Ellison, S. Monte Carlo simulation of expert judgments on human errors in chemical analysis—A case study of ICP–MS. Talanta 2014, 130, 462–469. [Google Scholar] [CrossRef]
- Sienkowski, S. Estimation of Random Variable Distribution Parameters by the Monte Carlo Method. Metrol. Meas. Syst. 2013, 20, 249–262. [Google Scholar] [CrossRef]
- Sabol, R.; Klein, P.; Ryba, T.; Hvizdos, L.; Varga, R.; Rovnak, M.; Sulla, I.; Mudronova, D.; Galik, J.; Polacek, I.; et al. Novel Applications of Bistable Magnetic Microwires. Acta Physica Polonica A 2017, 131, 1150–1152. [Google Scholar] [CrossRef]
- Özfirat, M.K.; Özkan, E.; Kahraman, B.; Şengün, B.; Yetkin, M.E. Integration of risk matrix and event tree analysis: A natural stone plant case. Sadhana 2017, 42, 1741–1749. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).