Travelling Waves Approach in a Parabolic Coupled System for Modelling the Behaviour of Substances in a Fuel Tank
Abstract
:1. Introduction
2. Materials and Methods
3. Discussions and Results
3.1. Analysis in the Travelling Waves (TW) Domain
3.2. TW Profiles
- The methodology is based on the Matlab function bvp4c which provides an implicit Runge–Kutta approach with an interpolant extension [22]. The collocation method requires the specification of boundary conditions, in this case, given by the stationary solutions at and ;
- The integration interval is , large enough to study the TW evolution in their domain, and not impacted by the boundary conditions that act as tractors;
- The considered error for computation is ;
- The integration domain has been divided into 100,000 nodes;
- To make the numerical assessment tractable and without loss of generality, particular values were taken for the involved parameters: , —and different values of n and m.
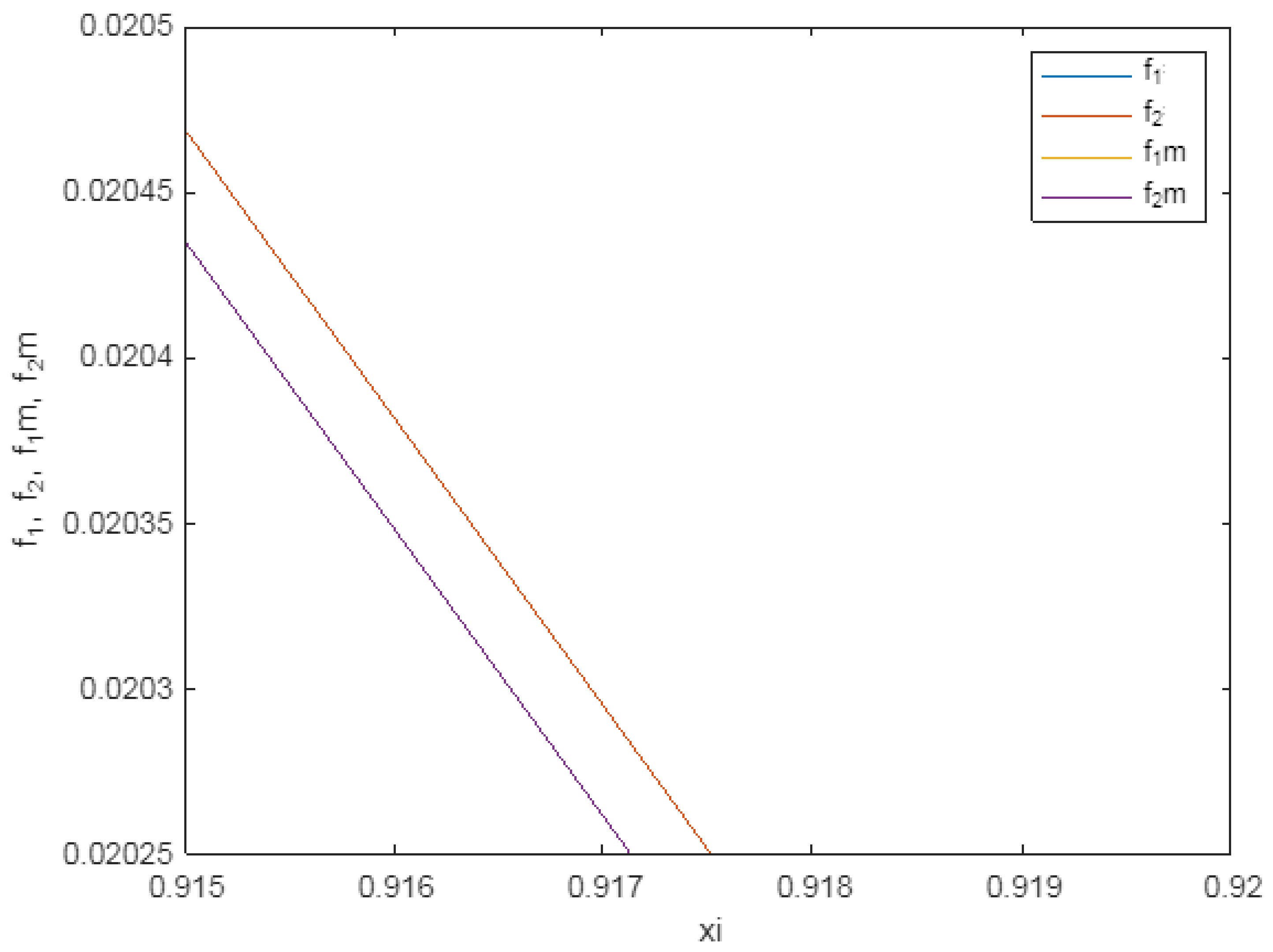
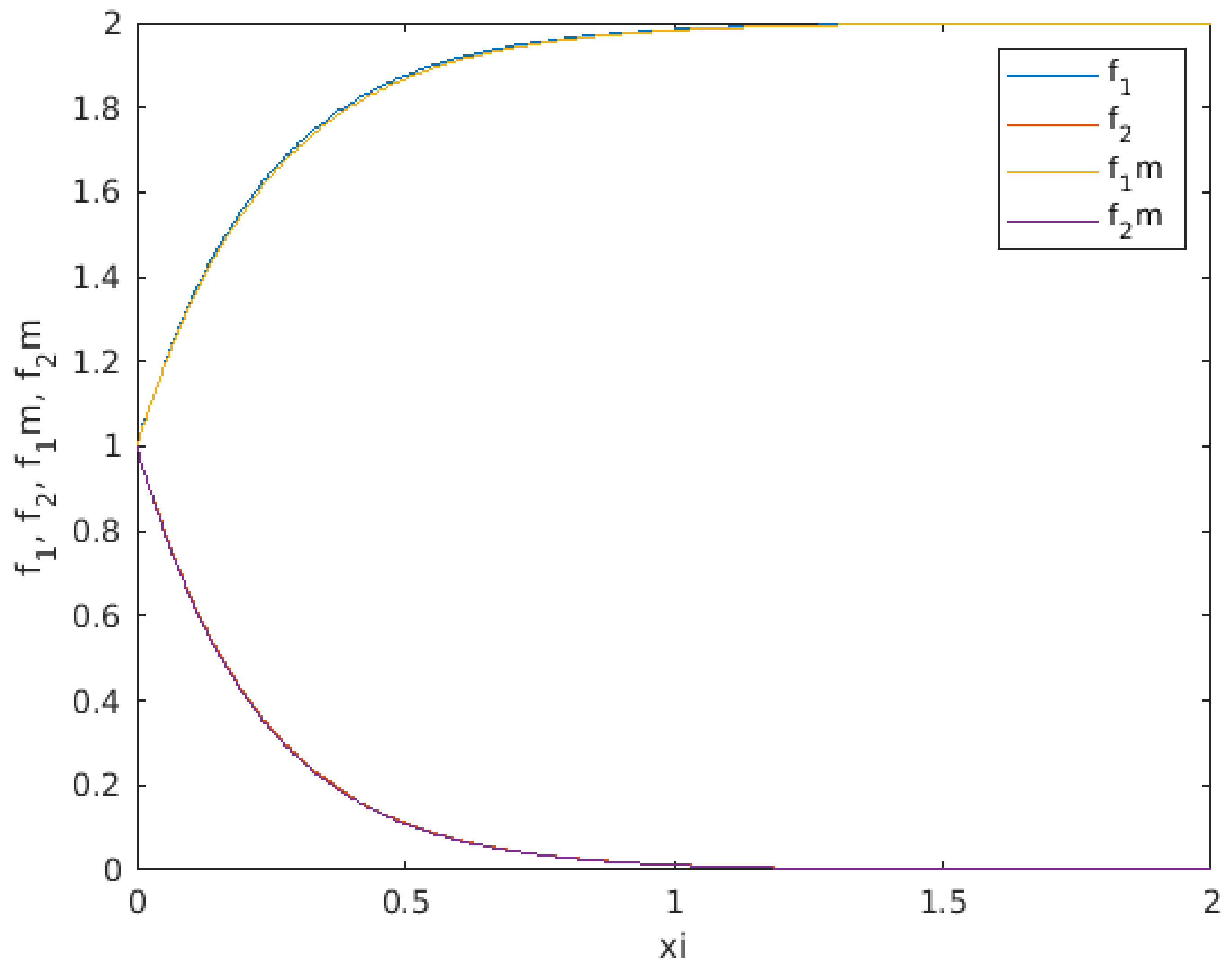
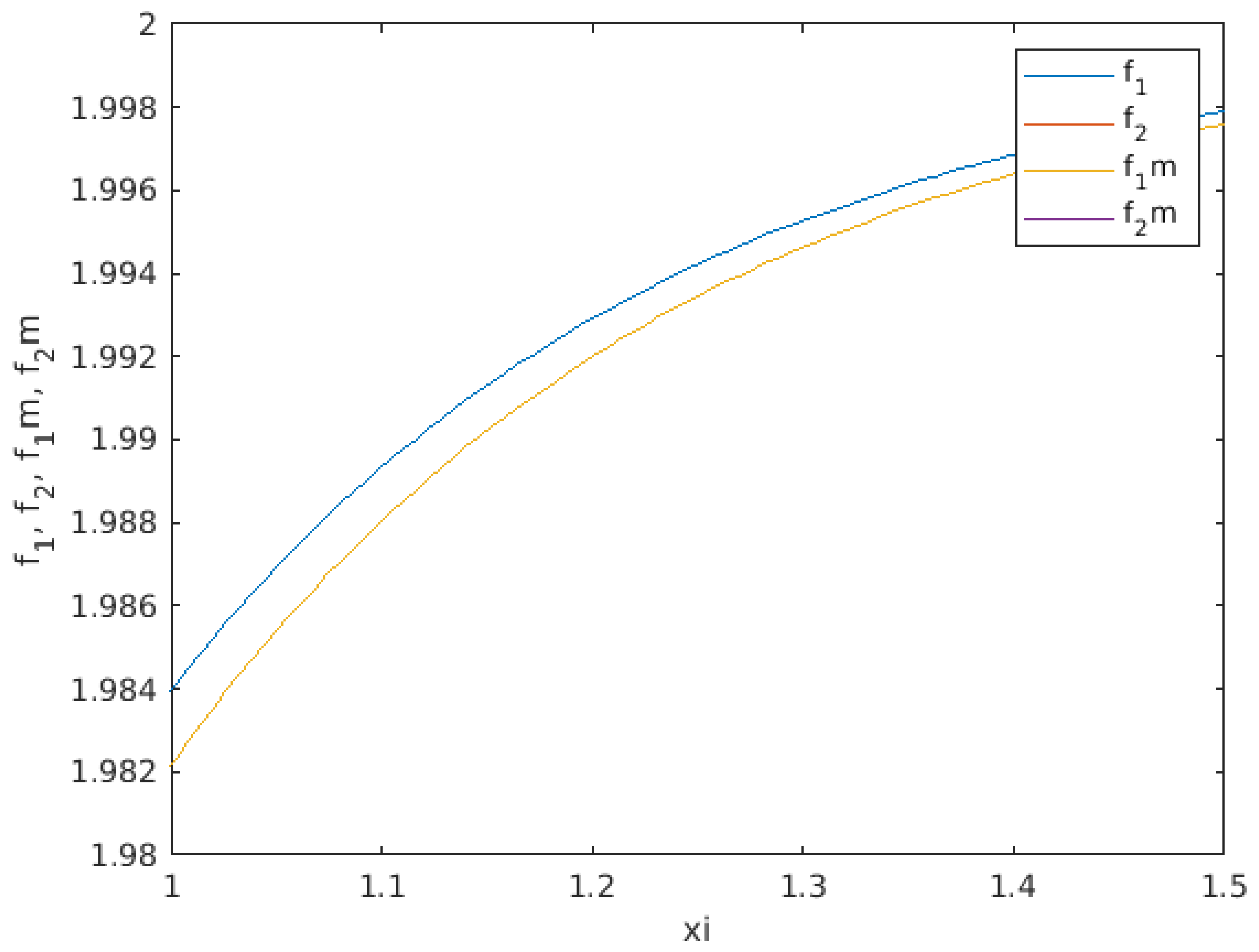
- Case with and —according to the results in Figure 1, Figure 2 and Figure 3, TW profiles to (20) are lower solutions compared to those for (19). Note that (as solution to (20)) is positive along the domain. Then, any TW moving with the exponential decaying term as per (25) is positive in the whole domain. In addition, we note that the solutions to (19) are very close to solutions to the linearised (20), which permits the validation of the goodness of the linearised exercise;
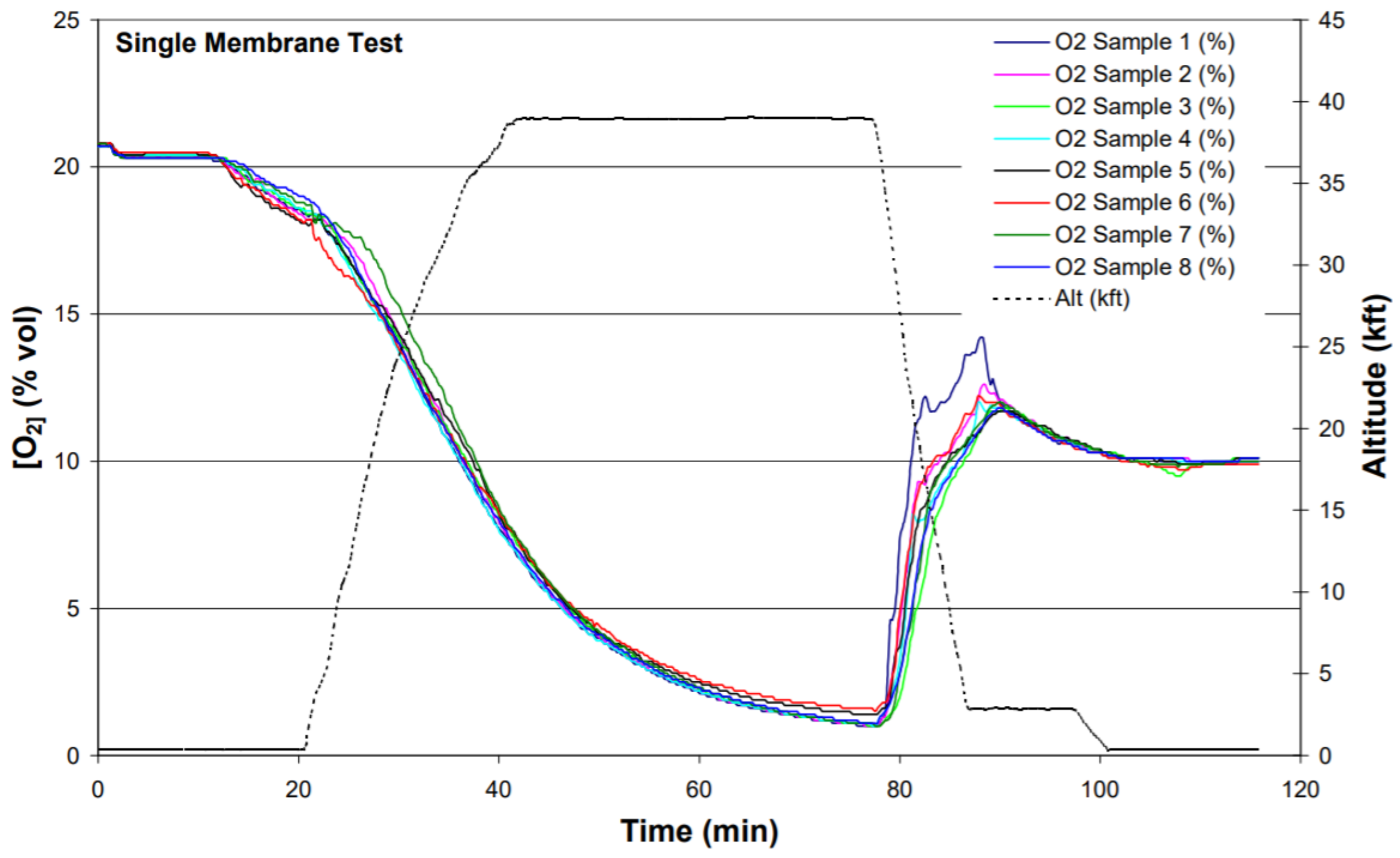
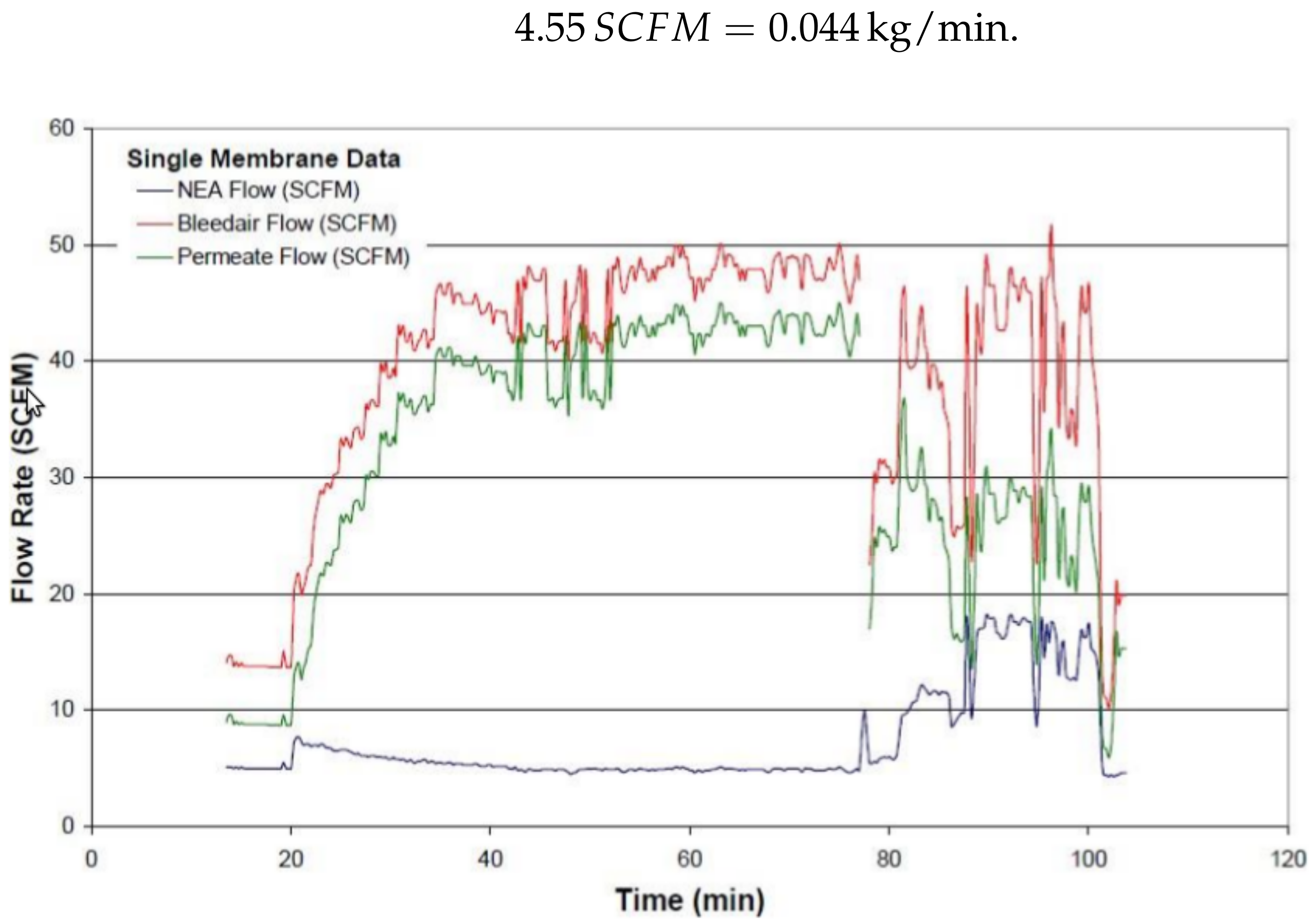
3.3. Application to a Fuel Tank Inerting Process
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Fisher, R.A. The advance of advantageous genes. Ann. Eugen. 1937, 7, 355–369. [Google Scholar] [CrossRef] [Green Version]
- Kolmogorov, A.N.; Petrovskii, I.G.; Piskunov, N.S. Study of the diffusion equation with growth of the quantity of matter and its application to a biological problem. Dyn. Curved Front. 1937, 1937, 105–130. [Google Scholar]
- Aronson, D. Density-dependent interaction-diffusion systems. In Dynamics and Modelling of Reactive Systems; Academic Press: New York, NY, USA, 1980. [Google Scholar]
- Aronson, D.; Weinberger, H. Nonlinear diffusion in population genetics, combustion and nerve propagation. In Partial Differential Equations and Related Topic; Springer: New York, NY, USA, 1975; pp. 5–49. [Google Scholar]
- Aronson, D.; Weinberger, H. Multidimensional nonlinear diffusion arising in population genetics. Adv. Math. 1978, 30, 33–76. [Google Scholar] [CrossRef] [Green Version]
- Cangiani, A.; Georgoulis, E.H.; Morozov, A.; Sutton, O.J. Revealing new dynamical patterns in a reaction–diffusion model with cyclic competition via a novel computational framework. Proc. R. Soc. 2018, 474. [Google Scholar] [CrossRef]
- Volpert, V.; Petrovskii, S. Reaction-diffusion waves in biology. Phys. Life 2009, 6, 267–310. [Google Scholar] [CrossRef]
- Barenblatt, G.I.; Zeldovich, Y.B. On stability of flame propagation. Prikl. Mat. Mekh. 1957, 21, 856–859. (In Russian) [Google Scholar]
- Federal Aviation Administration. Advisory Circular Ref. 25.981-1C. Fuel Tank Ignition Source Prevention Guidelines; Federal Aviation Administration: Washington, DC, USA, 2008.
- SAE International. Aircraft Fuel Tank Inerting Systems; SAE Ref ARP6078; SAE International: Warrendale, PA, USA, 2012. [Google Scholar]
- Cavage, W.M.; Bowman, T. Modeling in flight inert gas distribution in a 747 center wing fuel tank. In Proceedings of the 35th AIAA Fluid Dynamics Conference and Exhibit, Toronto, ON, Canada, 6–9 June 2005. [Google Scholar]
- Burns, M.; Cavage, W.M. Inerting of a Vented Aircraft Fuel Tank Test Article with Nitrogen Enriched Air; Report for the Federal Aviation Administration, Report No. DOT/FAA/AR-01/6; Federal Aviation Administration: Washington, DC, USA, 2001.
- Ghadirian, E.; Brown, J.; Wahiduzzaman, S. A Quasy-Steady Diffusion Based Model for Design and Analysis of Fuel Tank Evaporate Emissions; SAE Technical Paper Ref. 2019-01-0947; SAE International: Warrendale, PA, USA, 2019. [Google Scholar]
- Burns, M.; Cavage, W.; Hill, R.; Morrison, R. Flight-Testing of the FAA Onboard Inert Gas Generation System on an Airbus A320. DOT/FAA/AR-03/58; U.S. Department of Transportation Federal Aviation Administration: Washington, DC, USA, 2004.
- Feng, S.; Li, C.; Peng, X.; Wen, T.; Yan, Y.; Jiang, R.; Liu, W. Oxygen concentration variation in ullage influenced by dissolved oxygen evolution. Chin. J. Aeronaut. 2020. [Google Scholar] [CrossRef]
- Banerjee, R.A. A numerical study of combined heat and mass transfer in an inclined channel using the VOF multiphase model. Numer. Heat Transf. Part A Appl. 2007, 52, 163–183. [Google Scholar] [CrossRef]
- Karpinska, A.M.; Bridgeman, J. CFD-aided modelling of activated sludge systems—A critical review. Water Res. 2016, 88, 861–879. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kiselev, V.G. Fundamentals of diffusion MRI (Magnetic Resonance Imaging) Physics. NMR Biomed. 2017, 30, 3602. [Google Scholar] [CrossRef] [PubMed]
- Bhatti, M.; Zeeshan, A.; Ellahi, R.; Anwar Bég, O.; Kadir, A. Effects of coagulation on the two-phase peristaltic pumping of magnetized prandtl biofluid through an endoscopic annular geometry containing a porous medium. Chin. J. Phys. 2019, 58, 222–223. [Google Scholar] [CrossRef]
- Ellahi, R.; Hussain, F.; Ishtiaq, F.; Hussain, A. Peristaltic transport of Jeffrey fluid in a rectangular duct through a porous medium under the effect of partial slip: An application to upgrade industrial sieves/filters. Pramana J. Phys. 2019, 93, 34. [Google Scholar] [CrossRef]
- Pao, C.V. Nonlinear Parabolic and Elliptic Equations; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Enright, H.; Muir, P.H. A Runge-Kutta Type Boundary Value ODE Solver with Defect Control; Teh. Rep. 267/93; University of Toronto, Dept. of Computer Sciences: Toronto, ON, Canada, 1993. [Google Scholar]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; John Wiley & Sons: Hoboken, NJ, USA, 1960; p. 780. [Google Scholar]
- Kuchta, J.M. Oxygen Dilution Requirements for Inerting Aircraft Fuel Tanks; Bureau of Mines Safety Research Center: Washington, DC, USA, 1970; pp. 85–115. [Google Scholar]
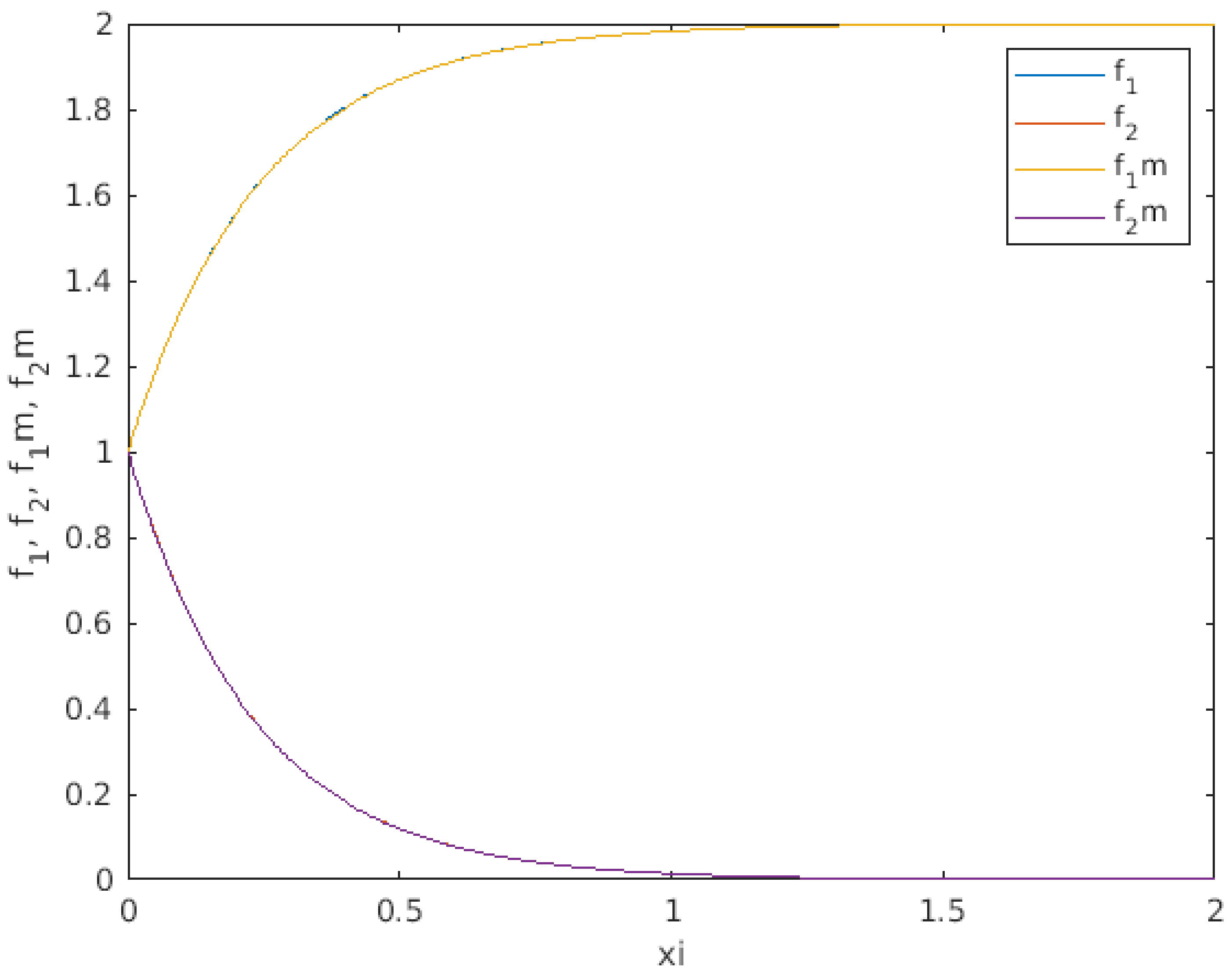
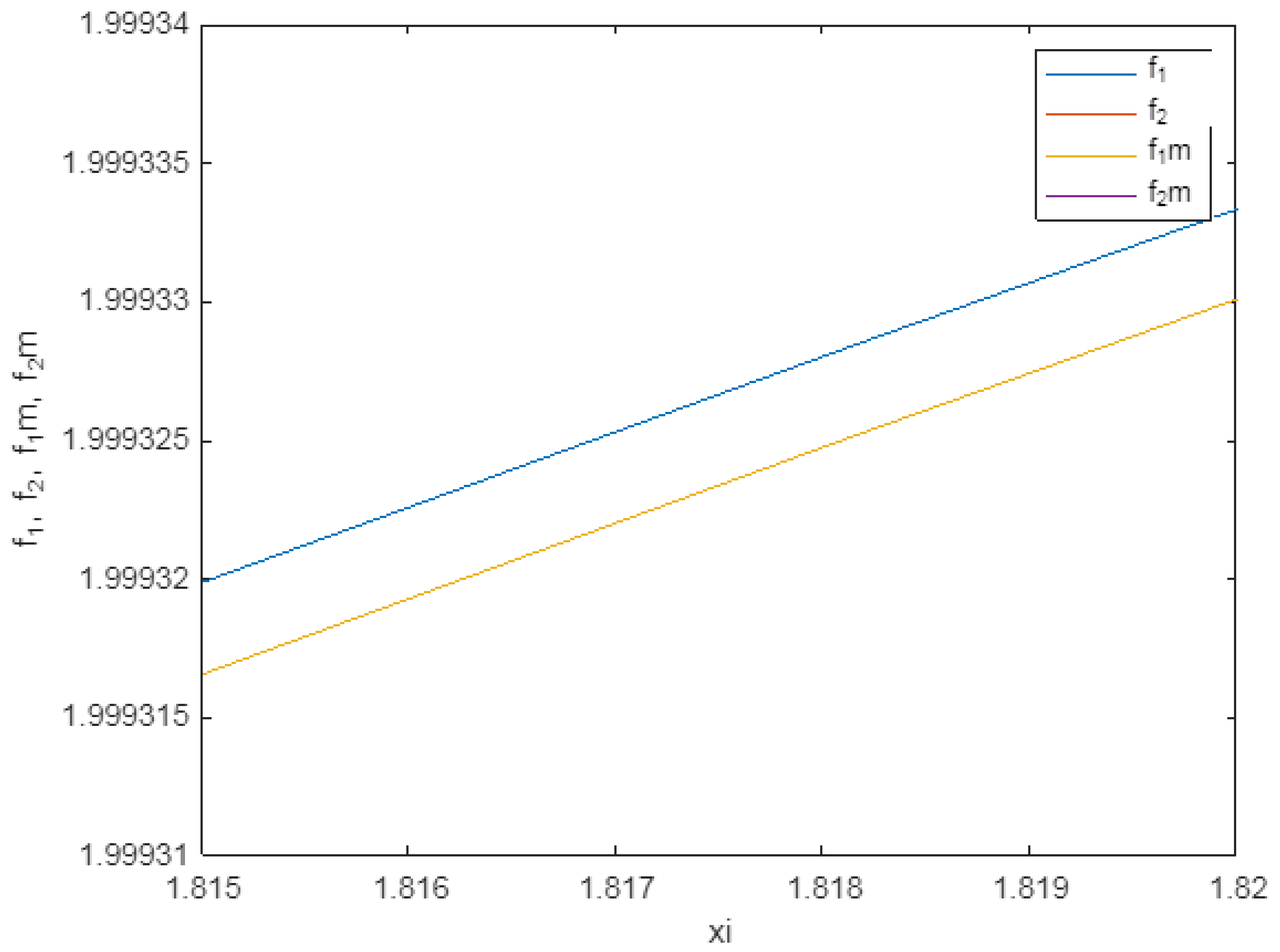

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palencia, J.L.D. Travelling Waves Approach in a Parabolic Coupled System for Modelling the Behaviour of Substances in a Fuel Tank. Appl. Sci. 2021, 11, 5846. https://doi.org/10.3390/app11135846
Palencia JLD. Travelling Waves Approach in a Parabolic Coupled System for Modelling the Behaviour of Substances in a Fuel Tank. Applied Sciences. 2021; 11(13):5846. https://doi.org/10.3390/app11135846
Chicago/Turabian StylePalencia, José Luis Díaz. 2021. "Travelling Waves Approach in a Parabolic Coupled System for Modelling the Behaviour of Substances in a Fuel Tank" Applied Sciences 11, no. 13: 5846. https://doi.org/10.3390/app11135846
APA StylePalencia, J. L. D. (2021). Travelling Waves Approach in a Parabolic Coupled System for Modelling the Behaviour of Substances in a Fuel Tank. Applied Sciences, 11(13), 5846. https://doi.org/10.3390/app11135846





