Superfluid Transition and Specific Heat of the 2D x-y Model: Monte Carlo Simulation
Abstract
:1. Introduction
2. Model and Methodology
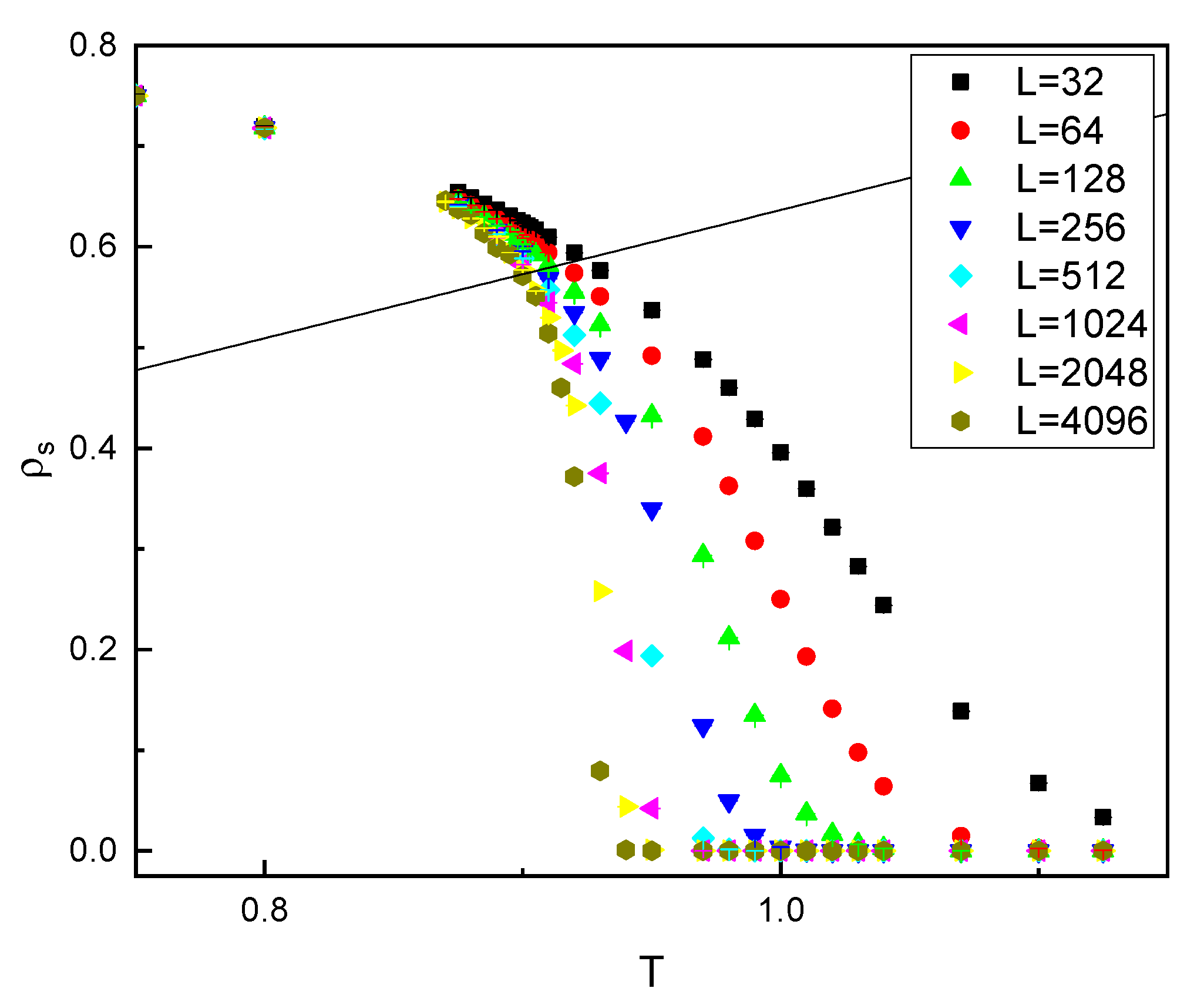
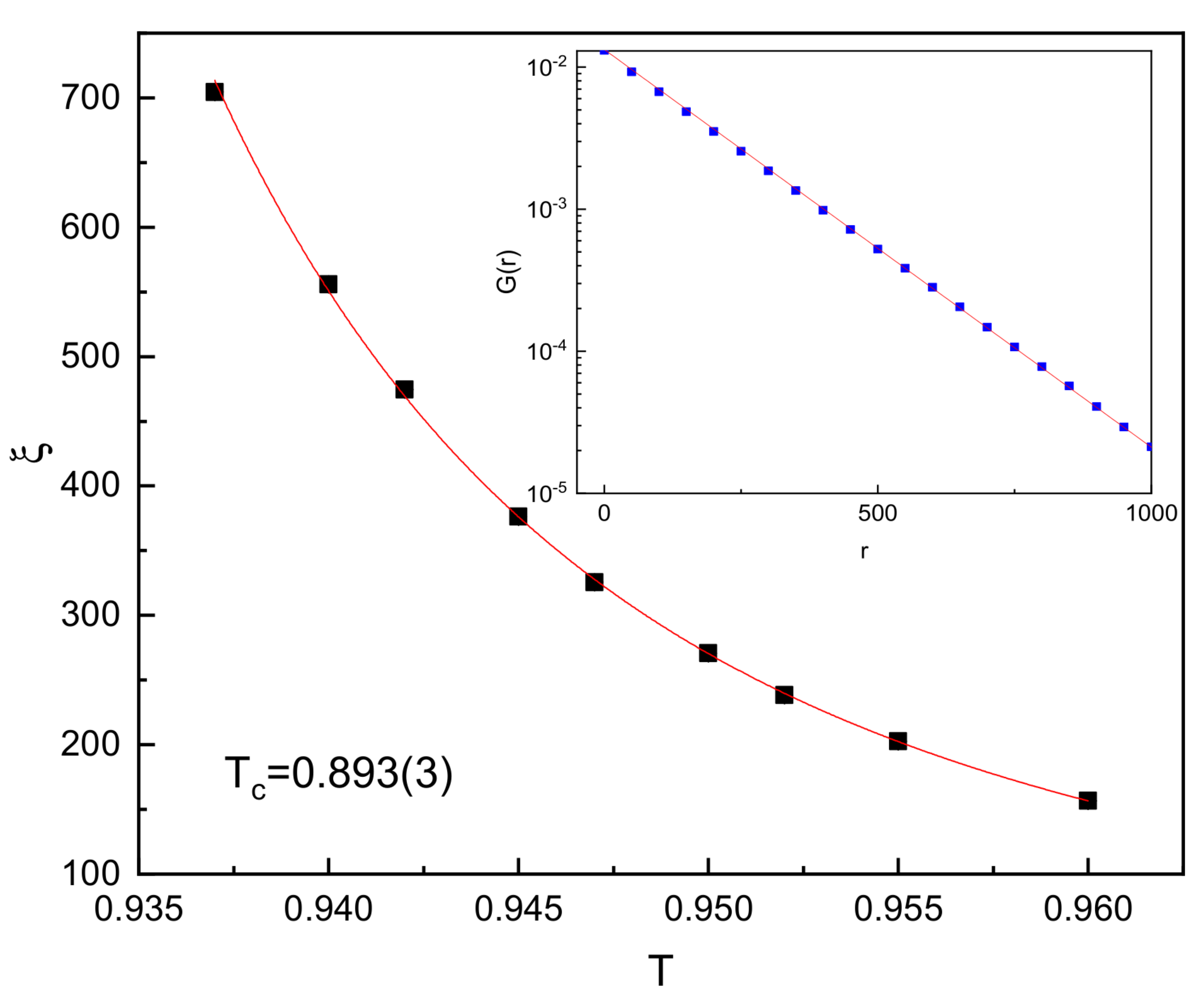
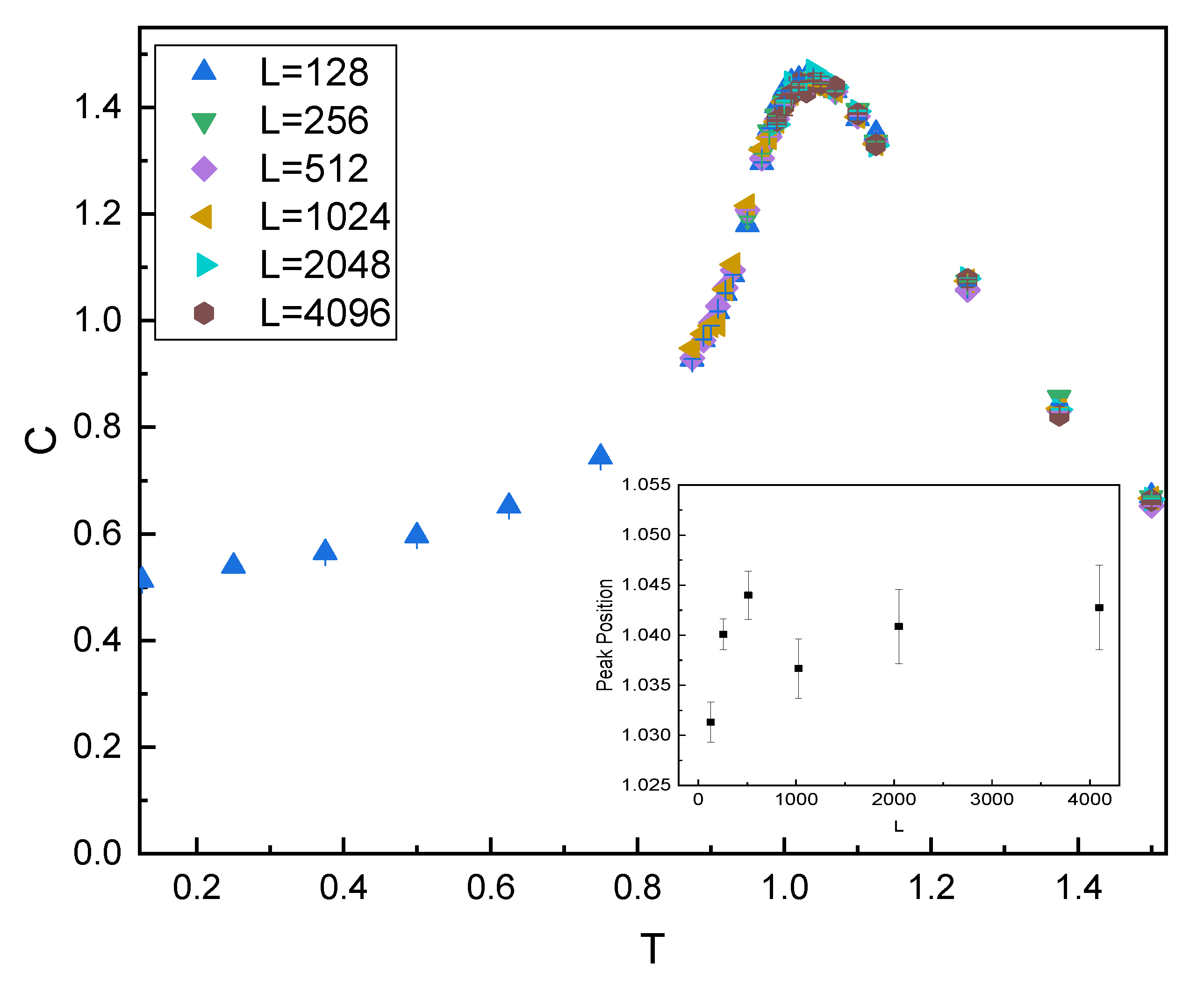
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kosterlitz, J.M.; Thouless, D.J. Long range order and metastability in two dimensional solids and superfluids. (Application of dislocation theory). J. Phys. C Solid State Phys. 1972, 5, L124. [Google Scholar] [CrossRef]
- Kosterlitz, J.M.; Thouless, D.J. Ordering, metastability and phase transitions in two-dimensional systems. J. Phys. C Solid State Phys. 1973, 6, 1181. [Google Scholar] [CrossRef]
- Kosterlitz, J.M.; Thouless, D.J. The critical properties of the two-dimensional xy model. J. Phys. C Solid State Phys. 1974, 7, 1046. [Google Scholar] [CrossRef]
- Nelson, D.R. XVI. Superfluidity and the two dimensional XY model. Phys. Rep. 1979, 49, 255–259. [Google Scholar] [CrossRef]
- Bishop, D.J.; Reppy, J.D. Study of the Superfluid Transition in Two-Dimensional 4He Films. Phys. Rev. Lett. 1978, 40, 1727–1730. [Google Scholar] [CrossRef]
- Agnolet, G.; McQueeney, D.F.; Reppy, J.D. Kosterlitz-Thouless transition in helium films. Phys. Rev. B 1989, 39, 8934–8958. [Google Scholar] [CrossRef] [Green Version]
- Csáthy, G.; Tulimieri, D.; Yoon, J.; Chan, M.H.W. Heat Capacity and Superfluid Density of Thin 4He Films on Porous Gold and on H2. Phys. Rev. Lett. 1998, 80, 4482–4485. [Google Scholar] [CrossRef]
- Boninsegni, M.; Cole, M.W.; Toigo, F. Helium Adsorption on a Lithium Substrate. Phys. Rev. Lett. 1999, 83, 2002–2005. [Google Scholar] [CrossRef]
- Van Cleve, E.; Taborek, P.; Rutledge, J.M. Helium Adsorption on Lithium Substrate. J. Low Temp. Phys. 2008, 150, 1–11. [Google Scholar] [CrossRef]
- Kosterlitz, J.M. Superfluidity in Thin Films of 4He. J. Low Temp. Phys. 2020, 201, 504–581. [Google Scholar] [CrossRef]
- Huberman, B.A.; Dash, J.G. Onset of superflow in thin helium films. Phys. Rev. B 1978, 17, 398–401. [Google Scholar] [CrossRef]
- Mon, K.K.; Saam, W.F. Two-layer XY model for 4He films. Phys. Rev. B 1980, 22, 3221–3229. [Google Scholar] [CrossRef]
- Schultka, N.; Manousakis, E. Specific heat of superfluids near the transition temperature. Phys. Rev. B 1995, 52, 7528. [Google Scholar] [CrossRef] [Green Version]
- Schultka, N.; Manousakis, E. Crossover from two- to three-dimensional behavior in superfluids. Phys. Rev. B 1995, 51, 11712–11720. [Google Scholar] [CrossRef] [Green Version]
- Komura, Y.; Okabe, Y. Large-Scale Monte Carlo Simulation of Two-Dimensional Classical XY Model Using Multiple GPUs. J. Phys. Soc. Jpn. 2012, 81, 113001. [Google Scholar] [CrossRef] [Green Version]
- Tobochnik, J.; Chester, G.V. Monte Carlo study of the planar spin model. Phys. Rev. B 1979, 20, 3761–3769. [Google Scholar] [CrossRef]
- Van Himbergen, J.E.; Chakravarty, S. Helicity modulus and specific heat of classical XY model in two dimensions. Phys. Rev. B 1981, 23, 359–361. [Google Scholar] [CrossRef]
- Evertz, H.G.; Landau, D.P. Critical dynamics in the two-dimensional classical XY model: A spin-dynamics study. Phys. Rev. B 1996, 54, 12302–12317. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gupta, R.; Baillie, C.F. Critical behavior of the two-dimensional XY model. Phys. Rev. B 1992, 45, 2883–2898. [Google Scholar] [CrossRef] [PubMed]
- Cuccoli, A.; Tognetti, V.; Vaia, R. Two-dimensional XXZ model on a square lattice: A Monte Carlo simulation. Phys. Rev. B 1995, 52, 10221–10231. [Google Scholar] [CrossRef]
- Hasenbusch, M. The two-dimensional XY model at the transition temperature: A high-precision Monte Carlo study. J. Phys. A Math. Gen. 2005, 38, 5869–5884. [Google Scholar] [CrossRef]
- Hsieh, Y.D.; Kao, Y.J.; Sandvik, A.W. Finite-size scaling method for the Berezinskii–Kosterlitz–Thouless transition. J. Stat. Mech. Theory Exp. 2013, 2013, P09001. [Google Scholar] [CrossRef] [Green Version]
- Wang, B.Z.; Hou, P.; Huang, C.J.; Deng, Y. Percolation of the two-dimensional XY model in the flow representation. arXiv 2020, arXiv:2010.14427. [Google Scholar]
- Steele, L.M.; Yeager, C.J.; Finotello, D. Precision specific-heat studies of thin superfluid films. Phys. Rev. Lett. 1993, 71, 3673–3676. [Google Scholar] [CrossRef] [PubMed]
- Boninsegni, M.; Moroni, S. Specific heat of thin 4He films on graphite. Phys. Rev. B 2020, 102, 235436. [Google Scholar] [CrossRef]
- Ceperley, D.M.; Pollock, E.L. Path-integral simulation of the superfluid transition in two-dimensional 4He. Phys. Rev. B 1989, 39, 2084–2093. [Google Scholar] [CrossRef] [PubMed]
- Prokof’ev, N.; Svistunov, B. Worm Algorithms for Classical Statistical Models. Phys. Rev. Lett. 2001, 87, 160601. [Google Scholar] [CrossRef] [Green Version]
- Pollock, E.L.; Ceperley, D.M. Path-integral computation of superfluid densities. Phys. Rev. B 1987, 36, 8343. [Google Scholar] [CrossRef]
- Nelson, D.R.; Kosterlitz, J.M. Universal Jump in the Superfluid Density of Two-Dimensional Superfluids. Phys. Rev. Lett. 1977, 39, 1201. [Google Scholar] [CrossRef] [Green Version]
- Prokof’ev, N.V.; Svistunov, B.V. Two definitions of superfluid density. Phys. Rev. B 2000, 61, 11282–11284. [Google Scholar] [CrossRef] [Green Version]
- Tomita, Y.; Okabe, Y. Probability-changing cluster algorithm for two-dimensional XY and clock models. Phys. Rev. B 2002, 65, 184405. [Google Scholar] [CrossRef] [Green Version]
- Janke, W.; Nather, K. High-precision Monte Carlo study of the two-dimensional XY Villain model. Phys. Rev. B 1993, 48, 7419. [Google Scholar] [CrossRef] [PubMed]
- Gould, H.; Tobochnik, J.; Wolfgang, C. An Introduction to Computer Simulation Methods: Applications to Physical Systems, 3rd ed.; Addison-Wesley Longman Publishing Co., Inc.: San Francisco, CA, USA, 2005. [Google Scholar] [CrossRef]
- Jakubczyk, P.; Eberlein, A. Thermodynamics of the two-dimensional XY model from functional renormalization. Phys. Rev. E 2016, 93, 062145. [Google Scholar] [CrossRef] [Green Version]
- Ota, S.; Ota, S.B.; Fahnle, M. Microcanonical Monte Carlo simulations for the two-dimensional XY model. J. Phys. Condens. Matter 1992, 4, 5411. [Google Scholar] [CrossRef]
- Ota, S.; Ota, S. Vortices in the 2d classical XY-model: A microcanonical Monte-Carlo simulation study. Phys. Lett. A 1995, 206, 133–136. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, P.H.; Boninsegni, M. Superfluid Transition and Specific Heat of the 2D x-y Model: Monte Carlo Simulation. Appl. Sci. 2021, 11, 4931. https://doi.org/10.3390/app11114931
Nguyen PH, Boninsegni M. Superfluid Transition and Specific Heat of the 2D x-y Model: Monte Carlo Simulation. Applied Sciences. 2021; 11(11):4931. https://doi.org/10.3390/app11114931
Chicago/Turabian StyleNguyen, Phong H., and Massimo Boninsegni. 2021. "Superfluid Transition and Specific Heat of the 2D x-y Model: Monte Carlo Simulation" Applied Sciences 11, no. 11: 4931. https://doi.org/10.3390/app11114931
APA StyleNguyen, P. H., & Boninsegni, M. (2021). Superfluid Transition and Specific Heat of the 2D x-y Model: Monte Carlo Simulation. Applied Sciences, 11(11), 4931. https://doi.org/10.3390/app11114931






