A Multiphysics Ray Optics Model for the Propagation of Electromagnetic Waves in Plasmas and the Design of Laser-Based Diagnostics in Nuclear Fusion Reactors
Abstract
1. Introduction
2. Modelling
2.1. Ray Optics
2.2. Interferometry
2.3. Polarimetry
2.4. Thomson Scattering (TS)
2.5. Interactions with the Boundaries and Other Elements
3. Validation on a Case Study
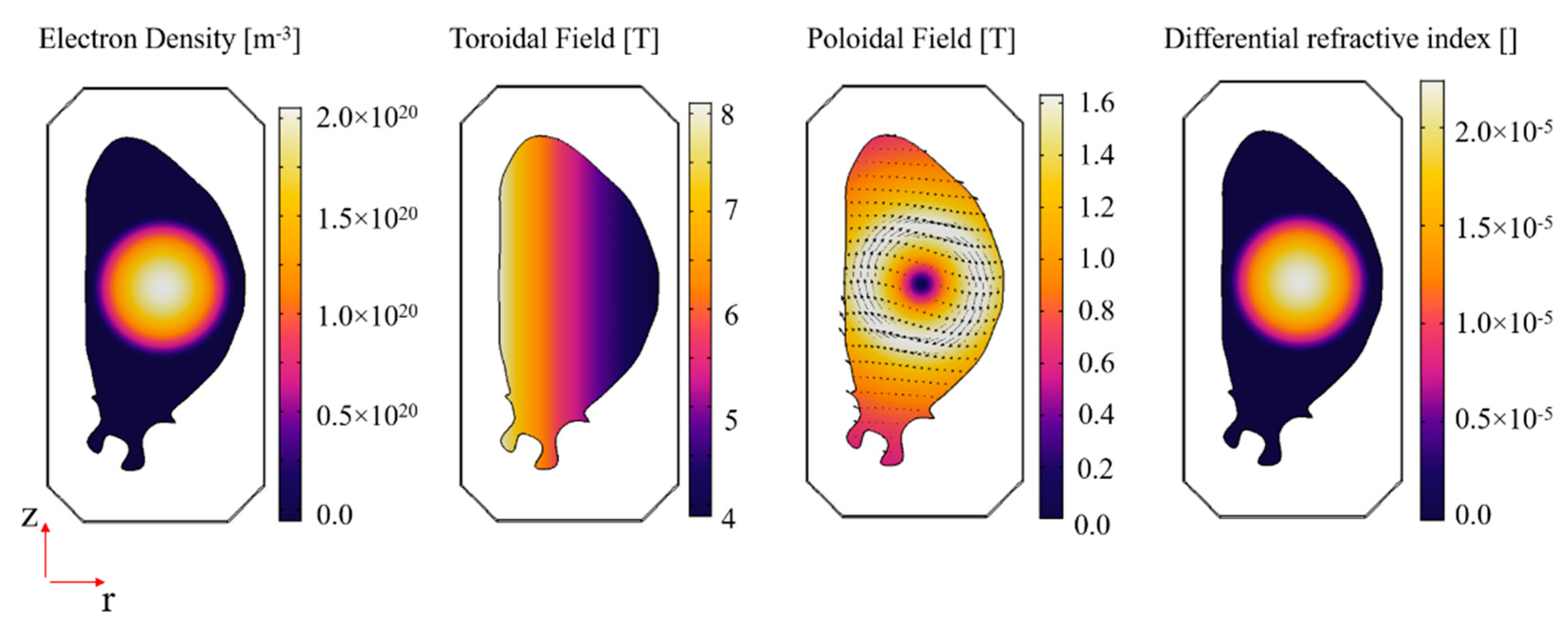
3.1. Geometry, Domain, Boundary Conditions and Mesh
3.2. Interferometer Simulations
3.3. Polarimeter Simulations
3.4. Thomson Scattering Simulations
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wesson, J. Tokamaks; Claredon Press: Oxford, UK, 2004. [Google Scholar]
- Igochine, V. Active Control of Magneto-Hydrodynamic Instabilities in Hot Plasmas; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Gelfusa, M.; Murari, A.; Lupelli, I.; Hawkes, N.; Gaudio, P.; Baruzzo, M.; Brix, M.; Craciunescu, T.; Drozdov, V.; Meigs, A.; et al. Influence of plasma diagnostics and constraints on the quality of equilibrium. Rev. Sci. Instrum. 2013, 84, 103508. [Google Scholar] [CrossRef]
- Murari, A.; Angelone, M.; Bonheure, G.; Cecil, E.; Craciunescu, T.; Darrow, D.; Edlington, T.; Ericsson, G.; Gatu-Johnson, M.; Gorini, G.; et al. New developments in the diagnostics for the fusion products on JET in preparation for ITER. Rev. Sci. Instrum. 2010, 81, 10E136. [Google Scholar] [CrossRef] [PubMed]
- Rigamonti, D.; Giacomelli, L.; Gorini, G.; Nocente, M.; Rebai, M.; Tardocchi, M.; Angelone, M.; Batistoni, P.; Cufar, A.; Ghani, Z.; et al. Neutron spectroscopy measurements of 14 MeV neutrons at unprecedented energy resolution and implications for deuterium-tritium fusion plasma diagnostics. Meas. Sci. Technol. 2018, 29, 045502. [Google Scholar] [CrossRef]
- Hutchinson, I.H. Principle of Plasma Diagnostics; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Barth, C.J.; Donné, A.J. Laser-aided plasma disagnostics. Fusion Sci. Technol. 2004, 45, 407–417. [Google Scholar] [CrossRef]
- Huber, A.; McCormick, K.; Andrew, P.; de Baar, M.R.; Beaumont, P.; Dalley, S.; Fink, J.; Fuchs, J.C.; Fullard, K.; Fundamenski, W.; et al. Improved radiation measurements on JET—First results from an upgraded bolometer system. J. Nucl. Mater. 2007, 363, 365–370. [Google Scholar] [CrossRef]
- Craciunescu, T.; Peluso, E.; Murari, A.; Gelfusa, M.; Contributors, J.E.T. Maximum likelihood bolometric tomography for the determination of the uncertainties in the radiation emission on JET TOKAMAK. Rev. Sci. Instrum. 2018, 89, 053504. [Google Scholar] [CrossRef]
- Peluso, E.; Craciunescu, T.; Murari, A.; Carvalho, P.; Gelfusa, M.; Contributors, J.E.T. A comprehensive study of the uncertainties in bolometric tomography on JET using the maximum likelihood method. Rev. Sci. Instrum. 2019, 90, 123502. [Google Scholar] [CrossRef]
- Peluso, E.; Craciunescu, T.; Gelfusa, M.; Murari, A.; Carvalho, P.J.; Gaudio, P.; Contributors, J.E.T. On the effects of missing chords and systematic errors on a new tomographic method for JET bolometry. Fusion Eng. Des. 2019, 146, 2124–2129. [Google Scholar] [CrossRef]
- Orsitto, F.P.; Villari, R.; Moro, F.; Todd, T.N.; Lilley, S.; Jenkins, I.; Felton, R.; Biel, W.; Silva, A.; Scholz, M.; et al. Diagnostics and control for the steady state and pulsed tokamak DEMO. Nucl. Fusion 2016, 56, 026009. [Google Scholar] [CrossRef]
- Button, K.J. Infrared and Millimeter Waves; Academic Press: New York, NY, USA, 1979. [Google Scholar]
- Van Zeeland, M.A.; Carlstrom, T.N.; Finkenthal, D.K.; Boivin, R.L.; Colio, A.; Du, D.; Gattuso, A.; Glass, F.; Muscatello, C.M.; O’Neill, R.; et al. Tests of a two-color interferometer and polarimeter for ITER density measurements. Plasma Phys. Control Fusion 2017, 59, 125005. [Google Scholar] [CrossRef]
- Segre, S.E. A review of plasma polarimetry—Theory and methods. Plasma Phys. Control Fusion 1999, 41, R57. [Google Scholar] [CrossRef]
- Orsitto, F.P.; Boboc, A.; Gaudio, P.; Gelfusa, M.; Giovannozzi, E.; Mazzotta, C.; Murari, A.; Contributors, J.E.T. Analysis of Faraday rotation in JET polarimetric measurements. Plasma Phys. Control Fusion 2011, 53, 035001. [Google Scholar] [CrossRef][Green Version]
- Boboc, A.; Gelfusa, M.; Murari, A.; Gaudio, P.; Contributors, J.E.T. Recent developments of the JET far-infrared interferometer-polarimeter diagnostic. Rev. Sci. Instrum. 2010, 81, 10D538. [Google Scholar] [CrossRef]
- Pasqualotto, R.; Nielsen, P.; Gowers, C.; Beurskens, M.; Kempenaars, M.; Carlstrom, T.; Johnson, D.; Contributors, J.E.T. High resolution Thomson scattering for Joint European Torus (JET). Rev. Sci. Instrum. 2004, 75, 3891–3893. [Google Scholar] [CrossRef]
- Froula, D.H.; Glenzer, S.H.; Luhmann, N.C., Jr.; Sheffield, J. Plasma Scattering of Electromagnetic Radiation: Theory and Measurement Techniques; Oxford Academic Press: Cambridge, MA, USA, 2011. [Google Scholar]
- Donné, A.J.H.; Graswinckel, M.F.; Cavinato, M.; Giudicotti, L.; Zilli, E.; Gil, C.; Koslowski, H.R.; McCarthy, P.; Nyhan, C.; Prunty, S.; et al. Poloidal polarimeter for current density measurements in ITER. Rev. Sci. Instrum. 2004, 75, 4694–4701. [Google Scholar]
- Costley, A.E.; Sugie, T.; Vayakis, G.; Walker, C.I. Technological challenges of ITER diagnostics. Fusion Eng. Des. 2005, 74, 109–119. [Google Scholar] [CrossRef]
- Walsh, M.; Andrew, P.; Barnsley, R.; Bertalot, L.; Boivin, R.; Bora, D.; Bouhamou, R.; Ciattaglia, S.; Costley, A.E.; Counsell, G.; et al. ITER diagnostic challenges. In Proceedings of the 24th Symposium of Fusion Engineering, Chicago, IL, USA, 26–30 June 2011. [Google Scholar]
- Biel, W.; Albanese, R.; Ambrosino, R.; Ariola, M.; Berkel, M.V.; Bolshakova, I.; Brunner, K.J.; Cavazzana, R.; Cecconello, M.; Conroy, S.; et al. Diagnostics for plasma control—From ITER to DEMO. Fusion Eng. Des. 2019, 146, 465–472. [Google Scholar] [CrossRef]
- Donné, A.J.; Costley, A.E.; Morris, A.W. Diagnostics for plasma control on DEMO: Challenges of implementation. Nucl. Fusion 2012, 52, 074015. [Google Scholar] [CrossRef]
- Litnovsky, A.; Voitsenya, V.S.; Reichle, R.; Walsh, M.; Razdobarin, A.; Dmitriev, A.; Babinov, N.; Marot, L.; Moser, L.; Yan, R.; et al. Diagnostic mirrors for ITER: Research in the frame of International Tokamak Physics Activity. Nucl. Fusion 2019, 59, 066029. [Google Scholar] [CrossRef]
- Petykiewicz, J. Wave Optics; Springer: The Netherlands, 1991. [Google Scholar]
- Mouroulis, P.; Macdonald, J. Macdonald, Geometrical Optics and Optical Design; Oxford University Press: New York, NY, USA, 1997. [Google Scholar]
- Bittencourt, J.A. Pundamental of Plasma Physics; Springer: New York, NY, USA, 2004. [Google Scholar]
- Gaudio, P.; Gelfusa, M.; Murari, A.; Conetta, F.; Orsitto, F.P.; Boboc, A. Analysis of JET Polarimeter With a Propagation Code Based on the Stokes Formalism. IEEE Trans. Plasma Sci. 2013, 41, 1575–1586. [Google Scholar] [CrossRef]
- LeBlanc, B.P. Thomson scattering density calibration by Rayleigh and rotational Raman scattering on NSTX. Rev. Sci. Instrum. 2008, 79, 10E737. [Google Scholar] [CrossRef] [PubMed]
- Matoba, T.; Itagaki, T.; Yamauchi, T.; Funahashi, A. Analytical Approximation in the Theory of Relativistic Thomson Scattering for High Temperature Plasma. Jpn. J. Appl. Phys. 1979, 18, 1127–1133. [Google Scholar] [CrossRef]
- Parke, E.; Mirnov, V.V.; Den Hartog, D.J. A polarization-based Thomson scattering technique. J. Instrum. 2013, 9, C02030. [Google Scholar] [CrossRef][Green Version]
- Mirnov, V.V.; Den Hartog, D.J.; Parke, E. Exact relativistic expressions for polarization of incoherent Thomson scattering. Phys. Plasmas 2016, 23, 052108. [Google Scholar] [CrossRef]
- Mirnov, V.V.; Den Hartog, D.J. Polarization of incoherent Thomson scattering for electron temperature measurement. Plasma Phys. Control Fusion 2017, 59, 063001. [Google Scholar] [CrossRef]
- ITER—The Way to New Energy. Available online: https://www.iter.org (accessed on 19 December 2020).
- Rossi, R.; Orsitto, F.P.; Spolladore, L.; Wyss, I.; Gaudio, P. On the interpretability and uncertainty propagation of polarimetric measurements in thermonuclear plasmas as a function of the input polarisation and laser wavelength. Plasma Phys. Control Fusion 2020, 62, 105019. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Spolladore, L.; Wyss, I.; Rossi, R.; Gaudio, P. A Multiphysics Ray Optics Model for the Propagation of Electromagnetic Waves in Plasmas and the Design of Laser-Based Diagnostics in Nuclear Fusion Reactors. Appl. Sci. 2021, 11, 434. https://doi.org/10.3390/app11010434
Spolladore L, Wyss I, Rossi R, Gaudio P. A Multiphysics Ray Optics Model for the Propagation of Electromagnetic Waves in Plasmas and the Design of Laser-Based Diagnostics in Nuclear Fusion Reactors. Applied Sciences. 2021; 11(1):434. https://doi.org/10.3390/app11010434
Chicago/Turabian StyleSpolladore, Luca, Ivan Wyss, Riccardo Rossi, and Pasquale Gaudio. 2021. "A Multiphysics Ray Optics Model for the Propagation of Electromagnetic Waves in Plasmas and the Design of Laser-Based Diagnostics in Nuclear Fusion Reactors" Applied Sciences 11, no. 1: 434. https://doi.org/10.3390/app11010434
APA StyleSpolladore, L., Wyss, I., Rossi, R., & Gaudio, P. (2021). A Multiphysics Ray Optics Model for the Propagation of Electromagnetic Waves in Plasmas and the Design of Laser-Based Diagnostics in Nuclear Fusion Reactors. Applied Sciences, 11(1), 434. https://doi.org/10.3390/app11010434





