Validation of EMT Digital Twin Models for Dynamic Voltage Performance Assessment of 66 kV Offshore Transmission Network
Abstract
1. Introduction
- Modified Droop Control: This strategy is common for standalone grids, where the parallel operation of voltage forming units is developed recently using the f/P (frequency/active power) and V/Q (voltage/reactive power) droop controls similar to the control in synchronous generators [7]. Few authors have named these droop control concepts as Virtual Synchronous Machines Without Inertia (VSCM0H) [8].
- Direct Voltage Control (DVC): DVC is a representation of the conventional current control approach towards a voltage control. DVC allows for the direct control of the AC converter voltage which in turn varies the current injected by the converter [9,10]. This approach provides continuous voltage control both in steady-state and dynamic scenarios.
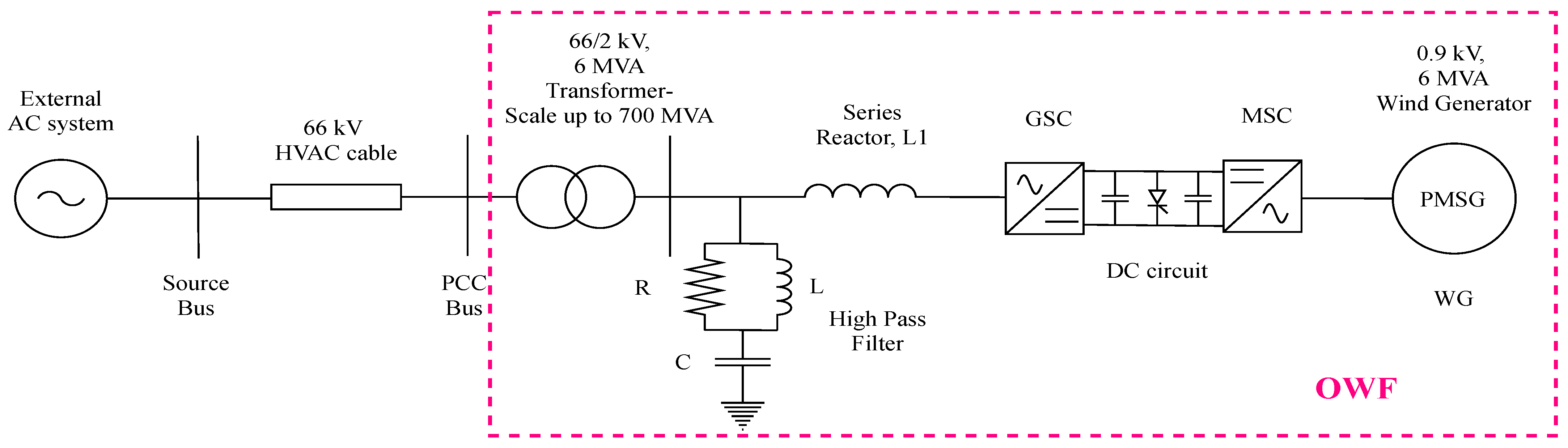
2. Overview of the Digital Twin Model in RSCAD
- An aggregated representation of ∼700 MW installed capacity OWF is modelled with the following elements:
- –
- Wind Generation System
- –
- High Pass filter (HPF) with series reactor
- –
- OWF transformer
- HVAC cables
- External AC system
2.1. Aggregated OWF
2.1.1. Wind Generation System
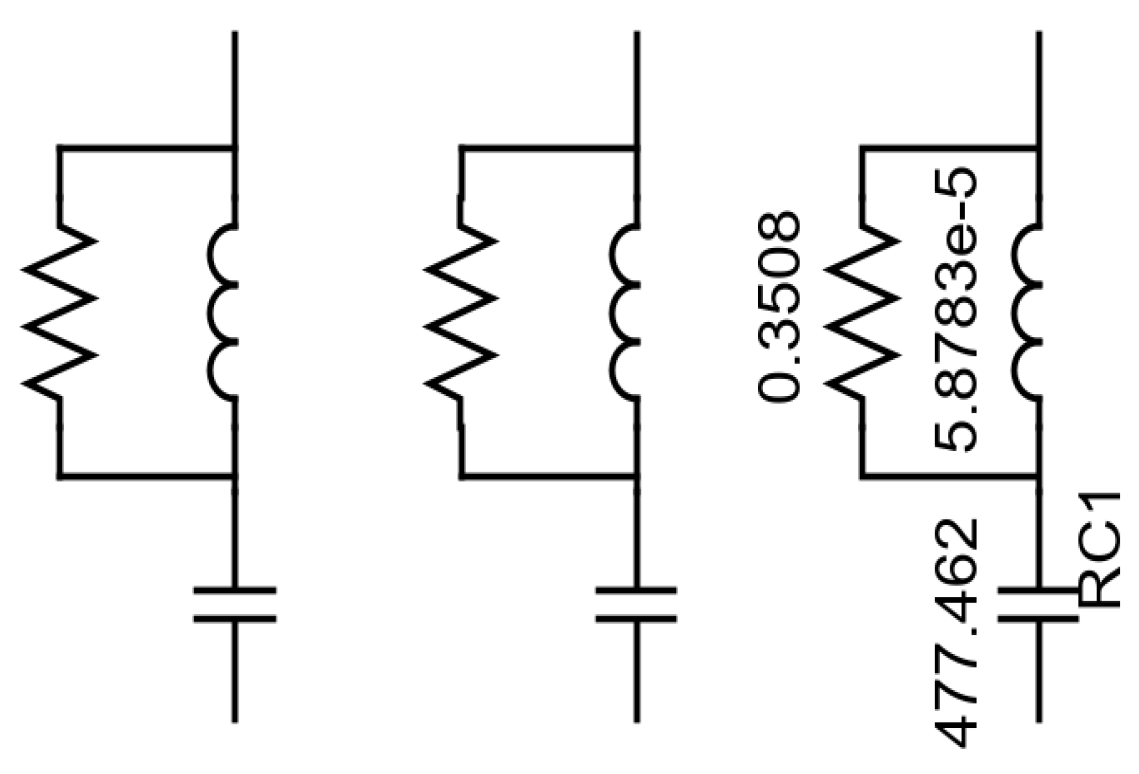
2.1.2. High Pass Filter (HPF) with Series Reactor
2.1.3. OWF Transformer
2.2. HVAC Cables
2.3. External AC System
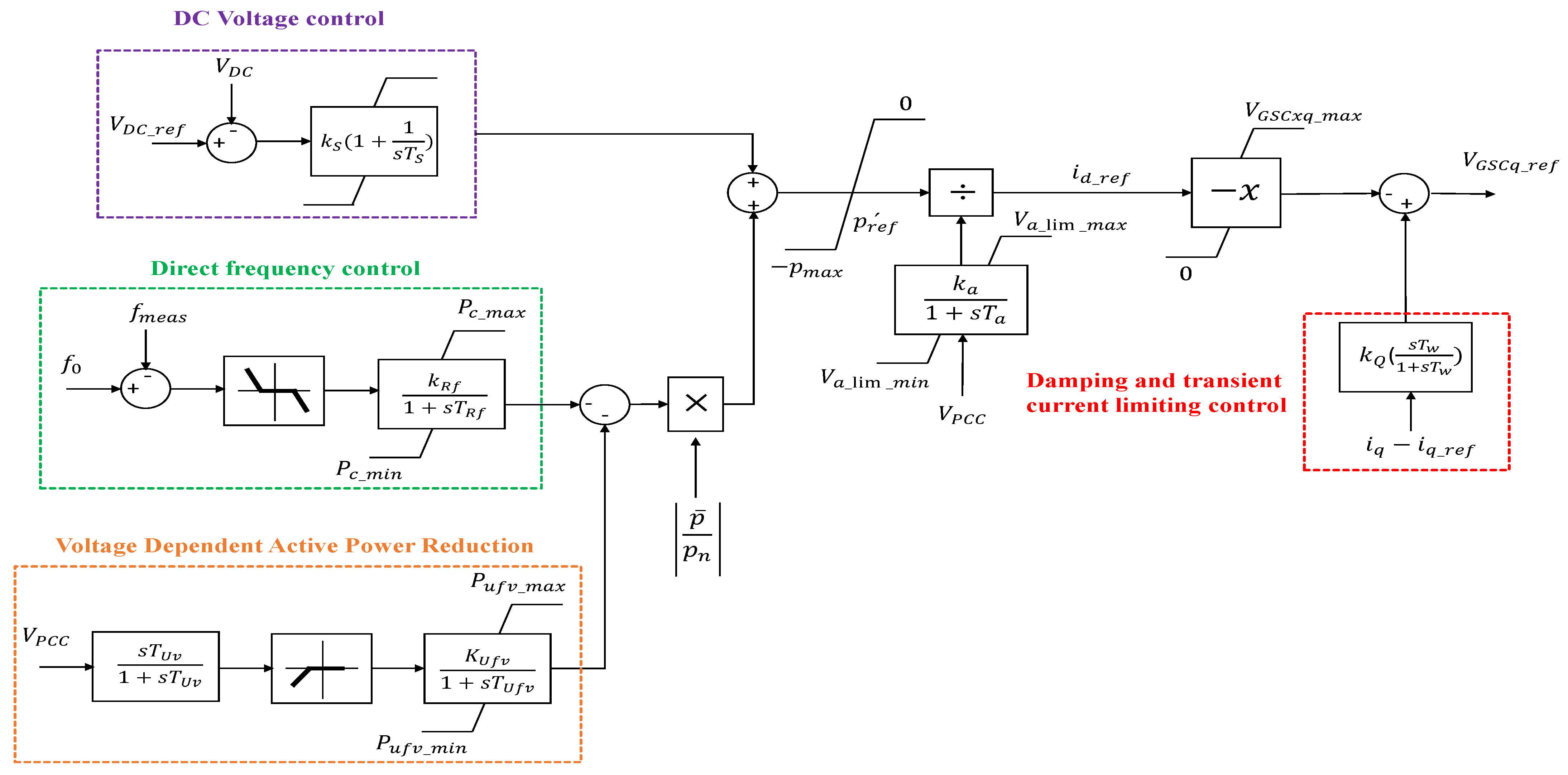
3. Control Structures
3.1. Implementation of DVC in RSCAD
3.1.1. Reactive Power Control
3.1.2. Active Power Control
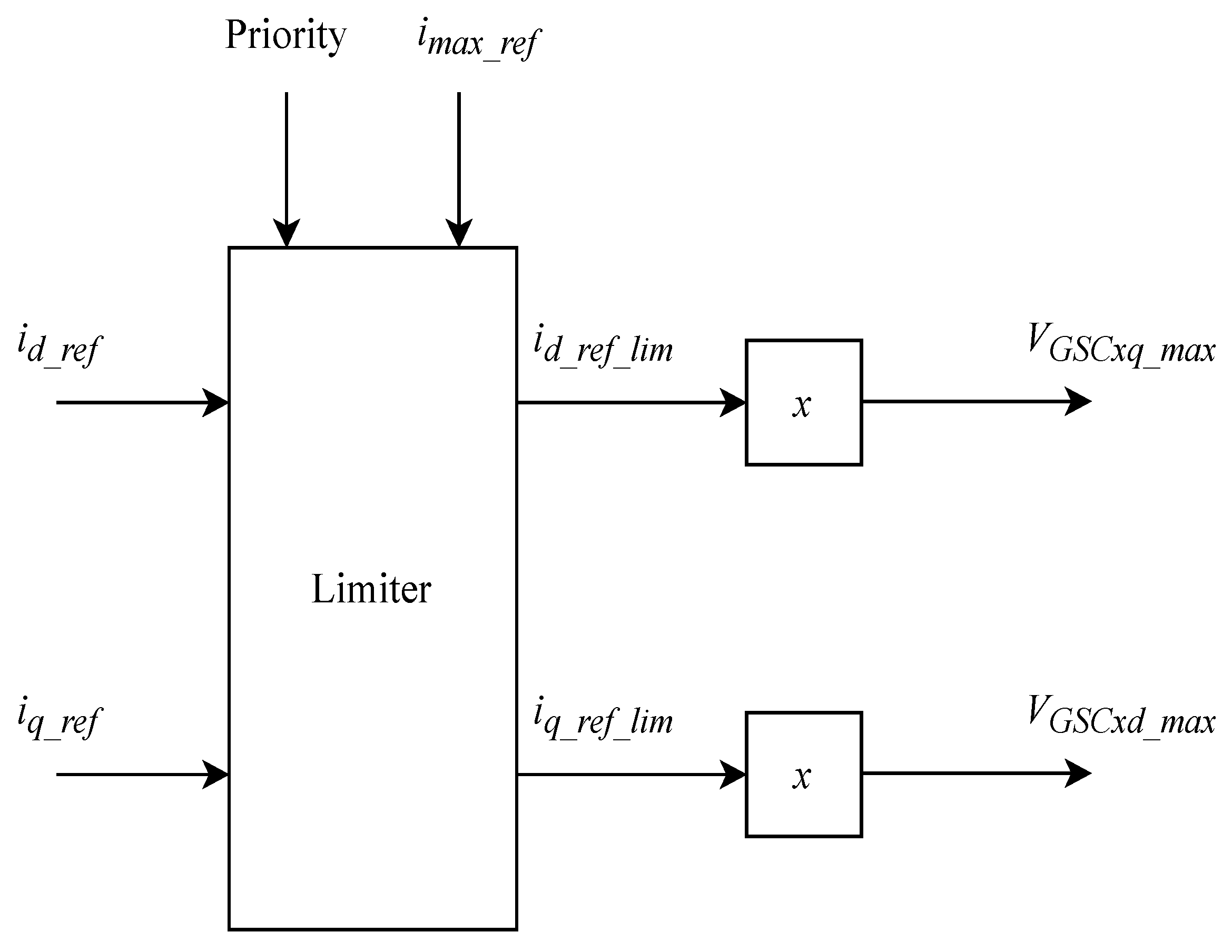
3.1.3. Current Limitation
3.1.4. Voltage Limitation
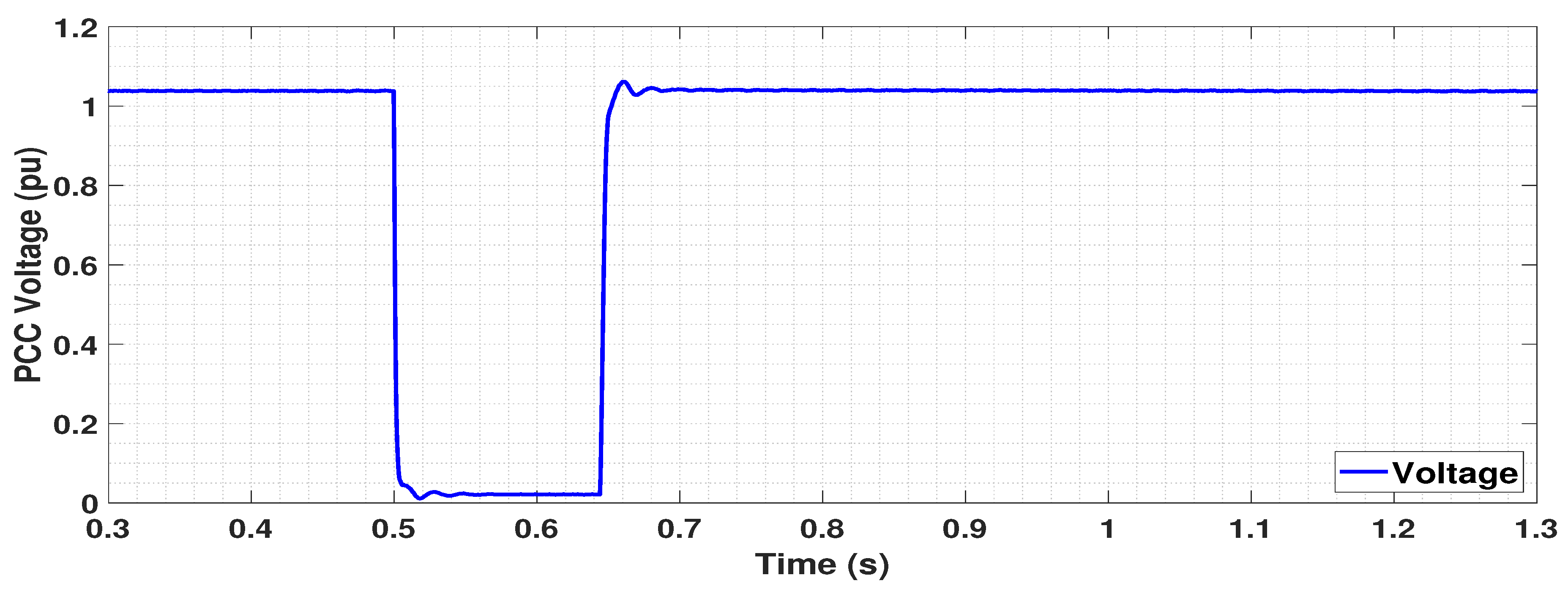
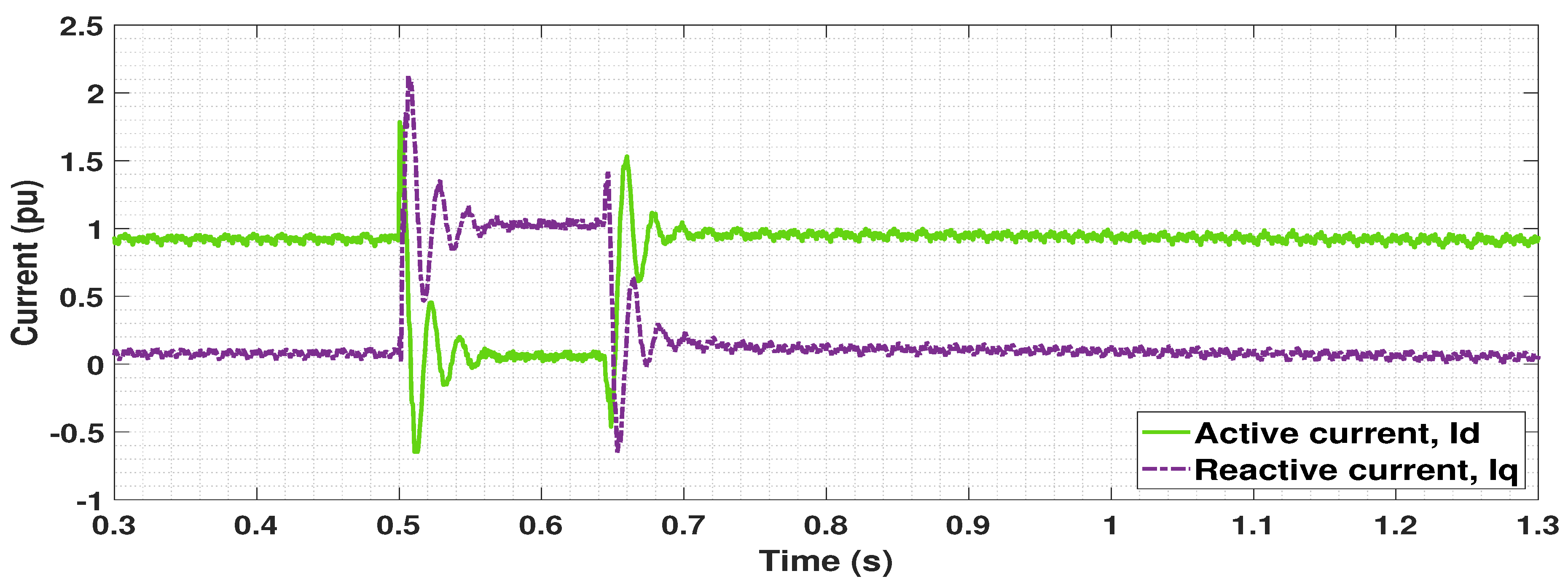
4. Analysis of the Dynamic Performance of 66 kV Test System
4.1. Three-Phase Line to Ground Fault
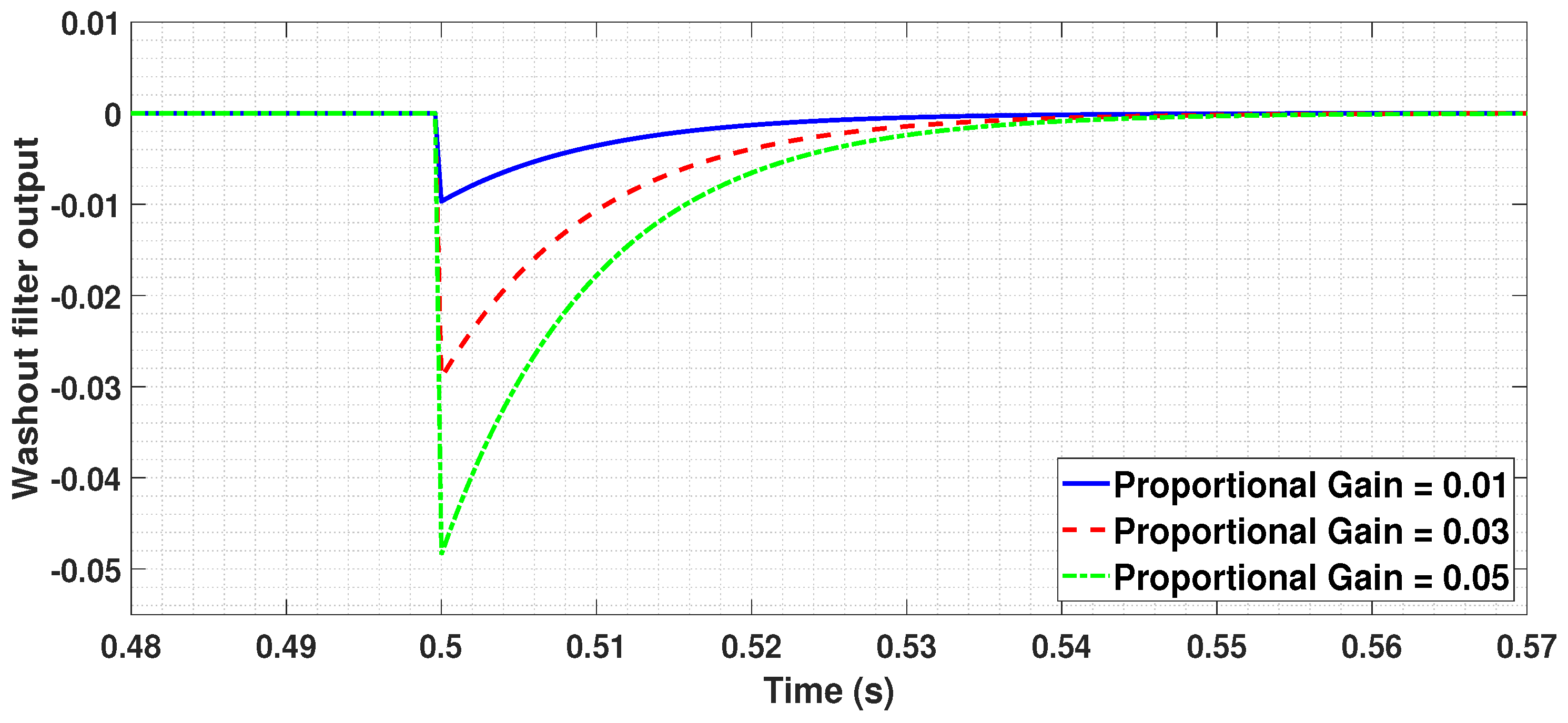
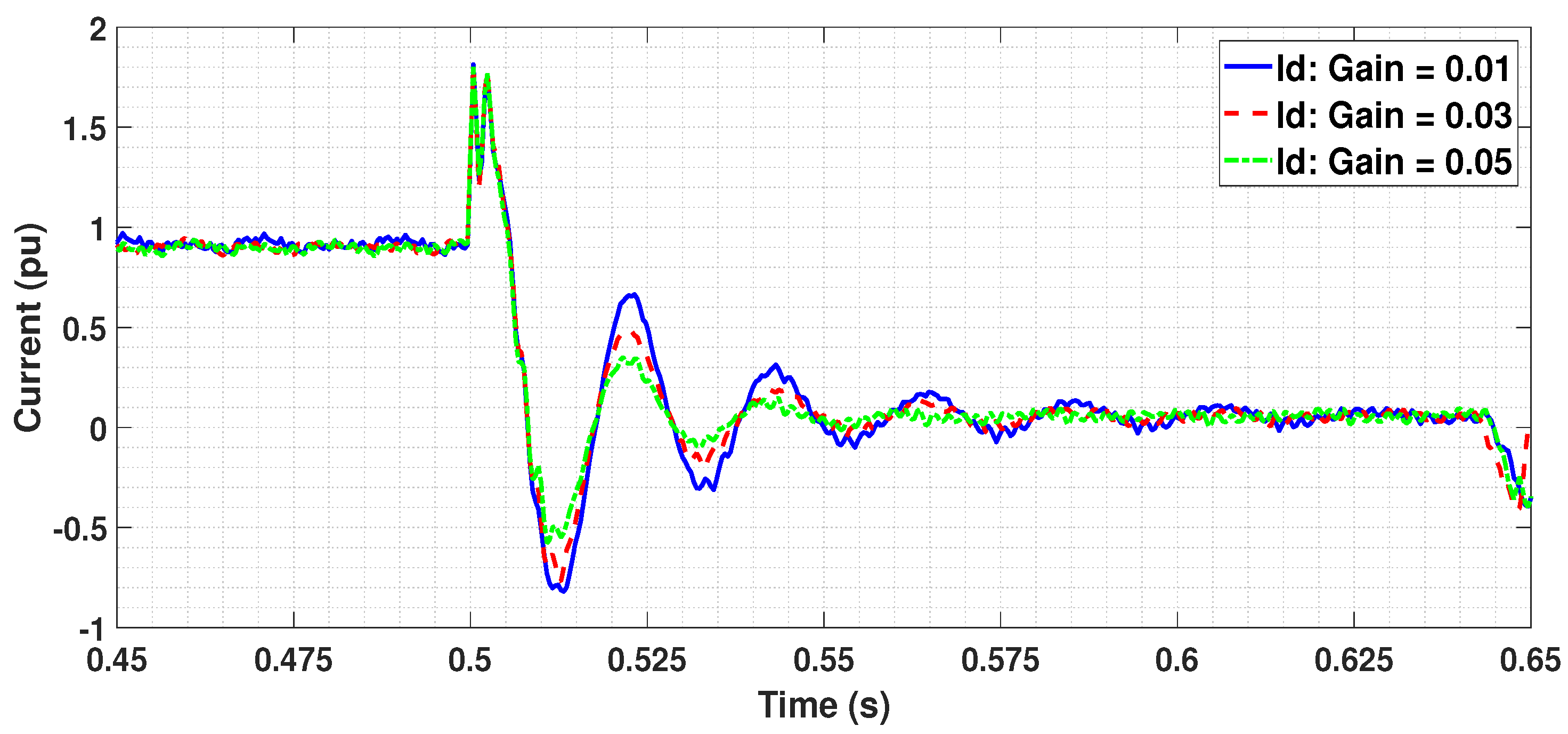
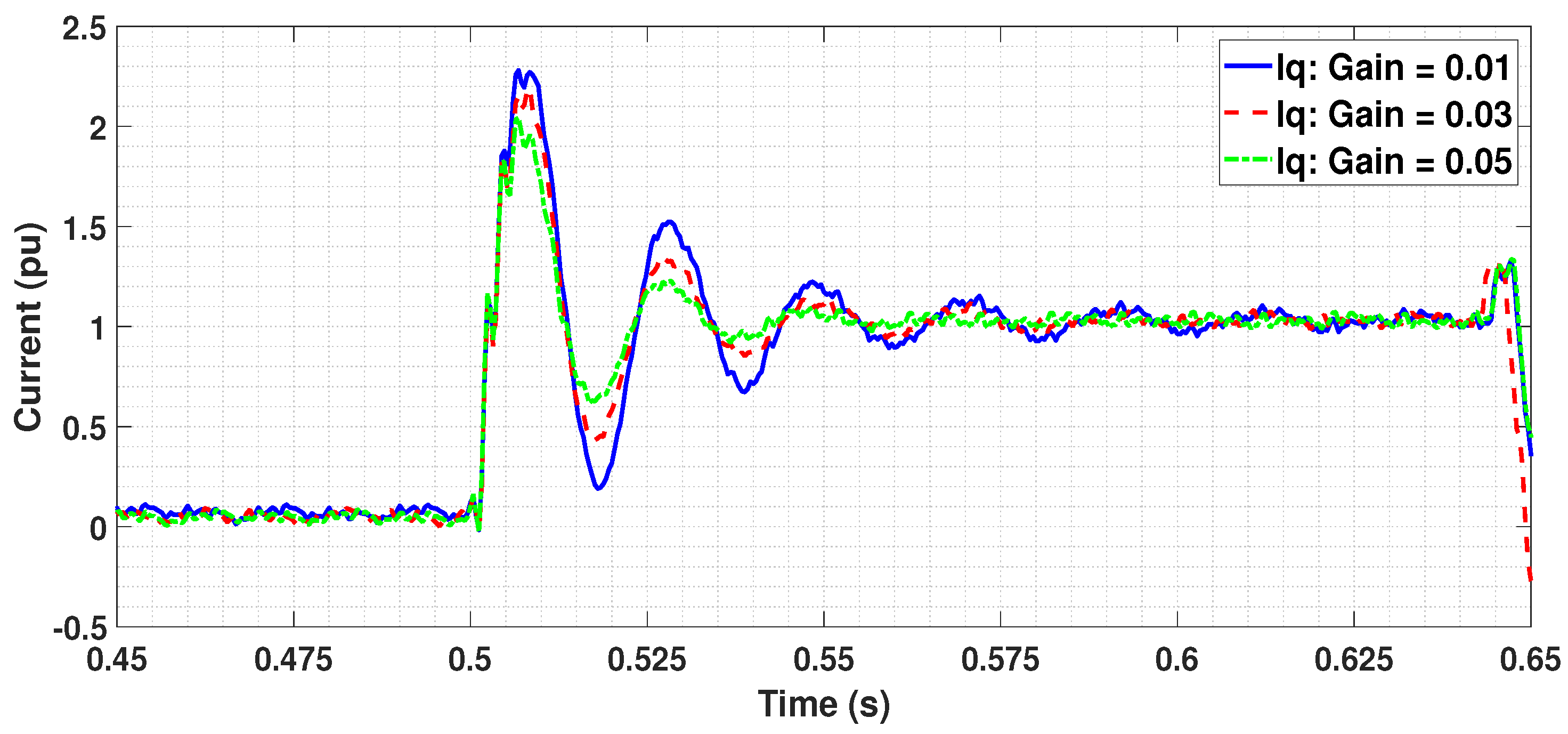
4.1.1. Parameter Selection for Washout Filters
5. Overview of the Digital Twin Model in PowerFactory
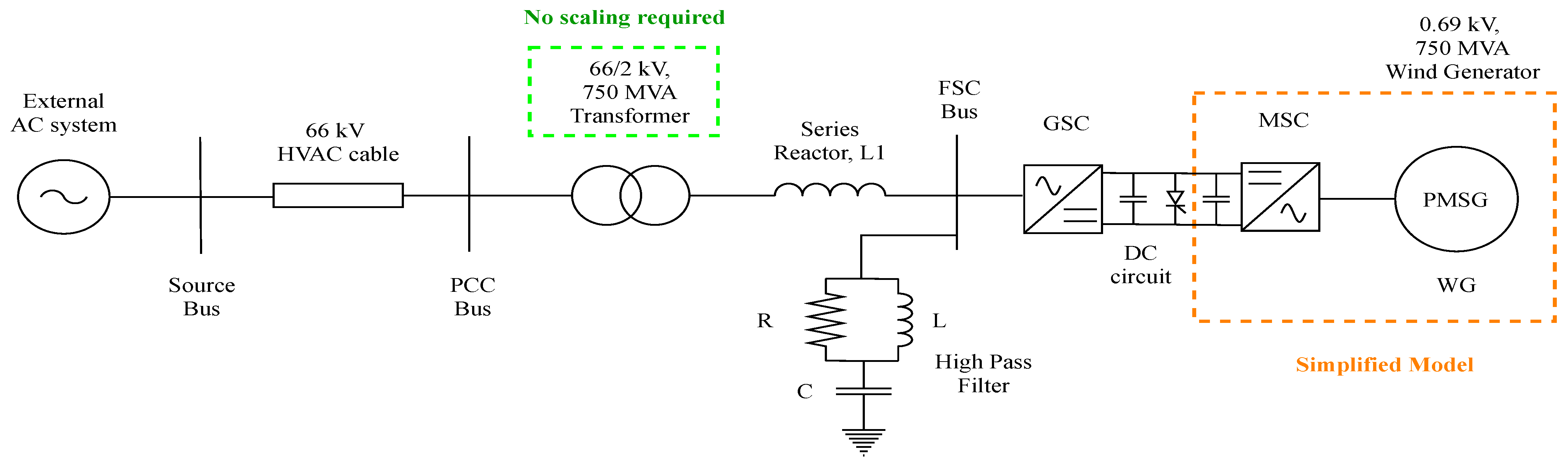
- A simplified model of Full Scale Converter (FSC) based Type-4 WG system consisting of the following elements:
- –
- DC circuit
- –
- GSC
- HPF with series reactor
- OWF transformer
- HVAC cables
- External AC system
5.1. Simplified FSC Based WG System
5.1.1. DC Circuit
5.1.2. GSC
5.2. HPF with Series Reactor
5.3. OWF Transformer
5.4. HVAC Cables
5.5. External AC System
5.6. Control Structures
6. Comparison of Models in RSCAD and PowerFactory
6.1. Selection of Time Step in RSCAD and PowerFactory
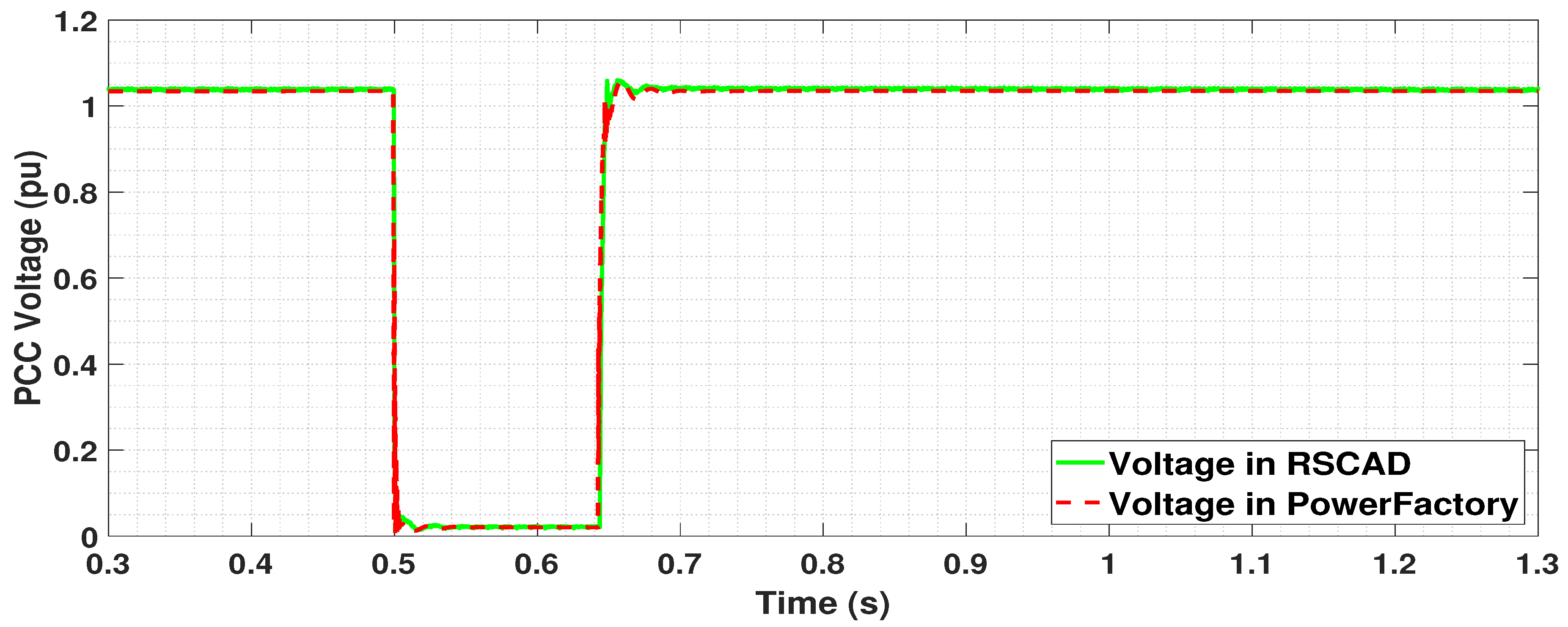
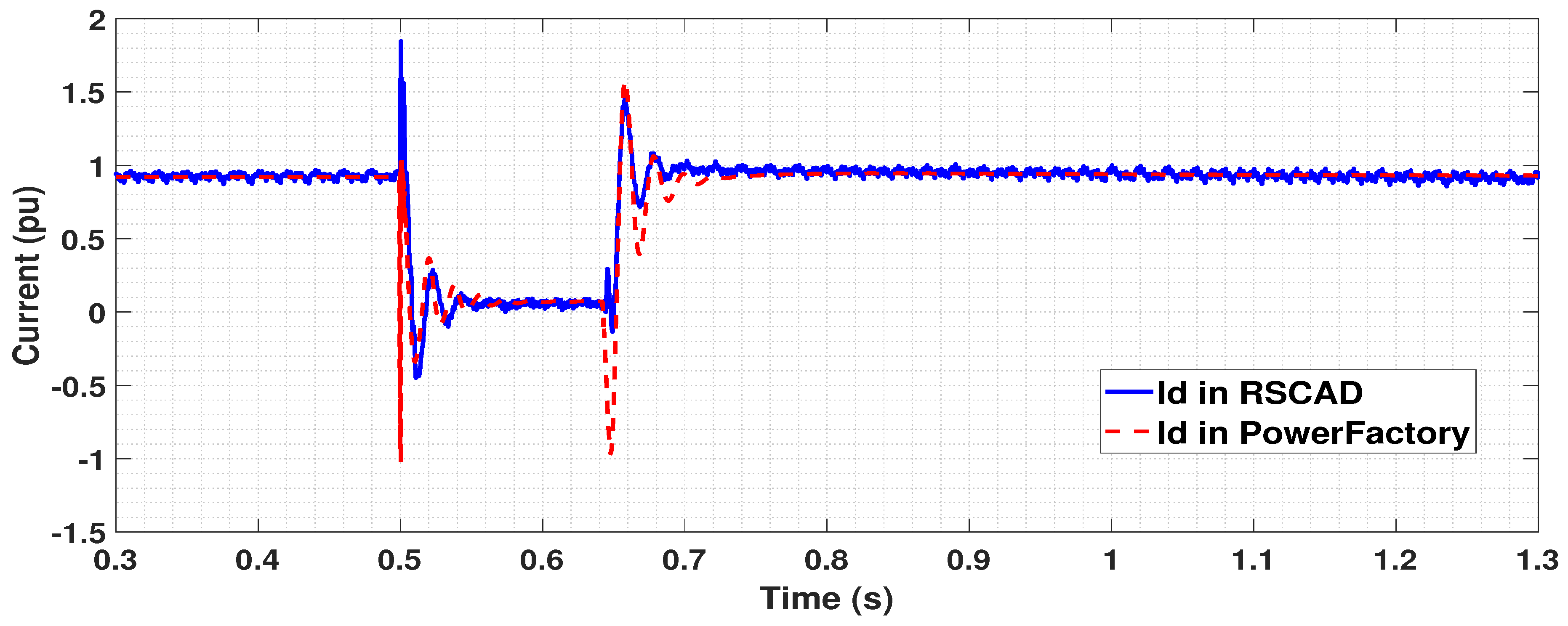
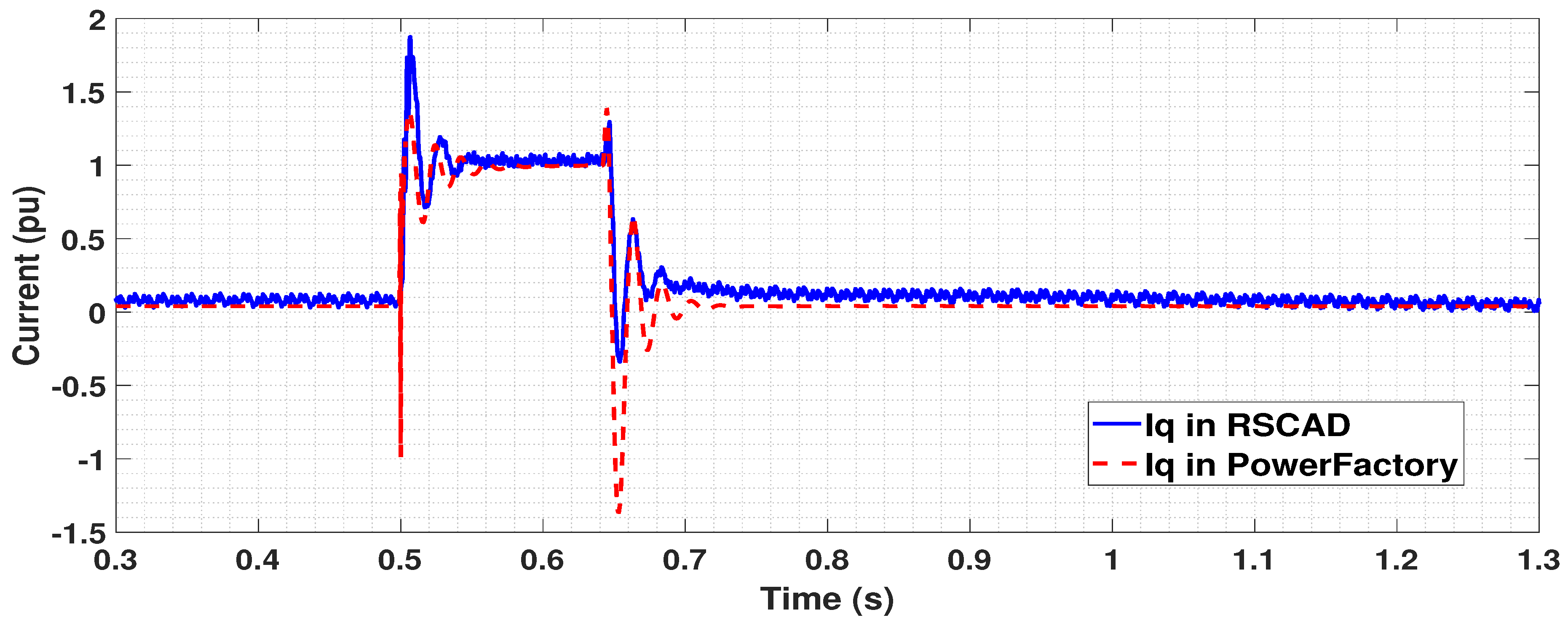
6.2. Event Comparison in RSCAD and PowerFactory
7. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Parameters | Description | Unit | Value |
|---|---|---|---|
| Static gain for slow global reactive power control | p.u. | 6.6 | |
| Maximum/minimum reactive power | p.u. | 0.31 | |
| Slow global reactive power control proportional gain | p.u. | 0.002 | |
| Slow global reactive power control integral time constant | s | 15 | |
| Fast local voltage control proportional gain | p.u. | 0.2 | |
| x | Converter reactance | p.u. | 0.1 |
| Washout filter proportional gain | p.u. | 0.05 | |
| Washout filter time constant | s | 0.01 | |
| Maximum q-axis converter control voltage |
| Parameters | Description | Unit | Value |
|---|---|---|---|
| DC voltage control proportional gain | p.u. | 1 | |
| DC voltage control integral time constant | p.u. | 0.1 | |
| Proportional gain for direct frequency control | p.u. | 1 | |
| First order delay for direct frequency control | s | 0.2 | |
| Washout time constant for the voltage dependent active power reduction | s | 60 | |
| Proportional gain for voltage dependent active power reduction | p.u. | 2 | |
| First order delay for the voltage dependent active power reduction | s | 0.005 | |
| x | Converter reactance | p.u. | 0.1 |
| Washout filter proportional gain | p.u. | 0.05 | |
| Washout filter time constant | s | 0.01 | |
| Voltage measurement delay | s | 5 | |
| Deadband for direct frequency control | Hz | 0.2 | |
| Deadband for voltage dependent active power reduction | p.u. | 0.1 | |
| Maximum d-axis converter control voltage |
| Parameters | Description | Unit | Value |
|---|---|---|---|
| Gain for current limitation | p.u. | 1.2 |
References
- UNFCCC. Adoption of the Paris agreement. COP. In Twenty-First Session of Conference of the Parties, Paris, France, 30 November–11 December 2015; UNFCCC: Rio de Janeiro, Brazil, 2015; Volume 30. [Google Scholar]
- Ecofys. Translate COP21: 2045 Outlook and Implications for Offshore Wind in the North Seas; Technical Report; Ecofys: Utrecht, The Netherlands, 2017. [Google Scholar]
- Weise, B.; Korai, A.; Constantin, A. Comparison of Selected Grid-Forming Converter Control Strategies for Use in Power Electronic Dominated Power Systems. In Proceedings of the 18th Wind Integration Workshop, Dublin, Ireland, 16–18 October 2019; pp. 16–18. [Google Scholar]
- Markovic, U.; Chu, Z.; Aristidou, P.; Hug, G. LQR-Based Adaptive Virtual Synchronous Machine for Power Systems with High Inverter Penetration. IEEE Trans. Sustain. Energy 2018, 10, 1501–1512. [Google Scholar] [CrossRef]
- Duckwitz, D.; Welck, F.; Glöckler, C. Operational Behavior of the Virtual Synchronous Machine. In Proceedings of the Energiewende in der Stromversorgung—Systemstabilität und Systemsicherheit—12. ETG/GMA-Tagung “Netzregelung und Systemführung”, Berlin, Germany, 26–27 September 2017. [Google Scholar]
- Lu, L.; Cutululis, N.A. Virtual synchronous machine control for wind turbines: A review. J. Phys. Conf. Ser. 2019, 1356, 012028. [Google Scholar] [CrossRef]
- Bouzid, A.E.M.; Sicard, P.; Yamane, A.; Paquin, J.N. Simulation of droop control strategy for parallel inverters in autonomous AC microgrids. In Proceedings of the 2016 8th International Conference on Modelling, Identification and Control (ICMIC), Algiers, Algeria, 15–17 November 2016; pp. 701–706. [Google Scholar]
- Yu, M.; Roscoe, A.J.; Booth, C.D.; Dysko, A.; Ierna, R.; Zhu, J.; Urdal, H. Use of an inertia-less Virtual Synchronous Machine within future power networks with high penetrations of converters. In Proceedings of the 2016 Power Systems Computation Conference (PSCC), Genoa, Italy, 20–24 June 2016; pp. 1–7. [Google Scholar]
- Korai, A.W. Dynamic Performance of Electrical Power Systems with High Penetration of Power Electronic Converters: Analysis and New Control Methods for Mitigation of Instability Threats and Restoration. Ph.D. Thesis, Universität Duisburg-Essen, Duisburg, Germany, 2019. [Google Scholar] [CrossRef]
- Erlich, I.; Korai, A.; Neumann, T.; Koochack Zadeh, M.; Vogt, S.; Buchhagen, C.; Rauscher, C.; Menze, A.; Jung, J. New Control of Wind Turbines Ensuring Stable and Secure Operation Following Islanding of Wind Farms. IEEE Trans. Energy Convers. 2017, 32, 1263–1271. [Google Scholar] [CrossRef]
- Duckwitz, D. Derivation of Requirements and Comparison of Inertia Emulation Methods for Converter-based Power PlantsPower System Inertia. Ph.D. Thesis, Universität Kassel, Kassel, Germany, 2019. [Google Scholar] [CrossRef]
- Liu, X.; Lindemann, A. Control of VSC-HVDC Connected Offshore Windfarms for Providing Synthetic Inertia. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 6, 1407–1417. [Google Scholar] [CrossRef]
- Abdelwahed, M.A.; El-Saadany, E.F. Power Sharing Control Strategy of Multiterminal VSC-HVDC Transmission Systems Utilizing Adaptive Voltage Droop. IEEE Trans. Sustain. Energy 2016, 8, 605–615. [Google Scholar] [CrossRef]
- DNV.GL. 66 kV Systems for Offshore Wind Farms; Technical Brochure; DNV.GL: Arnhem, The Netherlands, 2015. [Google Scholar]
- Lozada Ayala, P. Dynamic System Performance Analysis of a Novel Grid Connection Topology for Offshore Wind Farms Using MMC-HVDC Transmission. 2018. Available online: https://repository.tudelft.nl/islandora/object/uuid%3Abfd0ac94-fc5b-46e4-a049-742c5249138a (accessed on 27 December 2020).
- RTDS Technologies Inc. RSCAD Modules. Available online: https://knowledge.rtds.com/hc/en-us/articles/360037537653-RSCAD-Modules (accessed on 27 December 2020).
- The MIGRATE Project, A EU-Funded Project Under the Framework of European Union’s HORIZON 2020. 2020. Available online: https://www.h2020-migrate.eu/#:~:text=The%20MIGRATE%20Project&text=MIGRATE%20stands%20for%20Massive%20InteGRATion,especially%20in%20future%20faced%20with. (accessed on 27 December 2020).
- Sethi, S. Real-Time Implementation for Gird Forming Control of Type-4 Wind Turbine to Mitigate Voltage and Frequency Instabilities in High Renewable Penetration. Master’s Thesis, NTNU, Trondheim, Norway, 2019. [Google Scholar]
- Beres, R.N.; Wang, X.; Liserre, M.; Blaabjerg, F.; Bak, C.L. A Review of Passive Power Filters for Three-Phase Grid-Connected Voltage-Source Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 54–69. [Google Scholar] [CrossRef]
- Kothari, D.P.; Nagrath, I. Modern Power System Analysis; Tata McGraw-Hill Education: New Delhi, India, 2003. [Google Scholar]
- Guide for the Development of Models for HVDC Converters in a HVDC Grid. Technical Brochure, CIGRE Working Group B4.57. 2014. Available online: https://e-cigre.org/publication/604-guide-for-the-development-of-models-for-hvdc-converters-in-a-hvdc-grid (accessed on 27 December 2020).
- Erlich, I.; Korai, A. Description, Modelling and Simulation of a Benchmark System for Converter Dominated Grids (Part I). 2018. Available online: https://www.digsilent.de/en/faq-reader-powerfactory/do-you-have-an-example-of-a-fault-tolerant-power-system-with-98-share-of-renewables.html (accessed on 27 December 2020).
- Mohseni, M.; Islam, S.M. Review of international grid codes for wind power integration: Diversity, technology and a case for global standard. Renew. Sustain. Energy Rev. 2012, 16, 3876–3890. [Google Scholar] [CrossRef]


| Parameters | RSCAD | PowerFactory |
|---|---|---|
| WG model | PMSG | Simplified (Constant power model) |
| MSC control | Conventional current control | Not modelled |
| GSC model | VSC with PWM | Controlled Voltage Source with no PWM |
| Generated active power from WG | 6 MW | ∼700 MW |
| Scaling factor at transformer | 116 (cf. Section. Section 2.1.3) | Not applicable |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ganesh, S.; Perilla, A.; Torres, J.R.; Palensky, P.; van der Meijden, M. Validation of EMT Digital Twin Models for Dynamic Voltage Performance Assessment of 66 kV Offshore Transmission Network. Appl. Sci. 2021, 11, 244. https://doi.org/10.3390/app11010244
Ganesh S, Perilla A, Torres JR, Palensky P, van der Meijden M. Validation of EMT Digital Twin Models for Dynamic Voltage Performance Assessment of 66 kV Offshore Transmission Network. Applied Sciences. 2021; 11(1):244. https://doi.org/10.3390/app11010244
Chicago/Turabian StyleGanesh, Saran, Arcadio Perilla, Jose Rueda Torres, Peter Palensky, and Mart van der Meijden. 2021. "Validation of EMT Digital Twin Models for Dynamic Voltage Performance Assessment of 66 kV Offshore Transmission Network" Applied Sciences 11, no. 1: 244. https://doi.org/10.3390/app11010244
APA StyleGanesh, S., Perilla, A., Torres, J. R., Palensky, P., & van der Meijden, M. (2021). Validation of EMT Digital Twin Models for Dynamic Voltage Performance Assessment of 66 kV Offshore Transmission Network. Applied Sciences, 11(1), 244. https://doi.org/10.3390/app11010244








