Optimization of a T-Shaped MIMO Antenna for Reduction of EMI
Abstract
Featured Application
Abstract
1. Introduction
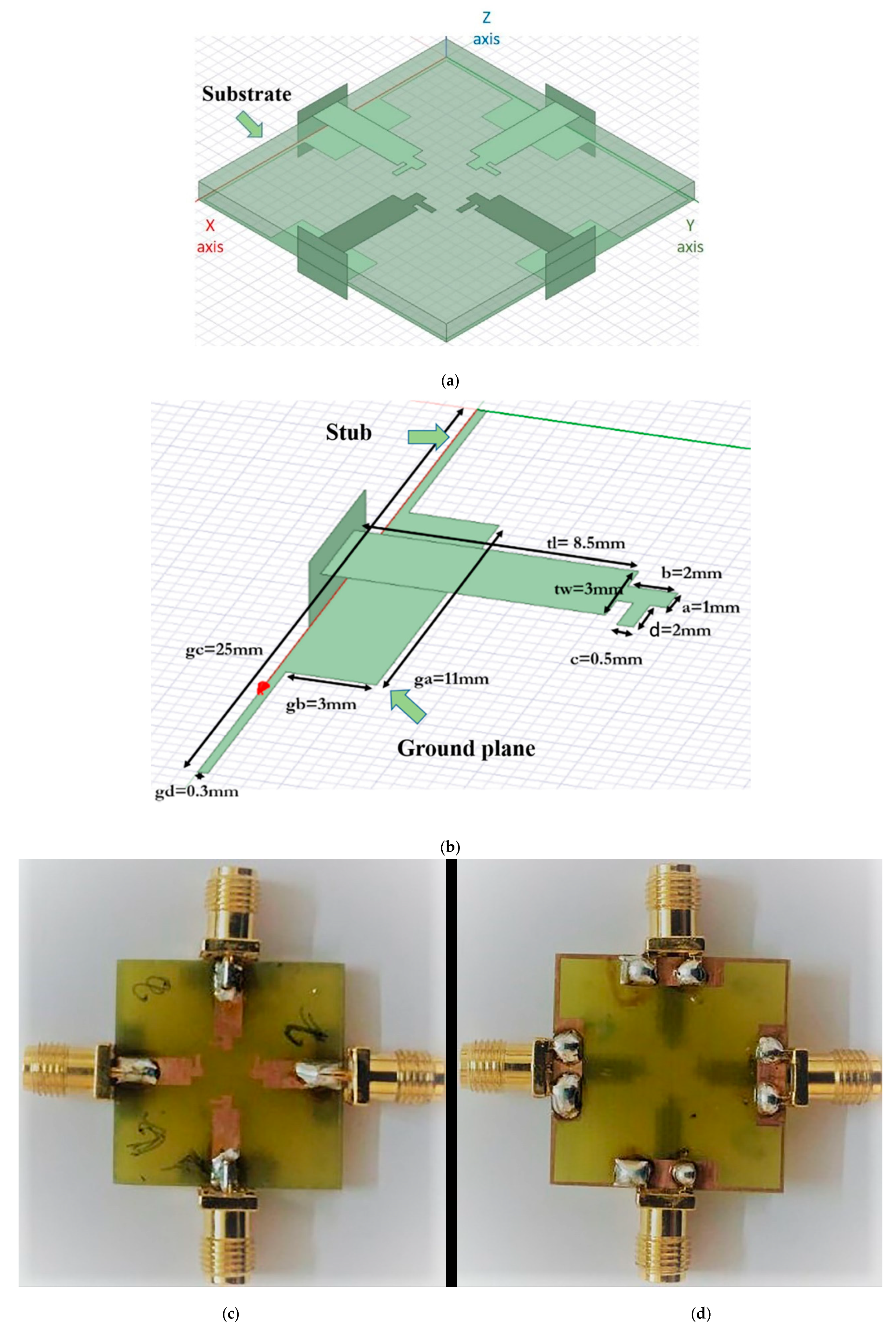
2. Antenna Design and Fabrication
3. 2K Factorial
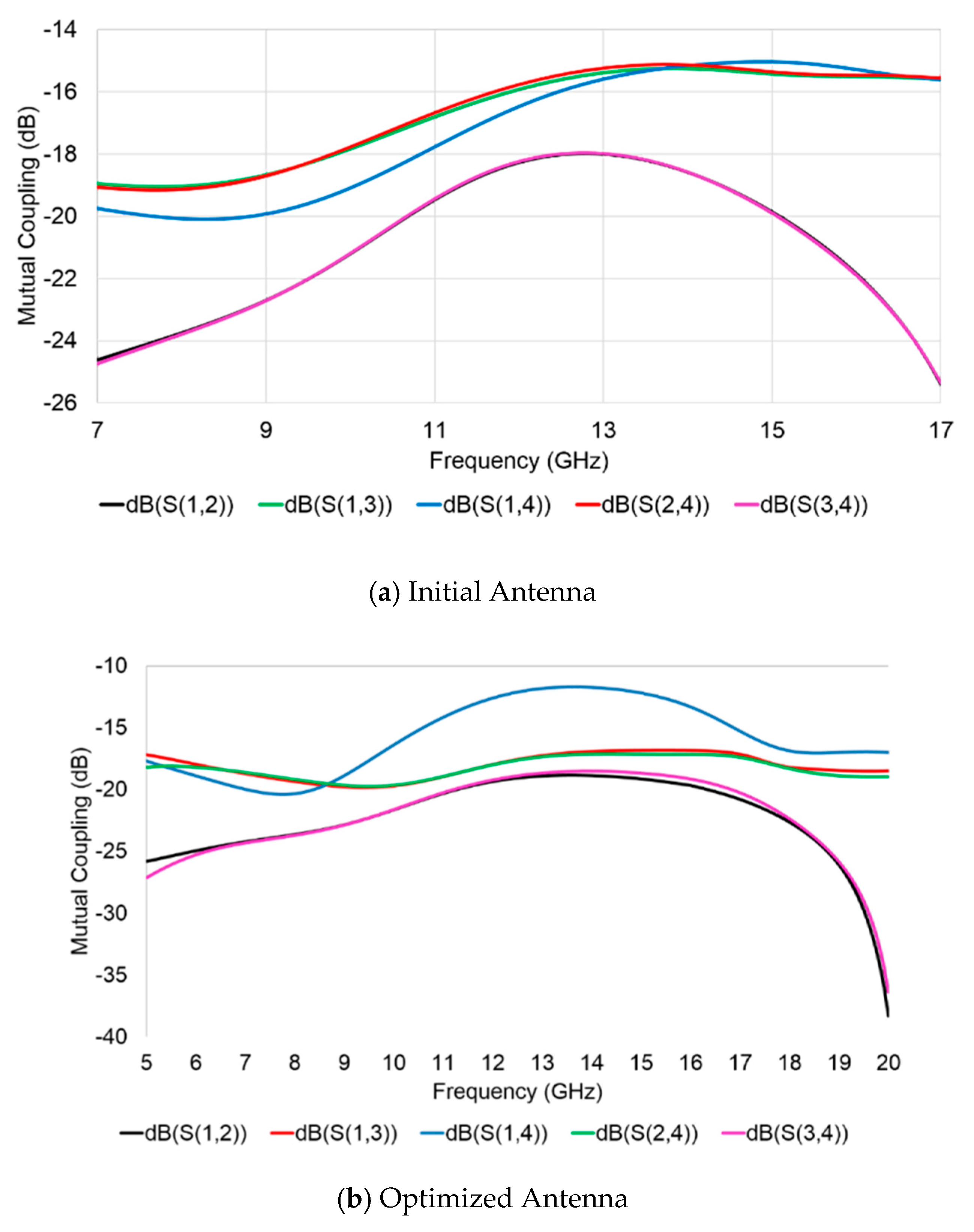
4. Optimization Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kragalott, M.; Kluskens, M.S.; Zolnick, D.A.; Dorsey, W.M.; Valenzi, J.A. A toolset independent hybrid method for calculating antenna coupling. IEEE Trans. Antennas Propag. 2011, 59, 443–451. [Google Scholar] [CrossRef]
- Saxena, S.; Kanaujia, B.K.; Dwari, S.; Kumar, S.; Tiwari, R. A Compact Dual-Polarized MIMO Antenna with Distinct Diversity Performance for UWB Applications. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 3096–3099. [Google Scholar] [CrossRef]
- Rajkumar, S.; Vivek Sivaraman, N.; Murali, S.; Selvan, K.T. Heptaband swastik arm antenna for MIMO applications. IET Microw. Antennas Propag. 2017, 11, 1255–1261. [Google Scholar] [CrossRef]
- Roshna, T.K.; Deepak, U.; Sajitha, V.R.; Vasudevan, K.; Mohanan, P. A compact UWB MIMO antenna with reflector to enhance isolation. IEEE Trans. Antennas Propag. 2015, 63, 1873–1877. [Google Scholar] [CrossRef]
- Krishna, R.V.S.R.; Kumar, R. A Dual-Polarized Square-Ring Slot Antenna for UWB, Imaging, and Radar Applications. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 195–198. [Google Scholar] [CrossRef]
- Jafri, S.I.; Saleem, R.; Shafique, M.F.; Brown, A.K. Compact reconfigurable multiple-input-multiple-output antenna for ultra wideband applications. IET Microw. Antennas Propag. 2016, 10, 413–419. [Google Scholar] [CrossRef]
- Khan, A.A.; Jamaluddin, M.H.; Aqeel, S.; Nasir, J.; Kazim, J.U.R.; Owais, O. Dual-band MIMO dielectric resonator antenna for WiMAX/WLAN applications. IET Microw. Antennas Propag. 2017, 11, 113–120. [Google Scholar] [CrossRef]
- Iqbal, A.; Saraereh, O.A.; Ahmad, A.W.; Bashir, S. Mutual Coupling Reduction Using F-Shaped Stubs in UWB-MIMO Antenna. IEEE Access 2017, 6, 2755–2759. [Google Scholar] [CrossRef]
- Tian, R.; Lau, B.K.; Ying, Z. Multiplexing efficiency of MIMO antennas. IEEE Antennas Wirel. Propag. Lett. 2011, 10, 183–186. [Google Scholar] [CrossRef]
- Liu, X.; Bialkowski, M.E. Effect of antenna mutual coupling on MIMO channel estimation and capacity. Int. J. Antennas Propag. 2010, 2010, 1–9. [Google Scholar] [CrossRef]
- Abdul Haleem, M. On the capacity and transmission techniques of massive MIMO Systems. Wirel. Commun. Mob. Comput. 2018, 2018, 1–9. [Google Scholar] [CrossRef]
- Khalighi, M.A.; Raoof, K.; Jourdain, G. Capacity of wireless communication systems employing antenna arrays, a tutorial study. Wirel. Pers. Commun. 2002, 23, 321–352. [Google Scholar] [CrossRef]
- Du, J.; Li, Y. Optimization of antenna configuration for MIMO systems. IEEE Trans. Commun. 2005, 53, 1451–1454. [Google Scholar] [CrossRef]
- Kiehbadroudinezhad, S.; Noordin, N.K.; Sali, A.; Abidin, Z.Z. Optimization of an antenna array using genetic algorithms. Astron. J. 2014, 147, 1–13. [Google Scholar] [CrossRef]
- Telzhensky, N.; Leviatan, Y. Novel method of UWB antenna optimization for specified input signal forms by means of genetic algorithm. IEEE Trans. Antennas Propag. 2006, 54, 2216–2225. [Google Scholar] [CrossRef]
- Binitha, S.; Siva Sathya, S. A survey of bio inspired optimization algorithms. Int. J. Soft Comput. Eng. 2012, 2, 137–151. [Google Scholar]
- Hussein, A.H.; Abdullah, H.H.; Salem, A.M.; Khamis, S.; Nasr, M. Optimum design of linear antenna arrays using a hybrid MoM/GA algorithm. IEEE Antennas Wirel. Propag. Lett. 2011, 10, 1232–1235. [Google Scholar] [CrossRef]
- Zhou, D.; Abd-Alhameed, R.A.; See, C.H.; Bin-Melha, M.S.; Zainal-Abdin, Z.B.; Excell, P.S. New antenna designs for wideband harmonic suppression using adaptive surface meshing and genetic algorithms. IET Microw. Antennas Propag. 2011, 5, 1054–1061. [Google Scholar] [CrossRef]
- Robinson, J.; Rahmat-Samii, Y. Particle swarm optimization in electromagnetics. IEEE Trans. Antennas Propag. 2004, 52, 397–407. [Google Scholar] [CrossRef]
- Mohammed, H.J.; Abdullah, A.S.; Ali, R.S.; Abd-Alhameed, R.A.; Abdulraheem, Y.I.; Noras, J.M. Design of a uniplanar printed triple band-rejected ultra-wideband antenna using particle swarm optimisation and the firefly algorithm. IET Microw. Antennas Propag. 2016, 10, 31–37. [Google Scholar] [CrossRef]
- Johnson, J.M.; Rahmat-Samii, Y. Genetic algorithms in engineering electromagnetics. IEEE Antennas Propag. Mag. 1997, 39, 7–21. [Google Scholar] [CrossRef]
- Panduro, M.A.; Brizuela, C.A. A comparative analysis of the performance of GA, PSO and DE for circular antenna arrays. In Proceedings of the IEEE Antennas and Propagation Society International Symposium, Charleston, SC, USA, 1–5 June 2009; IEEE: Piscataway, NJ, USA, 2009; pp. 1–4. [Google Scholar]
- Binelo, M.O.; De Almeida, A.L.F.; Cavalcanti, F.R.P. MIMO array capacity optimization using a genetic algorithm. IEEE Trans. Veh. Technol. 2011, 60, 2471–2481. [Google Scholar] [CrossRef]
- Oprime, P.C.; Pureza, V.M.M.; De Oliveira, S.C. Systematic sequencing of factorial experiments as an alternative to the random order. Gest. Prod. 2017, 24, 108–122. [Google Scholar] [CrossRef]
- Cavalcanti, F.R.P. Resource Allocation and MIMO for 4G and Beyond; Springer: New York, NY, USA, 2014. [Google Scholar]
- E Silva Neto, A.S.; de Macedo Dantas, M.L.; dos Santos Silva, J.; César Chaves Fernandes, H. Antenna for fifth generation (5G) using a EBG structure. In New Contributions in Information Systems and Technologies; Springer: Cham, Switzerland, 2015; pp. 33–38. [Google Scholar]
- Hoang, T.V.; Le, T.T.; Li, Q.Y.; Park, H.C. Quad-Band Circularly Polarized Antenna for 2.4/5.3/5.8-GHz WLAN and 3.5-GHz WiMAX Applications. IEEE Antennas Propag. Lett. 2016, 15, 1032–1035. [Google Scholar] [CrossRef]
- Liu, X.L.; Wang, Z.D.; Yin, Y.Z.; Ren, J.; Wu, J.J. A compact ultrawideband MIMO antenna using QSCA for high isolation. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 1497–1500. [Google Scholar] [CrossRef]
- Tang, T.C.; Lin, K.H. An ultrawideband MIMO antenna with dual band-notched function. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 1076–1079. [Google Scholar] [CrossRef]
- Kishk, A. Advancement in Microstrip Antennas with Recent Applications; InTech: Rijeka, Croatia, 2013. [Google Scholar]
- Hilow, H. Minimum cost linear trend free fractional factorial designs. J. Stat. Theory Pract. 2012, 6, 580–589. [Google Scholar] [CrossRef]
- Dahiru, T. P-Value, a true test of statistical significance? A cautionary note. Ann. Ibadan Postgrad. Med. 2008, 6, 21–26. [Google Scholar] [CrossRef]
- Mohammed, H.J.; Abdulsalam, F.; Abdulla, A.S.; Ali, R.S.; Abd-Alhameed, R.A.; Noras, J.M.; Abdulraheem, Y.I.; Ali, A.; Rodriguez, J.; Abdalla, A.M. Evaluation of genetic algorithms, particle swarm optimisation, and firefly algorithms in antenna design. In Proceedings of the 2016 13th International Conference on Synthesis, Modeling, Analysis and Simulation Methods and Applications to Circuit Design, SMACD 2016, Lisbon, Portugal, 27–30 June 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–4. [Google Scholar]
- John, M.; Evans, J.A.; Ammann, M.J.; Modro, J.C.; Chen, Z.N. Reduction of ground-plane-dependent effects on microstrip-fed printed rectangular monopoles. IET Microw. Antennas Propag. 2008, 2, 42–47. [Google Scholar] [CrossRef]

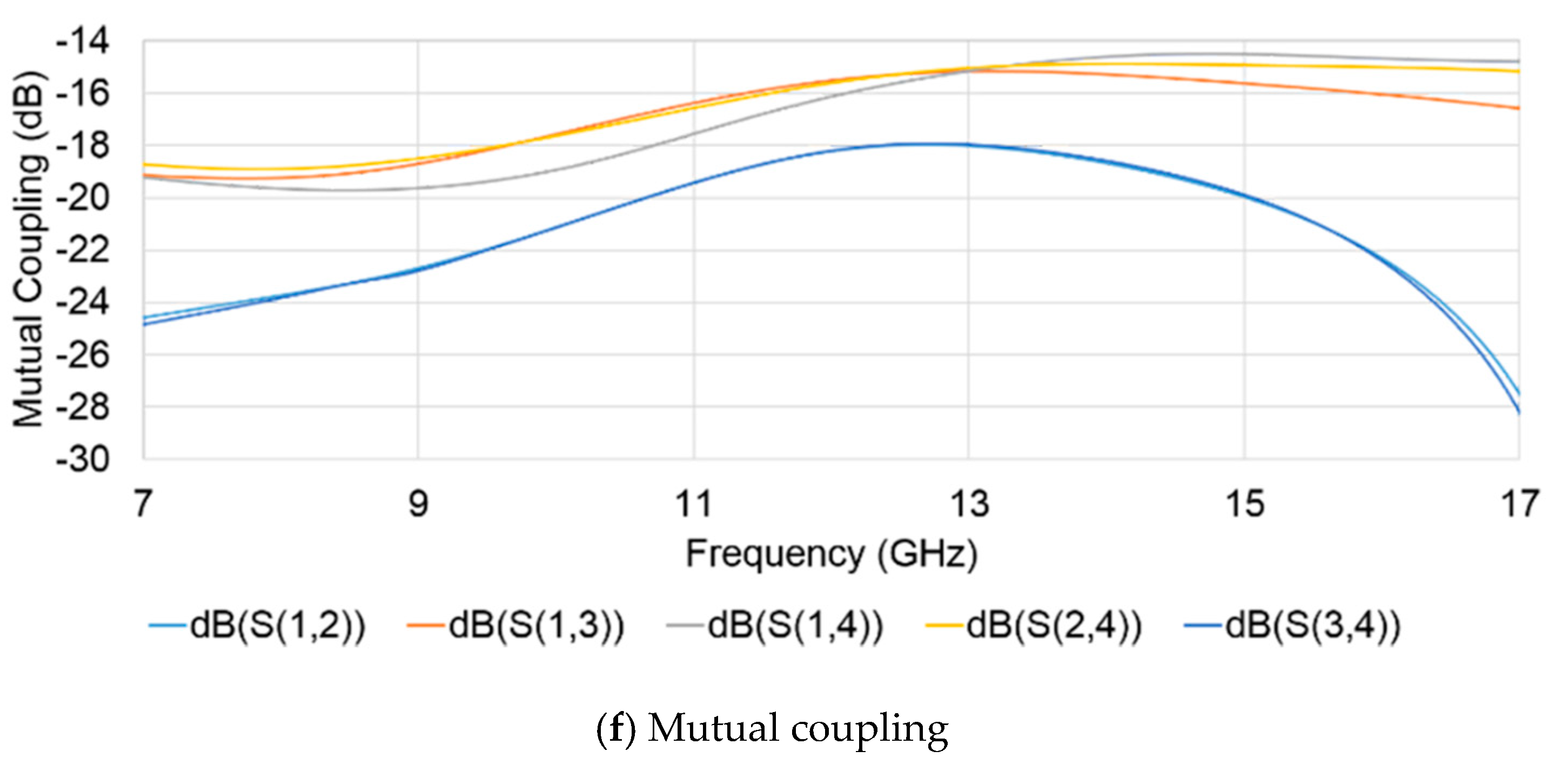
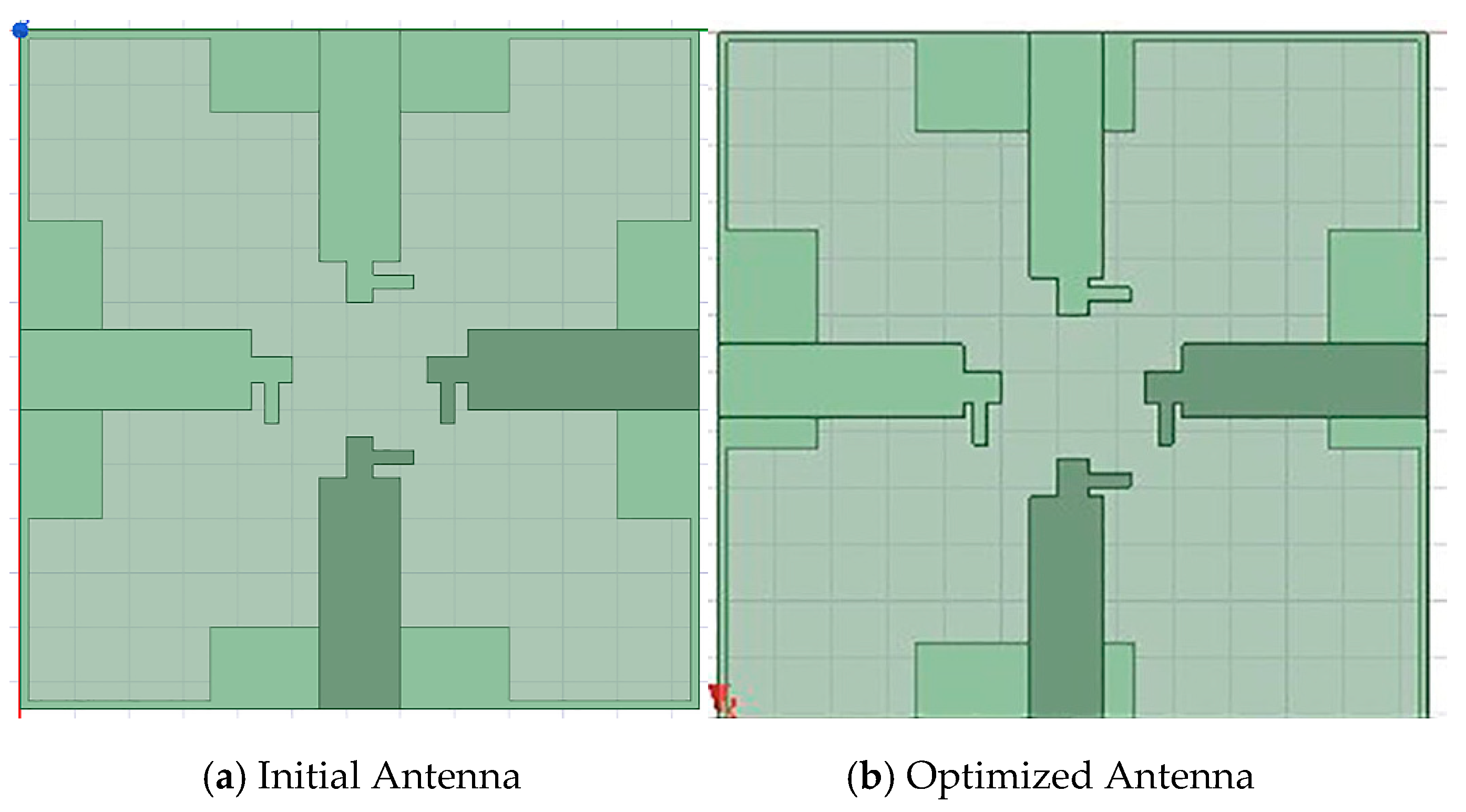
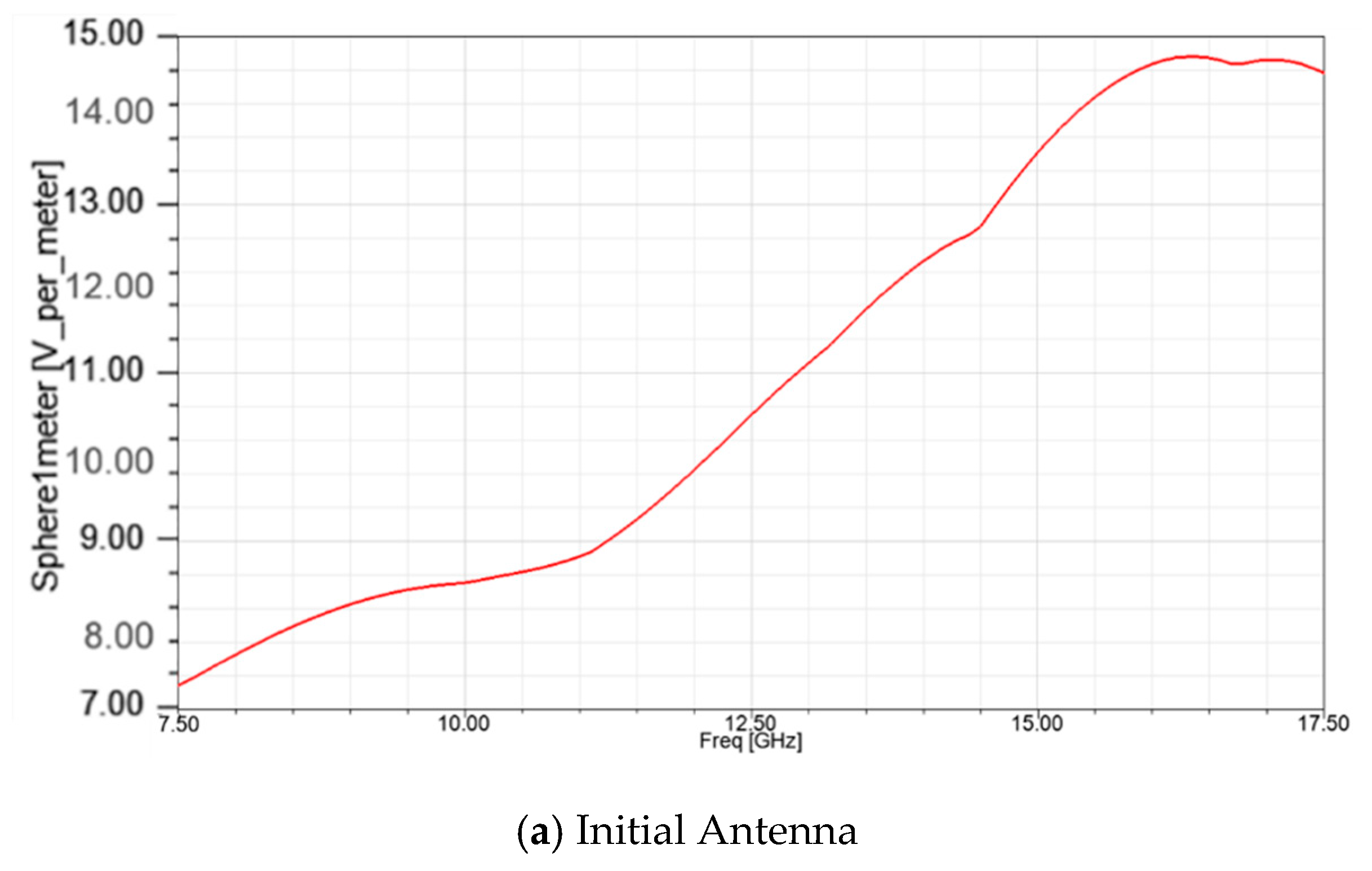
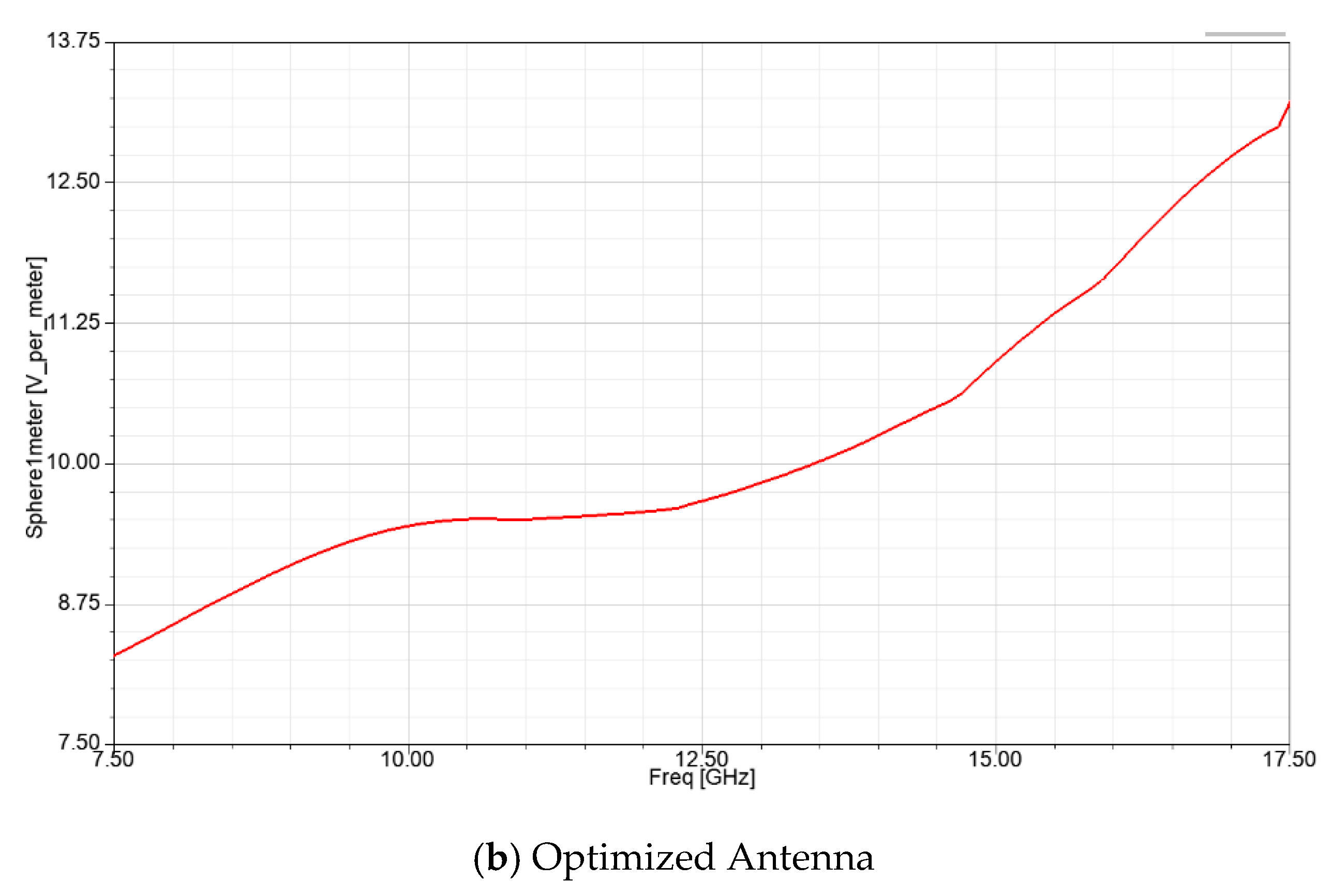

| The Unit for the Parameters Is mm | Return Loss (dB) | Bandwidth (GHz) | Maximum EMI (V/m) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A | b | c | d | tl | tw | ga | gb | gc | gd | |||
| 0.5 | 1 | 0.75 | 0.75 | 12.75 | 4.5 | 16.5 | 1.5 | 12.5 | 0.45 | −41.7 | 8.47 | 15.54 |
| 1.5 | 1 | 0.25 | 2.25 | 12.75 | 4.5 | 5.5 | 1.5 | 37.5 | 0.15 | −34.2 | 8.51 | 15.78 |
| 0.5 | 1 | 0.25 | 0.75 | 4.25 | 1.5 | 5.5 | 1.5 | 37.5 | 0.45 | −12.5 | 5.4 | 16.54 |
| Parameter (mm) | P Value (Return Loss (dB)) | P Value (Bandwidth (GHz)) | P Value (EMI (V/m)) |
|---|---|---|---|
| tl | 0.009 | 0.027 | 0.054 |
| tw | 0.012 | 0.048 | 0.089 |
| ga | 0.043 | 0.079 | 0.081 |
| gb | 0.014 | 0.010 | 0.142 |
| Parameter | Nominal Value as Initial Design (mm) | Optimized Value (mm) |
|---|---|---|
| tw | 3.0 | 2.55 |
| tl | 8.5 | 8.62 |
| ga | 11.0 | 7.62 |
| gb | 3.0 | 3.46 |
| Parameter | Initial Antenna Design | Optimized Antenna Design |
|---|---|---|
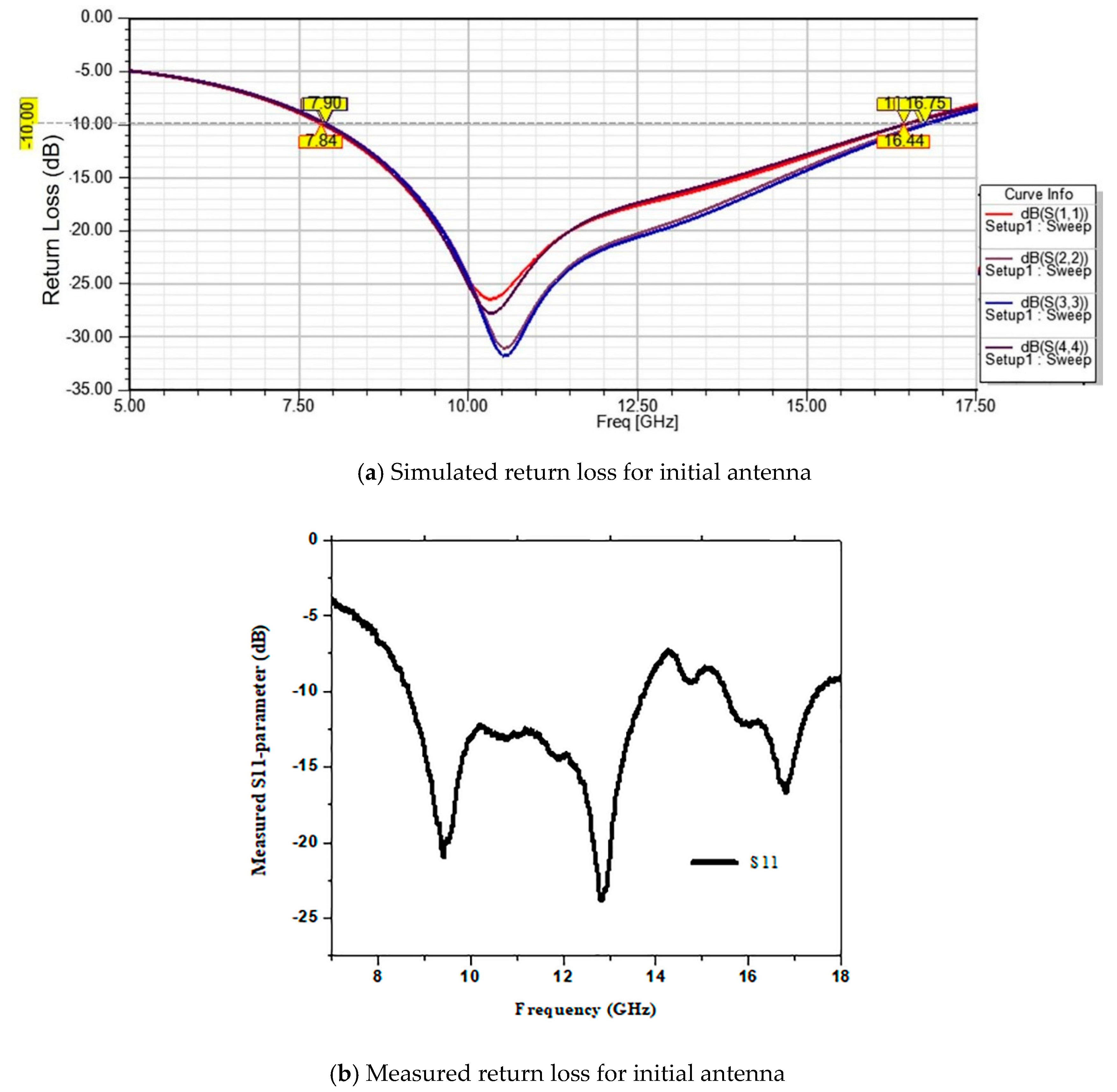
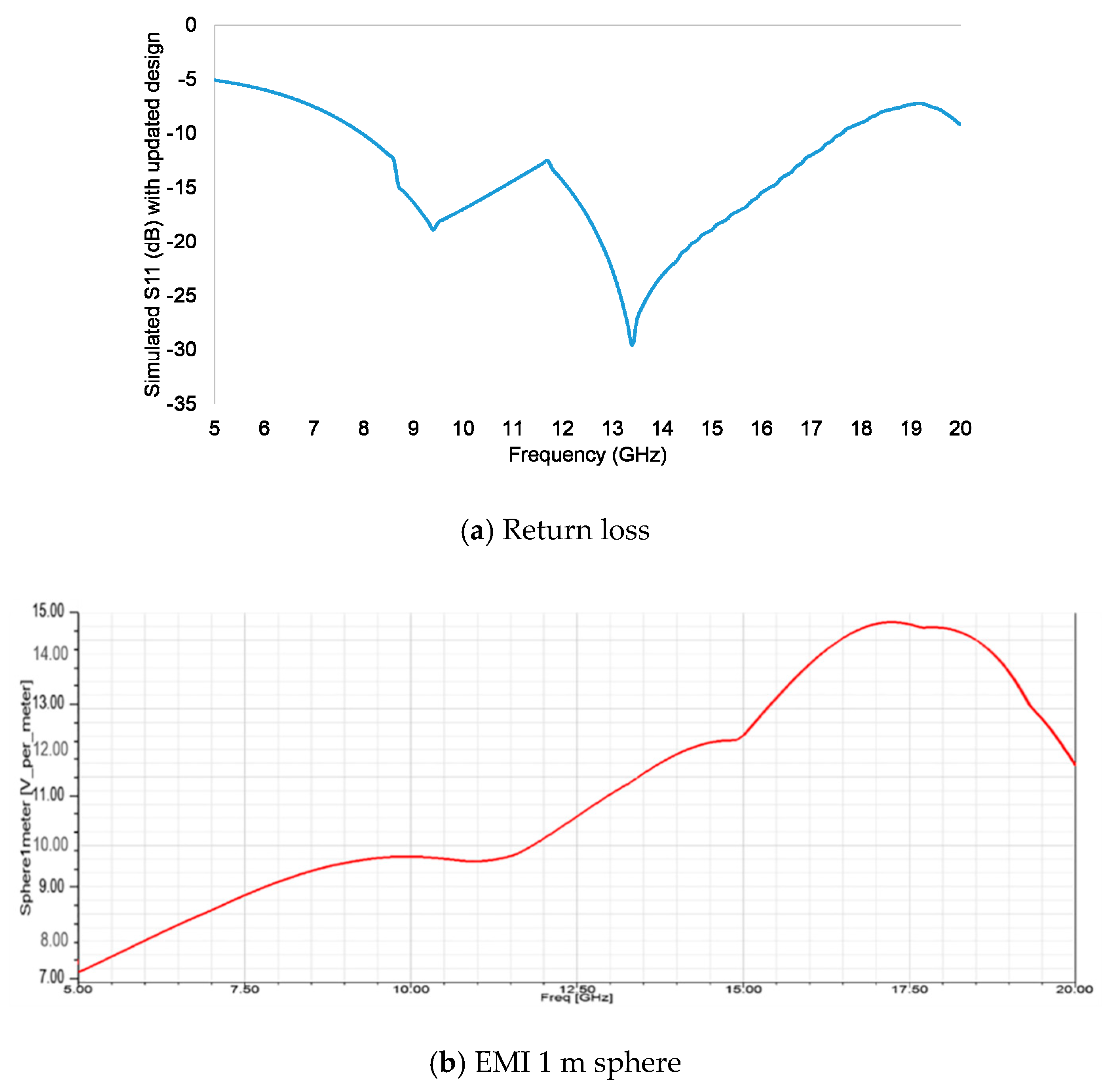
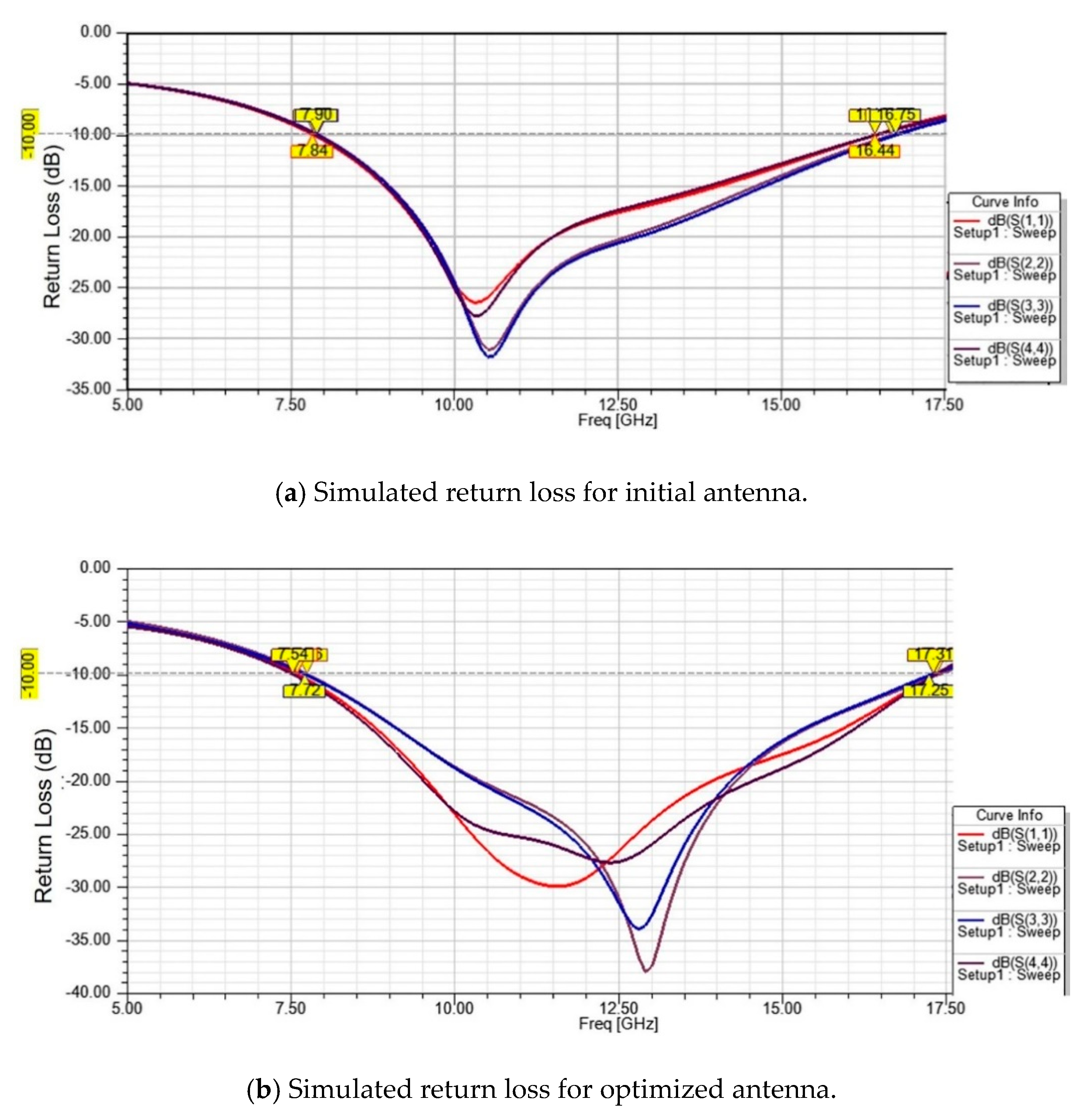
| Bandwidth (GHz) | 7.84–16.44 GHz | 7.72–17.25 GHz |
| Minimum return loss (dB) | −32 | −37 |
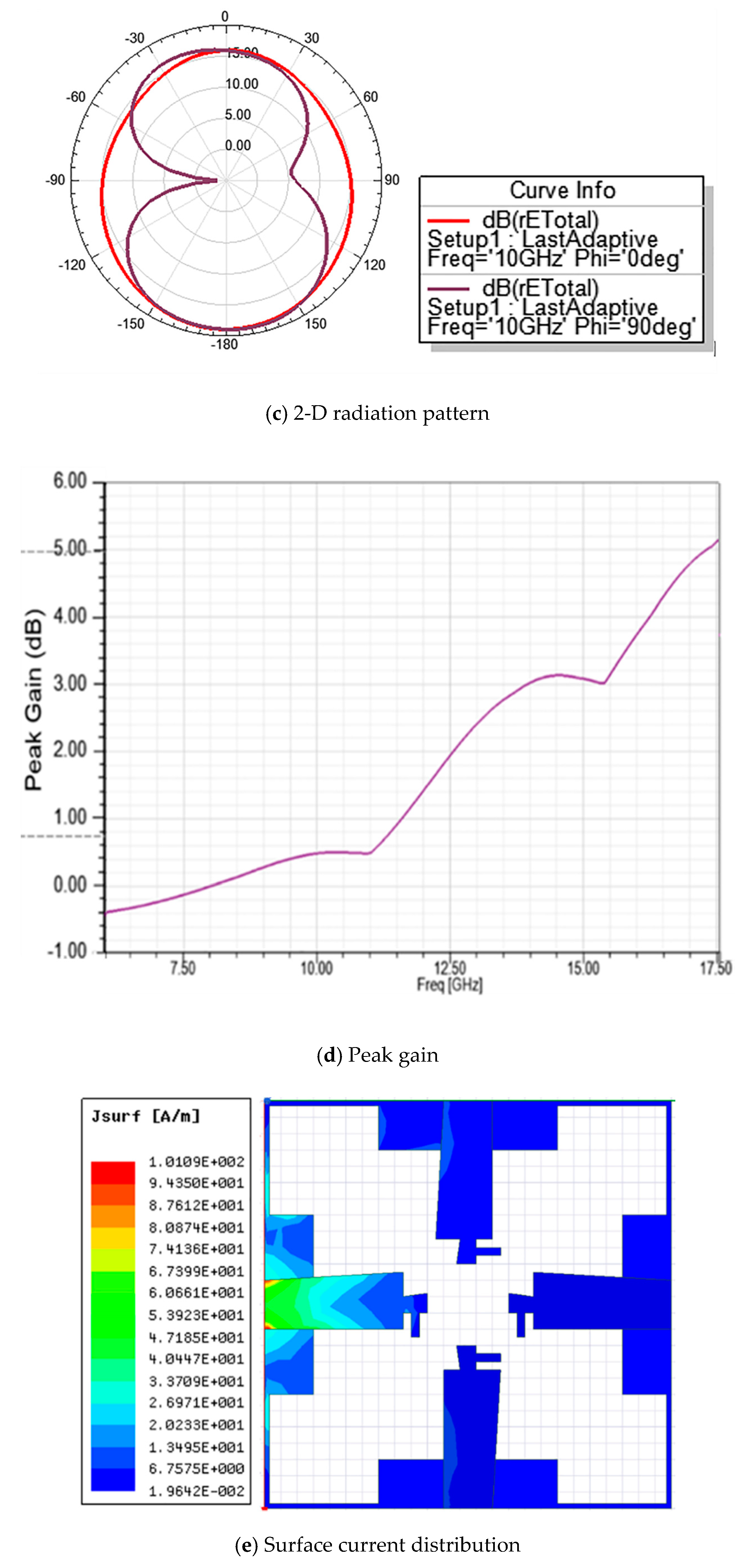
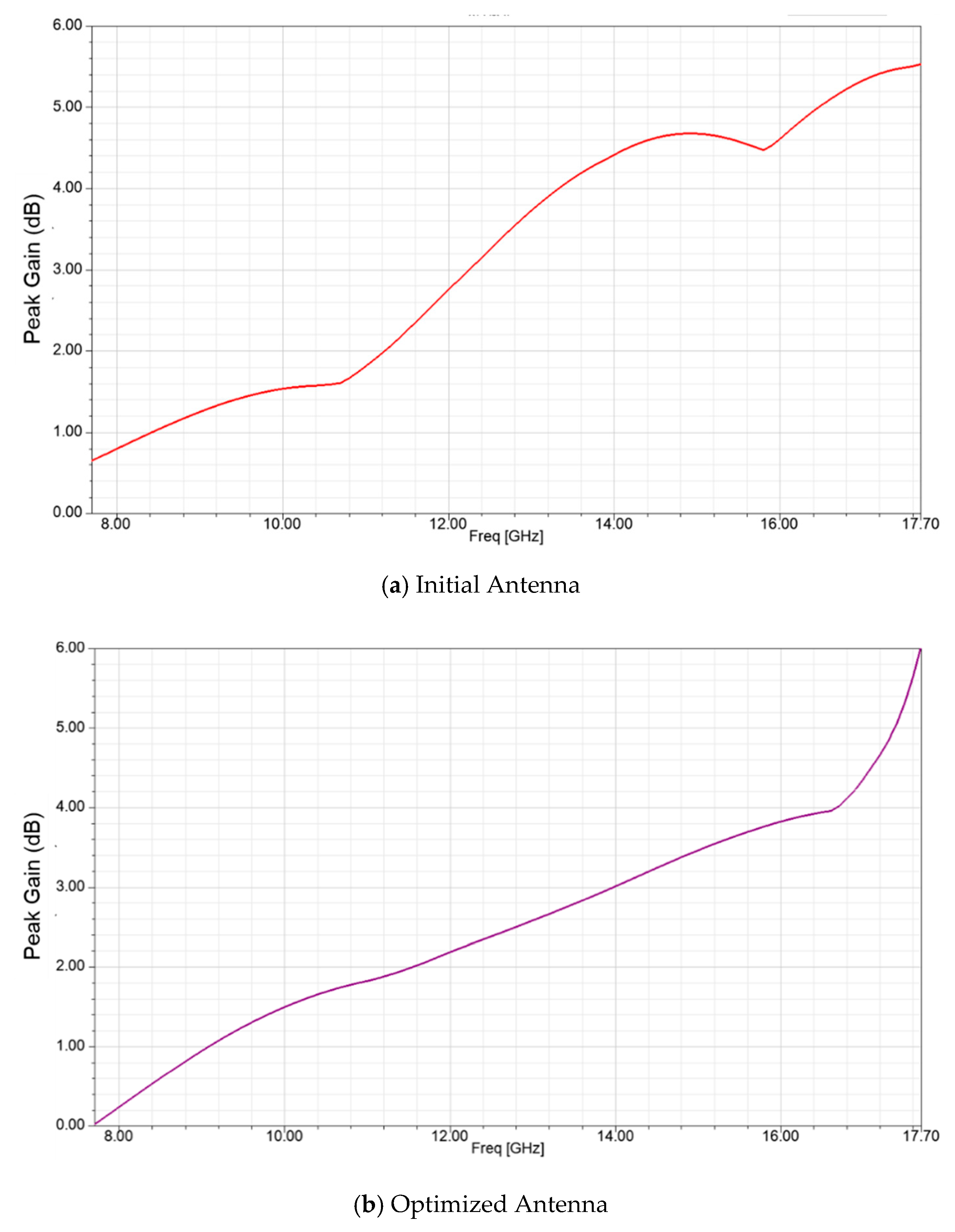
| Maximum peak gain (dB) | 5.55 | 5.99 |
| Maximum surface current density (A/m) | 125.19 | 82.083 |
| Maximum EMI (V/m) | 14.60 | 13.25 |
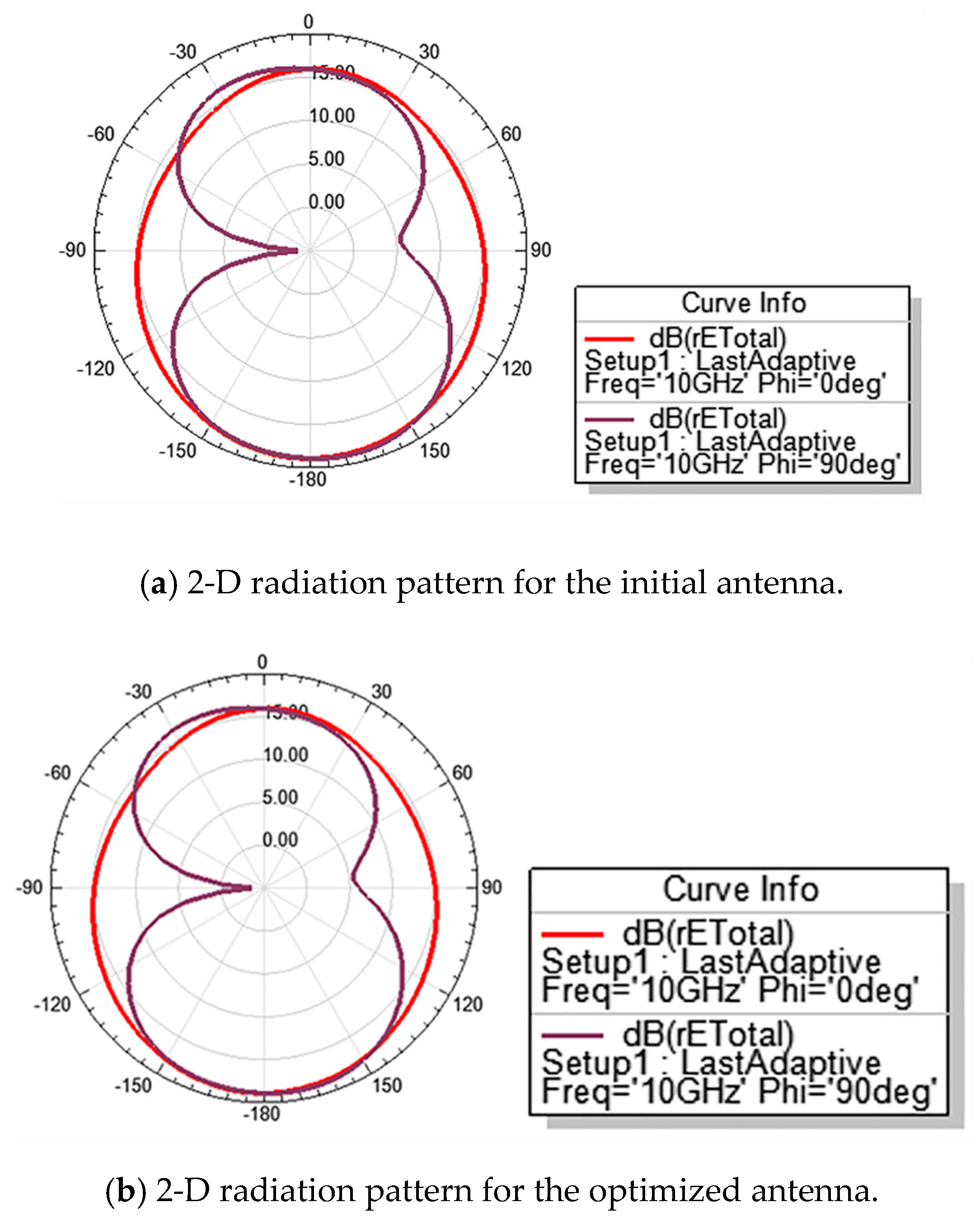
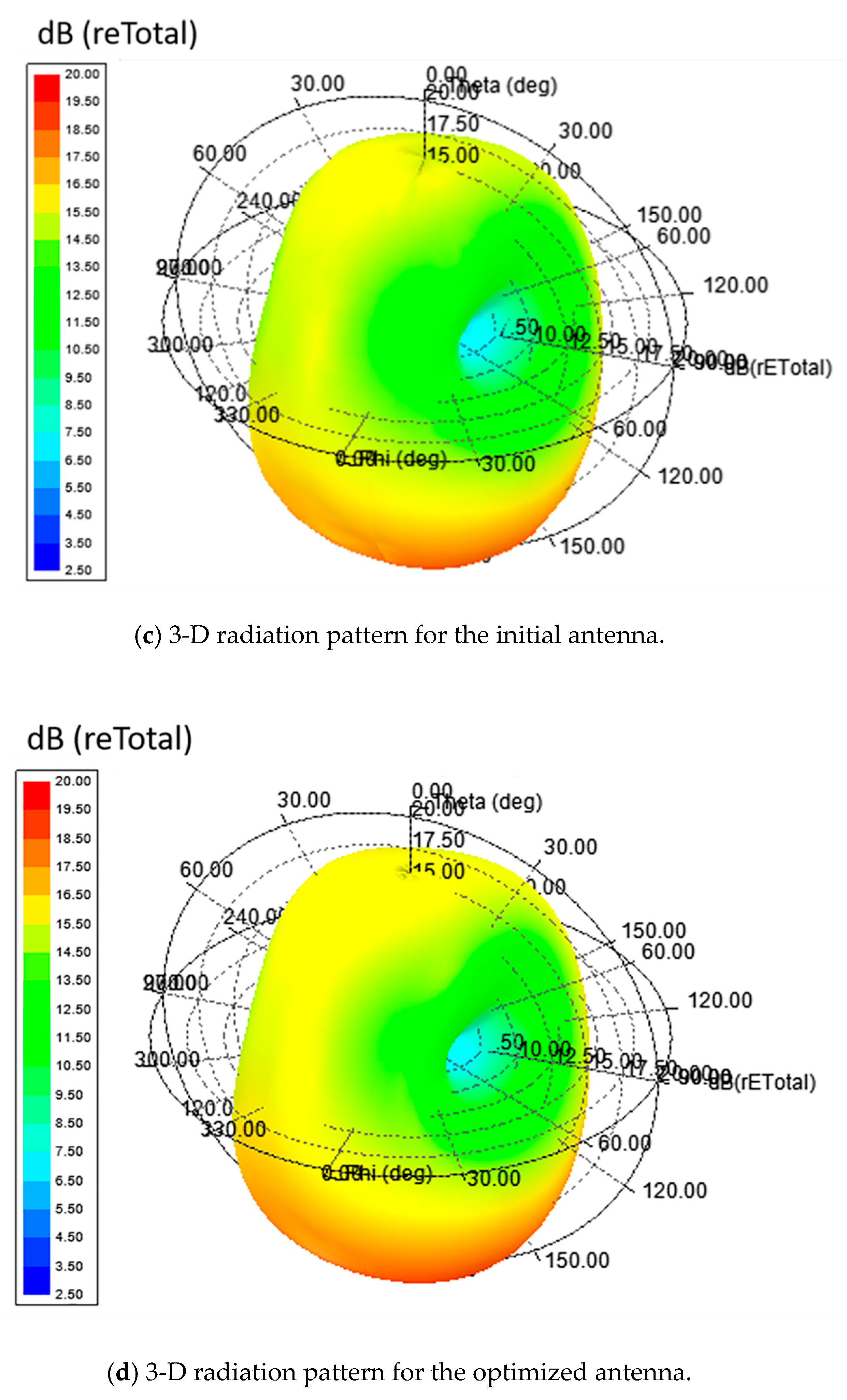
| Radiation intensity (dB) | 15.42 | 15.42 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kapoor, D.; Sangwan, V.; Tan, C.M.; Paliwal, V.; Tanwar, N. Optimization of a T-Shaped MIMO Antenna for Reduction of EMI. Appl. Sci. 2020, 10, 3117. https://doi.org/10.3390/app10093117
Kapoor D, Sangwan V, Tan CM, Paliwal V, Tanwar N. Optimization of a T-Shaped MIMO Antenna for Reduction of EMI. Applied Sciences. 2020; 10(9):3117. https://doi.org/10.3390/app10093117
Chicago/Turabian StyleKapoor, Dipesh, Vivek Sangwan, Cher Ming Tan, Vani Paliwal, and Nirdosh Tanwar. 2020. "Optimization of a T-Shaped MIMO Antenna for Reduction of EMI" Applied Sciences 10, no. 9: 3117. https://doi.org/10.3390/app10093117
APA StyleKapoor, D., Sangwan, V., Tan, C. M., Paliwal, V., & Tanwar, N. (2020). Optimization of a T-Shaped MIMO Antenna for Reduction of EMI. Applied Sciences, 10(9), 3117. https://doi.org/10.3390/app10093117






