Investigation on the Measurement Method for Output Torque of a Spherical Motor
Abstract
1. Introduction
2. Electromagnetic Theory and Simulation of the Output Torque of a Spherical Motor
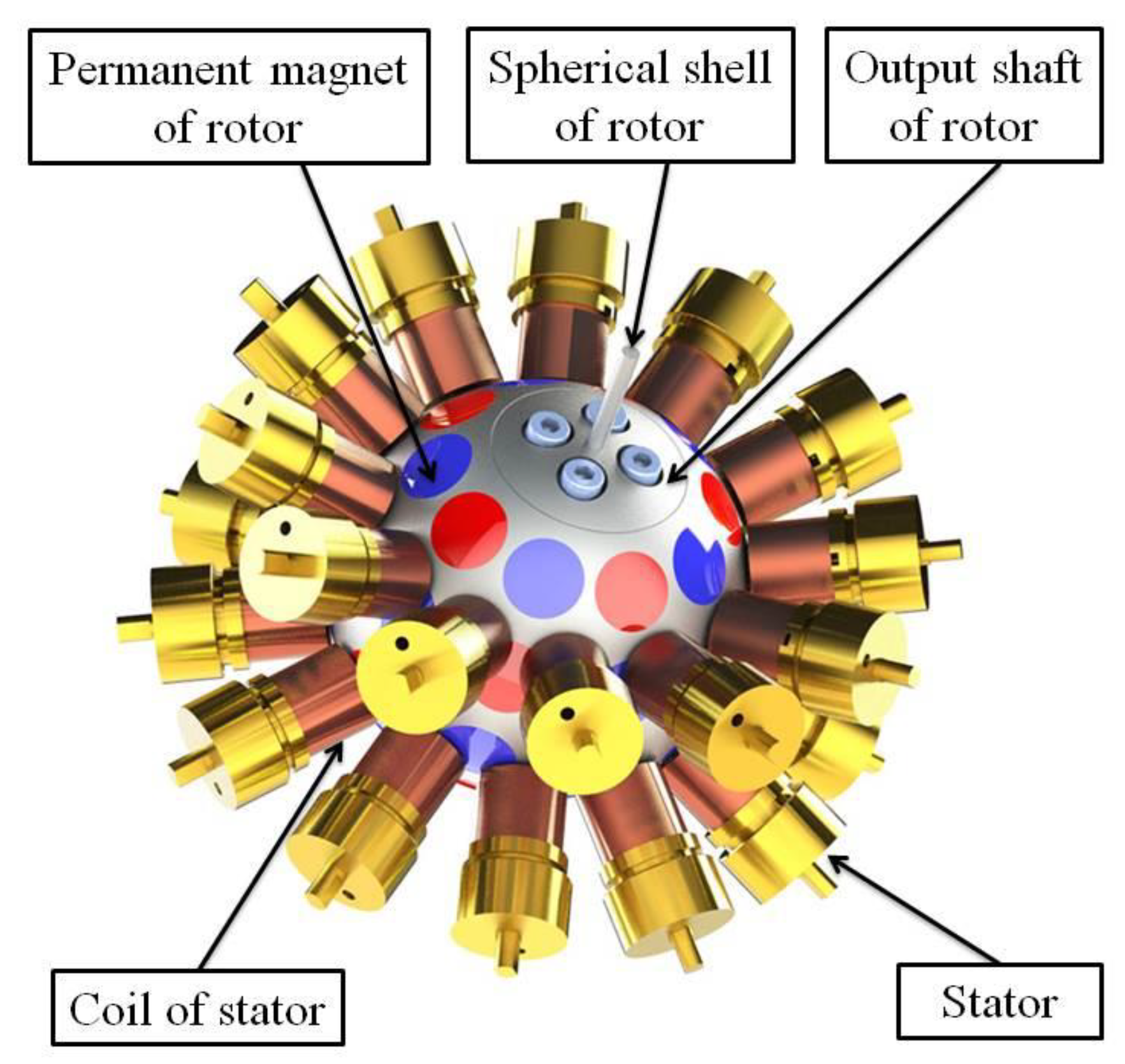
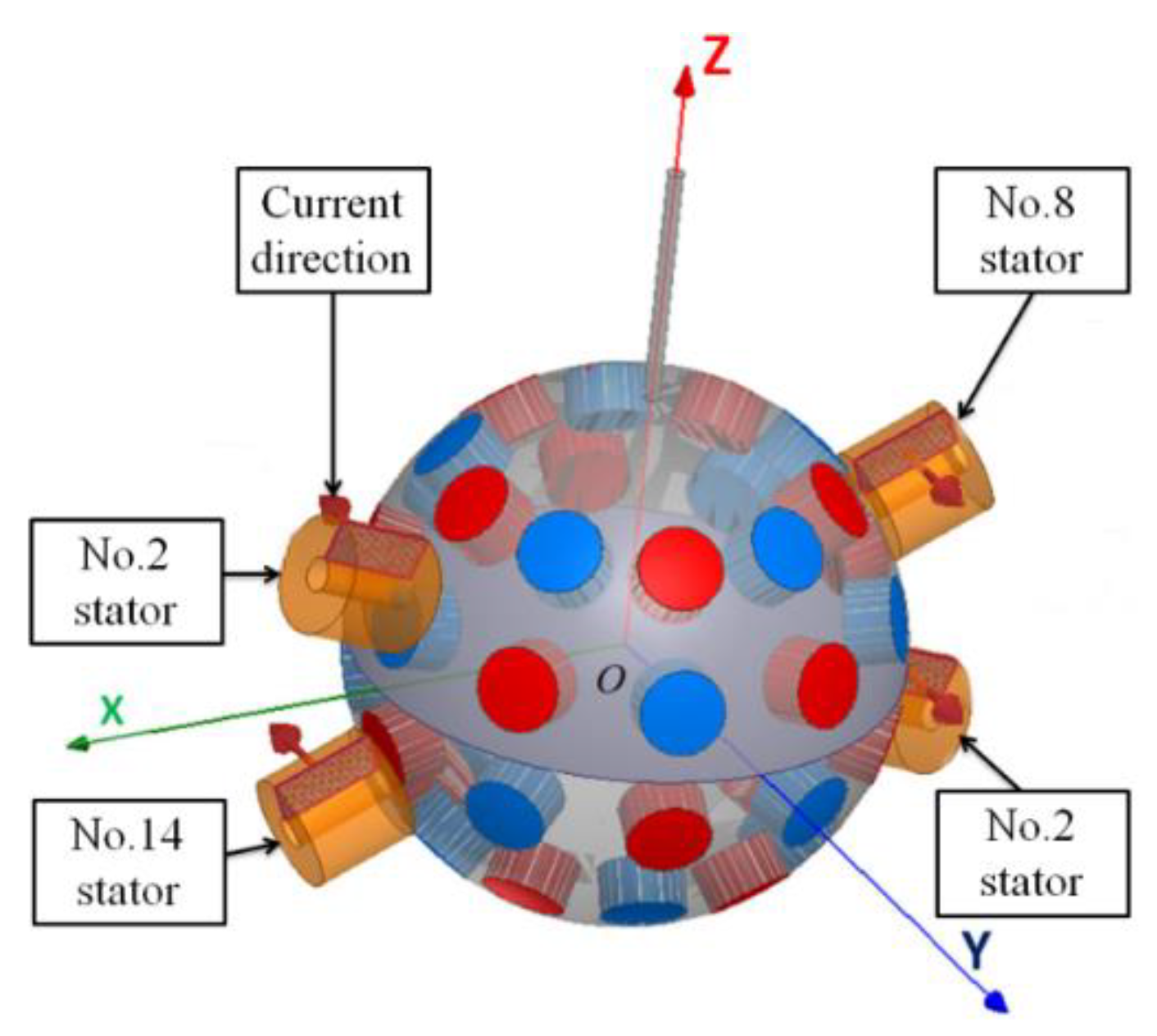
2.1. Motor Structure
2.2. Electromagnetic Theory of Output Torque
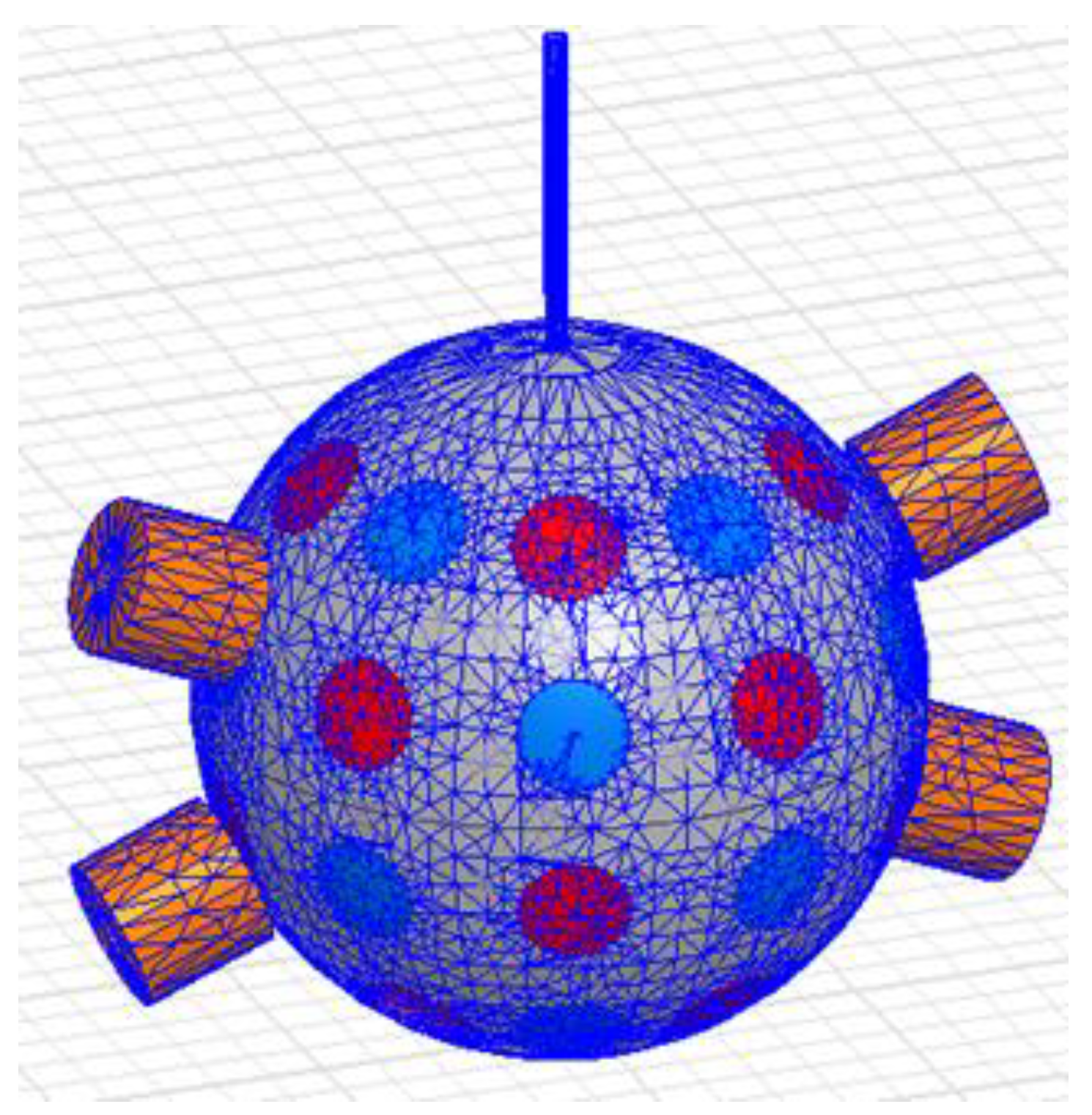
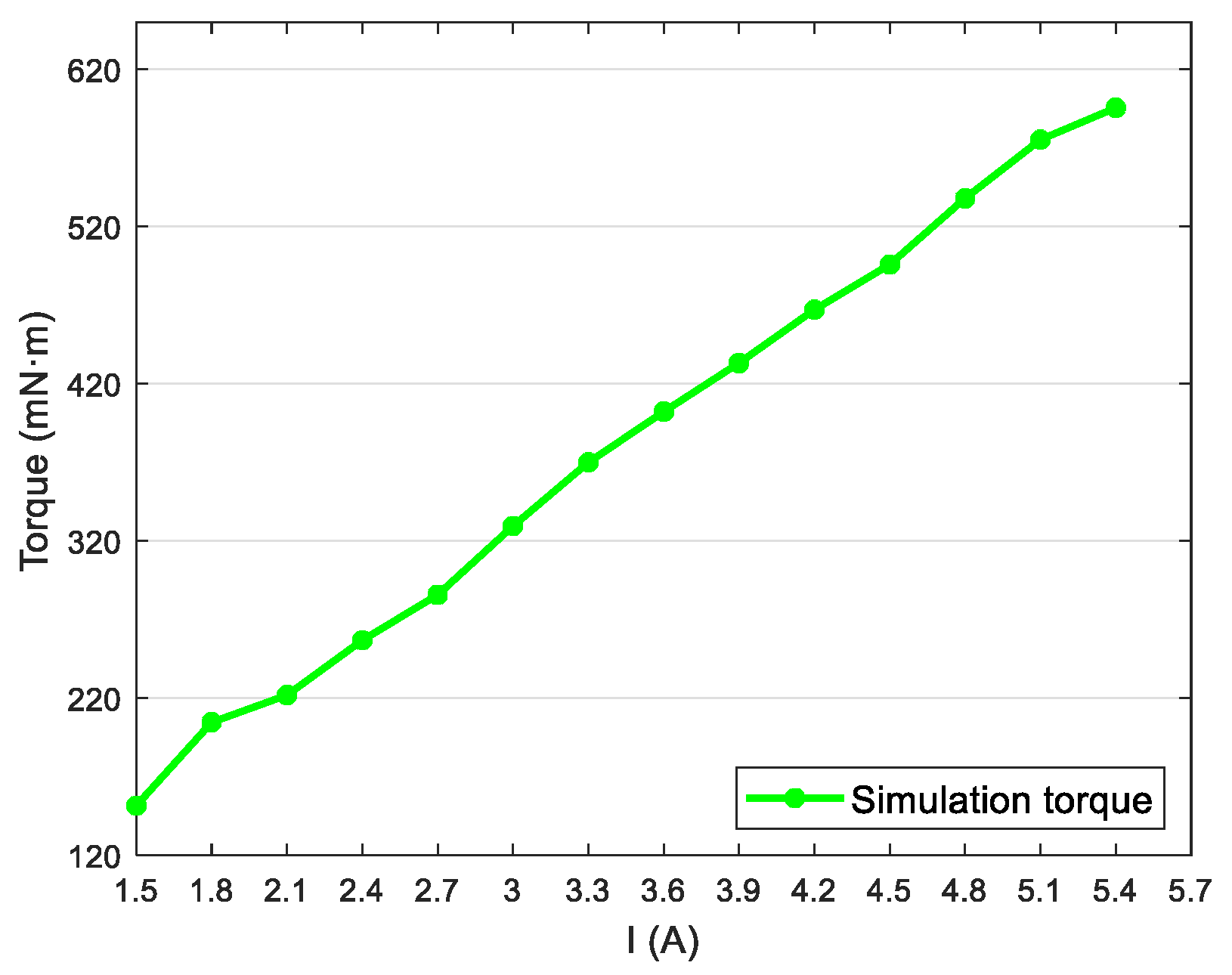
2.3. Torque Simulation
3. Experimental Test of Output Torque for a PMSM
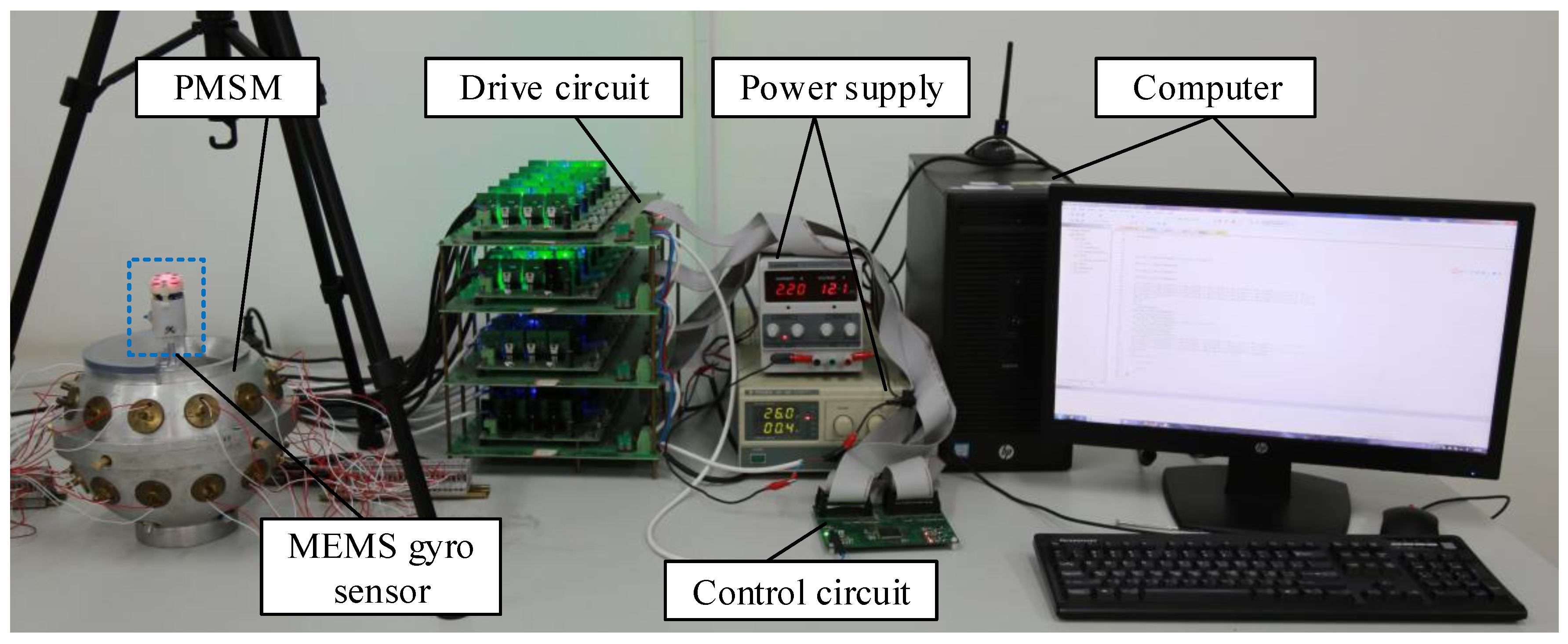
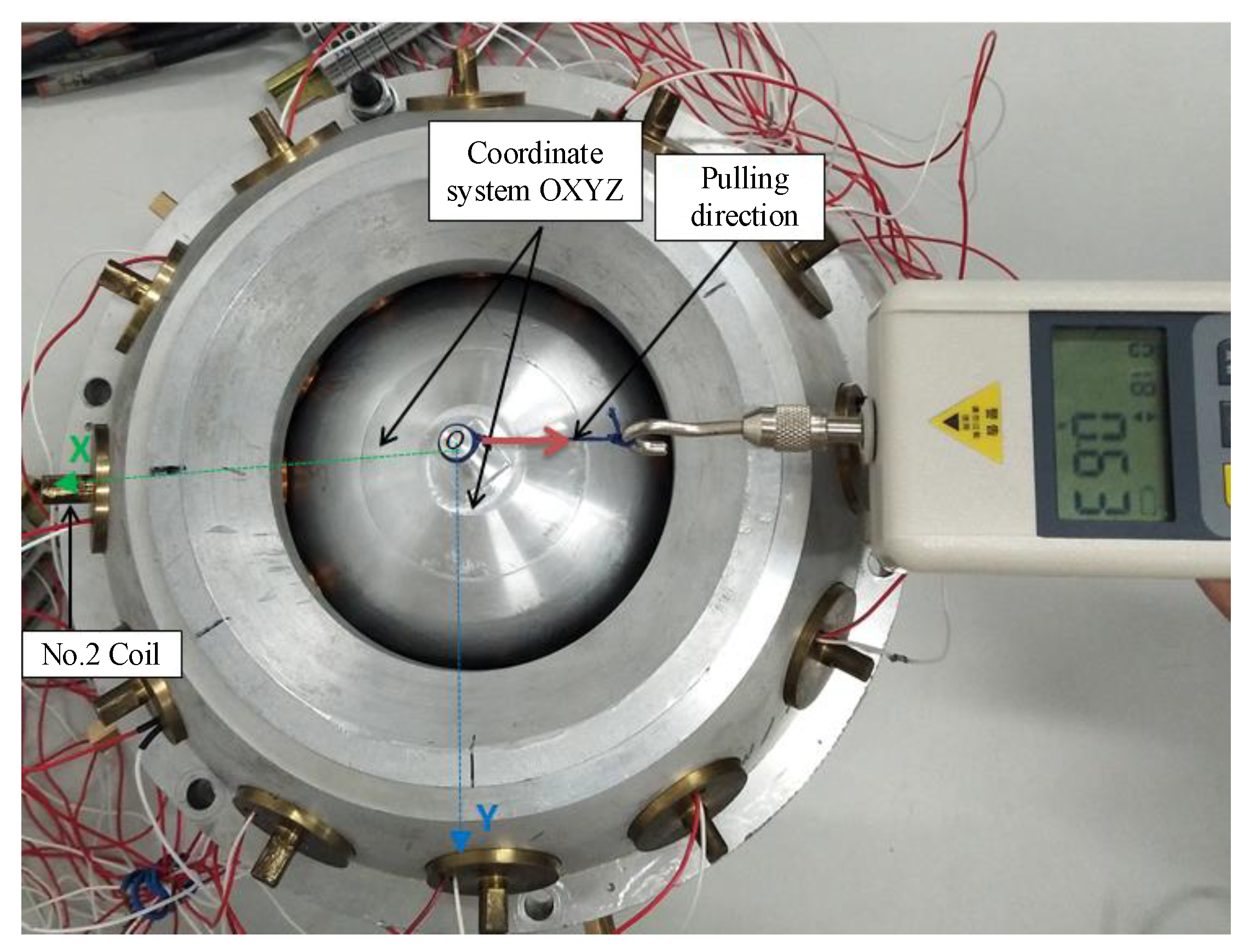
3.1. Experimental Setup
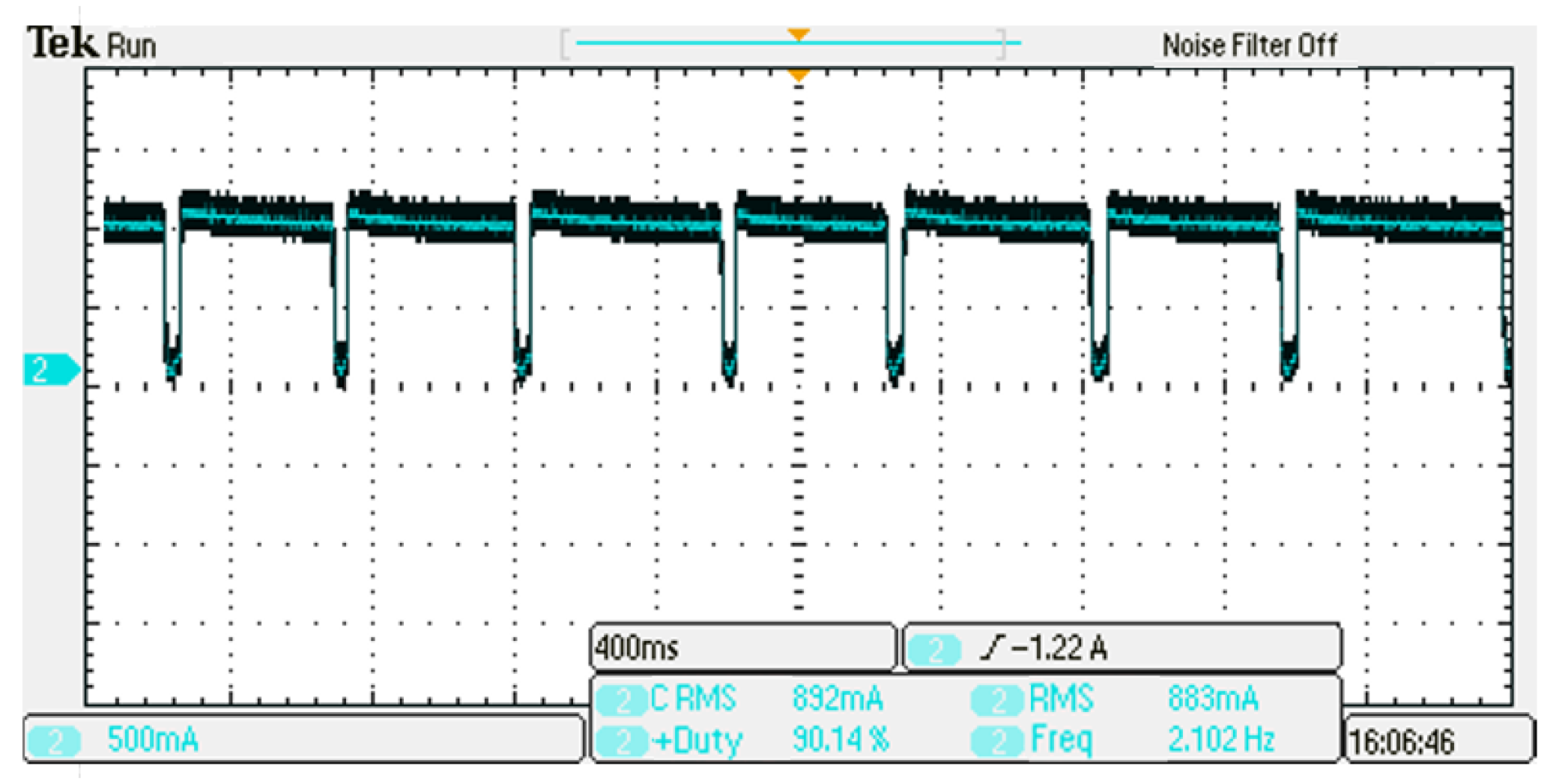
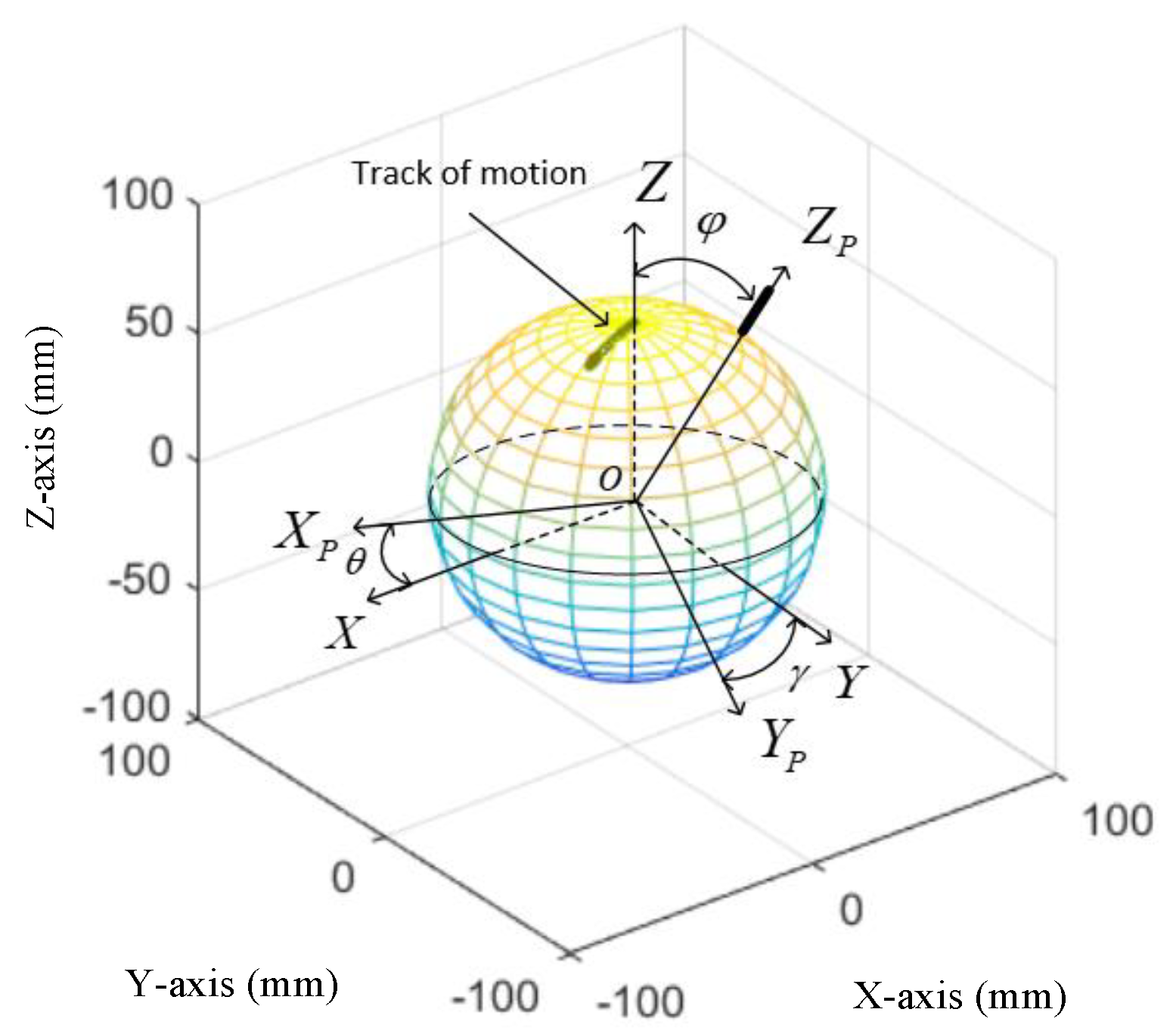
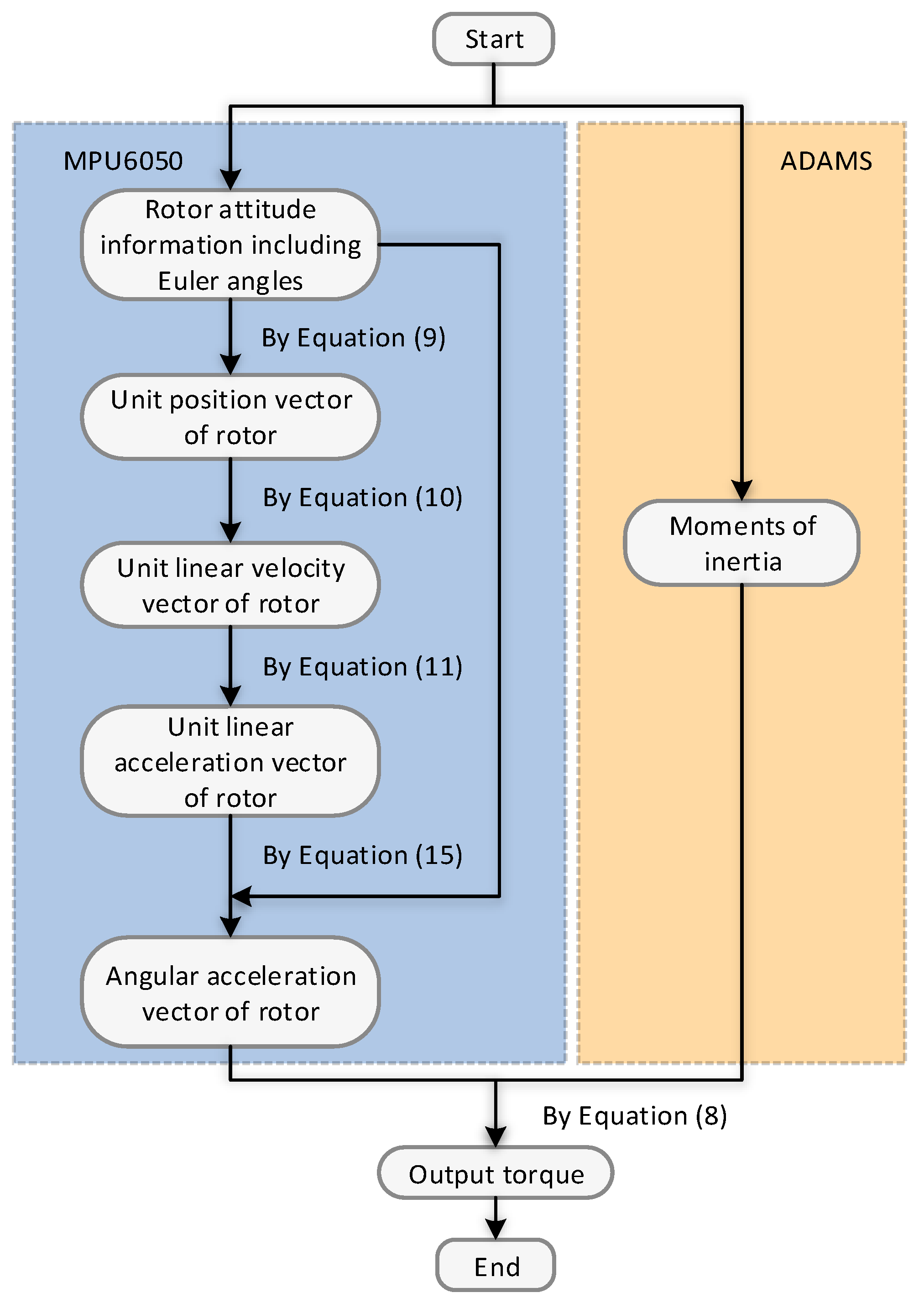
3.2. Principles of Experimentation
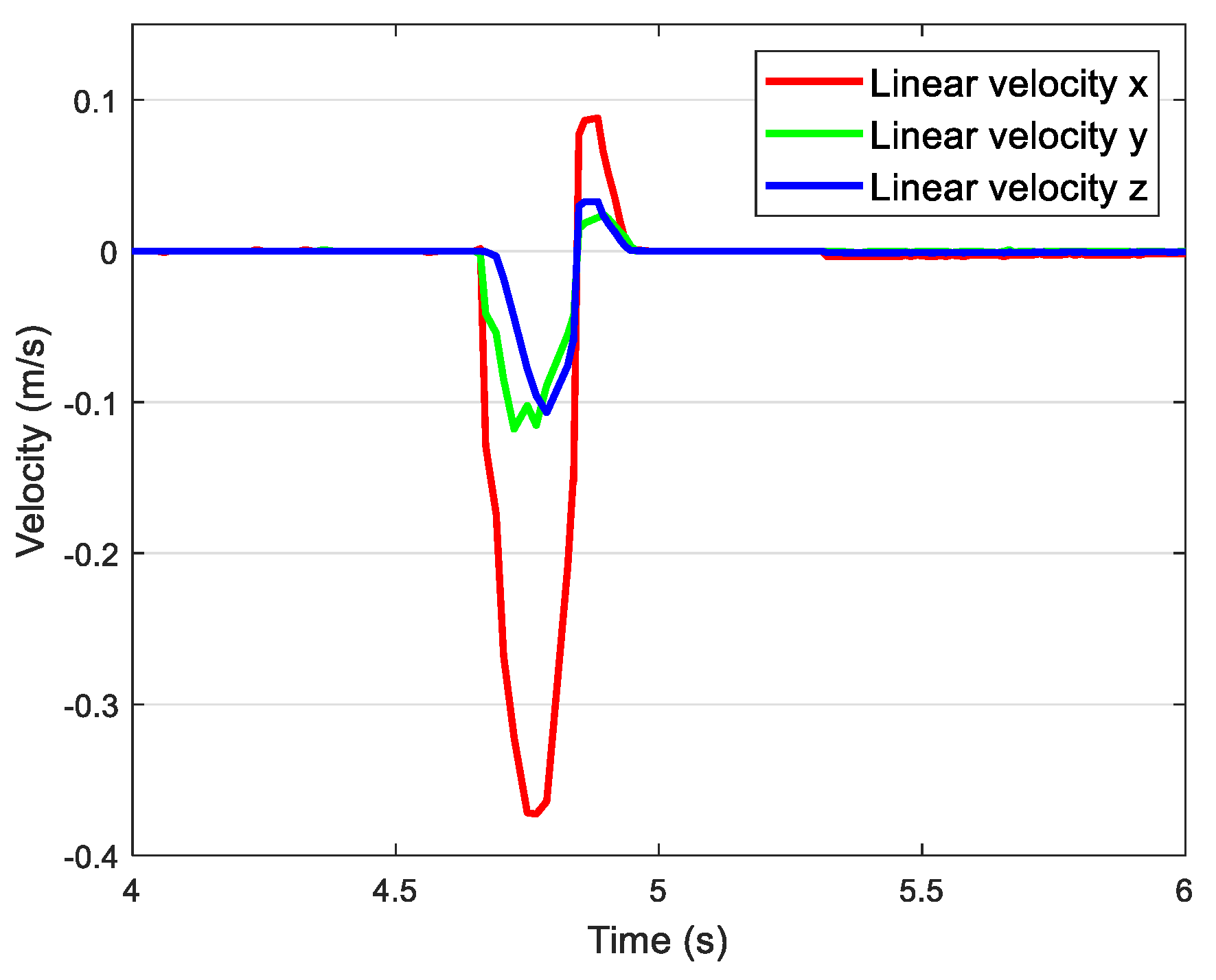
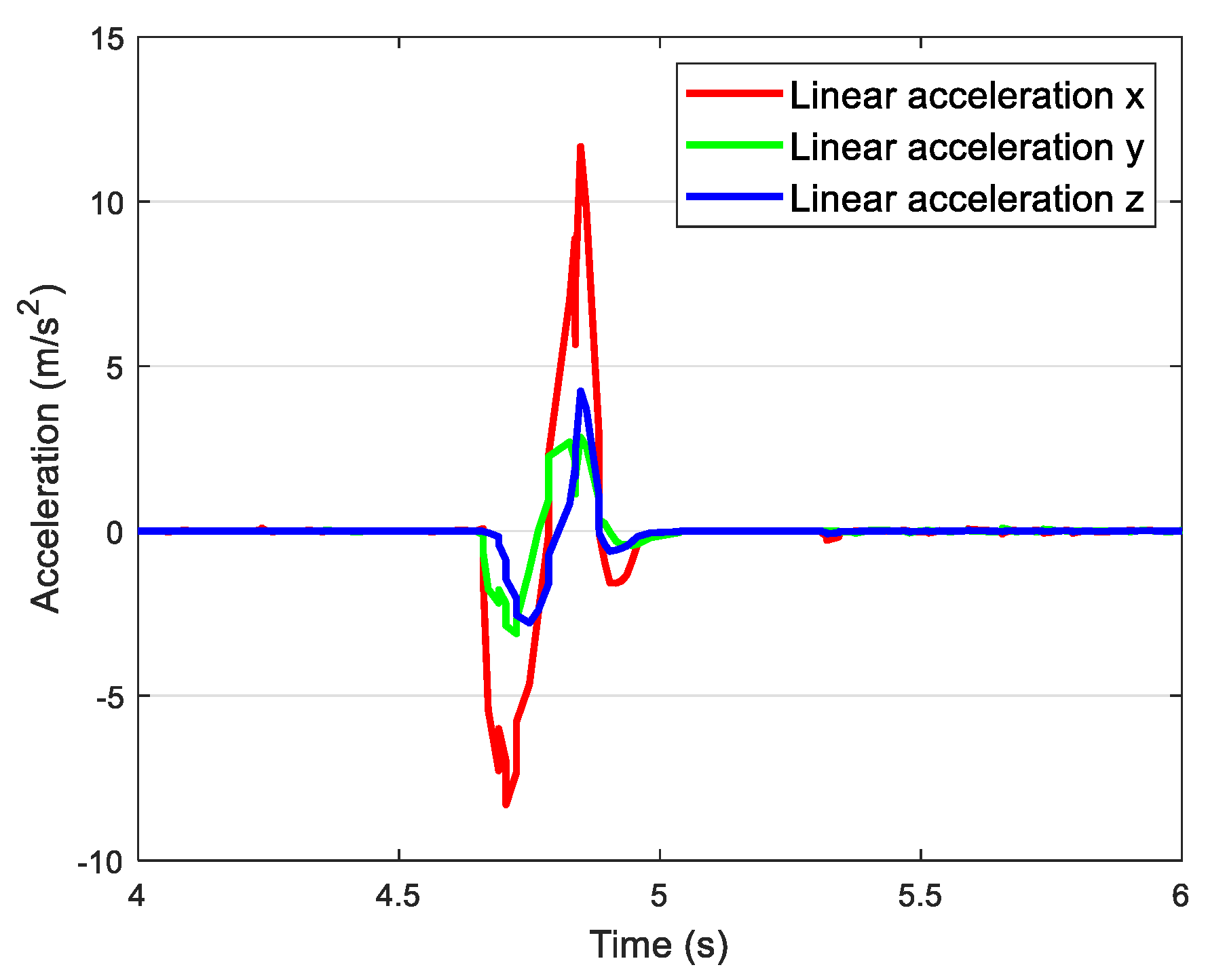
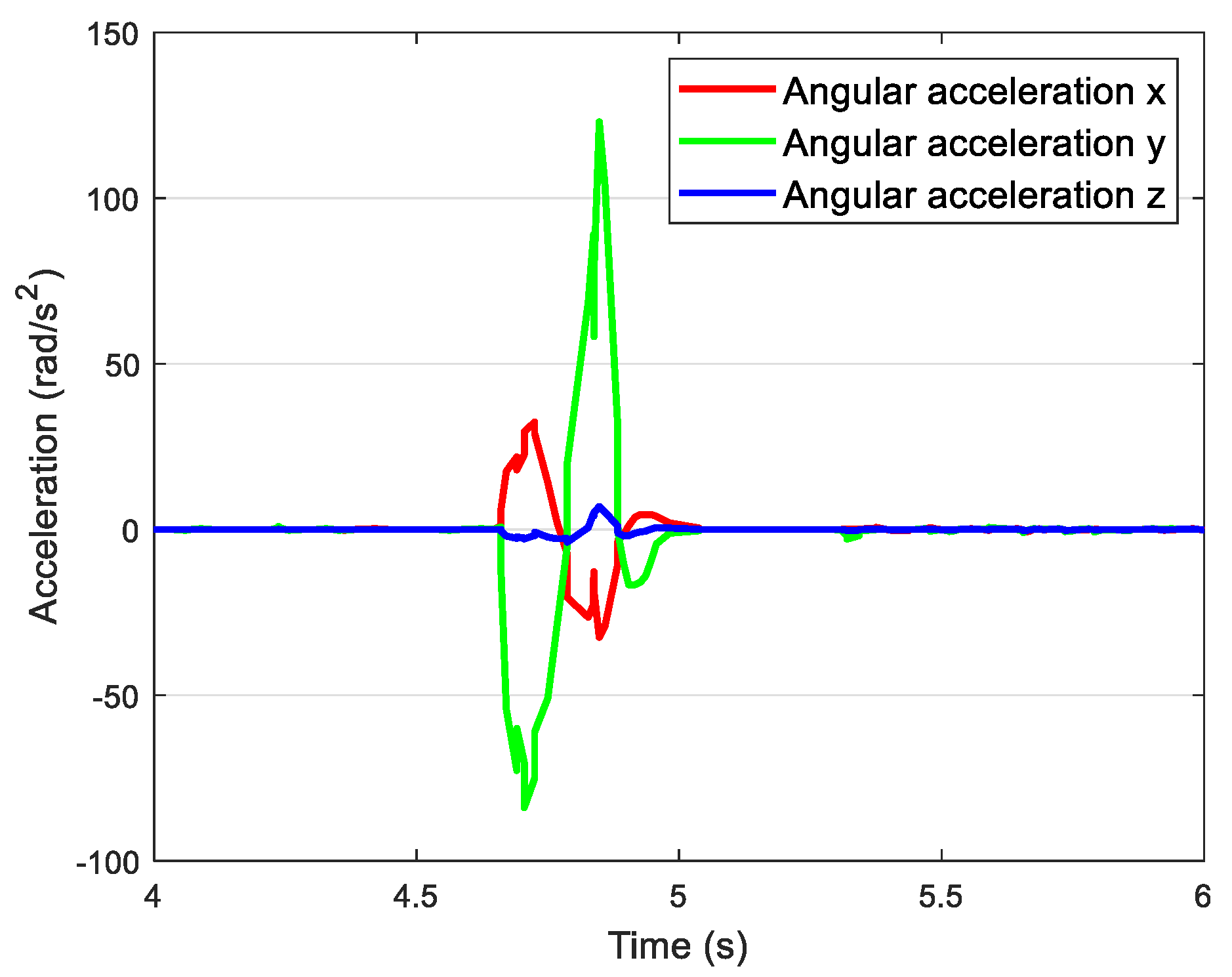
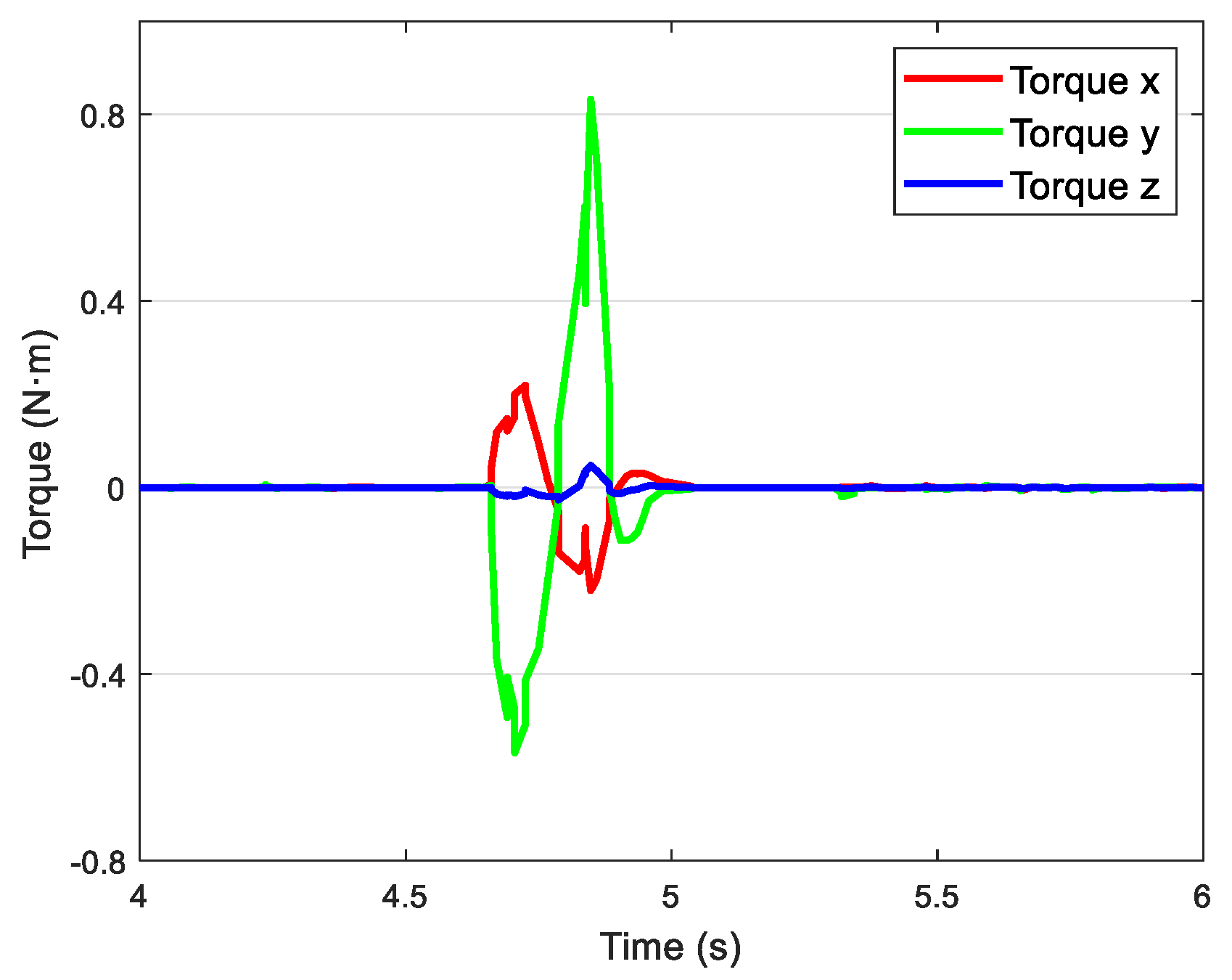
3.3. Experimental Results
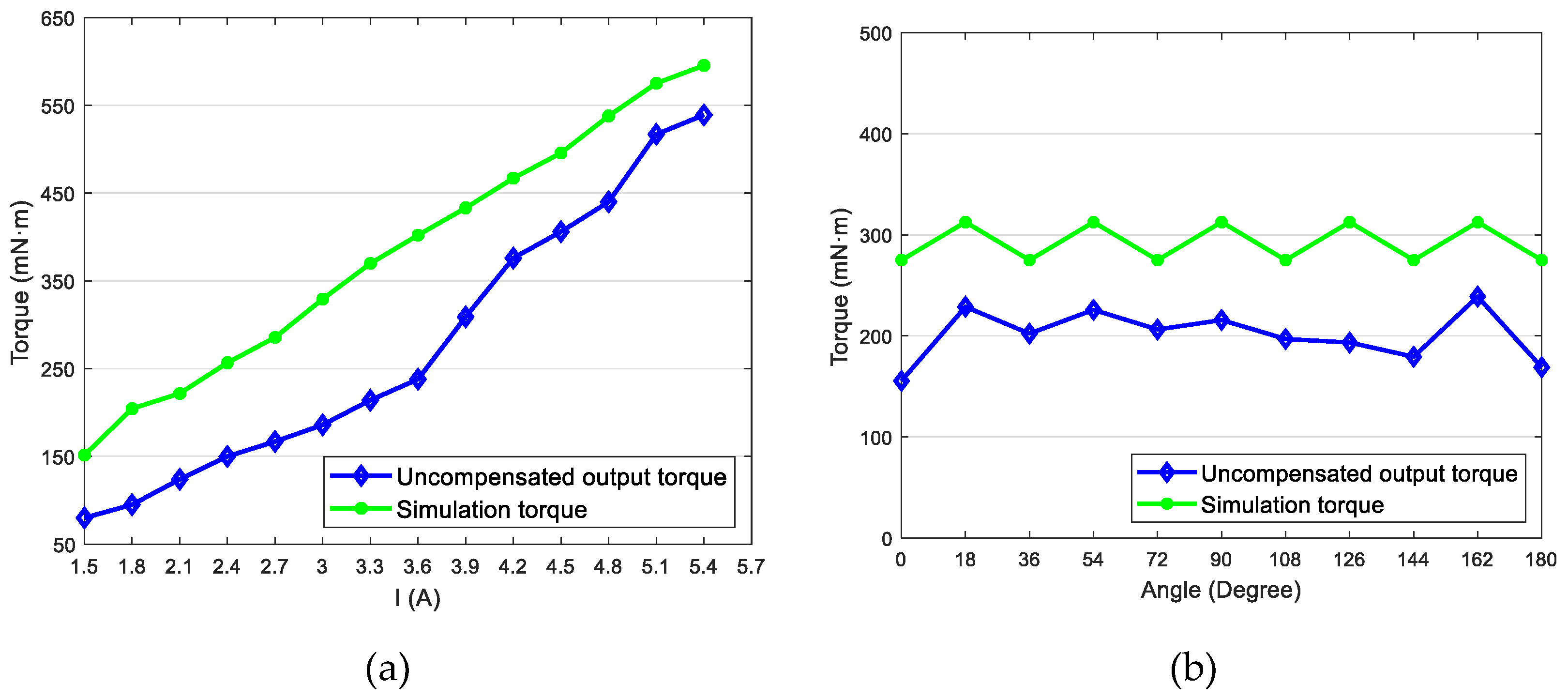
3.4. Comparison with Simulated Results
3.5. Error Analysis and Compensation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lee, K.M.; Vachtsevanos, K.; Kwan, C. Development of a Spherical Stepper Wrist Motor. J. Intell. Robot. Syst. 1998, 1, 225–242. [Google Scholar]
- Lee, K.M.; Son, H.S. Torque Model for Design and Control of a Spherical Wheel Motor. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Monterey, CA, USA, 24–28 July 2005. [Google Scholar]
- Xia, Y.; Li, G.; Qian, Z.; Ye, Q.; Zhang, Z. Research on rotor magnet loss in fractional-slot concentrated-windings permanent magnet motor. In Proceedings of the IEEE 11th Conference on Industrial Electronics and Applications, Hefei, China, 5–7 June 2016. [Google Scholar]
- Wang, A.; Wang, C.; Hu, C.; Qian, Z.; Ju, L.; Liu, J. An EKF for PMSM sensorless control based on noise model identification using Ant Colony Algorithm. In Proceedings of the 2009 International Conference on Electrical Machines and Systems, Tokyo, Japan, 15–18 November 2009. [Google Scholar]
- Rossini, L.; Onillon, E.; Chételat, O.; Perriard, Y. Force and Torque Analytical Models of a Reaction Sphere Actuator Based on Spherical Harmonic Rotation and Decomposition. IEEE ASME Trans. Mechatron. 2013, 18, 1006–1018. [Google Scholar] [CrossRef]
- Rossini, L.; Chételat, O.; Onillon, E.; Perriard, Y. An open-loop control strategy of a reaction sphere for satellite attitude control. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011. [Google Scholar]
- Yan, L.; Chen, I.M.; Yang, G.; Lee, K. Analytical and Experimental Investigation on the Magnetic Field and Torque of a Permanent Magnet Spherical Actuator. IEEE-ASME Trans. Mechatron. 2006, 11, 409–419. [Google Scholar]
- Yan, L.; Chen, I.M.; Yang, G.; Lee, K. Design and Analysis of a Permanent Magnet Spherical Actuator. IEEE ASME Trans. Mechatron. 2008, 13, 239–248. [Google Scholar] [CrossRef]
- Kumagai, M.; Ochiai, T. Development of a Robot Balancing on a Ball. In Proceedings of the 2008 International Conference on Control, Automation and Systems, Seoul, Korea, 14–17 October 2008. [Google Scholar]
- Kumagai, M. Torque Evaluation Method of Spherical Motors Using Six-Axis Force/Torque Sensor. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation, Stockholm, Sweden, 16–21 May 2016. [Google Scholar]
- Kasashima, N.; Ashida, K.; Yano, T.; Gofuku, A.; Shibata, M. Torque Control Method of an Electromagnetic Spherical Motor Using Torque Map. IEEE ASME Trans. Mechatron. 2016, 21, 2050–2060. [Google Scholar] [CrossRef]
- Qian, Z.; Wang, Q.; Ju, L.; Wang, A.; Liu, J. Torque Modeling and Control Algorithm of a Permanent Magnetic Spherical Motor. In Proceedings of the 2009 International Conference on Electrical Machines and Systems, Tokyo, Japan, 15–18 November 2009. [Google Scholar]
- Wang, W.; Wang, J.; Jewell, G.W.; Howe, D. Design and Control of a Novel Spherical Permanent Magnet Actuator with Three Degrees of Freedom. IEEE ASME Trans. Mechatron. 2003, 8, 457–468. [Google Scholar] [CrossRef]
- Lu, Y.; Hu, C.G.; Wang, Q.J.; Hong, Y.; Shen, W.X.; Zhou, C.Q. A New Rotor Position Measurement Method for Permanent Magnet Spherical Motors. Appl. Sci. 2018, 8, 2415. [Google Scholar] [CrossRef]
- Li, B.; Li, G.D.; Li, H.F. Magnetic Field Analysis of 3-DOF Permanent Magnetic Spherical Motor Using Magnetic Equivalent Circuit Method. IEEE Trans. Magn. 2011, 47, 2127–2133. [Google Scholar] [CrossRef]
- Changliang, X.; Peng, S.; Hongfeng, L.; Bin, L.; Tingna, S. Research on Torque Calculation Method of Permanent-Magnet Spherical Motor Based on the Finite-Element Method. IEEE Trans. Magn. 2009, 45, 2015–2022. [Google Scholar] [CrossRef]
- Liu, J.M.; Deng, H.Y.; Chen, W.H.; Bai, S.P. Robust dynamic decoupling control for permanent magnet spherical actuators based on extended state observer. IET Control Theory A 2017, 11, 619–631. [Google Scholar] [CrossRef]
- Wen, Y.; Li, G.; Wang, Q.; Guo, X. Robust Adaptive Sliding-Mode Control for Permanent Magnet Spherical Actuator with Uncertainty Using Dynamic Surface Approach. J. Electr. Eng. Technol. 2019, 14, 2341–2353. [Google Scholar] [CrossRef]
- Rong, Y.; Wang, Q.; Lu, S.; Li, G.; Lu, Y.; Xu, J. Improving attitude detection performance for spherical motors using a MEMS inertial measurement sensor. IET Electr. Power Appl. 2019, 13, 198–205. [Google Scholar] [CrossRef]



| Current of Each Coils (A) | Total Current(A) | |||
|---|---|---|---|---|
| No.14 | No.2 | No.20 | No.8 | |
| 0 | 0.4 | 0.049 | 1 | 1.45 |
| 0 | 0.9 | 0.052 | 0.95 | 1.90 |
| 0 | 1 | 0.024 | 1.01 | 2.03 |
| 0 | 1.15 | 0.052 | 1.17 | 2.37 |
| 1.12 | 0.45 | 0.042 | 1.06 | 2.67 |
| 1.03 | 0.96 | 0.023 | 1.01 | 3.02 |
| 1.12 | 1.12 | 0.030 | 1.12 | 3.39 |
| 1.22 | 1.22 | 0.039 | 1.21 | 3.69 |
| 1.31 | 1.31 | 0.054 | 1.3 | 3.97 |
| 1.42 | 1.42 | 0.054 | 1.4 | 4.29 |
| 1.5 | 1.5 | 0.026 | 1.5 | 4.53 |
| 1.63 | 1.62 | 0.054 | 1.6 | 4.90 |
| 1.74 | 1.69 | 0.054 | 1.77 | 5.25 |
| 1.8 | 1.78 | 0.037 | 1.8 | 5.42 |
| Total Stator Current (A) | |
|---|---|
| 1.5 | 47.19% |
| 1.8 | 53.54% |
| 2.1 | 44.09% |
| 2.4 | 41.57% |
| 2.7 | 41.53% |
| 3.0 | 43.53% |
| 3.3 | 42.14% |
| 3.6 | 40.81% |
| 3.9 | 28.66% |
| 4.2 | 19.49% |
| 4.5 | 18.10% |
| 4.8 | 18.20% |
| 5.1 | 10.11% |
| 5.4 | 9.48% |
| Angle (Degree) | |
|---|---|
| 0 | 43.44% |
| 18 | 26.81% |
| 36 | 26.43% |
| 54 | 27.76% |
| 72 | 24.96% |
| 90 | 30.99% |
| 108 | 28.37% |
| 126 | 38.11% |
| 144 | 34.75% |
| 162 | 17.17% |
| 180 | 38.55% |
| Number | Maximum Static Friction (mN·m) |
|---|---|
| 1 | 60.1 |
| 2 | 76.3 |
| 3 | 61.7 |
| 4 | 65.2 |
| 5 | 68.6 |
| 6 | 63.6 |
| 7 | 62.9 |
| 8 | 77.4 |
| 9 | 71.0 |
| 10 | 77.1 |
| 11 | 75.5 |
| 12 | 77.4 |
| 13 | 68.0 |
| 14 | 76.1 |
| 15 | 78.2 |
| 16 | 65.3 |
| 17 | 62.7 |
| 18 | 63.0 |
| 19 | 71.6 |
| 20 | 72.4 |
| Total Stator Current (A) | |
|---|---|
| 1.5 | 1.19% |
| 1.8 | 19.45% |
| 2.1 | 12.67% |
| 2.4 | 14.42% |
| 2.7 | 17.13% |
| 3.0 | 22.36% |
| 3.3 | 23.11% |
| 3.6 | 23.47% |
| 3.9 | 12.56% |
| 4.2 | 4.56% |
| 4.5 | 4.04% |
| 4.8 | 5.24% |
| 5.1 | 2.01% |
| 5.4 | 2.23% |
| Angle (Degree) | |
|---|---|
| 0 | 18.09% |
| 18 | 4.51% |
| 36 | 1.08% |
| 54 | 5.46% |
| 72 | 0.39% |
| 90 | 8.69% |
| 108 | 3.02% |
| 126 | 15.81% |
| 144 | 9.40% |
| 162 | 5.13% |
| 180 | 13.20% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, Y.; Li, G.; Wang, Q.; Tang, R.; Liu, Y.; Li, H. Investigation on the Measurement Method for Output Torque of a Spherical Motor. Appl. Sci. 2020, 10, 2510. https://doi.org/10.3390/app10072510
Wen Y, Li G, Wang Q, Tang R, Liu Y, Li H. Investigation on the Measurement Method for Output Torque of a Spherical Motor. Applied Sciences. 2020; 10(7):2510. https://doi.org/10.3390/app10072510
Chicago/Turabian StyleWen, Yan, Guoli Li, Qunjing Wang, Runyu Tang, Yongbin Liu, and Haolin Li. 2020. "Investigation on the Measurement Method for Output Torque of a Spherical Motor" Applied Sciences 10, no. 7: 2510. https://doi.org/10.3390/app10072510
APA StyleWen, Y., Li, G., Wang, Q., Tang, R., Liu, Y., & Li, H. (2020). Investigation on the Measurement Method for Output Torque of a Spherical Motor. Applied Sciences, 10(7), 2510. https://doi.org/10.3390/app10072510





