Modeling the Natural Convection Flow in a Square Porous Enclosure Filled with a Micropolar Nanofluid under Magnetohydrodynamic Conditions
Abstract
1. Introduction
2. Problem Formulation
3. Numerical Method
3.1. Solution Procedure
- Step 1: Compute the intermediate velocity components, through Equations (28) and (29), using vorticity values at the previous step (for the first iteration, the prescribed initial conditions are used).
- Step 2: Compute the correction potential and update the velocity field ().
- Step 3: Solve Equations (30) and (31) for the magnetic potential and temperature. Then, calculate the magnetic field components and , along with the temperature gradient .
- Step 4: Solve the coupled equations for the microrotation and vorticity, Equations (32) and (33), using the updated magnetic field and temperature gradient.
- Step 5: Repeat Steps 1–4, until the normalized root-mean-square error NRMSE() of all field variables becomes less that a predefined value (e.g., 10−5).
3.2. Vorticity Boundary Conditions
3.3. Zero Flux Temperature Boundary Conditions
4. Results and Discussion
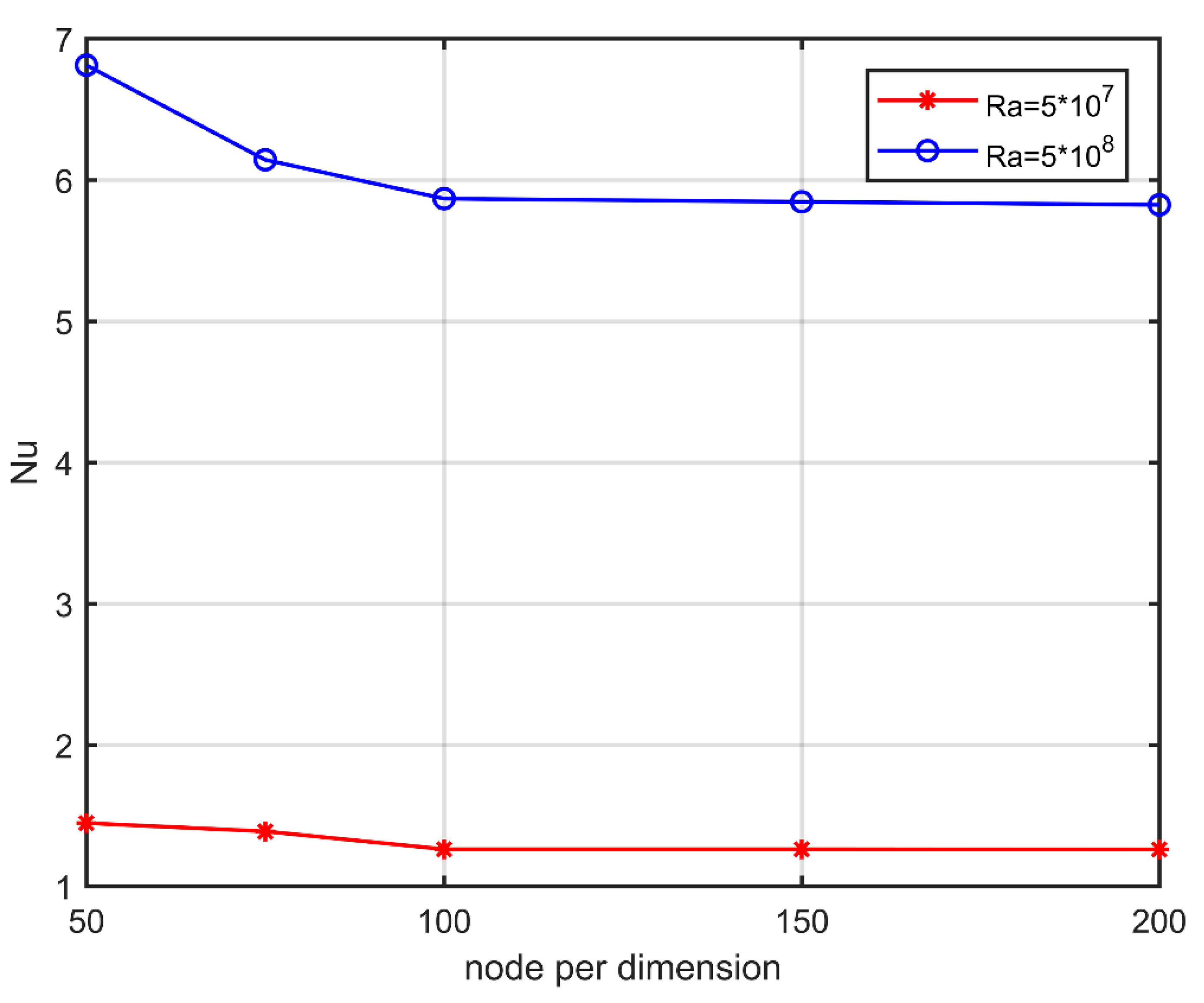
4.1. Code Verification
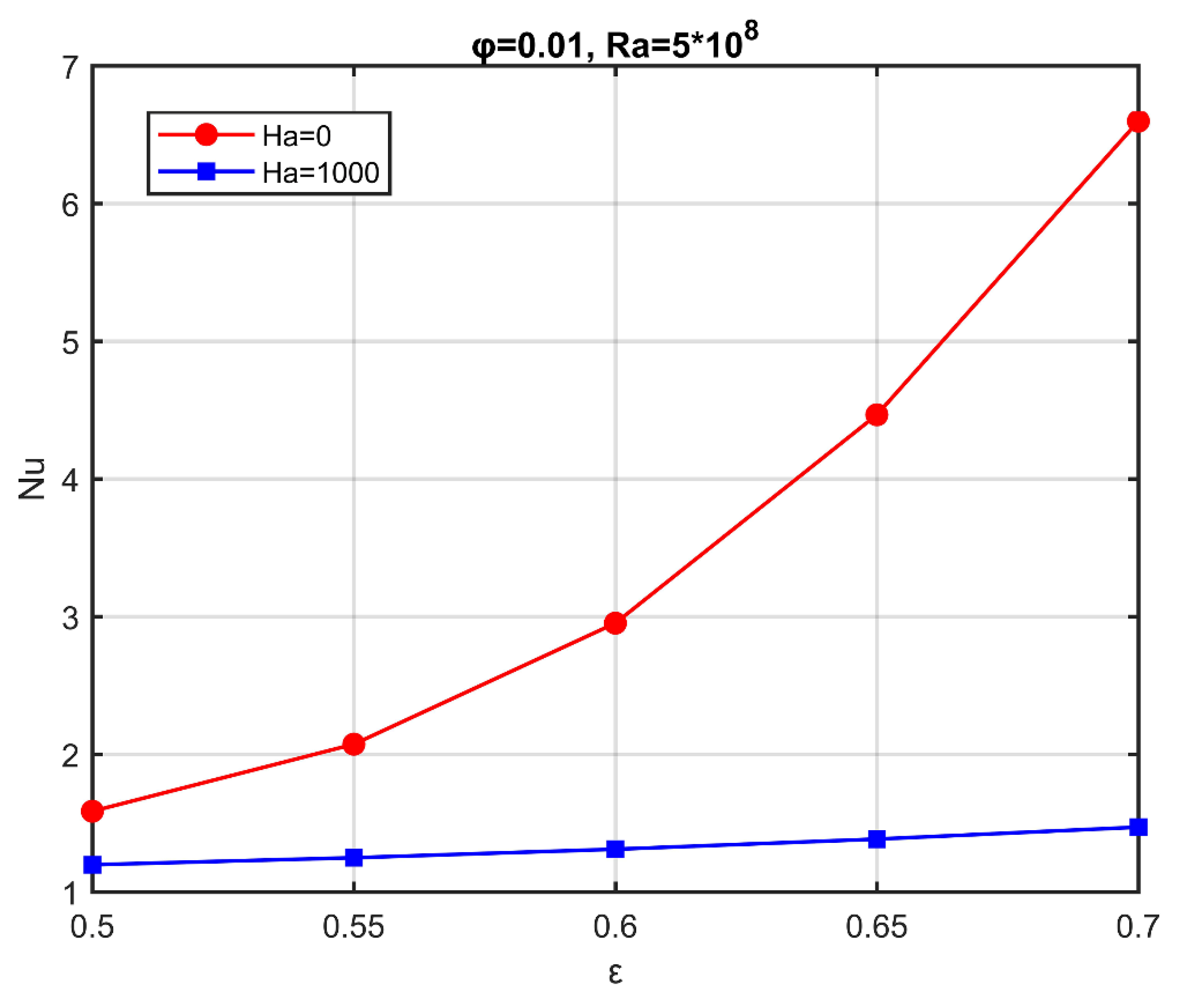
4.2. Effect of Porosity (ε)
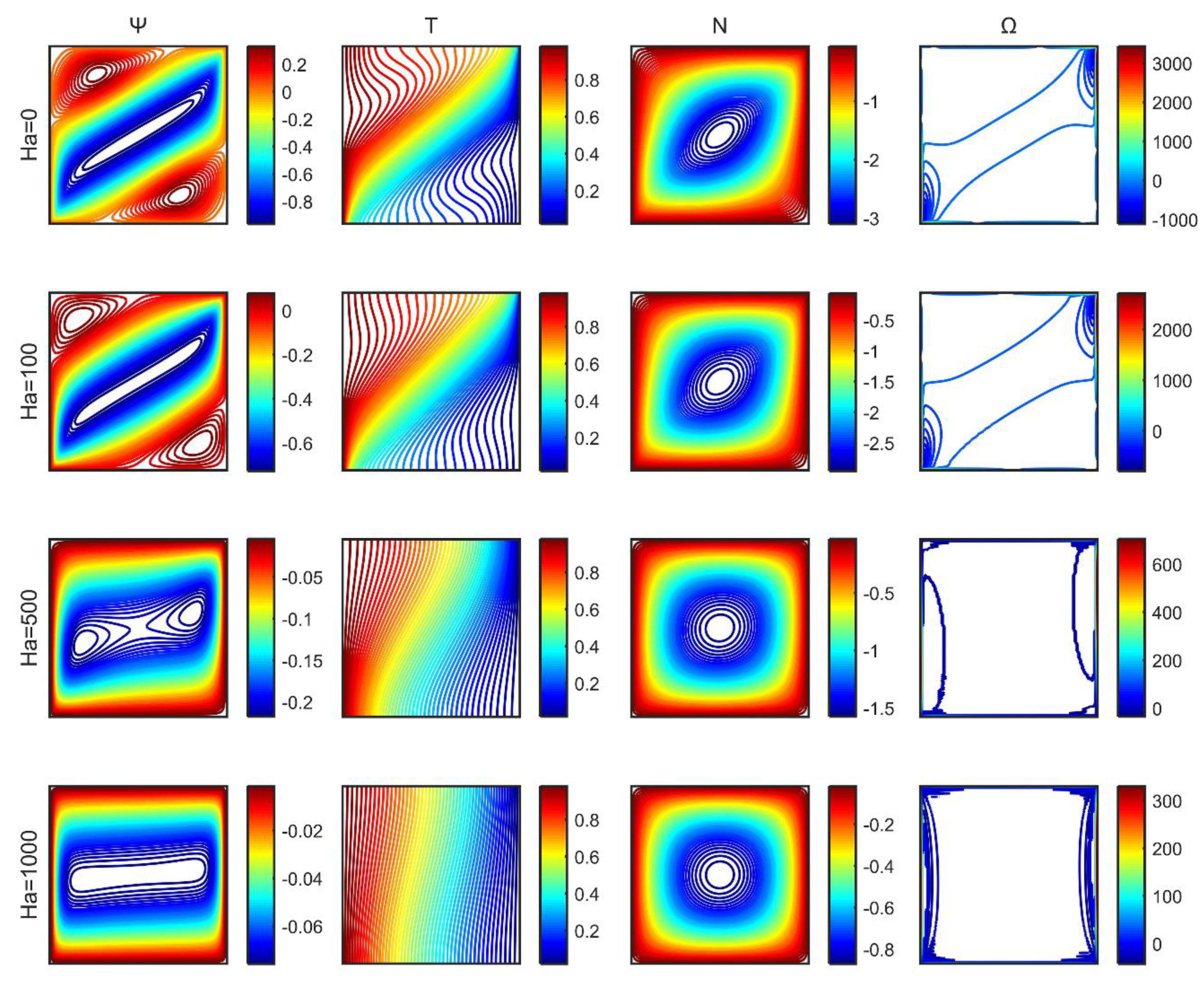
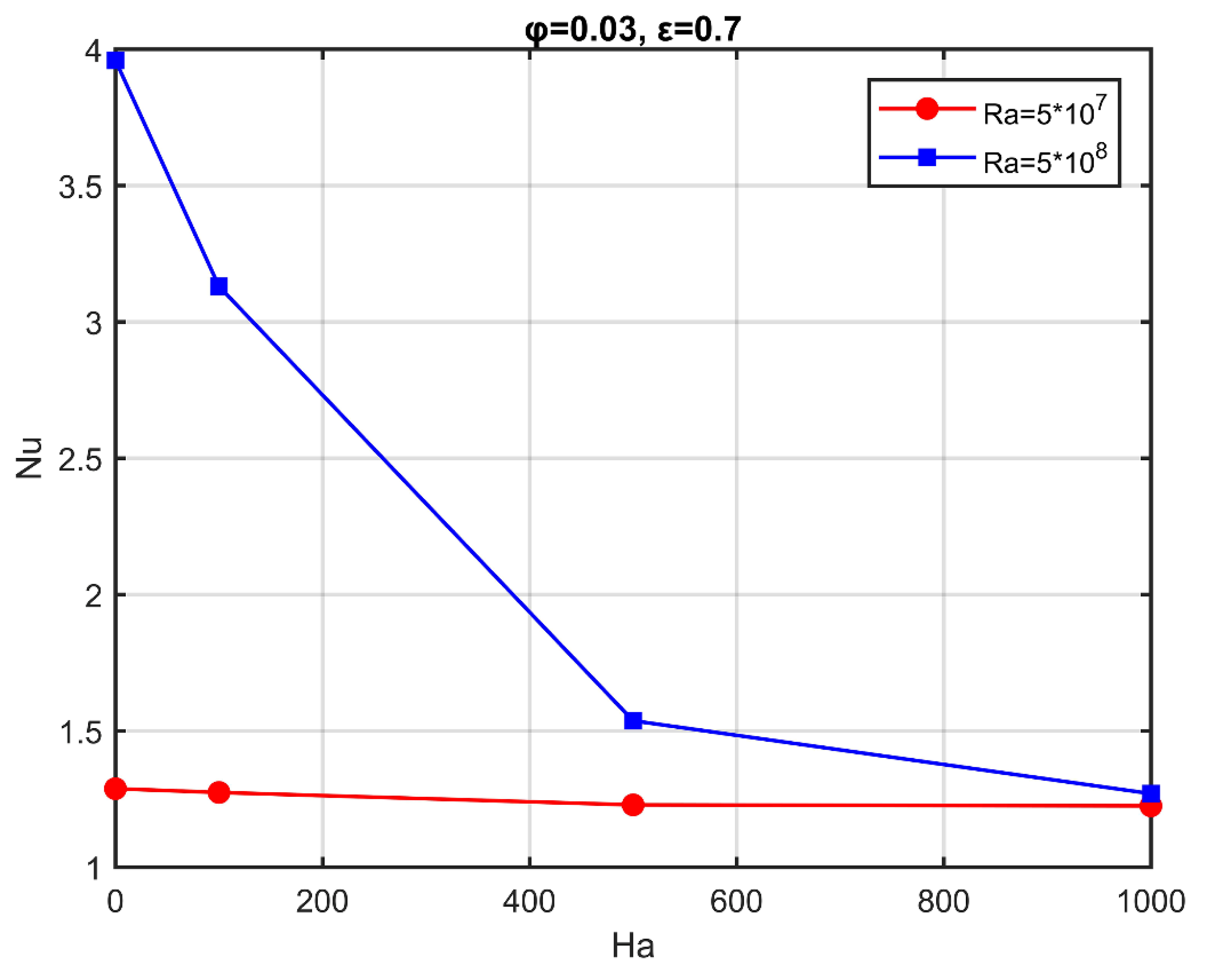
4.3. Effect of the Hartmann Number (Ha)
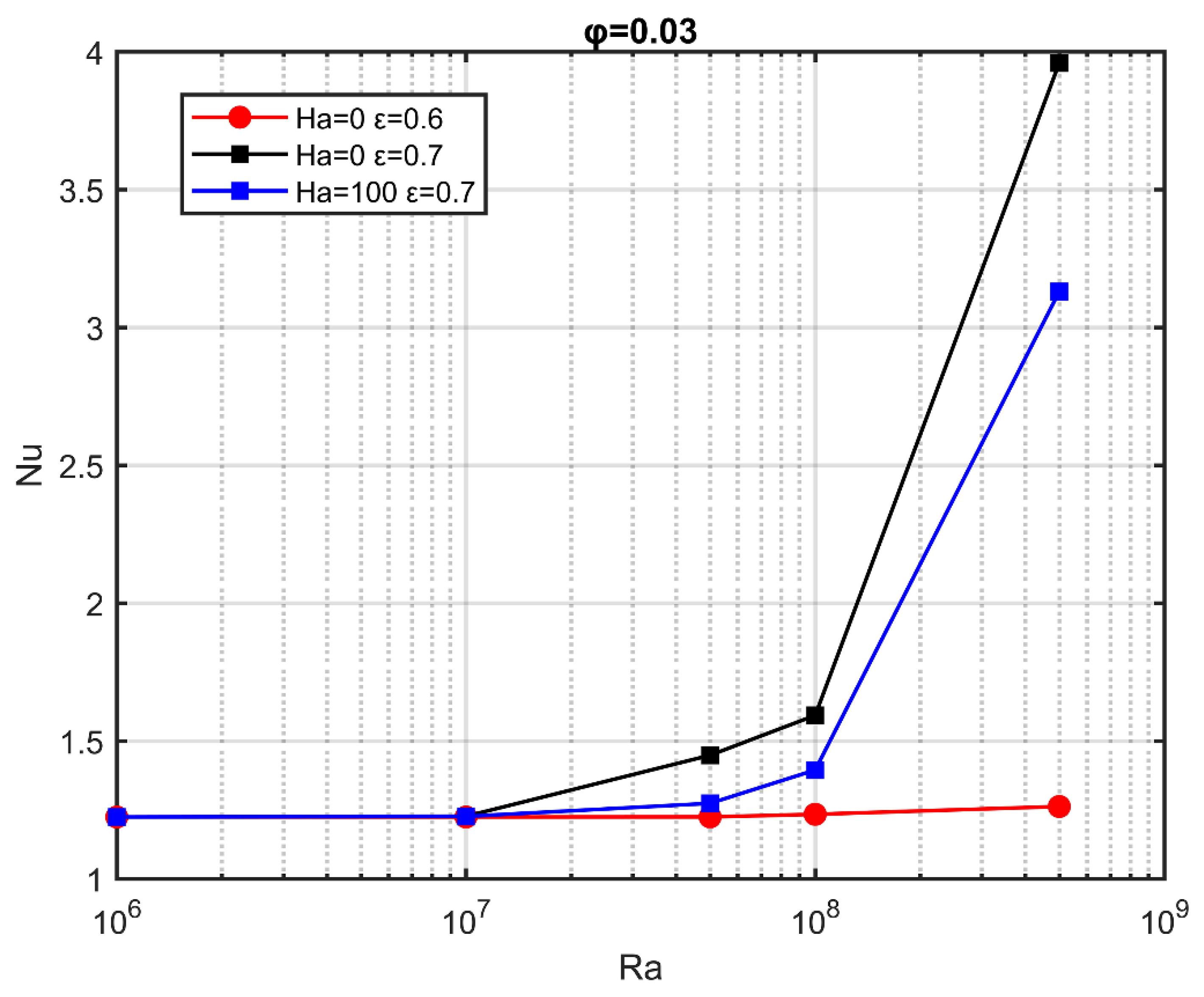
4.4. Effect of the Rayleigh Number (Ra)
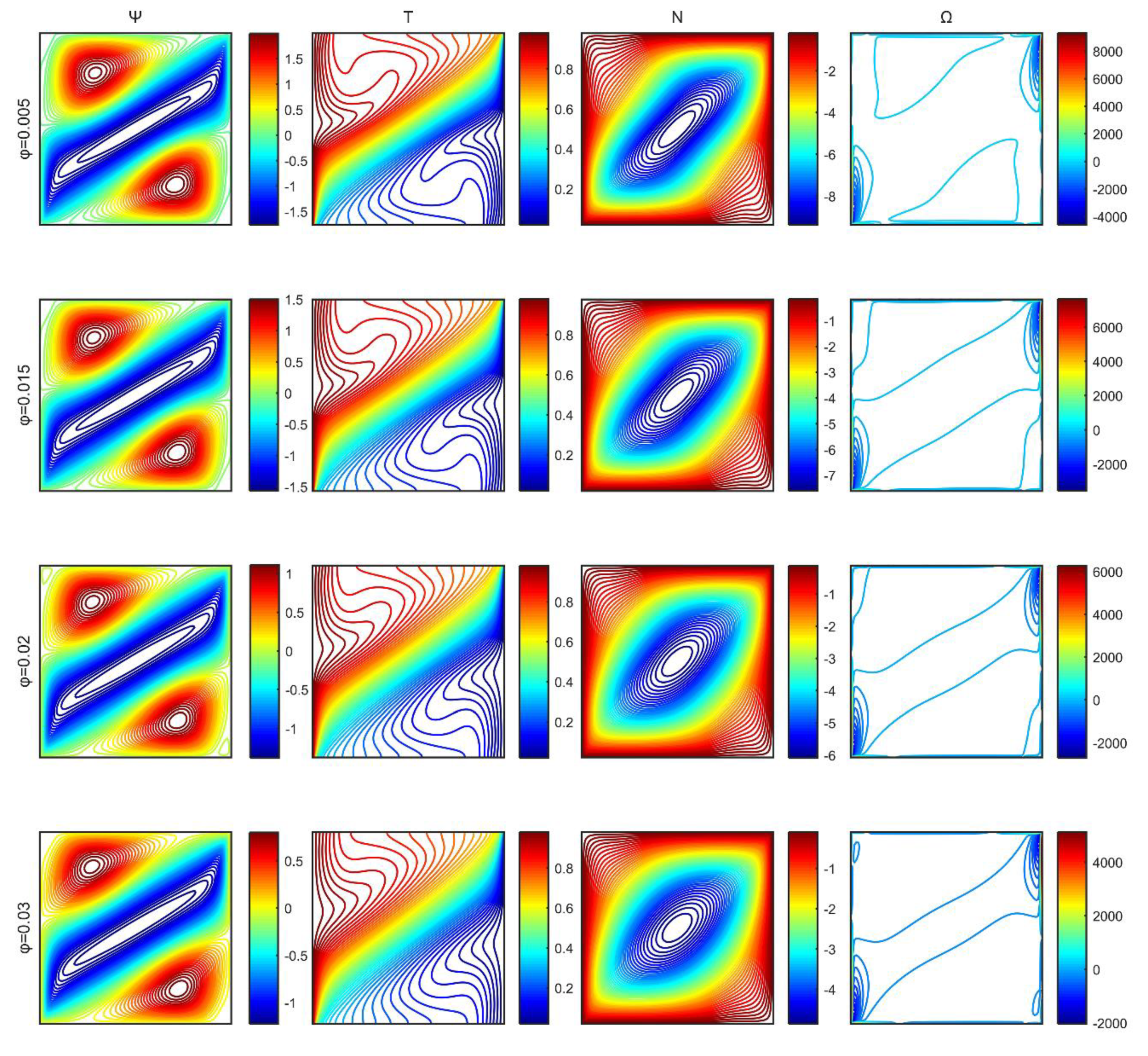
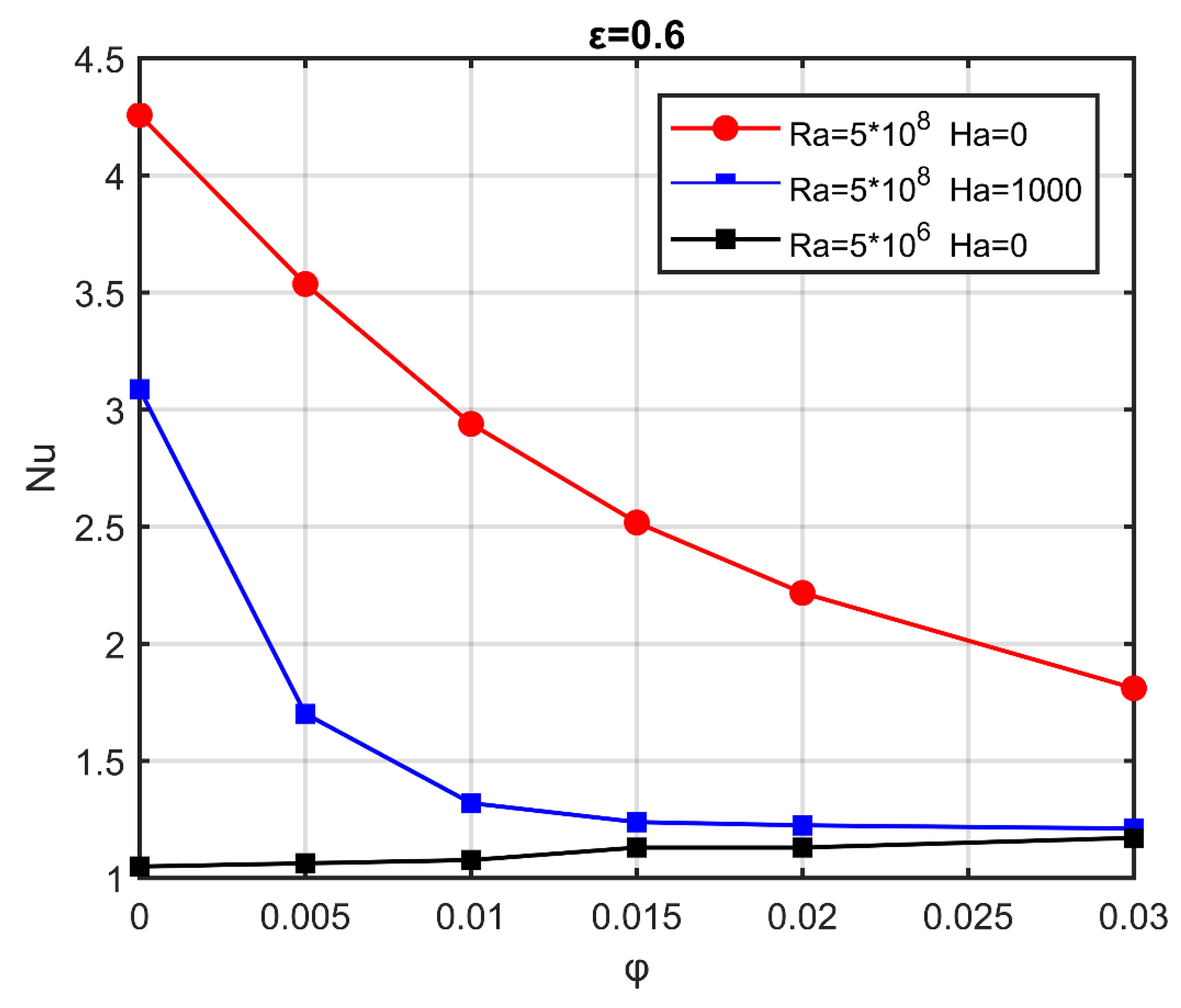
4.5. Effect of the Volume Fraction (φ)
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Melnikov, I.A.; Sviridov, E.V.; Sviridov, V.G.; Razuvanov, N.G. Experimental investigation of MHD heat transfer in a vertical round tube affected by transverse magnetic field. Fusion Eng. Des. 2016, 112, 505–512. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. MHD boundary layer slip flow along a stretching cylinder. Ain Shams Eng. J. 2013, 4, 317–324. [Google Scholar] [CrossRef]
- Jang, J.; Lee, S.S. Theoretical and experimental study of MHD (magnetohydrodynamic) micropump. Sens. Actuators A Phys. 2000, 80, 84–89. [Google Scholar] [CrossRef]
- Ostrach, S. Natural convection in enclosures. J. Heat Transf. 1988, 110, 1175–1190. [Google Scholar] [CrossRef]
- Jani, S.; Amini, M.; Mahmoodi, M. Numerical study of free convection heat transfer in a square cavity with a fin attached to its cold wall. Heat Transf. Res. 2011, 42. [Google Scholar] [CrossRef]
- Ghosh, B.C.; Ghosh, N. MHD flow of a visco-elastic fluid through porous medium. Int. J. Numer. Methods Heat Fluid Flow 2001, 11, 682–698. [Google Scholar] [CrossRef]
- Jha, B.K. Natural convection in unsteady MHD couette flow. Heat Mass Transf. 2001, 37, 329–331. [Google Scholar] [CrossRef]
- Duwairi, H.M.; Duwairi, R.M. Thermal radiation effects on MHD-Rayleigh flow with constant surface heat flux. Heat Mass Transf. 2004, 41, 51–57. [Google Scholar] [CrossRef]
- Di Piazza, I.; Ciofalo, M. MHD free convection in a liquid-metal filled cubic enclosure. I. Differential heating. Int. J. Heat Mass Transf. 2002, 45, 1477–1492. [Google Scholar] [CrossRef]
- Kenjereš, S.; Hanjalić, K. Numerical simulation of magnetic control of heat transfer in thermal convection. Int. J. Heat Fluid Flow 2004, 25, 559–568. [Google Scholar] [CrossRef]
- Kakaç, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Lee, J.-H.; Lee, S.-H.; Choi, C.; Jang, S.; Choi, S. A review of thermal conductivity data, mechanisms and models for nanofluids. Int. J. Micro Nano Scale Transp. 2011, 1, 269–322. [Google Scholar] [CrossRef]
- Eapen, J.; Rusconi, R.; Piazza, R.; Yip, S. The classical nature of thermal conduction in nanofluids. J. Heat Transf. 2010, 132. [Google Scholar] [CrossRef]
- Wong, K.V.; De Leon, O. Applications of nanofluids: Current and future. In Nanotechnology and Energy; Jenny Stanford Publishing: Singapore, 2017; pp. 105–132. [Google Scholar]
- Fan, J.; Wang, L. Review of heat conduction in nanofluids. J. Heat Transf. 2011, 133, 040801. [Google Scholar] [CrossRef]
- Putra, N.; Roetzel, W.; Das, S.K. Natural convection of nano-fluids. Heat Mass Transf. 2003, 39, 775–784. [Google Scholar] [CrossRef]
- Wen, D.; Ding, Y. Natural convective heat transfer of suspensions of titanium dioxide nanoparticles (nanofluids). IEEE Trans. Nanotechnol. 2006, 5, 220–227. [Google Scholar]
- Polidori, G.; Fohanno, S.; Nguyen, C.T. A note on heat transfer modelling of Newtonian nanofluids in laminar free convection. Int. J. Therm. Sci. 2007, 46, 739–744. [Google Scholar] [CrossRef]
- Mahmoudi, A.H.; Abu-Nada, E. Combined effect of magnetic field and nanofluid variable properties on heat transfer enhancement in natural convection. Numer. Heat Transf. Part A Appl. 2013, 63, 452–472. [Google Scholar] [CrossRef]
- Makinde, O.D. Effects of viscous dissipation and Newtonian heating on boundary-layer flow of nanofluids over a flat plate. Int. J. Numer. Methods Heat Fluid Flow 2013, 23, 1291–1303. [Google Scholar] [CrossRef]
- Jani, S.; Mahmoodi, M.; Amini, M.; Akbari, M. Free convection in rectangular enclosures containing nanofluid with nanoparticles of various diameters. 2014, 45, 145–169.
- Eringen, A.C. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Eringen, A.C. Microcontinuum field Theories: II. Fluent Media; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2001; Volume 2. [Google Scholar]
- Lukaszewicz, G. Micropolar Fluids: Theory and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Bourantas, G.C.; Loukopoulos, V.C. Modeling the natural convective flow of micropolar nanofluids. Int. J. Heat Mass Transf. 2014, 68, 35–41. [Google Scholar] [CrossRef]
- Baytas, A.C.; Pop, I. Free convection in oblique enclosures filled with a porous medium. Int. J. Heat Mass Transf. 1999, 42, 1047–1057. [Google Scholar] [CrossRef]
- Kramer, J.; Jecl, R.; Škerget, L. Boundary domain integral method for the study of double diffusive natural convection in porous media. Eng. Anal. Bound. Elem. 2007, 31, 897–905. [Google Scholar] [CrossRef]
- Kumari, M.; Nath, G. Unsteady natural convection from a horizontal annulus filled with a porous medium. Int. J. Heat Mass Transf. 2008, 51, 5001–5007. [Google Scholar] [CrossRef]
- Varol, Y.; Oztop, H.F.; Pop, I. Natural convection in a diagonally divided square cavity filled with a porous medium. Int. J. Therm. Sci. 2009, 48, 1405–1415. [Google Scholar] [CrossRef]
- Bourantas, G.C.; Skouras, E.D.; Loukopoulos, V.C.; Burganos, V.N. Heat transfer and natural convection of nanofluids in porous media. Eur. J. Mech. B Fluids 2014, 43, 45–56. [Google Scholar] [CrossRef]
- Oztop, H.F. Natural convection in partially cooled and inclined porous rectangular enclosures. Int. J. Therm. Sci. 2007, 46, 149–156. [Google Scholar] [CrossRef]
- Shehadeh, F.G.; Duwairi, H.M. MHD natural convection in porous media-filled enclosures. Appl. Math. Mech. 2009, 30, 1113–1120. [Google Scholar] [CrossRef]
- Kim, Y.J. Unsteady MHD convective heat transfer past a semi-infinite vertical porous moving plate with variable suction. Int. J. Eng. Sci. 2000, 38, 833–845. [Google Scholar] [CrossRef]
- Aminossadati, S.M.; Ghasemi, B. Natural convection cooling of a localised heat source at the bottom of a nanofluid-filled enclosure. Eur. J. Mech. B Fluids 2009, 28, 630–640. [Google Scholar] [CrossRef]
- Ganguly, S.; Sikdar, S.; Basu, S. Experimental investigation of the effective electrical conductivity of aluminum oxide nanofluids. Powder Technol. 2009, 196, 326–330. [Google Scholar] [CrossRef]
- Grobler, C.; Sharifpur, M.; Ghodsinezhad, H.; Capitani, R.; Meyer, J. Experimental study on cavity flow natural convection in a porous medium, saturated with an Al2O3 60% EG-40% Water nanofluid. In Proceedings of the 11th International Conference on Heat Transfer, Fluid Mechanics and Thermodynamics, Skukuza, South Africa, 20–23 July 2015; Volume 2015. [Google Scholar]
- Davidson, P.A. An Introduction to Magnetohydrodynamics; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Eringen, A.C. Simple microfluids. Int. J. Eng. Sci. 1964, 2, 205–217. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Malashetty, M.S.; Shivakumara, I.S.; Kulkarni, S. The onset of Lapwood—Brinkman convection using a thermal non-equilibrium model. Int. J. Heat Mass Transf. 2005, 48, 1155–1163. [Google Scholar] [CrossRef]
- Zadravec, M.; Hriberšek, M.; Škerget, L. Natural convection of micropolar fluid in an enclosure with boundary element method. Eng. Anal. Bound. Elem. 2009, 33, 485–492. [Google Scholar] [CrossRef]
- Ahmadi, G. Self-similar solution of imcompressible micropolar boundary layer flow over a semi-infinite plate. Int. J. Eng. Sci. 1976, 14, 639–646. [Google Scholar] [CrossRef]
- Rees, D.; Pop, I. Free convection boundary-layer flow of a micropolar fluid from a vertical flat plate. IMA J. Appl. Math. 1998, 61, 179–197. [Google Scholar] [CrossRef]
- Pak, B.C.; Cho, Y.I. Hydrodynamic and heat transfer study of dispersed fluids with submicron metallic oxide particles. Exp. Heat Transf. 1998, 11, 151–170. [Google Scholar] [CrossRef]
- Bourantas, G.; Skouras, E.; Loukopoulos, V.; Nikiforidis, G. Meshfree point collocation schemes for 2d steady state incompressible navier-stokes equations in velocity-vorticity formulation for high values of reynolds number. Comput. Model. Eng. Sci. 2010, 59, 31–63. [Google Scholar]
- Bourantas, G.C.; Skouras, E.D.; Loukopoulos, V.C.; Nikiforidis, G.C. An accurate, stable and efficient domain-type meshless method for the solution of MHD flow problems. J. Comput. Phys. 2009, 228, 8135–8160. [Google Scholar] [CrossRef]
- Bourantas, G.C.; Cheeseman, B.L.; Ramaswamy, R.; Sbalzarini, I.F. Using DC PSE operator discretization in Eulerian meshless collocation methods improves their robustness in complex geometries. Comput. Fluids 2016, 136, 285–300. [Google Scholar] [CrossRef]
- Zahab, Z.E.; Divo, E.; Kassab, A.J. A localized collocation meshless method (LCMM) for incompressible flows CFD modeling with applications to transient hemodynamics. Eng. Anal. Bound. Elem. 2009, 33, 1045–1061. [Google Scholar] [CrossRef]
- Schrader, B.; Reboux, S.; Sbalzarini, I.F. Discretization correction of general integral PSE Operators for particle methods. J. Comput. Phys. 2010, 229, 4159–4182. [Google Scholar] [CrossRef]
- Degond, P.; Mas-Gallic, S. The weighted particle method for convection-diffusion equations. I. The case of an isotropic viscosity. Math. Comput. 1989, 53, 485–507. [Google Scholar]
- Eldredge, J.D.; Leonard, A.; Colonius, T. A General deterministic treatment of derivatives in particle methods. J. Comput. Phys. 2002, 180, 686–709. [Google Scholar] [CrossRef]
- Weinan, E.; Liu, J.-G. Vorticity boundary condition and related issues for finite difference schemes. J. Comput. Phys. 1996, 124, 368–382. [Google Scholar]
- Bourantas, G.C.; Zwick, B.F.; Joldes, G.R.; Loukopoulos, V.C.; Tavner, A.C.; Wittek, A.; Miller, K. An explicit meshless point collocation solver for incompressible Navier-Stokes equations. Fluids 2019, 4, 164. [Google Scholar] [CrossRef]
- Ece, M.C.; Büyük, E. Natural-convection flow under a magnetic field in an inclined rectangular enclosure heated and cooled on adjacent walls. Fluid Dyn. Res. 2006, 38, 564–590. [Google Scholar] [CrossRef]



| Pure Water | 997.1 | 4179 | 0.613 | 21 | 3 |
| Alumina (Al2O3) | 3970 | 765 | 40 | 0.85 | |
| Glass spheres | 2800 | 13.96 | 0.7 | - |
| Gr | Nuavg (Ha = 0) | Nuavg (Ha = 100) |
|---|---|---|
| 103 | 3.745834 3.735471 | 3.678740 3.716622 |
| 104 | 4.771623 4.781083 | 3.680210 3.757865 |
| 105 | 6.677280 6.728638 | 3.885215 3.825346 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karagiannakis, N.P.; Bourantas, G.C.; Skouras, E.D.; Loukopoulos, V.C.; Miller, K.; Burganos, V.N. Modeling the Natural Convection Flow in a Square Porous Enclosure Filled with a Micropolar Nanofluid under Magnetohydrodynamic Conditions. Appl. Sci. 2020, 10, 1633. https://doi.org/10.3390/app10051633
Karagiannakis NP, Bourantas GC, Skouras ED, Loukopoulos VC, Miller K, Burganos VN. Modeling the Natural Convection Flow in a Square Porous Enclosure Filled with a Micropolar Nanofluid under Magnetohydrodynamic Conditions. Applied Sciences. 2020; 10(5):1633. https://doi.org/10.3390/app10051633
Chicago/Turabian StyleKaragiannakis, Nikolaos P., George C. Bourantas, Eugene D. Skouras, Vassilios C. Loukopoulos, Karol Miller, and Vasilis N. Burganos. 2020. "Modeling the Natural Convection Flow in a Square Porous Enclosure Filled with a Micropolar Nanofluid under Magnetohydrodynamic Conditions" Applied Sciences 10, no. 5: 1633. https://doi.org/10.3390/app10051633
APA StyleKaragiannakis, N. P., Bourantas, G. C., Skouras, E. D., Loukopoulos, V. C., Miller, K., & Burganos, V. N. (2020). Modeling the Natural Convection Flow in a Square Porous Enclosure Filled with a Micropolar Nanofluid under Magnetohydrodynamic Conditions. Applied Sciences, 10(5), 1633. https://doi.org/10.3390/app10051633






