A Probabilistic Capacity Model and Seismic Vulnerability Analysis of Wall Pier Bridges
Abstract
:1. Introduction
2. Finite Element Model of Wall Pier
3. Probabilistic Capability Model of Wall Piers
3.1. Wall Pier Damage Indexes
3.2. Probabilistic in-Plane Capability Model of Wall Piers
4. Seismic Vulnerability of Wall Pier Girder Bridges
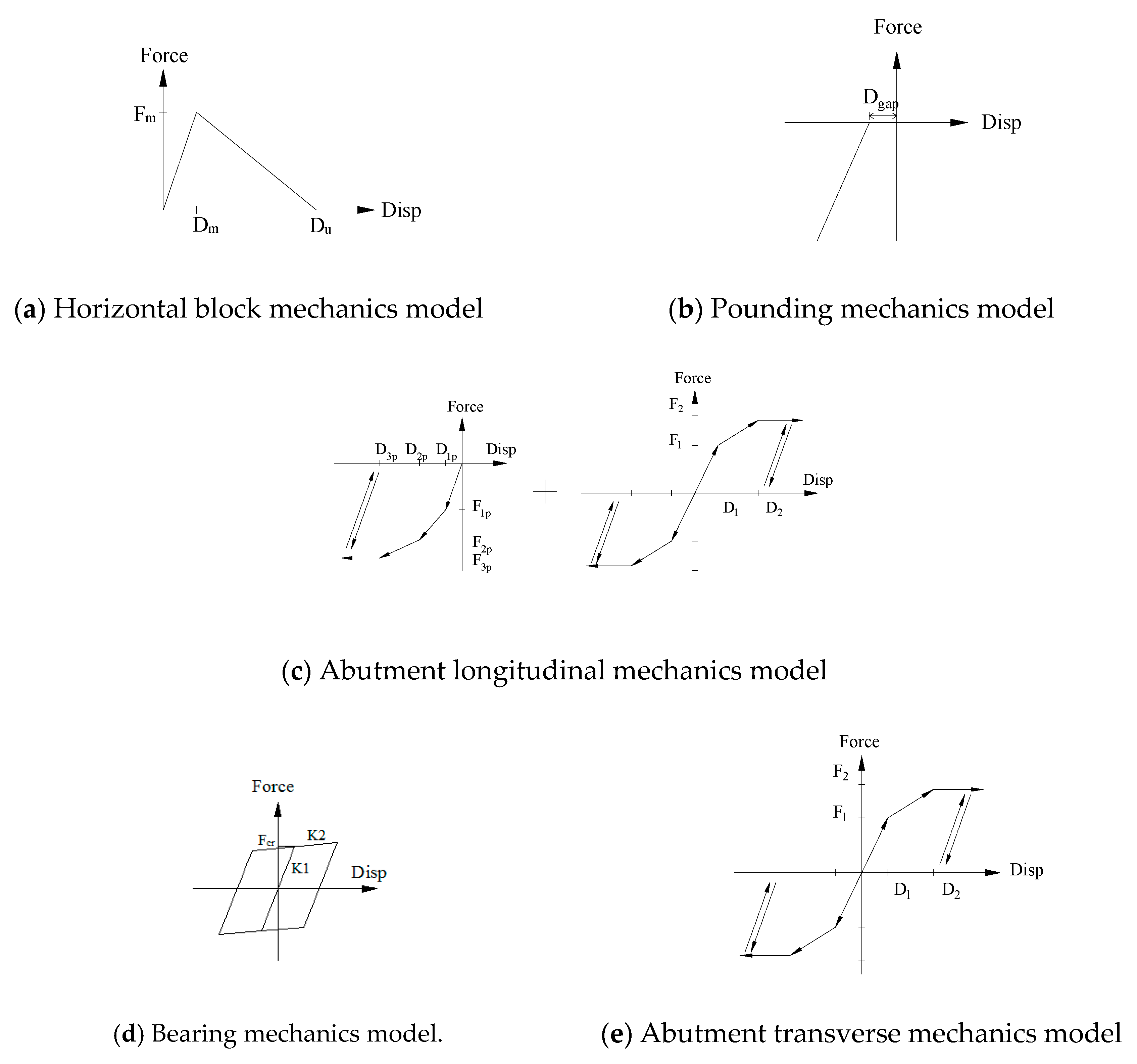
4.1. Bearing and Abutment Limit States
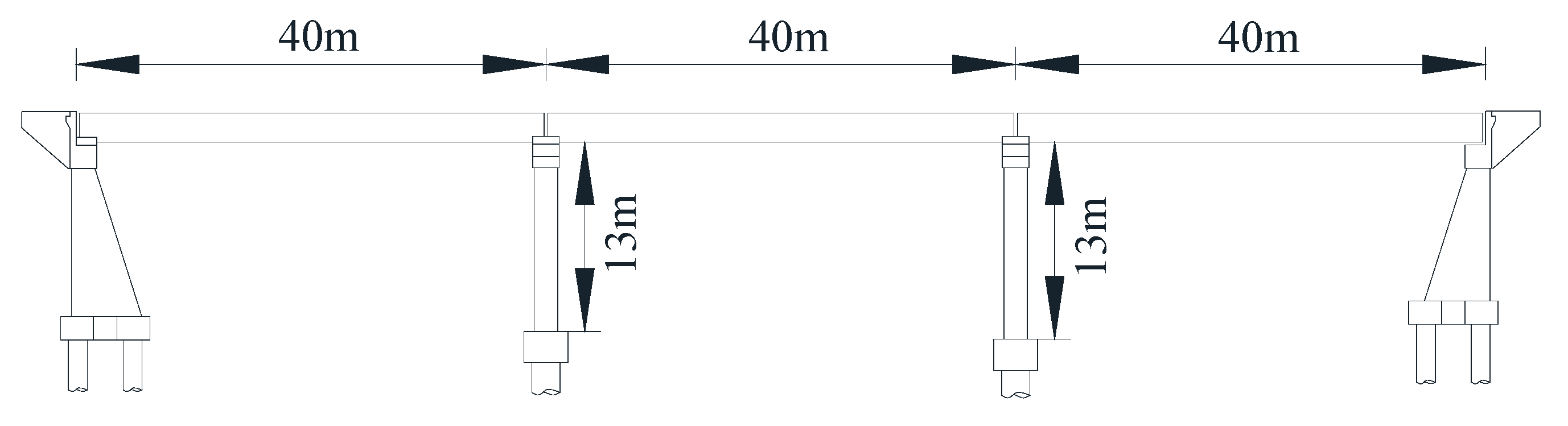
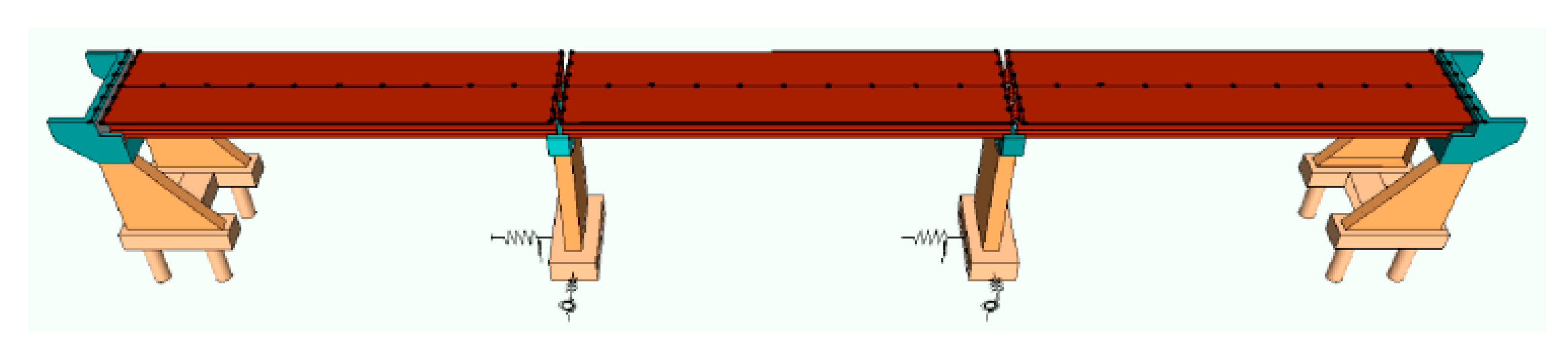
4.2. Bridge Sample Establishment
4.3. Time-History Analysis
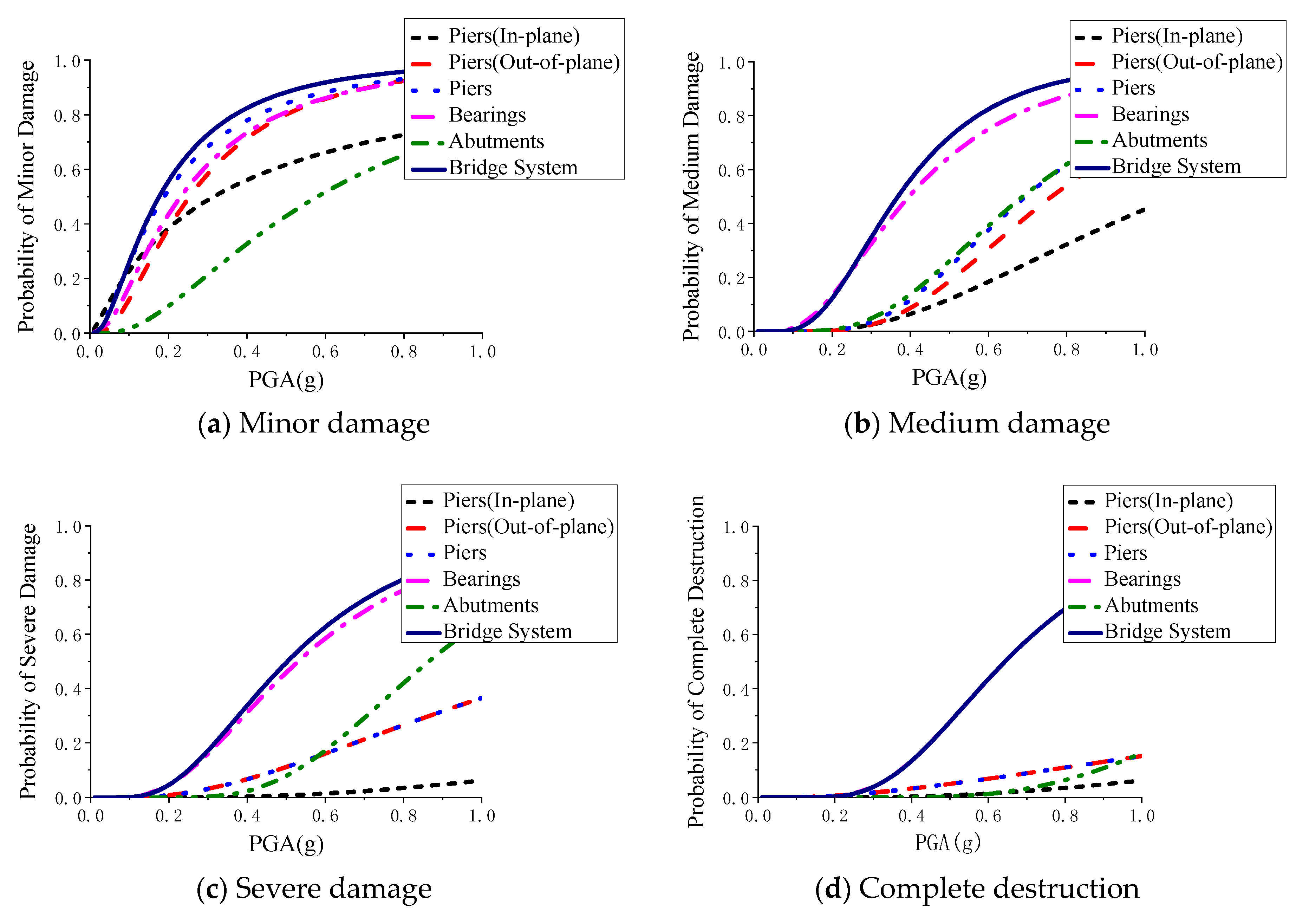
4.4. Seismic Vulnerability of Wall Pier Bridges
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bignell, J.L. Assessment of the Seismic Vulnerability of Wall Pier Supported Highway Bridges on Priority Emergency Routes in Southern Illinois. Ph.D. Dissertation, University of Illinois at Urbana-Champaign, Urbana, IL, USA, 2006. [Google Scholar]
- Bayat, M.; Daneshjoo, F.; Nistico, N. Probabilistic sensitivity analysis of multi-span highway bridges. Steel Compos. Struct. 2015, 1, 237–262. [Google Scholar] [CrossRef]
- Haroun, M.A.; Pardoen, G.C.; Shepherd, R.; Haggag, H.A.; Kazanjy, R.P. Cyclic Behavior of Bridge Pier Walls for Retrofit; University of California Irvine: Irvine, CA, USA, 1993. [Google Scholar]
- Aboutaha, R.S.; Engelhardt, M.D.; Jirsa, J.O.; Kreger, M.E. Rehabilitation of shear critical concrete columns by use of rectangular steel jackets. ACI Struct. J. 1999, 96, 68–78. [Google Scholar]
- Greifenhagen, C. Seismic Behavior of Lightly Reinforced Concrete Squat Shear Walls. Ph.D. Dissertation, Dresden University of Technology, Dresden, Germany, 2006. [Google Scholar]
- Hidalgo, P.A.; Ledezma, C.A.; Jordan, R.M. Seismic Behavior of Squat Reinforced Concrete Shear Walls. Earthq. Spectra 2002, 18, 287–308. [Google Scholar] [CrossRef]
- Baker, J.W. Efficient analytical fragility function fitting using dynamic structural analysis. Earthq. Spectra 2015, 31, 579–599. [Google Scholar] [CrossRef]
- Leborgne, M.R. Modeling the Post Shear Failure Behavior of Reinforced Concrete Columns. Ph.D. Dissertation, University of Texas at Austin, Austin, TX, USA, 2012. [Google Scholar]
- Sun, Z.; Li, H.; Wang, D.; SI, B. Discrimination criterion governing flexural-shear failure modes and improved seismic analysis model for RC bridge piers. China J. Highw. Transp. 2015, 28, 42–50. (In Chinese) [Google Scholar]
- Zhang, L.; Yang, H. Hysteresis shear models for shear-wall. World Inf. Earthq. Eng. 1999, 15, 9–16. (In Chinese) [Google Scholar]
- Orakcal, K. Nonlinear Modeling and Analysis of Slender Reinforced Concrete Walls. Ph.D. Dissertation, Department of Civil and Environmental Engineering, University of California Los Angeles, Los Angeles, CA, USA, 2004. [Google Scholar]
- Orakcal, K.; Conte, J.P.; Wallace, J.W. Flexural Modeling of Reinforced Concrete Structural Walls—Model Attributes. ACI Struct. J. 2004, 101, 688–698. [Google Scholar]
- Kolozvari, K.; Orakcal, K.; Wallace, J.W. Shear-Flexure Interaction Modeling of Reinforced Concrete Structural Walls and Columns under Reversed Cyclic Loading; Pacific Earthquake Engineering Research Center, University of California Berkeley: Berkeley, CA, USA, 2015; PEER Report No. 2015/12. [Google Scholar]
- Lu, X. Elasto-Plastic Analysis of Buildings Against Earthquake; China Architecture & Building Press: Beijing, China, 2015; pp. 62–63. (In Chinese) [Google Scholar]
- Zhang, H. Study on the Performance-based Seismic Design Method for Shear Wall Structures. Ph.D. Dissertation, Tongji University, Shanghai, China, 2007. (In Chinese). [Google Scholar]
- Berry, M.; Parrish, M.; Eberhard, M. PEER Structural Performance Database User’s Manual; Pacific Earthquake Engineering Research Center, University of California Berkeley: Berkeley, CA, USA, 2004; Available online: https://nisee.berkeley.edu/spd/ (accessed on 31 December 2019).
- FEWA; HAZUS-MH MR4. Technical Manual: Earthquake Model; Federal Emergency Management Agency: Washington, DC, USA, 2003. [Google Scholar]
- Sun, Y.; Zhuo, W.; Fang, Z. Definition and quantified description of seismic performance levels for regular bridges. J. Earthq. Eng. Eng. Vib. 2011, 31, 104–112. (In Chinese) [Google Scholar]
- Gardoni, P. Probabilistic Models and Fragility Estimates for Bridge Components and Systems. Ph.D. Dissertation, University of California Berkeley, Berkeley, CA, USA, 2002. [Google Scholar]
- Gelman, A.; Carlin, J.B.; Stern, H.S.; Dunson, D.B.; Vehtari, A.; Rubin, D.B. Bayesian Data Analysis, 3rd ed.; Chapman and Hall/CRC: New York, NY, USA, 2013. [Google Scholar]
- Zhu, L.; Elwood, K.J.; Haukaas, T. Classification and Seismic Safety Evaluation of Existing Reinforced Concrete Columns. J. Struct. Eng. 2007, 133, 1316–1330. [Google Scholar] [CrossRef]
- Japan Road Association. Specifications for Highway Bridges: Part V. Seismic Design; Maruzen: Tokyo, Japan, 2002. [Google Scholar]
- Zhang, J.; Huo, Y. Evaluating the effectiveness and optimum design of isolation devices for highway bridges using the fragility function method. Eng. Struct. 2009, 31, 1648–1660. [Google Scholar] [CrossRef]
- California Department of Transportation. Seismic Design Criterial Version 1.7; California Department of Transportation: Sacramento, CA, USA, 2013. [Google Scholar]
- Nielson, B.G. Analytical Fragility Curves for Highway Bridges in Moderate Seismic Zones. Ph.D. Dissertation, Georgia Institute of Technology, Atlanta, GA, USA, 2005. [Google Scholar]
- Xu, L.; Li, J. Effect of retainers on transverse seismic response of a standard continuous girder bridge. J. Highw. Transp. Res. Dev. 2013, 30, 53–59. (In Chinese) [Google Scholar]
- Xia, Q.; Luo, R. Bridge Impact Stiffness Values of Correction in the Earthquake. Open J. Transp. Technol. 2013, 2, 200–205. (In Chinese) [Google Scholar] [CrossRef]
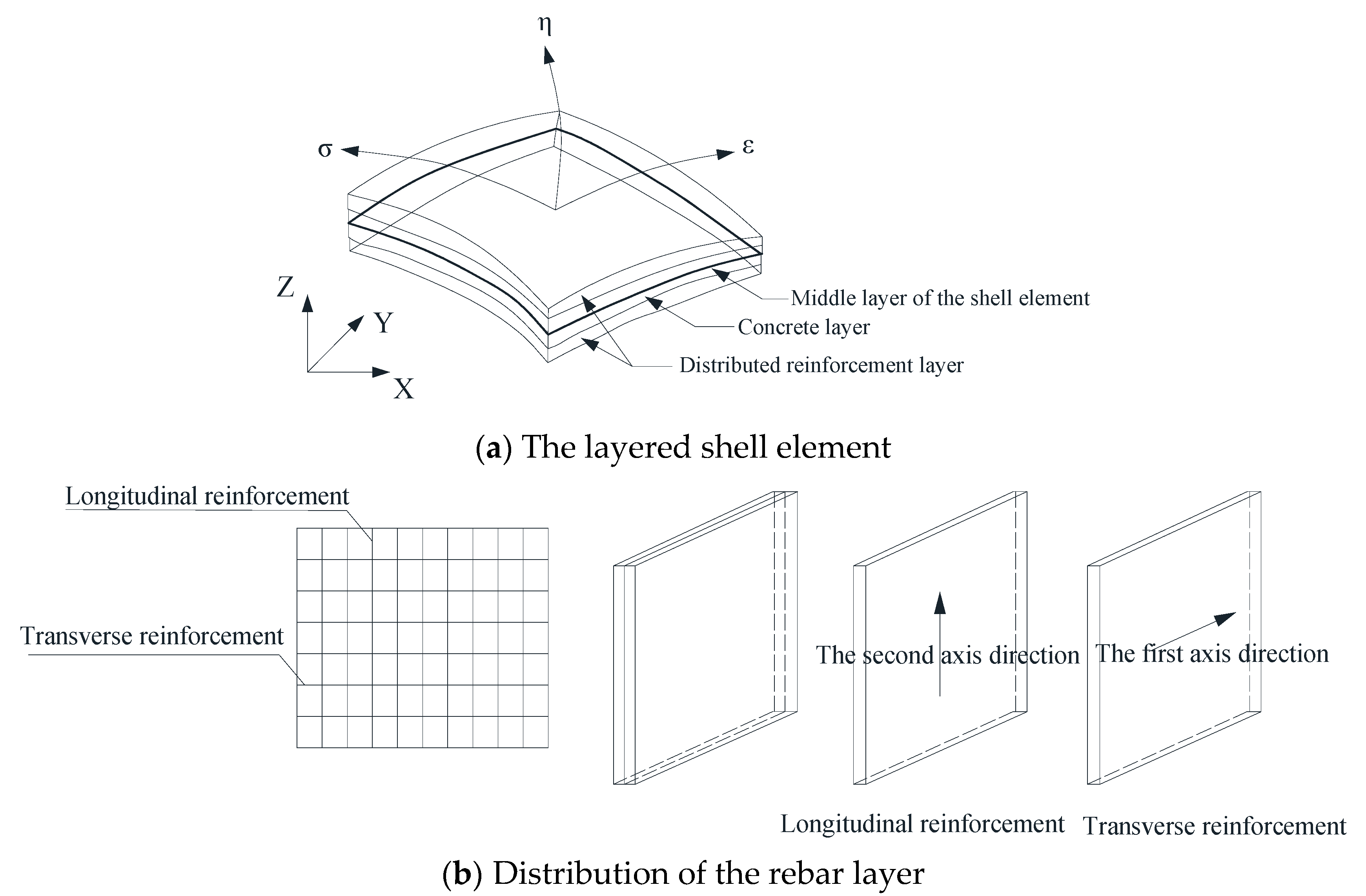

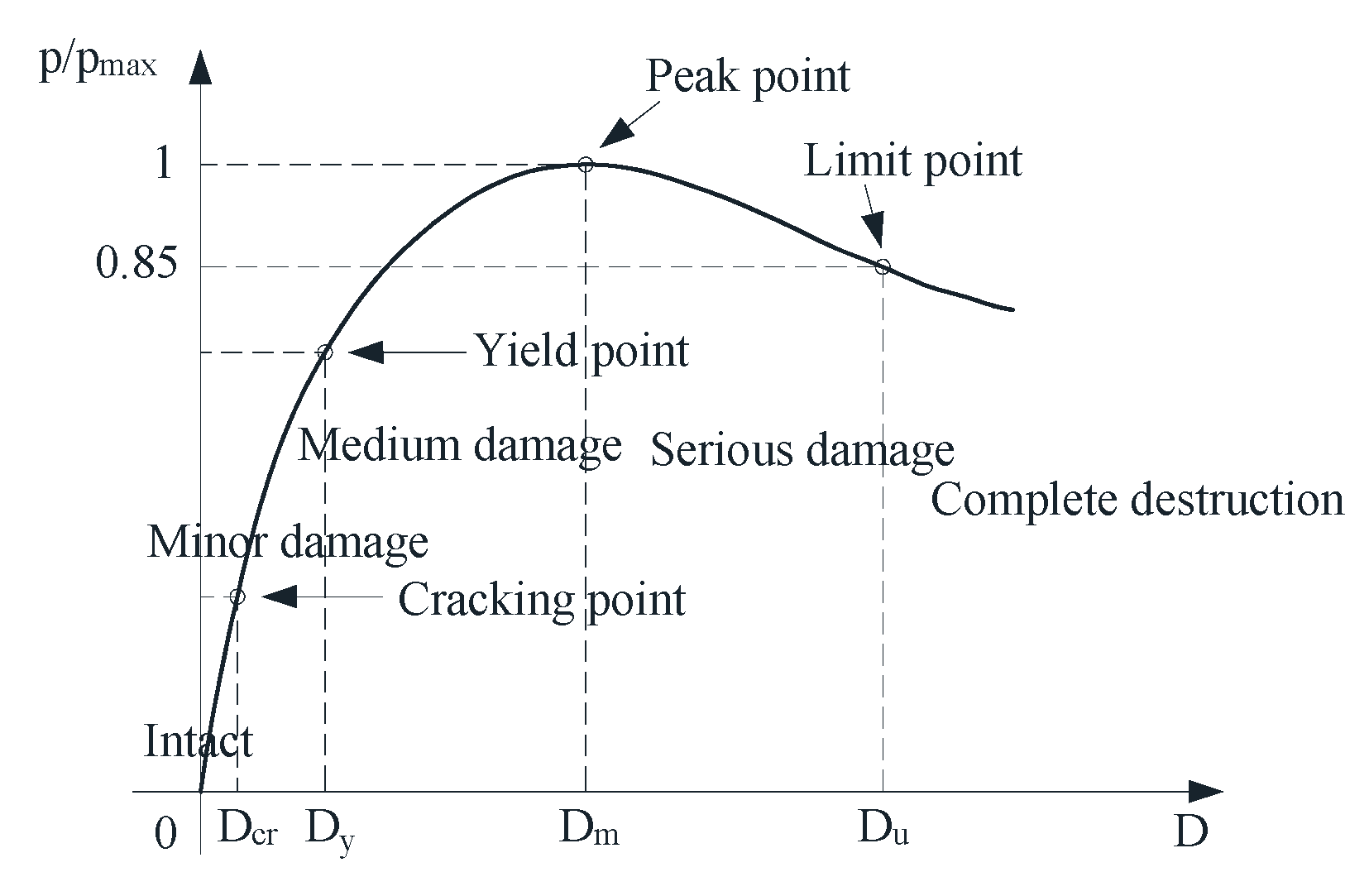
| Component Name | Section Size (cm) (Height × Width × Thickness) | Concrete Strength | Embedded Column Width (cm) | Axial Compression Ratio | Dark Column Reinforcement Ratio | Structural Reinforcement | Destruction Form | ||
|---|---|---|---|---|---|---|---|---|---|
| Vertical | Lateral | Vertical | Lateral | ||||||
| SW1-1 | 200 × 100 × 12.5 | C30 | 20 | 0.1 | 1.84% | 0.57% | 0.38% | 0.36% | Bending |
| SW2-1 | 100 × 100 × 12.5 | C40 | 20 | 0.3 | 1.84% | 0.57% | 0.38% | 0.36% | Shearing |
| Component Name | Section Size (cm) (Height × Width × Thick) | Concrete Strength (MPa) | Axial Compression Ratio | Reinforcement Ratio | Destruction Form |
|---|---|---|---|---|---|
| Park and Paulay | 178 × 60 × 40 | 26.9 | 0.1 | Vertical 1.88% Transverse 2.2% | bending damage |
| Wehbe | 234 × 61 × 38 | 27.2 | 0.098 | Vertical 2.22% Transverse 0.4% | bending damage |
| Wight03 | 88 × 31 × 15 | 26.1 | 0.147 | Vertical 2.45% Transverse 0.5% | bending and shearing |
| Wight04 | 88 × 31 × 15 | 26.1 | 0.147 | Vertical 2.45% Transverse 0.5% | bending and shearing |
| Performance Level | Degree of Damage | Seismic Performance Index |
|---|---|---|
| level 1 | Intact | MDR ≤ 0.11% |
| level 2 | Minor damage | 0.11% < MDR ≤ 0.38% |
| level 3 | Medium damage | 0.38% < MDR ≤ 0.84% |
| level 4 | Serious damage | 0.84% < MDR ≤ 2.23% |
| level 5 | Complete destruction | MDR > 2.23% |
| Level | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Aspect ratio | 3.2 | 3.8 | 4.4 | 5 |
| Pier width (m) | 4 | 5.33 | 6.67 | 8 |
| Vertical reinforcement ratio (%) | 0.5 | 0.63 | 0.77 | 0.9 |
| Lateral reinforcement ratio (%) | 0.2 | 0.27 | 0.33 | 0.4 |
| Axial pressure ratio | 0.02 | 0.04 | 0.06 | 0.08 |
| Shear span ratio | 1.6 | 2.07 | 2.53 | 3 |
| Reinforcement grade | HRB400 | HRB335 | — | — |
| Concrete marking | C40 | C35 | — | — |
| Performance Level | Damage State | Allowable Quantification of Shear Strain |
|---|---|---|
| level Ⅰ | intact | γα < 100% |
| level Ⅱ | minor damage | 100% ≤ γα < 150% |
| level Ⅲ | medium damage | 150% ≤ γα < 200% |
| level Ⅳ | serious damage | 200% ≤ γα < 250% |
| level Ⅴ | complete destruction | γα ≥ 250% |
| Performance Level | Damage State | Abutment Limit Displacement Quantification (mm) |
|---|---|---|
| level Ⅰ | intact | Δ < 25 |
| level Ⅱ | minor damage | 25 ≤ Δ < 50 |
| level Ⅲ | medium damage | 50 ≤ Δ < 100 |
| level Ⅳ | serious damage | 100 ≤ Δ < 150 |
| level Ⅴ | complete destruction | Δ ≥ 150 |
| Uncertainty Parameter | Distribution Type | Distribution Parameter | |
|---|---|---|---|
| α | β | ||
| C35 concrete compressive strength (MPa) | normal distribution | 35 | 4.5 |
| HRB335 steel yield strength (MPa) | logarithmic normal distribution | 5.81 | 0.1 |
| abutment initial stiffness (kN/mm/m) | uniform distribution | 11.5 | 28.5 |
| Scale factor of horizontal resistance coefficient (kN/m4) | uniform distribution | 60000 | 100000 |
| damping ratio | normal distribution | 0.045 | 0.0125 |
| vertical reinforcement ratio of piers (%) | uniform distribution | 0.55 | 0.85 |
| transverse reinforcement ratio (%) | uniform distribution | 0.2 | 0.4 |
| pier height (m) | uniform distribution | 11 | 16 |
| expansion joint width (cm) | normal distribution | 8 | 0.5 |
| shear elastic modulus (MPa) | normal distribution | 1.18 | 0.16 |
| Type of Ground Motions | Types | Fault Distance (R) | Intensity Magnitude | PGV/PGA |
|---|---|---|---|---|
| near field | pulse type | 0~20km | 6~8 | >0.15 |
| far field | non-pulse type | 20~100km | 6~8 | ≤0.15 |
| Component | Minor Damage | Medium Damage | Severe Damage | Complete Destruction | ||||
|---|---|---|---|---|---|---|---|---|
| Median Value (g) | Logarithmic Standard Deviation | Median Value (g) | Logarithmic Standard Deviation | Median Value (g) | Logarithmic Standard Deviation | Median value (g) | Logarithmic Standard Deviation | |
| Pier (out of plane) | 0.2541 | 0.8003 | 0.7618 | 0.4712 | 1.3108 | 0.7875 | 3.1159 | 1.1027 |
| Pier (in plane) | 0.316 | 1.5414 | 1.0808 | 0.6534 | 3.3743 | 0.7875 | 3.3743 | 0.7875 |
| Pier (overall) | 0.1892 | 0.9701 | 0.695 | 0.463 | 1.3108 | 0.7875 | 3.1159 | 1.1027 |
| Bearing | 0.2312 | 0.8775 | 0.3966 | 0.6135 | 0.5306 | 0.5739 | 0.6435 | 0.4278 |
| Abutment | 0.5759 | 0.8253 | 0.6877 | 0.4962 | 0.8648 | 0.3866 | 1.4911 | 0.4057 |
| Bridge system | 0.1767 | 0.8758 | 0.3682 | 0.5241 | 0.5031 | 0.5449 | 0.6435 | 0.4278 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Tu, Y.; He, L. A Probabilistic Capacity Model and Seismic Vulnerability Analysis of Wall Pier Bridges. Appl. Sci. 2020, 10, 926. https://doi.org/10.3390/app10030926
Chen L, Tu Y, He L. A Probabilistic Capacity Model and Seismic Vulnerability Analysis of Wall Pier Bridges. Applied Sciences. 2020; 10(3):926. https://doi.org/10.3390/app10030926
Chicago/Turabian StyleChen, Libo, Yi Tu, and Leqia He. 2020. "A Probabilistic Capacity Model and Seismic Vulnerability Analysis of Wall Pier Bridges" Applied Sciences 10, no. 3: 926. https://doi.org/10.3390/app10030926
APA StyleChen, L., Tu, Y., & He, L. (2020). A Probabilistic Capacity Model and Seismic Vulnerability Analysis of Wall Pier Bridges. Applied Sciences, 10(3), 926. https://doi.org/10.3390/app10030926





