1. Introduction
Since the pioneering experiment by Toms [
1], it is known that the additions of small (parts per million) amounts of long-chain flexible polymers to a turbulent flow can drastically reduce the transport energy by decreasing the turbulent drag. The effects are most evident in turbulent shear flow, in which dissolving the polymers in solution can reduce friction losses by as much as 80% compared to the solvent alone [
2]. After the discovery of the drag reduction (DR) phenomena, several comprehensive studies were carried out to understand the physical mechanisms of the interactions between the turbulent structures and polymer chains. Early comprehensive studies in this area come from Lumley [
3,
4], Hoyt [
5] and Virk [
6]. Lumley suggests that the DR phenomenon is the result of an increase in effective viscosity in an area outside the viscous sub-layer and in the buffer layer, caused by polymer chains stretching in a turbulent flow.
More recent studies have been proposed for the theory of the mechanisms of drag-reducing polymer additives [
7,
8]. Several Direct Numerical Simulation (DNS) studies were conducted to understand further the energy exchanges between the polymer chains and turbulence structures [
9,
10,
11,
12,
13,
14]. It is now known to at least low to moderate levels of DR, that the mechanism is the suppression of the near-wall streamwise vortices by polymers that stretch in the extensional flow, and then relax as they are rolled into other vortices, generating forces that tend to weaken these vortices. Quantitatively, this can be expressed as a polymer body force [
9], which is positive in the streamwise direction, with an opposite sign (anti-correlation) in the wall direction.
DNS is a great resource to explore the underlying mechanics of drag-reducing viscoelastic turbulent flows. However, for the majority of engineering motivations, DNS is not practical because of the high number of variables which requires a substantial expense of memory and CPU-time. This cost is more prevalent in high DR (HDR) schemes in which the near-wall velocity streaks become more elongated, requiring an increased demand on computational resources.
An alternative approach in capturing flow features at much less computational demands is the application of Reynolds-averaged Navier–Stokes (RANS) models, whose interest has increased in recent years. One of the original implementations of elastic effects within turbulence models was achieved by Pinho [
15] and Cruz et al. [
16]. Their work focused on low-Reynolds number
turbulence models, applying a Generalised Newtonian Fluid (GNF) constitutive equation involving dependency of the fluid strain hardening on the third invariant of the rate of deformation tensor. Following these studies, an anisotropic version was also developed which included an increased Reynolds stress anisotropy [
17], along with a Reynolds stress turbulence model [
18], both able to satisfactorily predict drag-reducing behavior. Nevertheless, the models are constrained because of the inelastic formulation of the GNF constitutive equations.
Further developments in viscoelastic RANS models became possible owing to the emergence of DNS data regarding turbulent viscoelastic fluids. The first elastic model was developed by Leighton et al. [
19], which was based on the finitely extensible nonlinear elastic with Peterlin closure (FENE-P) dumbbell constitutive equation model. Their study involved the development of a polymer strain–stress coupling based on the tensor expansion, which incorporated the conformation tensor and Reynolds stress. From this work, more attention arose to the FENE-P model given the molecular roots of the equations. Later, based on a-priori analysis of DNS data, Pinho et al. [
20] developed a low-Reynolds number
model for FENE-P fluids which could predict flow features up to the low drag reduction regime (LDR < 20%). Turbulent viscoelastic closures were proposed, including the non-linear term involving the conformation tensor and the strain rate fluctuations within the conformation tensor equation (denoted
following the nomenclature of Housiadas et al. [
21] and Li et al. [
22]); along with the viscoelastic turbulent transport term of the turbulent kinetic energy. One of the key difficulties that arose in this initial study was the decrease in the magnitude of turbulent kinetic energy as viscoelasticity increased, opposite to that found in the DNS literature [
23]. Subsequently, the model closures were improved by Resende et al. [
24] and the capacity of the model predictions were extended to the intermediate drag reduction regime (20% < IDR < 40%). However, the model closures involved complex damping functions and model constants which gave spurious results for the high drag reduction regime (HDR > 40%). Resende et al. [
25] applied the same viscoelastic closures to a low-Reynolds number
model with only a mathematical transformation of the governing terms involving
. The closures had identical limitations as the
model for predicting DR behaviour but demonstrated great versatility and robustness given its application to alternative two-equation models.
During this time, a
model for FENE-P fluids in fully developed channel flow was proposed by Iaccarino et al. [
26], following the initial studies of Dubief et al. [
27]. They introduced the idea of a turbulent polymer viscosity which accounts for the effects of viscoelasticity and turbulence on the polymer stress within the momentum equation. The reduction in the Reynolds shear stress is assumed by a-priori DNS data analysis from the decreasing
shown within the DNS studies [
9]. The model closure for the
is much simpler than the one developed by Resende et al. [
24], but contains only the trace and not the individual components. The model was later improved by Masoudian et al. [
28] and can predict flow features up to maximum DR (MDR). The key advancement of the closures were an
closure based on DNS analysis and comparisons to the local eddy viscosity peaks; the viscoelastic stress work in the turbulent kinetic energy equations; viscoelastic stress in the momentum equation; and a viscoelastic destruction term in the dissipation transport equation. The viscoelastic turbulent closures within the
equation (transverse viscoelastic stress work,
) should be strictly a function of
, which is a key component in the formulation of an effective polymer viscosity. However, because only the trace of the
term is present within the model, the closure had to be formulated using DNS analysis of alternative parameters.
Subsequently, after this study, a second-order Reynolds stress model for FENE-P fluids was proposed by Masoudian et al. [
29], extending on the idea of a correlation between the Reynolds stresses and the
components, similar to Leighton et al. [
19]. The model can predict all DR regimes but is generally unattractive due to the higher number of Newtonian terms resulting from higher-order modeling. Masoudian et al. [
30] then further improved the
model capabilities via the
term by introducing a simple extension to include heat transfer, along with removing wall dependence via the friction velocity. There are concerning features when one examines the Bousinesq-type
term, which has a zero
component, along with an opposite sign for
, both terms being crucial for the polymer shear stress in the momentum balance (see Appendix 1 in Pinho et al. [
20]). Further, the increase of
k in the buffer layer is small, meaning the decoupling of the
component may not be enough to decrease the eddy viscosity. This is compensated by an opposite trend in the dissipation rate,
, for increasing DR, which subsequently balances the momentum equations and causes the necessary increase in the velocity profiles.
An alternative approach in predicting DR flow features other than the use of higher-order models such as the
and Reynolds stress models mentioned previously, is that of a modified damping function or polymer eddy viscosity, accounting for the effect the polymer has on reducing the Reynolds shear stress. Tsukahara and Kawaguchi [
31] proposed a modified damping function for a low-Reynolds
model for fluids described by the Giesekus constitutive equation, following the same ideas as Pinho [
15] and Cruz. The closure was developed based on the energy-dissipative range and the dynamic characterization of the viscoelastic fluid. The model successfully captures the increase in the magnitude of the turbulent kinetic energy, along with the shift through the buffer layer. Although the magnitudes of
k are largely over-predicted in many cases, which is counterbalanced by a lack of closure for the viscoelastic destruction term. In some instances, the model predicts a DR of
with a DNS result of
. Resende et al. [
32] proposed a modified damping function for a low-Reynolds number
model for FENE-P fluids which can capture the increase of turbulent kinetic energy as flow viscoelasticity is increased, improving on model predictions made previously by Pinho et al. [
20] and Resende et al. [
24]. The study also improved largely on the
closure accuracy and simplicity formulated in the previous work. The model is able to predict flow features for a large range of rheological parameters but is limited to a friction Reynolds number of
, along with friction velocity still present in the model. For model applicability in flows with reattachment, the friction velocity dependence poses a problem as the values become null at these points and lead to spurious results or floating point errors within computational solvers.
In the present study, an improved
model for FENE-P fluids is proposed, validated for all drag reduction regimes (low, intermediate, high) and up to the largest friction Reynolds number (
) available in the DNS data. The important contribution to the current model is improved and simplified
term that removes complexity from the most recent model developed by Resende et al. [
32]; along with a modified damping function which accurately predicts the viscoelastic contributions near and away from the wall, effectively reducing the eddy viscosity and thickening the buffer-layer as DR increases. Further, a reformulation of the viscoelastic destruction term,
, which removes all friction velocity present in the previous
models. The model is assessed against DNS data covering a wide range of flow conditions in terms of the friction Weissenberg number,
, maximum polymer extension,
, viscosity ratio,
, and friction Reynolds number,
; along with comparisons against other turbulent FENE-P models within the literature.
The paper is organized as follows:
Section 2 introduces the instantaneous and time-averaged governing equations and identifies the viscoelastic terms that will require modeling;
Section 3 explains in detail the development of the viscoelastic turbulent closures;
Section 4 summarises the model;
Section 5 gives the numerical procedure applied;
Section 6 presents the results of the flow fields in fully developed channel flow, covering all range of DR and flow conditions; and finally in
Section 7, the main conclusions are presented.
2. Governing Equations
The governing equations for incompressible turbulent flow of dilute polymer solutions are the continuity and momentum equations respectively:
where the hat represents instantaneous quantities of velocity
, pressure
, stress tensor
, and fluid density
. The stress tensor is the sum of the Newtonian solvent which obeys Newton’s law of viscosity,
, with
representing the solvent viscosity coefficient, and polymeric contributions,
,
The kinematic viscosity is used alternatively throughout this study and is defined as
. The instantaneous rate of strain tensor,
, is defined as
The instantaneous polymer contributions are based on the FENE-P rheological dumbbell model [
33], with closure given by
with
known as the Peterlin function, and
is the trace of the instantaneous conformation tensor. The other parameters that are associated with the FENE-P model are:
, the relaxation time of the polymeric fluid;
, the maximum extensibility of the dumbbell model; and
, the polymer viscosity coefficient.
The behaviour of the instantaneous conformation tensor follows a hyperbolic differential equation of the form,
The Oldroyd’s upper convective derivative of the instantaneous conformation tensor is here denoted with . The local and advective derivatives are the first and second terms respectively. The bracketed term accounts for the effect of polymer stretching by the instantaneous flow.
The Reynolds averaging process [
34] is applied to the governing equations via a Reynolds decomposition of the flow fields such that,
; where the use of overbars or upper-case represents the averaged quantity; and primes or lower-case represent the instantaneous quantities. The continuity and momentum equations now take the form:
referred to as the Reynolds-averaged Navier–Stokes (RANS). The Reynolds stress tensor is
and requires a closure model. The Reynolds-averaged polymer stress is
and written fully as
where the additional term on the right requires a closure. The Peterlin function becomes
After Reynolds averaging, the instantaneous conformation tensor equation becomes
which is referred to as the Reynolds-averaged conformation evolution (RACE).
is the mean flow distortion term; it is non-zero, but requires no closure. The remaining two terms are named following the nomenclature of Li et al. [
22] and Housiadas et al. [
21]. They are labelled with
; representing the contribution to the transport of the conformation tensor due to the fluctuating advective terms; and
, which accounts for the interactions between the fluctuating components of the conformation tensor and the velocity gradient tensor.
Following the analysis of Pinho et al. [
20], the nonlinear fluctuating correlation of the average polymeric stress,
in Equation (
10) was shown to be negligible for LDR and HDR when compared with the linear part. This was later neglected in the models of Resende et al. [
24] and Masoudian et al. [
28] and is also neglected here. The
term can also be omitted for all DR regimes following a budget analysis of the RACE carried out by Housiadas et al. [
21] and Li et al. [
22]. The
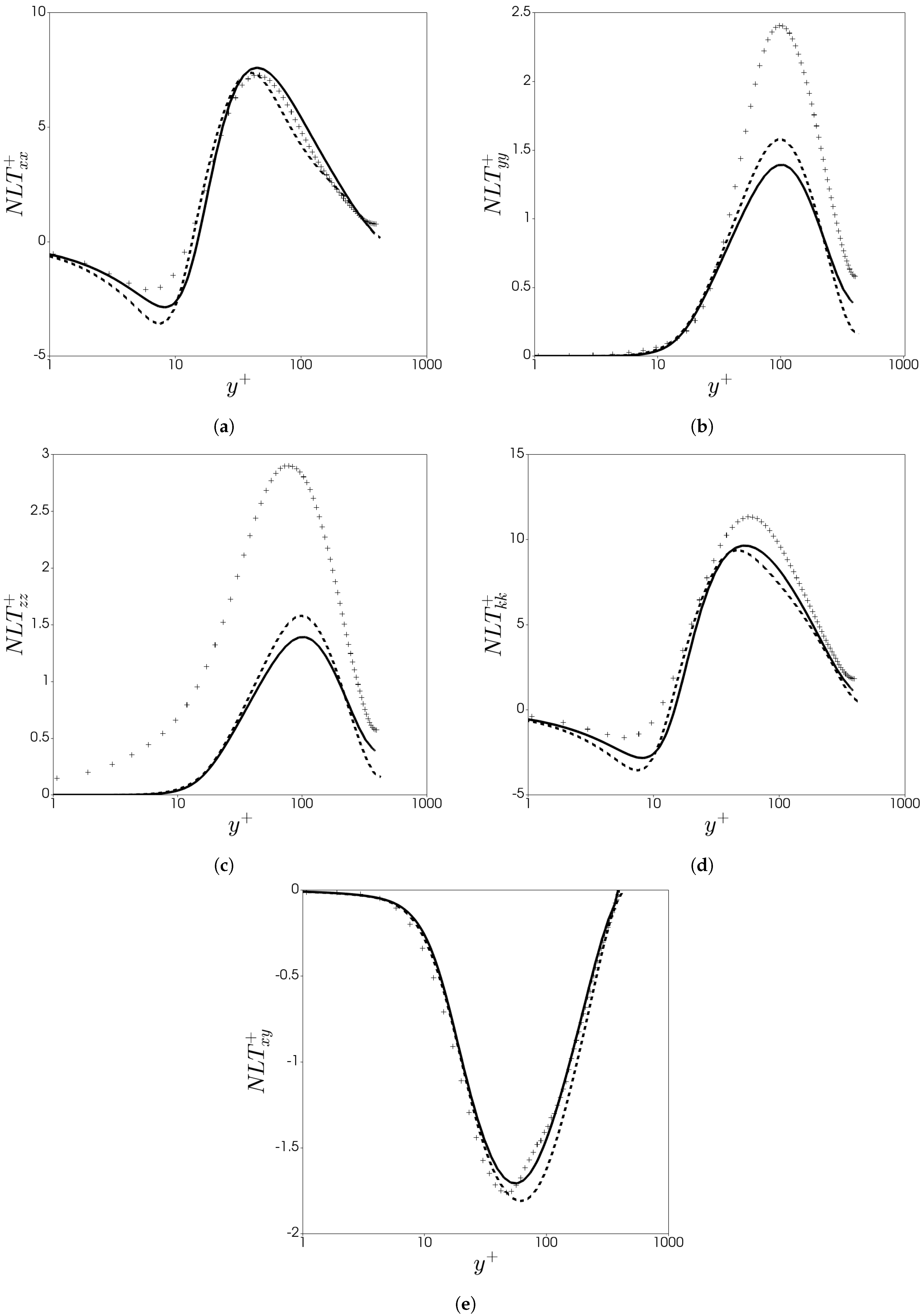
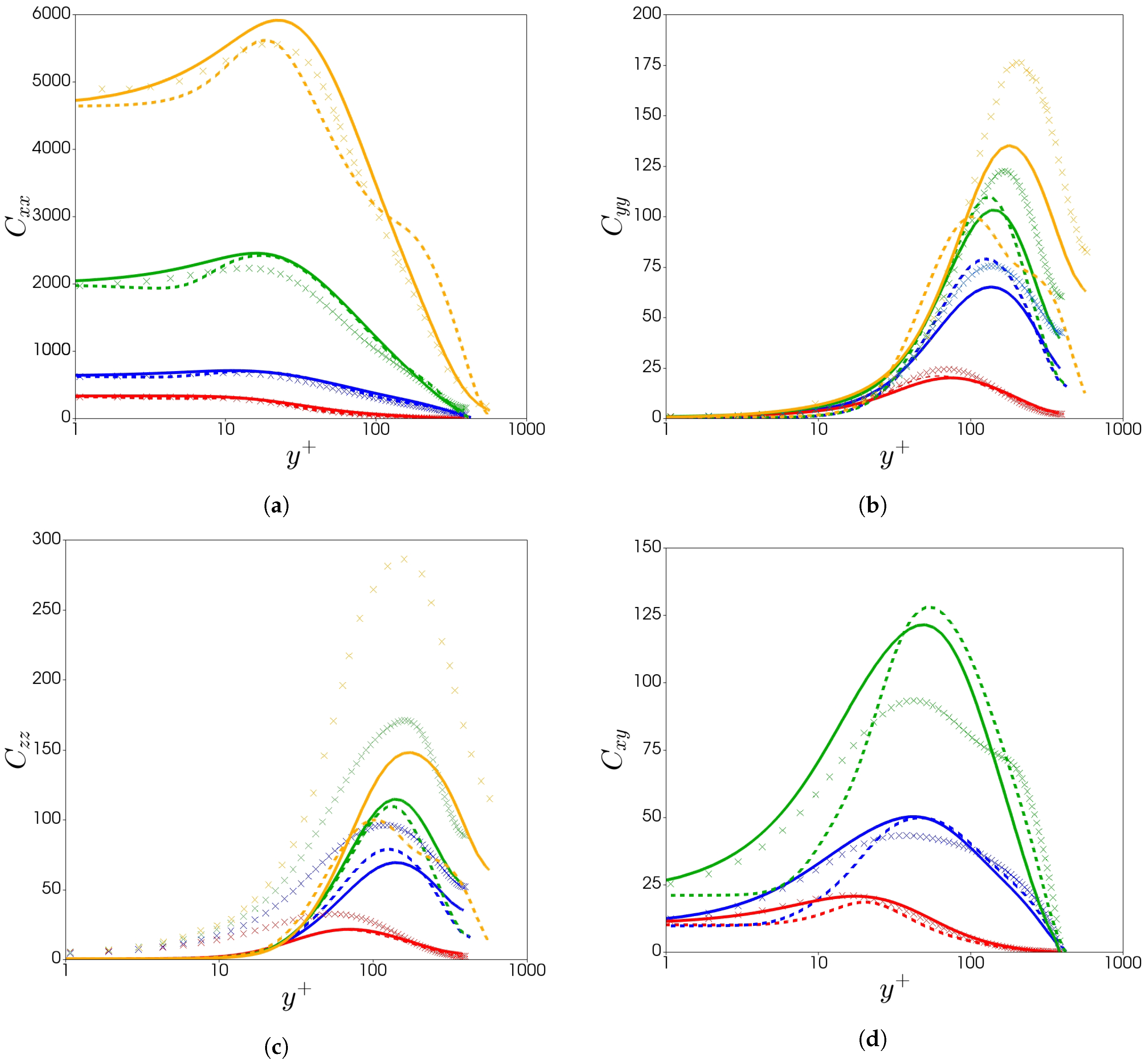
term cannot be neglected since it is a significant contributor to the RACE and therefore requires a suitable closure.
2.1. Model for the Reynolds Stress Tensor
The Reynolds stress tensor is computed by adopting the Boussinesq turbulent stress strain relationship,
where
k is the turbulent kinetic energy,
is the mean rate of strain tensor and
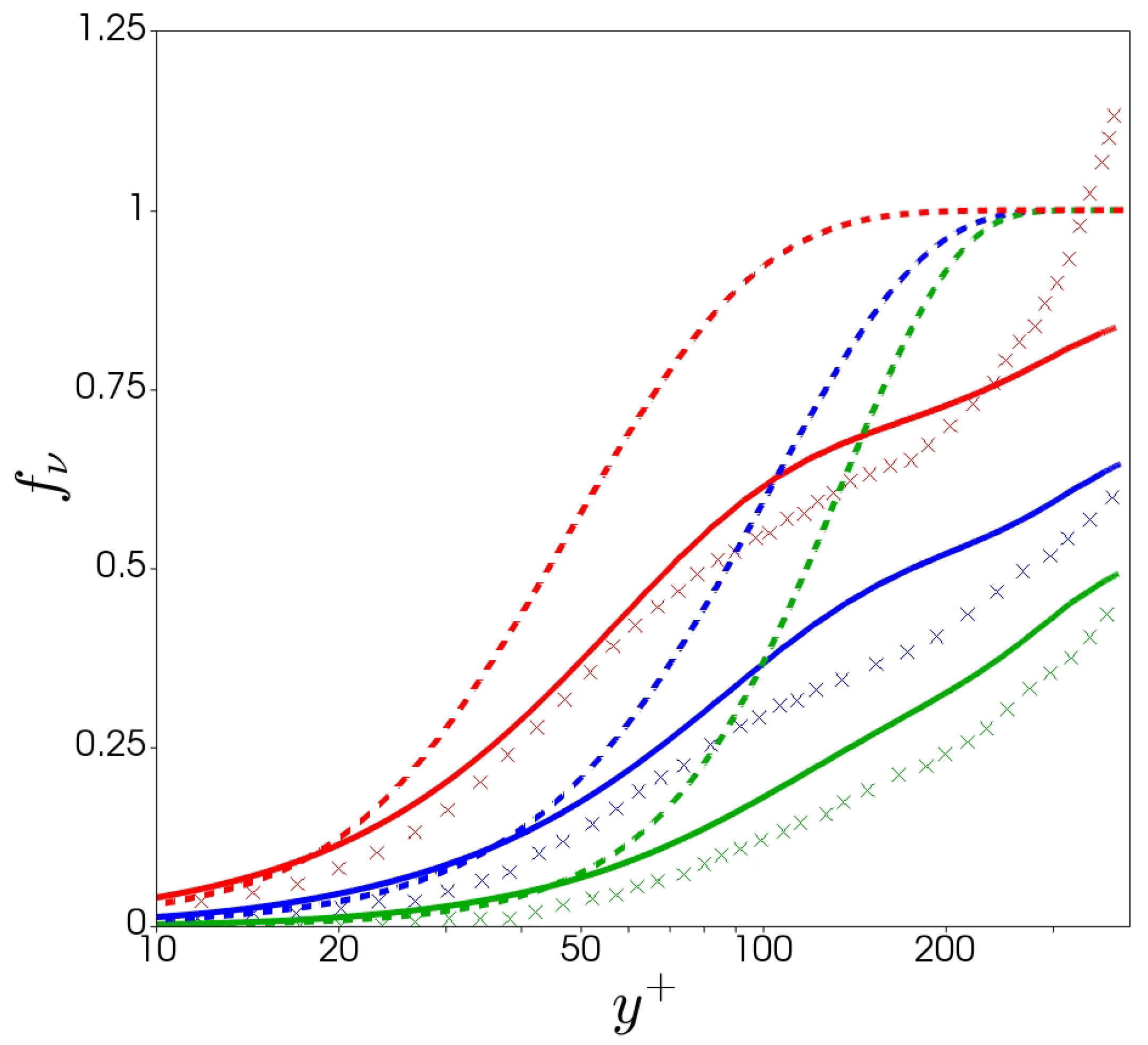
is the eddy viscosity.
is modelled by the typical isotropic
turbulence model for low Reynolds numbers, which includes a damping function
to account for near-wall effects:
where
is the viscous dissipation of
k by the Newtonian solvent,
and
. The dimensionless wall scaling is
, where
is the friction velocity,
y is the distance to the nearest wall, and
is the sum of solvent and polymer viscosity coefficients (
). The damping function requires additional modelling to capture the anisotropy of the drag reducing flow as a result of viscoelastic flow effects, to be discussed further in this study (
Section 3.2).
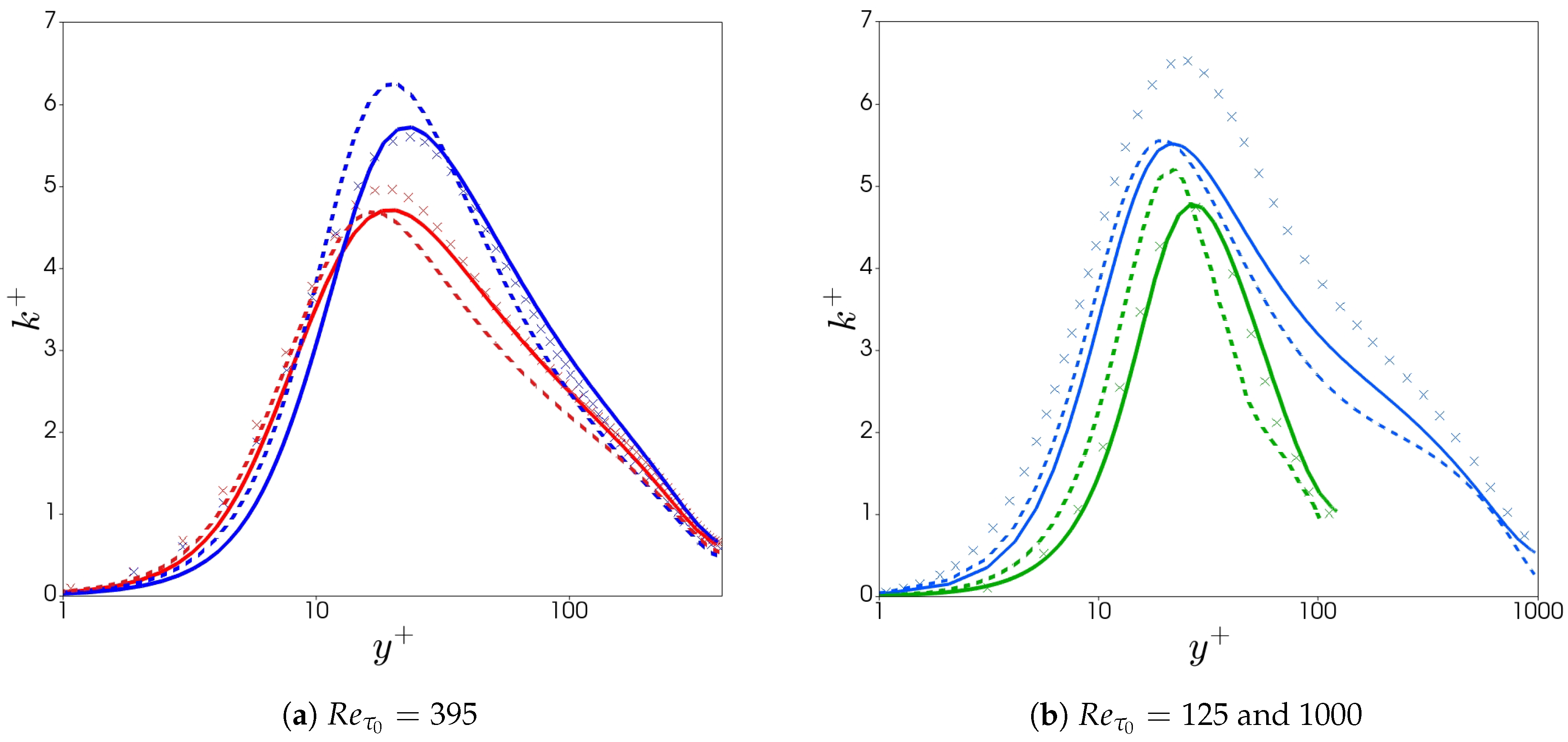
2.2. Transport Equation for the Turbulent Kinetic Energy
The governing transport equation for the turbulent kinetic energy of turbulent flow with FENE-P fluids is given by,
with
is the rate of production of
k.
The Newtonian closures of Equation (
19) are those present in the Nagano et al. [
35,
36] models. To increase numerical stability, a modified Newtonian rate of dissipation of
k is applied instead of the true dissipation, which are related by
. For better model performance and to correct for the turbulent diffusion near walls, a turbulent variable Prandtl number is added of the form,
with
and model constant
. More details of the form of Equation (
19) can be found in Pinho et al. [
20] and Resende et al. [
24].
The last two terms on the right side of the Equation (
19) are:
which are the viscoelastic turbulent transport and the viscoelastic stress work, respectively. They represent the fluctuating viscoelastic turbulent part of the
k transport equation and require suitable closure models.
A budget analysis for each term in the
k transport equation was performed by Pinho et al. [
20] for different regimes of DR. They demonstrated that the magnitude of
has more impact on the overall budget in the IDR, and also developed a closure. In the HDR, the amplitude of
is the same as
but has a different location in the buffer layer, in which the effects of
are overcome by turbulent diffusion, thus, revealing negligible effects to overall flow predictions. Masoudian et al. [
28] had chosen to neglect the
contributions in the
model and is also not included here as well.
2.3. Transport Equation for the Rate of Dissipation of Turbulent Kinetic Energy
The corresponding governing transport equation for the modified Newtonian rate of dissipation of
k is given by,
with
As mentioned in the previous sub-section, all terms are modelled in the Newtonian context (excluding
). The damping functions of Equation (
22) are
and
; with model coefficients
,
and
.
The last term in Equation (
22) is the viscoelastic contribution to the overall
balance. This term acts as a Newtonian destruction to the dissipation and is given by,
It has non-negligible effects on flow predictions for all DR regimes and thus requires a suitable model.
5. Numerical Procedure
This section presents the numerical methods applied in order to examine the viscoelastic turbulence model against the available DNS data identified within the literature. A new finite volume C++ computational solver was developed in the OpenFOAM software by modifying the sub-class files and introducing the FENE-P viscoelastic quantities such as: the polymer stress to the momentum equation; conformation tensor transport equation; and modified damping function to include elastic effects.
A fully-developed channel flow using half of the channel height,
h, is applied given the symmetry of the governing geometry. We assigned 100 cells in the transverse (wall) direction with approximately 10 cells located inside the viscous sublayer. This is to provide mesh independent results, with errors within 0.5% for the mean velocity and the friction factor compared with a very fine mesh, similarly with [
30]. The initial state of the simulation is the Newtonian solution until a steady-state solution was reached for each run case, except for HDR where a similar IDR developed case is applied to reduce computational time. Relaxation factors for the additional conformation tensor field are set to 0.2, along with residual control set to
. To improve numerical stability, an artificial diffusion term is added to the RACE of the form,
, where
denotes a constant, isotropic, artificial numerical diffusivity. In earlier studies [
10], the dimensionless artificial numerical diffusivity is taken to be
. Here,
and has negligible effect on mean values.
A pressure gradient is forced in the stream-wise direction to be unity, with periodic boundary conditions for all other flow fields, mimicking the DNS procedure of Li et al. [
22]. No-slip boundary conditions were imposed on the solid wall for the velocity field
U, along with
k and
set to zero (or very small,
). A Dirichlet boundary condition for
is reported in
Appendix A (similar to [
26], but for all components), which is imposed within OpenFOAM under the swak4Foam library using the groovyBC functionality developed by Gschaider [
45].
When normalizing the governing equations and inherently the various physical quantities, the velocity scale is taken to be the friction velocity (leading to the use of superscript +) and the length scale is the viscous length, . The conformation tensor is already in dimensionless form.
7. Conclusions
A viscoelastic turbulence model in fully-developed drag-reducing channel flow is improved, with turbulent eddies modeled under a
representation, along with polymeric solutions described by the finitely extensible nonlinear elastic-Peterlin (FENE-P) constitutive model. A new finite volume C++ computational solver was developed in the OpenFOAM software by modifying the
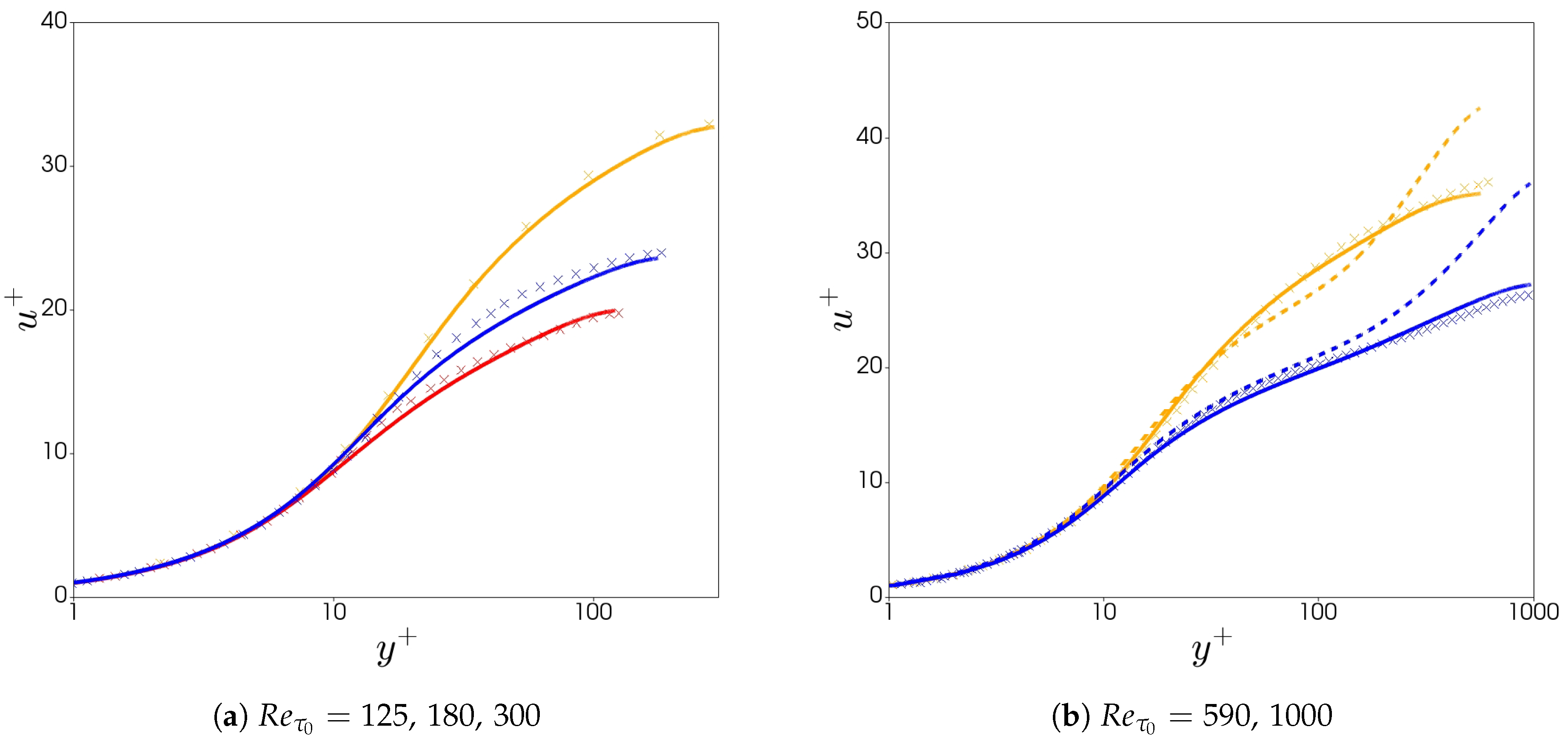
sub-class files and introducing the FENE-P viscoelastic quantities such as: the polymer stress to the momentum equation; conformation tensor transport equation; and modified damping function to include elastic effects. The model performance is evaluated against a variety of rheological parameters within the DNS data literature, including: friction Reynolds number
; Wiessenberg number
; and maximum molecular extensibility of the dumbbell chain
. The DNS data case (19) in
Table 1 (
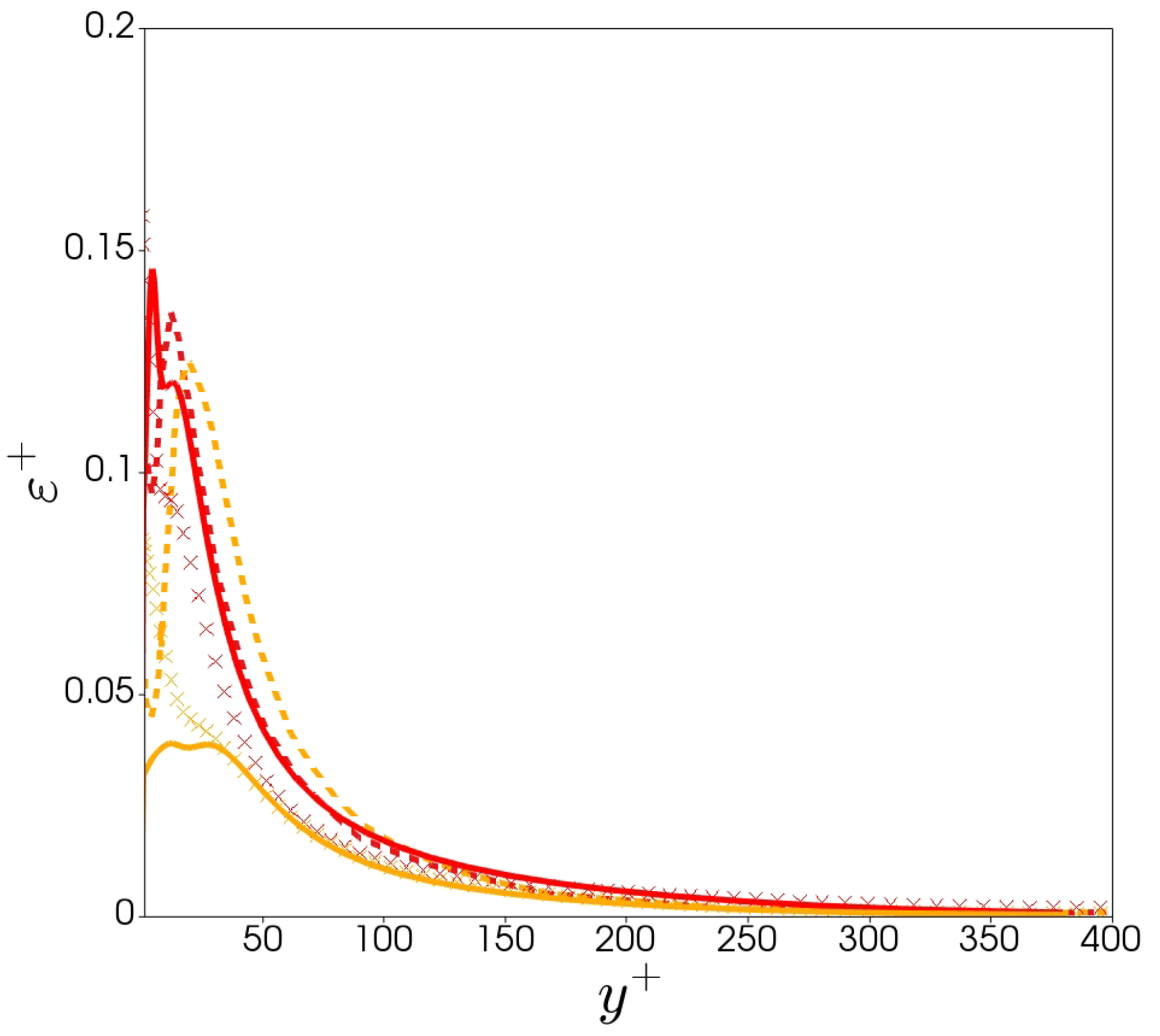
, DR = 37%) is used for the calibration of the closures developed for the turbulent cross-correlations identified in
Section 3. The model is capable of predicting all flow features for low and high Reynolds numbers at all regimes of DR and improves significantly on the model of Resende et al. [
32], with its ability to capture higher Reynolds numbers with simpler and physical-based closures.
The main feature is the formulation of the term which accounts for the interactions between the fluctuating components of the conformation tensor and the velocity gradient tensor. The advantage of the closure is the reduction in the complexity and use of damping functions in the dominant contribution, , modeled here to increase with turbulent kinetic energy as the flow viscoelasticity increases, demonstrating significant improvement with a range of rheological parameters and flow conditions.
Further improvements are developed for the viscoelastic destruction term, , within the dissipation rate transport equation. Modeled here with dependence on k and viscoelastic quantities, showing the ability to predict for low and high drag reduction.
An improved modified damping function, , is also presented, which is able to predict the global reduction of the eddy viscosity and shift away from the wall for increasing viscoelasticity, whilst also improving the profiles of turbulent kinetic energy.
Overall, predictions compare very well with a wide range of DNS data and significantly improves on capturing all flow features with simplicity and performance compared with the most recent
model developed by Resende et al. [
32]. The simplicity of the present model allows easy implementation into 3D codes and increases numerical stability. All friction velocity dependence is removed in the present model which is the first of its kind for damping function
models, whose main advantage is the realization of simulations in geometries with reattachment. Future work to extend to this study includes the development of an improved
model based on the present model [
25]. This would require the same concept of the modified damping function developed in this paper to be applied, with capabilities to predict flow behavior in industrially represented geometries such as pipes and constrictions.