Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width
Abstract
1. Introduction
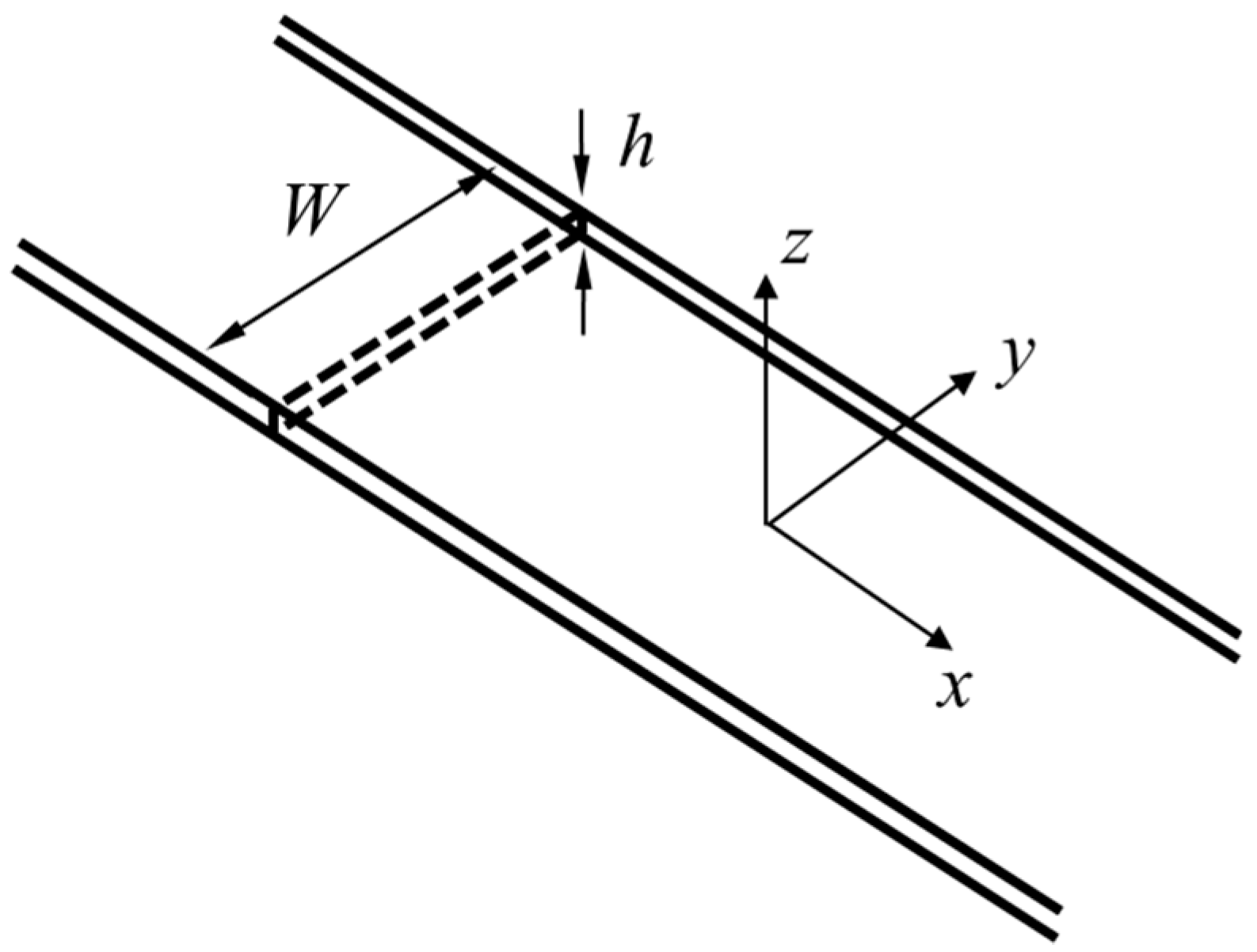
2. Lamb Wave Propagation in a Plate Strip with Finite Width
2.1. Semi-Analytical Finite Element(SAFE) Method
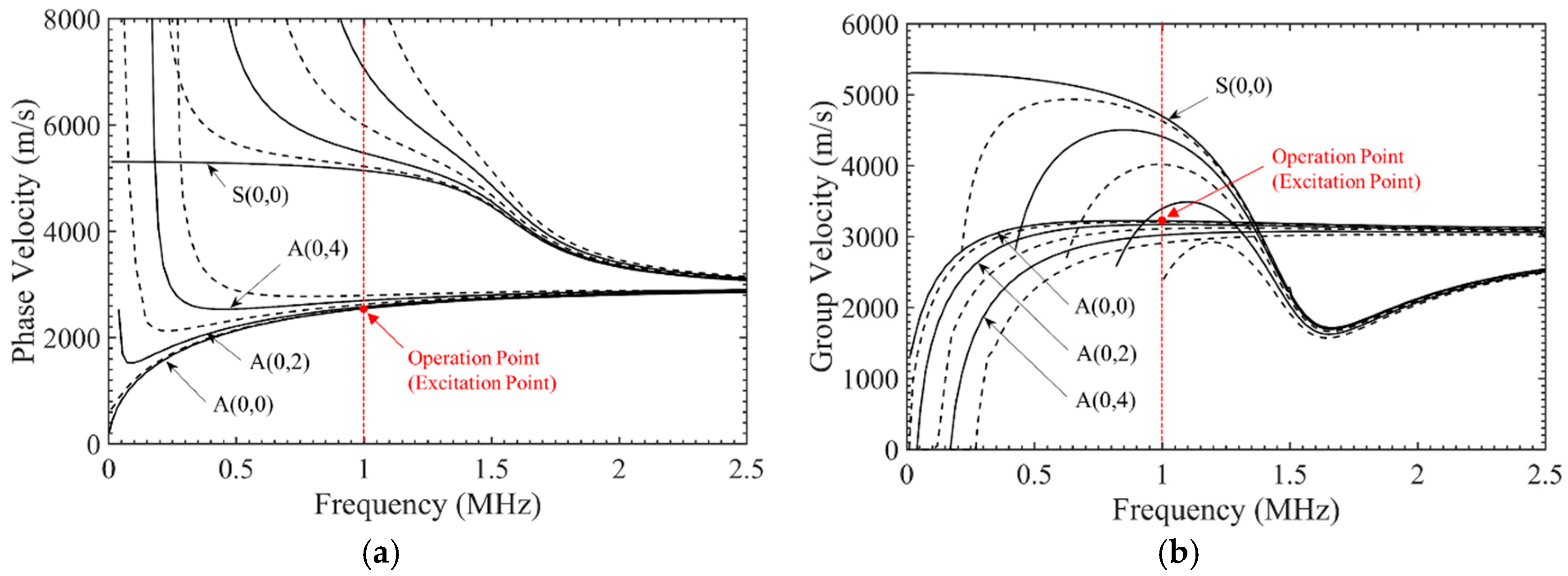
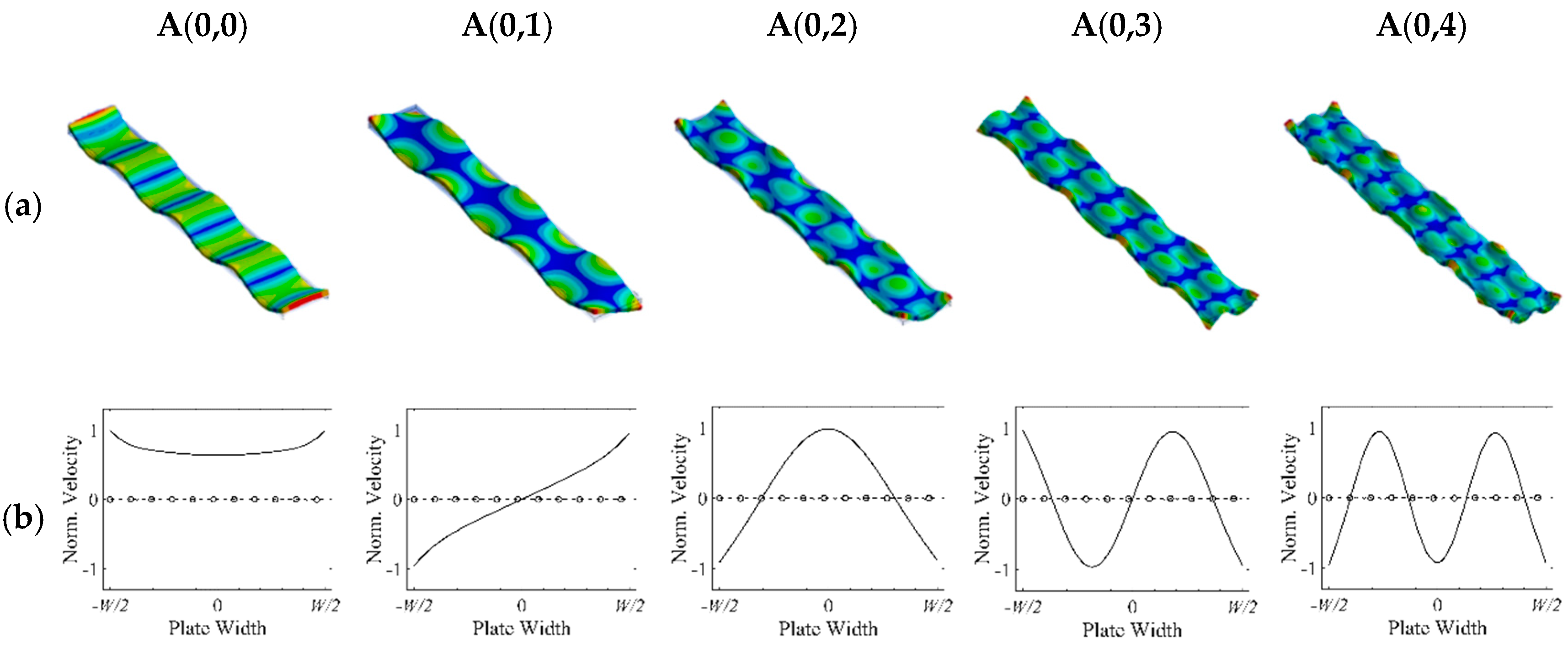
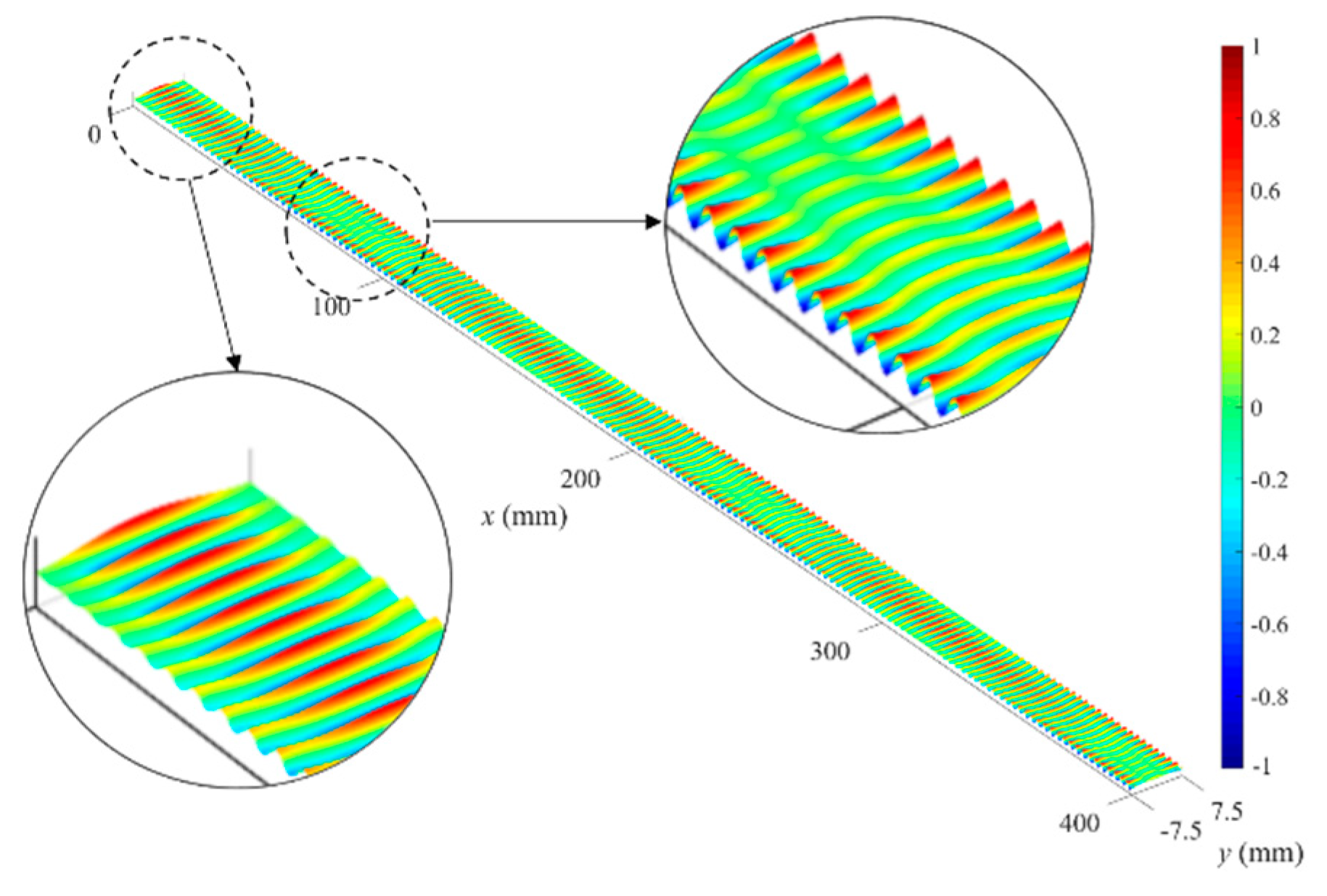
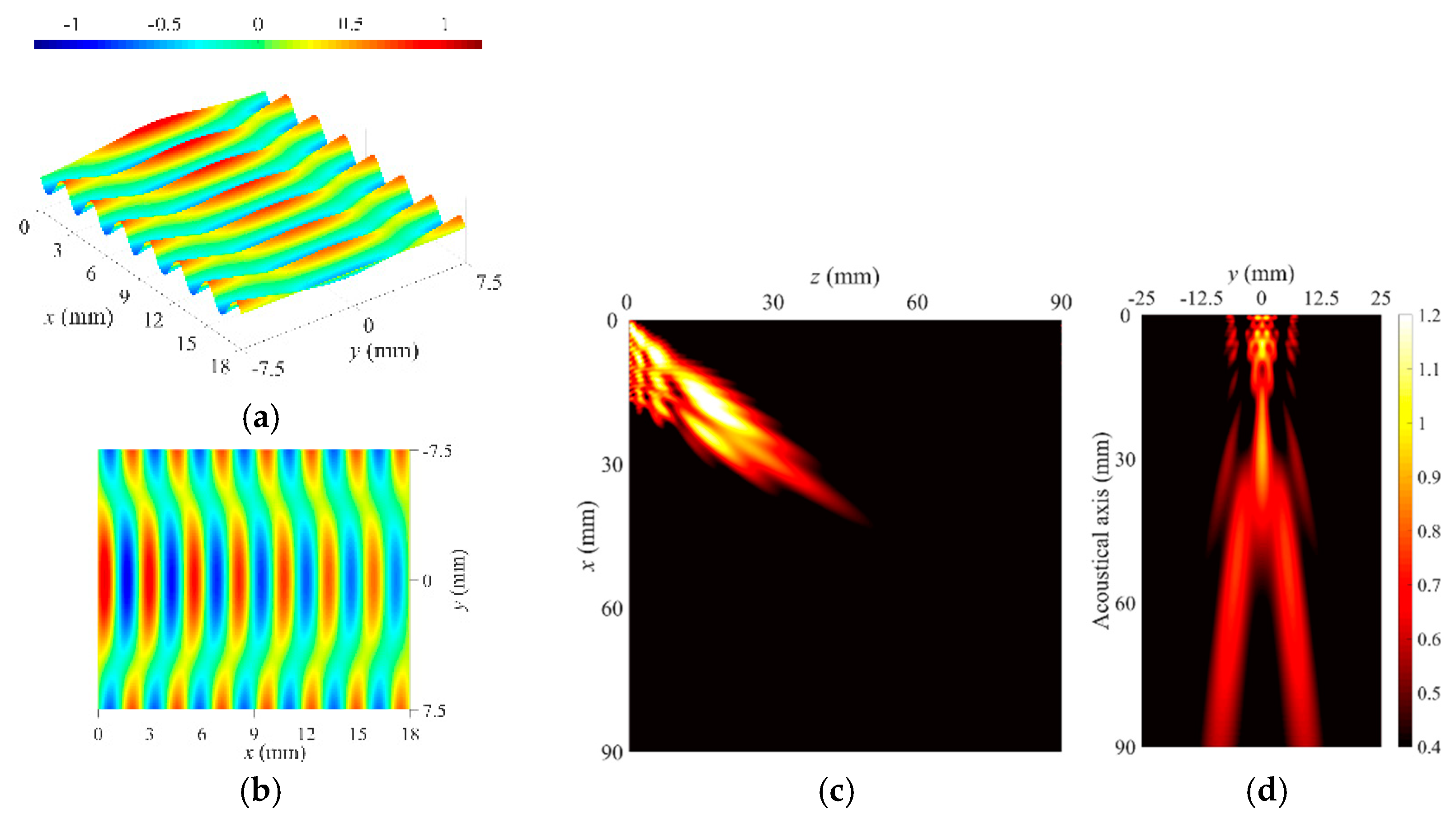
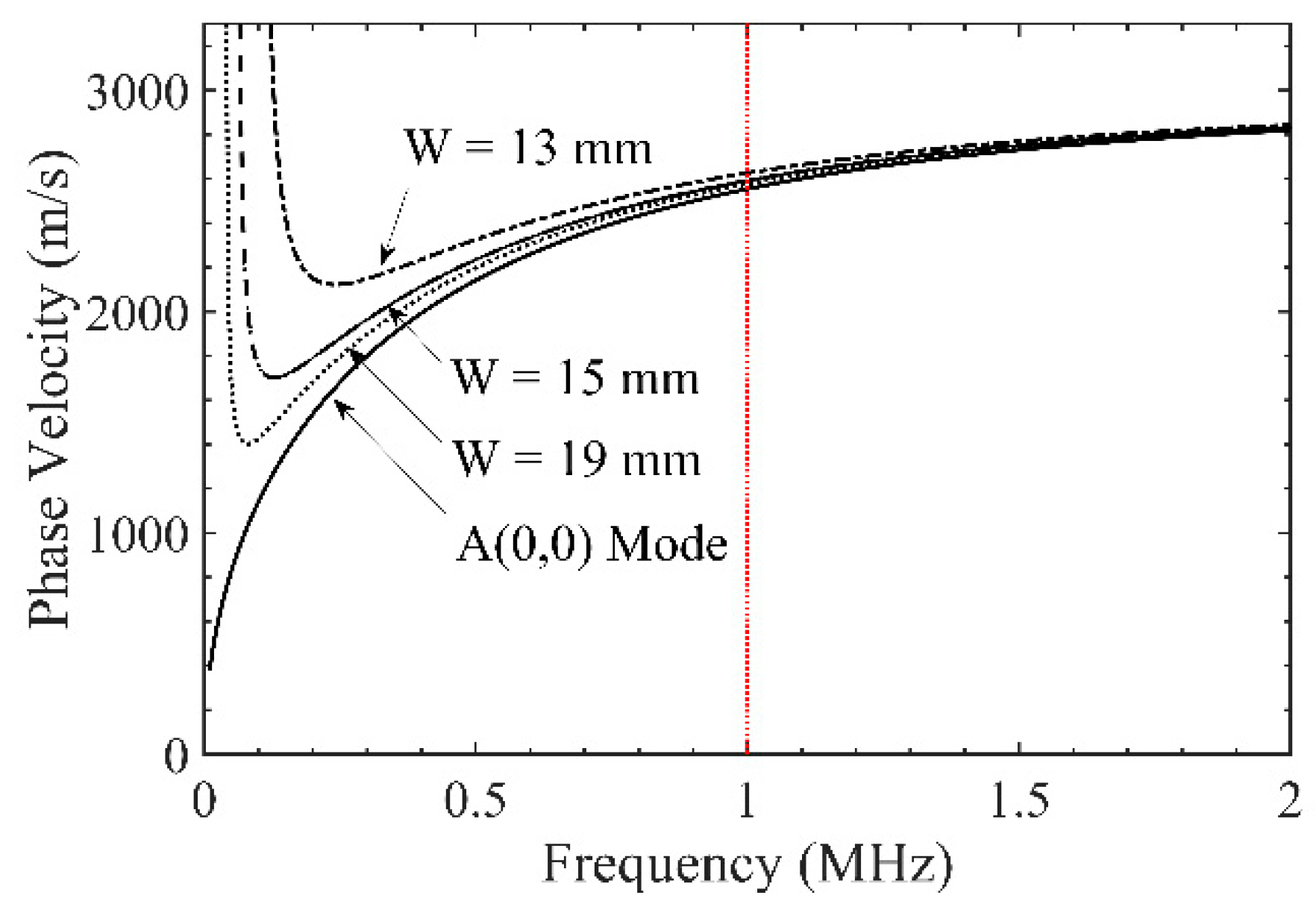
2.2. Dispersion Curves and Wave Structures
3. Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width
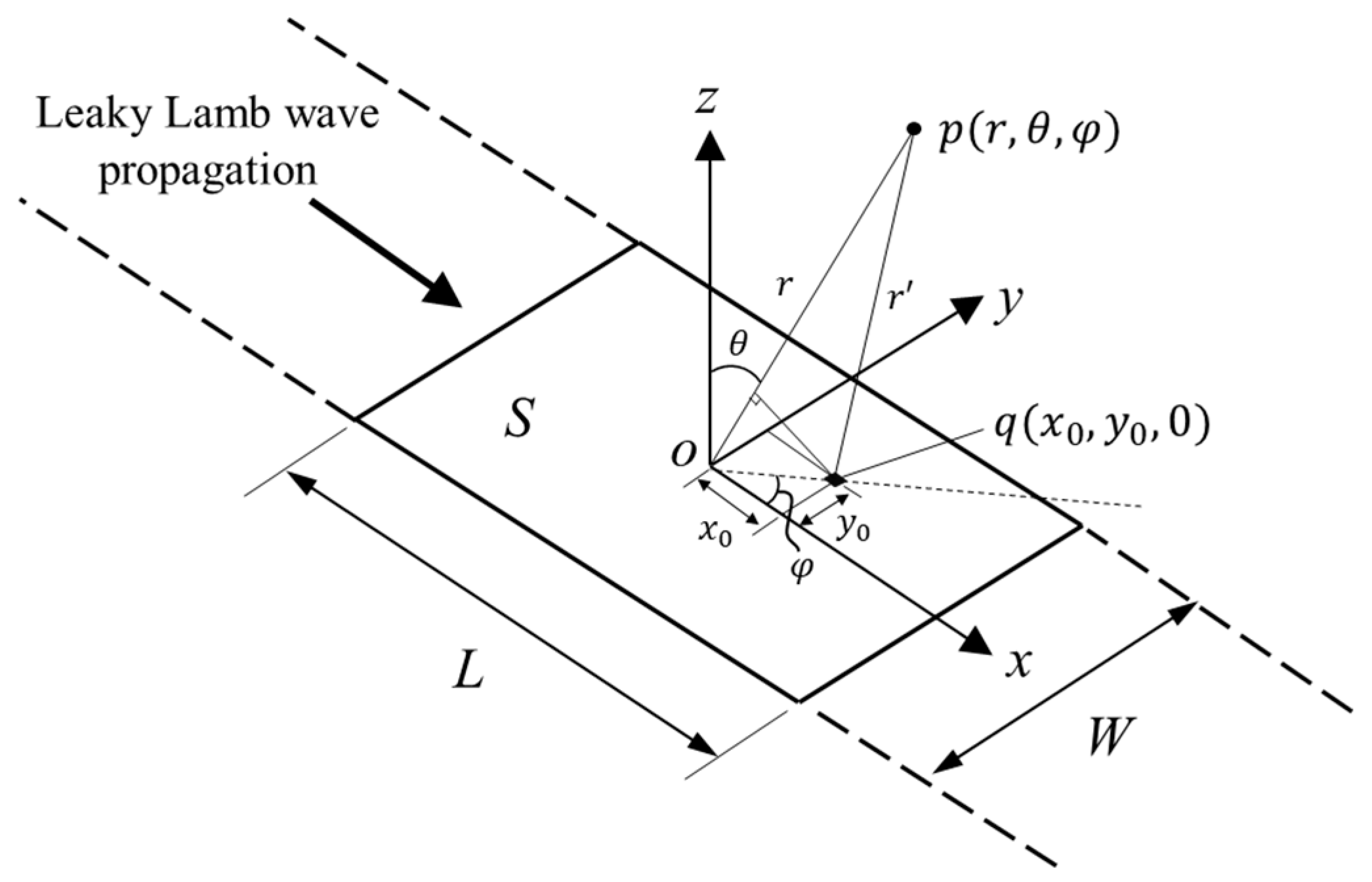
3.1. Rayleigh–Sommerfeld Integral Model
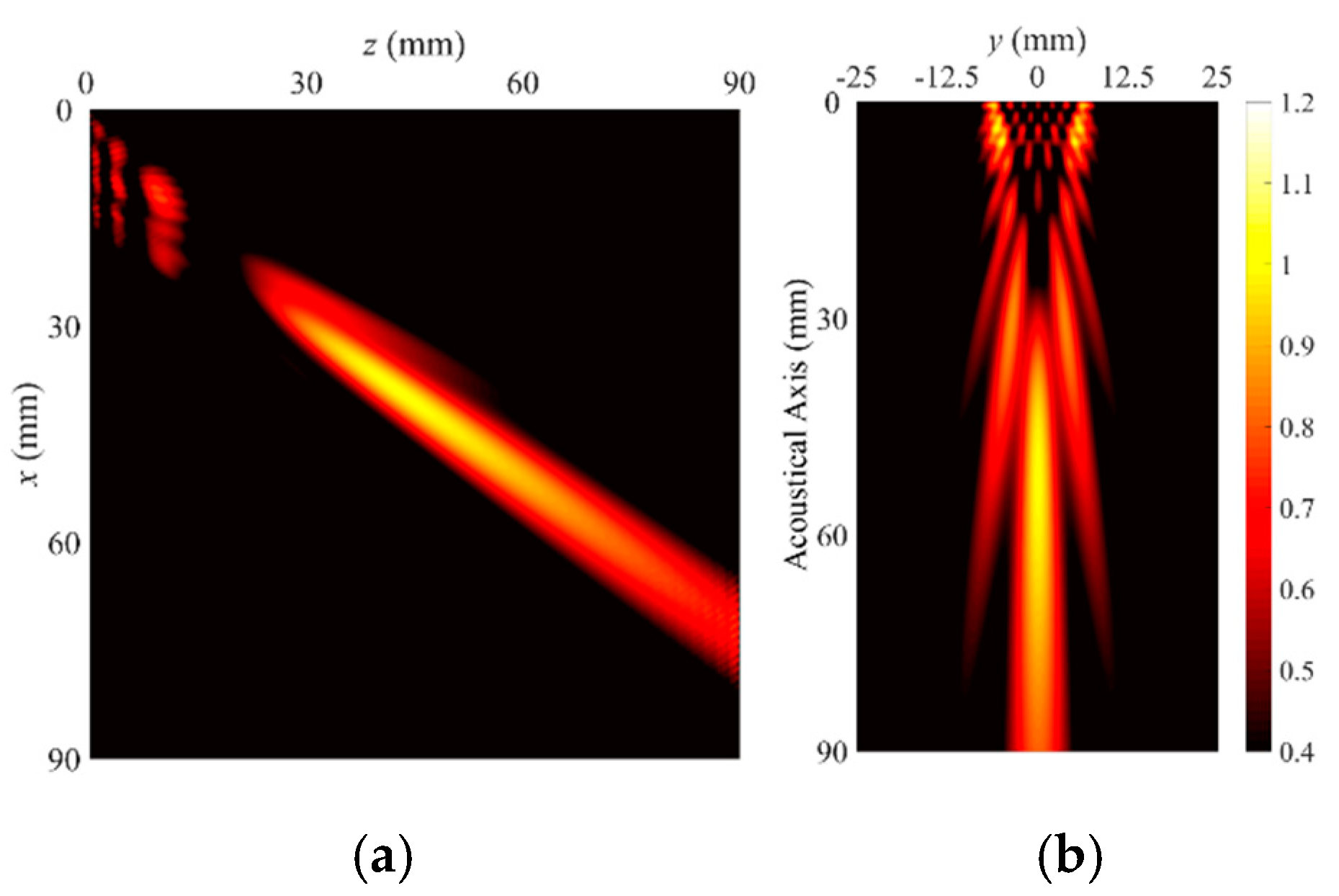
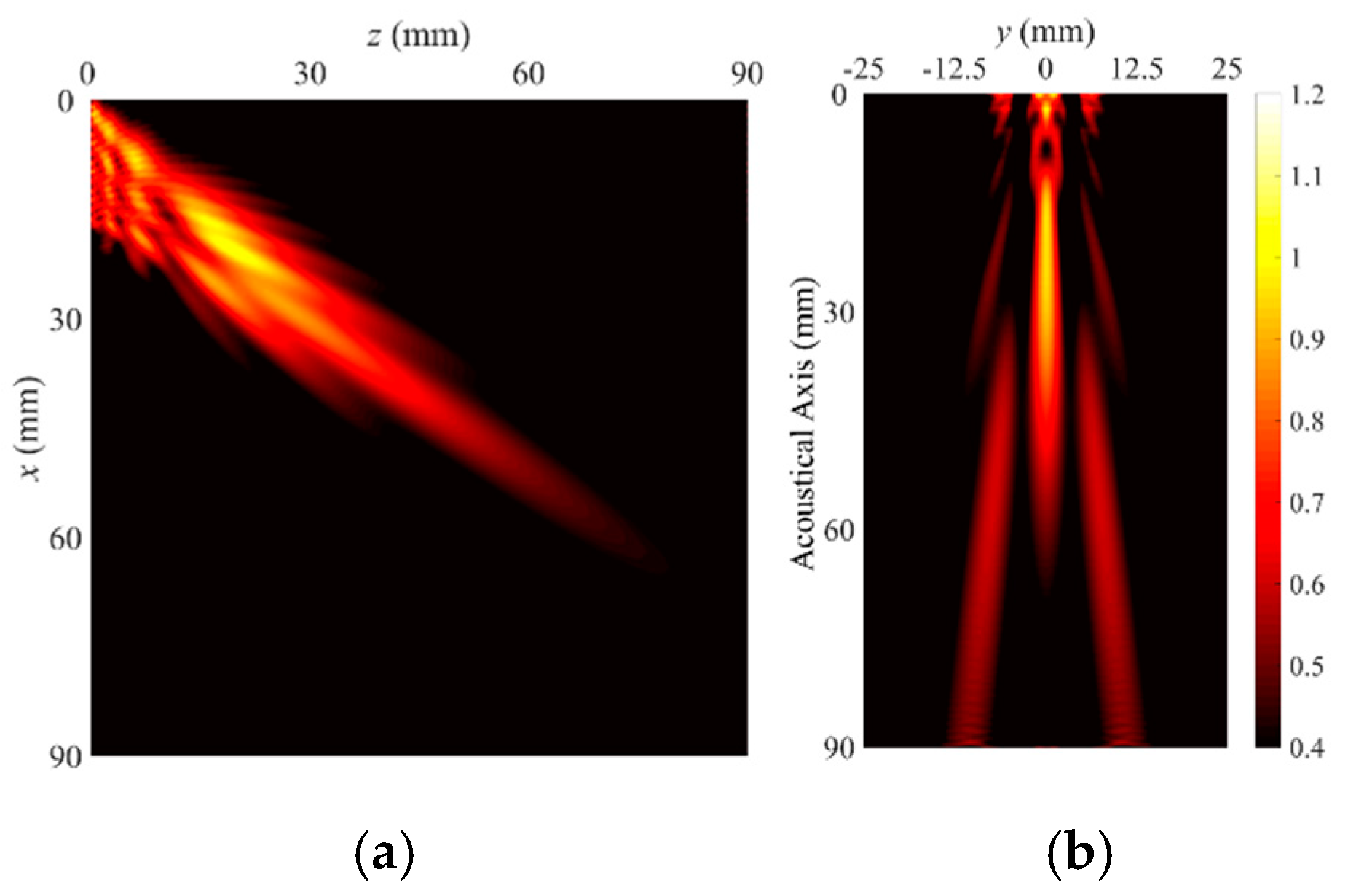
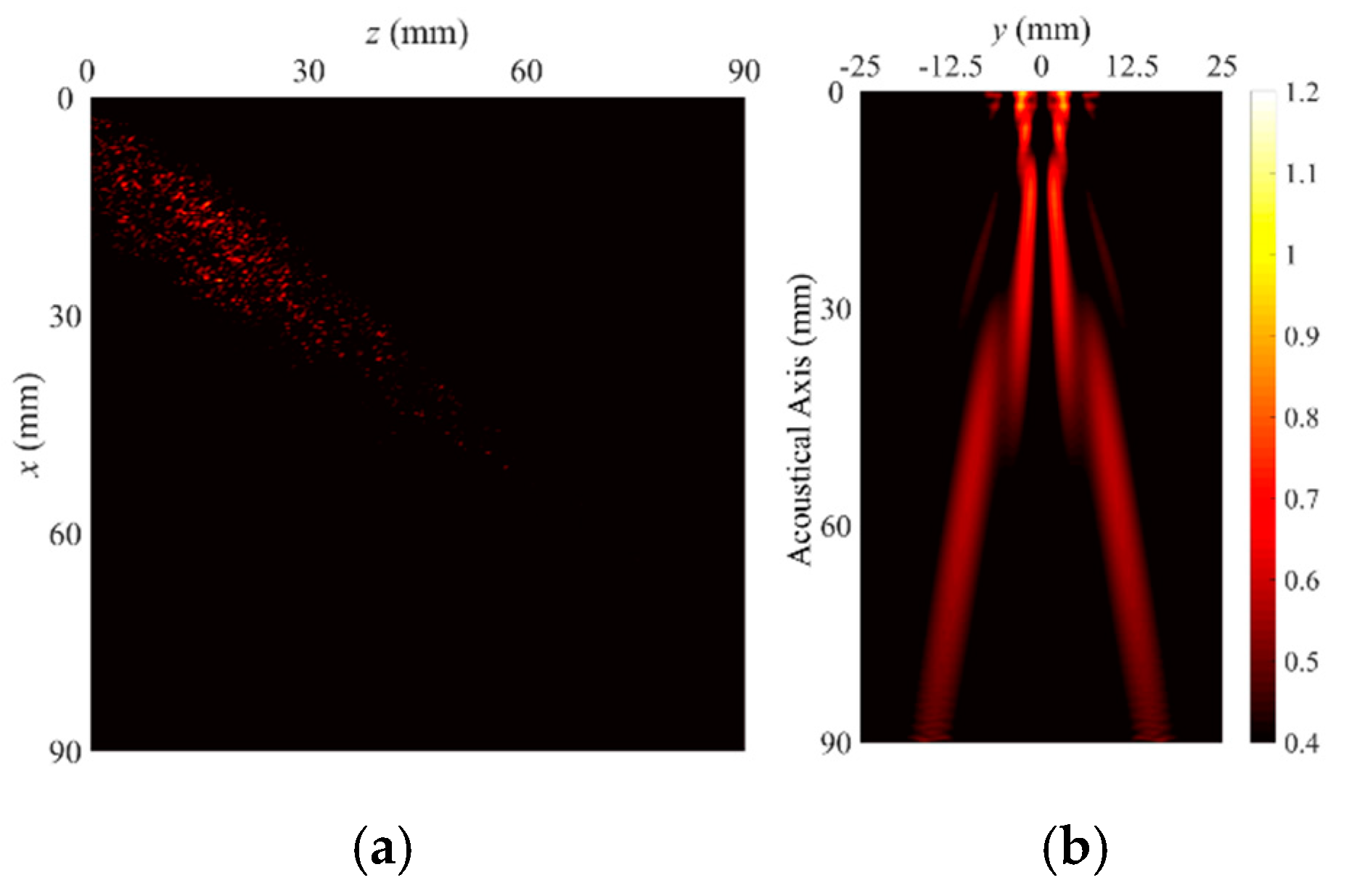
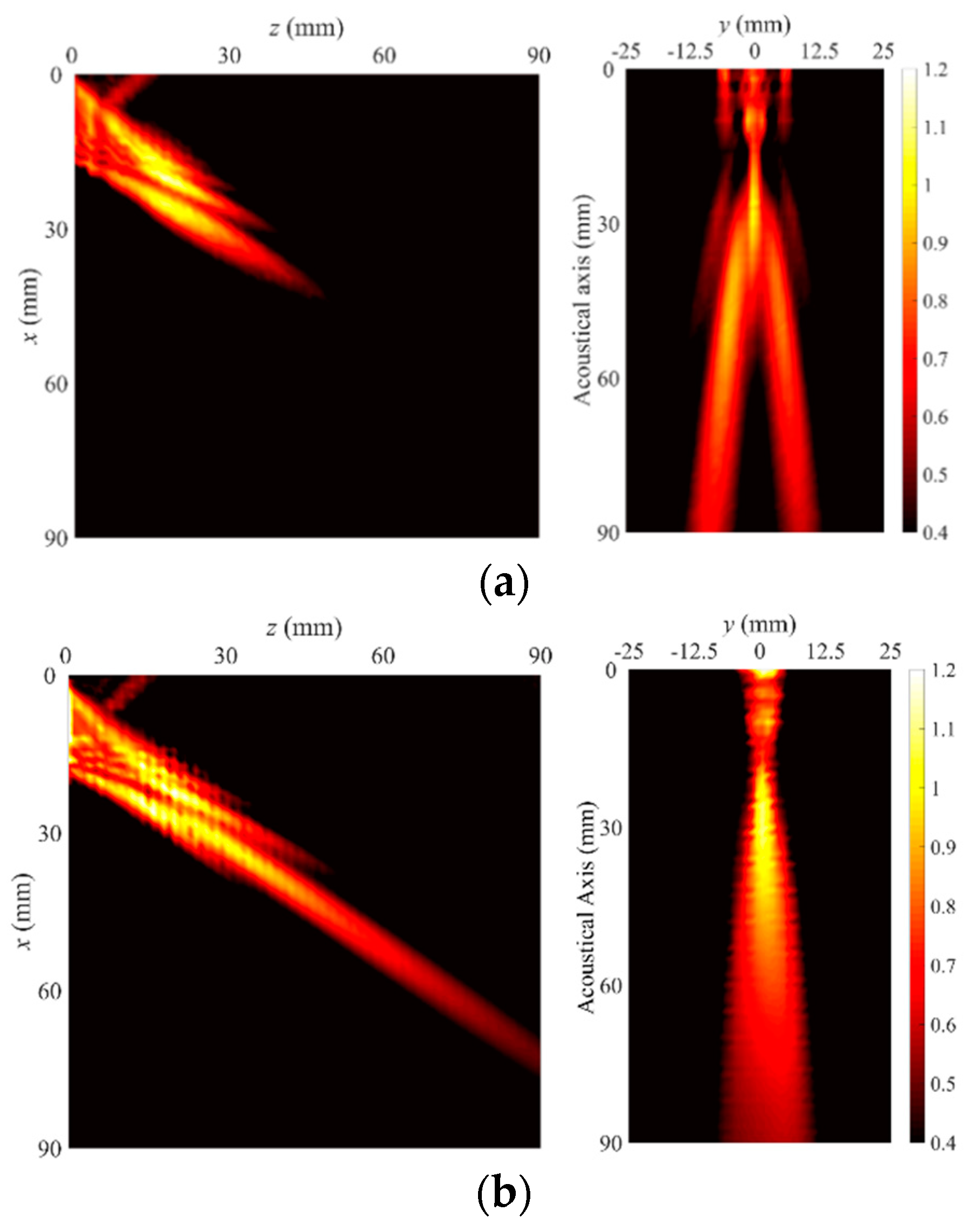
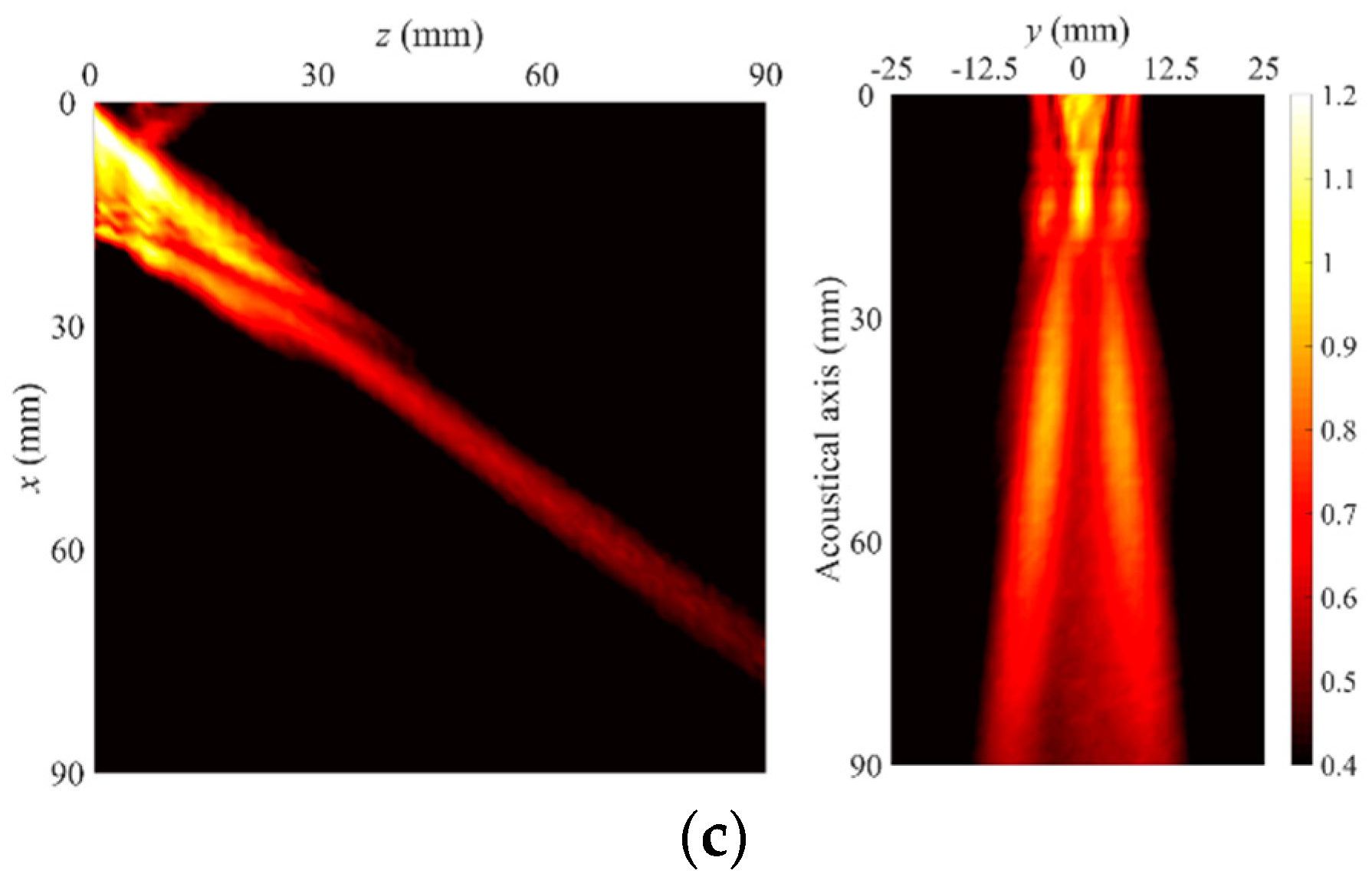
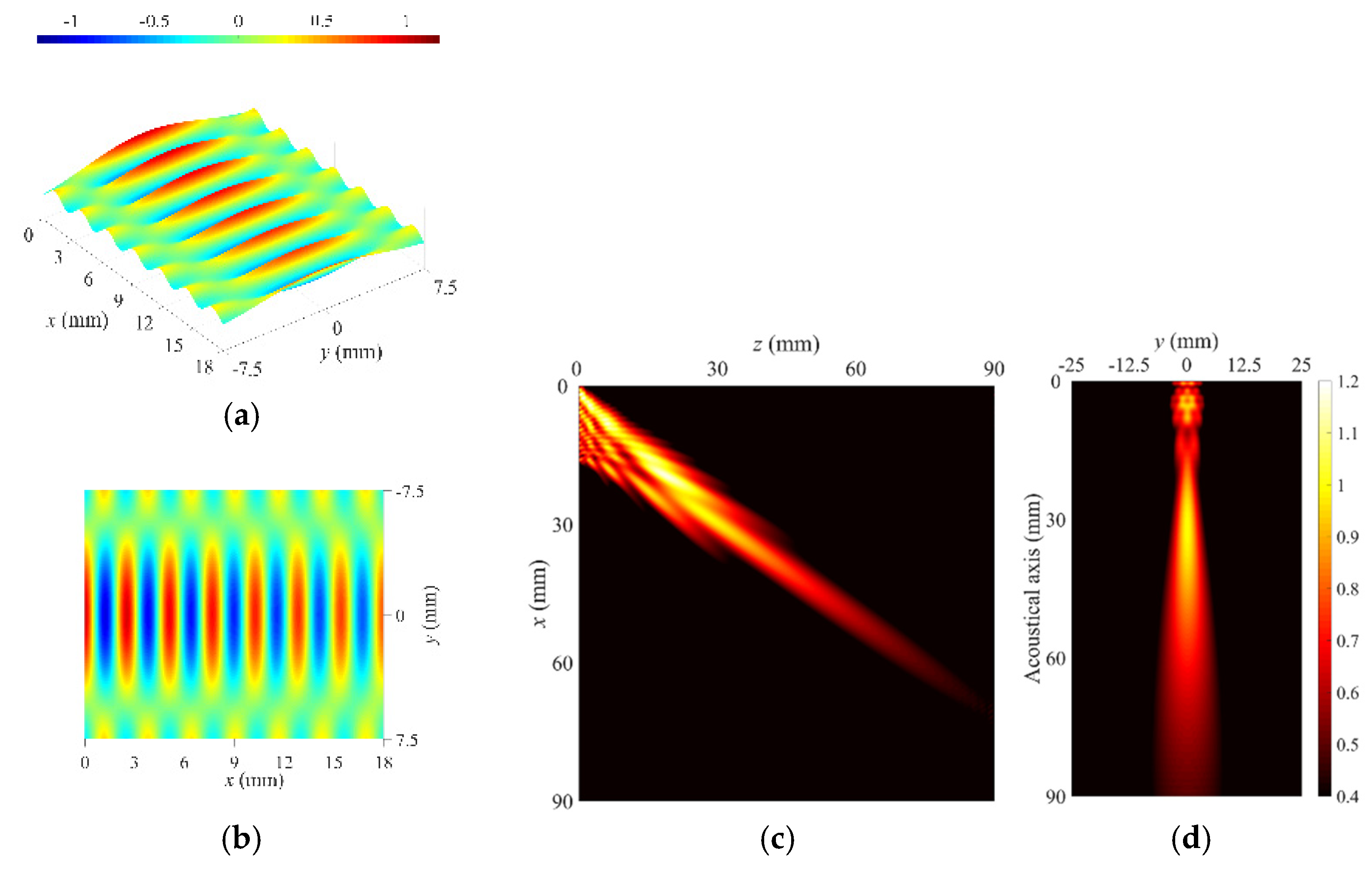
3.2. Leaky Radiation Beam Patterns and Beam Profiles
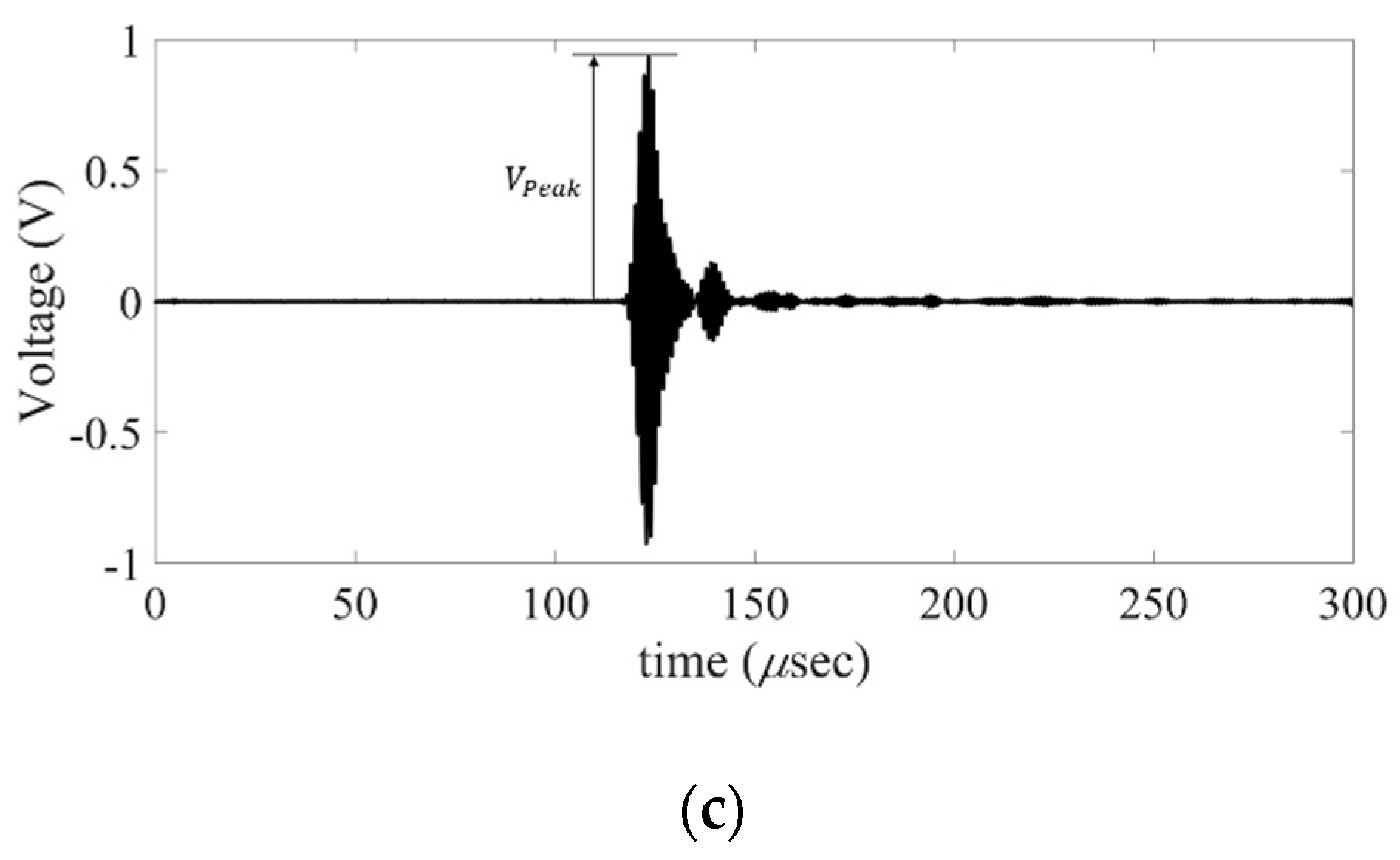
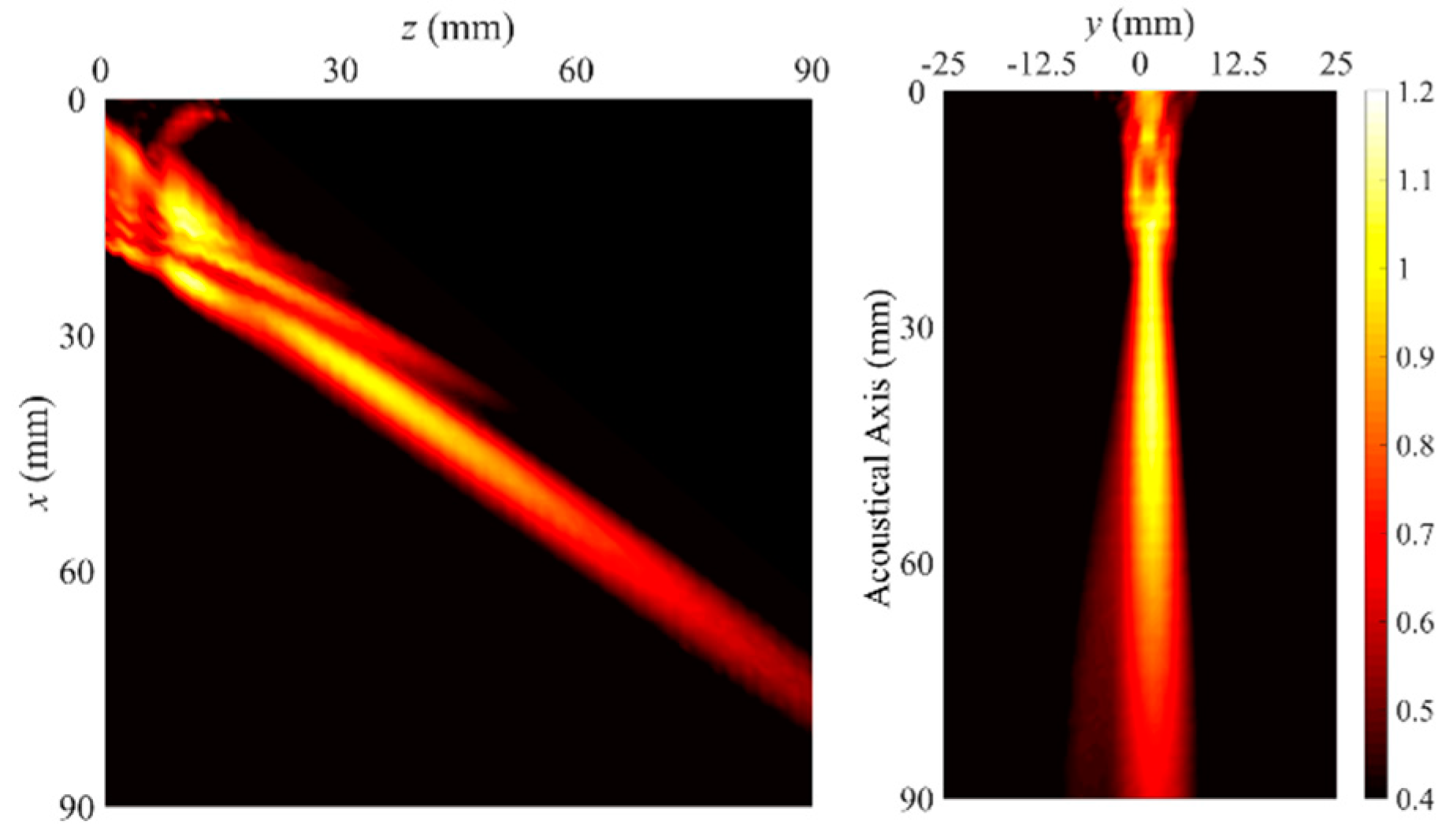
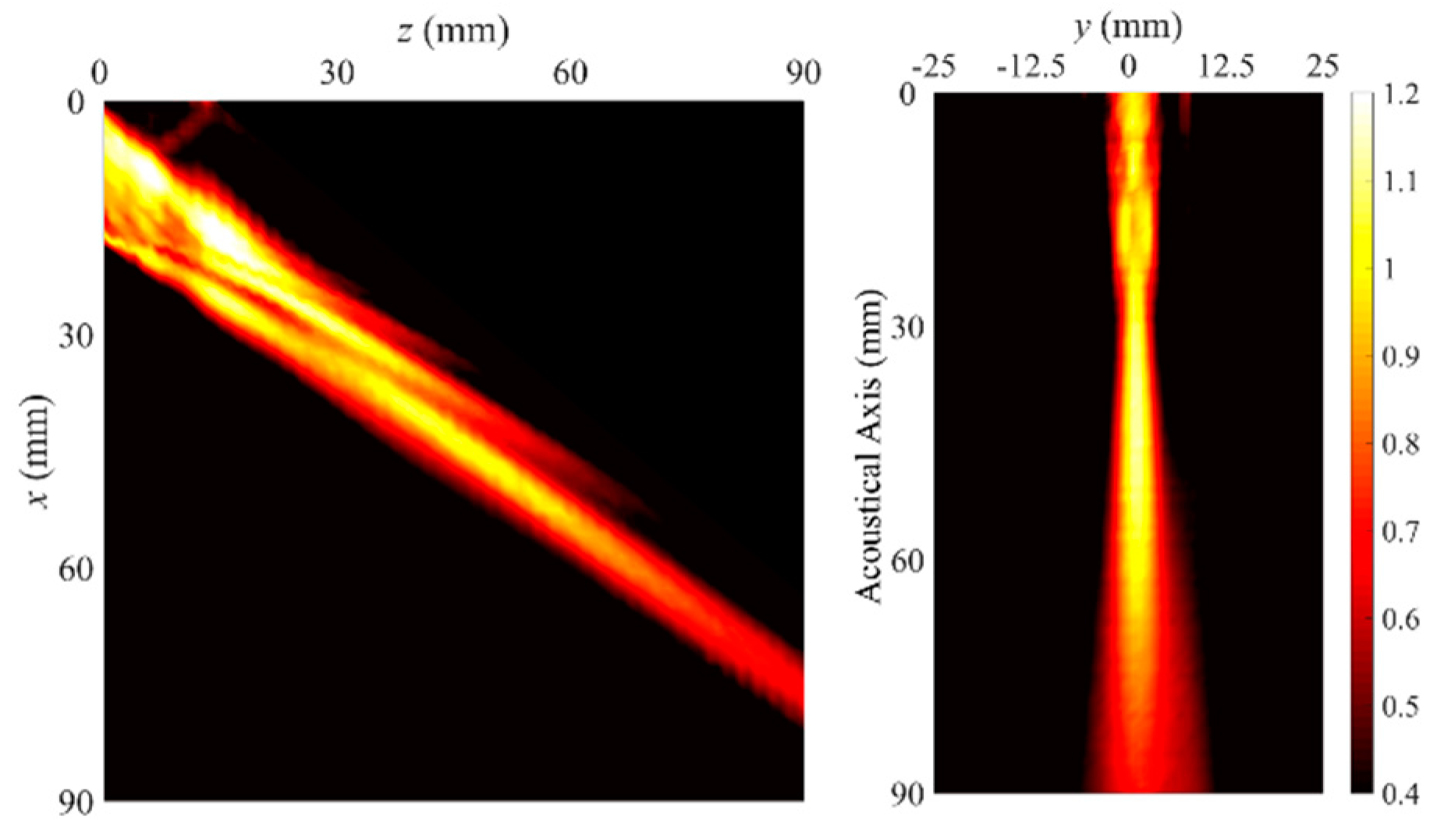
4. Beam Profile Measurements
4.1. Experimental Setup
4.2. Measured Beam Profiles and Effects of the Mode Superposition
5. Discussion and Design Direction for Performance Improvement of the Waveguide Sensor
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ditri, J.; Rose, J.L.; Chen, G. Mode selection criteria for defect detection optimization using Lamb waves. In Proceedings of the 18th Annual Review of Progress in Quantitative NDE; Thompson, D.O., Chimenti, D.E., Eds.; Plenum Press: New York, NY, USA, 1992; Volume 11, pp. 2109–2115. [Google Scholar]
- Cawley, P.; Alleyne, D.N. The use of Lamb waves for the long range inspection of large structure. Ultrasonics 1996, 34, 287–290. [Google Scholar] [CrossRef]
- Alleyne, D.N.; Cawley, P. Long range propagation of Lamb waves in chemical plant pipework. Mater. Eval. 1997, 55, 504–508. [Google Scholar]
- Na, W.-B.; Kundu, T. Underwater pipeline inspection using guided waves. J. Press. Vessel Technol. 2002, 124, 196–200. [Google Scholar] [CrossRef]
- Gao, H.; Rose, J.L. Ice detection and classification on an aircraft wing with ultrasonic shear horizontal guided waves. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 334–344. [Google Scholar]
- Chen, J.; Su, Z.; Cheng, L. Identification of corrosion damage in submerged structures using fundamental anti-symmetric Lamb waves. Smart Mater. Struct. 2010, 19, 015004. [Google Scholar] [CrossRef]
- Gao, H.; Rose, J.L. Goodness dispersion curves for ultrasonic guided wave based SHM: A sample problem in corrosion monitoring. Aeronaut. J. R. Aeronaut. Soc. 2010, 114, 49–56. [Google Scholar] [CrossRef]
- Leinov, E.; Lowe, M.-J.S.; Cawley, P. Investigation of guided wave propagation and attenuation in pipe buried in sand. J. Sound Vib. 2015, 347, 96–114. [Google Scholar] [CrossRef]
- Lynnworth, L.C.; Liu, Y.; Umina, J.A. Extensional bundle waveguide techniques for measuring flow of hot fluids. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 538–544. [Google Scholar] [CrossRef]
- Celga, F.B.; Cawley, P.; Allin, J.; Davies, J. High temperature(>500 °C) wall thickness monitoring using dry-coupled ultrasonic waveguide transducer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 156–167. [Google Scholar]
- Laws, M.; Ramadas, S.N.; Lynnworth, L.C.; Dixon, S. Parallel strip waveguide for ultrasonic flow measurement in harsh environments. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 697–708. [Google Scholar] [CrossRef]
- Sun, F.; Sun, Z.; Chen, Q.; Murayama, R.; Nishino, H. Mode conversion behavior of guided wave in a pipe inspection system based on a long waveguide. Sensors 2016, 16, 1–14. [Google Scholar] [CrossRef] [PubMed]
- Shah, H.; Balasubramaniam, K.; Rajagopal, R. In-situ process-and online structural health monitoring of composites using embedded acoustic waveguide sensors. J. Phys. Commun. 2017, 1, 1–11. [Google Scholar] [CrossRef]
- Griffin, J.W.; Bond, L.J.; Peters, T.J.; Denslow, K.M.; Posakony, G.J.; Sheen, S.H.; Chien, H.T.; Raptis, A.C. Under-Sodium Viewing: A Review of Ultrasonic Imaging Technology for Liquid Metal Fast Reactors; Pacific Northwest National Laboratory: Richland, WA, USA, 2009; pp. 1–64. [Google Scholar]
- Wang, K.; Chien, H.; Elmer, T.W.; Lawrence, W.P.; Engel, D.M.; Sheen, S. Development of ultrasonic waveguide techniques for under-sodium viewing. NDT E Int. 2012, 49, 71–76. [Google Scholar] [CrossRef]
- Watkins, R.D.; Deighton, M.O.; Gillespie, A.B.; Pike, R.B. A proposed method for generating and receiving narrow beams of ultrasound in the fast reactor liquid sodium environment. Ultrasonics 1982, 20, 7–12. [Google Scholar] [CrossRef]
- Watkins, R.D.; Barrett, L.M.; McKnight, J.A. Ultrasonic waveguide for use in the sodium coolant of fast reactors. Nucl. Energy 1988, 27, 85–89. [Google Scholar]
- Joo, Y.-S.; Park, C.-G.; Lee, J.-H.; Kim, J.-B. Development of ultrasonic waveguide sensor for under-sodium inspection in a sodium-cooled fast reactor. NDT E Int. 2011, 44, 239–246. [Google Scholar] [CrossRef]
- Joo, Y.-S.; Bae, J.-H.; Kim, J.-B.; Kim, J.-Y. Effects of beryllium coating layer on performance of the ultrasonic waveguide sensor. Ultrasonics 2013, 53, 387–395. [Google Scholar] [CrossRef]
- Kim, H.-W.; Joo, Y.-S.; Park, C.-G.; Kim, J.-B.; Bae, J.-H. Ultrasonic imaging in hot liquid sodium using a plate-type ultrasonic waveguide sensor. J. Nondestruct. Eval. 2014, 33, 676–683. [Google Scholar] [CrossRef]
- Joo, Y.-S. Under-sodium viewing techniques in sodium-cooled fast reactors. J. Korean Soc. Nondestruct. Test. 2012, 32, 439–447. [Google Scholar]
- Kim, H.-W.; Joo, Y.-S.; Park, S.-J.; Kim, S.-K. Ultrasonic ranging technique for obstacle monitoring above reactor core in prototype generation IV sodium-cooled fast reactor. Nucl. Eng. Technol. 2020, 52, 776–783. [Google Scholar] [CrossRef]
- Banerjee, S.; Kundu, T. Ultrasonic field modeling in plates immersed in fluid. Int. J. Sol. Struct. 2007, 44, 6013–6029. [Google Scholar] [CrossRef]
- Hayashi, T.; Inoue, D. Calculation of leaky Lamb waves with a semi-analytical finite element. Ultrasonics 2014, 54, 1460–1469. [Google Scholar] [CrossRef] [PubMed]
- Inoue, D.; Hayashi, T. Transient analysis of leaky Lamb waves with a semi-analytical finite element. Ultrasonics 2015, 62, 80–88. [Google Scholar] [CrossRef] [PubMed]
- An, Z.; Hu, J.; Mao, J.; Lian, G.; Wang, X. Optical visualization of leaky Lamb wave and its application in nondestructive testing. In Proceedings of the 2017 ICU Honolulu Sixth International Congress on Ultrasonics, Honolulu, HI, USA, 18–22 December 2017. [Google Scholar]
- Deighton, M.O.; Gillespie, A.-B.; Pike, R.-B.; Watkins, R.-D. Mode conversion of Rayleigh and Lamb waves to compression waves at a metal-liquid interface. Ultrasonics 1981, 19, 249–258. [Google Scholar] [CrossRef]
- Morse, R.W. Dispersion of compressional waves in isotropic rods of rectangular cross section. J. Acoust. Soc. Am. 1948, 20, 833–838. [Google Scholar] [CrossRef]
- Morse, R.W. The velocity of compressional waves in rods of rectangular cross section. J. Acoust. Soc. Am. 1950, 22, 219–223. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Fox, E.A. Vibrations and waves in elastic bars of rectangular cross section. Trans. ASME J. Appl. Mech. 1960, 27, 152–158. [Google Scholar] [CrossRef]
- Konenkov, Y.K. Plate waves and flexural oscillations of a plate. Sov. Phys. Acoust. 1960, 6, 52–59. [Google Scholar]
- Konenkov, Y.K.; Namukina, N.I.; Tartakovskii, B.D. Investigation of forced flexural vibrations of an elastic strip. Sov. Phys. Acoust. 1966, 11, 288–295. [Google Scholar]
- Fraser, W.B. Stress wave propagation in rectangular bars. Int. J. Solids Struct. 1969, 5, 379–397. [Google Scholar] [CrossRef]
- Krushynska, A.A.; Meleshko, V.V. Normal waves in elastic bars of rectangular cross section. J. Acoust. Soc. Am. 2011, 129, 1324–1335. [Google Scholar] [CrossRef]
- Hayashi, T.; Song, W.-J.; Rose, J.L. Guided wave dispersion curves for a bar with an arbitrary cross-section, a rod and rail example. Ultrasonics 2003, 41, 175–183. [Google Scholar] [CrossRef]
- Hayashi, T.; Tanaka, T. Lamb wave propagation in a plate with a finite width. Trans. Jpn. Soc. Mech. Eng. A 2006, 72, 149–154. [Google Scholar] [CrossRef]
- Mukdadi, O.M.; Datta, S.K.; Dunn, M.L. Elastic guided waves in a layered plate with a rectangular cross section. J. Press. Vessel Technol. 2002, 124, 319–325. [Google Scholar] [CrossRef]
- Hakoda, C.; Rose, J.; Shokouhi, P.; Lissenden, C. Using Floquet periodicity to easily calculate dispersion curves and wave structures of homogeneous waveguides. In 44th Annual Review of Progress in Quantitative NDE; Chimenti, D.E., Bond, L.J., Eds.; Springer: New York, NY, USA, 2017; Volume 37, pp. 020016:1–020016:10. [Google Scholar]
- Groth, E.B.; Iturrioz, I.; Clarke, T.-G.R. The dispersion curve applied in guided wave propagation in prismatic rods. Lat. Am. J. Solids Struct. 2018, 15, 1–27. [Google Scholar] [CrossRef]
- Pavlakovic, B.; Lowe, M. DISPERSE: User’s Manual Version 2.0.16B; Imperial College London: London, UK, 2003; pp. 1–209. [Google Scholar]
- Rayleigh, J.W.S. The Theory of Sound; Dover Publications: New York, NY, USA, 1945; Volume 2, pp. 1–520. [Google Scholar]
- Nakahata, K.; Kono, N. 3-D modelings of an ultrasonic phased array transducer and its radiation properties in solid. In Ultrasonic Waves; Santos, J.A., Ed.; IntechOpen: London, UK, 2012; Available online: http://www.intechopen.com/books/ultrasonic-waves/3-d-modelings-of-an-ultrasonic-phasedarray-transducer-and-its-radiation-properties-in-solid (accessed on 10 August 2019).
- Song, S.-J.; Kim, H.-J. Modeling of radiation beams from ultrasonic transducers in a single medium. J. Korean Soc. Nondestruct. Test. 2000, 20, 91–101. [Google Scholar]
- Kundu, T.; Placko, D.; Rahani, E.K.; Yanagita, T.; Dao, C.M. Ultrasonic field modeling: A comparison of analytical, semi-analytical, and numerical techniques. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 2795–2807. [Google Scholar] [CrossRef]
- Duan, W.; Niu, X.; Gan, T.-H.; Kanfoud, J.; Chen, H.-P. A numerical study on the excitation of guided waves in rectangular plates using multiple point sources. Metals 2017, 7, 1–21. [Google Scholar] [CrossRef]
- Serey, V.; Quaegebeur, N.; Micheau, P.; Masson, P.; Castaings, M.; Renier, M. Selective generation of ultrasonic guided waves in a bi-dimensional waveguide. SHM 2018, 18, 1–13. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Surrounding liquid (wave velocity) | Water (= 1480 m/s) |
| Leaky Lamb wave modes | A(0,n) |
| Plate dimension | Thickness: 1.5 mm Width: 15mm |
| Plate material (wave velocity) | SS304 (= 5800 m/s, = 3160 m/s) |
| Aperture size | Length : 18 mm Width : 15 mm |
| Excitation frequency | 1.0 MHz |
| Attenuation coefficient | 0.168 dB/mm at 1.0 MHz [40] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, S.-J.; Kim, H.-W.; Joo, Y.-S. Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width. Appl. Sci. 2020, 10, 8104. https://doi.org/10.3390/app10228104
Park S-J, Kim H-W, Joo Y-S. Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width. Applied Sciences. 2020; 10(22):8104. https://doi.org/10.3390/app10228104
Chicago/Turabian StylePark, Sang-Jin, Hoe-Woong Kim, and Young-Sang Joo. 2020. "Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width" Applied Sciences 10, no. 22: 8104. https://doi.org/10.3390/app10228104
APA StylePark, S.-J., Kim, H.-W., & Joo, Y.-S. (2020). Leaky Lamb Wave Radiation from a Waveguide Plate with Finite Width. Applied Sciences, 10(22), 8104. https://doi.org/10.3390/app10228104





