Seismic Effectiveness of Multiple Seismic Measures on a Continuous Girder Bridge
Abstract
1. Introduction
2. Theoretical Pounding Analysis of a Bridge
- (1)
- Initially, there is no pounding between the structures, and the pounding stiffness can be regarded as zero.
- (2)
- When the ends are pounding, the pounding stiffness will no longer be zero. If seismic measures are adopted on the bridge, the pounding stiffness will be the stiffness of the control measures.
3. Establishment of a Finite Element Model of a Bridge
3.1. Description of the Bridge
3.2. Simulation of the Bridge and Seismic Measures
3.2.1. Finite Element Model of the Bridge
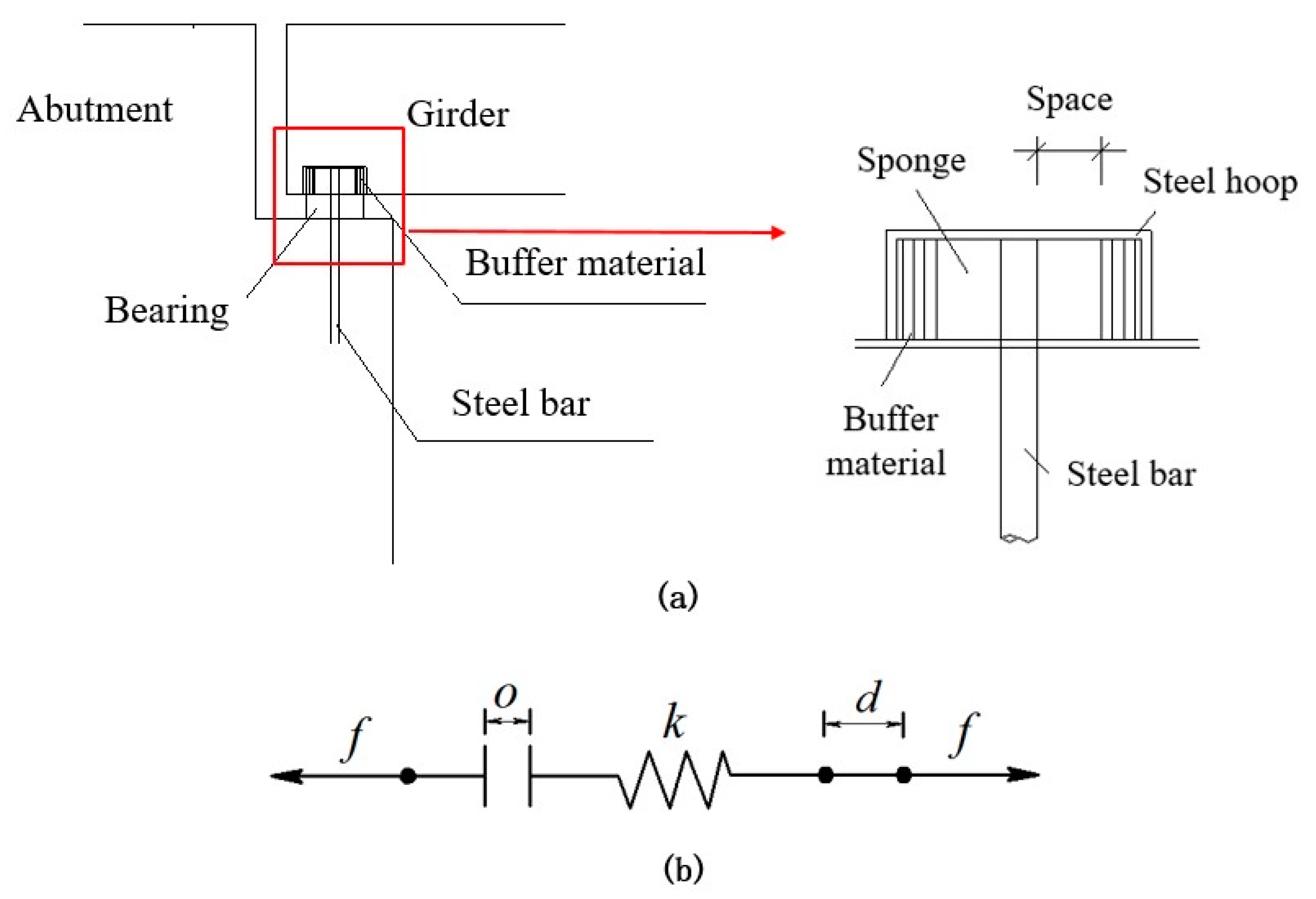
3.2.2. Finite Element Model of Restrainer
- is the initial design space of the restrainer,
- is the relative displacement of the superstructure and substructures of the bridge,
- is the stiffness of the restrainer,
- is the force of the restrainer, the value of which can calculated according to Equation (9):
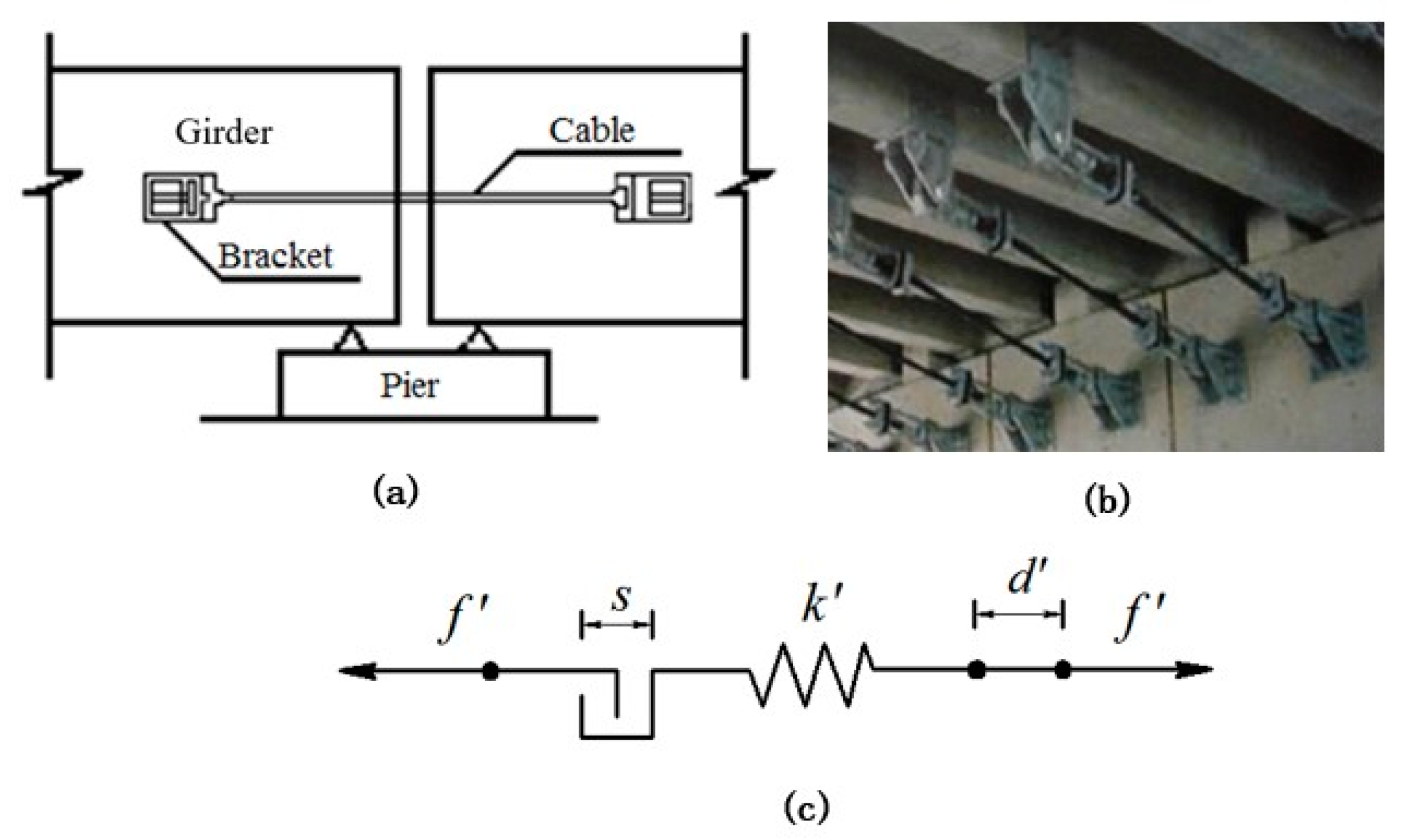
3.2.3. Selection and Simulation of Unseating Prevention Devices
- is the initial space of the unseating prevention device,
- is the relative displacement of superstructure and substructures of the bridge,
- is the stiffness of the unseating prevention device,
- is the force of the unseating prevention device, whose value is calculated according to Equation (10):
3.3. Case Settings
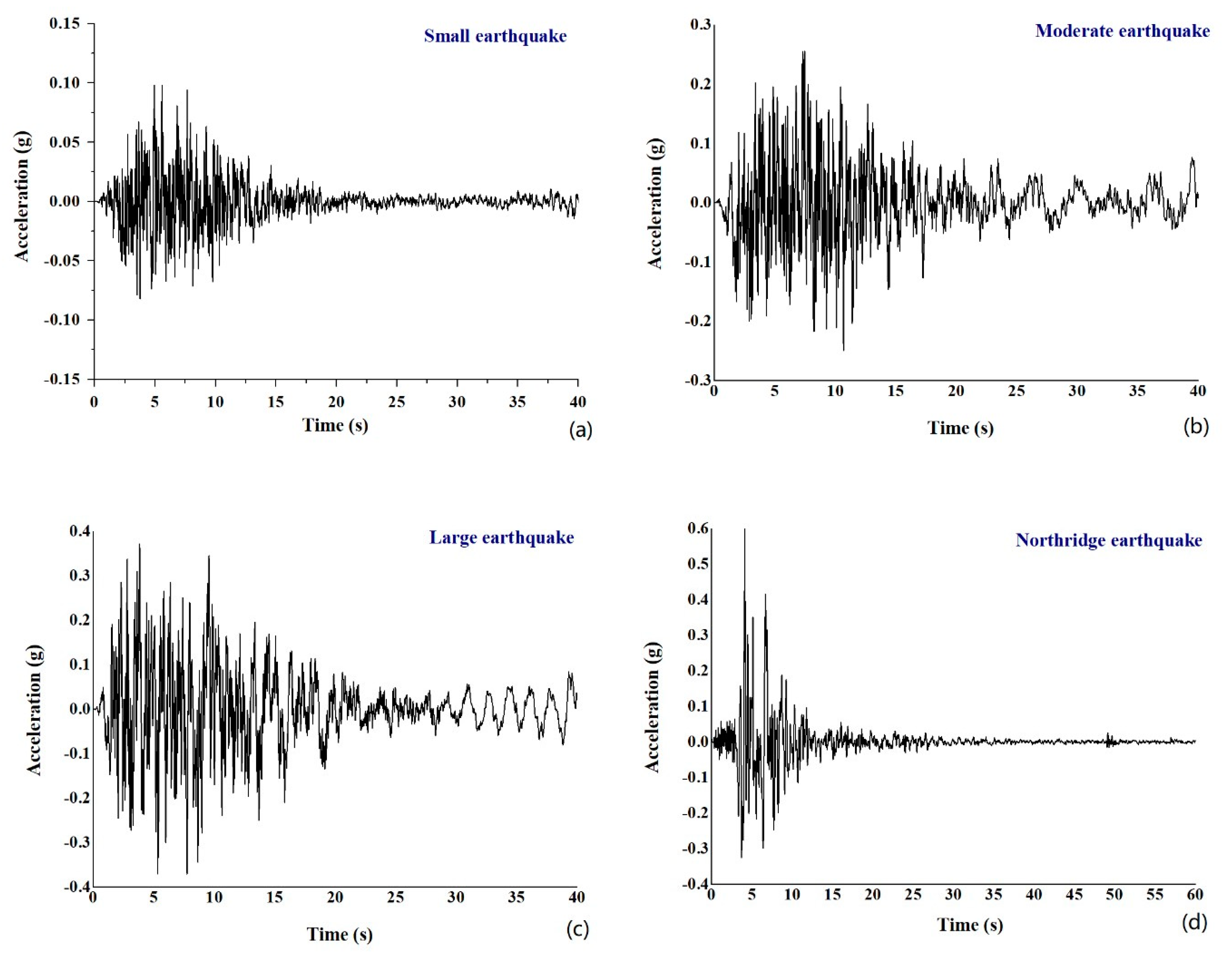
3.4. Earthquakes
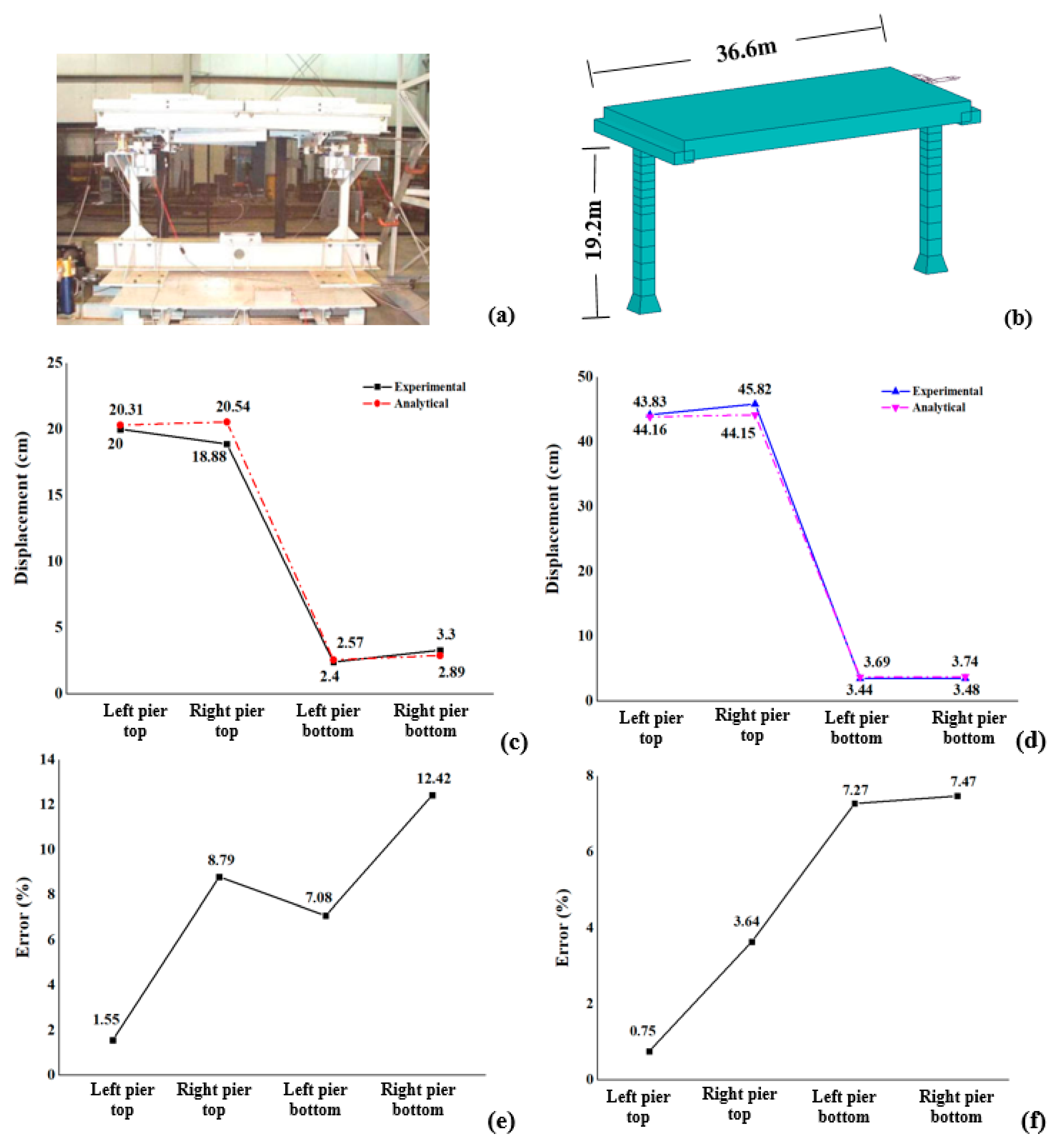
4. Model Validation
5. Calculation Analysis and Discussion
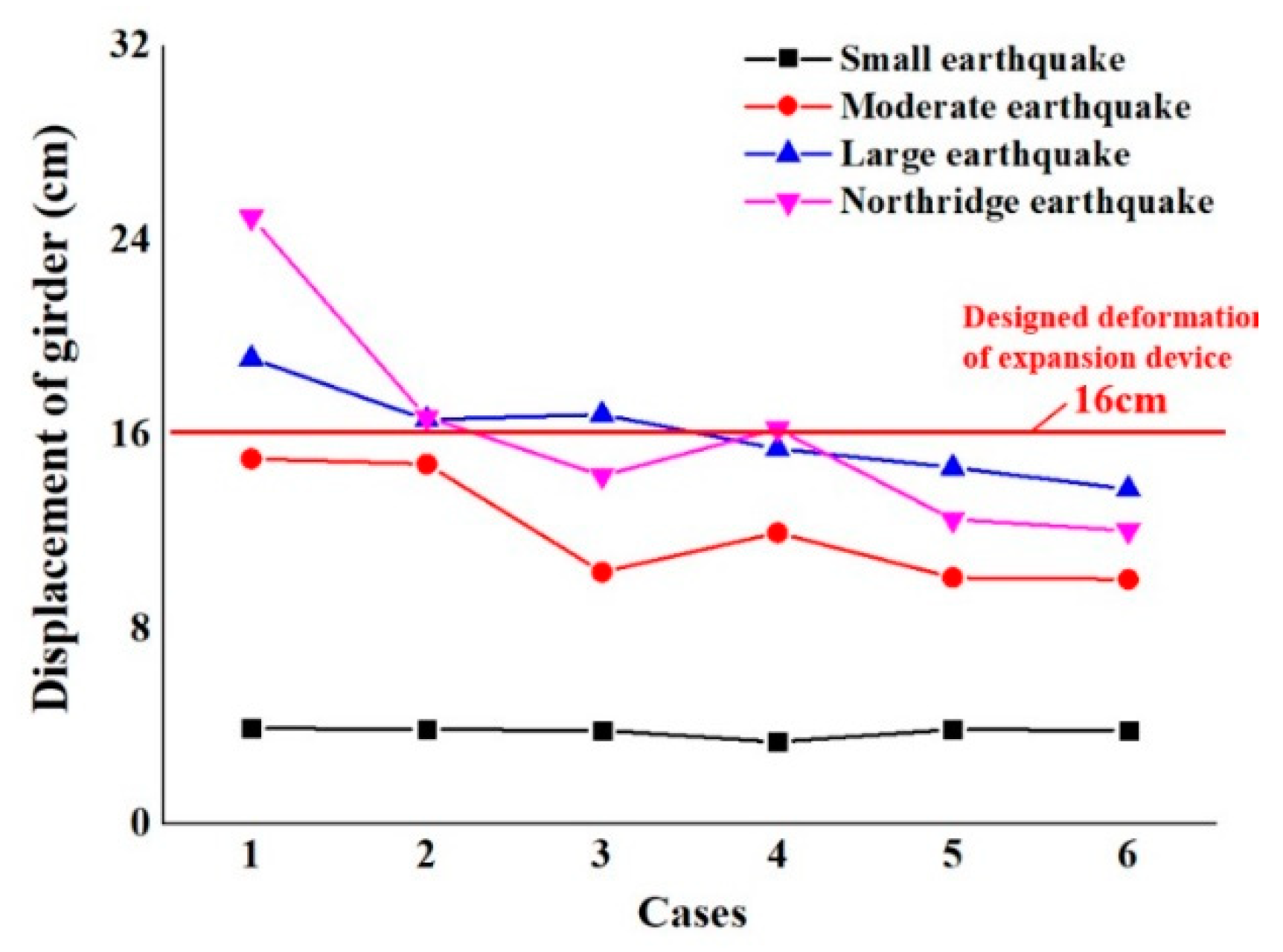
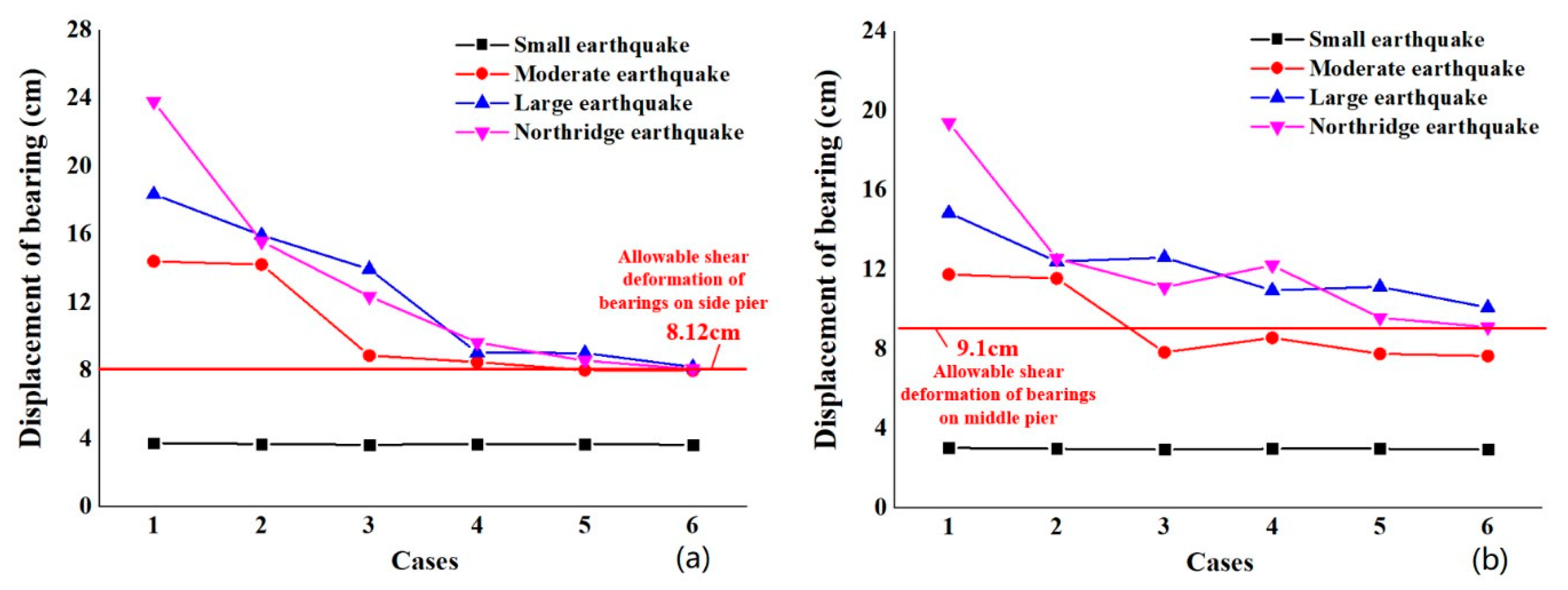
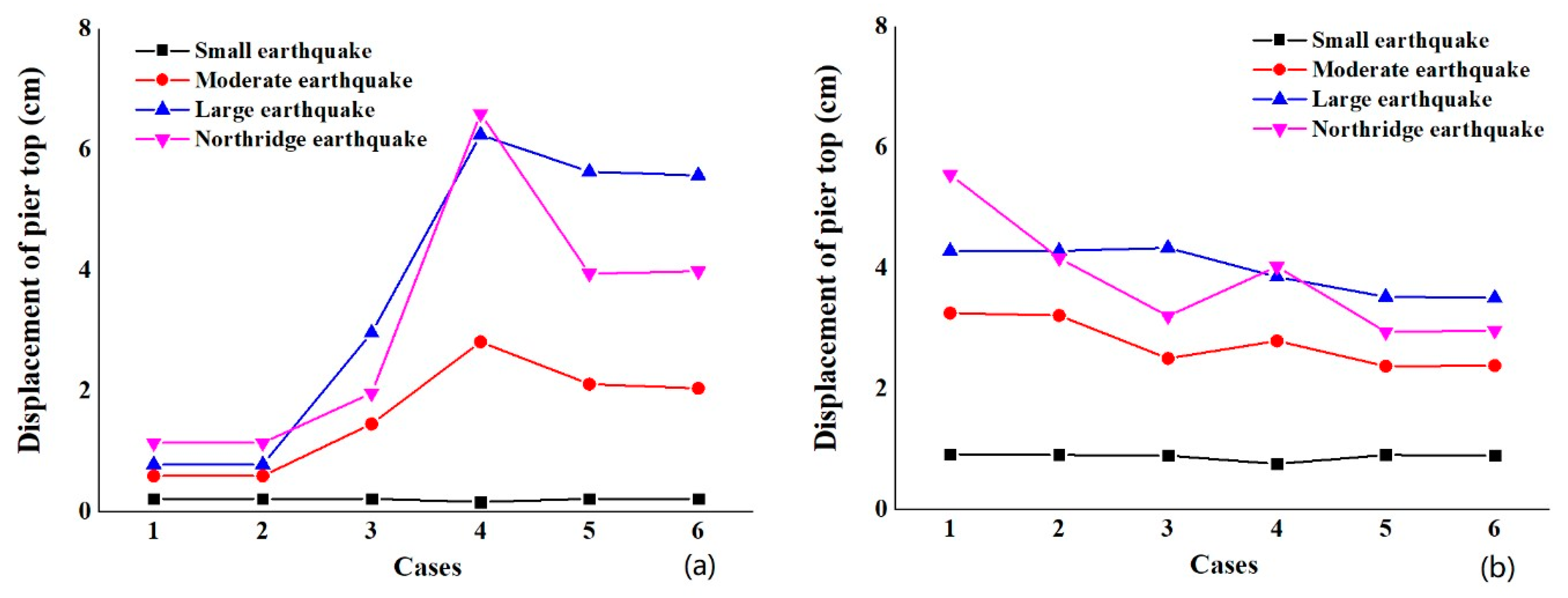
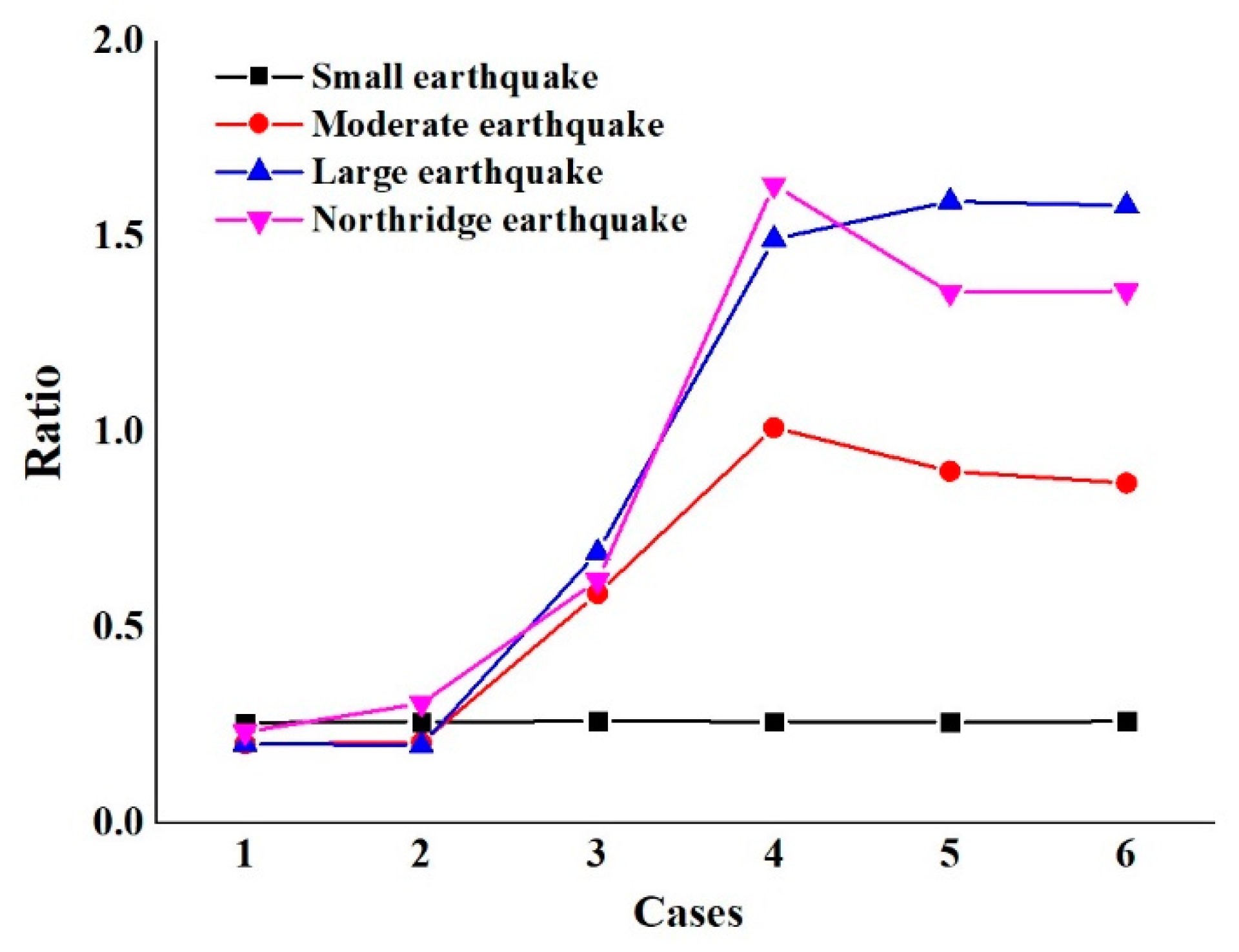
5.1. Displacement of Girders, Bearings, and Pier Top
5.2. Bending Moment
5.3. Internal Force
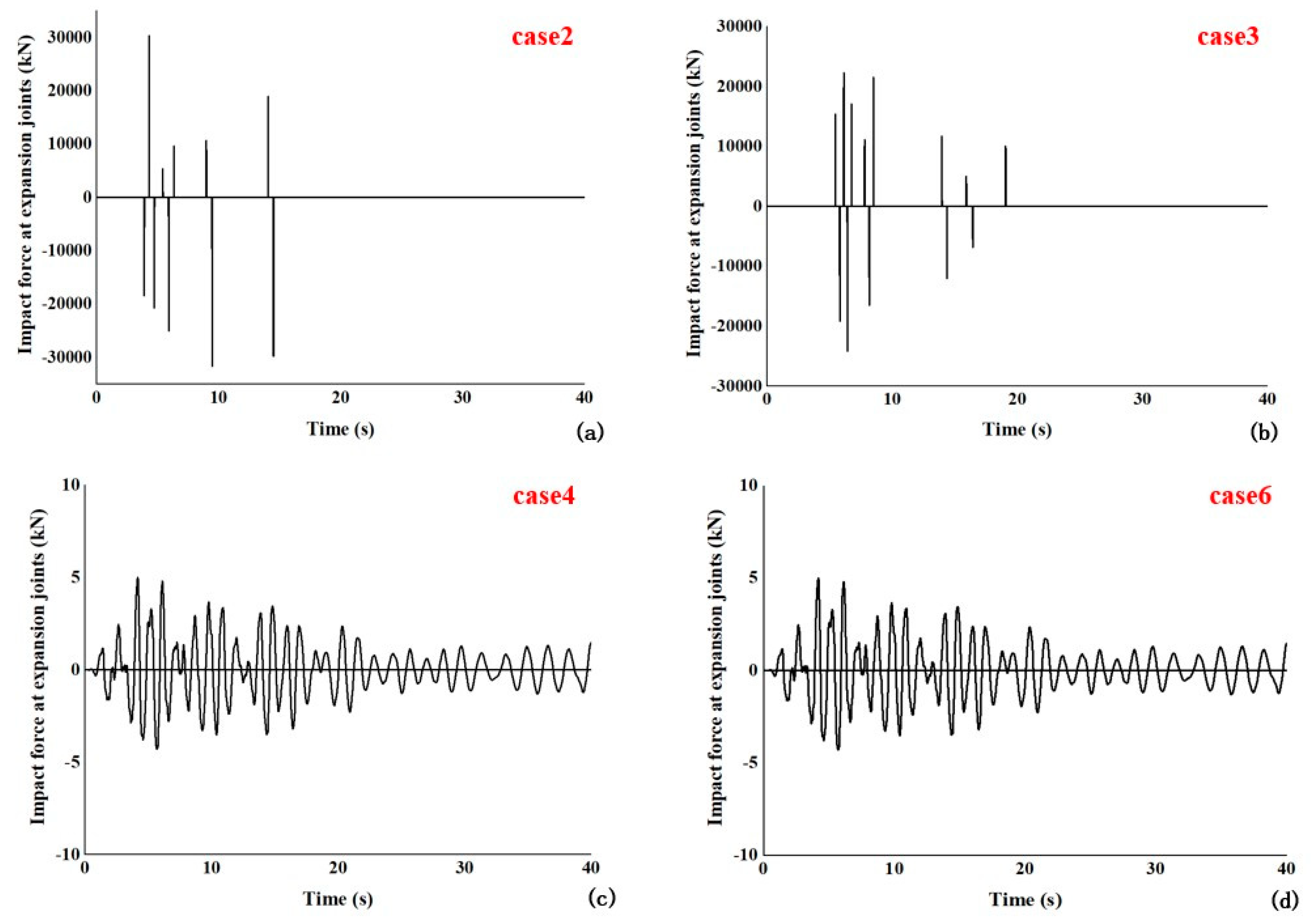
5.3.1. Impact Force at Expansion Joints
5.3.2. Internal Force of Seismic Measures
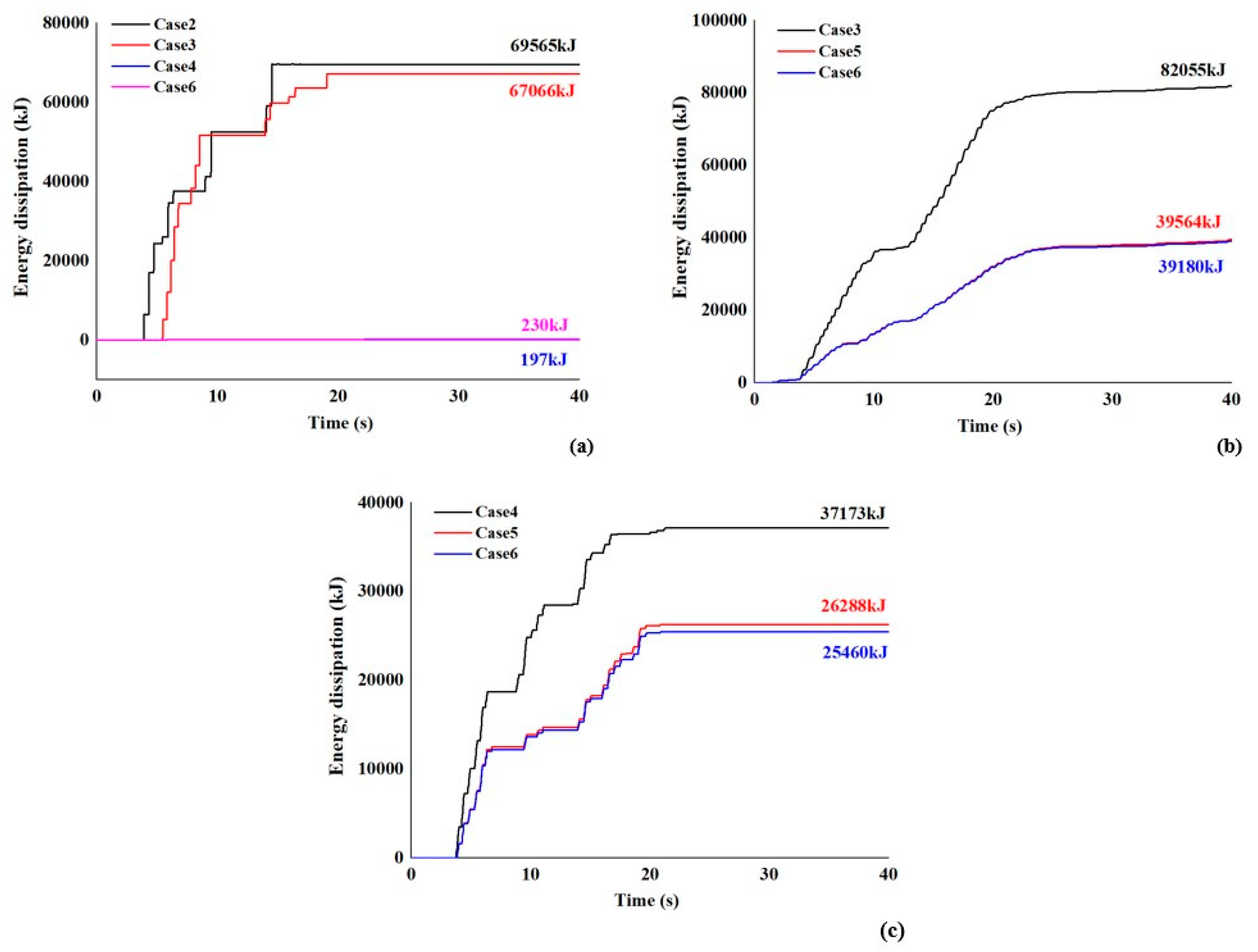
5.4. Energy Dissipation
6. Conclusions
- (1)
- Under larger earthquakes, the initial design of the bridge (Case 1) was found to be damaged by bearing failure, pounding, expansion device failure, and collapse damages without the prevention of seismic measures. For the expansion joint, its maximum displacement—24.92—was much more than its design displacement—16 cm—under the action of the Northridge earthquake. The sliding rubber bearings and laminated rubber bearings have a maximum displacement of 23.78 cm and 19.38 cm, respectively, which are more than their shear deformation of 8.12 cm and 9.1 cm, respectively. The maximum bending moment, 32,594 kN·m, at their bottoms of #2, #3, and #4 piers with laminated rubber bearings is four times the maximum bending moment, 7564 kN·m, at the bottoms of #1 and #5 piers with sliding rubber bearings. The seismic load is unevenly distributed between the piers, leading to a great difference in displacement at the top of each pier.
- (2)
- Damping expansion devices can weaken the seismic response of the bridge slightly; restrainers can effectively reduce the displacement of the bridge and improve the overall stiffness of the bridge under small and moderate earthquakes. However, if the seismic magnitude exceeds a moderate earthquake, the restrainer cannot be effectively limited to the excessive displacements of the bridge. The combination of the restrainer and unseating prevention was found to be more effective at controlling the excessive displacement of the bridge. The expansion joint and bearing have a maximum displacement reduction of 48% and 34%, respectively. As the displacements of bearings and expansion joints were reduced within their allowable deformation, the bridge was prevented from pounding and collapse during earthquakes. In addition, the moment at the bottom and the displacement at the top of #1 and #5 piers greatly increased, and even slightly exceeded those of #2, #3, and #4 piers; however, all the piers have a similar seismic response, and the seismic load is uniformly distributed between the piers.
- (3)
- Under the action of the earthquake, a large displacement occurs to the sliding rubber bearings (#1 and #5 piers), so a smaller seismic load is transferred to #1 and #5 piers. Thus, #2, #3, and #4 piers have a bigger maximum displacement and bending moment than #1 and #5 piers. The restrainer and installed unseating prevention devices increase the connection stiffness between the substructure and superstructure of the bridge with #1 and #5 piers, distribute a bigger seismic load to the substructure, and increase the displacement at the top and bending moment at the bottom of #1 and #5 piers by a factor of more than four compared with the preliminary design. This revealed that seismic measures could increase the overall stiffness of the bridge and more evenly distribute the impact force and seismic energy among piers caused by earthquakes.
- (4)
- Under the action of the large and Northridge earthquakes, there is a big impact force at the expansion joint of the bridge. As no seismic measure is taken, the maximum impact force at the expansion joint is 30,301 kN, which is much more than the designed internal force, 15,000 kN, so the expansion joint is seriously damaged. After the restrainer is installed, the impact force at the expansion joint decreases to some extent, but the impact still exists at the expansion joint. When the restrainer and the unseating prevention devices are used together, the impact force at the expansion joint almost disappears. Seismic measures also bear a large internal force in an earthquake. When the restrainer or unseating prevention devices are used with expansion devices, the internal force is large, and the bridge may be damaged even under the action of the large earthquake. When the expansion device, the restrainer, and the unseating prevention devices are used together, the internal force of the restrainer and the unseating prevention devices are reduced to some extent. Several seismic measures can share the impact force caused by the earthquake load, dissipate the earthquake energy, improve the seismic performance of the structure and seismic measures, and make the force on the structure more reasonable.
Author Contributions
Funding
Conflicts of Interest
References
- Ruangrassamee, A.; Kawashima, K. Control of nonlinear bridge response with pounding effect by variable dampers. Eng. Struct. 2003, 25, 593–606. [Google Scholar] [CrossRef]
- Schanack, F.; Valdebenito, G.; Alvial, J. Seismic damage to bridges during the 27 February 2010 magnitude 8.8 Chile earthquake. Earthq. Spectra 2012, 28, 301–315. [Google Scholar] [CrossRef]
- Di, S.L.; Da, P.F.; Guerrini, G.; CalviG, P.M.; Prota, C. Seismic performance of bridges during the 2016 Central Italy earthquakes. Bull. Earthq. Eng. 2019, 17, 5729–5761. [Google Scholar]
- Kawashima, K.; Takahashi, Y.; Hanbin, G.; Wu, Z.; Zhang, J. Reconnaissance report on damages of bridges in 2008 Wenchuan, China, earthquake. J. Earthq. Eng. 2009, 13, 965–996. [Google Scholar] [CrossRef]
- Jankowski, R.; Wilde, K.; Fujino, Y. Reduction of pounding effects in elevated bridges during earthquakes. Earthq. Eng. Struct. Dyn. 2015, 29, 195–212. [Google Scholar] [CrossRef]
- Selna, L.G.; Malvar, L.J.; Zelinski, R.J. Bridge retrofit testing, Hinge cable restrainers. J. Struct. Eng. 1989, 115, 920–934. [Google Scholar] [CrossRef]
- DesRoches, R.; Pfeifer, T.; Leon, R.T.; Lam, T. Full-scale tests of seismic cable restrainer retrofits for simply supported bridges. J. Bridge Eng. 2003, 8, 191–198. [Google Scholar] [CrossRef]
- Di Sarno, L.; Del Vecchio, C.; Maddaloni, G.; Prota, A. Shake table studies of an as-built RC bridge with smooth bars. Eng. Struct. 2017, 136, 355–368. [Google Scholar] [CrossRef]
- Kawashima, K.; Unjoh, S. The damage of highway bridges in the 1995 Hyogo-ken nanbu earthquake and its impact on Japanese seismic design. J. Earthq. Eng. 1997, 1, 505–541. [Google Scholar] [CrossRef]
- Goel, R.K.; Chopra, A.K. Role of shear keys in seismic behavior of bridges crossing fault-rupture zones. J. Bridge Eng. 2008, 13, 398–408. [Google Scholar] [CrossRef]
- Están, J.D.J.W.; Santa María, H.; Riddell, R.; Arrate, C. Influence of the use of external shear keys on the seismic behavior of Chilean highway bridges. Eng. Struct. 2017, 147, 613–624. [Google Scholar] [CrossRef]
- Liu, C.; Gao, R. Design method for steel restrainer bars on railway bridges subjected to spatially varying earthquakes. Eng. Struct. 2018, 159, 198–212. [Google Scholar] [CrossRef]
- Choi, E.; Park, J.; Yoon, S.-J.; Choi, D.-H.; Park, C. Comparison of seismic performance of three restrainers for multiple-span bridges using fragility analysis. Nonlinear Dyn. 2010, 61, 83–99. [Google Scholar] [CrossRef]
- Raheem, S.E.A. Pounding mitigation and unseating prevention at expansion joints of isolated multi-span bridges. Eng. Struct. 2009, 31, 2345–2356. [Google Scholar] [CrossRef]
- Alam, M.S.; Bhuiyan, M.A.R.; Billah, A.H.M.M. Seismic fragility assessment of SMA-bar restrained multi-span continuous highway bridge isolated by different laminated rubber bearings in medium to strong seismic risk zones. Bull. Earthq. Eng. 2012, 10, 1885–1909. [Google Scholar] [CrossRef]
- Zhu, S.; Qiu, C.X. Incremental dynamic analysis of highway bridges with novel shape memory alloy isolators. Adv. Struct. Eng. 2014, 17, 429–438. [Google Scholar] [CrossRef]
- Saiidi, M.; Randall, M.; Maragakis, E.; Isakovic, T. Seismic restrainer design methods for simply supported bridges. J. Bridge Eng. 2001, 6, 307–315. [Google Scholar] [CrossRef]
- DesRoches, R.; Fenves, G.L. Design of Seismic Cable Hinge Restrainers for Bridges. J. Struct. Eng. 2000, 126, 500–509. [Google Scholar] [CrossRef]
- DesRoches, R.; Fenves, G.L. Simplified restrainer design procedure for multiple-frame bridges. Earthq. Spectra 2001, 17, 551–567. [Google Scholar] [CrossRef]
- Vlassis, A.G.; Maragakis, E.; Saiidi, M. Experimental evaluation of longitudinal seismic performance of bridge restrainers at in-span hinges. J. Test. Eval. 2004, 32, 96–105. [Google Scholar] [CrossRef]
- Li, S.; Hedayati, D.F.; Wang, J.Q.; Alam, M.S. Displacement-Based Seismic Design of Steel, FRP, and SMA Cable Restrainers for Isolated Simply Supported Bridges. J. Bridge Eng. 2018, 23, 04018032. [Google Scholar] [CrossRef]
- Kawashima, K.; Watanabe, G. Effectiveness of cable-restrainer for mitigating rotation of a skewed bridge subjected to strong ground shaking. Doboku Gakkai Ronb. 2001, 675, 141–159. [Google Scholar] [CrossRef]
- Julian, F.D.R.; Hayashikawa, T.; Obata, T. Seismic performance of isolated curved steel viaducts equipped with deck unseating prevention cable restrainers. J. Constr. Steel Res. 2007, 63, 237–253. [Google Scholar] [CrossRef]
- Won, J.H.; Mha, H.S.; Cho, K.I.; Kim, S.-H. Effects of the restrainer upon bridge motions under seismic excitations. Eng. Struct. 2008, 30, 3532–3544. [Google Scholar] [CrossRef]
- Shrestha, B.; Hao, H.; Bi, K. Effectiveness of using rubber bumper and restrainer on mitigating pounding and unseating damage of bridge structures subjected to spatially varying ground motions. Eng. Struct. 2014, 79, 195–210. [Google Scholar] [CrossRef]
- Guo, J.J.; Zhong, J.; Dang, X.Z.; Yuan, W.C. Influence of Multidirectional Cable Restrainer on Seismic Fragility of a Curved Bridge. J. Bridge Eng. 2019, 24, 04019001. [Google Scholar] [CrossRef]
- Andrawes, B.; DesRoches, R. Comparison between shape memory alloy seismic restrainers and other bridge retrofit devices. J. Bridge Eng. 2007, 12, 700–709. [Google Scholar] [CrossRef]
- Ghosh, G.; Singh, Y.; Thakkar, S.K. Seismic response of a continuous bridge with bearing protection devices. Eng. Struct. 2011, 33, 1149–1156. [Google Scholar] [CrossRef]
- Kawashima, K.; Gaku, S. Effect of restrainers to mitigate pounding between adjacent decks subjected to a strong ground motion. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000. [Google Scholar]
- Polycarpou, P.C.; Komodromos, P. Numerical investigation of potential mitigation measures for poundings of seismically isolated buildings. Earthq. Struct. 2011, 2, 1–24. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Qin, X.X. Research on Bridge Damage-Reduction Seismic Performance with Variable Parameter Cable-Unseating Prevention Devices. Appl. Mech. Mater. 2014, 638, 977–982. [Google Scholar] [CrossRef]
- Zhu, P.; Abe, M.; Fujino, Y. Evaluation of pounding countermeasures and serviceability of elevated bridges during seismic excitation using 3D modelling. Earthq. Eng. Struct. Dyn. 2004, 33, 591–609. [Google Scholar] [CrossRef]
- Shrestha, B.; Hao, H.; Bi, K. Devices for protecting bridge superstructure from pounding and unseating damages: An overview. Struct. Infrastruct. Eng. 2017, 13, 313–330. [Google Scholar] [CrossRef]
- Xiang, N.; Li, J. Seismic performance of highway bridges with different transverse unseating-prevention devices. J. Bridge Eng. 2016, 21, 04016045. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Cui, Y.; Liu, J. Collapse-Prevention Design and Damage-Reduction Seismic Performance Analysis of Bridges. Appl. Mech. Mater. 2014, 640, 1997–2000. [Google Scholar] [CrossRef]
- Guan, Z.G.; Li, J.Z.; Xu, Y. Performance test of energy dissipation bearing and its application in seismic control of a long-span bridge. J. Bridge Eng. 2009, 15, 622–630. [Google Scholar] [CrossRef]
- Padgett, J.E.; Nielson, B.G.; DesRoches, R. Selection of optimal intensity measures in probabilistic seismic demand models of highway bridge portfolios. Earthq. Eng. Struct. Dyn. 2008, 37, 711–725. [Google Scholar] [CrossRef]
- Guo, A.; Li, Z.; Li, H.; Ou, J. Experimental and analytical study on pounding reduction of base-isolated highway bridges using MR dampers. Earthq. Eng. Struct. Dyn. 2009, 38, 1307–1333. [Google Scholar] [CrossRef]
- Andrawes, B.; Reginald, D. Unseating prevention for multiple frame bridges using superelastic devices. Smart Mater. Struct. 2005, 14, S60. [Google Scholar] [CrossRef]
- Wang, L.; Su, J.W.; Gu, Z.L.; Tang, L.Y. Numerical Study on Flow Field and Pollutant Dispersion in an Ideal Street Canyon within a Real Tree Model at Different Wind Velocities. Comput. Math. Appl. 2020. [Google Scholar] [CrossRef]
- Cheng, C.T. Shaking table tests of a self-centering designed bridge substructure. Eng. Struct. 2008, 30, 3426–3433. [Google Scholar] [CrossRef]
- Johnson, R.; Padgett, J.E.; Maragakis, M.E.; Desroches, R. Large scale testing of nitinol shape memory alloy devices for retrofitting of bridges. Smart Mater. Struct. 2008, 17, 035018. [Google Scholar] [CrossRef]
- Sahasrabudhe, S.; Nagarajaiah, S. Experimental study of sliding base-isolated buildings with magnetorheological dampers in near-fault earthquakes. J. Struct. Eng. 2005, 131, 1025–1034. [Google Scholar] [CrossRef]
- Sahasrabudhe, S.; Nagarajaiah, S. Semi-active control of sliding isolated bridges using MR dampers: An experimental and numerical study. Earthq. Eng. Struct. Dyn. 2010, 34, 965–983. [Google Scholar] [CrossRef]
- Xiang, N.; Alam, M.S.; Li, J. Shake table studies of a highway bridge model by allowing the sliding of laminated-rubber bearings with and without restraining devices. Eng. Struct. 2018, 171, 583–601. [Google Scholar] [CrossRef]
- Yi, J.; Yang, H.; Li, J. Experimental and numerical study on isolated simply-supported bridges subjected to a fault rupture. Soil Dyn. Earthq. Eng. 2019, 127, 105819. [Google Scholar] [CrossRef]
- Kim, Y.; Park, G. Nonlinear dynamic response structural optimization using equivalent static loads. Comput. Method Appl. Mech. Eng. 2010, 199, 660–676. [Google Scholar] [CrossRef]
- Wang, X.Y.; Zhu, B.; Cui, S. Research on collapse process of cable-stayed bridges under strong seismic excitations. Shock Vib. 2017, 2017, 7185281. [Google Scholar] [CrossRef]
- Jiang, X.; Kolstein, M.H.; Bijlaard, F.S.K. Experimental and numerical study on mechanical behavior of an adhesively-bonded joint of FRP–steel composite bridge under shear loading. Compos. Struct. 2014, 108, 387–399. [Google Scholar] [CrossRef]
- Lee, T.Y.; Hung, W.H.; Chung, K.J. Seismic-induced collapse simulation of bridges using simple implicit dynamic analysis. Eng. Struct. 2018, 177, 1–11. [Google Scholar] [CrossRef]
- Xiang, N.; Goto, Y.; Obata, M.; ShahriaAlam, M. Passive seismic unseating prevention strategies implemented in highway bridges: A state-of-the-art review. Eng. Struct. 2019, 197, 77–93. [Google Scholar] [CrossRef]
- DesRoches, R.; Muthukumar, S. Effect of pounding and restrainers on seismic response of multiple-frame bridges. J. Struct. Eng. 2002, 128, 860–869. [Google Scholar] [CrossRef]
- Chouw, N.; Hao, H. Seismic design of bridge structures with allowance for large relative girder movements to avoid pounding. Bull. N. Z. Soc. Earthq. Eng. 2009, 42, 75–85. [Google Scholar] [CrossRef]



| Parameters of Bearings | Slide Rubber Bearings | Laminated Rubber Bearings |
|---|---|---|
| Plane size of bearings (mm) | 400 × 450 | 400 × 450 |
| Maximum bearing pressure/(kN) | 1716 | 3481 |
| Height of bearings (mm) | 116 | 130 |
| Total thickness of rubber layer (mm) | 82 | 95 |
| Longitudinal displacement of bearing without braking force (mm) | / | 45 |
| Maximum longitudinal displacement of bearing considering braking force (mm) | 90 | 63 |
| Allowable shear deformation (mm) | 81.2 | 91 |
| Primary Design Bridge | Expansion Device | Restrainer | Unseating Prevention Device | |
|---|---|---|---|---|
| Case 1 | Yes | No | No | No |
| Case 2 | Yes | Yes | No | No |
| Case 3 | Yes | Yes | Yes | No |
| Case 4 | Yes | Yes | No | Yes |
| Case 5 | Yes | No | Yes | Yes |
| Case 6 | Yes | Yes | Yes | Yes |
| Seismic Measures | Small Earthquake | Moderate Earthquake | Large Earthquake | Northridge Earthquake | |
|---|---|---|---|---|---|
| Case 2 | Expansion devices | 1 | 3 | 30,301 | 29,973 |
| Case 3 | Expansion devices | 1 | 3 | 28,973 | 4 |
| Restrainer | 49 | 582 | 1209 | 941 | |
| Case 4 | Expansion devices | 1 | 3 | 5 | 5 |
| Unseating prevention device | 0 | 1814 | 3213 | 3691 | |
| Case 5 | Restrainer | 52 | 511 | 594 | 544 |
| Unseating prevention device | 0 | 703 | 2587 | 1461 | |
| Case 6 | Expansion devices | 1 | 3 | 5 | 3 |
| Restrainer | 49 | 509 | 593 | 548 | |
| Unseating prevention device | 0 | 667 | 2589 | 1556 |
| Earthquakes | Positions | Displacement of Girder (cm) | Case6/Case1 (%) | Displacement of Pier Top (cm) | Case6/Case1 (%) | Bearing DisplacEment (cm) | Case 6/Case 1 (%) | Bending Moment of Pier Bottom (kN·m) | Case 6/Case 1 (%) |
|---|---|---|---|---|---|---|---|---|---|
| Small Earthquake | Side pier | 3.83 | 0.98 | 0.21 | 1.00 | 3.62 | 0.98 | 1377 | 1.00 |
| middle pier | 3.83 | 0.98 | 0.89 | 0.98 | 2.93 | 0.97 | 5298 | 0.99 | |
| Moderate Earthquake | Side pier | 10.03 | 0.67 | 2.04 | 3.46 | 7.97 | 0.55 | 12,363 | 3.20 |
| middle pier | 10.03 | 0.67 | 2.38 | 0.73 | 7.64 | 0.65 | 14,232 | 0.74 | |
| Large Earthquake | Side pier | 13.75 | 0.72 | 5.57 | 7.14 | 8.18 | 0.45 | 32,803 | 6.43 |
| middle pier | 13.57 | 0.71 | 3.5 | 0.82 | 10.07 | 0.68 | 20,783 | 0.82 | |
| Northridge Earthquake | Side pier | 12.05 | 0.48 | 3.98 | 3.49 | 8.07 | 0.34 | 23,924 | 3.16 |
| middle pier | 12.05 | 0.48 | 2.96 | 0.53 | 9.09 | 0.47 | 17,592 | 0.54 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Shi, Y.; Liu, D. Seismic Effectiveness of Multiple Seismic Measures on a Continuous Girder Bridge. Appl. Sci. 2020, 10, 624. https://doi.org/10.3390/app10020624
Zhang Y, Shi Y, Liu D. Seismic Effectiveness of Multiple Seismic Measures on a Continuous Girder Bridge. Applied Sciences. 2020; 10(2):624. https://doi.org/10.3390/app10020624
Chicago/Turabian StyleZhang, Yumin, Yun Shi, and Dengke Liu. 2020. "Seismic Effectiveness of Multiple Seismic Measures on a Continuous Girder Bridge" Applied Sciences 10, no. 2: 624. https://doi.org/10.3390/app10020624
APA StyleZhang, Y., Shi, Y., & Liu, D. (2020). Seismic Effectiveness of Multiple Seismic Measures on a Continuous Girder Bridge. Applied Sciences, 10(2), 624. https://doi.org/10.3390/app10020624





