Propagation of Rectangular Multi-Gaussian Schell-Model Array Beams through Free Space and Non-Kolmogorov Turbulence
Abstract
1. Introduction
2. Propagation Analysis of MGSM Array Beams
2.1. Analytical Expressions of MGSM Array Beams
2.2. Propagation Theory
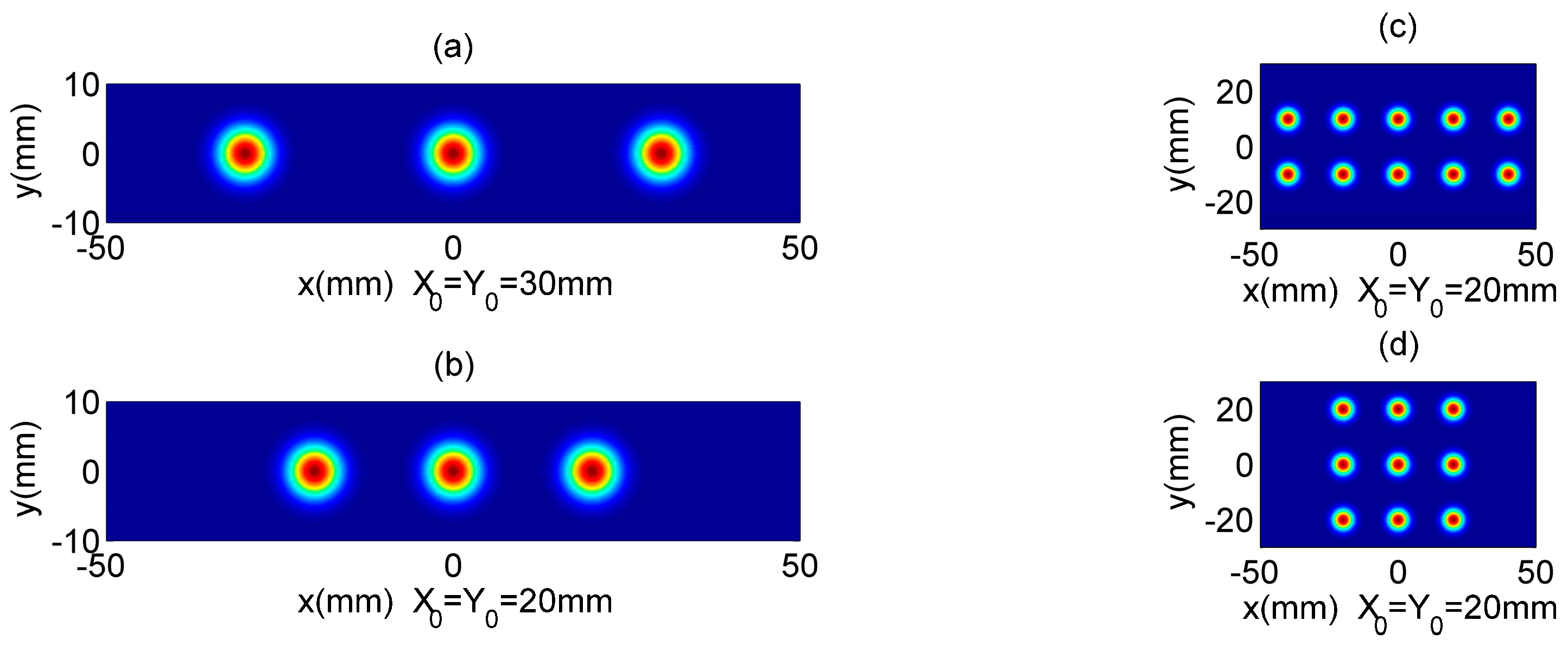
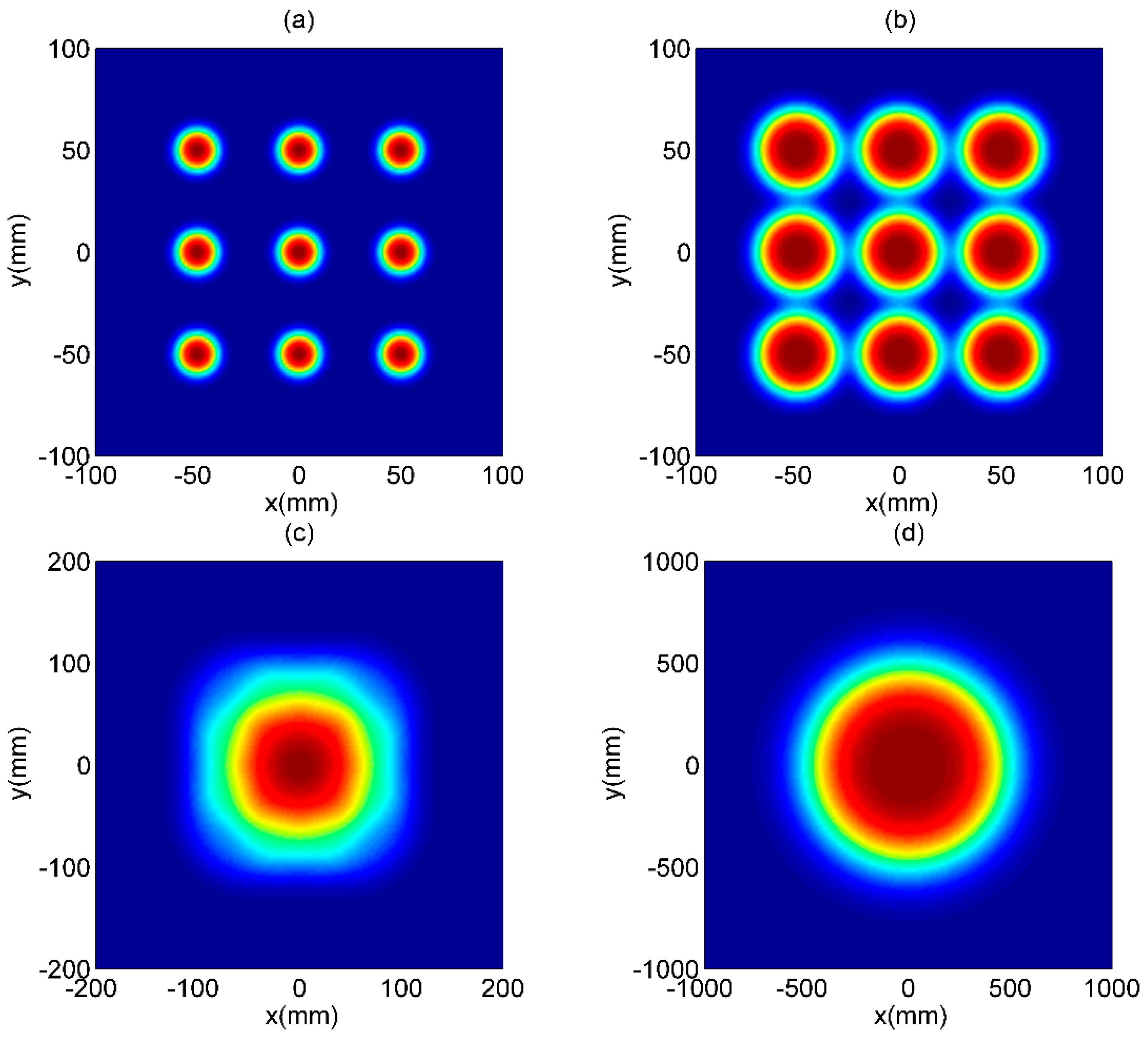
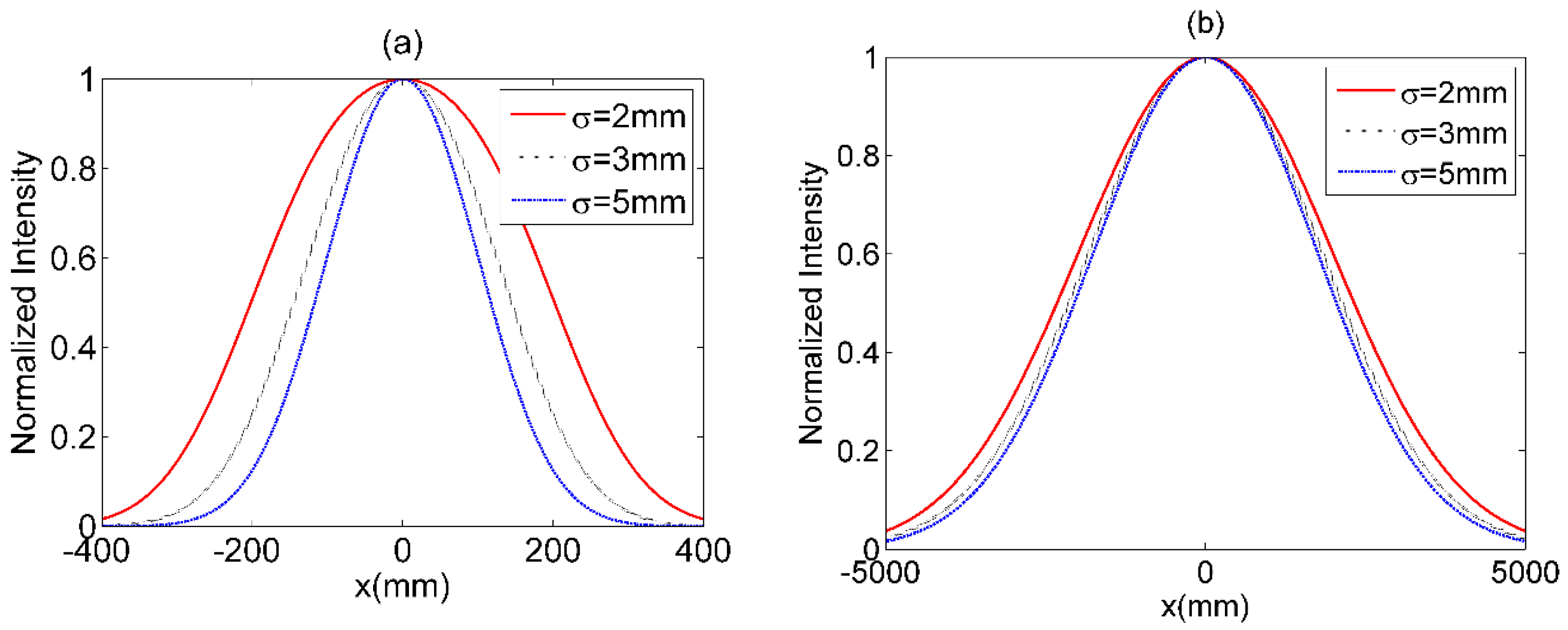
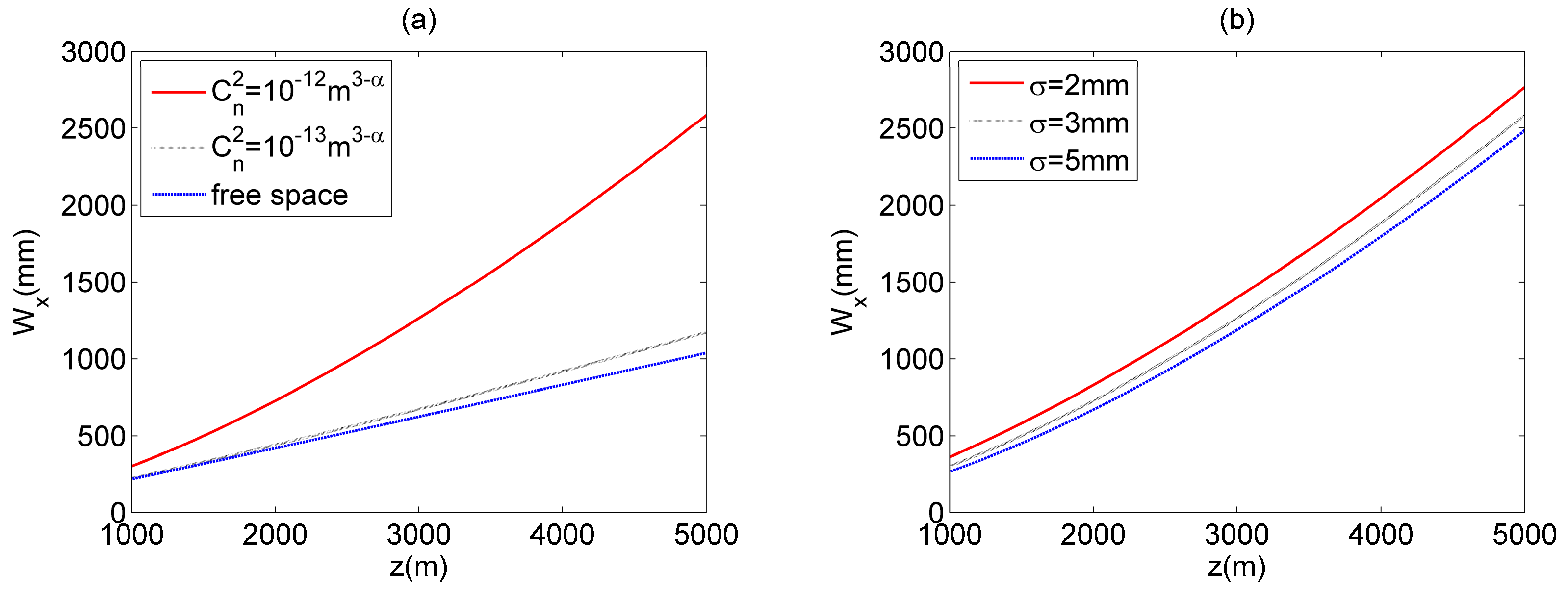
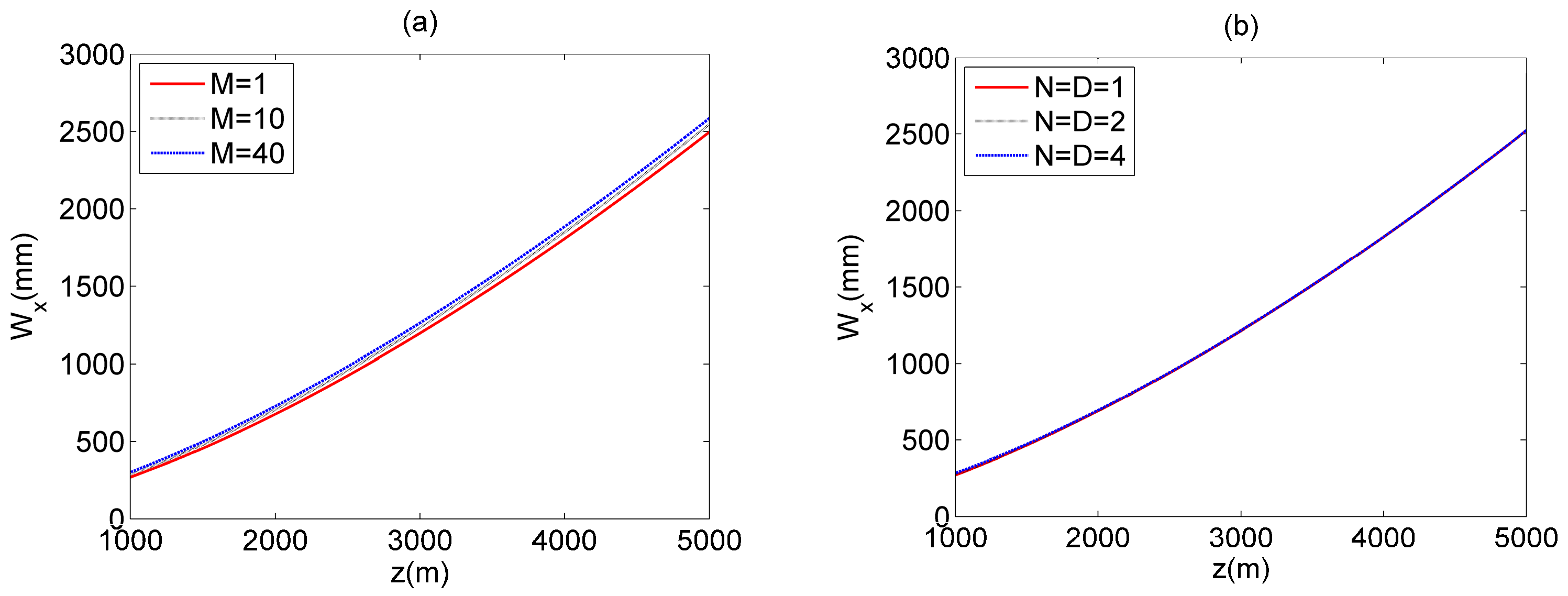
3. Numerical Examples
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Eyyuboglu, H.T.; Baykal, Y. Average intensity and spreading of cosh-Gaussian laser beams in the turbulent atmosphere. Appl. Opt. 2005, 44, 976–983. [Google Scholar] [CrossRef] [PubMed]
- Cai, Y.J.; Lin, Q.; Baykal, Y.; Eyyuboglu, H.T. Off-axis Gaussian Schell-model beam and partially coherent laser array beam in a turbulent atmosphere. Opt. Commun. 2007, 278, 157–167. [Google Scholar] [CrossRef]
- Zhu, Y.B.; Zhao, D.M.; Du, X.Y. Propagation of stochastic Gaussian-Schell model array beams in turbulent atmosphere. Opt. Express 2008, 16, 18437–18442. [Google Scholar] [CrossRef]
- Zhou, G. Average intensity and spreading of super Lorentz-Gauss modes in turbulent atmosphere. Appl. Phys. B 2010, 101, 371–379. [Google Scholar] [CrossRef]
- Wang, D.; Wang, F.; Cai, Y.; Chen, J. Evolution properties of the complex degree of coherence of a partially coherent Laguerre–Gaussian beam in turbulent atmosphere. J. Mod. Opt. 2012, 59, 372–380. [Google Scholar] [CrossRef]
- Zhang, L.C.; Yin, X.; Zhu, Y. Polarization fluctuations of partially coherent Hermite-Gaussian beams in a slant turbulent channel. Opt. Int. J. Light Electron Opt. 2014, 125, 3272–3276. [Google Scholar] [CrossRef]
- Liu, D.; Wang, Y.; Wang, G.; Yin, H. Propagation Properties of a Partially Coherent Flat-Topped Vortex Hollow Beam in Turbulent Atmosphere. J. Opt. Soc. Korea 2016, 20, 1–7. [Google Scholar] [CrossRef]
- Liu, D.; Luo, X.; Wang, G.; Wang, Y. Spectral and Coherence Properties of Spectrally Partially Coherent Gaussian Schell-model Pulsed Beams Propagating in Turbulent Atmosphere. Curr. Opt. Photonics 2017, 1, 271–277. [Google Scholar]
- Liu, Y.; Zhang, K.; Chen, Z.; Pu, J. Scintillation index of double vortex beams in turbulent atmosphere. Opt. Int. J. Light Electron Opt. 2019, 181, 571–574. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, X.; Yuan, X. Performance analysis of sinh-Gaussian vortex beams propagation in turbulent atmosphere. Opt. Commun. 2019, 440, 100–105. [Google Scholar] [CrossRef]
- Malik, M.; O’Sullivan, M.; Rodenburg, B.; Mirhosseini, M.; Leach, J.; Lavery, M.P.J.; Padgett, M.J.; Boyd, R.W. Influence of atmospheric turbulence on optical communications using orbital angular momentum for encoding. Opt. Express 2012, 20, 13195–13200. [Google Scholar] [CrossRef]
- Toselli, I.; Andrews, L.C.; Phillips, R.L.; Ferrero, V. Free-space optical system performance for laser beam propagation through non-Kolmogorov turbulence. Opt. Eng. 2008, 47, 026003. [Google Scholar]
- Shchepakina, E.; Korotkova, O. Second-order statistics of stochastic electromagnetic beams propagating through non-Kolmogorov turbulence. Opt. Express 2010, 18, 10650–10658. [Google Scholar] [CrossRef]
- Tang, H.; Ou, B. Average spreading of a linear Gaussian-Schell model beam array in non-Kolmogorov turbulence. Appl. Phys. B 2011, 104, 1007–1012. [Google Scholar] [CrossRef]
- Jiang, Y.S.; Wang, S.H.; Zhang, J.H.; Ou, J.; Tang, H. Spiral spectrum of Laguerre-Gaussian beam propagation in non-Kolmogorov turbulence. Opt. Commun. 2013, 303, 38–41. [Google Scholar] [CrossRef]
- Xu, H.F.; Zhang, Z.; Qu, J.; Huang, W. Propagation factors of cosine-Gaussian-correlated Schell-model beams in non-Kolmogorov turbulence. Opt. Express 2014, 22, 22479–22489. [Google Scholar] [CrossRef]
- Wang, X.; Yao, M.; Yi, X.; Qiu, Z.; Liu, Z. Spreading and evolution behavior of coherent vortices of multi-Gaussian Schell-model vortex beams propagating through non-Kolmogorov turbulence. Opt. Laser Technol. 2017, 87, 99–107. [Google Scholar] [CrossRef]
- Song, Z.-Z.; Liu, Z.-J.; Zhou, K.-Y.; Sun, Q.-G.; Liu, S.-T. Propagation factor of electromagnetic concentric rings Schell-model beams in non-Kolmogorov turbulence. Chin. Phys. B 2017, 26, 024201. [Google Scholar] [CrossRef]
- Zhi, D.; Tao, R.M.; Zhou, P.; Ma, Y.X.; Wu, W.M.; Wang, X.L.; Si, L. Propagation of ring Airy Gaussian beams with optical vortices through anisotropic non-Kolmogorov turbulence. Opt. Commun. 2017, 387, 157–165. [Google Scholar] [CrossRef]
- Tang, L.; Wang, H.; Zhang, X.; Zhu, S. Propagation properties of partially coherent Lommel beams in non-Kolmogorov turbulence. Opt. Commun. 2018, 427, 79–84. [Google Scholar] [CrossRef]
- Huang, Y.; Zeng, A. Effect of anisotropic non-Kolmogorov turbulence on the evolution behavior of Gaussian Schell-model vortex beams. Opt. Commun. 2019, 436, 63–68. [Google Scholar] [CrossRef]
- Yu, L.; Zhang, Y.X. Beam spreading and wander of partially coherent Lommel-Gaussian beam in turbulent biological tissue. J. Quant. Spectrosc. Radiat. Transf. 2018, 217, 315–320. [Google Scholar] [CrossRef]
- Song, X.; Wang, H.; Xiong, J.; Wang, K.; Zhang, X.; Luo, K.; Wu, L. Experimental observation of quantum Talbot effect. Phys. Rev. Lett. 2011, 107, 033902. [Google Scholar] [CrossRef]
- Zhang, Y.; Wen, J.; Zhu, S.; Xiao, M. Nonlinear Talbot effect. Phys. Rev. Lett. 2010, 104, 183901. [Google Scholar] [CrossRef]
- Wei, G.-X.; Lu, L.-L.; Guo, C.-S. Generation of optical vortex array based on the fractional Talbot effect. Opt. Commun. 2009, 282, 2665–2669. [Google Scholar] [CrossRef]
- Sarenac, D.; Cory, D.G.; Nsofini, J.; Hincks, I.; Miguel, P.; Arif, M.; Clark, C.W.; Huber, M.G.; Pushin, D.A. Generation of a Lattice of Spin-Orbit Beams via Coherent Averaging. Phys. Rev. Lett. 2018, 121, 183602. [Google Scholar] [CrossRef]
- Eyyuboğlu, H.T.; Baykal, Y.; Cai, Y. Scintillations of laser array beams. Appl. Phys. B 2008, 91, 265–271. [Google Scholar] [CrossRef]
- Lu, L.; Ji, X.L.; Deng, J.P.; Li, X.Q. A further study on the spreading and directionality of Gaussian array beams in non-Kolmogorov turbulence. Chin. Phys. B 2014, 23, 064209. [Google Scholar] [CrossRef]
- Zhou, G.Q. Propagation of a radial phased-locked Lorentz beam array in turbulent atmosphere. Opt. Express 2011, 19, 24699–24711. [Google Scholar] [CrossRef]
- Lu, L.; Wang, Z.Q.; Zhang, J.H.; Zhang, P.F.; Qiao, C.H.; Fan, C.Y.; Ji, X.L. Average intensity of M x N Gaussian array beams in oceanic turbulence. Appl. Opt. 2015, 54, 7500–7507. [Google Scholar] [CrossRef]
- Wang, F.; Liu, X.L.; Cai, Y.J. Propagation of Partially Coherent Beam in Turbulent Atmosphere: A Review. Prog. Electromagn. Res. 2015, 150, 123–143. [Google Scholar] [CrossRef]
- Mao, Y.H.; Mei, Z.R.; Gu, J.G. Propagation of Gaussian Schell-model Array beams in free space and atmospheric turbulence. Opt. Laser Technol. 2016, 86, 14–20. [Google Scholar] [CrossRef]
- Huang, Y.P.; Zeng, A.P.; Gao, Z.H.; Zhang, B. Beam wander of partially coherent array beams through non-Kolmogorov turbulence. Opt. Lett. 2015, 40, 1619–1622. [Google Scholar] [CrossRef]
- Song, Z.Z.; Liu, Z.J.; Zhou, K.Y.; Sun, Q.G.; Liu, S.T. Propagation properties of Gaussian Schell-model array beams in non-Kolmogorov turbulence. J. Opt. 2016, 18, 105601. [Google Scholar] [CrossRef]
- Razzaghi, D.; Hajiesmaeilbaigi, F.; Alavinejad, M. Spectrum changes of phase-locked partially coherent flat topped laser beam array propagating in turbulent atmosphere. Opt. Int. J. Light Electron Opt. 2013, 124, 2135–2139. [Google Scholar] [CrossRef]
- Liu, H.L.; Lu, Y.F.; Xia, J.; Chen, D.; He, W.; Pu, X.Y. Radial phased-locked partially coherent flat-topped vortex beam array in non-Kolmogorov medium. Opt. Express 2016, 24, 19695–19712. [Google Scholar] [CrossRef]
- Kashani, F.D.; Yousefi, M. Analyzing the propagation behavior of coherence and polarization degrees of a phase-locked partially coherent radial flat-topped array laser beam in underwater turbulence. Appl. Opt. 2016, 55, 6311–6320. [Google Scholar] [CrossRef]
- Liu, D.; Wang, Y.; Zhong, H. Average intensity of radial phased-locked partially coherent standard Hermite-Gaussian beam in oceanic turbulence. Opt. Laser Technol. 2018, 106, 495–505. [Google Scholar] [CrossRef]
- Liu, D.; Wang, Y. Evolution properties of a radial phased-locked partially coherent Lorentz-Gauss array beam in oceanic turbulence. Opt. Laser Technol. 2018, 103, 33–41. [Google Scholar] [CrossRef]
- Liu, D.; Zhong, H.; Wang, G.; Yin, H.; Wang, Y. Propagation of a radial phase-locked partially coherent elegant Laguerre–Gaussian beam array in non-Kolmogorov medium. Appl. Phys. B 2019, 125, 52. [Google Scholar] [CrossRef]
- Huang, Y.; Yuan, Y.S.; Liu, X.L.; Zeng, J.; Wang, F.; Yu, J.Y.; Liu, L.; Cai, Y.J. Propagation of Optical Coherence Vortex Lattices in Turbulent Atmosphere. Appl. Sci. 2018, 8, 2476. [Google Scholar] [CrossRef]
- Mei, Z.R.; Korotkova, O.; Shchepakina, E. Electromagnetic multi-Gaussian Schell-model beams. J. Opt. 2013, 15, 025705. [Google Scholar] [CrossRef]
- Cang, J.; Fang, X.; Liu, X. Propagation properties of multi-Gaussian Schell-model beams through ABCD optical systems and in atmospheric turbulence. Opt. Laser Technol. 2013, 50, 65–70. [Google Scholar] [CrossRef]
- Zhang, H.; Fu, W. Polarization properties of Square Multi-Gaussian Schell-Model beam propagating through non-Kolmogorov turbulence. Opt. Int. J. Light Electron Opt. 2017, 134, 161–169. [Google Scholar] [CrossRef]
- Zhou, Y.J.; Zhao, D.M. Propagation properties of a twisted rectangular multi-Gaussian Schell-model beam in free space and oceanic turbulence. Appl. Opt. 2018, 57, 8978–8983. [Google Scholar] [CrossRef]
- Liu, D.; Wang, Y. Properties of a random electromagnetic multi-Gaussian Schell-model vortex beam in oceanic turbulence. Appl. Phys. B 2018, 124, 176. [Google Scholar] [CrossRef]
- Korotkova, O.; Shchepakina, E. Rectangular Multi-Gaussian Schell-Model beams in atmospheric turbulence. J. Opt. 2014, 16, 045704. [Google Scholar] [CrossRef]
- Zhang, Y.T.; Ding, C.L.; Pan, L.Z.; Cai, Y.J. Laser arrays of partially coherent beams with multi-Gaussian correlation function. J. Quant. Spectrosc. Radiat. Transf. 2018, 218, 1–11. [Google Scholar] [CrossRef]
- Korotkova, O.; Sahin, S.; Shchepakina, E. Multi-Gaussian Schell-model beams. J. Opt. Soc. Am. A 2012, 29, 2159–2164. [Google Scholar] [CrossRef]
- Wolf, E. Unified theory of coherence and polarization of random electromagnetic beams. Phys. Lett. A 2003, 312, 263–267. [Google Scholar] [CrossRef]
- Carter, W. Spot size and divergence for Hermite Gaussian beams of any order. Appl. Opt. 1980, 19, 1027–1029. [Google Scholar] [CrossRef]
- Eyyuboglu, H.T.; Baykal, Y.; Sermutlu, E. Convergence of general beams into Gaussian-intensity profiles after propagation in turbulent atmosphere. Opt. Commun. 2006, 265, 399–405. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, X.; Liu, D.; Wang, Y.; Yin, H.; Zhong, H.; Wang, G. Propagation of Rectangular Multi-Gaussian Schell-Model Array Beams through Free Space and Non-Kolmogorov Turbulence. Appl. Sci. 2020, 10, 450. https://doi.org/10.3390/app10020450
Ma X, Liu D, Wang Y, Yin H, Zhong H, Wang G. Propagation of Rectangular Multi-Gaussian Schell-Model Array Beams through Free Space and Non-Kolmogorov Turbulence. Applied Sciences. 2020; 10(2):450. https://doi.org/10.3390/app10020450
Chicago/Turabian StyleMa, Xiaolu, Dajun Liu, Yaochuan Wang, Hongming Yin, Haiyang Zhong, and Guiqiu Wang. 2020. "Propagation of Rectangular Multi-Gaussian Schell-Model Array Beams through Free Space and Non-Kolmogorov Turbulence" Applied Sciences 10, no. 2: 450. https://doi.org/10.3390/app10020450
APA StyleMa, X., Liu, D., Wang, Y., Yin, H., Zhong, H., & Wang, G. (2020). Propagation of Rectangular Multi-Gaussian Schell-Model Array Beams through Free Space and Non-Kolmogorov Turbulence. Applied Sciences, 10(2), 450. https://doi.org/10.3390/app10020450




