Advances in Projectile Penetration Mechanism in Soil Media
Abstract
1. Introduction
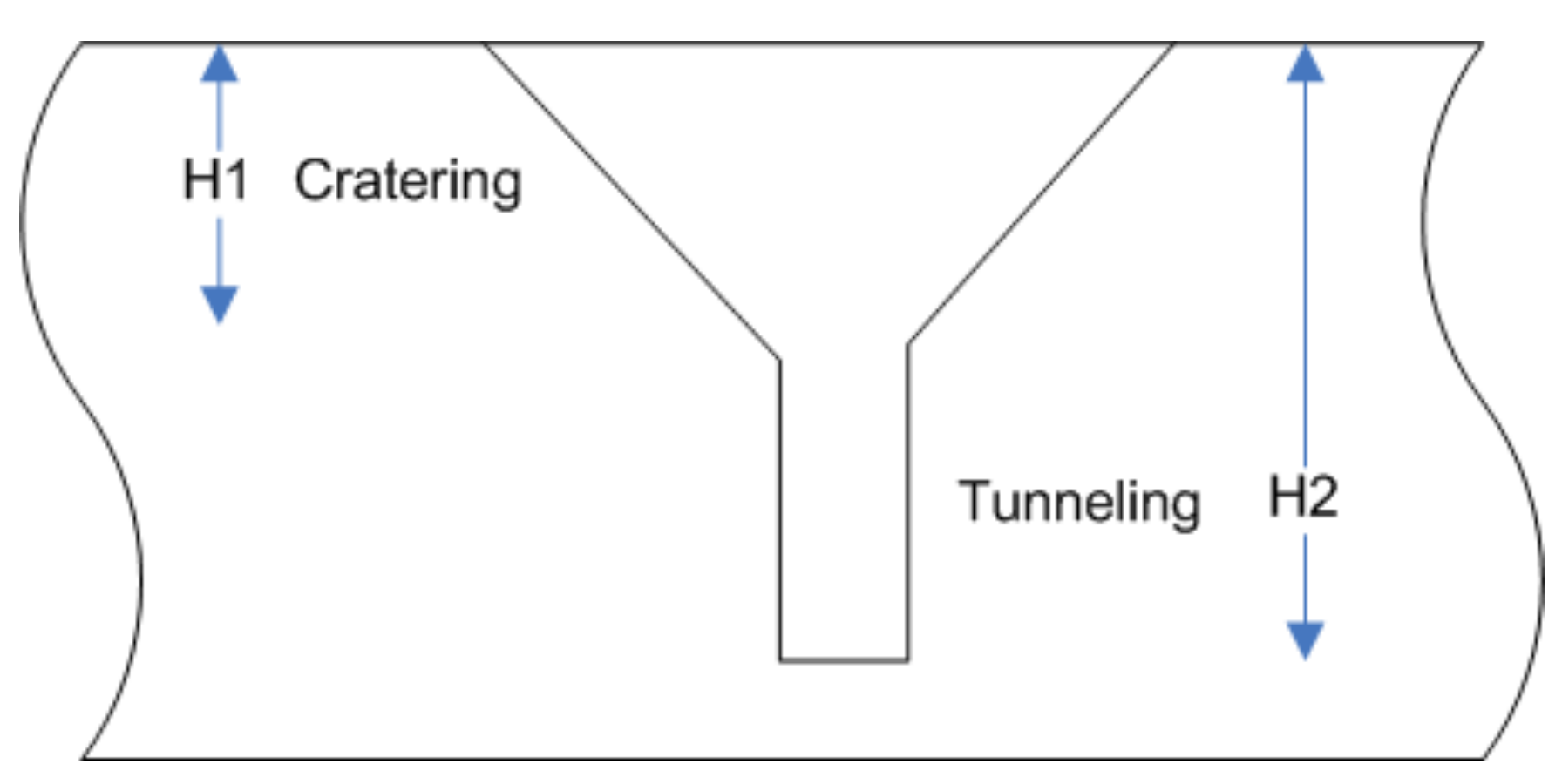
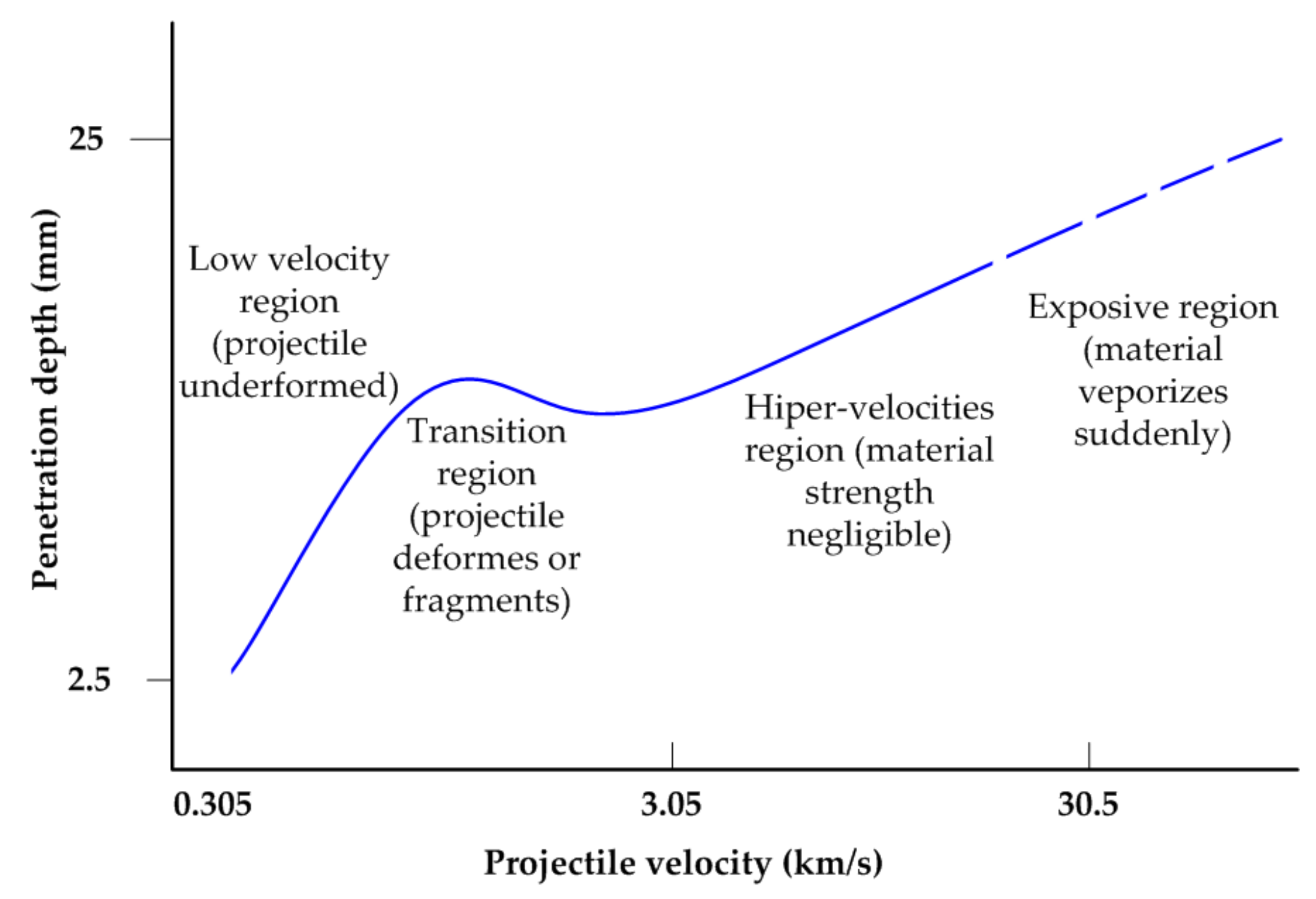
Projectile Penetration Process Parameters
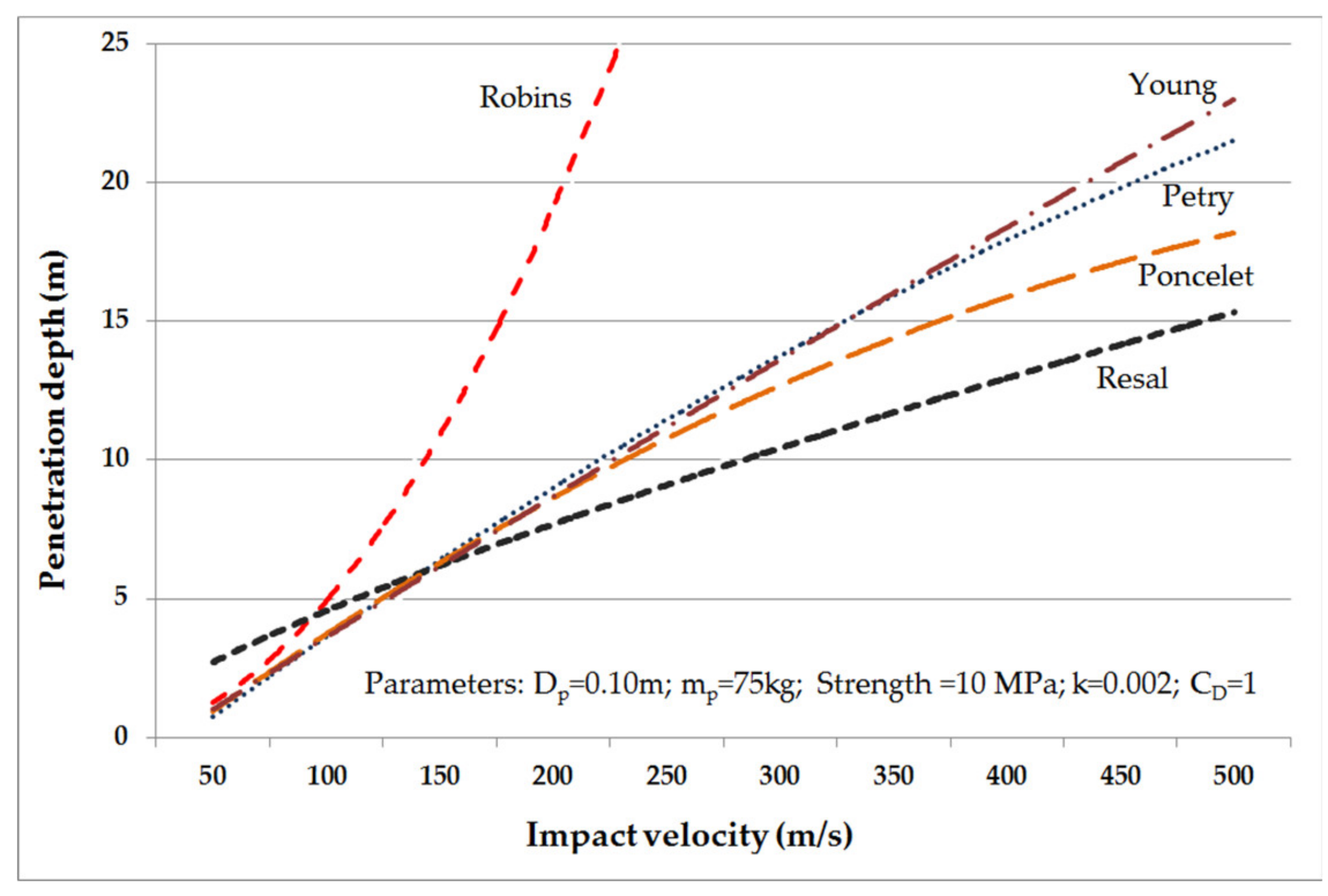
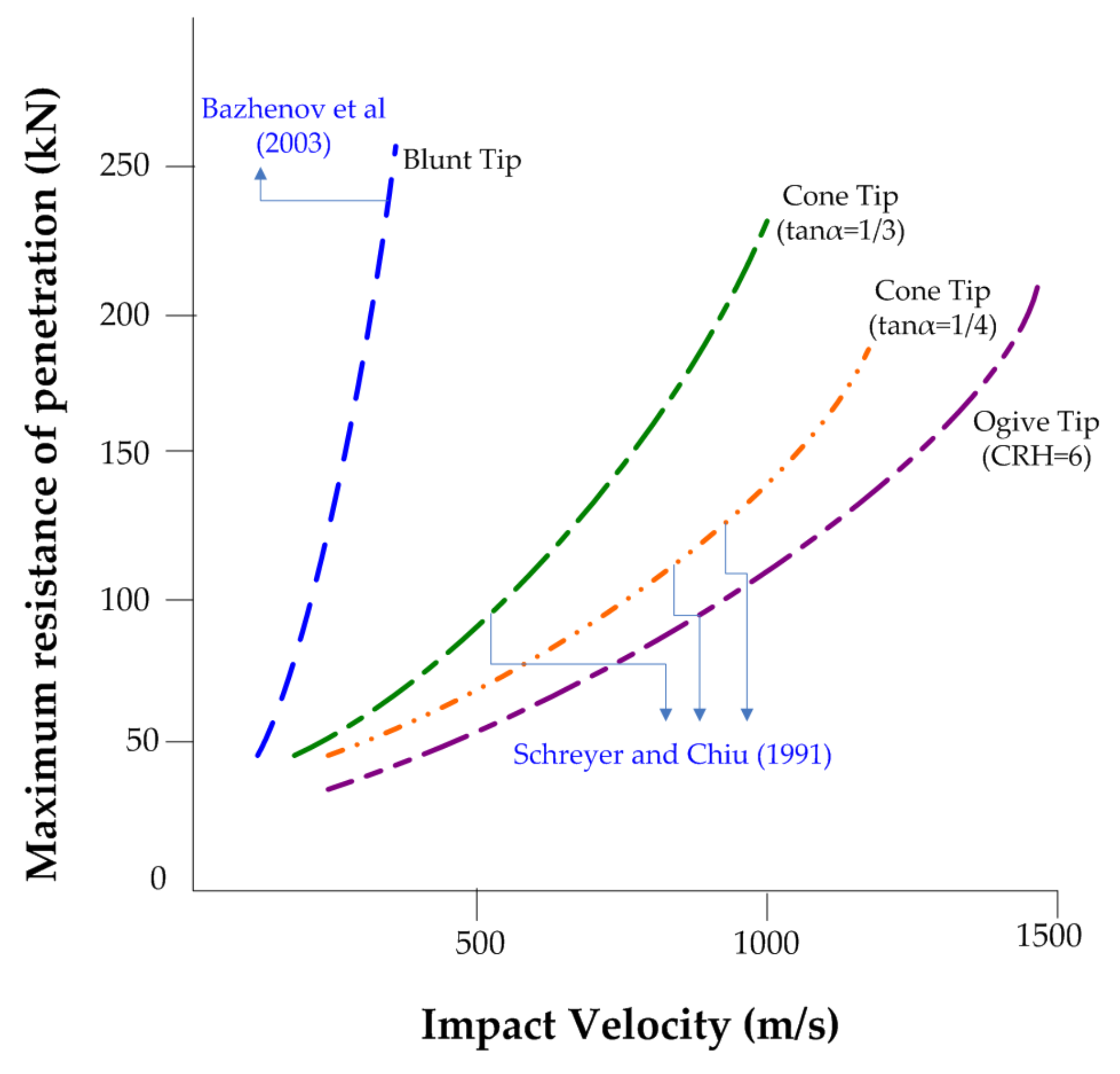
2. Analytical Methods
2.1. Classification of Methods
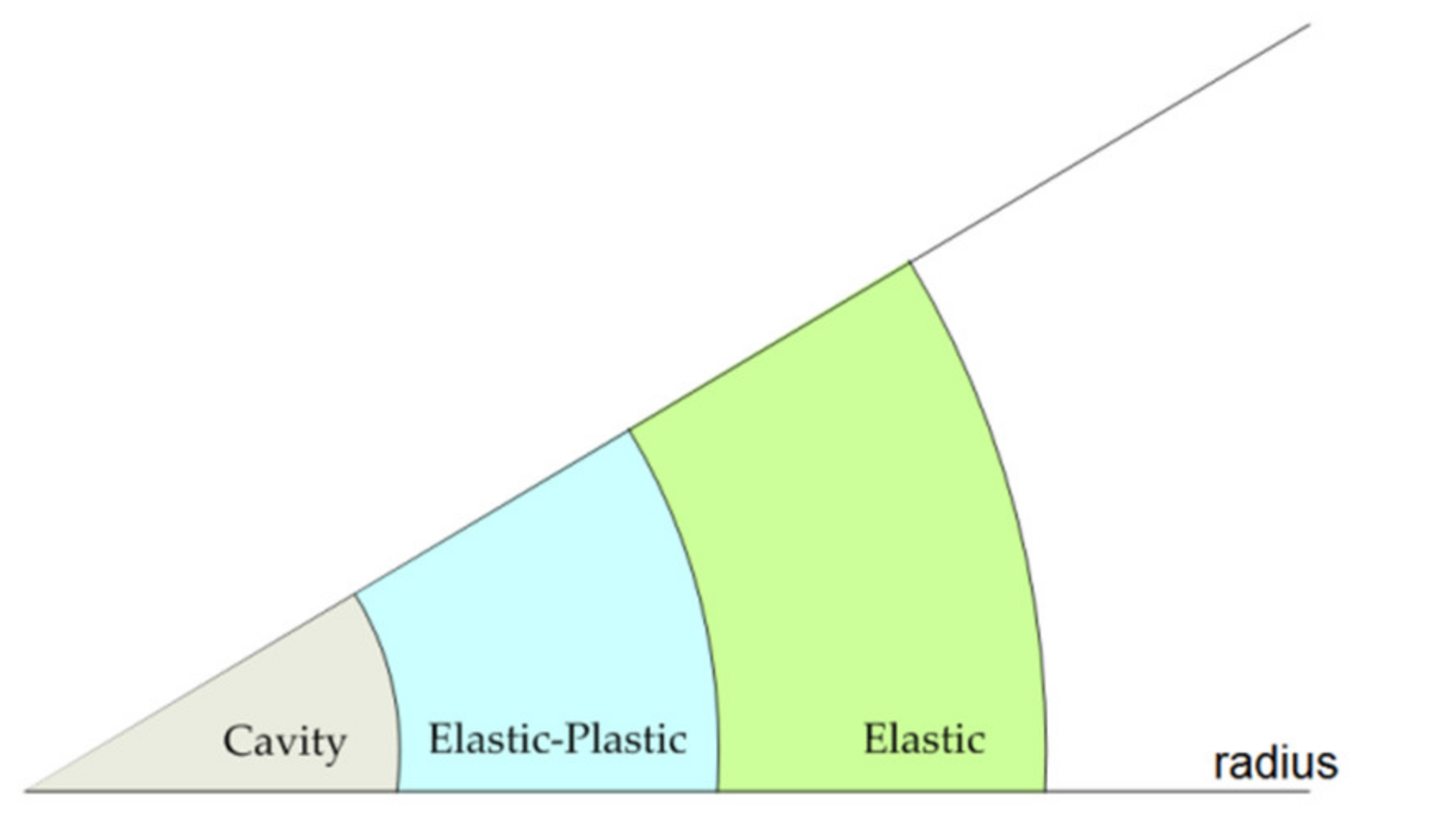
2.2. Granular Target Media
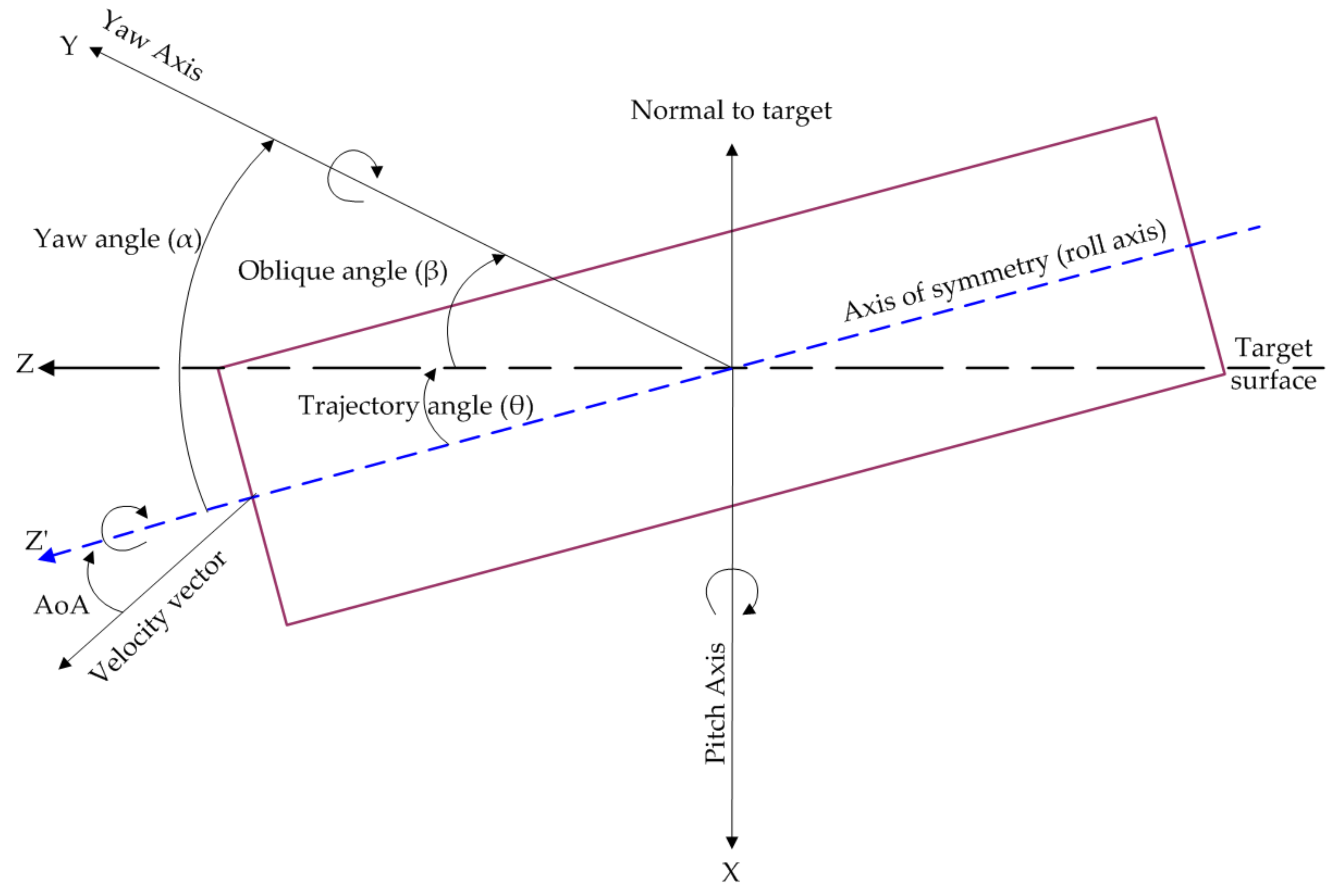
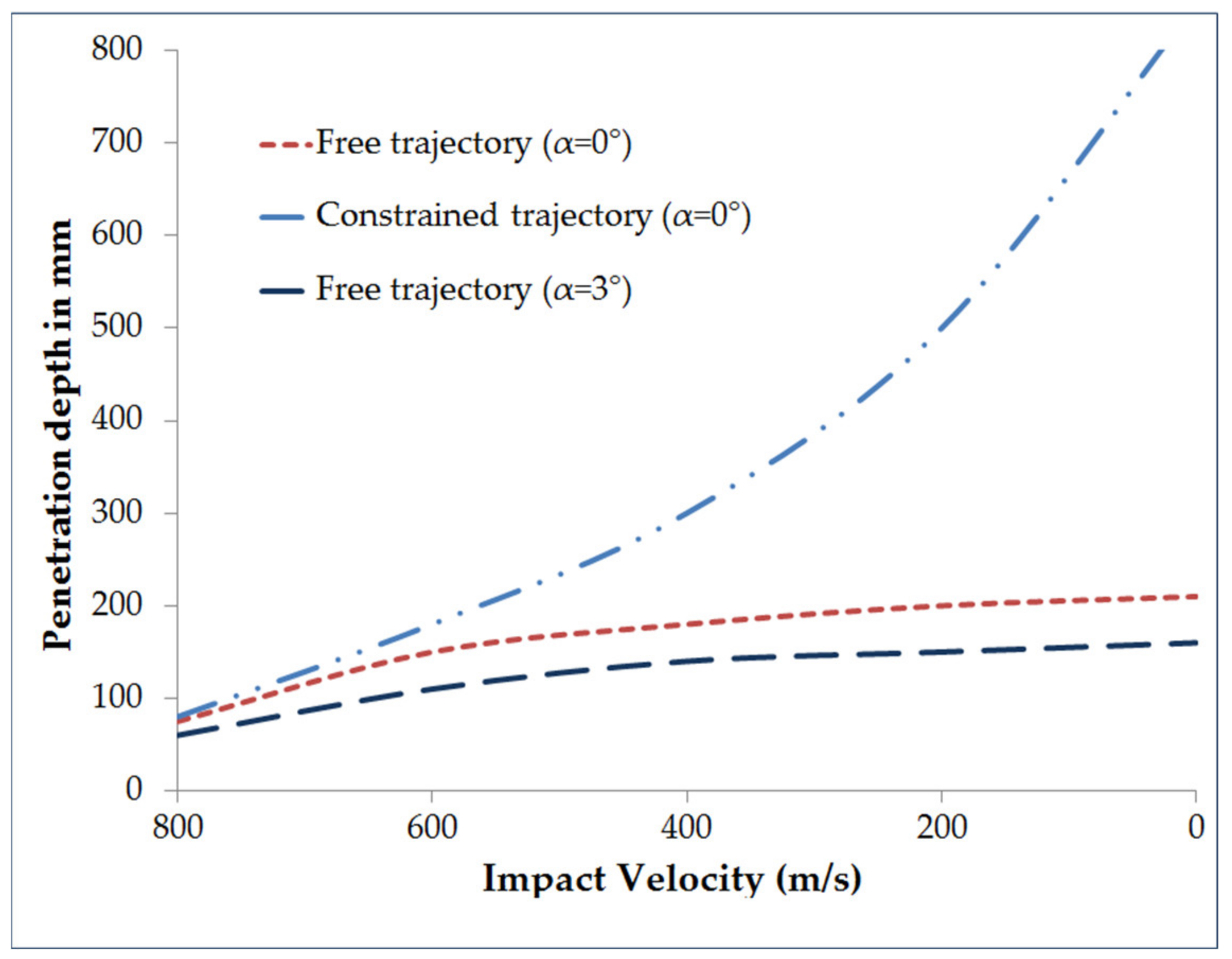
2.3. Projectile Non-Normal Impact and Stability
2.4. Multi-Stage and Multi-layers Penetration Modelling
3. Computational Methods
Hybrid and Coupled Numerical Methods
4. Experimental Methods
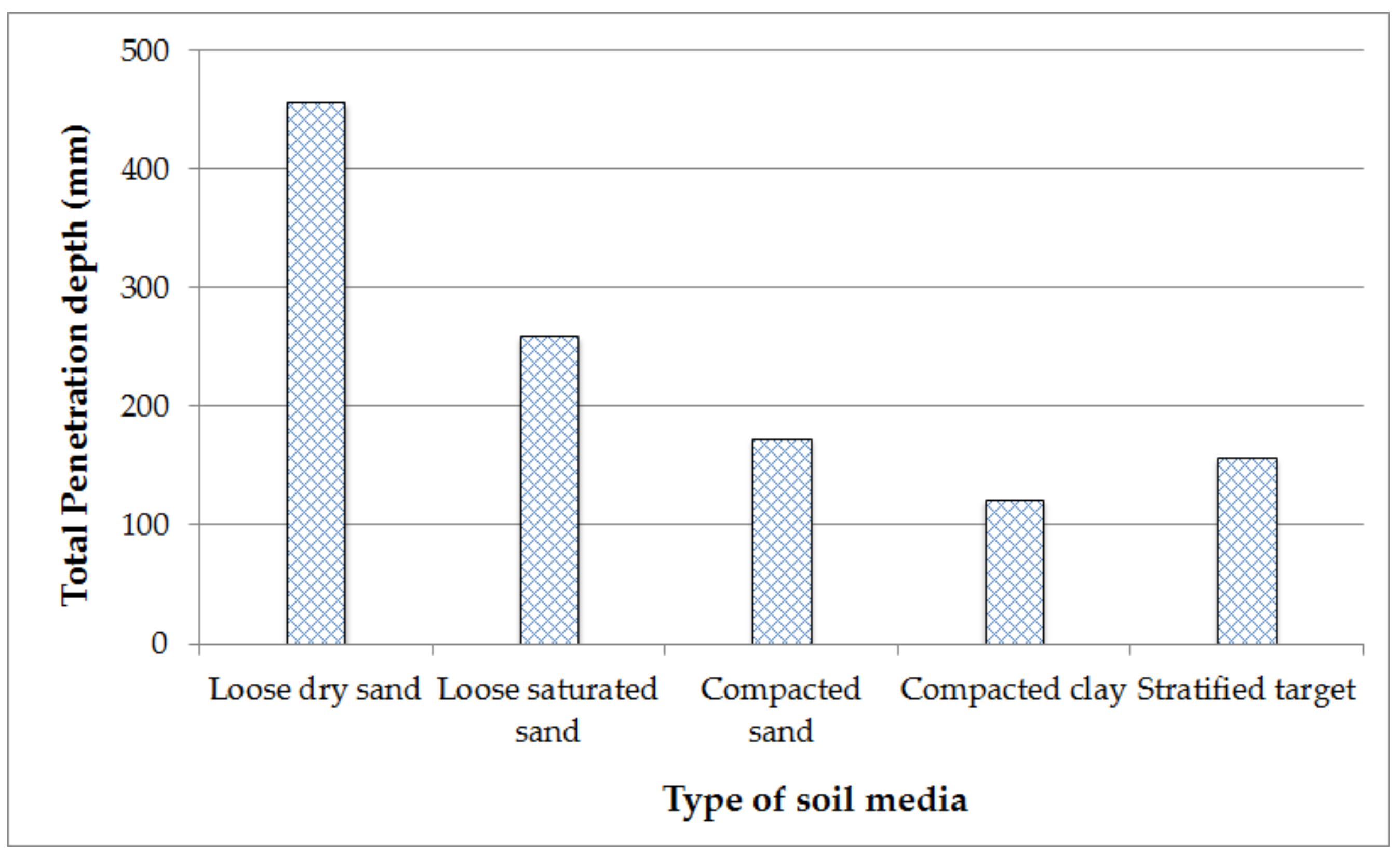
4.1. Natural Soil/Synthetic Soil Models and Prototypes
4.2. Impact Penetration Based Soil Investigation
4.3. Local and Bulk Behavior of Soil Media
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Description |
| A, B and C | Model Constant (A/Cv is static target resistance term, Bv2 is drag term), |
| A | Projectile Area |
| Bmax | Maximum hardness at crater bottom |
| D10* | effective grain size of soil, |
| E, | Material Elastic modulus, |
| F | net resistant force |
| F1, F2, F3 | respectively dissipative, dynamic, and static components of resistant force |
| & | Normal and tangential forces acting on particle i by contacting particle j, respectively; |
| and | Resultant force and moment on the projectile, |
| G | gravity acceleration |
| Ii, and ωi (ω) | Moment of inertia and angular velocity of particle i |
| Kϕ | coefficient (>1) |
| L, D | Projectile length, Diameter |
| Rolling moment; | |
| Twisting moment | |
| P (=ρs g h) | hydrostatic-like pressure |
| Pr | Pressure |
| Pst | term of static resistance; |
| Rt | Target resistance to penetration |
| R(t), (t), (t) | Disc internal boundary radial values of displacement, velocity, and acceleration |
| S | Soil penetrability number representing soil properties, |
| Yp | dynamic flow stress of projectile, |
| a | Hole radius, |
| c | cohesion of soil c, |
| d | Penetration depth of a cone, |
| dS | Infinitesimal area element pointing normal to the intruder surface, |
| h | Heaviside function, |
| l1, l2 | first and second characteristic length |
| kϕ | coefficient (>1) α to the internal friction coefficient φ, |
| m (mi) and v (vi) | mass and velocity of projectile (particle), |
| p(t) | Interaction pressure, positive in compression; |
| s | Extent of plastic zone in projectile, |
| t | Rise time of impact, |
| z | unit vector in z-direction, |
| σy | yield stress |
| α and β | nose geometry factors |
| ρs | packing density of the granular matter |
| η | a parameter dependent on grain properties; |
| ρp | Density of projectile, |
| αc | [1+ (h/Rc)] |
| τo | principal stress difference at failure; |
| λ | Projectile aspect ratio |
| εL. | volumetric locking strain; |
| ρo | initial density; |
| ρL | current locking density; |
| ρ, | Initial or current |
| ρ* | max. density reached during loading. |
| σr, σθ | radial and hoop components of the Cauchy stress |
| Angular momentum vector about the mass centre of projectile frame, | |
| Normal stress, | |
| ) | shear stress on projectile surface, |
| μ | coefficient of kinetic friction, or Factor of friction between projectile and soil, or Tangent of angle of repose of granular material, |
| vector from the center of particle i to the contact point with particle j; | |
| θ | apex angle of a wedge or a cone, |
| φ | angle of internal friction of soil, |
| ρ (ρt), s | density of soil (or target) and, degree of saturation of soil |
| θ | Semi-vertex angle of cone-nose projectile in degrees” |
| ν | Poisson’s ratio |
Appendix A
| General Formulations (Newton’s 2nd Law of motion) | |
| Forrestal and Luk [45] | ) |
| Poncelet [164] | |
| Resal [137] | |
| Local friction force model (LFFM) [38] | dFd = Kϕ P. dS = μ P dS |
| Ambroso et al. [165] | |
| DEM (Rolling spring-dashpot-slider model) [166] | |
| General Formulations (conservation of momentum) | |
| Walker-Anderson model [37] | |
| Hydro-dynamic theory steady state, incompressible model [30] |
| Modified Hydro-dynamic theory model [31] | |
| Tate-Alekseevskii model (Modified Bernoulli’s Equation) [34,35] | |
| Grigoryan [167] | |
| Spherical Cavity Expansion (SCE) [168] | |
| Cylindrical Cavity Expansion (CCE) or Disc or Orthogonal layers model [42] | , |
| Modified Archimedes’ law [46] | |
| Non-linear differential area force law (DAFL) [65] | ,
, |
| Dimensional Similitude Analysis [169] |
Appendix B
References
- Öhman, T.; Aittola, M.; Kostama, V.-P.; Raitala, J. The Preliminary Analysis of Polygonal Impact Craters within Greater Hellas Region, Mars. In Impact Tectonics; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Lobo-Guerrero, S.; Vallejo, L.E. Influence of pile shape and pile interaction on the crushable behavior of granular materials around driven piles: DEM analyses. Granul. Matter 2007, 9, 241. [Google Scholar] [CrossRef]
- Ng, K.; Ksaibati, R. Understanding the Responses of Surrounding Soils to Pile Setup from Medium-Scale Experiments and Cone Penetration Tests. Transp. Infrastruct. Geotechnol. 2018, 5, 129–145. [Google Scholar] [CrossRef]
- Maciejewski, J.; Jarzȩbowski, A.; Tra̧mpczyński, W. Study on the efficiency of the digging process using the model of excavator bucket. J. Terramechanics 2003, 40, 221–233. [Google Scholar] [CrossRef]
- Aguilar, J.; Goldman, D.I. Robophysical study of jumping dynamics on granular media. Nat. Phys. 2016, 12, 278–283. [Google Scholar] [CrossRef]
- Van der Meer, D. Impact on Granular Beds. Annu. Rev. Fluid Mech. 2017, 49, 463–484. [Google Scholar] [CrossRef]
- Hou, M.; Peng, Z.; Liu, R.; Wu, Y.; Tian, Y.; Lu, K.; Chan, C.K. Projectile impact and penetration in loose granular bed. Sci. Technol. Adv. Mater. 2005, 6, 855. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Alsaleh, M.I.; Alshibli, K.A. Evolving internal length scales in plastic strain localization for granular materials. Int. J. Plast. 2005, 21, 2000–2024. [Google Scholar] [CrossRef]
- Kadanoff, L.P. Built upon sand: Theoretical ideas inspired by granular flows. Rev. Mod. Phys. 1999, 71, 435. [Google Scholar] [CrossRef]
- Goey, C.H.; Pei, C.; Wu, C.-Y. DEM Modelling of Subsidence of a Solid Particle in Granular Media. In Discrete Element Modelling of Particulate Media; Royal Society of Chemistry: London, UK, 2012. [Google Scholar]
- Iskander, M.; Bless, S.; Omidvar, M. Rapid Penetration into Granular Media: Visualizing the Fundamental Physics of Rapid Earth Penetration; Elsevier: Amsterdam, The Netherlands, 2015; ISBN 9780128011553. [Google Scholar]
- Omidvar, M.; Iskander, M.; Bless, S. Response of granular media to rapid penetration. Int. J. Impact Eng. 2014, 66, 60–82. [Google Scholar] [CrossRef]
- Backman, M.E.; Goldsmith, W. The mechanics of penetration of projectiles into targets. Int. J. Eng. Sci. 1978, 16, 1–99. [Google Scholar] [CrossRef]
- Hopkins, H.G. Dynamic expansion of spherical cavities in metals. Prog. Solid Mech. 1960, 1, 85–164. [Google Scholar]
- O’Loughlin, C.D.; Nazem, M.; Chow, S.H.; Randolph, M.F.; Gaudin, C.; White, D.J. Dynamic embedment of projectiles in clay. Aust. Geomech. J. 2014, 49, 133–145. [Google Scholar]
- Heuzé, F.E. An overview of projectile penetration into geological materials, with emphasis on rocks. Int. J. Rock Mech. Min. Sci. 1990, 27, 1–14. [Google Scholar] [CrossRef]
- Peng, Y.; Wu, H.; Fang, Q.; Kong, X.Z. Modified spherical cavity expansion model for projectile penetration into concrete targets. Acta Mech. Sin. 2019, 35, 518–534. [Google Scholar] [CrossRef]
- Galanov, B.A.; Kartuzov, V.V.; Ivanov, S.M. A Model for Penetration Resistance in Brittle Materials Taking Into Account Strain Hardening of Solid Phase in Mescal-Zone Powder Material. Procedia Eng. 2015, 103, 135–142. [Google Scholar] [CrossRef][Green Version]
- Liu, W.H.; Hu, Z.J.; Ye, L.Y.; Liu, Y.F. The Study of Projectile Penetration into Aluminum Armor Plate. Adv. Mater. Res. 2011, 284–286, 2517–2520. [Google Scholar] [CrossRef]
- Cheon, J.; Choi, Y. Influence of Projectile Velocity on Penetration into a Steel Plate. Int. J. Precis. Eng. Manuf. 2020, 21, 137–144. [Google Scholar] [CrossRef]
- Manes, A.; Bresciani, L.M.; Giglio, M. Ballistic performance of multi-layered fabric composite plates impacted by different 7.62 mm caliber projectiles. Procedia Eng. 2014, 88, 208–215. [Google Scholar] [CrossRef]
- Chen, S. Anti-penetration simulation for HDPE honeycomb protection structure. Chem. Eng. Trans. 2017, 62, 49–54. [Google Scholar]
- Miller, J.E.; Christiansen, E.L.; Davis, B.A.; Deighton, K.D. Ballistic performance model of crater formation in monolithic, porous thermal protection systems. Procedia Eng. 2015, 103, 398–404. [Google Scholar] [CrossRef][Green Version]
- Feng, Y.; Blumenfeld, R.; Liu, C. Support of modified Archimedes’ law theory in granular media. Soft Matter 2019, 15, 3008–3017. [Google Scholar] [CrossRef] [PubMed]
- Tapia, F.; Espíndola, D.; Hamm, E.; Melo, F. Effect of packing fraction on shear band formation in a granular material forced by a penetrometer. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2013, 87, 014201. [Google Scholar] [CrossRef] [PubMed]
- Nazhat, Y.; Airey, D. The kinematics of granular soils subjected to rapid impact loading. Granul. Matter 2015, 17, 1–20. [Google Scholar] [CrossRef]
- Anderson, C.E., Jr. Analytical models for penetration mechanics: A Review. Int. J. Impact Eng. 2017, 108, 3–26. [Google Scholar] [CrossRef]
- Yu, H.S. Cavity Expansion Methods in Geomechanics; Springer Science & Busines Media: Berlin, Germany, 2013. [Google Scholar]
- Yankelevsky, D.Z. Effect of Soil Parameters on High Velocity Projectile Penetration. In Proceedings of the International Conferences on Recent Advances in Geotechnical Earthquake Engineering and Soil Dynamics. 10, St. Louis, MO, USA, 29 April 1981; Available online: http://scholarsmine.mst.edu/icrageesd/01icrageesd/session04b/10 (accessed on 21 August 2020).
- Stilp, A.J.; Hohler, V. Long rod penetration mechanics Chapter 5. In High Velocity Impact Dynamics; Zukas, J.A., Ed.; John Wiley & Sons: New York, NY, USA, 1990. [Google Scholar]
- Christman, D.R.; Gehring, J.W. Analysis of high-velocity projectile penetration mechanics. J. Appl. Phys. 1966, 37, 1579–1587. [Google Scholar] [CrossRef]
- Recht, R.F.; Ipson, T.W. Ballistic perforation dynamics. J. Appl. Mech. 1963, 30, 384–390. [Google Scholar] [CrossRef]
- Walker, J.D. Hypervelocity penetration modeling: Momentum vs. energy and energy transfer mechanisms. Int. J. Impact Eng. 2001, 26, 809–822. [Google Scholar] [CrossRef]
- Tate, A. Long rod penetration models—Part II. Extensions to the hydro-dynamic theory of penetration. Int. J. Mech. Sci. 1986, 28, 599–612. [Google Scholar] [CrossRef]
- Alekseevskii, V.P. Penetration of a Rod into a Target at High Velocity. Combust. Explos. Shock Waves 1993, 2, 63–66. [Google Scholar] [CrossRef]
- Ravid, M.; Bodner, S.R.; Holcman, I. Analysis of very high-speed impact. Int. J. Eng. Sci. 1987, 25, 473–482. [Google Scholar] [CrossRef]
- Walker, J.D.; Anderson, C.E., Jr. A time-dependent model for long-rod penetration. Int. J. Impact Eng. 1995, 16, 19–48. [Google Scholar] [CrossRef]
- Brzinski, T.; Mayor, P.; Durian, D. Depth-dependent resistance of granular media to vertical penetration. Phys. Rev. Lett. 2013, 111, 168002. [Google Scholar] [CrossRef] [PubMed]
- Jaeger, H.M.; Nagel, S.R. Physics of the granular state. Science 1992, 255, 1523–1531. [Google Scholar] [CrossRef] [PubMed]
- Sabetamal, H.; Nazem, M.; Carter, J.P.; Sloan, S.W. Large deformation dynamic analysis of saturated porous media with applications to penetration problems. Comput. Geotech. 2014, 55, 117–131. [Google Scholar] [CrossRef]
- Yankelevsky, D.Z.; Adin, M.A. A simplified analytical method for soil penetration analysis. Int. J. Numer. Anal. Methods Geomech. 1980, 4, 233–254. [Google Scholar] [CrossRef]
- Guo, X.J.; He, T.; Wen, H.M. Cylindrical cavity expansion penetration model for concrete targets with shear dilatancy. J. Eng. Mech. 2013, 139, 1260–1267. [Google Scholar] [CrossRef]
- Forrestal, M.J. Penetration into dry porous rock. Int. J. Solids Struct. 1986, 22, 1485–1500. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Tzou, D.Y. Spherical cavity-expansion penetration model for concrete targets. Int. J. Solids Struct. 1997, 34, 4127–4146. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Luk, V.K. Penetration into Soil Targets. Int. J. Impact Eng. 1992, 12, 427–444. [Google Scholar] [CrossRef]
- Bless, S.; Omidvar, M.; Iskander, M. High-Speed Penetration of Granular Geomaterials; Elsevier: Amsterdam, The Netherlands, 2015; p. 93. [Google Scholar]
- Kang, W.; Feng, Y.; Liu, C.; Blumenfeld, R. Archimedes’ law explains penetration of solids into granular media. Nat. Commun. 2018, 9, 1101. [Google Scholar] [CrossRef]
- Seguin, A.; Gondret, P. Drag force in a cold or hot granular medium. Phys. Rev. E 2017, 96, 032905. [Google Scholar] [CrossRef]
- Khan, M.A. Mechanics of projectile penetration into non-cohesive soil targets. Int. J. Civ. Eng. 2015, 13, 28–39. [Google Scholar]
- Huang, K.; Delfin, D.H.; Rech, F.; Dichtl, V.; Hidalgo, R.C. The role of initial speed in projectile impacts into light granular media. Sci. Rep. 2020, 10, 3207. [Google Scholar] [CrossRef] [PubMed]
- Singh, A.; Magnanimo, V.; Saitoh, K.; Luding, S. Effect of cohesion on shear banding in quasi-static granular materials. Phys. Rev. E 2014, 90, 022202. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Ran, X.; Blumenfeld, R. Vertical dynamics of a horizontally oscillating active object in a two-dimensional granular medium. Phys. Rev. E 2016, 94, 062906. [Google Scholar] [CrossRef]
- Vinutha, H.; Sastry, S. Disentangling the role of structure and friction in shear jamming. Nat. Phys. 2016, 12, 578–583. [Google Scholar] [CrossRef]
- Voorena, V.; Borga, J.; Sandusky, H.; Felts, J. Sand Penetration: A Near Nose Investigation of a Sand Penetration Event Andrew. Procedia Eng. 2013, 58, 601–607. [Google Scholar] [CrossRef]
- Macek, R.W.; Duffey, T.A. Finite cavity expansion method for nearsurface effects and layering during earth penetration. Int. J. Impact Eng. 2000, 24, 239–258. [Google Scholar] [CrossRef]
- Jiang, M.J.; Sun, Y.G. Cavity expansion analyses of crushable granular materials with state-dependent dilatancy. Int. J. Numer. Anal. Methods Geomech. 2012, 36, 723–742. [Google Scholar] [CrossRef]
- Kotov, V.L.; Balandin, V.V.; Bragov, A.M.; Linnik, E.Y.; Balandin, V.V. Using a local interaction model to determine the resistance to penetration of projectiles into sandy soil. J. Appl. Mech. Tech. Phys. 2013, 54, 612–621. [Google Scholar] [CrossRef]
- Bernard, R.S.; Creighton, D. Projectile Penetration in Soil and rock: Analysis for Non-Normal Impact, Technical Report; SL-79-15AD-A081044; US Army Waterways Experiment Station: Vieksburg, MS, USA, 1979. [Google Scholar]
- Young, C.W. Simplified Analytical Model of Penetration with Lateral Loading e User’s Guide; Report No. SAND98-0978; Sandia National Laboratories: Albuquerque, NM, USA, 1998; p. 61. [Google Scholar]
- Rosenberg, Z.; Dekel, E. Terminal Ballistics; Springer: Berlin/Heidelberg, Germany, 2012; p. 395. [Google Scholar]
- Wen, H.M. Predicting the Penetration and Perforation of Targets Struck by Projectiles at Normal Incidence. Mech. Struct. Mach. 2002, 30, 543–577. [Google Scholar] [CrossRef]
- Goldsmith, W. Non-ideal projectile impact on targets. Int. J. Impact Eng. 1999, 22, 95–395. [Google Scholar] [CrossRef]
- Collins, A.L.; Addiss, J.W.; Walley, S.M.; Promratana, K.; Bobaru, F.; Proud, W.G.; Williamson, D.M. The effect of nose shape on the internal flow fields during ballistic penetration of sand. Int. J. Impact Eng. 2011, 38, 951–963. [Google Scholar] [CrossRef]
- Ben-Dor, G.; Dubinsky, A.; Elperin, T. Some inverse problems in penetration mechanics. Mech. Based Des. Struct. Mach. 2010, 38, 468–480. [Google Scholar] [CrossRef]
- Gao, X.; Li, Q.M. Trajectory instability and convergence of the curvilinear motion of a hard projectile in deep penetration. Int. J. Mech. Sci. 2017, 121, 123–142. [Google Scholar] [CrossRef]
- Dwivedi, S.K.; Teeter, R.D.; Felice, C.W.; Gupta, Y.M. Two dimensional mesoscale simulations of projectile instability during penetration in dry sand. J. Appl. Phys. 2008, 104, 083502. [Google Scholar] [CrossRef]
- Simonov, I.V.; Osipenko, K.Y. Stability, paths, and dynamic bending of a blunt body of revolution penetrating an elastoplastic medium. J. Appl. Mech. Tech. Phys. 2004, 45, 428–439. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Luk, V.K. Dynamic spherical cavity-expansion in a compressible elastic-plastic solid. J. Appl. Mech. 1988, 55, 275–279. [Google Scholar] [CrossRef]
- Masri, R.; Durban, D. Dynamic spherical cavity expansion in an elasloplastic compressible mises solid. J. Appl. Mech. Trans. ASME 2005, 72, 887–898. [Google Scholar] [CrossRef]
- Ravid, M.; Bodner, S.R. Dynamic perforation of viscoplastic plates by rigid projectiles. Int. J. Eng. Sci. 1983, 21, 577–591. [Google Scholar] [CrossRef]
- Frew, D.J.; Forrestal, M.J.; Hanchak, S.J. Penetration Experiments with Limestone Targets and Ogive-Nose Steel Projectiles. J. Appl. Mech. 2000, 67, 841–845. [Google Scholar] [CrossRef]
- Orphal, D.L. Phase three penetration. Int. J. Impact Eng. 1997, 20, 601–616. [Google Scholar] [CrossRef]
- Goldman, D.I.; Umbanhowar, P. Scaling and dynamics of sphere and disk impact into granular media. Phys. Rev. E 2008, 77, 021308-1–021308-14. [Google Scholar] [CrossRef] [PubMed]
- Siddiqui, N.A.; Abbas, H. Mechanics of missile penetration into geo-materials. Struct. Eng. Mech. 2002, 13, 639–652. [Google Scholar] [CrossRef]
- Kondic, L.; Fang, X.; Losert, W.; O’Hern, C.S.; Behringer, R.P. Microstructure evolution during impact on granular matter. Phys. Rev. E 2012, 85, 011305–1–011305-17. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Shi, C.; Liu, Z.; Wen, D. Theoretical Research Progress in High-Velocity/Hypervelocity Impact on Semi-Infinite Targets. Shock Vib. 2015, 2015, 1–15. [Google Scholar] [CrossRef]
- Silling, S.A.; Epton, M.; Weckner, O.; Xu, J.; Askari, E. Peridynamic states and constitutive modelling. J. Elast. 2007, 88, 151–184. [Google Scholar] [CrossRef]
- Borg, J.P.; Vogler, T.J. Mesoscale simulations of a dart penetrating sand. Int. J. Impact Eng. 2008, 35, 1435–1440. [Google Scholar] [CrossRef]
- Herrmann, W. Constitutive equation for the dynamic compaction of ductile porous materials. J. Appl. Phys. 1969, 40, 2490–2499. [Google Scholar] [CrossRef]
- Grady, D.E.; Winfree, N.A.; Kerley, G.I.; Wilson, L.T.; Kuhns, L.D. Computational modeling and wave propagation in media with inelastic deforming microstructure. J. Phys. IV Fr. 2000, 10, 15–20. [Google Scholar] [CrossRef]
- Wunnemann, K.; Collins, G.S.; Melosh, H.J. A strain-based porosity model for use in hydrocode simulations of impacts and implications for transient crater growth in porous targets. Icarus 2006, 180, 514–527. [Google Scholar] [CrossRef]
- Borg, J.P.; Morrissey, M.P.; Perich, C.A.; Vogler, T.J.; Chhabildas, L.C. In situ velocity and stress characterization of a projectile penetrating a sand target: Experimental measurements and continuum simulations. Int. J. Impact Eng. 2013, 51, 23–35. [Google Scholar] [CrossRef]
- Bazhenov, V.G.; Bragov, A.M.; Konstantinov, A.Y.; Kotov, V.L. Comparative analysis of methods for modeling the penetration and plane-parallel motion of conical projectiles in soil. J. Appl. Mech. Tech. Phys. 2015, 56, 381–390. [Google Scholar] [CrossRef]
- Paik, K.; Salgado, R.; Lee, J.; Kim, B. Behaviorofopen-and closed-ended piles driven into sands. J. Geotech. Geoenviron. Eng. 2003, 129, 296–306. [Google Scholar] [CrossRef]
- Ko, J.; Jeong, S.; Lee, J.K. Large deformation FE analysis of driven steel pipe piles with soil plugging. Comput. Geotech. 2016, 71, 82–97. [Google Scholar] [CrossRef]
- Rahbek, D.B. Finite Element Simulations of Drop Indentations into Oily Clay; FFI-rapport 2014/02221; Norwegian Defense Research Establishment (FFI): Kjeller, Norway, 2015; Available online: http://hdl.handle.net/20.500.12242/1118 (accessed on 26 August 2020).
- Carter, J.P.; Mazem, M. Analysis of dynamic penetration of soils. In Proceedings of the From Materials to Structures: Advancement through Innovation-Proceedings of the 22nd Australasian Conference on the Mechanics of Structures and Materials, ACMSM, Sydney, Australia, 11–14 December 2012. [Google Scholar]
- Danielson, K.T.; Adley, M.D. A meshless treatment of three-dimensional penetrator targets for parallel computation. Comput. Mech. 2000, 25, 267–273. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Seguin, A.; Bertho, Y.; Gondret, P.; Crassous, J. Sphere penetration by impact in a granular medium: A collisional process. Europhys. Lett. 2009, 88, 44002–1–44002-6. [Google Scholar] [CrossRef]
- Tran, Q.A.; Chevalier, B.; Breul, P. Discrete modeling of penetration tests in constant velocity and impact conditions. Comput. Geotech. 2016, 71, 12–18. [Google Scholar] [CrossRef]
- Holmen, J.K.; Olovsson, L.; Børvik, T. Discrete modelling of low-velocity penetration in sand. Comput. Geotech. 2017, 86, 21–32. [Google Scholar] [CrossRef]
- IMPETUS Afea, A.S. IMPETUS Afea Solver. 2016. Available online: http://www.impetusafea.com (accessed on 21 August 2020).
- Børvik, T.; Dey, S.; Olovsson, L. Penetration of granular materials by small-arms bullets. Int. J. Impact Eng. 2015, 75, 123–139. [Google Scholar] [CrossRef]
- Takita, H.; Sumita, I. Low-velocity impact cratering experiments in a wet sand target. Phys. Rev. E 2013, 88, 022203. [Google Scholar] [CrossRef] [PubMed]
- Yang, A.; Li, J.; Qu, H.; Pan, Y.; Kang, Y.; Zhang, Y. Numerical Simulation of Hypervelocity Impact FEM-SPH Algorithm Based on Large Deformation of Material. Preprints 2016. [Google Scholar] [CrossRef][Green Version]
- Li, Q.M.; Flores-Johnson, E.A. Hard projectile penetration and trajectory stability. Int. J. Impact Eng. 2011, 38, 815–823. [Google Scholar] [CrossRef][Green Version]
- Fang, Q.; Kong, X.; Wu, H.; Yan, H. Predictions of projectile penetration and perforation by DAFL with the free surface effect. Struct. Shock Impact XII 2012, 126, 249. [Google Scholar] [CrossRef]
- Abelev, A.; Simeonov, J.; Valent, P. Numerical investigation of dynamic free-fall penetrometers in soft cohesive marine sediments using a finite element approach. In Proceedings of the OCEANS 2009, MTS/IEEE Biloxi—Marine Technology for Our Future: Global and Local Challenges, Biloxi, MS, USA, 26–29 October 2009; pp. 2626–2633. [Google Scholar]
- Abelev, A.; Tubbs, K.; Valent, P. Numerical investigation of dynamic free-fall penetrometers in soft cohesive marine sediments using a finite difference approach. In Proceedings of the OCEANS 2009, MTS/IEEE Biloxi—Marine Technology for Our Future: Global and Local Challenges, Biloxi, MS, USA, 26–29 October 2009; pp. 2616–2625. [Google Scholar]
- Yongxiang, D.; Shunshan, F. Numerical simulation of flat-nose projectile penetrating concrete and soil target. In Proceedings of the 3rd WSEAS International Conference on Applied and Theoretical Mechanics, Tenerife, Canary Islands, Spain, 14–16 December 2007. [Google Scholar]
- Ogawa, K.; Takeda, S.; Kobayashi, H.; Tanigaki, K. Simulation of dynamic response of projectile and granular target. In EPJ Web of Conferences; EDP Sciences: Les Ulis, France, 2015; Volume 94, p. 0404. [Google Scholar]
- Flores-Johnson, E.A.; Wang, S.; Maggi, F.; El Zein, A.; Gan, Y.; Nguyen, G.D.; Shen, L. Discrete element simulation of dynamic behaviour of partially saturated sand. Int. J. Mech. Mater. Des. 2016, 12, 495–507. [Google Scholar] [CrossRef]
- Rabinovich, Y.I.; Esayanur, M.S.; Moudgil, B.M. Capillary forces between two spheres with a fixed volume liquid bridge: Theory and experiment. Langmuir 2005, 21, 10992–10997. [Google Scholar] [CrossRef]
- Yankelevsky, D.Z. Projectile penetration through a narrow drill in soil. Int. J. Impact Eng. 1983, 1, 377–391. [Google Scholar] [CrossRef]
- Yu, H.S.; Mictchell, J.K. Analysis of cone resistance: Review of methods. J. Geotech. Geoenviron. Eng. ASCE 1998, 124, 140–149. [Google Scholar] [CrossRef]
- Thigpen, L. Projectile penetration of elastic-plastic earth media. J. Geotech. Eng. ASCE 1974, 100, 279–294. [Google Scholar] [CrossRef]
- True, D.G. Penetration of Projectiles into Seafloor Soils; Final Report No. CEL-TR-822; Naval. Civil Engineering Lab.: Port Hueneme, CA, USA, 1975. [Google Scholar]
- Teland, J.A.; Moxnes, J.F. Analytical Cavity Expansion Penetration Models Compared with Numerical Simulations; Technical Report; Norwegian Defence Research Establishment: Kjeller, Norway, 2003. [Google Scholar]
- Thompson, J.B. Low-Velocity Impact Penetration of Low-Cohesion Soil Deposits. Ph.D. Thesis, University of California, Berkeley, CA, USA, 1975. [Google Scholar]
- Liu, J.; Huang, F.; Xu, K.; Liu, L.; Zuo, T.; Pi, A. Influence of Mass Ratio on Forward and Reverse Ballistic Impact Equivalence: Experiments, Simulations, and Mechanism Analysis. Exp. Mech. 2017, 57, 387–404. [Google Scholar] [CrossRef]
- Uehara, J.S.; Ambroso, M.A.; Ojha, R.P.; Durian, D.J. Low-speed impact craters in loose granular media. Phys. Rev. Lett. 2003, 90, 194301. [Google Scholar] [CrossRef] [PubMed]
- Clark, A.H.; Kondic, L.; Behringer, R.P. Particle scale dynamics in granular impact. Phys. Rev. Lett. 2012, 109, 238302–1–238302–5. [Google Scholar] [CrossRef] [PubMed]
- Nordstrom, K.N.; Lim, E.; Harrington, M.; Losert, W. Granular dynamics during impact. Phys. Rev. Lett. 2014, 112, 228002–1–228002–5. [Google Scholar] [CrossRef]
- Zacny, K.; Bualat, M.; Lee, P.; Alvarez, L.; Fong, T.; Deans, M.; VanGundy, L.; Lees, D. Using percussive, dynamic, and static soil penetrometers to assess geotechnical properties and the depth to ground ice of the mars and lunar analog terrains on the Devon Island, Canadian Arctic. In Earth and Space 2012: Engineering, Science, Construction, and Operations in Challenging Environments; Zacny, K., Mall, R.B., Binienda, W., Eds.; ASCE: Pasadena, CA, USA, 2012; pp. 284–294. [Google Scholar]
- Melzer, K.-J. Measuring Soil Properties in Vehicle Mobility Research: Cone Penetration Resistance and Relative Density; Technical Report; U.S. Army Engineer Waterways Experiment Station, CE: Vicksburg, MS, USA, 1970. [Google Scholar]
- Braslau, D. Partitioning of energy in hypervelocity impact against loose sand target. J. Geophys. Res. 1970, 75, 3987–3999. [Google Scholar] [CrossRef]
- Pournaghiazar, M.; Russell, A.; Khalili, N. Linking cone penetration resistances measured in calibration chambers and the field. Géotech. Lett. 2012, 2, 29–35. [Google Scholar] [CrossRef]
- Bivin, Y.K.; Simonov, I.V. Mechanics of dynamic penetration into soil medium. Mech. Solids 2010, 45, 892–920. [Google Scholar] [CrossRef]
- Bless, S.; Peden, B.; Guzman, I.; Omidvar, M.; Iskander, M. Poncelet coefficients of granular media. In Dynamic Behavior of Materials; Song, B., Casem, D., Kimberley, J., Eds.; Proceedings of the Society for Experimental Mechanics Series, 1; Springer: Lombard, IL, USA, 2014; pp. 373–380. [Google Scholar]
- Bazhenov, V.G.; Bragov, A.M.; Kotov, V.L. Experimental-theoretical study of the penetration of rigid projectiles and identification of soil properties. J. App. Mech. Tech. Phys. 2009, 50, 1011. [Google Scholar] [CrossRef]
- Bragova, А.М.; Balandina, V.V.; Igumnova, L.А.; Кotova, V.L.; Kruszkab, L.; Lomunova, А.K. Impact and penetration of cylindrical bodies into dry and water-saturated sand. Int. J. Impact Eng. 2018, 122, 197–208. [Google Scholar] [CrossRef]
- Savvateev, A.F.; Budin, A.V.; Kolikov, V.A.; Rutberg, P.G. High-Seed Penetration into Sand. Int. J. Impact Eng. 2001, 26, 675–681. [Google Scholar] [CrossRef]
- Miyai, S.; Kobayakawa, M.; Tsuji, T.; Tanaka, T. Influence of particle size on vertical plate penetration into dense cohesion-less granular materials (large-scale DEM simulation using real particle size). Granul. Matter 2019, 21, 105. [Google Scholar] [CrossRef]
- O’Loughlin, C.D.; Nazem, S.H.; Chow, M.F.; Randolph, C.; Gaudin, D.J.W. Dynamic Embedment of Projectiles in Clay. Aust. Geomech. 2009, 49, 133–145. [Google Scholar]
- Richardson, M.D.; O’Loughlin, C.D.; Randolph, M.F.; Gaudin, C. Setup following installation of dynamic anchors in normally consolidated clay. J. Geotech. Geoenviron. Eng. 2009, 135, 487–496. [Google Scholar] [CrossRef]
- Christensen, B.K. Twenty Five Years of Penetration Records at Sandia National Laboratories-PENTDB: A Relational Database; Report SAND 88-1402; Sandia National Laboratories: Albuquerque, NM, USA, 1989; p. 53. [Google Scholar]
- Cooper, W.L. Communication of Stresses by Chains of Grains in High-Speed Particulate Media Impacts; Proulx, T., Ed.; Dynamic Behavior of Materials, Conference Proceedings of the Society for Experimental Mechanics Series; Springer: New York, NY, USA, 2011; Volume 1. [Google Scholar] [CrossRef]
- Baker, W.E.; Westine, P.S.; Dodge, F.T. Similarity Methods in Engineering Dynamics: Theory and Practice of Scale Modeling; Spartan Books: Rochell Park, NJ, USA, 1973. [Google Scholar]
- Awoshika, K.; Cox, W.R. An Application of Similitude to Model Design of a Soil-Projectile System; NASA Contractor Report CR-1210; Department of Civil Engineering, The University of Texas at Austin, to National Aeronautics and Space Administration, Langley Research Centre: Hampton, VA, USA, November 1968. [Google Scholar]
- Anandarajah, A.; Kim, Y.S.; Thompson, P.Y.; Rosengren, P. Progress in Geotechnical Dynamic Centrifuge Modeling; Final Report No. ESL-TR-8462; Air Force Engrg. Services Ctr.: Tyndall Air Force Base, FL, USA, 1985. [Google Scholar]
- Taylor, T.; Fragaszy, R.J.; Ho, C.L. Projectile Penetration in Granular Soils. J. Geotech. Eng. 1991, 117, 658–672. [Google Scholar] [CrossRef]
- True, D.G. Undrained Vertical Penetration into Ocean Bottom Soils. Ph.D. Thesis, University of California, Berkeley, CA, USA, 1976. Available online: http://search.proquest.com/docview/302795036 (accessed on 26 May 2020).
- Freeman, T.J.; Burdett, J.R.F. Deep Ocean Model Penetrator Experiments. Report CD-NA-10-502, 1986-EN-C; Directorate-General for Science, Research and Development: Brussels, Belgium. Available online: http://bookshop.europa.eu/en/deep-ocean-model-penetrator-experiments.-report-pbCDNA10502/ (accessed on 26 May 2020).
- Hossain, M.S.; O’Loughlin, C.D.; Kim, Y. Dynamic installation and monotonic pullout of a torpedo anchor in calcareous silt. Géotechnique 2015, 65, 77–90. [Google Scholar] [CrossRef]
- Robins, B. New Principles of Gunnery (Originially published in 1742). In Mathematical Tracts of the Late Benjamin Ribins; James, W., Ed.; Wright Printer, St. John’s Square: London, UK, 1761; Volume 1, p. 341. [Google Scholar]
- Resal, H. Sur la penetration d’un projectile dans les semi-fluides et les solides. Compte Rendus 1895, 120, 397–401. [Google Scholar]
- Faulstich, A.J.; Herring, H.J. Two Aspects of Earth Penetration: Measurement of Resistance to Burial and Theoretical Prediction of Penetration in Stratiform Soil; Report No. NOLTR 69-116; United States Naval Ordnance Laboratory: White Oak, MD, USA, 1969; p. 48. [Google Scholar]
- Ezzein, F.M.; Bathurst, R.J. A transparent sand for geotechnical laboratory modeling. Geotech. Test. J. 2011, 34, 590–601. [Google Scholar] [CrossRef]
- Ads, A.; Iskander, M.; Bless, S. Soil–projectile interaction during penetration of a transparent clay simulant. Acta Geotech. 2020, 15, 815–826. [Google Scholar] [CrossRef]
- Omidvar, M.; Iskander, M.; Bless, S. Soil–projectile interactions during low velocity penetration. Int. J. Impact Eng. 2016, 93, 211–221. [Google Scholar] [CrossRef]
- Brown, E.; Zhang, H.; Forman, N.A.; Maynor, B.W.; Betts, D.E.; DeSimone, J.M.; Jaeger, H.M. Shear thickening and jamming in densely packed suspensions of different particle shapes. Phys. Rev. E 2011, 84, 031408. [Google Scholar] [CrossRef]
- Bi, D.; Zhang, J.; Chakraborty, B.; Behringer, R.P. Jamming by shear. Nature 2011, 480, 355–358. [Google Scholar] [CrossRef] [PubMed]
- Albert, I.; Sample, J.G.; Morss, A.J.; Rajagopalan, S.; Barabási, A.L.; Schiffer, P. Granular drag on a discrete object: Shape effects on jamming. Phys. Rev. E 2001, 64, 061303. [Google Scholar] [CrossRef]
- Caudle, W.N.; Pope, A.Y.; McNeill, R.L.; Margason, B.E. The Feasibility of Rapid Soil Investigations using High-Speed, Earth-Penetrating Projectiles. In Proceedings of the International Symposium on Wave Propagation and Dynamic Properties of Earth Materials, Albuquerque, NM, USA, 23–25 August 1967. [Google Scholar]
- Lunne, T.; Robertson, P.K.; Powell, J.J.M. Cone Penetration Testing in Geotechnical Practice; Taylor & Francis: London, UK, 1997. [Google Scholar]
- Stark, N.; Hanff, H.; Kopf, A. Nimrod: A tool for rapid geotechnical characterization of surface sediments. Sea Technol. 2009, 50, 10–14. [Google Scholar]
- Lieng, J.T.; Tjelta, T.I.; Skaugset, K. Installation of two prototype deep penetrating anchors at the Gjøa Field in the North Sea. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 2010; pp. 1999–2007. [Google Scholar] [CrossRef]
- Stephan, S.; Kaul, N.; Villinger, H. Validation of impact penetrometer data by cone penetration testing and shallow seismic data within the regional geology of the Southern North Sea. Geo-Mar. Lett. 2015, 35, 203–219. [Google Scholar] [CrossRef]
- Chow, S.H.; Airey, D.W. Free-falling penetrometers: A laboratory investigation in clay. J. Geotech. Geo-Environ. Eng. 2014, 140, 201–214. [Google Scholar] [CrossRef]
- Chian, S.C.; Tan, B.C.V.; Sarma, A. Projectile penetration into sand: Relative density of sand and projectile nose shape and mass. Int. J. Impact Eng. 2017, 103, 29–37. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Lee, L.M.; Jenrette, L.M. Laboratory-scale penetration experiments into geological targets to impact velocities of 2.1 km/s. J. Appl. Mech. ASME 1986, 53, 317–320. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Hanchak, S.J. Penetration limits velocity for ogive nose projectiles and limestone targets. J. Appl. Mech. ASME 2002, 69, 853–864. [Google Scholar] [CrossRef]
- Hearst, J.R.; Lynch, C.S. Measurement of in-situ strength using projectile penetration. Int. J. Rock Mech. Min. Sci. Geomech. 1994, 31, 243–251. [Google Scholar] [CrossRef]
- Ben-Dor, G.; Dubinsky, A.; Elperin, T. Engineering models of high-speed penetration into geological shields. Central Eur. J. Eng. 2014, 4, 1–19. [Google Scholar] [CrossRef]
- Nechitailo, N. Hypervelocity Penetration into Soil. Procedia Eng. 2015, 103, 427–435. [Google Scholar] [CrossRef][Green Version]
- Lohse, D.; van der Meer, D. Structures in sand streams. Nature 2009, 459, 1064–1065. [Google Scholar] [CrossRef]
- Parab, N.D.; Claus, B.; Hudspeth, M.C.; Black, J.T.; Mondal, A.; Sun, J.; Fezzaa, K.; Xiao, X.; Luo, S.N.; Chen, W. Experimental assessment of fracture of individual sand particles at different loading rates. Int. J. Impact Eng. 2014, 68, 8–14. [Google Scholar] [CrossRef]
- Bernard, R.S.; Creighton, D.C. Non-Normal Impact and Penetration: Analysis for Hard Targets and Small Angles of Attack; Technical Report S-78-14; U.S. Army Engineer Waterways Experiment Station Soils and Pavements Laboratory: Vieksburg, MS, USA, 1978. [Google Scholar]
- Byers, R.K.; Yarrington, P.; Chabai, A.J. Dynamic penetration of soil media by slender projectiles. Int. J. Eng. Sci. 1978, 16, 835–844. [Google Scholar] [CrossRef]
- Deboeuf, S.; Gondret, P.; Rabaud, M. Dynamics of grain ejection by sphere impact on a granular bed. arXiv 2008, arXiv:0808.2295. [Google Scholar] [CrossRef] [PubMed]
- Boudet, J.F.; Amarouchene, Y.; Kellay, H. Dynamics of impact cratering in shallow sand layers. Phys. Rev. Lett. 2006, 96, 158001. [Google Scholar] [CrossRef]
- Cintala, M.J.; Berthoud, L.; Horz, F. Ejection-velocity distribution from impact into coarse-grained sand. Meteor. Planet. Sci. 1999, 34, 605–623. [Google Scholar] [CrossRef]
- Poncelet, J.V. Introduction Ii la Mecanique Industrielle. Brussels, Belgium, 2nd ed.; Meline, Cans et Compagnie: Brussels, Belgium, 1839; p. 271. [Google Scholar]
- Ambroso, M.A.; Santore, C.R.; Abate, A.R.; Durian, D.J. Penetration depth for shallow impact cratering. Phys. Rev. E 2002, 71, 051305. [Google Scholar] [CrossRef] [PubMed]
- Jiang, M.; Shen, Z.; Wang, J. A novel three-dimensional contact model for granulates incorporating rolling and twisting resistances. Comput. Geotech. 2015, 65, 147–163. [Google Scholar] [CrossRef]
- Grigoryan, S.S. On basic concepts of soil dynamics. J. Appl. Math. Mech. 1960, 24, 1604–1627. [Google Scholar] [CrossRef]
- Buchely, M.; Maranon, A. Spherical Cavity Expansion Approach for the Study of Rigid-Penetrator’s Impact Problems. Appl. Mech. 2020, 1, 3. [Google Scholar] [CrossRef]
- Euler, L. Neue Grundsatze der Artillerie, 1st ed.; Reprinted in Euler’s Opera Omnia; Druck und Verlag Von B. G. Teubner: Berlin, Germany, 1922; p. 484. [Google Scholar]
- Petry, L. Monographies de Systemes D’artillerie; Meline, Cans et Compagnie: Brussels, Belgium, 1910. [Google Scholar]
- Patterson, W.J.; Albuquerque, N.M.; Sandia Laboratories. Untitled Letter Report to: Bernard, R.S. oil Dynamics Division; U.S. Army Engineer Waterways Experiment Station, CE: Vicksburg, MS, USA, 26 August 1976. [Google Scholar]
- Charters, A.C.; Summers, J.L. Comments on the Phenomena of High-Speed Impact; NOLR1238, U.S. Naval Ordance Lab.: White Oak, MD, USA, 1959; pp. 200–221. [Google Scholar]
- Young, C.W. Depth Prediction for Earth-Penetrating Projectiles. J. Soil Mech. Found. Div. Am. Soc. Civ. Eng. 1969, 95, 803–817. [Google Scholar]
- Adeli, H.; Amin, A.M.; Sierakowski, R.L. Earth penetration by solid impactors. Shock Vib. Dig. 1986, 18, 14–22. [Google Scholar] [CrossRef]
- Crull, M.; Taylor, L.; Tipton, J. Estimating ordnance penetration into earth. In Proceedings of the UXO Forum’99, Atlanta, GA, USA, 25–27 May 1999. [Google Scholar]
- Pensky, O.G. Mathematical Modelling of Dynamics of Driving Rigid Bodies into Continuous Medium using Heat Impulse Machines. Ph.D. Thesis, Perm State University, Perm, Russian, 2007. (In Russian). [Google Scholar]
- Richmond, P.W. Influence of Nose Shape and L/D Ratio on Projectile Penetration in Frozen Soil; Special Report No. 80-17; U.S. Army Cold Regions Research and Engineering Laboratory: Hanover, NH, USA, 1981. [Google Scholar]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmed, M.; AlQadhi, S.; Mallick, J.; Desmukh, M.N.; Hang, H.T. Advances in Projectile Penetration Mechanism in Soil Media. Appl. Sci. 2020, 10, 6810. https://doi.org/10.3390/app10196810
Ahmed M, AlQadhi S, Mallick J, Desmukh MN, Hang HT. Advances in Projectile Penetration Mechanism in Soil Media. Applied Sciences. 2020; 10(19):6810. https://doi.org/10.3390/app10196810
Chicago/Turabian StyleAhmed, Mohd., Saeed AlQadhi, Javed Mallick, Mohammed Noor Desmukh, and Hoang Thi Hang. 2020. "Advances in Projectile Penetration Mechanism in Soil Media" Applied Sciences 10, no. 19: 6810. https://doi.org/10.3390/app10196810
APA StyleAhmed, M., AlQadhi, S., Mallick, J., Desmukh, M. N., & Hang, H. T. (2020). Advances in Projectile Penetration Mechanism in Soil Media. Applied Sciences, 10(19), 6810. https://doi.org/10.3390/app10196810





