Design and Optimization of Lattice Structures: A Review
Abstract
1. Introduction
- (1)
- The manuscript especially distinguishes the differences between lattices and foams and honeycombs from aspects of structure and properties. Additionally, the manuscript divides lattice structures into cellular structures.
- (2)
- The manuscript reviews the definition and classification, properties and applications, and manufacturing methods of lattice structures. Especially, there are some shortcomings in the definition of lattice structures in some literature. Therefore, based on the understanding of lattice structures, the definition is given new content, which makes the definition more perfect.
- (3)
- The most excellent work is to reclassify the lattice structures, which is divided into uniform lattice structure and non-uniform lattice structure. The design and optimization methods of lattice structure are reviewed comprehensively over existing literature.
- (4)
- The manuscript also emphasizes the lattice structure of non-uniform Poisson’s ratio, which is rarely involved in other literature. The lattice structures with different Poisson’s ratios are discussed in detail.
2. Lattice Structures
2.1. The Definition and Classification of Lattice Structure
2.2. Properties and Applications of Lattice Structures
2.2.1. Properties
2.2.2. Applications
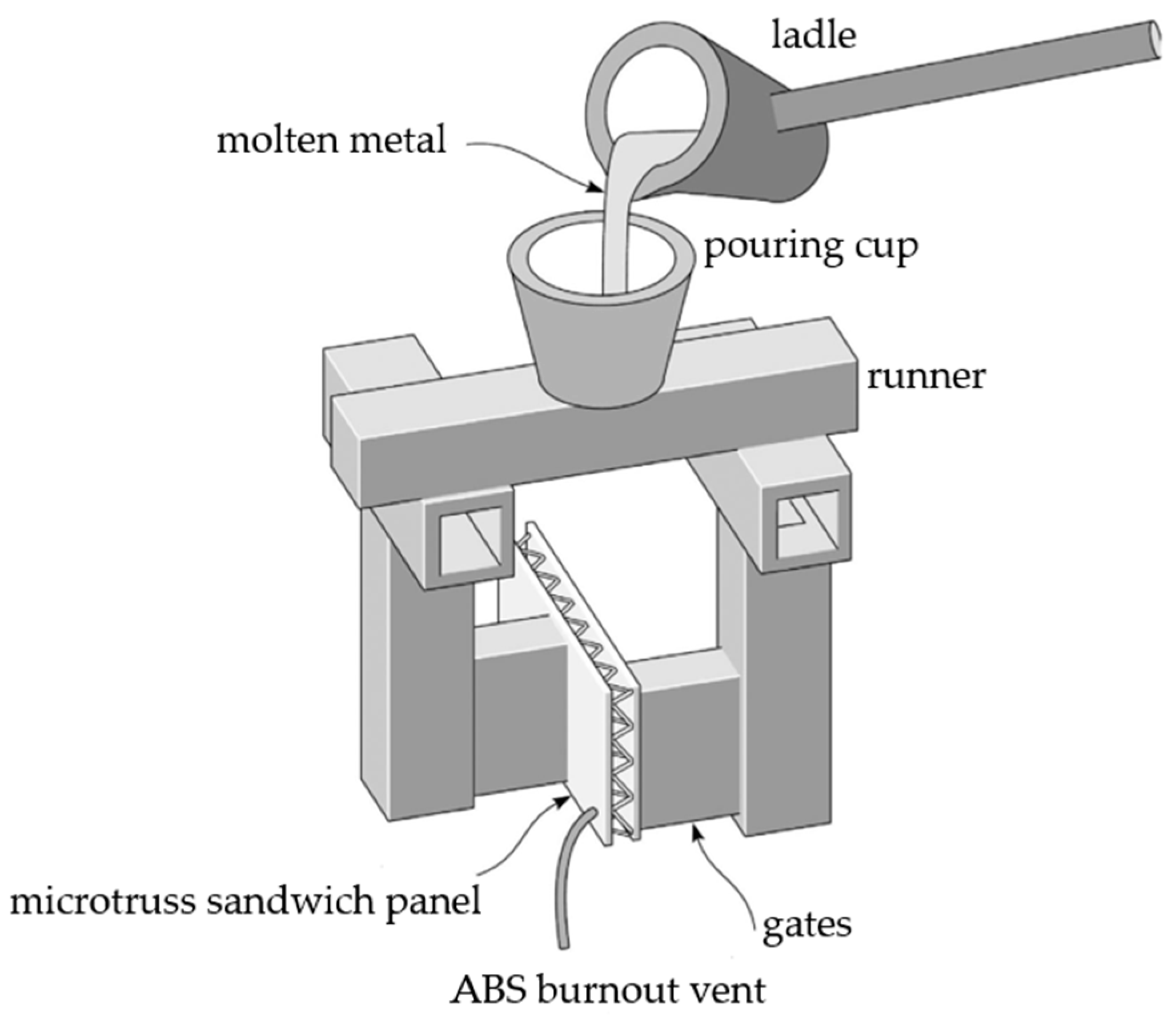
2.3. Manufacturing Methods of Lattice Structures
3. Design and Optimization of Uniform Lattice Structures
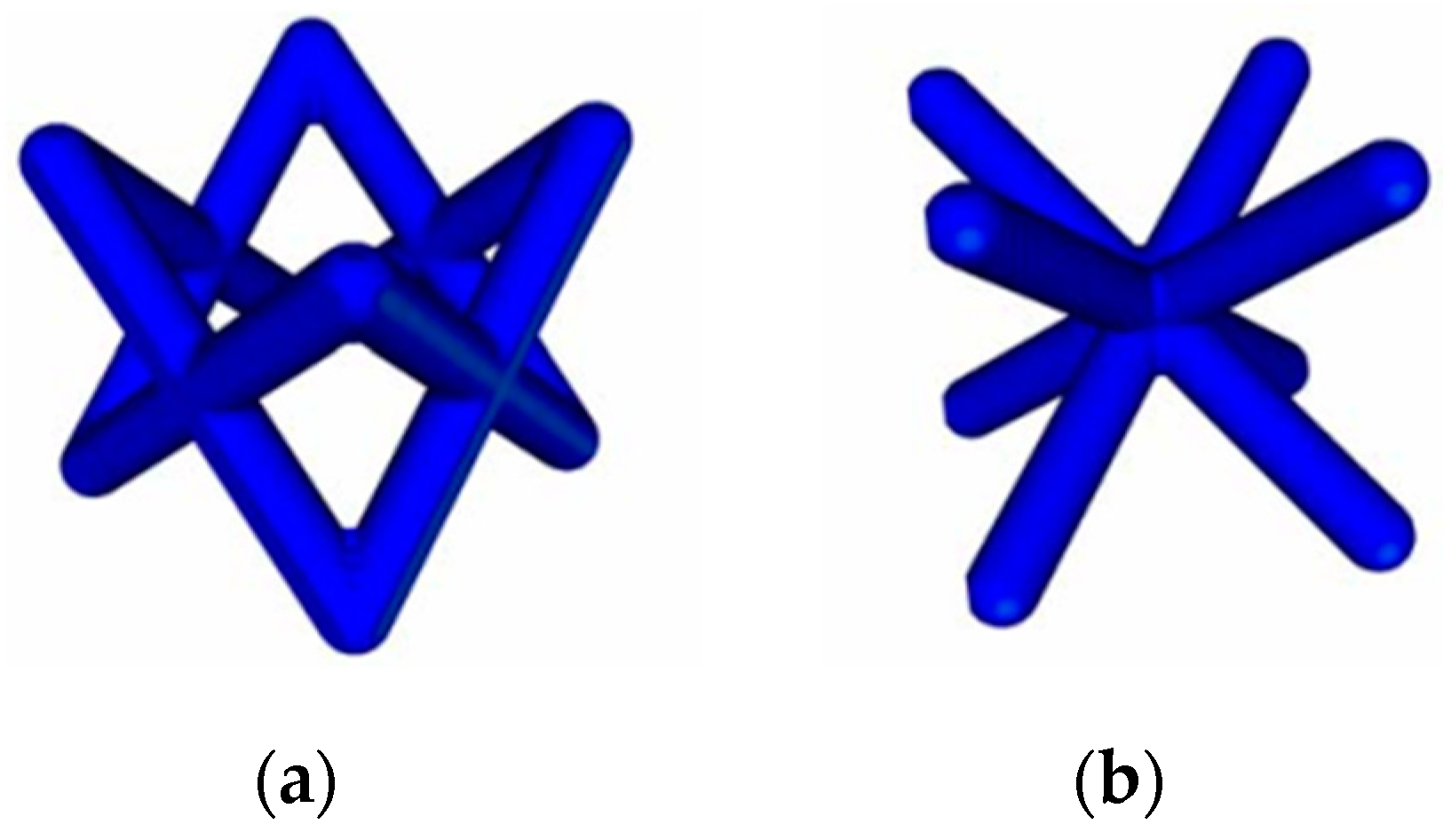
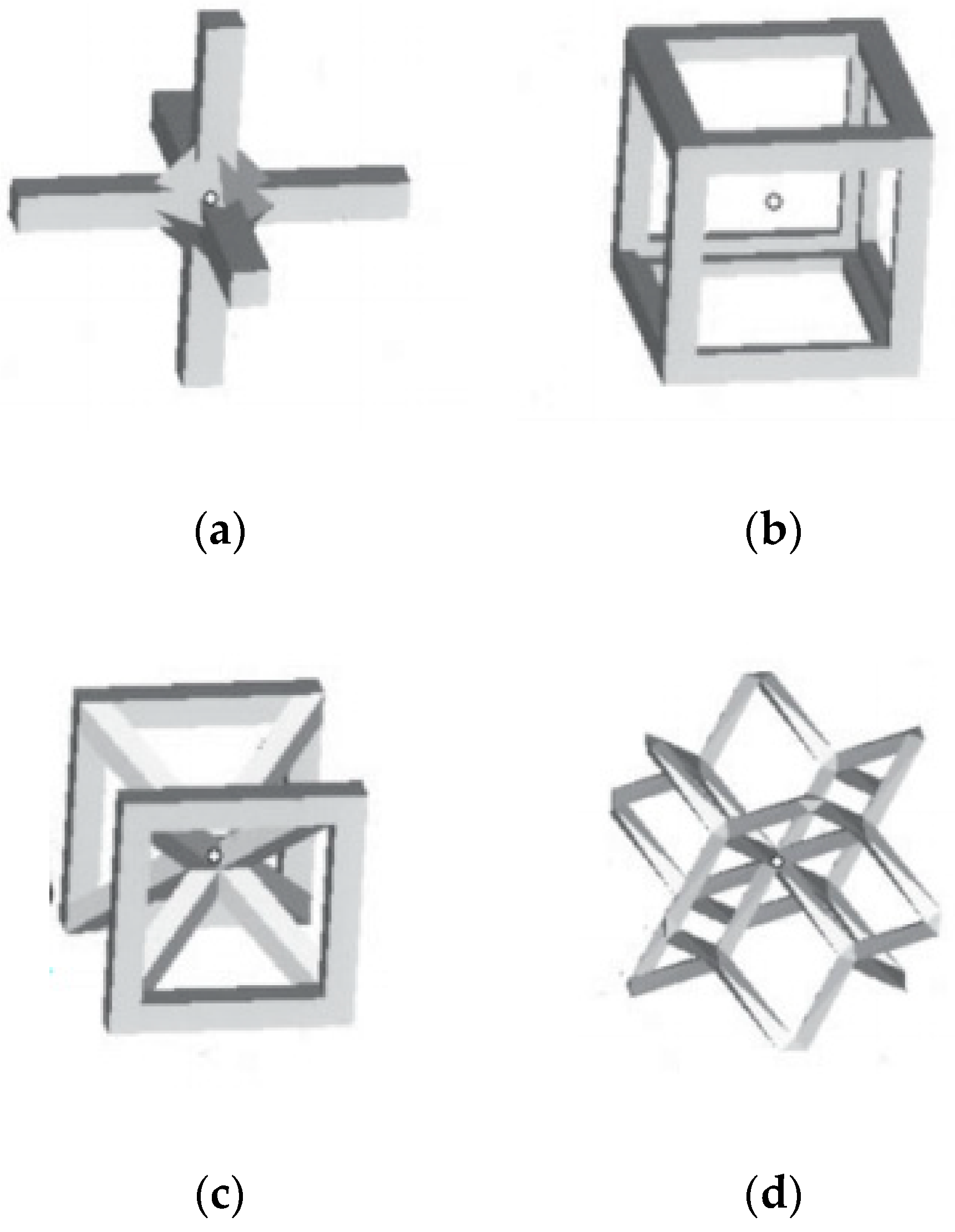
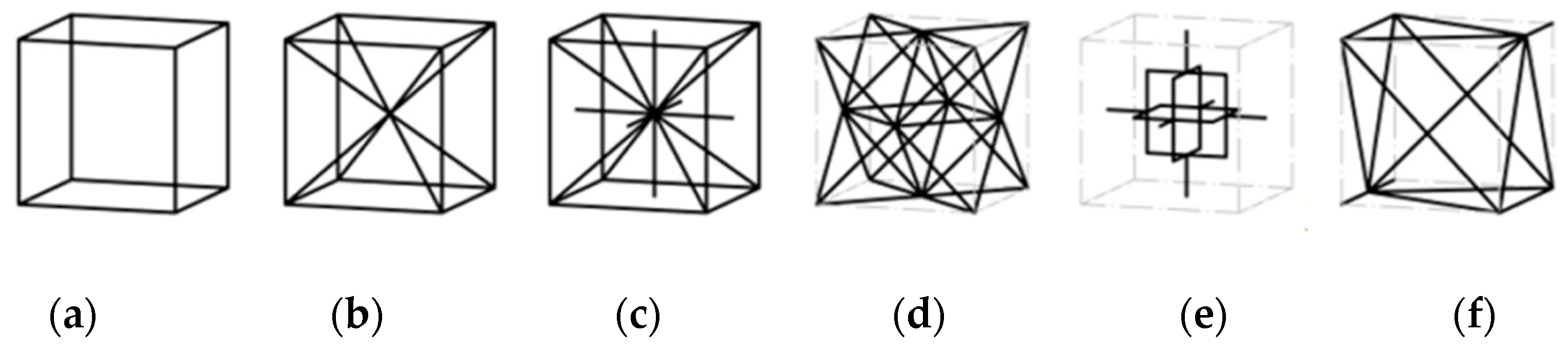
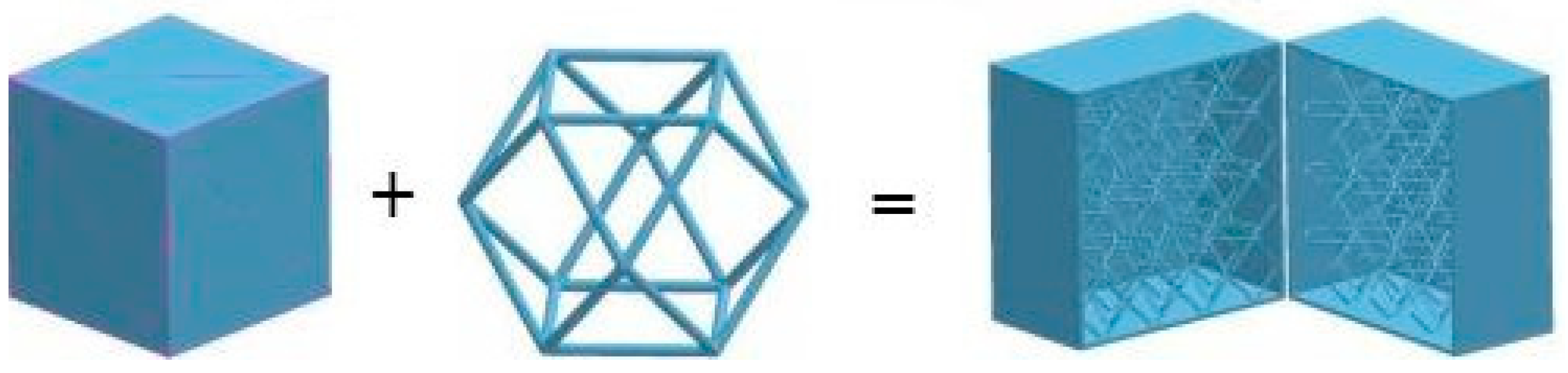
3.1. Unit Cell Design Based on Geometric Wireframe
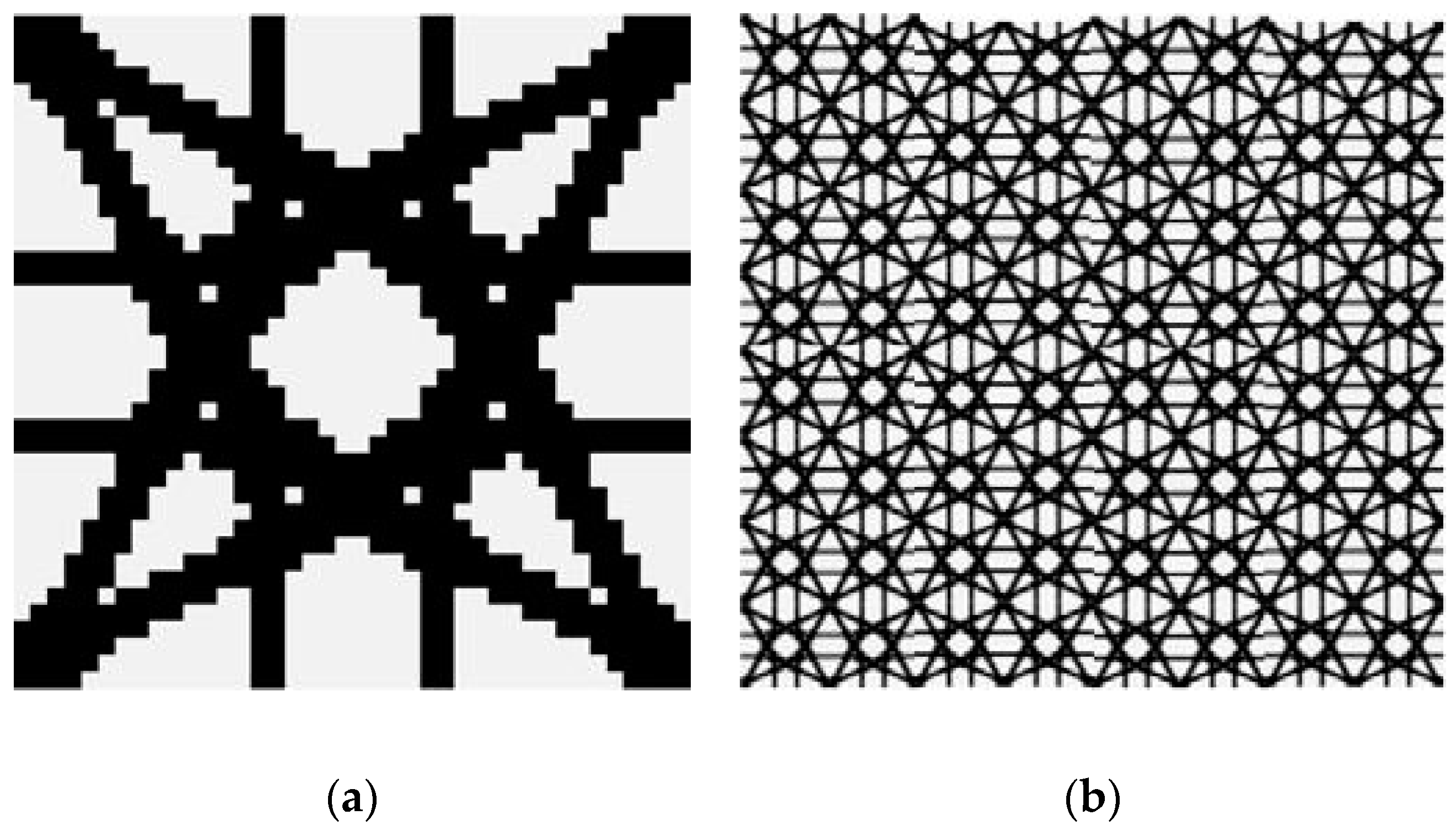
3.2. Unit Cell Design Based on Mathematical Algorithm
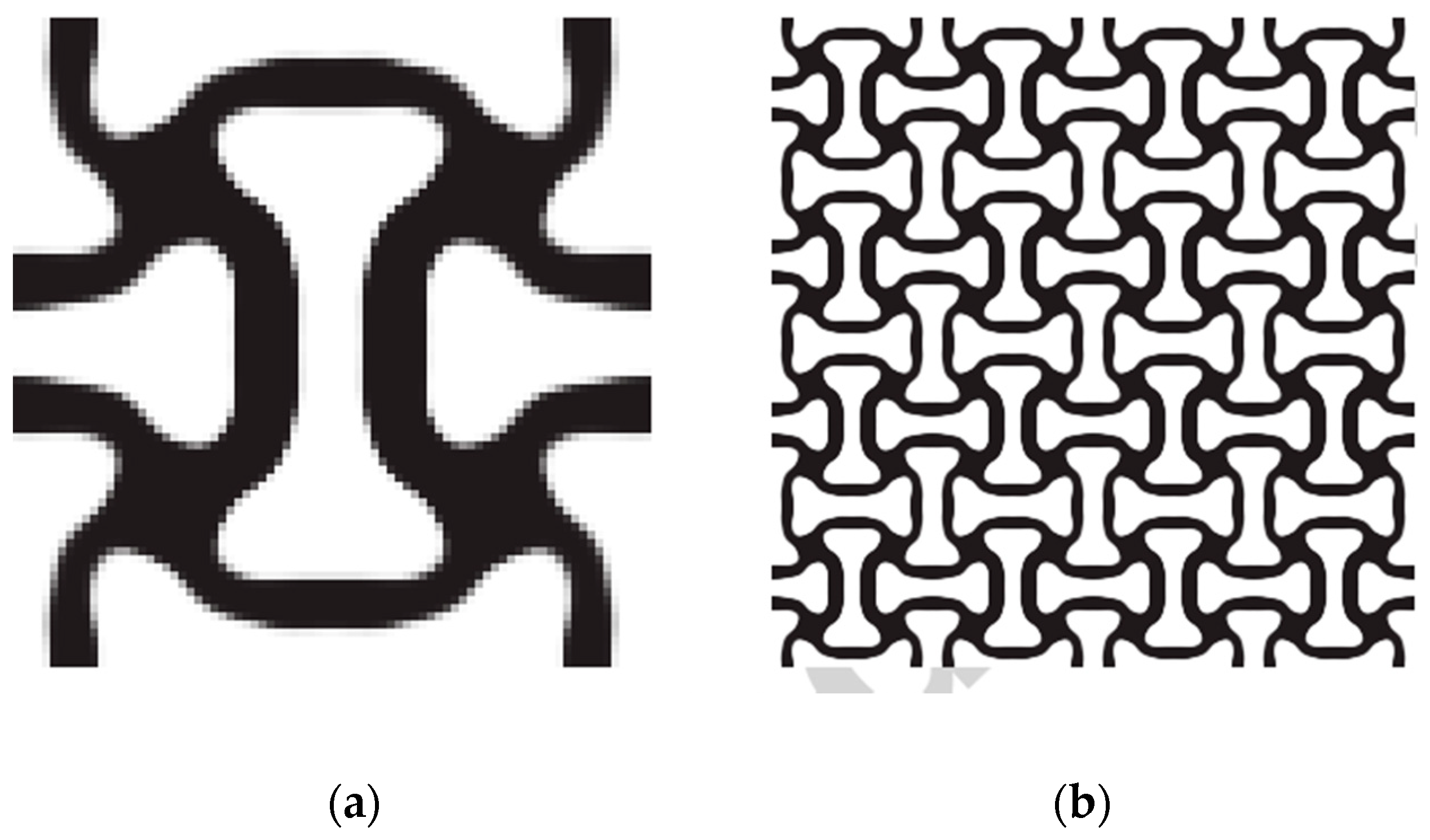
3.3. Unit Cell Design Based on Topology Optimization
4. Design and Optimization of Non-Uniform Lattices
4.1. Non-Uniform Lattice Structures Based on Functional Gradient Design
4.2. Non-Uniform Lattice Structure Based on Structural Optimization
5. Summary and Overview
- (1)
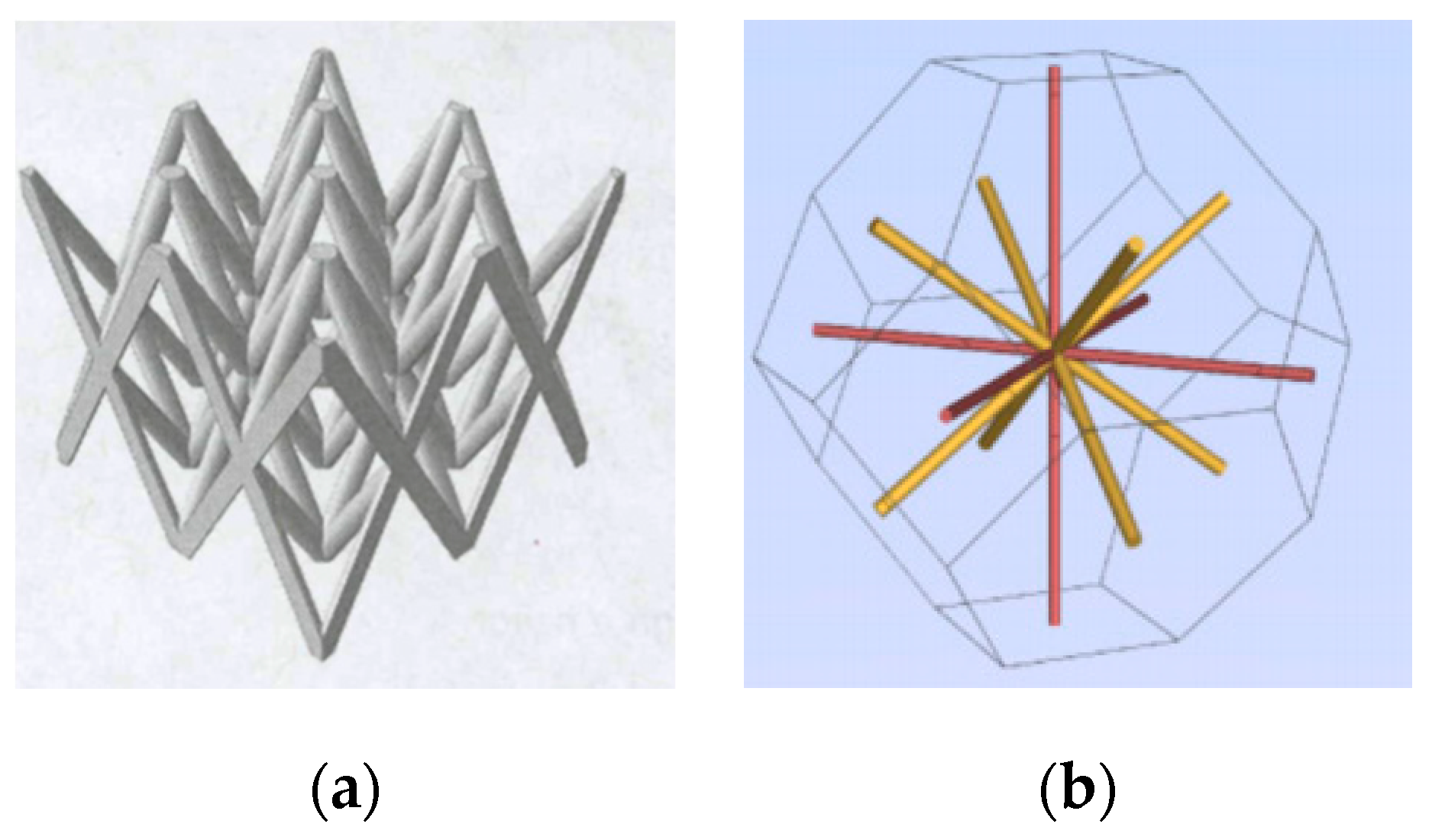
- In the current researches of lattice structures, the design of cell topological shape is insufficient, and most of the literature are still studying the existing cell structures. The performance of cell structure is directly related to lattice structure, so it is necessary to design cell topology with special performance. Leonardi et al. [206] made a preliminary exploration of the potentialities of H-C and H-C+D lattice structures.
- (2)
- In the topology optimization of lattice structures, most researcher are still focused on the strut’s sizes. The single optimization is not conducive to the design of lattice structures. Only from the point of view of optimizing struts, the lattice structures cannot meet the specific requirements. Kazemi et al. [179] proposed the geometry projection method, to optimizing the multi-material lattice structures. This is a new method to optimize lattice structure, which enriches the method of lattice topology optimization.
- (1)
- Multi-material lattice structures. Because additive manufacturing technology adopts the manufacturing method of material layer printing, different materials can be used to manufacture lattice structure in order to meet the demand of using performance. For example, with the wide application of composite materials, a variety of composite materials can be mixed for designing lattice structures.
- (2)
- Multi-structure lattice structures. At present, the research of Poisson’s ratio structure has changed from uniform Poisson’s ratio to non-uniform Poisson’s ratio. From the former positive Poisson’s ratio structure to the present negative Poisson’s ratio structure, the design and optimization of multiple structures in lattice structures are bound to be the future research trend.
- (3)
- Multi-method to design the lattice structures. With the development of CAD technology and programming language, the design of lattice structures in the future will rely heavily on computer technology to develop lattice structure databases with different topology of unit cells. At the same time, equipped with detailed performance parameters using standards for various industries.
- (4)
- Micro-scale lattice structures. Because of different design methods and fabricating methods (such as additive manufacturing technology) of lattice structures, the scales of lattice structures are divided into macro size (>0.5 mm), micro size (0.1 mm–5 mm), micro size (micron level: 100 nm–100 um; nano level: <100 nm) [74,207]. Micro-nano scale lattice structures can be realized in the future, and will be a hot spot in research field.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A



References
- Gibson, L.J. Modelling the mechanical behavior of cellular materials. Mater.Sci. Eng. A 1989, 110, 1–36. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 1997; pp. 16–21. [Google Scholar]
- Evans, A.G.; Hutchinson, J.W.; Ashby, M.F. Multifunctionality of cellular metal systems. Prog. Mater. Sci. 1998, 43, 171–221. [Google Scholar] [CrossRef]
- Yan, C.; Hao, L.; Hussein, A.; Bubb, S.L.; Young, P.; Raymont, D. Evaluation of light-weight AlSi10Mg periodic cellular lattice structures fabricated via direct metal laser sintering. J. Mater. Process. Technol. 2014, 214, 856–864. [Google Scholar] [CrossRef]
- Ashby, M. The properties of foams and lattices. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2005, 364, 15–30. [Google Scholar] [CrossRef]
- Bhate, D. Four Questions in Cellular Material Design. Materials 2019, 12, 1060. [Google Scholar] [CrossRef]
- Tao, W.; Leu, M.C. Design of lattice structure for additive manufacturing. In Proceedings of the 2016 International Symposium on Flexible Automation Cleveland (ISFA 2016), Cleveland, OH, USA, 1–3 August 2016; pp. 326–332. [Google Scholar]
- Ashby, M.F.; Evans, T.; Fleck, N.A.; Hutchinson, J.W.; Wadley, H.N.G.; Gibson, L.J. Metal Foams: A Design Guide; Butterworth-Heinemann: Oxford, UK, 2000; pp. 1–3. [Google Scholar]
- Davies, G.J.; Zhen, S. Metallic foams: Their production, properties and applications. J. Mater. Sci. 1983, 18, 1899–1911. [Google Scholar] [CrossRef]
- Tang, Y.; Zhao, Y.F. A survey of the design methods for additive manufacturing to improve functional performance. Rapid Prototyp. J. 2016, 22, 569–590. [Google Scholar] [CrossRef]
- Chen, Q.; Pugno, N.M.; Zhao, K.; Li, Z. Mechanical properties of a hollow-cylindrical-joint honeycomb. Compos. Struct. 2014, 109, 68–74. [Google Scholar] [CrossRef]
- Boldrin, L.; Hummel, S.; Scarpa, F.; Di Maio, D.; Lira, C.; Ruzzene, M.; Remillat, C.; Lim, T.-C.; Rajasekaran, R.; Patsias, S. Dynamic behaviour of auxetic gradient composite hexagonal honeycombs. Compos. Struct. 2016, 149, 114–124. [Google Scholar] [CrossRef]
- Ingrole, A.; Hao, A.; Liang, R. Design and modeling of auxetic and hybrid honeycomb structures for in-plane property enhancement. Mater. Des. 2017, 117, 72–83. [Google Scholar] [CrossRef]
- Wang, T.; Li, Z.; Wang, L.; Ma, Z.-D.; Hulbert, G.M. Dynamic Crushing Analysis of a Three-Dimensional Re-Entrant Auxetic Cellular Structure. Materials 2019, 12, 460. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Harrysson, O.; West, H.; Cormier, D. Mechanical properties of 3D re-entrant honeycomb auxetic structures realized via additive manufacturing. Int. J. Solids Struct. 2015, 69, 475–490. [Google Scholar] [CrossRef]
- Lira, C.; Innocenti, P.; Scarpa, F. Transverse elastic shear of auxetic multi re-entrant honeycombs. Compos. Struct. 2009, 90, 314–322. [Google Scholar] [CrossRef]
- Qi, D.; Lu, Q.; He, C.; Li, Y.; Wu, W.; Xiao, D. Impact energy absorption of functionally graded chiral honeycomb structures. Extreme Mech. Lett. 2019, 32, 100568. [Google Scholar] [CrossRef]
- X, L.; By, C.; Vbc, T.; Te, T. A New Chiral Beam Element for Modelling Chiral Honeycombs. J. Appl. Mech. Eng. 2017, 6. [Google Scholar] [CrossRef]
- Scarpa, F.; Blain, S.; Lew, T.; Perrott, D.; Ruzzene, M.; Yates, J.R. Elastic buckling of hexagonal chiral cell honeycombs. Compos. Part A Appl. Sci. Manuf. 2007, 38, 280–289. [Google Scholar] [CrossRef]
- Rezaei, R.; Karamooz-Ravari, M.R.; Badrossamay, M.; Kadkhodaei, M. Mechanical characterization and finite element modeling of polylactic acid BCC-Z cellular lattice structures fabricated by fused deposition modeling. Proc. IMECHE Part. C J. Mech. Eng. Sci. 2016, 203–210, 1989–1996. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Ashby, M.F.; Fleck, N.A. Foam topology: Bending versus stretching dominated architectures. Acta Mater. 2001, 49, 1035–1040. [Google Scholar] [CrossRef]
- Yang, L.; Cormier, D.; West, H.; Harrysson, O.; Knowlson, K. Non-stochastic Ti–6Al–4V foam structures with negative Poisson’s ratio. Mater. Sci. Eng. A Struct. 2012, 558, 579–585. [Google Scholar] [CrossRef]
- Dong, G.; Tang, Y.; Zhao, Y.F. A Survey of Modeling of Lattice Structures Fabricated by Additive Manufacturing. J. Mech. Des. Trans. ASME 2017, 139, 100906. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Fleck, N.A.; Ashby, M.F. Effective properties of the octet-truss lattice material. J. Mech. Phys. Solids. 2001, 49, 1747–1769. [Google Scholar] [CrossRef]
- Queheillalt, D.T.; Wadley, H.N.G. Cellular metal lattices with hollow trusses. Acta Mater. 2005, 53, 303–313. [Google Scholar] [CrossRef]
- Clough, E.C.; Ensberg, J.; Eckel, Z.C.; Ro, C.J.; Schaedler, T.A. Mechanical performance of hollow tetrahedral truss cores. Int. J. Solids Struct. 2016, 91, 115–126. [Google Scholar] [CrossRef]
- Han, Y.F.; Lu, W.F. A novel design method for nonuniform lattice structures based on topology optimization. J. Mech. Des. Trans. ASME 2018, 140, 091403. [Google Scholar] [CrossRef]
- Wadley, H.N.G. Cellular Metals Manufacturing. Adv. Eng. Mater. 2002, 40, 726–733. [Google Scholar] [CrossRef]
- Bienvenu, Y. Application and future of solid foams. C. R. Phys. 2014, 15, 719–730. [Google Scholar] [CrossRef]
- Hassan, M.R.; Scarpa, F.; Ruzzene, M.; Mohammed, N. Smart shape memory alloy chiral honeycomb. Mater. Sci. Eng. A Struct. 2008, 481, 654–657. [Google Scholar] [CrossRef]
- Mousanezhad, D.; Haghpanah, B.; Ghosh, R.; Hamouda, A.; Nayeb-Hashemi, H.; Vaziri, A. Elastic properties of chiral, anti-chiral, and hierarchical honeycombs: A simple energy-based approach. Theor. Appl. Mech. Lett. 2016, 6, 81–96. [Google Scholar] [CrossRef]
- Zhou, Z.; Zhou, J.; Fan, H. Plastic analyses of thin-walled steel honeycombs with re-entrant deformation style. Mater. Sci. Eng. A Struct. 2017, 688, 123–133. [Google Scholar] [CrossRef]
- Alderson, A.; Alderson, K.; Chirima, G.; Ravirala, N.; Zied, K. The in-plane linear elastic constants and out-of-plane bending of 3-coordinated ligament and cylinder-ligament honeycombs. Compos. Sci. Technol. 2010, 70, 1034–1041. [Google Scholar] [CrossRef]
- Mir, M.; Ali, M.N.; Sami, J.; Ansari, U. Review of Mechanics and Applications of Auxetic Structures. Adv. Mater. Sci. Eng. 2014. [Google Scholar] [CrossRef]
- Khan, S.Z.; Masood, S.H.; Cottam, R. Mechanical properties in tensile loading of H13 re-entrant honeycomb auxetic structure manufactured by direct metal deposition. MATEC Web Conf. 2015, 34, 01004. [Google Scholar] [CrossRef]
- Murray, G.; Gandhi, F.; Hayden, E. Polymer Filled Honeycombs to Achieve a Structural Material with Appreciable Damping. J. Intell. Mater. Syst. Struct. 2013, 23, 703–718. [Google Scholar] [CrossRef]
- Vaidya, U.K.; Ulven, C.; Pillay, S.; Ricks, H. Impact Damage of Partially Foam-filled Co-injected Honeycomb Core Sandwich Composites. J. Compos. Mater. 2003, 37, 611–626. [Google Scholar] [CrossRef]
- Takemiya, H. Field vibration mitigation by honeycomb WIB for pile foundations of a high-speed train viaduct. Soil. Dyn. Earthq. Eng. 2004, 24, 69–87. [Google Scholar] [CrossRef]
- Murray, G.J.; Gandhi, F. Auxetic honeycombs with lossy polymeric infills for high damping structural materials. J. Intell. Mater. Syst. Struct. 2013, 24, 1090–1104. [Google Scholar] [CrossRef]
- Evans, A.; He, M.; Deshpande, V.; Hutchinson, J.; Jacobsen, A.; Carter, W. Concepts for enhanced energy absorption using hollow micro-lattices. Int. J. Impact Eng. 2010, 37, 947–959. [Google Scholar] [CrossRef]
- Schaedler, T.A.; Ro, C.J.; Sorensen, A.E.; Eckel, Z.; Yang, S.S.; Carter, W.B.; Jacobsen, A.J. Designing metallic microlattices for energy absorber applications. Adv. Eng. Mater. 2014, 16, 276–283. [Google Scholar] [CrossRef]
- Ozdemir, Z.; Hernandez-Nava, E.; Tyas, A.; Warren, J.A.; Fay, S.D.; Goodall, R.; Todd, I.; Askes, H. Energy absorption in lattice structures in dynamics: Experiments. Int. J. Impact Eng. 2016, 89, 49–61. [Google Scholar] [CrossRef]
- Maloney, K.; Fink, K.D.; Schaedler, T.A.; Kolodziejska, J.A.; Jacobsen, A.J.; Roper, C.S. Multifunctional heat exchangers derived from three-dimensional micro-lattice structures. Int. J. Heat Mass Trans. 2012, 55, 2486–2493. [Google Scholar] [CrossRef]
- Lu, T.J.; Valdevit, L.; Evans, A.G. Active cooling by metallic sandwich structures with periodic cores. Prog. Mater. Sci. 2005, 50, 798–815. [Google Scholar] [CrossRef]
- Wadley, H.N.G.; Queheillalt, D.T. Thermal Applications of Cellular Lattice Structures. Mater. Sci. Forum 2007, 539, 242–247. [Google Scholar] [CrossRef]
- Valdevit, L.; Pantano, A.; Stone, H.; Evans, A. Optimal active cooling performance of metallic sandwich panels with prismatic cores. Int. J. Heat Mass Transf. 2006, 49, 3819–3830. [Google Scholar] [CrossRef]
- Yin, S.; Chen, H.; Wu, Y.; Li, Y.; Xu, J. Introducing composite lattice core sandwich structure as an alternative proposal for engine hood. Compos. Struct. 2018, 201, 131–140. [Google Scholar] [CrossRef]
- Melchels, F.P.W.; Feijen, J.; Grijpma, D.W. A review on stereolithography and its applications in biomedical engineering. Biomaterials 2010, 31, 6121–6130. [Google Scholar] [CrossRef]
- Jetté, B.; Brailovski, V.; Dumas, M.; Simoneau, C.; Terriault, P. Femoral stem incorporating a diamond cubic lattice structure: Design, manufacture and testing. J. Mech. Behav. Biomed. 2018, 77, 58–72. [Google Scholar] [CrossRef]
- Dumas, M.; Terriault, P.; Brailovski, V. Modelling and characterization of a porosity graded lattice structure for additively manufactured biomaterials. Mater. Des. 2017, 121, 383–392. [Google Scholar] [CrossRef]
- Heinl, P.; Müller, L.; Körner, C.; Singer, R.F.; Müller, F.A. Cellular Ti–6Al–4V structures with interconnected macro porosity for bone implants fabricated by selective electron beam melting. Acta Biomater. 2008, 4, 1536–1544. [Google Scholar] [CrossRef]
- Chen, W.M.; Lee, S.J.; Lee, P.V.S. Failure analysis of an additive manufactured porous titanium structure for orthopedic implant applications. Mater. Sci. Forum 2016, 863, 45–49. [Google Scholar] [CrossRef]
- Gorguluarslan, R.M.; Choi, S.K.; Saldana, C.J. Uncertainty quantification and validation of 3D lattice scaffolds for computer-aided biomedical applications. J. Mech. Behav. Biomed. 2017, 71, 428–440. [Google Scholar] [CrossRef]
- Soro, N.; Attar, H.; Brodie, E.; Veidt, M.; Molotnikov, A.; Dargusch, M.S. Evaluation of the mechanical compatibility of additively manufactured porous Ti-25Ta alloy for load-bearing implant applications. J. Mech. Behav. Biomed. 2019, 97, 149–158. [Google Scholar] [CrossRef] [PubMed]
- Rahmani, R.; Antonov, M.; Kollo, L.; Holovenko, Y.; Prashanth, K.G. Mechanical behavior of Ti6Al4V scaffolds filled with CaSiO3 for implant applications. Appl. Sci. 2019, 9, 3844. [Google Scholar] [CrossRef]
- Moon, S.K.; Tan, Y.E.; Hwang, J.; Yoon, Y.-J. Application of 3D printing technology for designing light-weight unmanned aerial vehicle wing structures. Int. J. Precis. Eng. Manuf. Technol. 2014, 1, 223–228. [Google Scholar] [CrossRef]
- Magerramova, L.; Volkov, M.; Afonin, A.; Svinareva, M.; Kalinin, D. Application of light lattice structures for gas turbine engine fan blades. In Proceedings of the 31st congress of the international council of the aeronautical sciences (ICAS), Belo Horizonte, Brazil, 9–14 September 2018; pp. 1–10. [Google Scholar]
- Lou, J.; Ma, L.; Wu, L.Z. Free vibration analysis of simply supported sandwich beams with lattice truss core. Mater. Sci. Eng. B Solid-State Mater. Adv. Technol. 2012, 177, 1712–1716. [Google Scholar] [CrossRef]
- Xu, M.; Qiu, Z. Free vibration analysis and optimization of composite lattice truss core sandwich beams with interval parameters. Compos. Struct. 2013, 106, 85–95. [Google Scholar] [CrossRef]
- Yin, S.; Wu, L.; Yang, J.; Ma, L.; Nutt, S. Damping and low-velocity impact behavior of filled composite pyramidal lattice structures. J. Compos. Mater. 2014, 48, 1789–1800. [Google Scholar] [CrossRef]
- Cheng, L.; Liang, X.; Belski, E.; Wang, X.; Sietins, J.M.; Ludwick, S.; To, A.C.; Ludwick, S.J. Natural frequency optimization of variable-density additive manufactured lattice structure: Theory and experimental validation. J. Manuf. Sci. Eng. Trans. ASME 2018, 140, 100502. [Google Scholar] [CrossRef]
- Horn, T.J.; Harrysson, O.L.A. Overview of current additive manufacturing technologies and selected applications. Sci. Prog. 2012, 95, 255–282. [Google Scholar] [CrossRef]
- Barynin, V.A.; Bunakov, V.A.; Rasin, A.F.; Vasiliev, V.V. Aerospace composite lattice structures. In Proceedings of the 120th International Conference on Composite Materials, Paris, France, 5–9 July 1999. [Google Scholar]
- Vasiliev, V.V.; Razin, A.F. Anisogrid composite lattice structures for spacecraft and aircraft applications. Compos. Struct. 2006, 76, 182–189. [Google Scholar] [CrossRef]
- Vasiliev, V.V.; Barynin, V.A.; Razin, A.F. Anisogrid composite lattice structures-Development and aerospace applications. Compos. Struct. 2012, 94, 1117–1127. [Google Scholar] [CrossRef]
- Nazir, A.; Abate, K.M.; Kumar, A.; Jeng, J.-Y. A state-of-the-art review on types, design, optimization, and additive manufacturing of cellular structures. Int. J. Adv. Manuf. Technol. 2019, 104, 3489–3510. [Google Scholar] [CrossRef]
- Gianpaolo, S.; Stefano, R.; Roberto, M.; Conchen, G. Geometric Modeling of Cellular Materials for Additive Manufacturing in Biomedical Field: A Review. Appl. Bionics Biomech. 2018. [Google Scholar] [CrossRef]
- Tamburrino, F.; Graziosi, S.; Bordegoni, M. The Design Process of Additively Manufactured Mesoscale Lattice Structures: A Review. J. Comput. Inf. Sci. Eng. 2018, 18, 040801. [Google Scholar] [CrossRef]
- Schaedler, T.A.; Carter, W.B. Architected Cellular Materials. Annu. Rev. Mater. Res. 2016, 46, 187–210. [Google Scholar] [CrossRef]
- Gorguluarslan, R.M.; Gandhi, U.; Mandapati, R.; Choi, S.-K. Design and fabrication of periodic lattice-based cellular structures. Comput. Aided. Des. Appl. 2015, 13, 50–62. [Google Scholar] [CrossRef]
- Zok, F.W.; Latture, R.M.; Begley, M.R. Periodic truss structures. J. Mech. Phys. Solids 2016, 96, 184–203. [Google Scholar] [CrossRef]
- Helou, M.; Vongbunyong, S.; Kara, S. Finite Element Analysis and Validation of Cellular Structures. Procedia CIRP 2016, 50, 94–99. [Google Scholar] [CrossRef]
- Seharing, A.; Azman, A.H.; Abdullah, S. A review on integration of lightweight gradient lattice structures in additive manufacturing parts. Adv. Mech. Eng. 2020, 12, 1–21. [Google Scholar] [CrossRef]
- Helou, M.; Kara, S. Design, analysis and manufacturing of lattice structures: An overview. Int. J. Comput. Integr. Manuf. 2017, 31, 243–261. [Google Scholar] [CrossRef]
- Wang, H.; Rosen, D.W. Parametric Modeling Method for Truss Structures. In Proceedings of the DETC’02 ASME 2002 Design Engineering Technical Conferences and Computer and Information in Engineering Conference, Montreal, QC, Canada, 29 September–2 October 2002; pp. 759–767. [Google Scholar]
- Xiao, L.; Song, W. Additively-manufactured functionally graded Ti-6Al-4V lattice structures with high strength under static and dynamic loading: Experiments. Int. J. Impact Eng. 2018, 111, 255–272. [Google Scholar] [CrossRef]
- Han, Y.; Lu, W. Evolutionary design of nonuniform cellular structures with optimized Poisson’s ratio distribution. Mater. Des. 2017, 141, 384–394. [Google Scholar] [CrossRef]
- Han, Y.; Lu, W. Optimization design of nonuniform cellular structures for additive manufacturing. In Proceedings of the ASME 2018 13th International Manufacturing Science and Engineering Conference MSEC2018 College Station, College Station, TX, USA, 18–22 June 2018. V001T01A033. [Google Scholar]
- Radman, A.; Huang, X.; Xie, Y.M. Topology optimization of functionally graded cellular materials. J. Mater. Sci. 2013, 48, 1503–1510. [Google Scholar] [CrossRef]
- Daynes, S.; Feih, S.; Lu, W.F.; Wei, J. Optimization of functionally graded lattice structures using isostatic lines. Mater. Des. 2017, 127, 215–223. [Google Scholar] [CrossRef]
- Lira, C.; Scarpa, F.; Rajasekaran, R. A Gradient Cellular Core for Aeroengine Fan Blades Based on Auxetic Configurations. J. Intell. Mater. Syst. Struct. 2011, 22, 907–917. [Google Scholar] [CrossRef]
- Bauer, J.; Hengsbach, S.; Tesari, I.; Schwaiger, R.; Kraft, O. High-strength cellular ceramic composites with 3D microarchitecture. Proc. Natl. Acad. Sci. USA 2014, 111, 2453–2458. [Google Scholar] [CrossRef]
- Wendy, X.; Greer, J.R. Ultra-strong architected Cu meso-lattices. Extrem. Mech. Lett. 2015, 2, 7–14. [Google Scholar] [CrossRef]
- Messner, M.C. Optimal lattice-structured materials. J. Mech. Phys. Solids 2016, 96, 162–183. [Google Scholar] [CrossRef]
- Hou, A.; Gramoll, K. Compressive Strength of Composite Lattice Structures. J. Reinf. Plast. Comp. 2016, 17, 462–493. [Google Scholar] [CrossRef]
- Takezawa, A.; Koizumi, Y.; Kobashi, M. High-stiffness and strength porous maraging steel via topology optimization and selective laser melting. Addit. Manuf. 2017, 18, 194–202. [Google Scholar] [CrossRef]
- Parthasarathy, J.; Starly, B.; Raman, S.; Christensen, A. Mechanical Evaluation of Porous Titanium (Ti6Al4V) Structures with Electron Beam Melting (EBM). J. Mech. Behav. Biomed. Mater. 2010, 3, 249–259. [Google Scholar] [CrossRef]
- Mahshid, R.; Hansen, H.N.; Hojbjerre, K.L. Strength analysis and modeling of cellular lattice structures manufactured using selective laser melting for tooling applications. Mater. Des. 2016, 104, 276–283. [Google Scholar] [CrossRef]
- Tancogne-Dejean, T.; Spierings, A.B.; Mohr, D. Additively-manufactured metallic micro-lattice materials for high specific energy absorption under static and dynamic loading. Acta Mater. 2016, 116, 14–28. [Google Scholar] [CrossRef]
- Al-Saedi, D.S.; Masood, S.H.; Faizan-Ur-Rab, M.; Alomarah, A.; Ponnusamy, P. Mechanical properties and energy absorption capability of functionally graded F2BCC lattice fabricated by SLM. Mater. Des. 2018, 144, 32–44. [Google Scholar] [CrossRef]
- Maskery, I.; Aboulkhair, N.T.; Aremu, A.; Tuck, C.; Ashcroft, I. Compressive failure modes and energy absorption in additively manufactured double gyroid lattices. Addit. Manuf. 2017, 16, 24–29. [Google Scholar] [CrossRef]
- Syam, W.P.; Jianwei, W.; Zhao, B.; Maskery, I.; Elmadih, W.; Leach, R.K. Design and analysis of strut-based lattice structures for vibration isolation. Precis. Eng. 2018, 52, 494–506. [Google Scholar] [CrossRef]
- Elmadih, W.; Syam, W.P.; Maskery, I.; Chronopoulos, D.; Leach, R.K. Mechanical vibration bandgaps in surface-based lattices. Addit. Manuf. 2019, 25, 421–429. [Google Scholar] [CrossRef]
- D’Alessandro, L.; Zega, V.; Ardito, R.; Corigliano, A. 3D auxetic single material periodic structure with ultra-wide tunable bandgap. Sci. Rep.-UK 2018, 8, 2262. [Google Scholar] [CrossRef]
- D’Alessandro, L.; Belloni, E.; Ardito, R.; Braghin, F.; Corigliano, A. Mechanical low-frequency filter via modes separation in 3D periodic structures. Appl. Phys. Lett. 2017, 111, 231902. [Google Scholar] [CrossRef]
- D’Alessandro, L.; Belloni, E.; Ardito, R.; Corigliano, A.; Braghin, F. Modeling and experimental verification of an ultra-wide bandgap in 3D phononic crystal. Appl. Phys. Lett. 2016, 109, 221907. [Google Scholar] [CrossRef]
- Cheng, X.; Wei, K.; He, R.; Pei, Y.; Fang, D. The equivalent thermal conductivity of lattice core sandwich structure: A predictive model. Appl. Therm. Eng. 2016, 93, 236–243. [Google Scholar] [CrossRef]
- Catchpole-Smith, S.; Sélo, R.; Davis, A.; Ashcroft, I.; Tuck, C.; Clare, A. Thermal Conductivity of TPMS Lattice Structures Manufactured via Laser Powder Bed Fusion. Addit. Manuf. 2019, 30, 100846. [Google Scholar] [CrossRef]
- Fink, K.D.; Kolodziejska, J.A.; Jacobsen, A.J.; Roper, C.S. Fluid dynamics of flow through microscale lattice structures formed from self-propagating photopolymer waveguides. Aiche. J. 2011, 57, 2636–2646. [Google Scholar] [CrossRef]
- Do, G.; Geißelbrecht, M.; Schwieger, W.; Freund, H. Additive manufacturing of interpenetrating periodic open cellular structures (interPOCS) with in operando adjustable flow characteristics. Chem. Eng. Process. 2019, 148, 107786. [Google Scholar] [CrossRef]
- Jamshidinia, M.; Wang, L.; Tong, W.; Ajlouni, R.; Kovacevic, R. Fatigue properties of a dental implant produced by electron beam melting® (EBM). J. Mater. Process. Tech. 2015, 226, 255–263. [Google Scholar] [CrossRef]
- Zargarian, A.; Esfahanian, M.; Kadkhodapour, J.; Ziaei-Rad, S. Numerical simulation of the fatigue behavior of additive manufactured titanium porous lattice structures. Mater. Sci. Eng. C Mater. 2016, 60, 339–347. [Google Scholar] [CrossRef]
- Zargarian, A.; Esfahanian, M.; Kadkhodapour, J.; Ziaei-Rad, S.; Zamani, D. On the fatigue behavior of additive manufactured lattice structures. Theor. Appl. Fract. Mech. 2019, 100, 225–232. [Google Scholar] [CrossRef]
- Ren, D.; Li, S.; Wang, H.; Hou, W.; Hao, Y.; Jin, W.; Yang, R.; Misra, R.D.K.; Murr, L.E.; Zhao, S. Fatigue behavior of Ti-6Al-4V cellular structures fabricated by additive manufacturing technique. J. Mater. Sci. Technol. 2018, 35, 285–294. [Google Scholar] [CrossRef]
- Köhnen, P.; Haase, C.; Bültmann, J.; Ziegler, S.; Schleifenbaum, J.H.; Bleck, W. Mechanical properties and deformation behavior of additively manufactured lattice structures of stainless steel. Mater. Des. 2018, 145, 205–217. [Google Scholar] [CrossRef]
- Zhao, S.; Li, S.; Hou, W.; Hao, Y.; Yang, R.; Misra, R. The influence of cell morphology on the compressive fatigue behavior of Ti-6Al-4V meshes fabricated by electron beam melting. J. Mech. Behav. Biomed. 2016, 59, 251–264. [Google Scholar] [CrossRef]
- Zhao, S.; Li, S.; Wang, S.; Hou, W.; Li, Y.; Zhang, L.-C.; Hao, Y.; Yang, R.; Misra, R.; Murr, L. Compressive and fatigue behavior of functionally graded Ti-6Al-4V meshes fabricated by electron beam melting. Acta Mater. 2018, 150, 1–15. [Google Scholar] [CrossRef]
- Totaro, G.; Gürdal, Z. Optimal design of composite lattice shell structures for aerospace applications. Aerosp. Sci. Technol. 2009, 13, 157–164. [Google Scholar] [CrossRef]
- Zhang, J.H.; Zhang, W.H.; Xia, L. Topology Optimization in Aircraft and Aerospace Structures Design. Arch. Comput. Methods Eng. 2016, 23, 595–622. [Google Scholar]
- Zhu, L.; Li, N.; Childs, P.R.N. Light-weighting in aerospace component and system design. Propuls. Power Res. 2018, 7, 103–119. [Google Scholar] [CrossRef]
- Podshivalov, L.; Gomes, C.M.; Zocca, A.; Guenster, J.; Bar-Yoseph, P.; Fischer, A. Design, analysis and additive manufacturing of porous structures for biocompatible micro-scale scaffolds. Procedia CIRP 2013, 5, 247–252. [Google Scholar] [CrossRef]
- Arabnejad, S.; Johnston, R.B.; Pura, J.A.; Singh, B.; Tanzer, M.; Pasini, D. High-strength porous biomaterials for bone replacement: A strategy to assess the interplay between cell morphology, mechanical properties, bone ingrowth and manufacturing constraints. Acta Biomater. 2016, 30, 345–356. [Google Scholar] [CrossRef] [PubMed]
- Dantas, A.C.; Scalabrin, D.H.; De Farias, R.; Barbosa, A.A.; Ferraz, A.V.; Wirth, C. Design of Highly Porous Hydroxyapatite Scaffolds by Conversion of 3D Printed Gypsum Structures—A Comparison Study. Procedia CIRP 2015, 49, 55–60. [Google Scholar] [CrossRef]
- Du Plessis, A.; Yadroitsava, I.; Yadroitsev, I.; Le Roux, S.; Blaine, D. Numerical comparison of lattice unit cell designs for medical implants by additive manufacturing. Virtual Phys. Prototyp. 2018, 13, 1–16. [Google Scholar] [CrossRef]
- Zhang, D.Z.; Zhang, D.Z.; Zhang, P.; Zhao, M.; Jafar, S. Mechanical properties of optimized diamond lattice structure for bone scaffolds fabricated via selective laser melting. Materials 2018, 11, 374. [Google Scholar]
- Wu, J.; Aage, N.; Westermann, R.; Sigmund, O.; Westermann, R. Infill Optimization for Additive Manufacturing-Approaching Bone-Like Porous Structures. IEEE Trans. Vis. Comput. Graph. 2018, 24, 1127–1140. [Google Scholar] [CrossRef]
- Zhang, B.; Pei, X.; Zhou, C.; Fan, Y.; Jiang, Q.; Ronca, A.; D’Amora, U.; Chen, Y.; Li, H.; Sun, Y.; et al. The biomimetic design and 3D printing of customized mechanical properties porous Ti6Al4V scaffold for load-bearing bone reconstruction. Mater. Des. 2018, 152, 30–39. [Google Scholar] [CrossRef]
- Zadpoor, A.A. Meta-biomaterials. Biomater. Sci.-UK 2020, 8, 18–38. [Google Scholar] [CrossRef] [PubMed]
- Zadpoor, A.A. Additively manufactured porous metallic biomaterials. J. Mater. Chem. B 2019, 7, 4088–4117. [Google Scholar] [CrossRef] [PubMed]
- Du Plessis, A.; Broeckhoven, C.; Yadroitsava, I.; Yadroitsev, I.; Hands, C.H.; Kunju, R.; Bhate, D. Beautiful and Functional: A Review of Biomimetic Design in Additive Manufacturing. Addit. Manuf. 2019, 27, 408–427. [Google Scholar] [CrossRef]
- Cancer Patient Receives 3D Printed Ribs in World-First Surgery. Available online: http://blog.csiro.au/cancer-patient-receives-3d-printed-ribs-in-world-first-surgery/ (accessed on 11 September 2015).
- Queheillalt, D.T.; Murty, Y.; Wadley, H.N.G. Mechanical properties of an extruded pyramidal lattice truss sandwich structure. Scr. Mater. 2008, 58, 76–79. [Google Scholar] [CrossRef]
- Kooistra, G.W.; Wadley, H.N.G. Lattice truss structures from expanded metal sheet. Mater. Des. 2007, 28, 507–514. [Google Scholar] [CrossRef]
- Kooistra, G.W.; Deshpande, V.S.; Wadley, H.N.G. Compressive behavior of age hardenable tetrahedral lattice truss structures made from aluminum. Acta Mater. 2004, 52, 4229–4237. [Google Scholar] [CrossRef]
- Zhao, B.; Li, Z.Q.; Hou, H.L.; Han, X.; Liao, J.; Tan, Z.; Bai, B.; Bai, L. Research Progress on Fabrication Methods of Metal Three Dimensional Lattice. Rare Met. Mater. Eng. 2016, 45, 2189–2220. [Google Scholar]
- Wadley, H.N.G. Multifunctional periodic cellular metals. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2006, 364, 31–68. [Google Scholar] [CrossRef]
- Yan, C.; Hao, L.; Hussein, A.; Raymont, D. Evaluations of cellular lattice structures manufactured using selective laser melting. Int. J. Mach. Tools Manuf. 2012, 62, 32–38. [Google Scholar] [CrossRef]
- Cerardi, A.; Caneri, M.; Meneghello, R.; Concheri, G.; Ricotta, M. Mechanical characterization of polyamide cellular structures fabricated using selective laser sintering technologies. Mater. Des. 2013, 46, 910–915. [Google Scholar] [CrossRef]
- Tang, Y.; Zhou, Y.; Hoff, T.; Garon, M.; Zhao, Y.F. Elastic modulus of 316 stainless steel lattice structure fabricated via binder jetting process. Mater. Sci. Technol. 2016, 32, 648–656. [Google Scholar] [CrossRef]
- Xiao, L.; Song, W.-D.; Hu, M.; Li, P. Compressive properties and micro-structural characteristics of Ti–6Al–4V fabricated by electron beam melting and selective laser melting. Mater. Sci. Eng. A Struct. 2019, 764, 138204. [Google Scholar] [CrossRef]
- Wally, Z.J.; Haque, A.M.; Feteira, A.; Claeyssens, F.; Goodall, R.; Reilly, G.C. Selective laser melting processed Ti6Al4V lattices with graded porosities for dental applications. J. Mech. Behave. Biomed. 2018, 90, 20–29. [Google Scholar] [CrossRef] [PubMed]
- Gangireddy, S.; Komarasamy, M.; Faierson, E.J.; Mishra, R.S. High strain rate mechanical behavior of Ti-6A1-4V octet lattice structures additively manufactured by selective laser melting (SLM). Mater. Sci. Eng. A-Struct. 2019, 745, 231–239. [Google Scholar] [CrossRef]
- Yan, X.; Li, Q.; Yin, S.; Chen, Z.; Jenkins, R.; Chen, C.; Wang, J.; Ma, W.; Bolot, R.; Lupoi, R.; et al. Mechanical and invitro study of an isotropic Ti6Al4V lattice structure fabricated using selective laser melting. J. Alloys Compd. 2019, 782, 209–223. [Google Scholar] [CrossRef]
- Bai, L.; Zhang, J.; Chen, X.; Yi, C.; Chen, R.; Zhang, Z. Configuration Optimization Design of Ti6Al4V Lattice Structure Formed by SLM. Materials 2018, 11, 1856. [Google Scholar] [CrossRef]
- Bai, L.; Zhang, J.; Xiong, Y.; Chen, X.; Sun, Y.; Gong, C.; Pu, H.; Wu, X.; Luo, J. Influence of unit cell pose on the mechanical properties of Ti6Al4V lattice structures manufactured by selective laser melting. Addit. Manuf. 2020, 34, 101222. [Google Scholar] [CrossRef]
- Dong, Z.; Liu, Y.; Li, W.; Liang, J. Orientation dependency for microstructure, geometric accuracy and mechanical properties of selective laser melting AlSi10Mg lattices. J. Alloys Compd. 2019, 791, 490–500. [Google Scholar] [CrossRef]
- Liu, M.; Takata, N.; Suzuki, A.; Kobashi, M. Development of gradient microstructure in the lattice structure of AlSi10Mg alloy fabricated by selective laser melting. J. Mater. Sci. Technol. 2020, 36, 106–117. [Google Scholar] [CrossRef]
- Yu, T.; Hyer, H.; Sohn, Y.; Bai, Y.; Wu, D. Structure-property relationship in high strength and lightweight AlSi10Mg microlattices fabricated by selective laser melting. Mater. Des. 2019, 182, 108062. [Google Scholar] [CrossRef]
- Caravaggi, P.; Liverani, E.; Leardini, A.; Fortunato, A.; Belvedere, C.; Baruffaldi, F.; Fini, M.; Parrilli, A.; Mattioli-Belmonte, M.; Tomesani, L.; et al. CoCr porous scaffolds manufactured via selective laser melting in orthopedics: Topographical, mechanical, and biological characterization. J. Biomed. Mater. Res. Part B 2019, 107, 2343–2353. [Google Scholar] [CrossRef]
- Xiao, Z.; Yang, Y.; Xiao, R.; Bai, Y.; Song, C.; Wang, D. Evaluation of topology-optimized lattice structures manufactured via selective laser melting. Mater. Des. 2018, 143, 27–37. [Google Scholar] [CrossRef]
- Zhong, T.; He, K.; Li, H.; Yang, L. Mechanical properties of lightweight 316L stainless steel lattice structures fabricated by selective laser melting. Mater. Des. 2019, 181, 108076. [Google Scholar] [CrossRef]
- Schneider, J.; Kumar, S. Multiscale characterization and constitutive parameters identification of polyamide (PA12) processed via selective laser sintering. Polym. Test. 2020, 86, 106357. [Google Scholar] [CrossRef]
- Xin, J.; Xi, L.G.; Meng, Z. Optimal design of three-dimensional non-uniform nylon lattice structures for selective laser sintering manufacturing. Adv. Mech. Eng. 2018, 10. [Google Scholar] [CrossRef]
- Fina, F.; Goyanes, A.; Madla, C.M.; Awad, A.; Trenfield, S.J.; Kuek, J.M.; Patel, P.; Gaisford, S.; Basit, A.W. 3D printing of drug-loaded gyroid lattices using selective laser sintering. Int. J. Pharm. 2018, 547, 44–52. [Google Scholar] [CrossRef] [PubMed]
- Xue, J.; Gao, L.; Hu, X.; Cao, K.; Zhou, W.; Wang, W.; Lu, Y. Stereolithographic 3D Printing-Based Hierarchically Cellular Lattices for High-Performance Quasi-Solid Supercapacitor. Nano-Micro Lett. 2019, 11. [Google Scholar] [CrossRef]
- Yang, W.; An, J.; Chua, C.K.; Zhou, K. Acoustic absorptions of multifunctional polymeric cellular structures based on triply periodic minimal surfaces fabricated by stereolithography. Virtual Phys. Prototyp. 2020, 15, 242–249. [Google Scholar] [CrossRef]
- Dar, U.A.; Mian, H.H.; Abid, M.; Topa, A.; Sheikh, M.Z.; Bilal, M. Experimental and numerical investigation of compressive behavior of lattice structures manufactured through projection micro stereolithography. Mater. Today Commun. 2020. [Google Scholar] [CrossRef]
- Dong, G.; Wijaya, G.; Tang, Y.; Zhao, Y.F. Optimizing process parameters of fused deposition modeling by Taguchi method for the fabrication of lattice structures. Addit. Manuf. 2018, 19, 62–72. [Google Scholar] [CrossRef]
- Tao, Y.; Pan, L.; Liu, D.; Li, P. A Case Study: Mechanical Modeling Optimization of Cellular Structure Fabricated Using Wood Flour-Filled Polylactic Acid Composites with Fused Deposition Modeling. Compos. Struct. 2019, 216, 360–365. [Google Scholar] [CrossRef]
- Khan, S.Z.; Masood, S.H.; Ibrahim, E.; Ahmad, Z. Compressive behaviour of Neovius Triply Periodic Minimal Surface cellular structure manufactured by fused deposition modelling. Virtual Phys. Prototyp. 2019, 14, 360–370. [Google Scholar] [CrossRef]
- Akihiro, T.; Kazuo, Y.; Yuichiro, K.; Zhang, X.P.; Mitsuru, K. Isotropic Ti–6Al–4V Lattice via Topology Optimization and Electron-Beam Melting. Addit. Manuf. 2018, 22, 629–642. [Google Scholar]
- Ataee, A.; Li, Y.; Fraser, D.; Song, G.F.; Wen, C. Anisotropic Ti-6Al-4V gyroid scaffolds manufactured by electron beam melting (EBM) for bone implant applications. Mater. Des. 2018, 137, 45–354. [Google Scholar] [CrossRef]
- Yang, K.; Wang, J.; Jia, L.; Yang, G.Y.; Tang, H.P.; Li, Y.Y. Additive manufacturing of Ti-6Al-4V lattice structures with high structural integrity under large compressive deformation. J. Mater. Sci. Technol. 2018, 35, 305–308. [Google Scholar] [CrossRef]
- Hyun, S.; Karlsson, A.M.; Torquato, S.; Evans, A.G. Simulated properties of Kagomé and tetragonal truss core panels. Int. J. Solids Struct. 2003, 40, 6989–6998. [Google Scholar] [CrossRef]
- Brooks, W.; Sutcliffe, C.; Cantwell, W.; Fox, P.; Todd, J. Rapid Design and Manufacture of Ultralight Cellular Materials. In Proceedings of the Solid Freeform Fabrication Symposium, Austin, TX, USA, 1–3 August 2005; pp. 231–241. [Google Scholar]
- Wettergreen, M.A.; Bucklen, B.S.; Starly, B.; Yuksel, E.; Sun, W.; Liebschner, M.A.K. Creation of a unit block library of architectures for use in assembled scaffold engineering. Cad Comput. Aided Des. 2005, 37, 1141–1149. [Google Scholar] [CrossRef]
- Chen, Y. 3D Texture Mapping for Rapid Manufacturing. Comput. Aided Des. Appl. 2007, 4, 761–771. [Google Scholar] [CrossRef]
- Murr, L.E.; Gaytan, S.M.; Medina, F.; Lopez, H.; Martinez, E.; Machado, B.I.; Hermandz, D.H.; Martinez, L.; Lopez, M.I.; Wicken, R.B.; et al. Next-generation biomedical implants using additive manufacturing of complex, cellular and functional mesh arrays. Philos. Trans. 2010, 368, 1999–2032. [Google Scholar] [CrossRef]
- Pettermann, H.E.; Hüsing, J. Modeling and simulation of relaxation in viscoelastic open cell materials and structures. Int. J. Solids Struct. 2012, 49, 2848–2853. [Google Scholar] [CrossRef]
- Hammetter, C.I.; Rinaldi, R.G.; Zok, F.W. Pyramidal lattice structures for high strength and energy absorption. J. Appl. Mech. Trans. Asme 2013, 80, 041015. [Google Scholar] [CrossRef]
- Gurtner, G.; Durand, M. Stiffest elastic networks. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014. [Google Scholar] [CrossRef]
- Li, S.J.; Xu, Q.S.; Wang, Z.; Hou, W.T.; Hao, Y.L.; Yang, R.; Murr, L.E. Influence of cell shape on mechanical properties of Ti-6Al-4V meshes fabricated by electron beam melting method. Acta Biomater. 2014, 10, 4537–4547. [Google Scholar] [CrossRef] [PubMed]
- Dong, L.; Deshpande, V.; Wadley, H. Mechanical response of Ti-6Al-4V octet-truss lattice structures. Int. J. Solids Struct. 2015, 60, 107–124. [Google Scholar] [CrossRef]
- Savio, G.; Meneghell, R.; Concheri, G. Optimization of lattice structures for Additive Manufacturing Technologies. In Proceedings of the International Joint Conference on Mechanics, Design Engineering & Advanced Manufacturing (JCM 2016), Catania, Italy, 14–16 September 2016; pp. 213–222. [Google Scholar]
- Beyer, C.; Figueroa, D. Design and Analysis of Lattice Structures for Additive Manufacturing. J. Manuf. Sci. Eng. Trans. Asme 2016, 138, 121014. [Google Scholar] [CrossRef]
- Lord, E.A.; Mackay, A.L. Periodic minimal surfaces of cubic symmetry. Curr. Sci. 2003, 85, 346–362. [Google Scholar]
- Yang, L.; Yan, C.; Han, C.J.; Chen, P.; Yang, S.F.; Shi, Y.S. Mechanical response of a triply periodic minimal surface cellular structures manufactured by selective laser melting. Int. J. Mech. Sci. 2018, 148, 149–157. [Google Scholar] [CrossRef]
- Maskery, I.; Sturm, L.; Aremu, A.O.; Panesar, A.; Williams, C.B.; Tuck, C.J.; Wildman, R.D.; Ashcroft, I.A.; Hague, R.J.M. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing. Polymer 2017, 152, 62–71. [Google Scholar] [CrossRef]
- Jung, Y.; Chu, K.T.; Torquato, S. A variational level set approach for surface area minimization of triply-periodic surfaces. J. Comput. Phys. 2007, 223, 711–730. [Google Scholar] [CrossRef][Green Version]
- Gandy, P.J.F.; Bardhan, S.; Mackay, A.L.; Klinowski, J. Nodal surface approximations to the P, G, D and I-WP triply periodic minimal surfaces. Chem. Phys. Lett. 2001, 336, 187–195. [Google Scholar] [CrossRef]
- Wang, Y. Periodic surface modeling for computer aided nano design. Cad Comput. Aided Des. 2007, 39, 179–189. [Google Scholar] [CrossRef]
- Kapfer, S.C.; Hyde, S.T.; Mecke, K.; Arns, C.H.; Schröder-Turk, G.E. Minimal surface scaffold designs for tissue engineering. Biomaterials 2011, 32, 6875–6882. [Google Scholar] [CrossRef] [PubMed]
- Hussein, A.; Hao, L.; Yan, C.; Everson, R.; Young, P. Advanced lattice support structures for metal additive manufacturing. J. Mater. Process. Technol. 2013, 213, 1019–1026. [Google Scholar] [CrossRef]
- Montazerian, H.; Davoodi, E.; Asadi-Eydivand, M.; Kadkhodapour, J.; Solati-Hashjin, M. Porous scaffold internal architecture design based on minimal surfaces: A compromise between permeability and elastic properties. Mater. Des. 2017, 126, 98–114. [Google Scholar] [CrossRef]
- Hao, L.; Raymont, D.; Yan, C.; Hussein, A.; Young, P. Design and additive manufacturing of cellular lattice structures. In Proceedings of the 5th International Conference on Advanced Research and Rapid Prototyping (VRAP), Leiria, Portugal, 28 September–1 October 2011; pp. 249–254. [Google Scholar]
- Abu Al-Rub, R.K.; Rowshan, R.; Abu Al-Rub, R.K. Topology-mechanical property relationship of 3D printed strut, skeletal, and sheet based periodic metallic cellular materials. Addit. Manuf. 2018, 19, 167–183. [Google Scholar]
- Savio, G.; Meneghello, R.; Concheri, G. Design of variable thickness triply periodic surfaces for additive manufacturing. Prog. Addit. Manuf. 2019, 4, 290–291. [Google Scholar] [CrossRef]
- Al-Ketan, O.; Abu Al-Rub, R.K. Multifunctional mechanical-metamaterials based on triply periodic minimal surface lattices: A review. Adv. Eng. Mater. 2019, 21, 1900524. [Google Scholar] [CrossRef]
- Kazemi, H.; Vaziri, A.; Julián, A. Norato. Multi-material topology optimization of lattice structures using geometry projection. Comput. Methods Appl. Mech. 2020, 363, 112895. [Google Scholar] [CrossRef]
- Clausen, A.; Wang, F.; Jensen, J.S.; Sigmund, O.; Lewis, J. Topology Optimized Architectures with Programmable Poisson’s Ratio over Large Deformations. Adv. Mater. 2015, 27, 5523–5527. [Google Scholar] [CrossRef]
- Rodrigues, H.C. Topology Optimization of Structures: Applications in the Simulation and Design of Cellular Materials. In Proceedings of the “Enhancement and Promotion of Computational Methods in Engineering and Science X”, Sanya, China, 21–23 August 2006; pp. 125–136. [Google Scholar]
- Wang, F.; Sigmund, O.; Jensen, J.S. Design of materials with prescribed nonlinear properties. J. Mech. Phys. Solids 2014, 69, 156–174. [Google Scholar] [CrossRef]
- Sigmund, O.; Trans, P.; Lond, R.S. Topology optimization: A tool for the tailoring of structures and materials Topology optimization: A tool for the tailoring of structures and materials. Philos. Trans. R. Soc. Lond. A 2000, 358, 211–227. [Google Scholar] [CrossRef]
- Fantini, M.; Curto, M.; De Crescenzio, F. A method to design biomimetic scaffolds for bone tissue engineering based on Voronoi lattices. Virtual Phys. Prototyp. 2016, 11, 77–90. [Google Scholar] [CrossRef]
- Gómez, S.; Vlad, M.D.; López, J.; Fernández, E. Design and properties of 3D scaffolds for bone tissue engineering. Acta Biomater. 2016, 42, 341–350. [Google Scholar] [CrossRef] [PubMed]
- Wang, G.; Shen, L.; Zhao, J.; Liang, H.; Wang, C. Design and Compressive Behavior of Controllable Irregular Porous Scaffolds: Based on Voronoi-Tessellation and for Additive Manufacturing. Acs Biomater. Sci. Eng. 2018, 4, 719–727. [Google Scholar] [CrossRef]
- Du, Y.; Liang, H.; Xie, D.; Mao, N.; Zhao, J.F.; Tian, Z.J.; Wang, C.J.; Shen, L.D. Design and statistical analysis of irregular porous scaffolds for orthopedic reconstruction based on voronoi tessellation and fabricated via selective laser melting (SLM). Mater. Chem. Phys. 2020, 239, 1–9. [Google Scholar] [CrossRef]
- Lu, L.; Sharf, A.; Zhao, H.S.; Wei, Y.; Fan, Q.N.; Chen, X.L.; Savoye, Y.; Tu, C.H.; CohenOr, D.; Chen, B.Q. Build-to-last: Strength to weight 3D printed objects. Acm Trans. Graph. 2014, 3, 1–10. [Google Scholar] [CrossRef]
- Ying, J.; Lu, L.; Tian, L.; Yan, X.; Chen, B.Q. Anisotropic porous structure modeling for 3D printed objects. Comput. Graph. 2018, 70, 157–164. [Google Scholar] [CrossRef]
- Sienkiewicz, J.A.; Płatek, P.; Jiang, F.; Sun, X.; Rusinek, A. Investigations on the Mechanical Response of Gradient Lattice Structures Manufactured via SLM. Metals 2020, 10, 213. [Google Scholar] [CrossRef]
- Nguyen, J.; Park, S.; Rosen, D. Heuristic optimization method for cellular structure design of light weight components. Int. J. Precis. Eng. Manuf. 2013, 14, 1071–1078. [Google Scholar] [CrossRef]
- Tang, Y.; Kurtz, A.; Zhao, Y.F. Bidirectional Evolutionary Structural Optimization (BESO) based design method for lattice structure to be fabricated by additive manufacturing. Cad Comput. Aided Des. 2015, 69, 91–101. [Google Scholar] [CrossRef]
- Salonitis, K.; Chantzis, D.; Kappatos, V. A hybrid finite element analysis and evolutionary computation method for the design of lightweight lattice components with optimized strut diameter. Int. J. Adv. Manuf. Technol. 2017, 90, 2689–2701. [Google Scholar] [CrossRef]
- Tang, Y.; Dong, G.; Zhou, Q.; Zhao, Y.F. Lattice Structure Design and Optimization with Additive Manufacturing Constraints. IEEE Trans. Autom. Sci. Eng. 2018, 15, 1546–1562. [Google Scholar] [CrossRef]
- Pantz, O.; Trabelsi, K. A post-trement of the homogenization method for shape optimization. Siam J. Control Optim. 2008, 47, 1380–1398. [Google Scholar] [CrossRef]
- Pantz, O.; Trabelsi, K. Construction of minimization sequences for shape optimization. In Proceedings of the 15th International Conference on Methods and Models in Automation and Robotics (MMAR), Miedzyzdroje, Poland, 23–26 August 2010; pp. 278–283. [Google Scholar]
- Verners, O.; Dobelis, M. Shape Optimization of a Superelement of Hexagonal Lattice Structure. Constr. Sci. 2010, 11, 66–76. [Google Scholar]
- Reinhart, G.; Teufelhart, S. Load-adapted design of generative manufactured lattice structures. Phys. Procedia 2011, 12, 385–392. [Google Scholar] [CrossRef]
- Reinhart, G.; Teufelhart, S. Optimization of mechanical loaded lattice structures by orientating their struts along the flux of force. Procedia CIRP 2013, 12, 175–180. [Google Scholar] [CrossRef]
- Janushevskis, A.; Melnikovs, A.; Boyko, A. Shape optimization of 3D mechanical systems using metamodels. Adv. Mater. Res. 2013, 705, 429–435. [Google Scholar] [CrossRef]
- Koizumi, Y.; Okazaki, A.; Chiba, A.; Kato, T.; Takezawa, A. Cellular lattices of biomedical Co-Cr-Mo-alloy fabricated by electron beam melting with the aid of shape optimization. Addit. Manuf. 2016, 12, 305–313. [Google Scholar] [CrossRef]
- Zhang, L.; Feih, S.; Daynes, S.; Wang, Y.Q.; Michael, Y.; Wei, J.; Lu, W.F. Buckling optimization of Kagome lattice cores with free-form trusses. Mater. Des. 2018, 145, 144–155. [Google Scholar] [CrossRef]
- Wang, H.; Wu, M. Study on the Shape Optimization of Cable-Stiffened Single-Layer Latticed Shells. Int. J. Steel Struct. 2018, 18, 924–934. [Google Scholar] [CrossRef]
- Dapogny, C.; Estevez, R.; Faure, A.; Michailidis, G. Shape and topology optimization considering anisotropic features induced by additive manufacturing processes. Comput. Methods Appl. Mech. Eng. 2019, 344, 626–665. [Google Scholar] [CrossRef]
- Zhang, P.; Toman, J.; Yu, Y.Q.; Biyikli, E.; Tu, A.C. Efficient design-optimization of variable-density hexagonal cellular structure by additive manufacturing: Theory and validation. J. Manuf. Sci. Eng. Trans. Asme. 2015, 137, 41–45. [Google Scholar] [CrossRef]
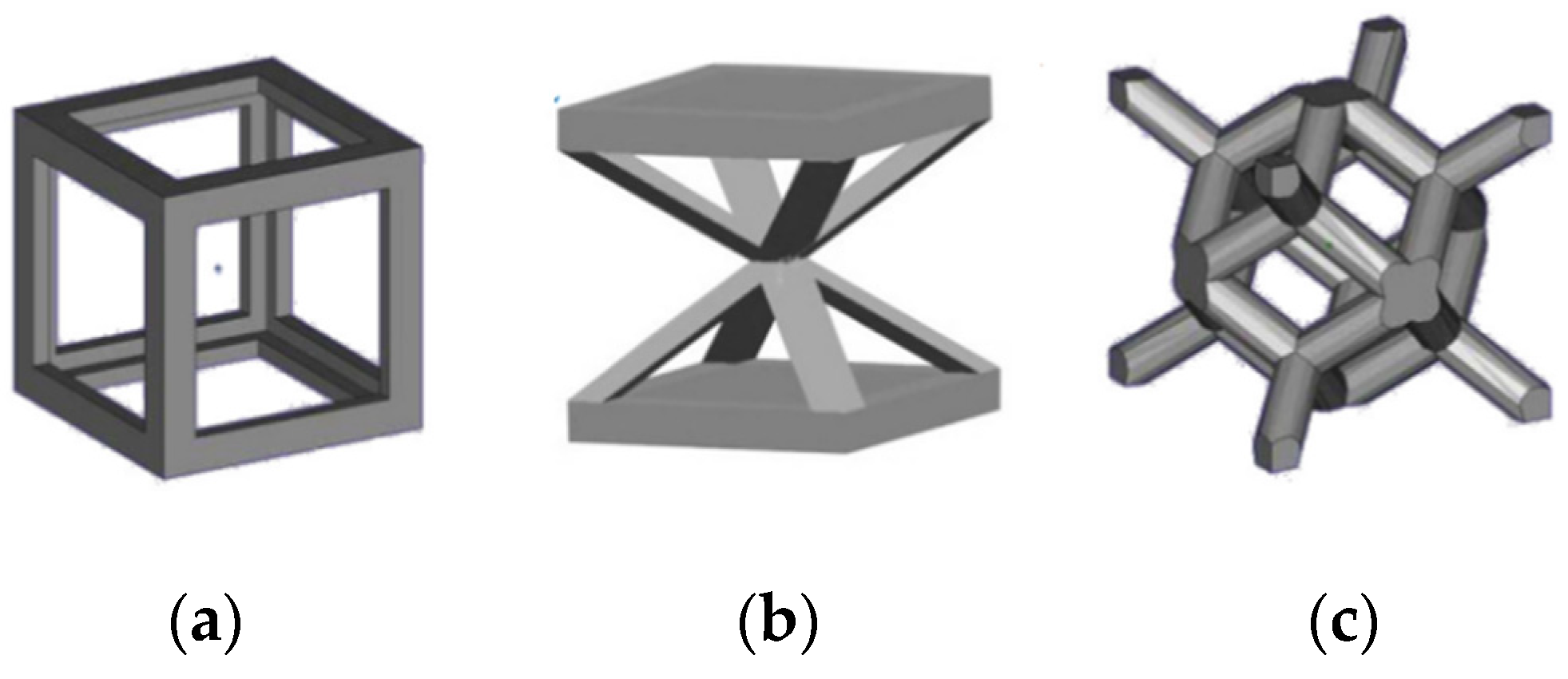
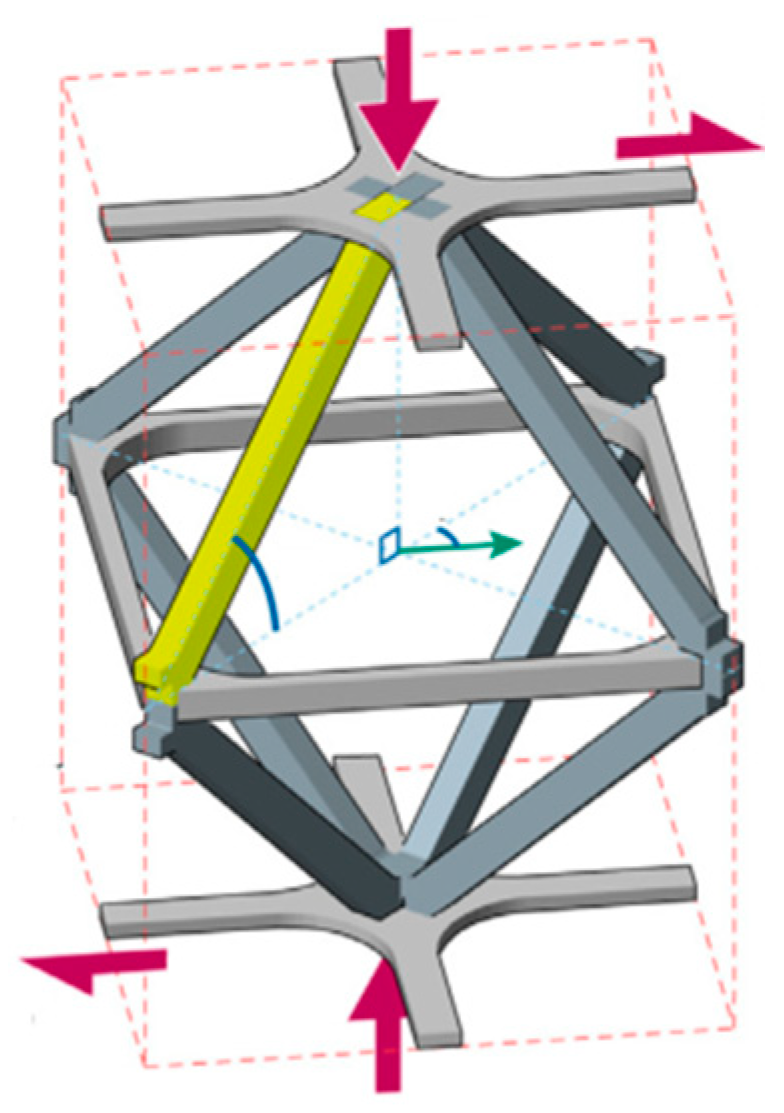
- Leonardi, F.; Graziosi, S.; Casati, R.; Tamburrino, F.; Bordegoni, M. Additive Manufacturing of Heterogeneous Lattice Structures: An Experimental Exploration. In Proceedings of the 22nd International Conference on Engineering Design (ICED19), Delft, The Netherlands, 5–8 August 2019; pp. 669–678. [Google Scholar]
- Vyatskikh, A.; Delalande, S.; Kudo, A.; Zhang, X.; Greer, J.R. Additive manufacturing of 3D nano-architected metals. Nat. Commun. 2018, 9, 3864–3870. [Google Scholar] [CrossRef] [PubMed]
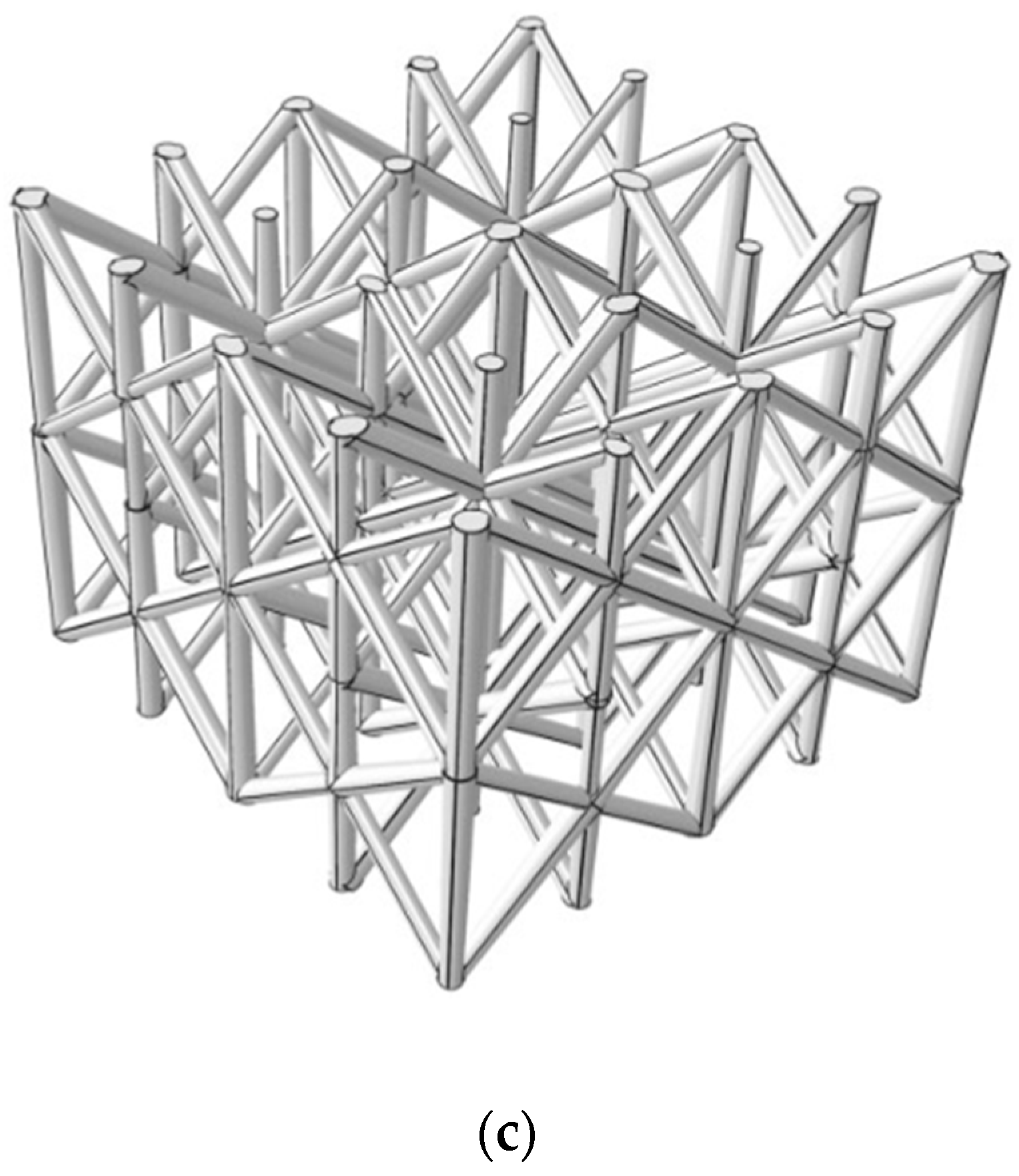
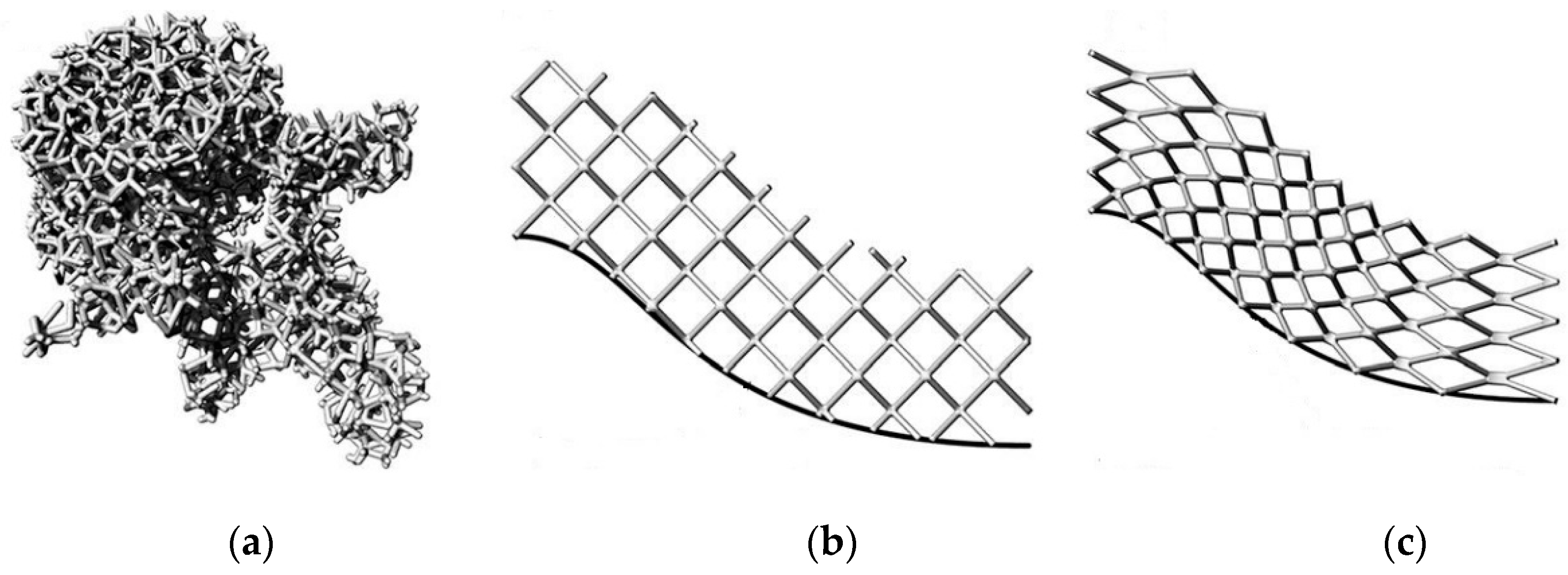
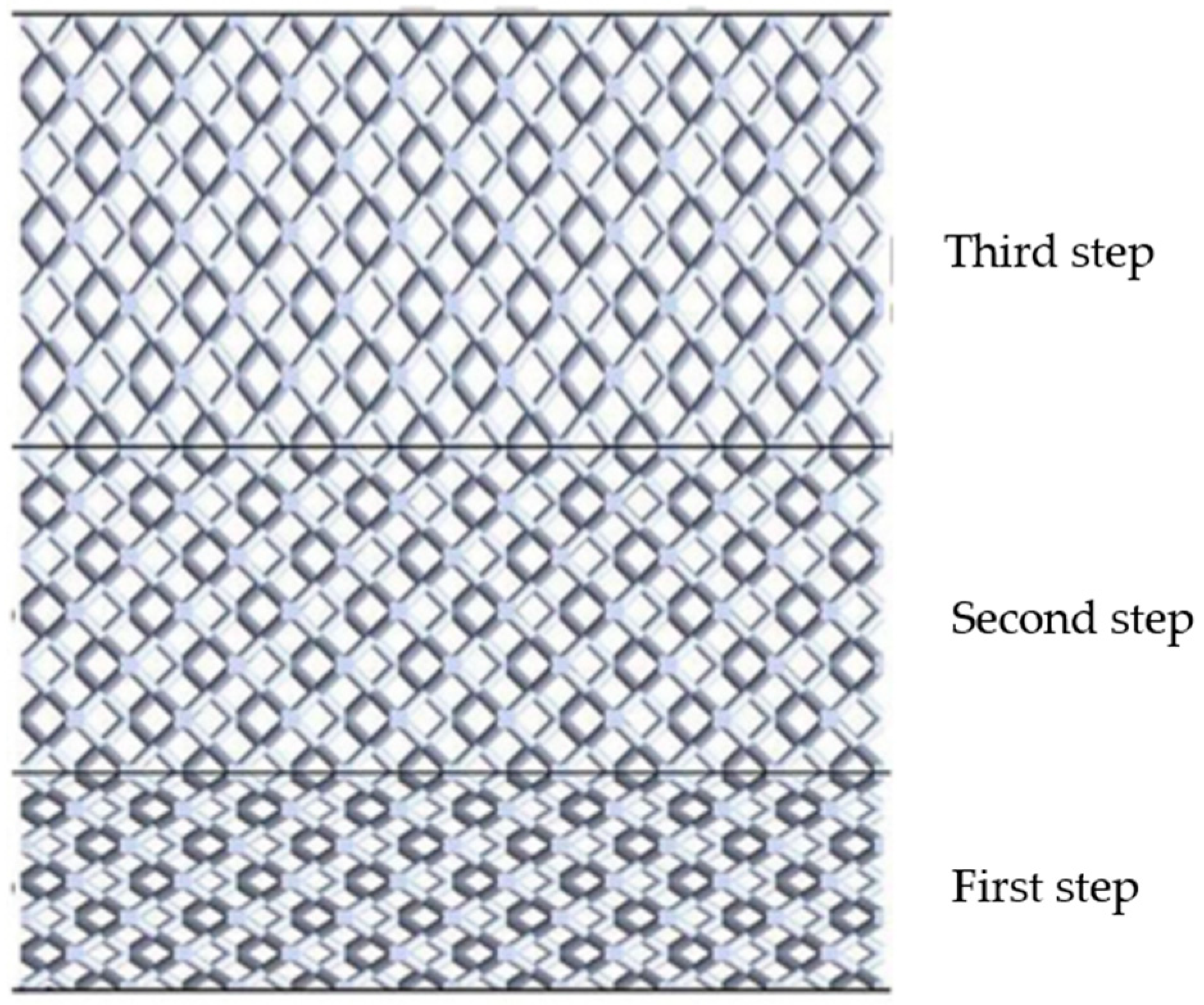


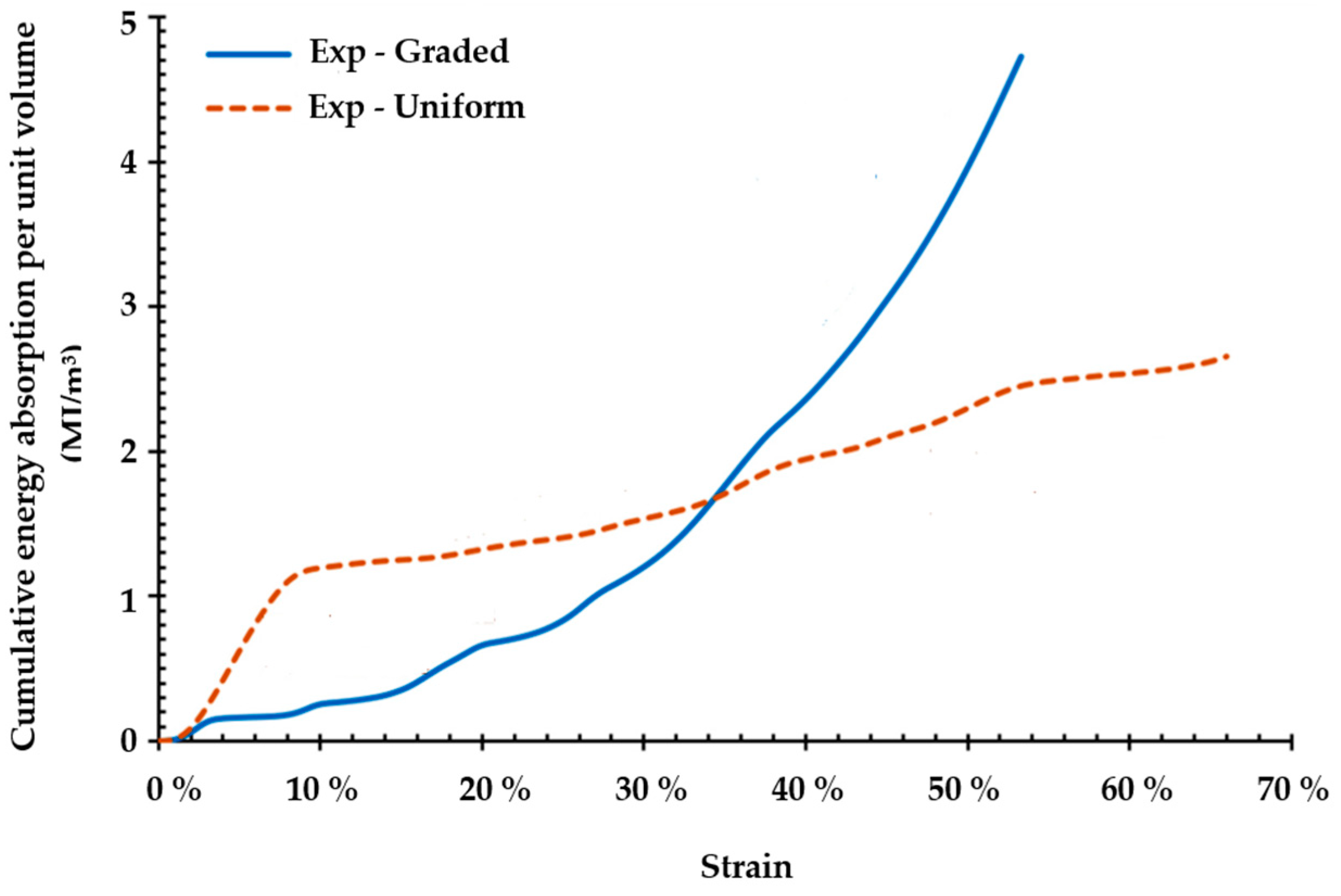






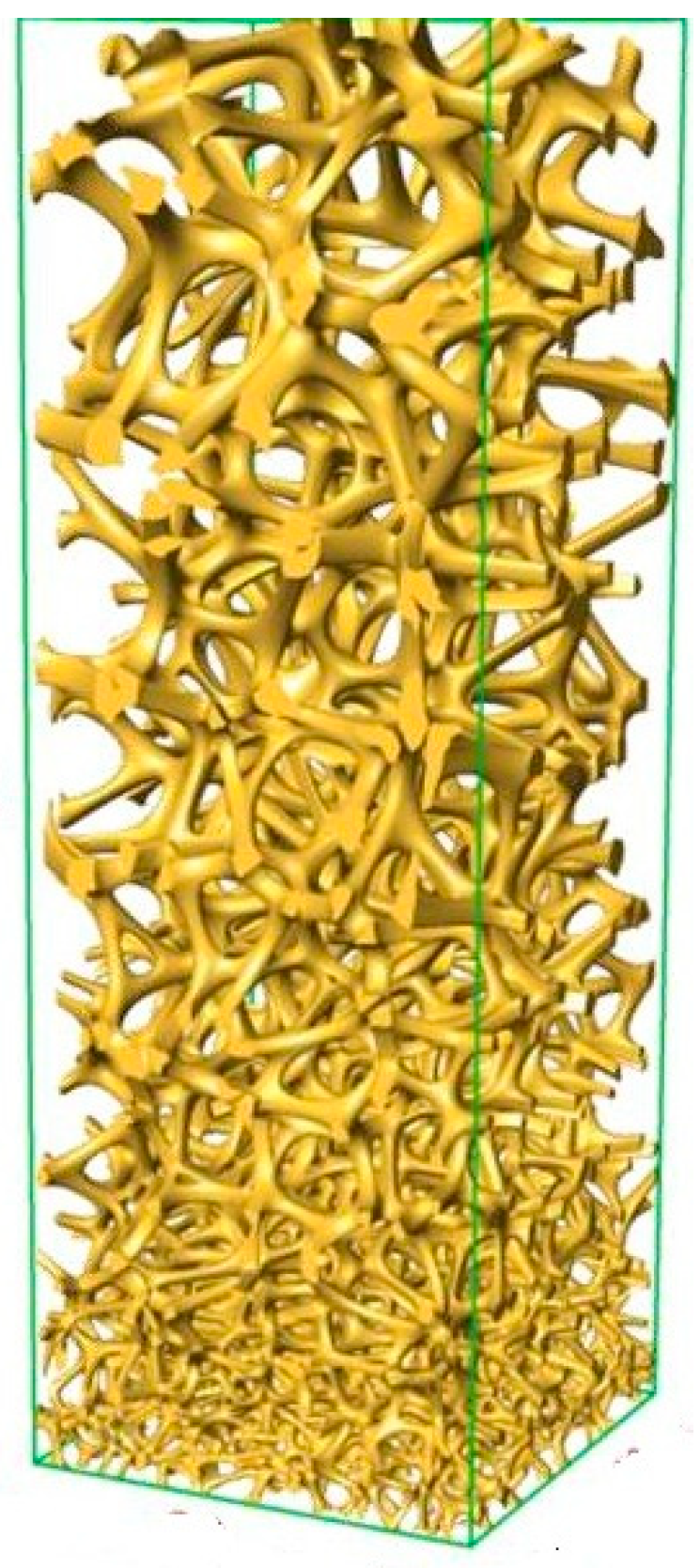
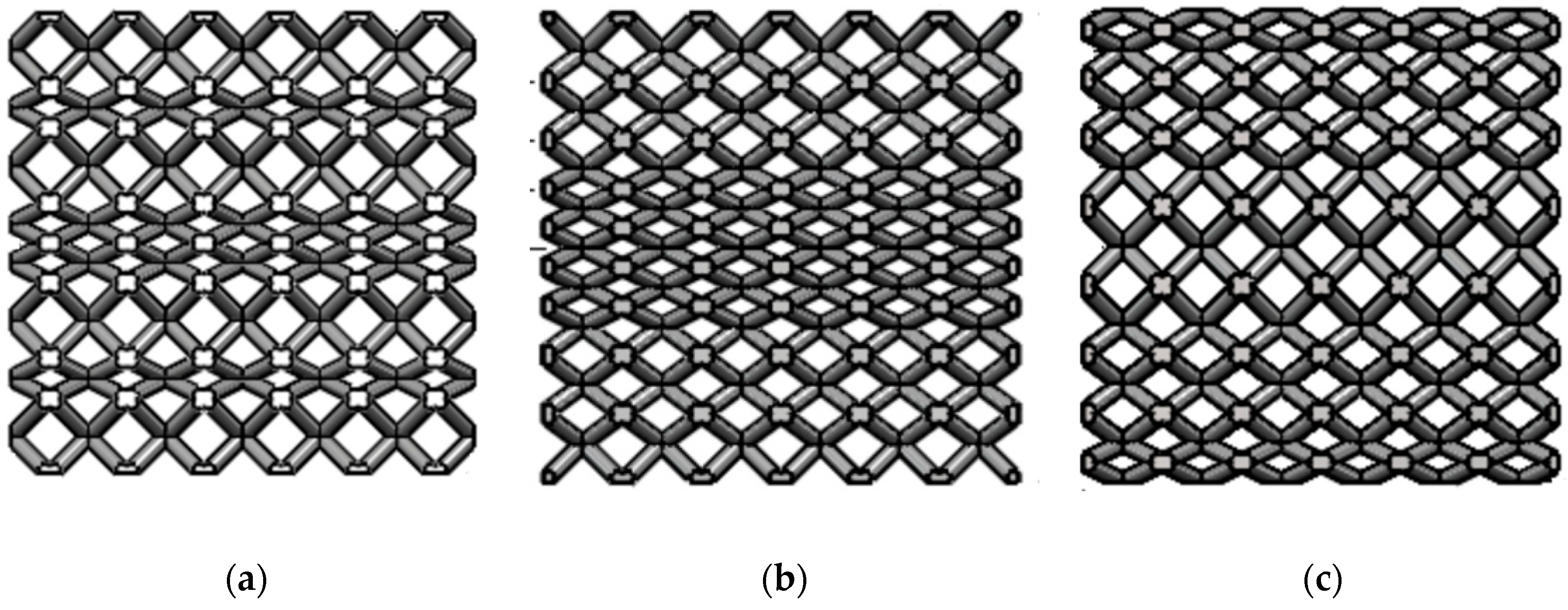
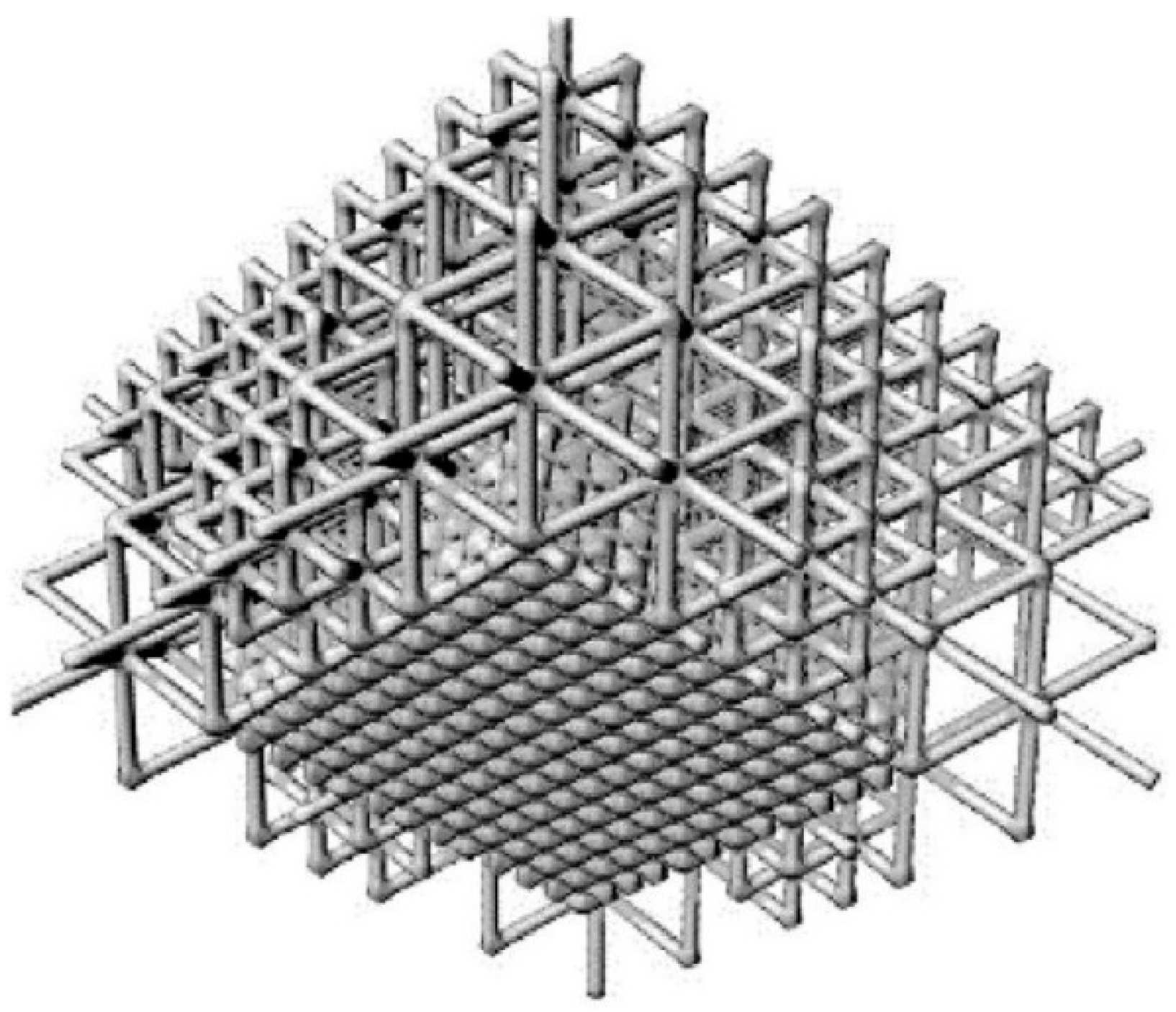
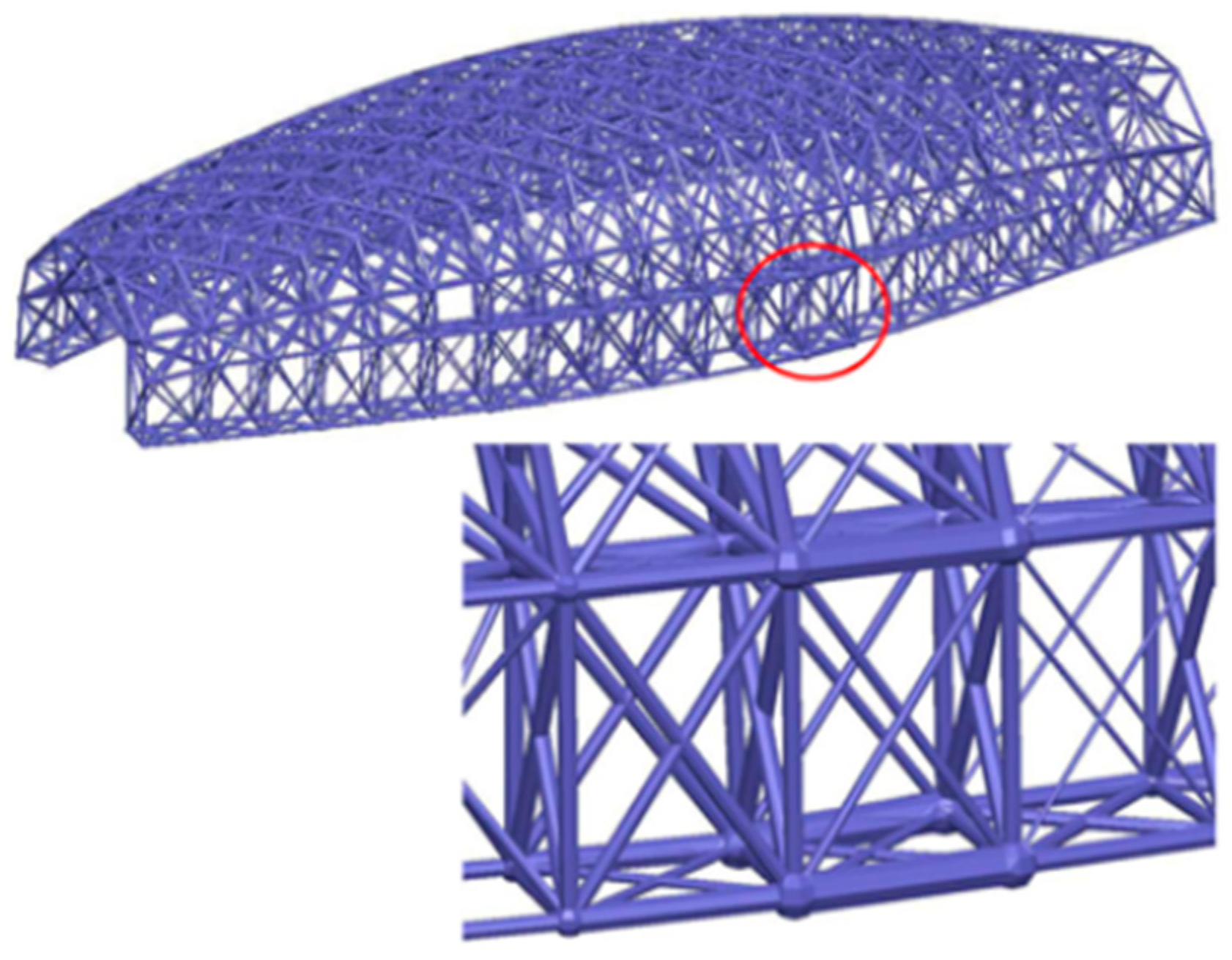
| Type of Cellular Structures | Applications |
|---|---|
| Foams | Energy absorbers [1,2,8,9], Filters [9,29], Silencers [9], Flame arresters [9], Heaters and heat exchangers [9,29], Eelectro-chemical devices [9,29]. |
| Honeycombs | Energy absorbers [1,2,15,30,31,32,33], Biomedical implants [30,31,32], Filters [34,35], Sensors [34,35], Actuators [34,35], Vibration absorber or damper [36,37,38,39]. |
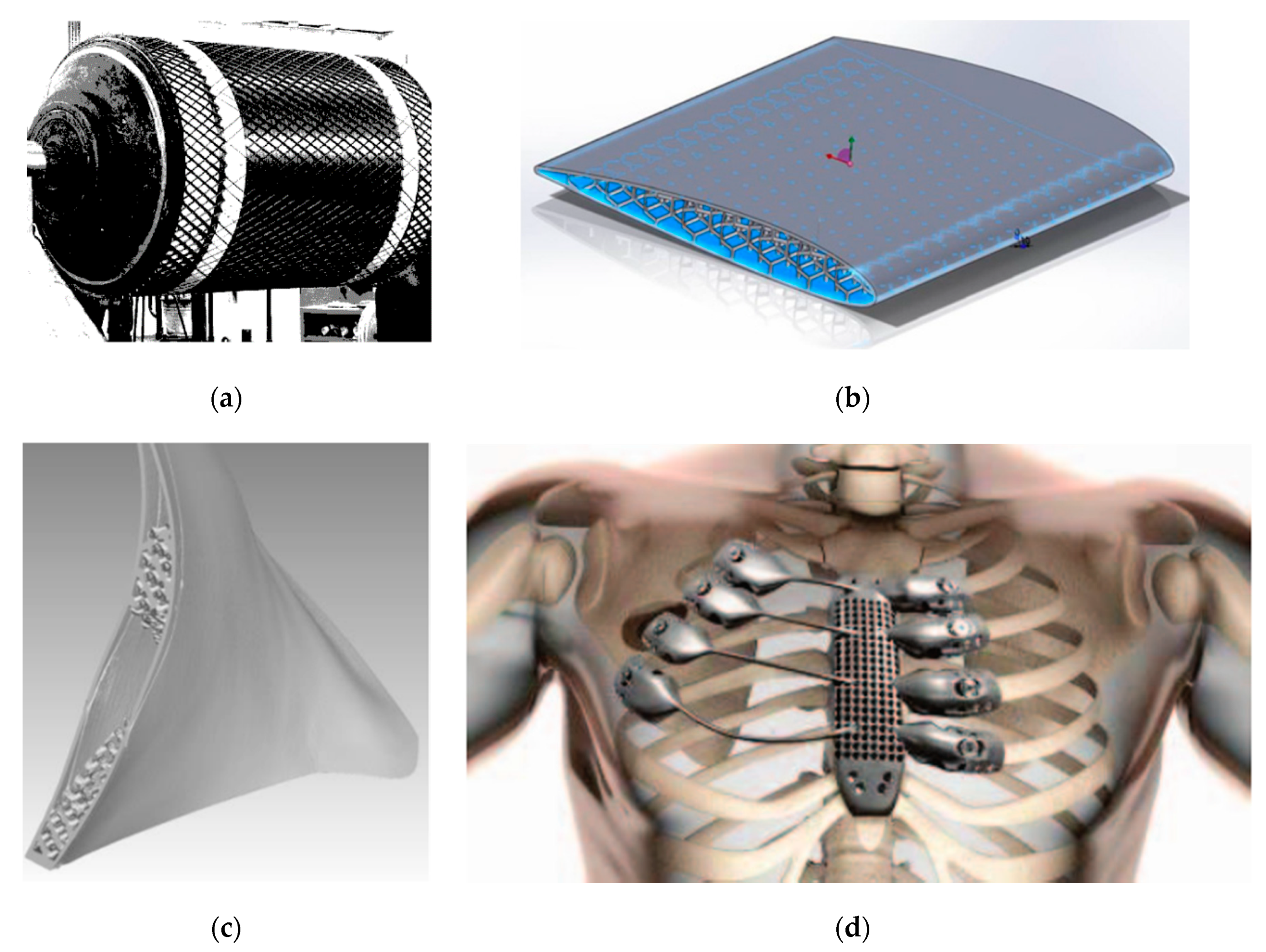
| Lattices | Energy absorbers [1,2,40,41,42], Heaters and heat exchangers [23,27,43,44,45,46], Engine hood [47], Biomedical implants [48,49,50,51,52,53,54,55], Wings [56], Gas turbine engine fan blades [57], Vibration absorber [58,59,60,61], Robotic system [62], Spacecraft and aircraft structures (i.e., fuselage, rib) [63,64,65]. |
| AM Process | Material | Lattice Structures | Reference |
|---|---|---|---|
| SLM | Ti-6Al-4V |  | [130] |
 | [131] | ||
 | [132] | ||
 | [133] | ||
 | [134] | ||
 | [135] | ||
| AlSi10Mg |  | [136] | |
 | [137] | ||
  | [138] | ||
| CoCr alloy |   | [139] | |
| 316L stainless steel |    | [140] | |
  | [141] | ||
| SLS | Polyamide powder |  | [142] |
| Nylon |  | [143] | |
| Paracetamol |  | [144] | |
| SLA | Polymer |  | [145] |
   | [146] | ||
 | [147] | ||
| FDM | Acrylonitrile Butadiene Styrene (ABS) |  | [148] |
  | [149] | ||
| PLA filaments |    | [150] | |
| EBM | Ti-6Al-4V |   | [151] |
 | [152] | ||
 | [153] |
| Condition | Interpretation |
|---|---|
| f(x, y, z) = 0 | On surface |
| f(x, y, z) < 0 | Inside |
| f(x, y, z) > 0 | Outside |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, C.; Han, Y.; Lu, J. Design and Optimization of Lattice Structures: A Review. Appl. Sci. 2020, 10, 6374. https://doi.org/10.3390/app10186374
Pan C, Han Y, Lu J. Design and Optimization of Lattice Structures: A Review. Applied Sciences. 2020; 10(18):6374. https://doi.org/10.3390/app10186374
Chicago/Turabian StylePan, Chen, Yafeng Han, and Jiping Lu. 2020. "Design and Optimization of Lattice Structures: A Review" Applied Sciences 10, no. 18: 6374. https://doi.org/10.3390/app10186374
APA StylePan, C., Han, Y., & Lu, J. (2020). Design and Optimization of Lattice Structures: A Review. Applied Sciences, 10(18), 6374. https://doi.org/10.3390/app10186374