Rocking Blocks Stability under Critical Pulses from Near-Fault Earthquakes Using a Novel Energy Based Approach
Abstract
Featured Application
Abstract
1. Introduction
1.1. Theoretical and Numerical Investigations of Rocking Blocks Stability
1.2. Experimental and Empirical Investigations of Rocking Blocks Stability
1.3. Representation of the Dominant Pulse of Near-Fault Earthquakes
1.4. Archaeoseimological Relevance
1.5. The Need for Efficient Scalar Intensity Measures
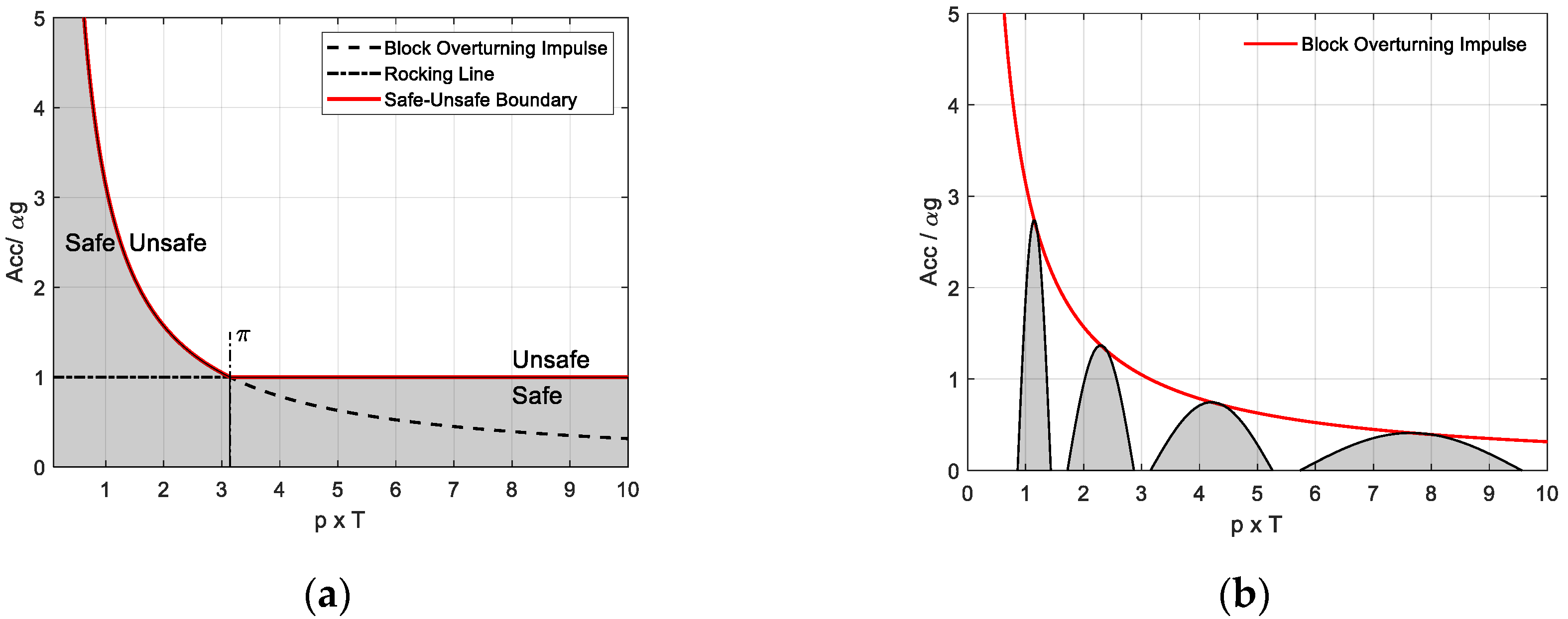
2. Theoretical Model
3. Numerical Verification
- (a)
- For periods greater roughly than 0.4 s for the 0.5 m block and 0.6 s for the 1 m block, the base and the top of the block move together. Inception of rocking is indicated by a minor lag between the two displacements followed by rapid increase over one or two impacts leading to overturning.
- (b)
- For periods smaller than 0.4 s for the 0.5 m block and 0.6 s for the 1 m block, multiple rocking takes place followed by overturning. The rocking response exhibits an apparent period that is different from the period of the applied excitation with an increase in the magnitude of rocking, akin to resonance, which leads to overturning after a few cycles.
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Housner, G.W. The Behavior of Inverted Pendulum Structures during Earthquakes. Bull. Seismol. Soc. Am. 1963, 53, 403–417. [Google Scholar]
- Mallet, R. Great Neapolitan Earthquake of 1857: The First Principles of Observational Seismology as Developed in the Report to the Royal Society of London of the Expedition Made by Command of the Society Into the Interior of the Kingdom of Naples, to Investigate the Circumstances of the Great Earthquake of December 1857; Chapman and Hall: London, UK, 1862; Volume 2. [Google Scholar]
- Milne, J.; Omori, F. On the overturning and fracturing of Brick and other Columns by horizontally applied Motion. Seismol. J. Jpn. 1893, 17, 59–86. [Google Scholar]
- Kirkpatrick, P. Seismic measurements by the overthrow of columns. Bull. Seismol. Soc. Am. 1927, 17, 95–109. [Google Scholar]
- Yim, C.S.; Chopra, A.K.; Penzien, J. Rocking response of rigid blocks to earthquakes. Earthq. Eng. Struct. Dyn. 1980, 8, 565–587. [Google Scholar] [CrossRef]
- Yim, S.C.; Lin, H. Nonlinear impact and chaotic response of slender rocking objects. J. Eng. Mech. 1991, 117, 2079–2100. [Google Scholar] [CrossRef]
- Ishiyama, Y. Motions of rigid bodies and criteria for overturning by earthquake excitations. Earthq. Eng. Struct. Dyn. 1982, 10, 635–650. [Google Scholar] [CrossRef]
- Spanos, P.D.; Koh, A.S. Rocking of rigid blocks due to harmonic shaking. J. Eng. Mech. 1984, 110, 1627–1642. [Google Scholar] [CrossRef]
- Tso, W.K.; Wong, C.M. Steady state rocking response of rigid blocks part 1: Analysis. Earthq. Eng. Struct. Dyn. 1989, 18, 89–106. [Google Scholar] [CrossRef]
- Wong, C.M.; Tso, W.K. Steady state rocking response of rigid blocks Part 2: Experiment. Earthq. Eng. Struct. Dyn. 1989, 18, 107–120. [Google Scholar] [CrossRef]
- Hogan, S.J. On the dynamics of rigid-block motion under harmonic forcing. Proc. R. Soc. Lond. A Math. Phys. Sci. 1989, 425, 441–476. [Google Scholar]
- Shenton, H.W., III; Jones, N.P. Base excitation of rigid bodies. I: Formulation. J. Eng. Mech. 1991, 117, 2286–2306. [Google Scholar] [CrossRef]
- Shenton, H.W., III; Jones, N.P. Base excitation of rigid bodies. II: Periodic slide-rock response. J. Eng. Mech. 1991, 117, 2307–2328. [Google Scholar] [CrossRef]
- Shenton, H.W., III. Criteria for initiation of slide, rock, and slide-rock rigid-body modes. J. Eng. Mech. 1996, 122, 690–693. [Google Scholar] [CrossRef]
- Shi, B.; Anooshehpoor, A.; Zeng, Y.; Brune, J.N. Rocking and overturning of precariously balanced rocks by earthquakes. Bull. Seismol. Soc. Am. 1996, 86, 1364–1371. [Google Scholar]
- Makris, N.; Roussos, Y. Rocking Response of Rigid Blocks under Near-Source Ground Motions. Géotechnique 2000, 50, 243–262. [Google Scholar] [CrossRef]
- Zhang, J.; Makris, N. Rocking response of free-standing blocks under cycloidal pulses. J. Eng. Mech. 2001, 127, 473–483. [Google Scholar] [CrossRef]
- Dimitrakopoulos, E.G.; DeJong, M.J. Revisiting the rocking block: Closed-form solutions and similarity laws. Proc. R. Soc. A Math. Phys. Eng. Sci. 2012, 468, 2294–2318. [Google Scholar] [CrossRef]
- Voyagaki, E.; Psycharis, I.; Mylonakis, G. Rocking Response and Overturning Criteria for Free Standing Blocks to Single-Lobe Pulses. Soil Dyn. Earthq. Eng. 2013, 46, 85–95. [Google Scholar] [CrossRef]
- Dimitrakopoulos, E.G.; Fung, E.D.W. Closed-form rocking overturning conditions for a family of pulse ground motions. Proc. R. Soc. A Math. Phys. Eng. Sci. 2016, 472, 20160662. [Google Scholar] [CrossRef]
- Nabeshima, K.; Taniguchi, R.; Kojima, K.; Takewaki, I. Closed-form overturning limit of rigid block under critical near-fault ground motions. Front. Built Environ. 2016, 2, 9. [Google Scholar] [CrossRef]
- Ther, T.; Kollar, L. Overturning of rigid blocks for earthquake excitation. Bull. Earthq. Eng. 2018, 16, 1607–1631. [Google Scholar] [CrossRef]
- Giouvanidis, A.I.; Dimitrakopoulos, E.G. Rocking amplification and strong-motion duration. Earthq. Eng. Struct. Dyn. 2018, 47, 2094–2116. [Google Scholar] [CrossRef]
- Aslam, M.; Godden, W.G.; Scalise, D.T. Earthquake rocking response of rigid bodies. J. Struct. Div. ASCE 1980, 106, 377–392. [Google Scholar]
- Lipscombe, P.R.; Pellegrino, S. Free rocking of prismatic blocks. J. Eng. Mech. 1993, 119, 1387–1410. [Google Scholar] [CrossRef]
- Manos, G.C.; Demosthenous, M. Dynamic response of rigid bodies subjected to horizontal base motion. In Proceedings of the 10th WCEE, Madrid, Spain, 19–24 July 1992; pp. 2817–2821. [Google Scholar]
- Prieto, F.; Lourenço, P.B.; Oliveira, C.S. Impulsive Dirac-delta forces in the rocking motion. Earthq. Eng. Struct. Dyn. 2004, 33, 839–857. [Google Scholar] [CrossRef]
- Prieto, F.; Lourenço, P.B. On the rocking behavior of rigid objects. Meccanica 2005, 40, 121–133. [Google Scholar] [CrossRef][Green Version]
- Peña, F.; Prieto, F.; Lourenço, P.B.; Campos Costa, A.; Lemos, J.V. On the dynamics of rocking motion of single rigid-block structures. Earthq. Eng. Struct. Dyn. 2007, 36, 2383–2399. [Google Scholar] [CrossRef]
- Peña, F.; Lourenço, P.B.; Campos-Costa, A. Experimental dynamic behavior of free-standing multi-block structures under seismic loadings. J. Earthq. Eng. 2008, 12, 953–979. [Google Scholar] [CrossRef]
- Purvance, M.D.; Anooshehpoor, A.; Brune, J.N. Freestanding block overturning fragilities: Numerical simulation and experimental validation. Earthq. Eng. Struct. Dyn. 2008, 37, 791–808. [Google Scholar] [CrossRef]
- Konstantinidis, D.; Makris, N. Experimental and analytical studies on the response of freestanding laboratory equipment to earthquake shaking. Earthq. Eng. Struct. Dyn. 2009, 38, 827–848. [Google Scholar] [CrossRef]
- Kafle, B.; Lam, N.T.; Gad, E.F.; Wilson, J. Displacement controlled rocking behaviour of rigid objects. Earthq. Eng. Struct. Dyn. 2011, 40, 1653–1669. [Google Scholar] [CrossRef]
- Baratta, A.; Corbi, I.; Corbi, O. Towards a seismic worst scenario approach for rocking systems: Analytical and experimental set-up for dynamic response. Acta Mech. 2013, 224, 691–705. [Google Scholar] [CrossRef]
- Saraswat, A.; Reddy, G.R.; Ghosh, A.K.; Ghosh, S. Effects of base excitation frequency on the stability of a freestanding rigid block. Acta Mech. 2016, 227, 795–812. [Google Scholar] [CrossRef]
- Gesualdo, A.; Iannuzzo, A.; Minutolo, V.; Monaco, M. Rocking of freestanding objects: Theoretical and experimental comparisons. J. Theor. Appl. Mech. 2018, 56, 977–991. [Google Scholar] [CrossRef]
- Arredondo, C.; Jaimes, M.A.; Reinoso, E. A Simplified Model to Evaluate the Dynamic Rocking Behavior of Irregular Free-Standing Rigid Bodies Calibrated with Experimental Shaking-Table Tests. J. Earthq. Eng. 2019, 23, 46–71. [Google Scholar] [CrossRef]
- Al Abadi, H.; Paton-Cole, V.; Gad, E.; Lam, N.; Patel, V. Rocking Behavior of Irregular Free-Standing Objects Subjected to Earthquake Motion. J. Earthq. Eng. 2019, 23, 793–809. [Google Scholar] [CrossRef]
- Di Sarno, L.; Magliulo, G.; D’Angela, D.; Cosenza, E. Experimental assessment of the seismic performance of hospital cabinets using shake table testing. Earthq. Eng. Struct. Dyn. 2019, 48, 103–123. [Google Scholar] [CrossRef]
- Reggiani Manzo, N.; Vassiliou, M.F. Displacement-based analysis and design of rocking structures. Earthq. Eng. Struct. Dyn. 2019, 48, 1613–1629. [Google Scholar] [CrossRef]
- Durukal, E. Critical evaluation of strong motion in Kocaeli and Düzce (Turkey) earthquakes. Soil Dyn. Earthq. Eng. 2002, 22, 589–609. [Google Scholar] [CrossRef]
- Godschalk, D.R. Urban hazard mitigation: Creating resilient cities. Nat. Hazards Rev. 2003, 4, 136–143. [Google Scholar] [CrossRef]
- Rashed, T.; Weeks, J. Assessing vulnerability to earthquake hazards through spatial multicriteria analysis of urban areas. Int. J. Geogr. Inform. Sci. 2003, 17, 547–576. [Google Scholar] [CrossRef]
- Godschalk, D.; Xu, C. Urban hazard mitigation: Creating resilient cities. Urban Plan. Int. 2015, 2, 22–29. [Google Scholar] [CrossRef]
- Hall, J.F.; Heaton, T.H.; Halling, M.W.; Wald, D.J. Near-source ground motion and its effects on flexible buildings. Earthq. Spec. 1995, 11, 569–605. [Google Scholar] [CrossRef]
- Somerville, P.G.; Smith, N.F.; Graves, R.W.; Abrahamson, N.A. Modification of empirical strong ground motion attenuation relations to include the amplitude and duration effects of rupture directivity. Seismol. Res. Lett. 1997, 68, 199–222. [Google Scholar] [CrossRef]
- Kalkan, E.; Kunnath, S.K. Effects of fling step and forward directivity on seismic response of buildings. Earthq. Spec. 2006, 22, 367–390. [Google Scholar] [CrossRef]
- Somerville, P.G. Magnitude scaling of the near fault rupture directivity pulse. Phys. Earth Planet. Int. 2003, 137, 201–212. [Google Scholar] [CrossRef]
- Somerville, P.G. Engineering characterization of near fault ground motions. In Proceedings of the NZSEE 2005 Conference, Wairakei, New Zealand, 11–13 March 2005. [Google Scholar]
- Chen, K.; Avouac, J.P.; Aati, S.; Milliner, C.; Zheng, F.; Shi, C. Cascading and pulse-like ruptures during the 2019 Ridgecrest earthquakes in the Eastern California Shear Zone. Nat. Commun. 2020, 11, 1–8. [Google Scholar] [CrossRef]
- Hough, S.E.; Thompson, E.; Parker, G.A.; Graves, R.W.; Hudnut, K.W.; Patton, J.; Blake, K. Near-field ground motions from the July 2019 Ridgecrest, California, earthquake sequence. Seismol. Res. Lett. 2020, 91, 1542–1555. [Google Scholar] [CrossRef]
- Sasani, M.; Bertero, V.V. Importance of Severe Pulse-Type Ground Motions in Performance-Based Engineering: Historical and Critical. In Proceedings of the 12th World Conference on Earthquake Engineering, New Zealand Society for Earthquake Engineering, Upper Hutt, New Zealand, 30 January–4 February 2000. [Google Scholar]
- Alavi, B.; Krawinkler, H. Behavior of moment-resisting frame structures subjected to near-fault ground motions. Earthq. Eng. Struct. Dyn. 2004, 33, 687–706. [Google Scholar] [CrossRef]
- Mollaioli, F.; Bruno, S.; Decanini, L.D.; Panza, G.F. Characterization of the dynamic response of structures to damaging pulse-type near-fault ground motions. Meccanica 2006, 41, 23–46. [Google Scholar] [CrossRef]
- Tong, M.; Rzhevsky, V.; Dai, J.; Lee, G.C.; Qi, J.; Qi, X. Near-fault ground motions with prominent acceleration pulses: Pulse characteristics and ductility demand. Earthq. Eng. Eng. Vib. 2007, 6, 215–223. [Google Scholar] [CrossRef]
- Xie, L.; Xu, L.; Adrian, R.M. Representation of near-fault pulse-type ground motions. Earthq. Eng. Eng. Vib. 2005, 4, 191–199. [Google Scholar] [CrossRef]
- Kojima, K.; Fujita, K.; Takewaki, I. Double and Triple Impulses for Capturing Critical Elastic-Plastic Response Properties and Robustness of Building Structures Under Near-Fault Ground Motions. In Resilient Structures and Infrastructure; Springer: Singapore, 2019; pp. 225–242. [Google Scholar]
- Arroyo, D.; Ordaz, M. Use of corrected sinusoidal pulses to estimate inelastic demands of elasto-perfectly plastic oscillators subjected to narrow-band motions. J. Earthq. Eng. 2007, 11, 303–325. [Google Scholar] [CrossRef]
- Zhang, Y.; Hu, Y.; Zhao, F.; Liang, J.; Yang, C. Identification of acceleration pulses in near-fault ground motion using the EMD method. Earthq. Eng. Eng. Vib. 2005, 4, 201–212. [Google Scholar] [CrossRef]
- Mavroeidis, G.P.; Papageorgiou, A.S. A mathematical representation of near-fault ground motions. Bull. Seismol. Soc. Am. 2003, 93, 1099–1131. [Google Scholar] [CrossRef]
- Baker, J.W. Quantitative classification of near-fault ground motions using wavelet analysis. Bull. Seismol. Soc. Am. 2007, 97, 1486–1501. [Google Scholar] [CrossRef]
- Shahi, S.K.; Baker, J.W. An efficient algorithm to identify strong-velocity pulses in multicomponent ground motions. Bull. Seismol. Soc. Am. 2014, 104, 2456–2466. [Google Scholar] [CrossRef]
- Makris, N.; Vassiliou, M.F. Sizing the slenderness of free-standing rocking columns to withstand earthquake shaking. Arch. Appl. Mech. 2012, 82, 1497–1511. [Google Scholar] [CrossRef]
- Yamamoto, M.; Sato, Y.; Inoue, S. Extraction method using sinusoidal waves to simplify earthquake ground motion. Jpn. Archit. Rev. 2018, 1, 322–330. [Google Scholar] [CrossRef]
- Makris, N.; Roussos, Y. Rocking Response and Overturning of Equipment under Horizontal Pulse-Type Motions; Pacific Earthquake Engineering Research Center: Berkeley, CA, USA, 1998. [Google Scholar]
- Francaviglia, V.; Augusti, G.; Sepe, V. Did Earthquakes fell Aksum obelisks? Ann. Geophys.-Italy 1995, 38, 983–1000. [Google Scholar]
- Erdik, M.; Durukal, E.; Ertürk, N.; Sungay, B. Earthquake risk mitigation in Istanbul museums. Nat. Hazards 2010, 53, 97–108. [Google Scholar] [CrossRef]
- Ambraseys, N.; Psycharis, I.N. Earthquake Stability of Columns and Statues. J. Earthq. Eng. 2011, 15, 685–710. [Google Scholar] [CrossRef]
- Galadini, F.; Hinzen, K.G.; Stiros, S. Archaeoseismology: Methodological issues and procedure. J. Seismol. 2006, 10, 395–414. [Google Scholar] [CrossRef]
- Sintubin, M. Archaeoseismology: Past, present and future. Quatern. Int. 2011, 242, 4–10. [Google Scholar] [CrossRef]
- Anooshehpoor, A.; Heaton, T.H.; Shi, B.; Brune, J.N. Estimates of the ground accelerations at Point Reyes Station during the 1906 San Francisco earthquake. Bull. Seismol. Soc. Am. 1999, 89, 845–853. [Google Scholar]
- Hinzen, K.G. Simulation of toppling columns in archaeoseismology. Bull. Seismol. Soc. Am. 2009, 99, 2855–2875. [Google Scholar] [CrossRef]
- De Silva, F.; Sica, S.; Silvestri, F.; Aversa, S. Estimation of the ground shaking from the response of rigid bodies. Ann. Geophy. 2016, 59. [Google Scholar] [CrossRef]
- Anooshehpoor, A.; Brune, J.N.; Zeng, Y. Methodology for obtaining constraints on ground motion from precariously balanced rocks. Bull. Seismol. Soc. Am. 2004, 94, 285–303. [Google Scholar] [CrossRef]
- Casapulla, C.; Giresini, L.; Lourenço, P.B. Rocking and kinematic approaches for rigid block analysis of masonry walls: State of the art and recent developments. Buildings 2017, 7, 69. [Google Scholar] [CrossRef]
- Tabbara, M.; Karam, G. Experimental, numerical and theoretical investigation of the rocking response of Baalbek columns under harmonic excitations. J. Earthq. Eng. 2019. [Google Scholar] [CrossRef]
- Drosos, V.; Anastasopoulos, I. Shaking Table Testing of Multidrum Columns and Portals. Earthq. Eng. Struct. Dyn. 2014, 43, 1703–1723. [Google Scholar] [CrossRef]
- Itasca Consulting Group Inc. UDEC-Universal Distinct Element Code, Version 6.0–User’s Guide; Itasca: Minneapolis, MN, USA, 2014. [Google Scholar]
- Tabbara, M.; Karam, G. Experimental Investigation of the Stability of Colonnades under Harmonic Excitation. In Proceedings of the 16th European Conference on Earthquake Engineering (16ECEE), Thessaloniki, Greece, 18–21 June 2018. [Google Scholar]
- Psycharis, I.; Papastamatiou, D.; Alexandris, A. Parametric Investigation of the Stability of Classical Columns under Harmonic and Earthquake Excitations. Earthq. Eng. Struct. Dyn. 2000, 29, 1093–1109. [Google Scholar] [CrossRef]
- Dimitri, R.; Lorenzis, L.; Zavarise, G. Numerical Study on the Dynamic Behavior of Masonry Columns and Arches on Buttresses with the Discrete Element Method. Eng. Struct. 2011, 33, 3172–3188. [Google Scholar] [CrossRef]
- Makris, N.; Kampas, G. Size versus slenderness: Two competing parameters in the seismic stability of free-standing rocking columns. Bull. Seismol. Soc. Am. 2016, 106, 104–122. [Google Scholar] [CrossRef]
- Ther, T.; Kollar, L. Refinement of Housner’s Model on Rocking Blocks. Bull. Earthq. Eng. 2017, 15, 2305–2319. [Google Scholar] [CrossRef]
- Tabbara, M.; Karam, G.; Jello, J.; Beaino, C. Numerical Investigation of the Wobbling Response of a Free Standing Multi-drum Column under Harmonic Excitations. Presented at the 9th Turkish Conference on Earthquake Engineering (9TCEE), Istanbul, Turkey, 1–3 June 2020. Postponed to June 2021 due to COVID19. [Google Scholar]
- Tabbara, M.; Karam, G.; Jello, J.; Beaino, C. Rocking, Wobbling and Overturning of the Multi-drum Columns of Baalbek under Periodic Pulses. J. Seismol. 2020. submitted. [Google Scholar]
- Ohnaka, M. Frictional Characteristics of Typical Rocks. J. Phys. Earth 1975, 23, 87–112. [Google Scholar] [CrossRef][Green Version]
- Foti, D.; Vacca, V. Rocking of Multiblock Stone Classical Columns. Earthq. Resist. Eng. Struct. 2017, 172, 1. [Google Scholar]
- Anderson, J.G.; Biasi, G.P.; Brune, J.N. Precarious rocks: Providing upper limits on past ground shaking from earthquakes. In Earthquake Hazard, Risk and Disasters; Shroder, J.F., Wyss, M., Eds.; Elsevier: Amsterdam, The Netherlands, 2014; pp. 377–403. [Google Scholar]
- Ther, T.; Kollár, L.P. Model for multiblock columns subjected to base excitation. Earthq. Eng. Struct. Dyn. 2018, 47, 418–437. [Google Scholar] [CrossRef]
- Kounadis, A.N. On the rocking–sliding instability of rigid blocks under ground excitation: Some new findings. Soil Dyn. Earthq. Eng. 2015, 75, 246–258. [Google Scholar] [CrossRef]
- Kavvadias, I.E.; Vasiliadis, L.K.; Elenas, A. Seismic response parametric study of ancient rocking columns. Int. J. Archit. Herit. 2017, 11, 791–804. [Google Scholar] [CrossRef]
- Pappas, A.; Sextos, A.; Da Porto, F.; Modena, C. Efficiency of alternative intensity measures for the seismic assessment of monolithic free-standing columns. Bull. Earthq. Eng. 2017, 15, 1635–1659. [Google Scholar] [CrossRef]
- DeJong, M.J. Seismic Assessment Strategies for Masonry Structures. Chapter 6: Analytical Modeling: Rocking Structures. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2009. [Google Scholar]





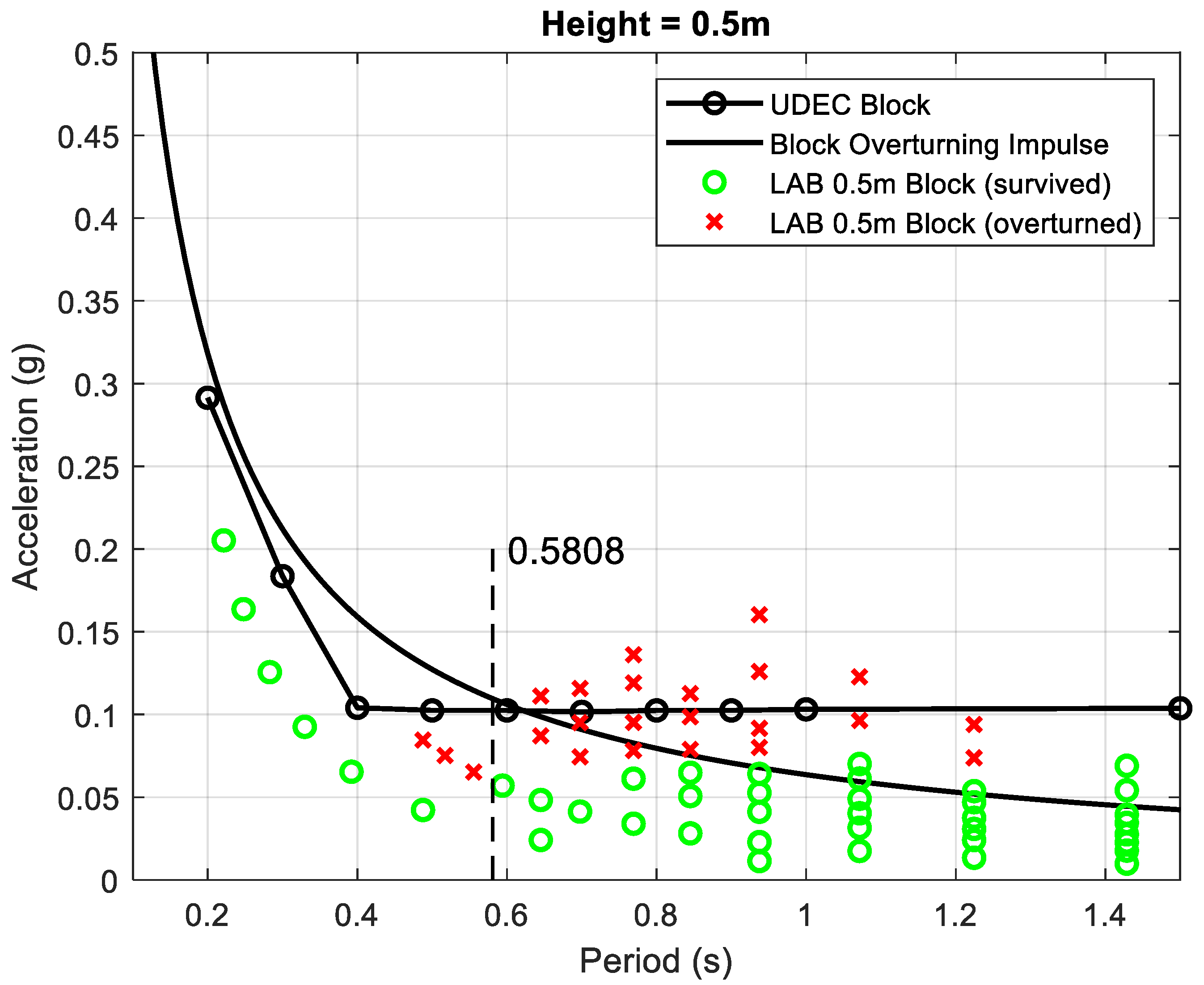



| T (s) | Velocity Amplitude (m/s) | Acceleration Amplitude (g) | Block, 0.5 m, 25 cycles, Displacement History (Base and Top) |
|---|---|---|---|
| 0.2 | 0.091 | 0.2914 |  |
| 0.3 | 0.086 | 0.1836 |  |
| 0.4 | 0.065 | 0.1041 |  |
| 0.6 | 0.096 | 0.1025 |  |
| 0.9 | 0.144 | 0.1025 |  |
| 1.5 | 0.243 | 0.1038 |  |
| T (s) | Velocity Amplitude (m/s) | Acceleration Amplitude (g) | Block, 1 m, 25 cycles, Displacement History (Base and Top) |
|---|---|---|---|
| 0.3 | 0.127 | 0.2711 |  |
| 0.4 | 0.116 | 0.1857 |  |
| 0.5 | 0.092 | 0.1178 |  |
| 0.6 | 0.097 | 0.1035 |  |
| 1.0 | 0.164 | 0.1050 |  |
| 1.5 | 0.248 | 0.1059 |  |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karam, G.; Tabbara, M. Rocking Blocks Stability under Critical Pulses from Near-Fault Earthquakes Using a Novel Energy Based Approach. Appl. Sci. 2020, 10, 5924. https://doi.org/10.3390/app10175924
Karam G, Tabbara M. Rocking Blocks Stability under Critical Pulses from Near-Fault Earthquakes Using a Novel Energy Based Approach. Applied Sciences. 2020; 10(17):5924. https://doi.org/10.3390/app10175924
Chicago/Turabian StyleKaram, Gebran, and Mazen Tabbara. 2020. "Rocking Blocks Stability under Critical Pulses from Near-Fault Earthquakes Using a Novel Energy Based Approach" Applied Sciences 10, no. 17: 5924. https://doi.org/10.3390/app10175924
APA StyleKaram, G., & Tabbara, M. (2020). Rocking Blocks Stability under Critical Pulses from Near-Fault Earthquakes Using a Novel Energy Based Approach. Applied Sciences, 10(17), 5924. https://doi.org/10.3390/app10175924





