New Indices for Evaluating Vibration Characteristics of Flexible-Joint Robots
Abstract
1. Introduction
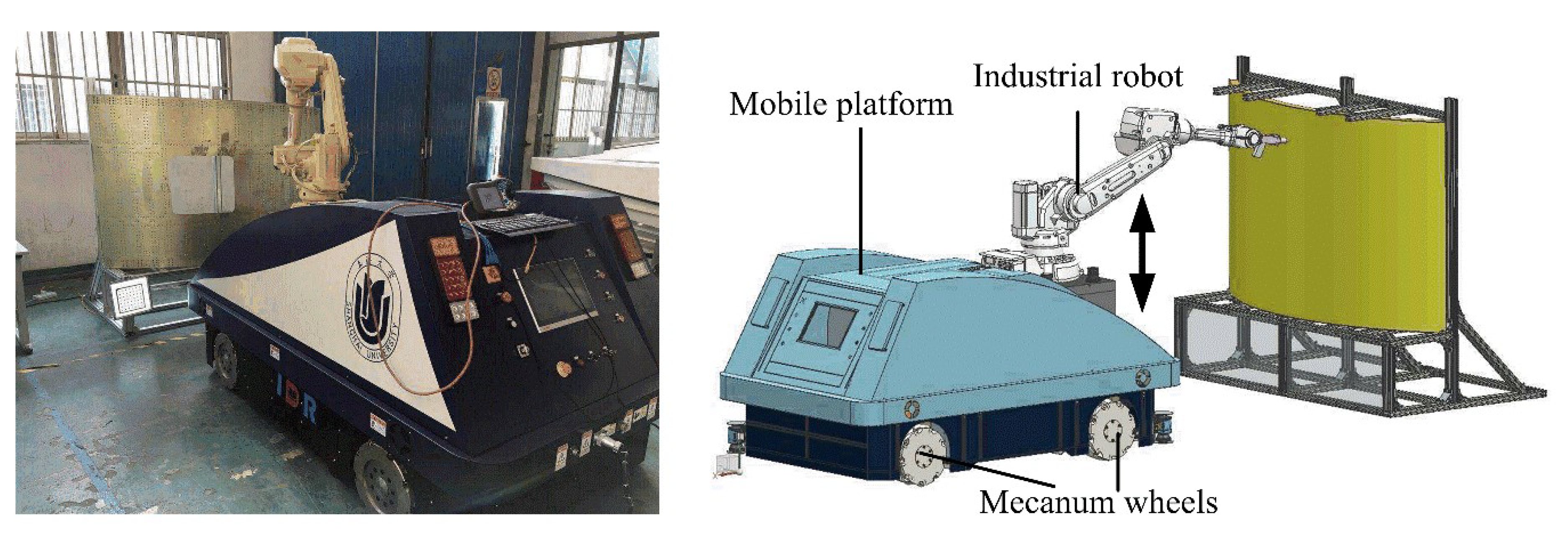
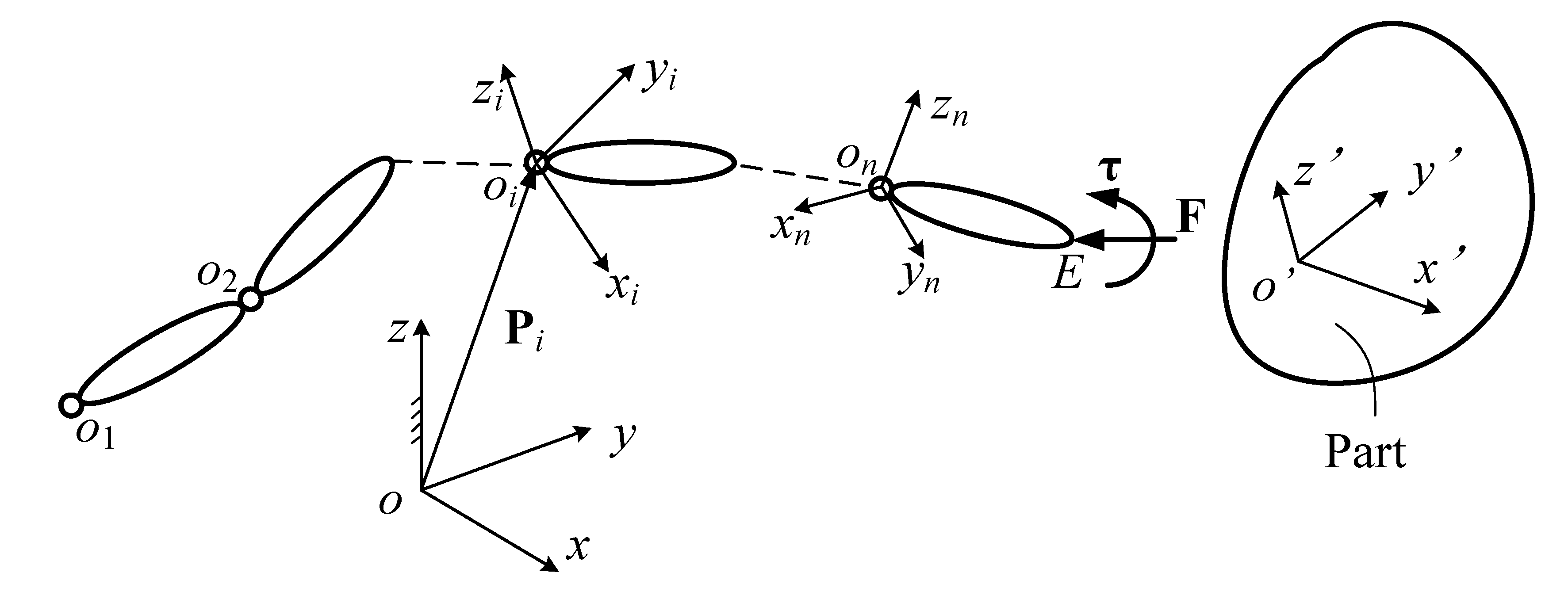
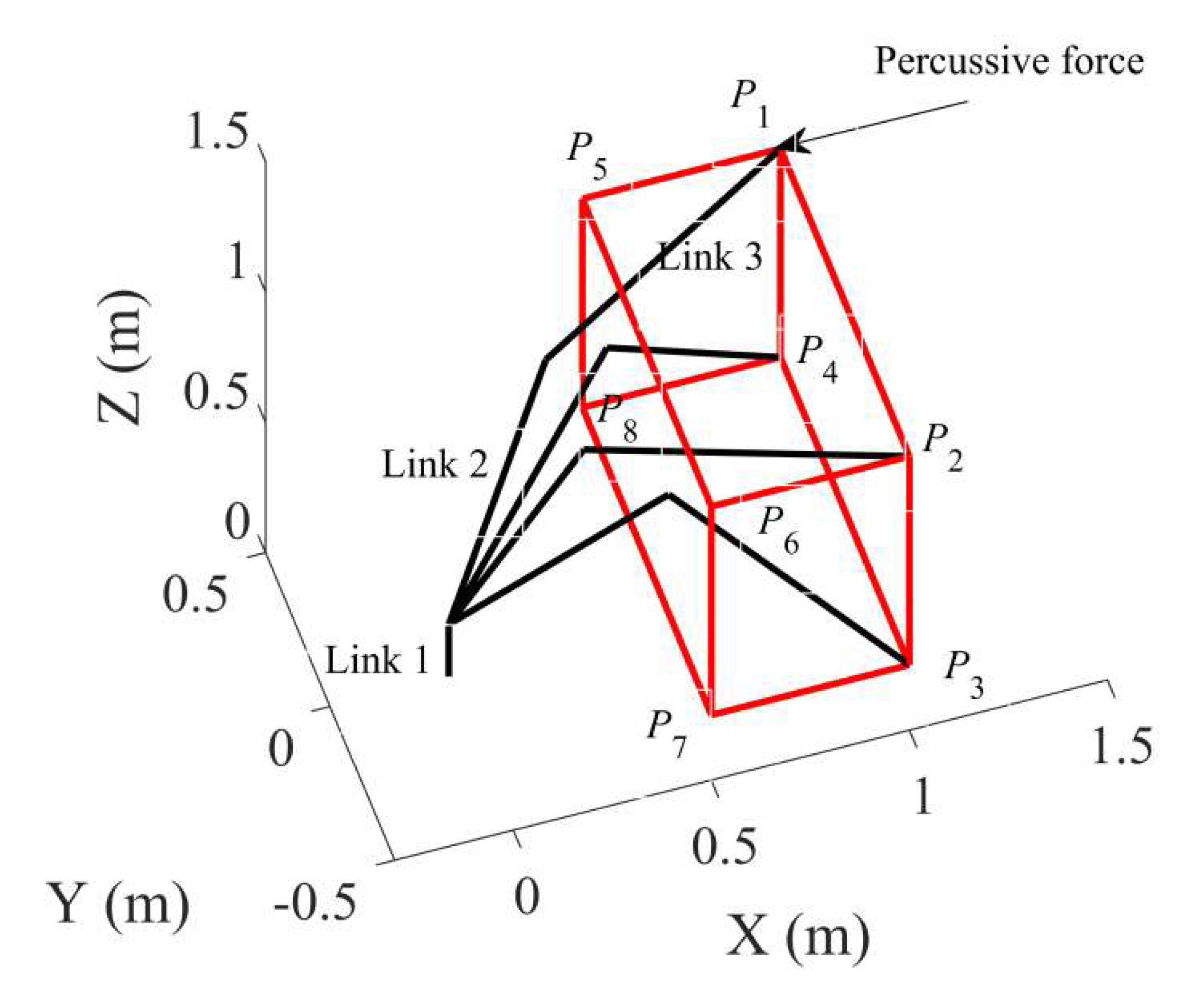
2. System Description
3. Theoretical Development
3.1. Equations of Motion
3.2. Frequency Response Functions
3.3. Frequency Response Ratios
4. Numerical Simulation
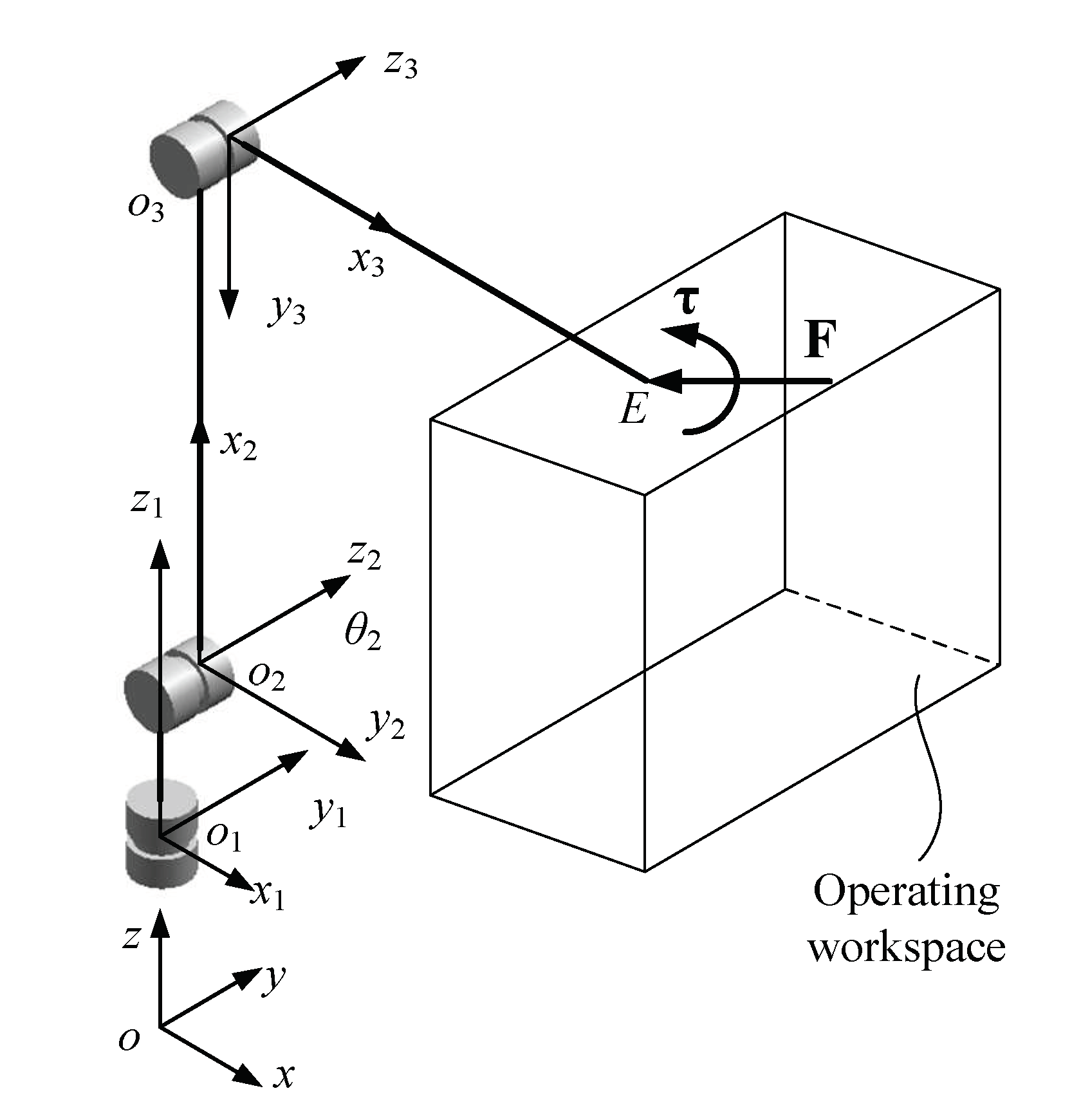
4.1. System Parameters
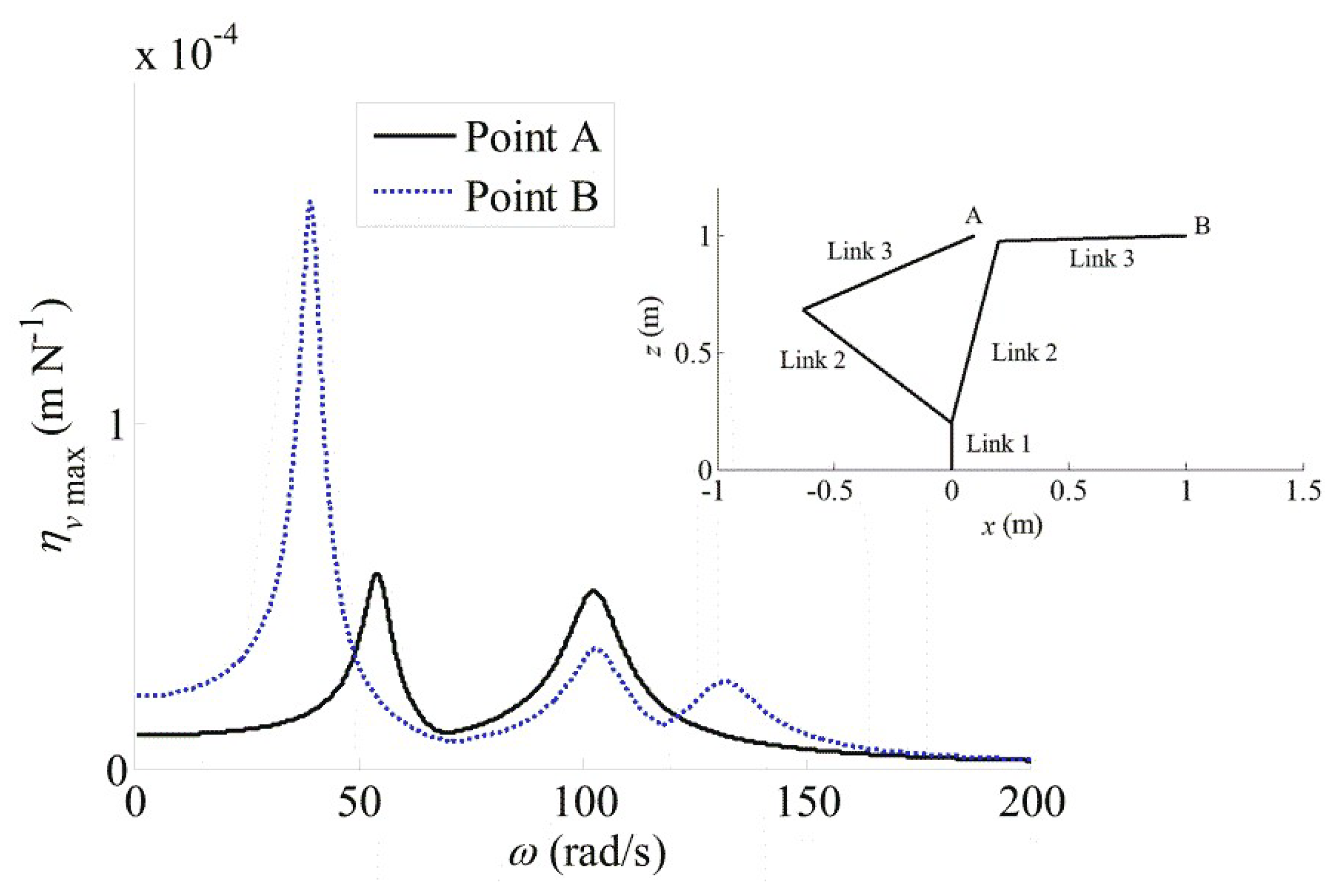
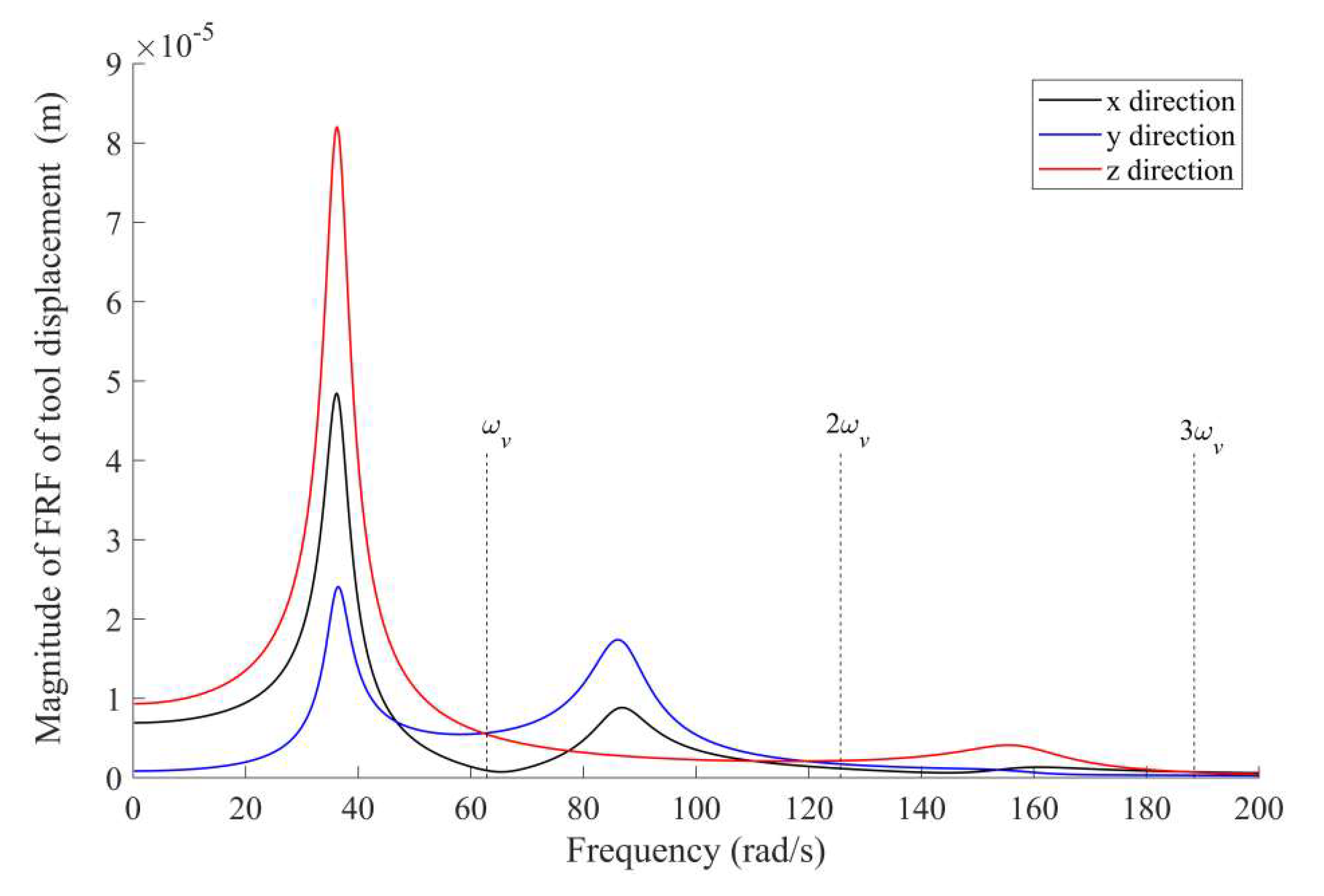
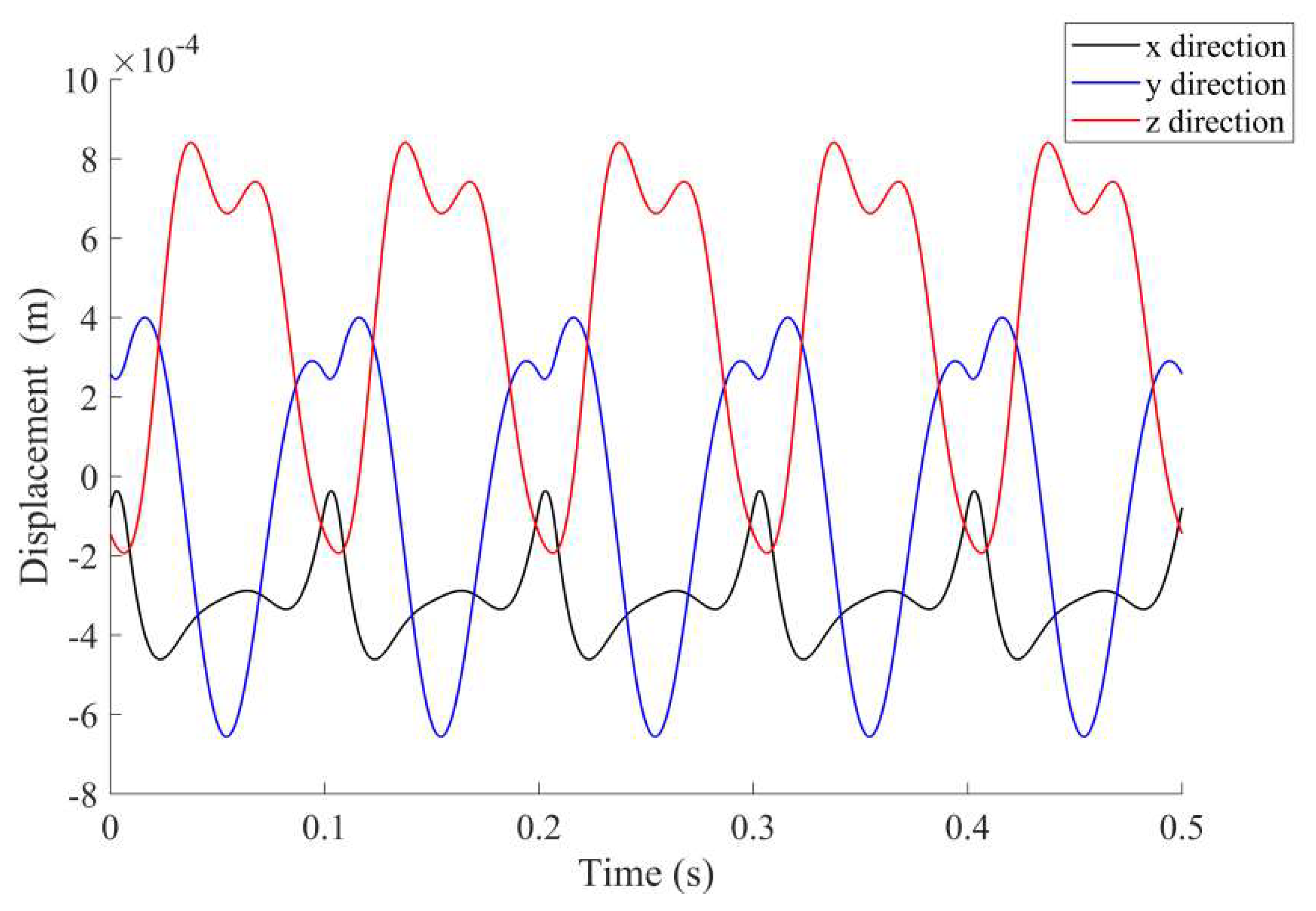
4.2. Performance Indices for Given Positions
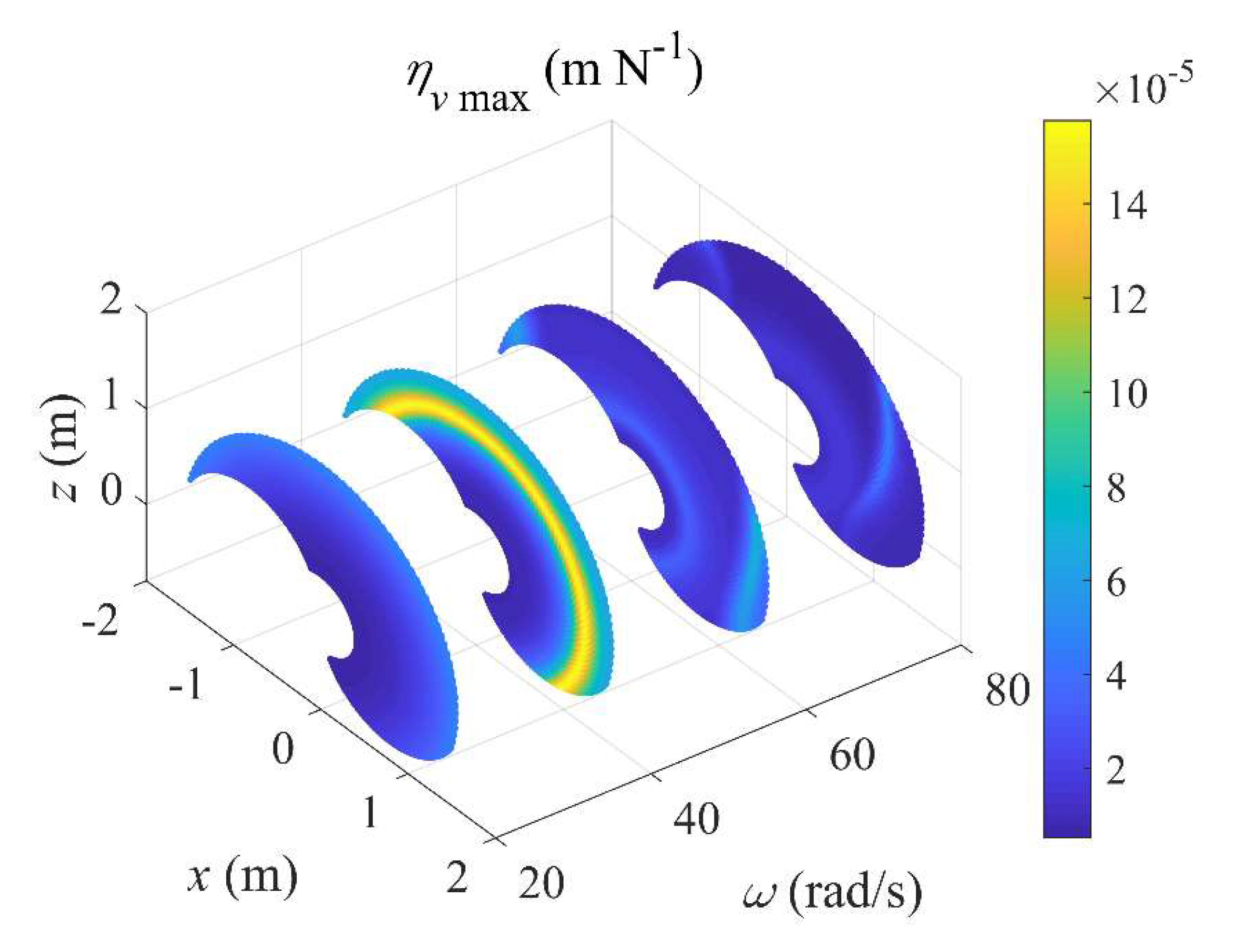
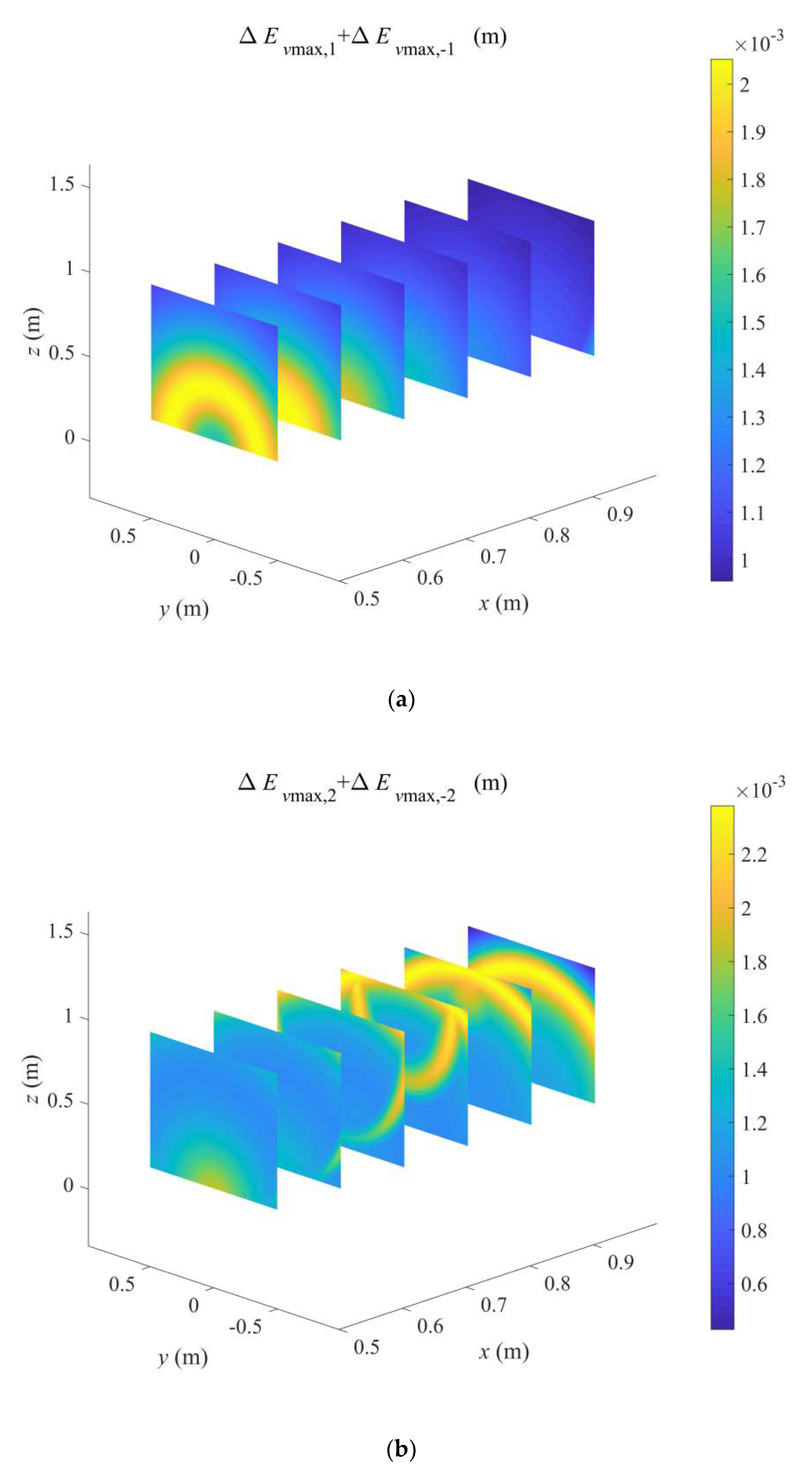
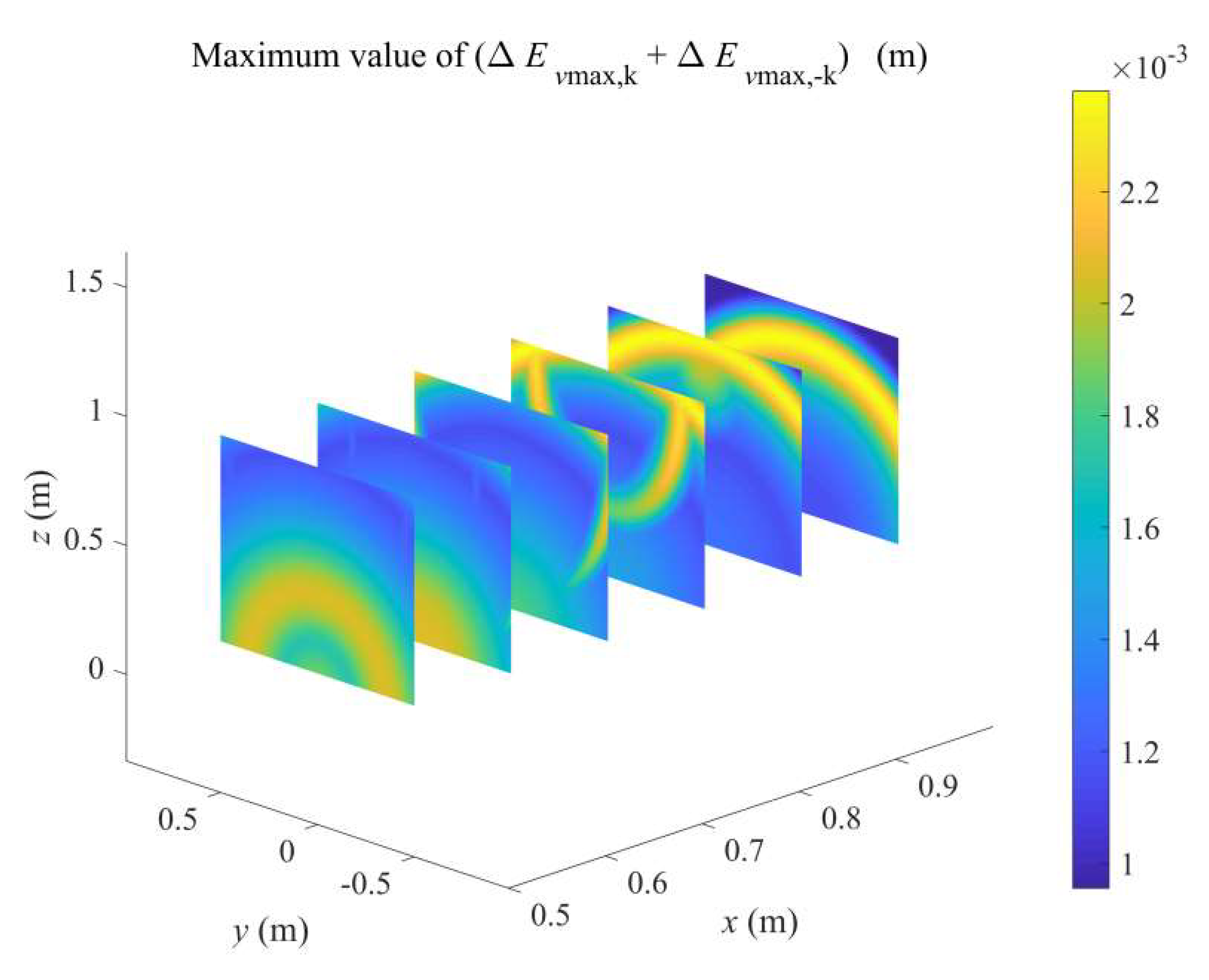
4.3. Distributions of Performance Indices
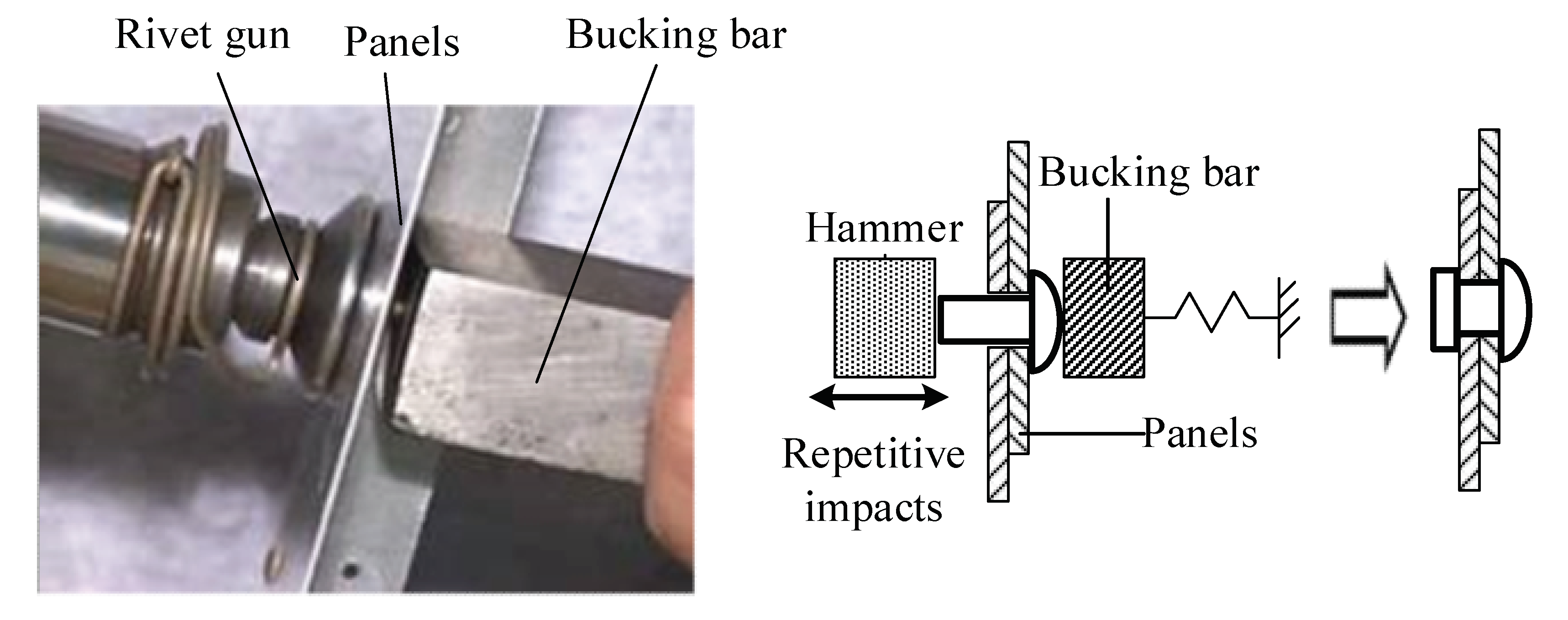
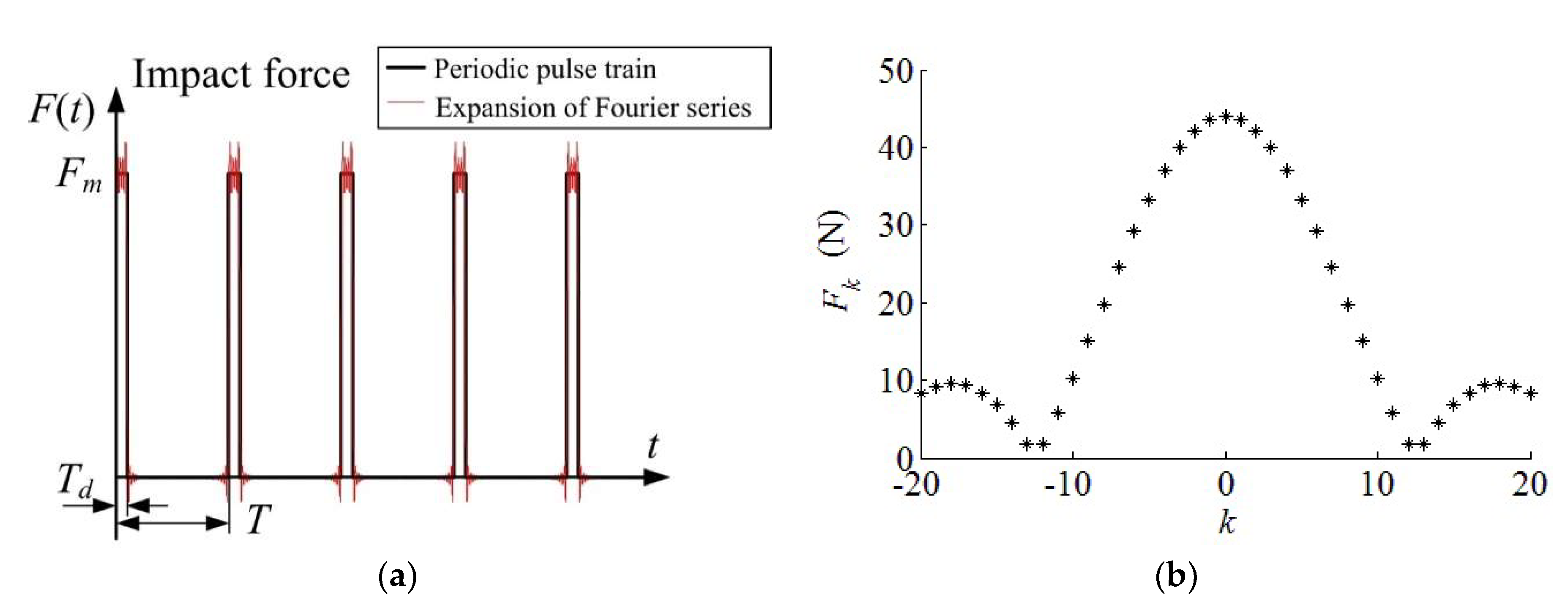
4.4. Performance Evaluation for Percussive Riveting
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Nof, S.Y. Handbook of Industrial Robotics, 2nd ed.; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Lehmann, C.; Pellicciari, M.; Drust, M.; Gunnink, J.W. Machining with industrial robots: The COMET project approach. Robot. Smart Manuf. 2013, 371, 27–36. [Google Scholar]
- Brunete, A.; Gambao, E.; Koskinen, J.; Heikkilä, T.; Kaldestad, K.B.; Tyapin, I.; Hovland, G.; Surdilovic, D.; Hernando, M.; Bottero, A.; et al. Hard material small-batch industrial machining robot. Robot. Comput. Manuf. 2018, 54, 185–199. [Google Scholar] [CrossRef]
- Boeing: A Futuristic View of the 777 Fuselage Build. The Boeing Company: Chicago. Available online: http://www.boeing.com/features/2014/07/bca-777-fuselage-07-14-14.page (accessed on 20 May 2020).
- Chen, Y.H.; Dong, F.H. Robot machining: Recent development and future research issues. Int. J. Adv. Manuf. Tech. 2013, 66, 1489–1497. [Google Scholar] [CrossRef]
- Barnfather, J.D.; Goodfellow, M.J.; Abram, T. Achievable tolerances in robotic feature machining operations using a low-cost hexapod. Int. J. Adv. Manuf. Tech. 2017, 95, 1–16. [Google Scholar] [CrossRef]
- Schneider, U.; Drust, M.; Ansaloni, M. Improving robotic machining accuracy through experimental error investigation and modular compensation. Int. J. Adv. Manuf. Technol. 2016, 85, 1–13. [Google Scholar] [CrossRef]
- Barnfather, J.D.; Goodfellow, M.J.; Abram, T. Development and testing of an error compensation algorithm for photogrammetry assisted robotic machining. Measurement 2016, 94, 561–577. [Google Scholar] [CrossRef]
- Barnfather, J.D.; Goodfellow, M.J.; Abram, T. Photogrammetric measurement process capability for metrology assisted robotic machining. Measurement 2016, 78, 29–41. [Google Scholar] [CrossRef]
- Leali, F.; Vergnano, A.; Pini, F.; Pellicciari, M.; Berselli, G. A workcell calibration method for enhancing accuracy in robot machining of aerospace parts. Int. J. Adv. Manuf. Technol. 2016, 85, 47–55. [Google Scholar] [CrossRef]
- Marie, S.; Courteille, E.; Maurine, P. Elasto-geometrical modeling and calibration of robot manipulators: Application to machining and forming applications. Mech. Mach. Theory 2013, 69, 13–43. [Google Scholar] [CrossRef]
- Barnfather, J.D.; Abram, T. Efficient compensation of dimensional errors in robotic machining using imperfect point cloud part inspection data. Measurement 2018, 117, 176–185. [Google Scholar] [CrossRef]
- Slavkovic, N.R.; Milutinovic, D.S.; Glavonjic, M.M. A method for off-line compensation of cutting force-induced errors in robotic machining by tool path modification. Int. J. Adv. Manuf. Technol. 2014, 70, 2083–2096. [Google Scholar] [CrossRef]
- Belchior, J.; Guillo, M.; Courteille, E.; Maurine, P.; Leotoing, L.; Guines, D. Off-line compensation of the tool path deviations on robotic machining: Application to incremental sheet forming. Robot. Comput. Manuf. 2013, 29, 58–69. [Google Scholar] [CrossRef]
- Mejri, S.; Gagnol, V.; Le, T.; Sabourin, P.L.; Ray, P.; Paultre, P. Dynamic characterization of machining robot and stability analysis. Int. J. Adv. Manuf. Technol. 2016, 82, 351–359. [Google Scholar] [CrossRef]
- Tian, Y.; Wang, B.; Liu, J.; Chen, F.; Yang, S.; Wang, W.; Li, L. Research on layout and operational pose optimization of robot grinding system based on optimal stiffness performance. J. Adv. Mech. Des. Syst. 2017, 11. [Google Scholar] [CrossRef]
- Guo, Y.; Dong, H.; Ke, Y. Stiffness-oriented posture optimization in robotic machining applications. Robot. Comput. Manuf. 2015, 35, 69–76. [Google Scholar] [CrossRef]
- Li, Y.; Ji, J.; Guo, S.; Xi, F. Process parameter optimization of a mobile robotic percussive riveting system with flexible joints. J. Comput. Nonlin. Dyn. 2017, 12, 061005. [Google Scholar] [CrossRef]
- Zaghbani, I.; Songmene, V.; Bonev, I. An experimental study on the vibration response of a robotic machining system. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2013, 227, 866–880. [Google Scholar] [CrossRef]
- Tratar, J.; Pusavec, F.; Kopac, J. Tool wear in terms of vibration effects in milling medium-density fibreboard with an industrial robot. J. Mech. Sci. Technol. 2014, 28, 4421–4429. [Google Scholar] [CrossRef]
- Nie, S.; Li, Y.; Shuai, G.; Tao, S.; Xi, F. Modeling and simulation for fatigue life analysis of robots with flexible joints under percussive impact forces. Robot. Comput. Manuf. 2016, 37, 292–301. [Google Scholar] [CrossRef]
- Mousavi, S.; Gagnol, V.; Bouzgarrou, B.C.; Ray, P. Model-based stability prediction of a machining robot. In New Advances in Mechanisms, Mechanical Transmissions and Robotics; Corves, B., Lovasz, E.-C., Husing, M., Maniu, I., Gruescu, C., Eds.; Springer: Berlin, Germany, 2017; Volume 46, pp. 379–387. [Google Scholar]
- Wang, G.; Dong, H.; Guo, Y.; Ke, Y. Chatter mechanism and stability analysis of robotic boring. Int. J. Adv. Manuf. Technol. 2017, 91, 411–421. [Google Scholar] [CrossRef]
- Li, J.; Li, B.; Shen, N.; Qian, Y.H.; Guo, Z.M. Effect of the cutter path and the workpiece clamping position on the stability of the robotic milling system. Int. J. Adv. Manuf. Technol. 2016, 89, 2919–2933. [Google Scholar] [CrossRef]
- Tunc, L.T.; Stoddart, D. Tool path pattern and feed direction selection in robotic milling for increased chatter-free material removal rate. Int. J. Adv. Manuf. Technol. 2017, 89, 2907–2918. [Google Scholar] [CrossRef]
- Siciliano, B.; Khatib, O. Springer Handbook of Robotics; Springer: Berlin, Germany, 2008; pp. 987–1008. [Google Scholar]
- Gosselin, C.M.; Angeles, J. A global performance index for the kinematic optimization of robotic manipulators. ASME J. Mech. Des. 1991, 113, 220–226. [Google Scholar] [CrossRef]
- Koeppe, R.; Yoshikawa, T. Dynamic manipulability analysis of compliant motion. In Proceedings of the IEEE International Conference on Intelligent Robots and Systems, Grenoble, France, 8–12 September 1997. [Google Scholar]
- Chiacchio, P. Dynamic manipulability ellipsoid for redundant manipulators. Robotica 2000, 18, 381–387. [Google Scholar] [CrossRef]
- Graettinger, T.J.; Krogh, B.H. The acceleration radius: A global performance measure for robotic manipulators. Int. J. Robot. Autom. 1988, 4, 60–69. [Google Scholar] [CrossRef]
- Kurazume, R.; Hasegawa, T. A new index of serial-link manipulator performance combining dynamic manipulability and manipulating force ellipsoids. IEEE Trans. Robot. 2006, 22, 1022–1028. [Google Scholar] [CrossRef]
- Liu, X.J.; Jin, Z.L.; Gao, F. Optimum design of 3-DOF spherical parallel manipulators with respect to the conditioning and stiffness indices. Mech. Mach. Theory 2000, 35, 1257–1267. [Google Scholar] [CrossRef]
- Courteille, E.; Deblaise, D.; Maurine, P. Design optimization of a delta-like parallel robot through global stiffness performance evaluation. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 15 November 2009. [Google Scholar]
- Scalera, L.; Palomba, I.; Wehrle, E.; Gasparetto, A.; Vidoni, R. Natural motion for energy saving in robotic and mechatronic systems. Appl. Sci. 2019, 9, 3516. [Google Scholar] [CrossRef]
- Zhang, F. Matrix Theory: Basic Results and Techniques, 2nd ed.; Springer: New York, NY, USA, 2011; pp. 253–292. [Google Scholar]
- Behi, F.; Tesar, D. Parametric identification for industrial manipulators using experimental modal analysis. IEEE Trans. Robot. Autom. 1991, 7, 642–652. [Google Scholar] [CrossRef]
- Zhang, D.; Wei, B. Novel Design and Applications of Robotics Technologies; IGI Global: Hershey, PA, USA, 2019; pp. 26–65. [Google Scholar]


| Link | Masses (kg) | Position Vector of CG (m) | Inertia Tensors (kg-m2) |
|---|---|---|---|
| 1 | 80 | (0, 0, 0.1) | diag(0.9, 1, 1) |
| 2 | 40 | (0.4, 0, 0) | diag(0.6, 6.5, 6.5) |
| 3 | 55 | (0.4, 0, 0) | diag(0.8, 9.5, 9.5) |
| i | ||||
|---|---|---|---|---|
| 1 | 0 | 0 | 0.2 | 0 |
| 2 | 0 | 0 | ||
| 3 | 0 | 0.8 | 0 | 0 |
| Indices | ω = 20 Hz | ω = 30 Hz | ω = 40 Hz | ω = 50 Hz | ω = 60 Hz | ω = 70 Hz | ω = 80 Hz |
|---|---|---|---|---|---|---|---|
| Mean value of ηυmax (10−5 m/N) | 2.2786 | 4.3875 | 6.3616 | 3.2873 | 2.2236 | 1.4321 | 1.2691 |
| Maximum value of ηυmax (10−5 m/N) | 4.5850 | 13.100 | 15.770 | 8.0613 | 7.2806 | 5.8410 | 4.7531 |
| Mean value of ηwmax (10−5/N) | 2.2145 | 3.9172 | 5.7468 | 3.3286 | 2.2313 | 1.5149 | 1.4133 |
| Maximum value of ηwmax (10−5/N) | 3.4936 | 9.7238 | 13.869 | 9.0598 | 5.0308 | 4.4893 | 4.0502 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, P.; Li, Y. New Indices for Evaluating Vibration Characteristics of Flexible-Joint Robots. Appl. Sci. 2020, 10, 4895. https://doi.org/10.3390/app10144895
Zhang P, Li Y. New Indices for Evaluating Vibration Characteristics of Flexible-Joint Robots. Applied Sciences. 2020; 10(14):4895. https://doi.org/10.3390/app10144895
Chicago/Turabian StyleZhang, Ping, and Yuwen Li. 2020. "New Indices for Evaluating Vibration Characteristics of Flexible-Joint Robots" Applied Sciences 10, no. 14: 4895. https://doi.org/10.3390/app10144895
APA StyleZhang, P., & Li, Y. (2020). New Indices for Evaluating Vibration Characteristics of Flexible-Joint Robots. Applied Sciences, 10(14), 4895. https://doi.org/10.3390/app10144895




