Simulation of Lid-Driven Cavity Flow with Internal Circular Obstacles
Abstract
:1. Introduction
2. Numerical Method
2.1. Governing Equation
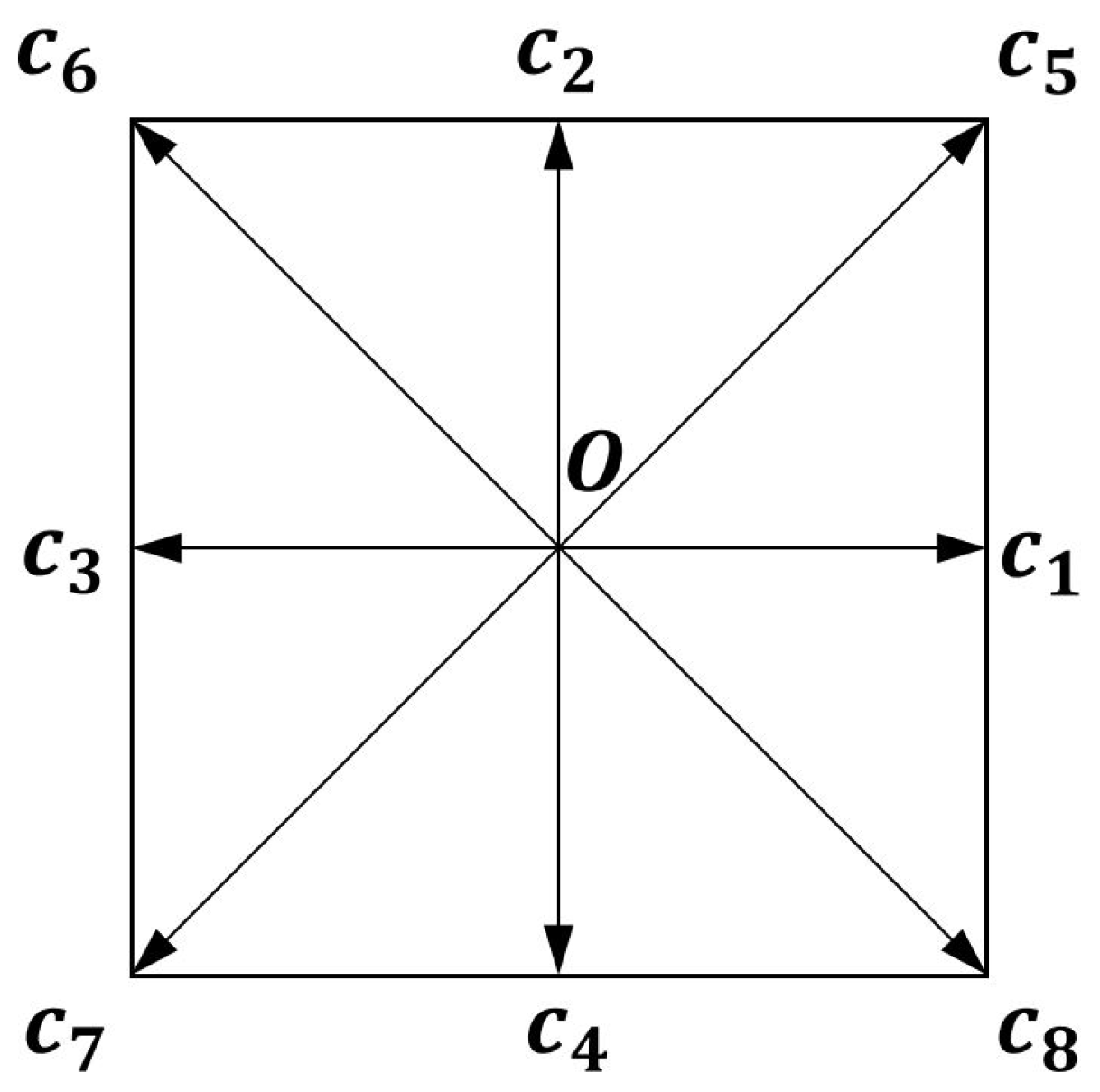
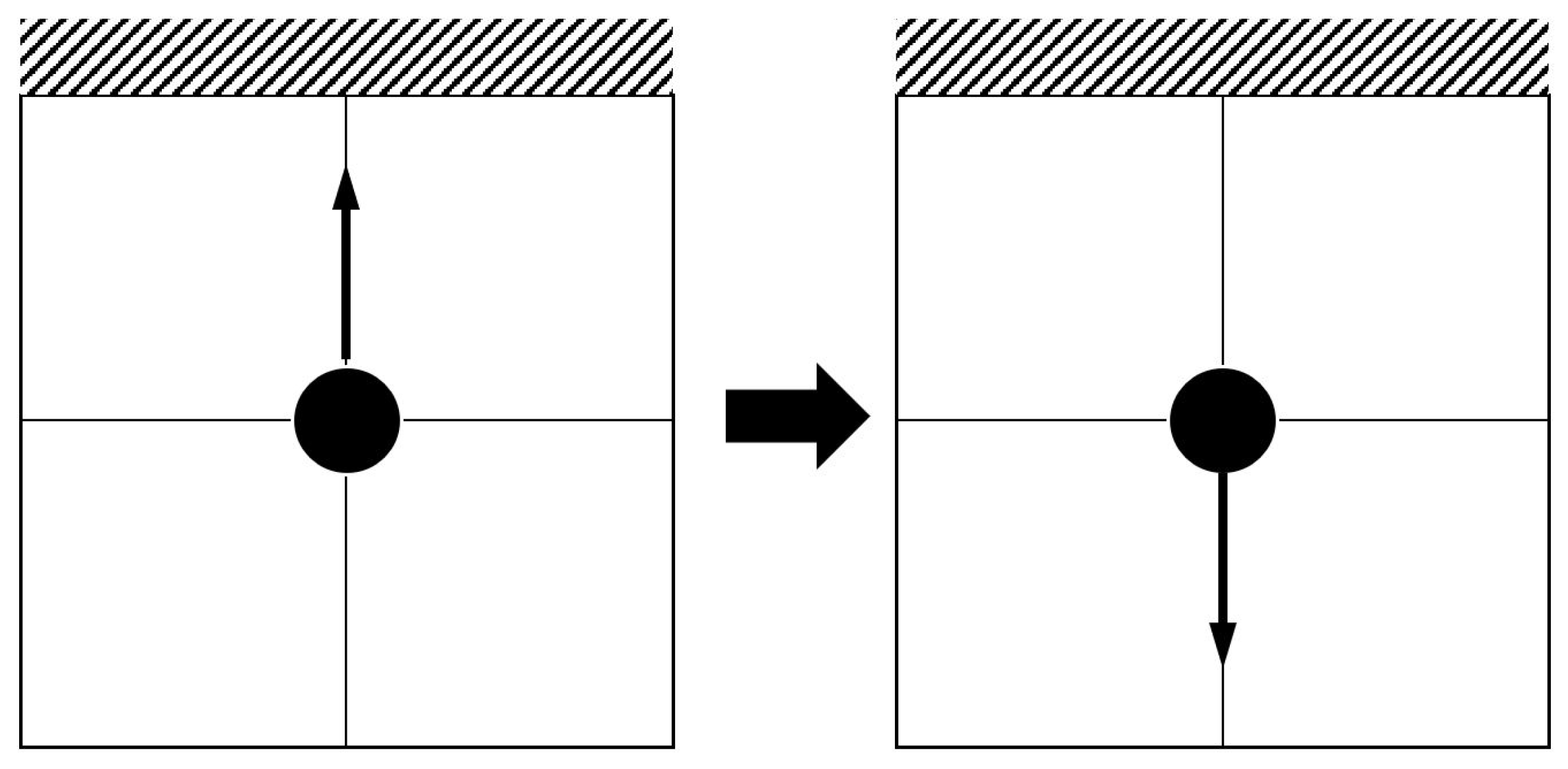
2.2. Boundary Conditions
3. Results and Discussion
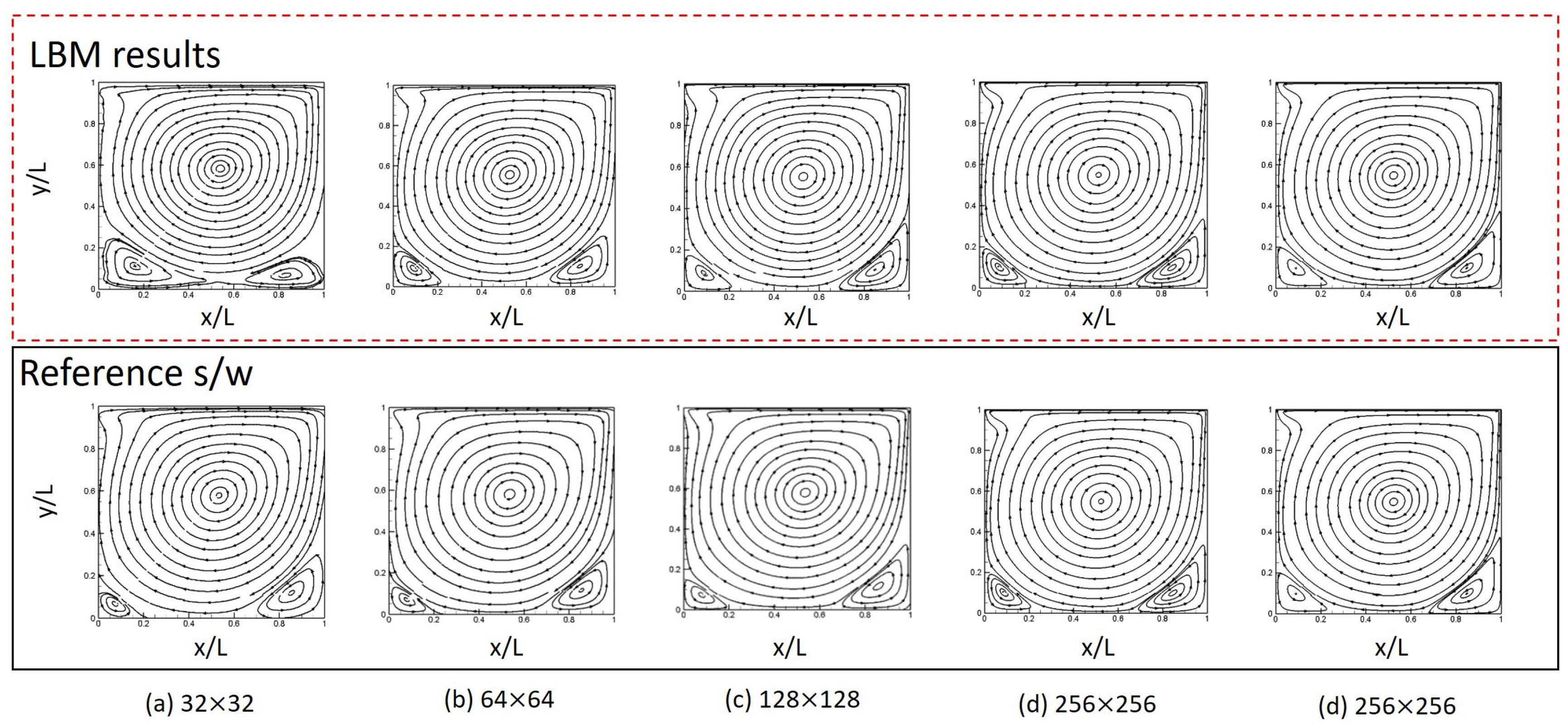
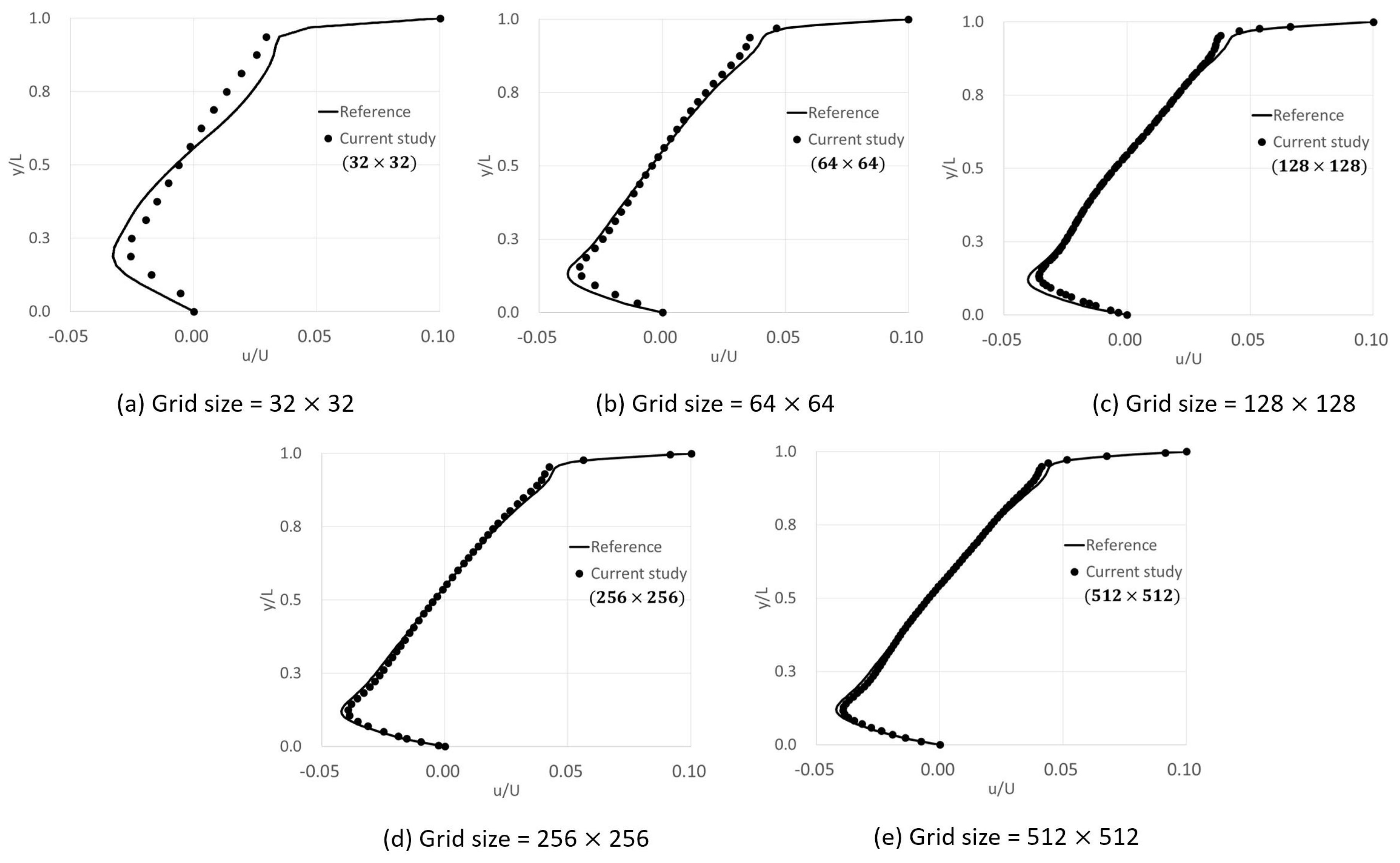
3.1. Mesh Dependence Check: Mesh Size
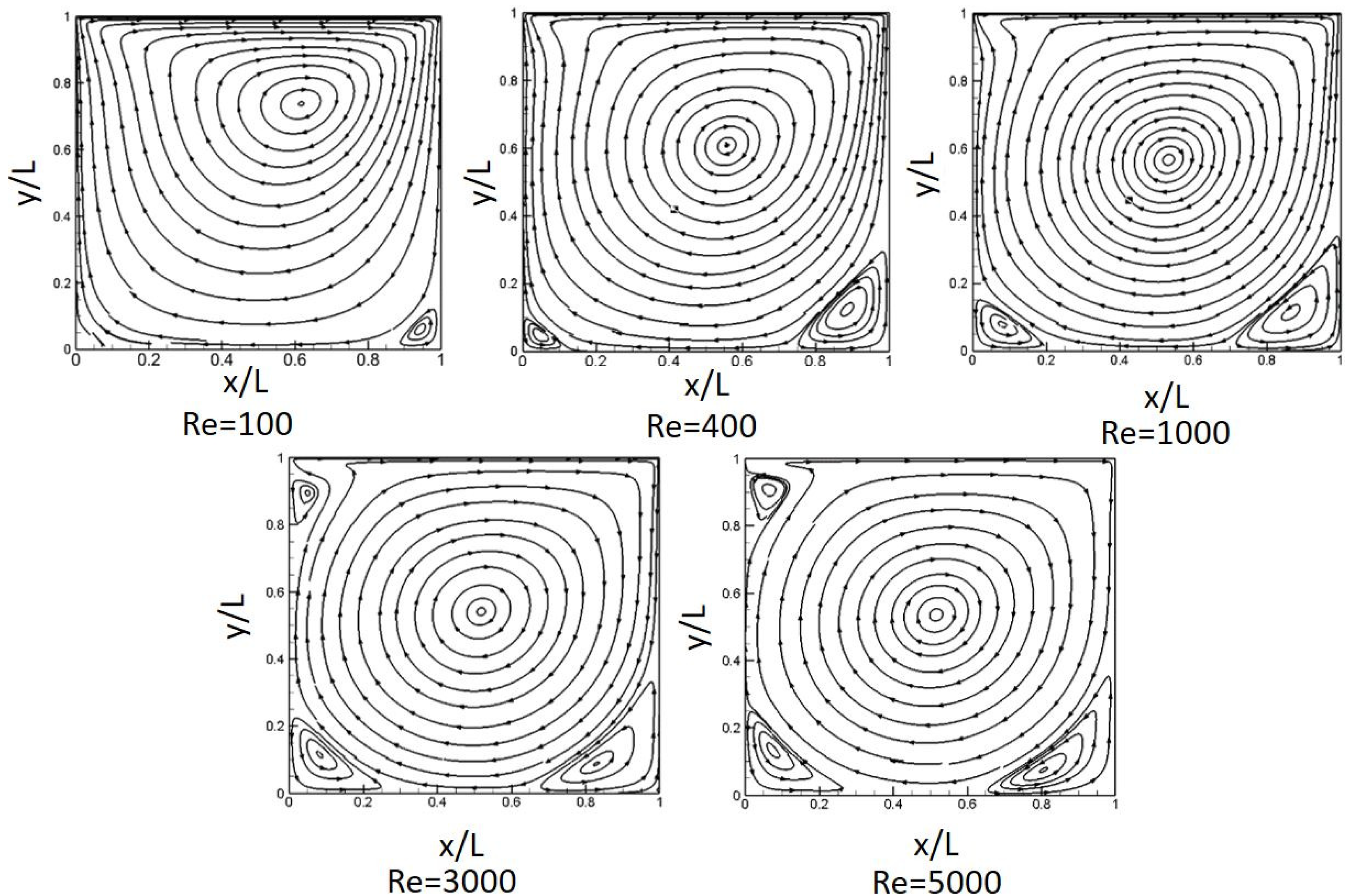
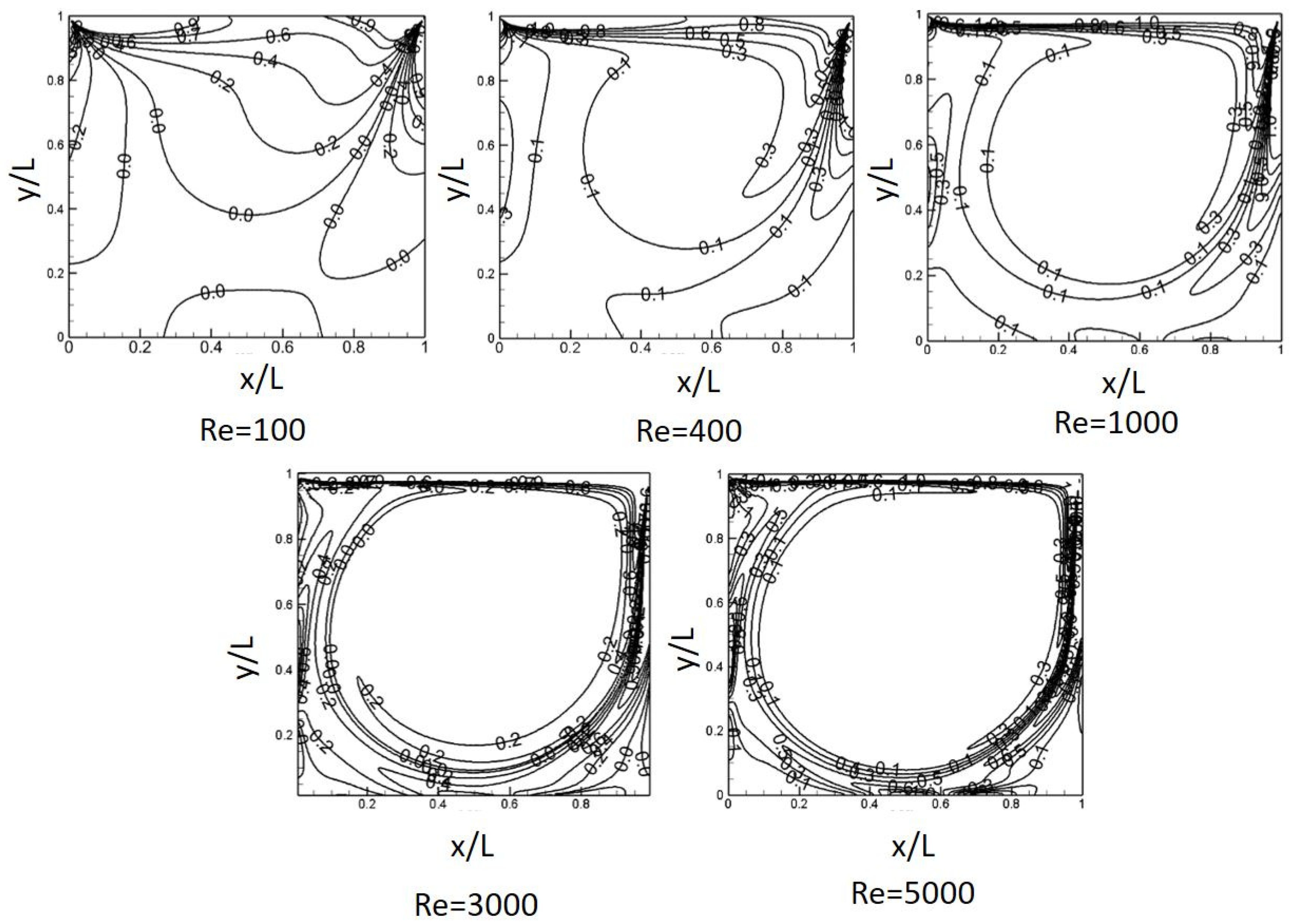
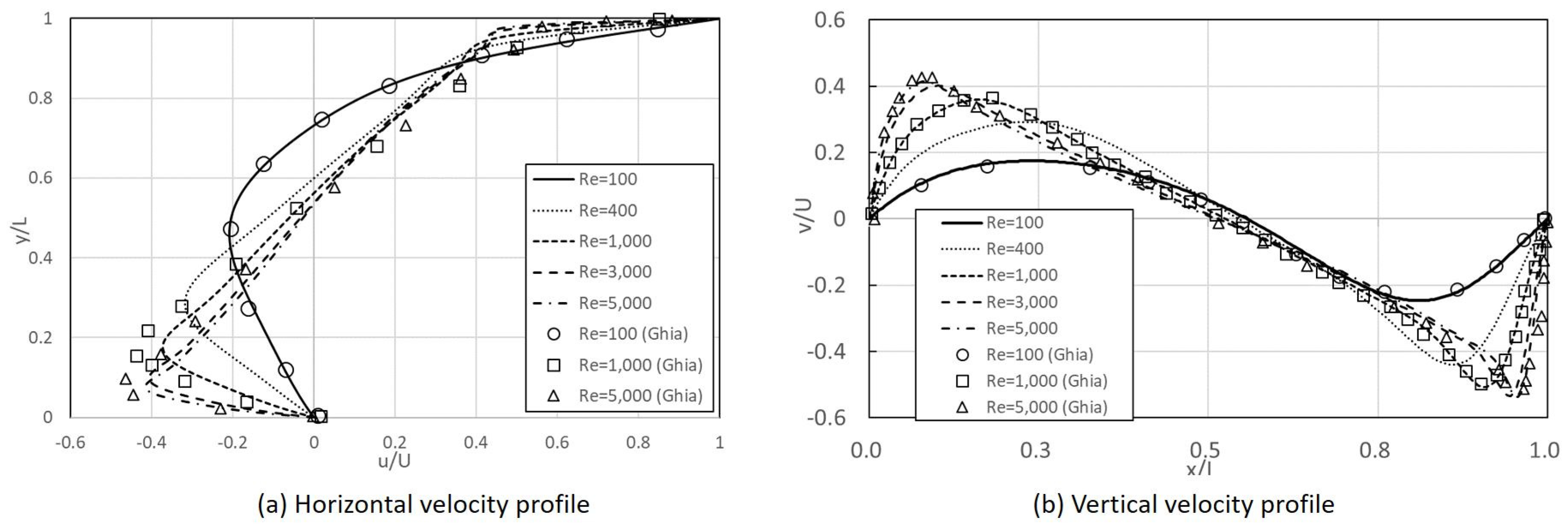
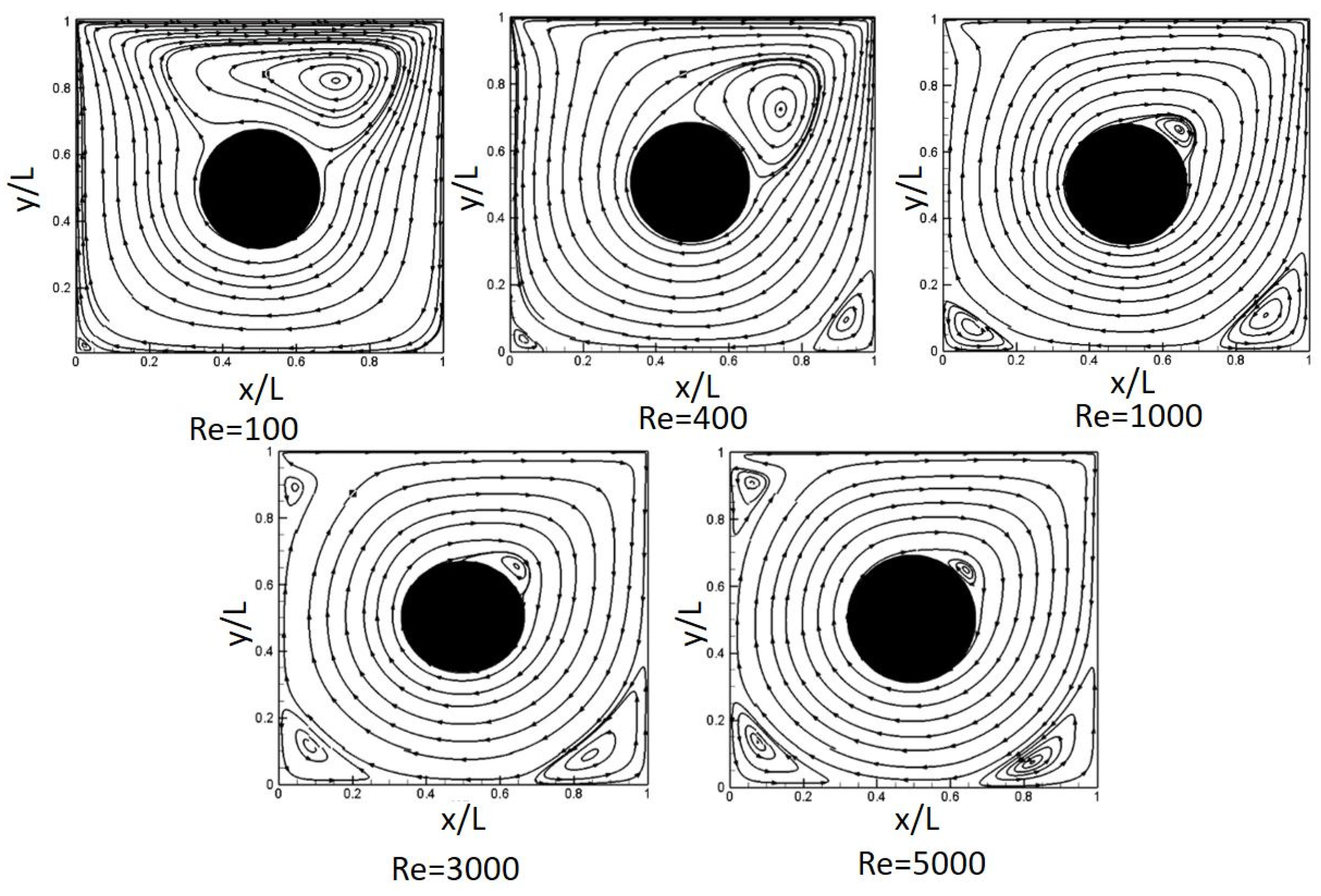
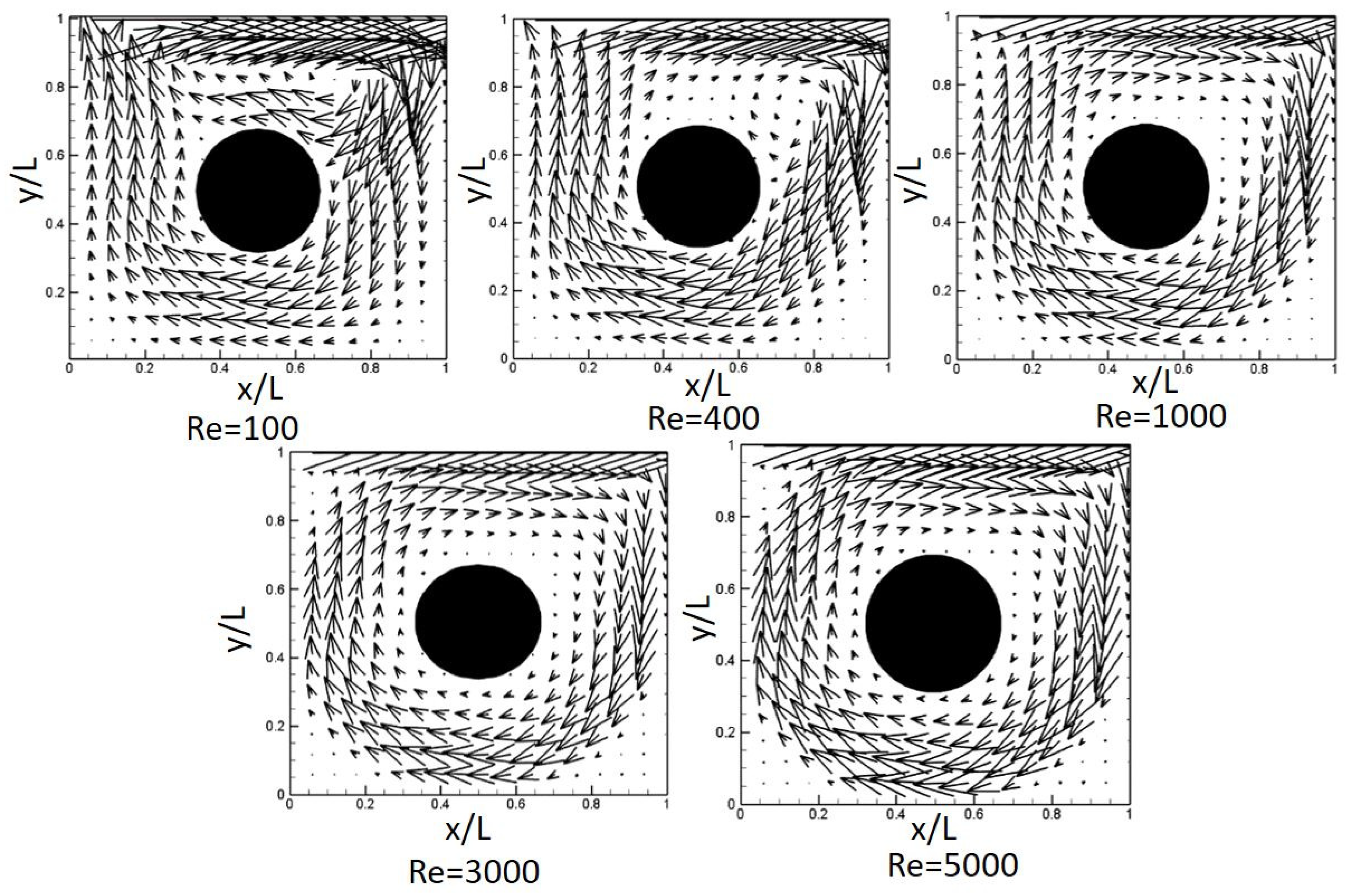
3.2. Lid-Driven Square-Cavity Flow without Obstacles
3.3. Location of Vortex Centers and Their Comparison
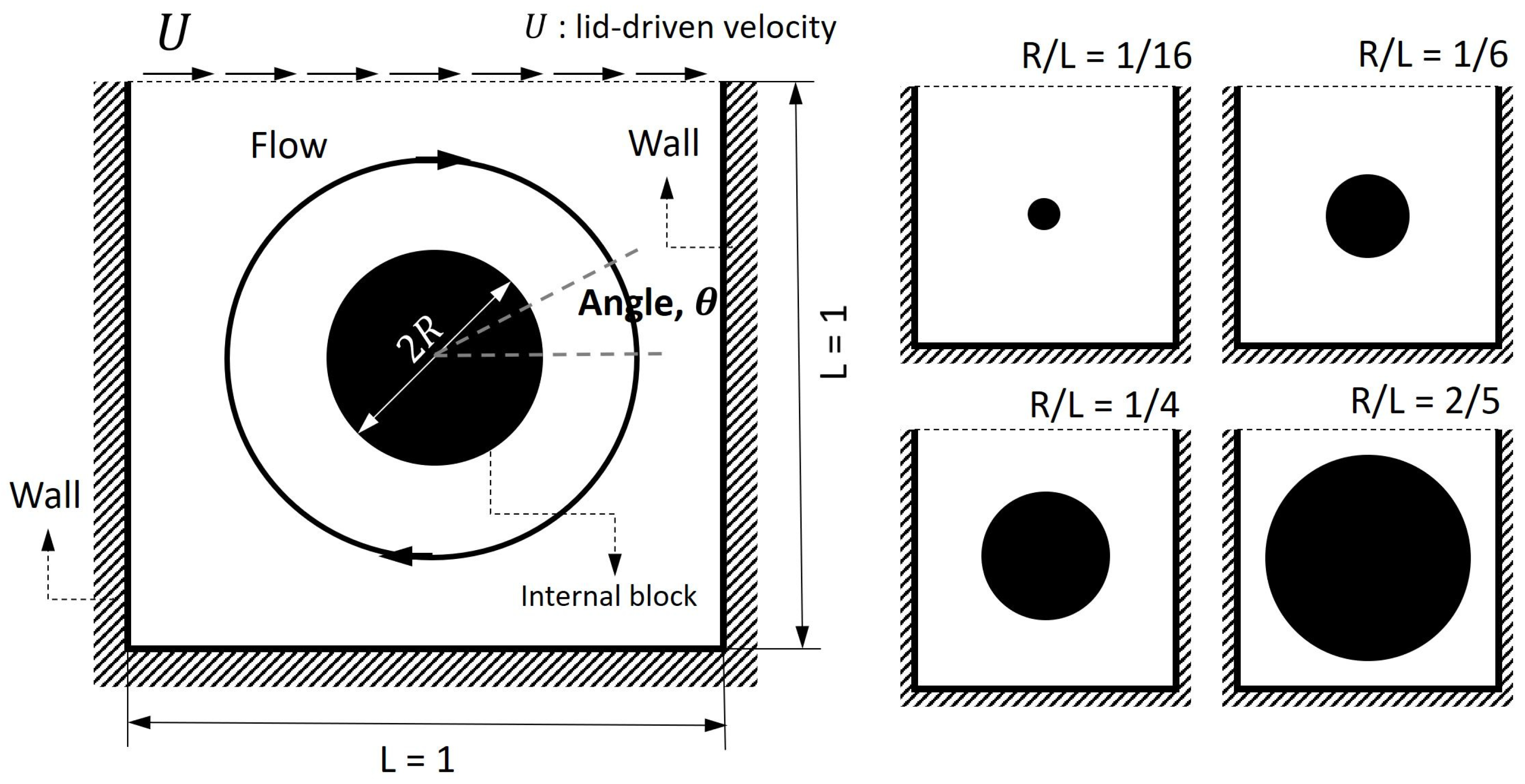
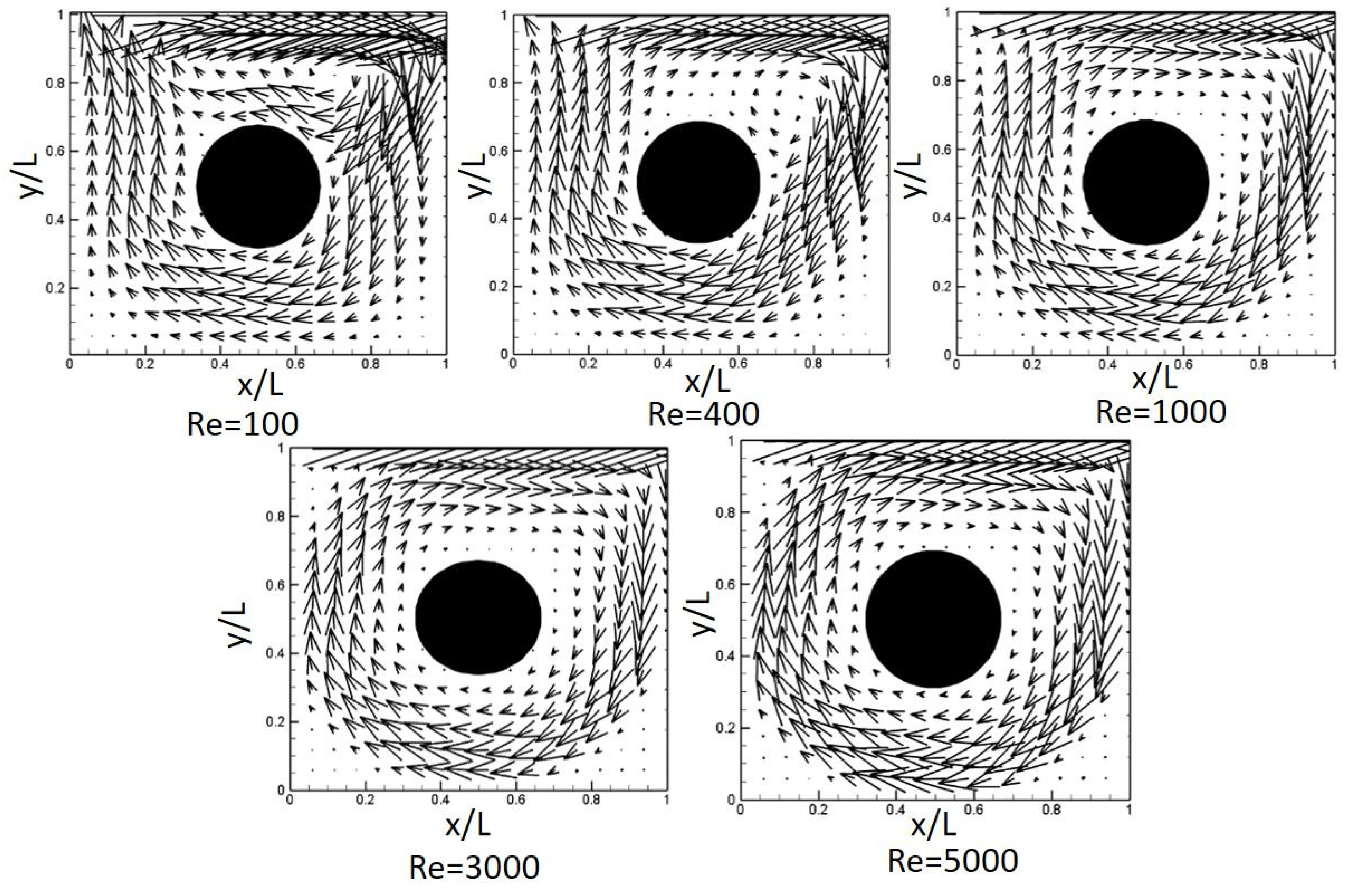
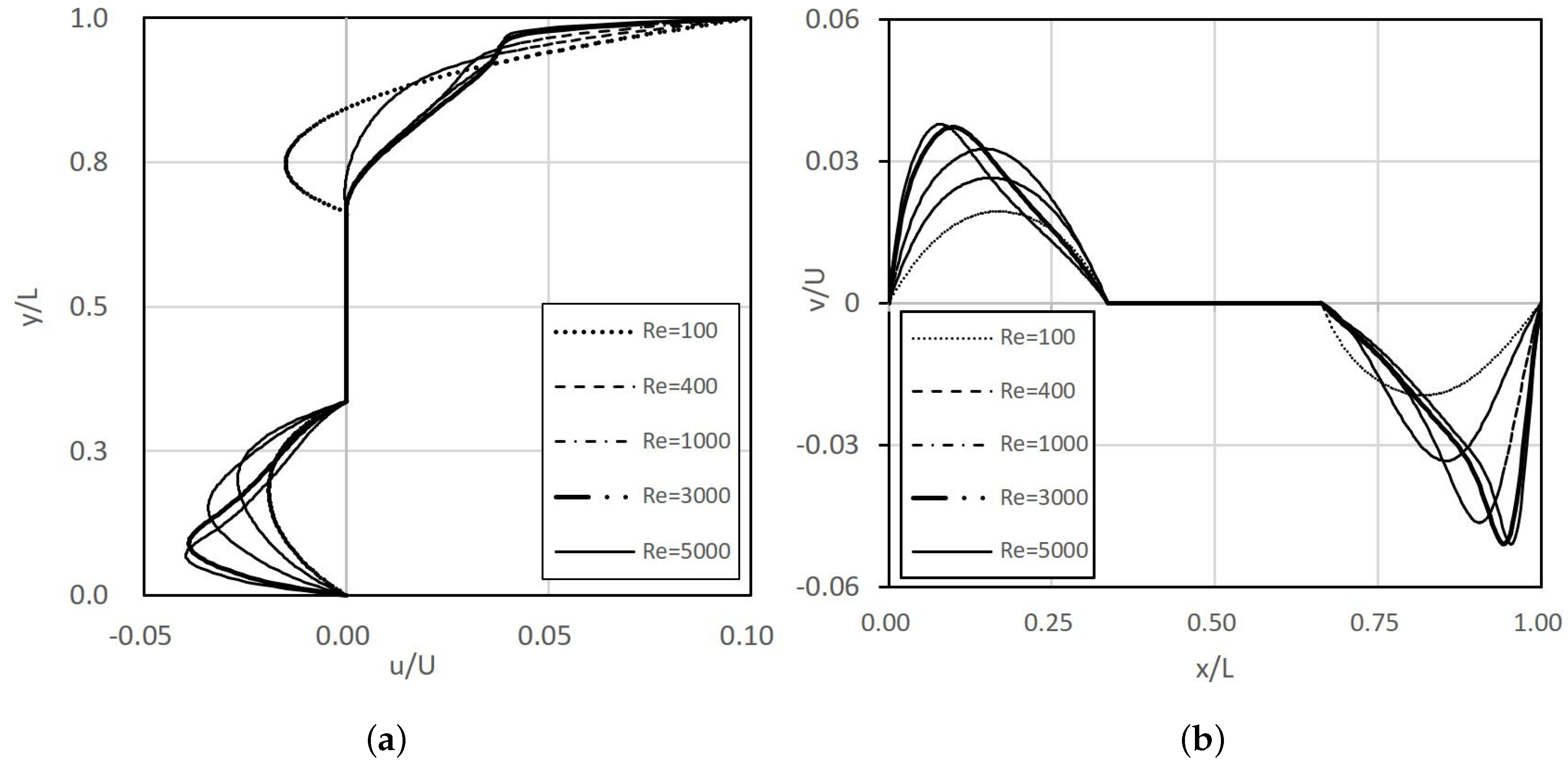
3.4. Flow Characteristics inside a Cavity with an Internal Obstacle ( = 1/6)
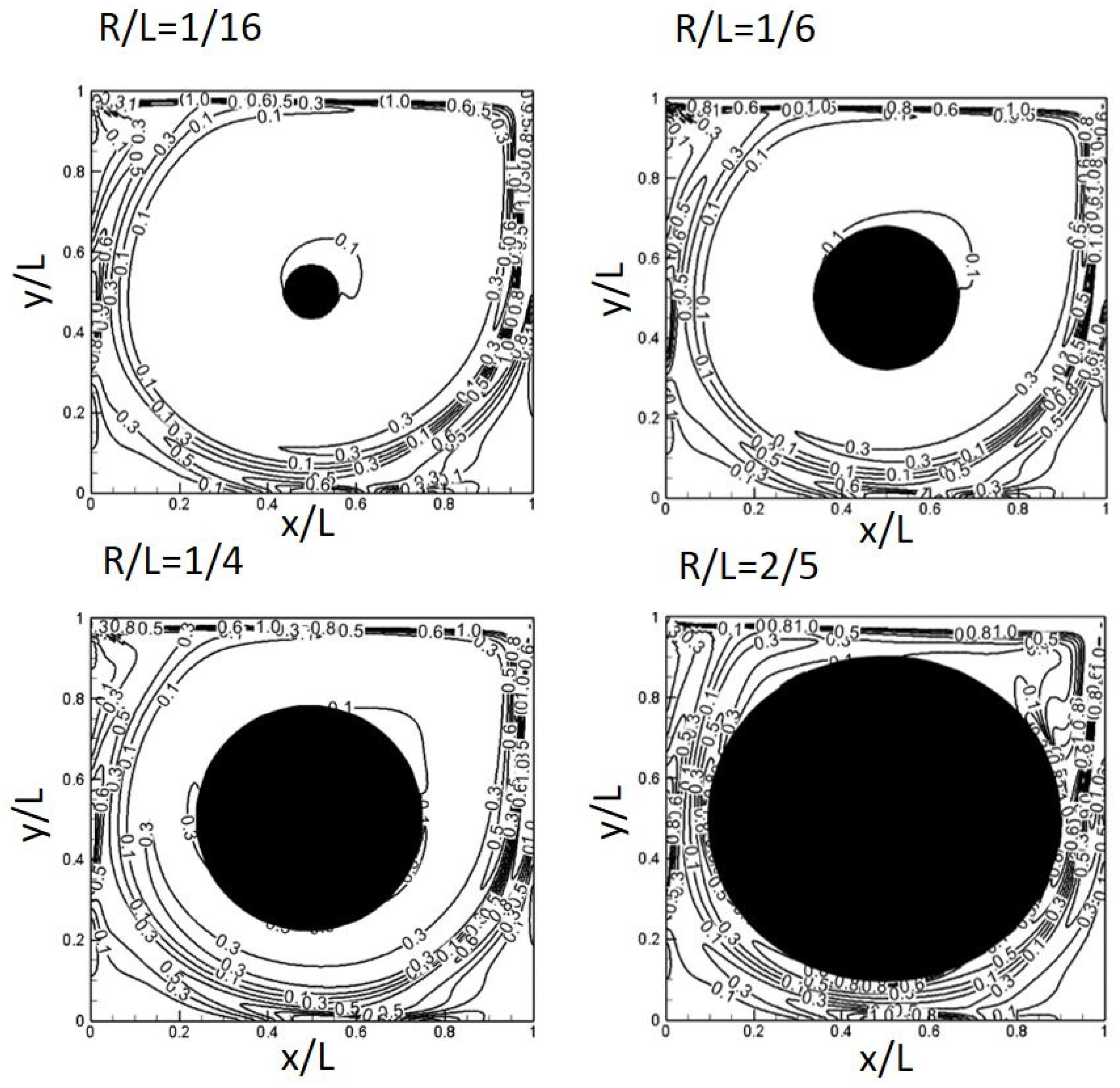
3.5. Flow Characteristics inside a Cavity with Varying Sizes of Internal Obstacles ( = 1/16, 1/6, 1/4, and 2/5)
4. Conclusions
- In order to observe the effect of on the flow inside the lid-driven square cavity, the magnitude of the viscosity in is increased by a factor of 50 in order to vary from 100 to 5000. As increases, the primary vortices inside the cavity without an obstacle move towards the center of the cavity, and the secondary vortices at the bottom corners increase in size. However, the core area in the central region is almost constant and fully developed in the range of = 3000–5000.
- In order to analyze the vortex variation inside a cavity, the stream function (symbol ) as well as the locations of the primary and secondary vortices were compared with existing data. The location of the vortex centers obtained in this study have some scatter depending on the location comparing with the previous work, but the deviation of location is, in the majority of cases, less than 20%.
- For the cavity with internal obstacles, as Re number increased, the secondary vortices close to the internal obstacles became smaller owing to the strong primary vortices. Depending on the obstacle size ( = 1/16, 1/6, 1/4, and 2/5), however, secondary vortices were induced at each corner of the cavity and remained stationary, but the secondary vortices close to the top region of the obstacle became larger as the obstacle size was increased.
- In order to analyze the velocity variation around the obstacle, speeds around obstacles of various sizes ( = 1/16, 1/6, 1/4, and 2/5) were compared at = 5000. Interestingly, depending on the size of the obstacle, the magnitude of the circumferential velocity showed a significant variation. In the case of = 1/16, the magnitude of the surface velocity () is less than 0.001 in the range of , whereas the other half has a greater magnitude over , which tends to be similar in the other cases as well.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Guo, Z.L.; Shin, B.C.; Wang, N. Lattice BGK model for incompressible Navier-Stokes equation. J. Comput. Phys. 2000, 165, 288–306. [Google Scholar] [CrossRef]
- Ghia, U.; Ghia, K.N.; Shin, C.T. High-Re solutions for incompressible flow using the Navier-Stokes equations and a multigrid method. J. Comput. Phys. 1982, 48, 387–411. [Google Scholar] [CrossRef]
- Hasert, M.; Bernsdorf, J.; Roller, S. Lattice Boltzmann simulation of non-Darcy flow in porous media. Procedia Comput. Sci. 2011, 4, 1048–1057. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.; Chen, H.; Martinez, D.O.; Matthaeus, W.H. Lattice Boltzmann model for simulation of magnetohydrodynamics. Phys. Rev. Lett. 1991, 67, 3776–3779. [Google Scholar] [CrossRef] [PubMed]
- Yan, Y.Y.; Zu, Y.Q.; Dong, B. LAM, a useful tool for mesoscale modelling of single-phase and multiphase flow. Appl. Therm. Eng. 2011, 31, 649–655. [Google Scholar] [CrossRef]
- Chang, C.; Liu, C.H.; Lin, C.A. Boundary conditions for lattice Boltzmann simulations with complex geometry flows. Comput. Math. Appl. 2009, 58, 940–949. [Google Scholar] [CrossRef] [Green Version]
- Shankar, P.N.; Deshpande, M.D. Fluid Mechanics in the driven cavity. Annu. Rev. Fluid Mech. 2000, 32, 93–136. [Google Scholar] [CrossRef] [Green Version]
- Erturk, E.; Corke, T.; Gokcol, C. Numerical Solutions of 2-D Steady Incompressible Driven Cavity Flow in a driven skewed cavity High Reynolds Numbers. I. J. Numer. Methods Fluids 2005, 48, 747–774. [Google Scholar] [CrossRef] [Green Version]
- Hou, S.; Zhou, Q.; Chen, S.; Doolen, G.; Cogley, A.C. Simulation of cavity flow by the lattice Boltzmann method. J. Comput. Phys. 1995, 118, 329–347. [Google Scholar] [CrossRef] [Green Version]
- Aslan, E.; Taymaz, I.; Benim, A.C. Investigation of the Lattice Boltzmann SRT and MRT Stability for Lid Driven Cavity Flow. J. Mater. Mech. Manuf. 2014, 2, 317–324. [Google Scholar]
- Lin, L.S.; Chen, Y.C.; Lin, C.A. Multi relaxation time lattice Boltzmann simulations of deep lid driven cavity flows at different aspect ratios. Comput. Fluids 2011, 45, 233–240. [Google Scholar] [CrossRef]
- Su, S.W.; Lai, M.C.; Lin, C.A. An immersed boundary technique for simulating complex flows with rigid boundary. Comput. Fluids 2007, 36, 313–324. [Google Scholar] [CrossRef]
- Gupta, M.M.; Kalita, J.C. A new paradigm for solving Navier-stokes equations, streamfunction-velocity formulation. J. Comput. Phys. 2005, 207, 25–68. [Google Scholar] [CrossRef]
- Barragy, E.; Carey, G.F. Stream function-vorticity driven cavity solution using p finite elements. Comput. Fluids 1997, 26, 453–468. [Google Scholar] [CrossRef]
- Bennacer, R.; Reggio, M.; Pellerin, N.; Ma, X.Y. Differentiated heated lid driven cavity interacting with tube a Lattice Boltzmann Study. Therm. Sci. 2017, 21, 89–104. [Google Scholar] [CrossRef] [Green Version]
- Kareem, A.K.; Gao, S. Mixed convection heat transfer of turbulent flow in a three-dimensional lid-driven cavity with a rotating cylinder. I J. Heat Mass Transf. 2017, 112, 185–200. [Google Scholar] [CrossRef] [Green Version]
- Bruneau, C.H.; Saad, M. The 2D lid-driven cavity problem revisited. Comput. Fluids 2006, 35, 326–348. [Google Scholar] [CrossRef]





| Re | Result | Left Bottom Vortex | Primary Vortex | Right Bottom Vortex | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| x | y | x | y | x | y | |||||
| Current work | 0.034 | 0.037 | 2.06 × 10 | 0.61 | 0.74 | −0.12 | 0.95 | 0.065 | 1.2 × 10 | |
| 100 | GHIA | 0.031 | 0.04 | 1.80 × 10 | 0.62 | 0.73 | −0.11 | 0.94 | 0.062 | 1.3 × 10 |
| Deviation [%] | 8.8 | 8.1 | 12.6 | 1.6 | 1.4 | 8.3 | 1.1 | 4.6 | 8.3 | |
| Current work | 0.056 | 0.05 | 1.3 × 10 | 0.56 | 0.61 | −0.12 | 0.88 | 0.14 | 6.5 × 10 | |
| 400 | GHIA | 0.051 | 0.04 | 1.4 × 10 | 0.54 | 0.60 | −0.11 | 0.89 | 0.12 | 6.4 × 10 |
| Deviation [%] | 8.9 | 20.0 | 7.7 | 3.6 | 1.6 | 8.3 | 1.1 | 14.3 | 1.5 | |
| Current work | 0.084 | 0.07 | 3.1 × 10 | 0.53 | 0.57 | −0.12 | 0.86 | 0.12 | 1.73 × 10 | |
| 1000 | GHIA | 0.085 | 0.08 | 2.3 × 10 | 0.51 | 0.56 | −0.11 | 0.84 | 0.10 | 1.75 × 10 |
| Deviation [%] | 1.2 | 14.3 | 25.8 | 3.8 | 1.8 | 8.3 | 2.3 | 16.7 | 1.2 | |
| Current work | 0.078 | 0.11 | 1.0 × 10 | 0.52 | 0.54 | −0.11 | 0.83 | 0.095 | 2.9 × 10 | |
| 3000 | GHIA | 0.086 | 0.14 | 9.8 × 10 | 0.53 | 0.55 | −0.12 | 0.81 | 0.085 | 3.1 × 10 |
| Deviation [%] | 10.3 | 27.3 | 2.0 | 1.9 | 1.9 | 9.1 | 2.4 | 10.5 | 6.9 | |
| Current work | 0.071 | 0.14 | 1.3 × 10 | 0.51 | 0.56 | −0.14 | 0.81 | 0.08 | 3.06 × 10 | |
| 5000 | GHIA | 0.070 | 0.13 | 1.4 × 10 | 0.52 | 0.53 | −0.11 | 0.80 | 0.07 | 3.08 × 10 |
| Deviation [%] | 1.4 | 7.1 | 7.7 | 2.0 | 5.4 | 21.4 | 1.2 | 12.5 | 0.7 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, T.; Lim, H.-C. Simulation of Lid-Driven Cavity Flow with Internal Circular Obstacles. Appl. Sci. 2020, 10, 4583. https://doi.org/10.3390/app10134583
Huang T, Lim H-C. Simulation of Lid-Driven Cavity Flow with Internal Circular Obstacles. Applied Sciences. 2020; 10(13):4583. https://doi.org/10.3390/app10134583
Chicago/Turabian StyleHuang, Tingting, and Hee-Chang Lim. 2020. "Simulation of Lid-Driven Cavity Flow with Internal Circular Obstacles" Applied Sciences 10, no. 13: 4583. https://doi.org/10.3390/app10134583
APA StyleHuang, T., & Lim, H.-C. (2020). Simulation of Lid-Driven Cavity Flow with Internal Circular Obstacles. Applied Sciences, 10(13), 4583. https://doi.org/10.3390/app10134583






